Dispersive Optical Solitons for Stochastic Fokas-Lenells Equation With Multiplicative White Noise
Abstract
1. Introduction
2. Governing Model
3. On Solving Equation (1) by MMSE
4. On Solving Equation (1) by MSC
5. On Solving Equation (1) by MJEE
6. Ansatze Involving Hyperbolic Functions
6.1. Combo Bright-Dark Solitons
6.2. Combo Dark-Bright Solitons
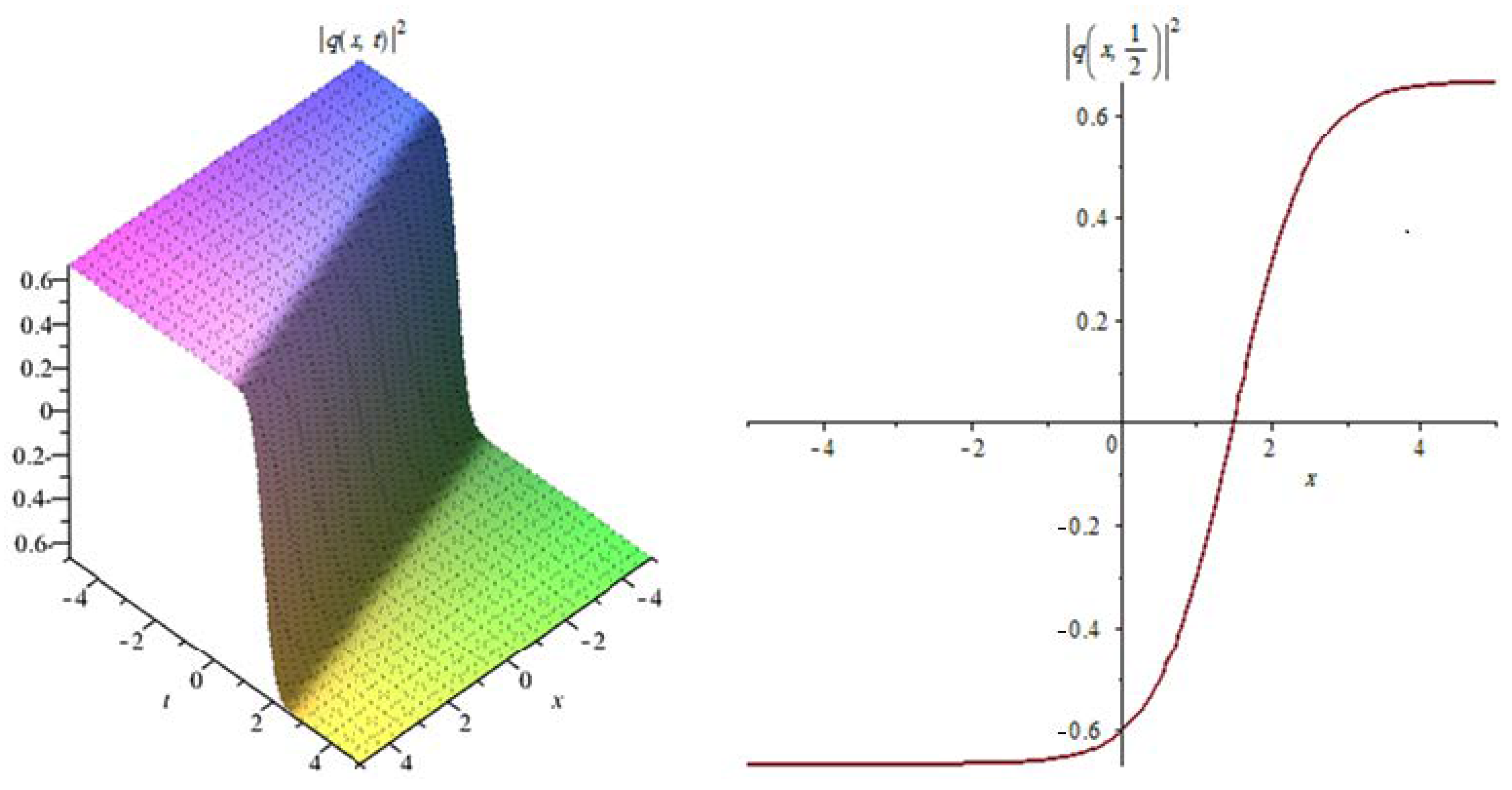
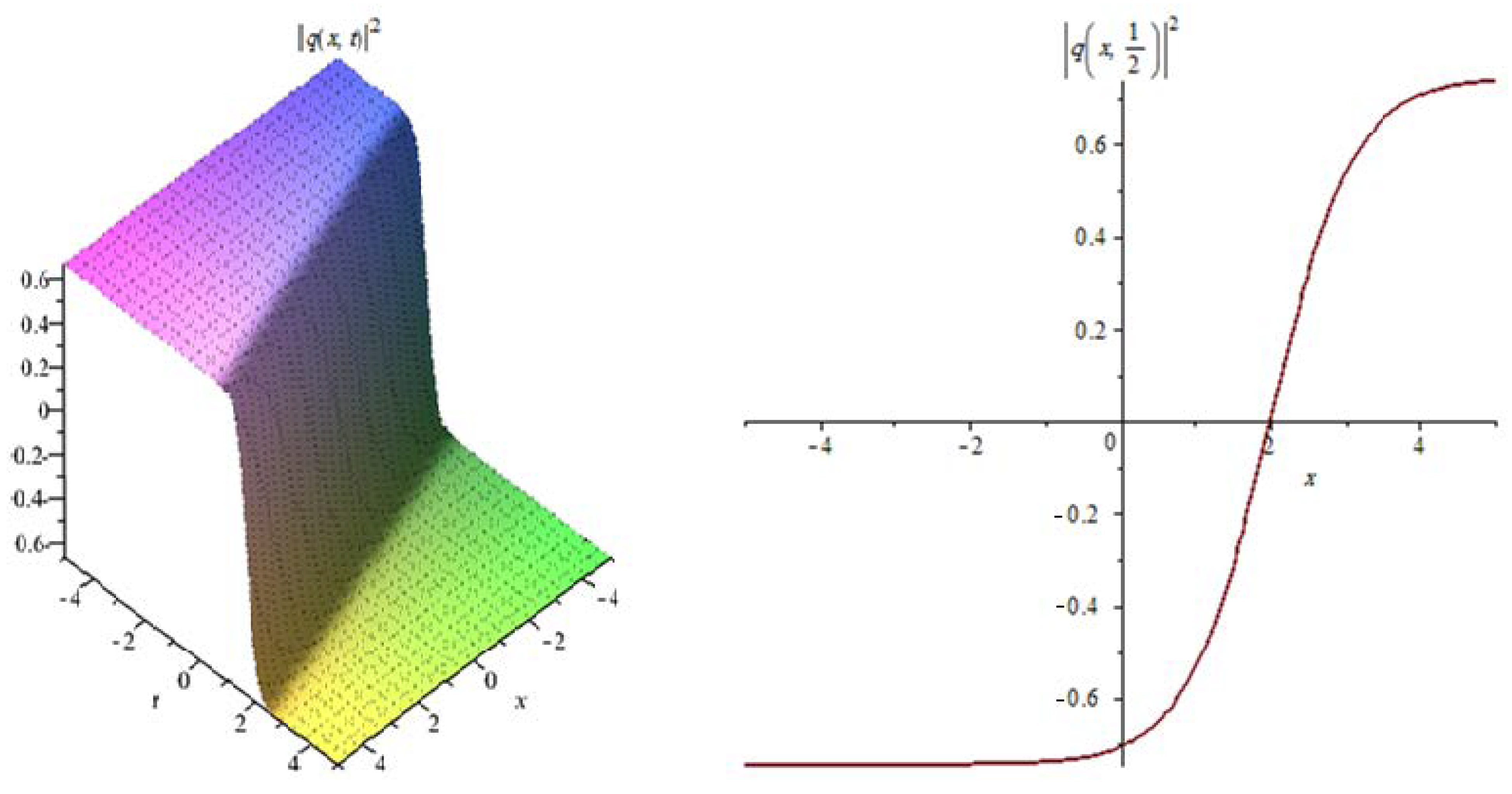
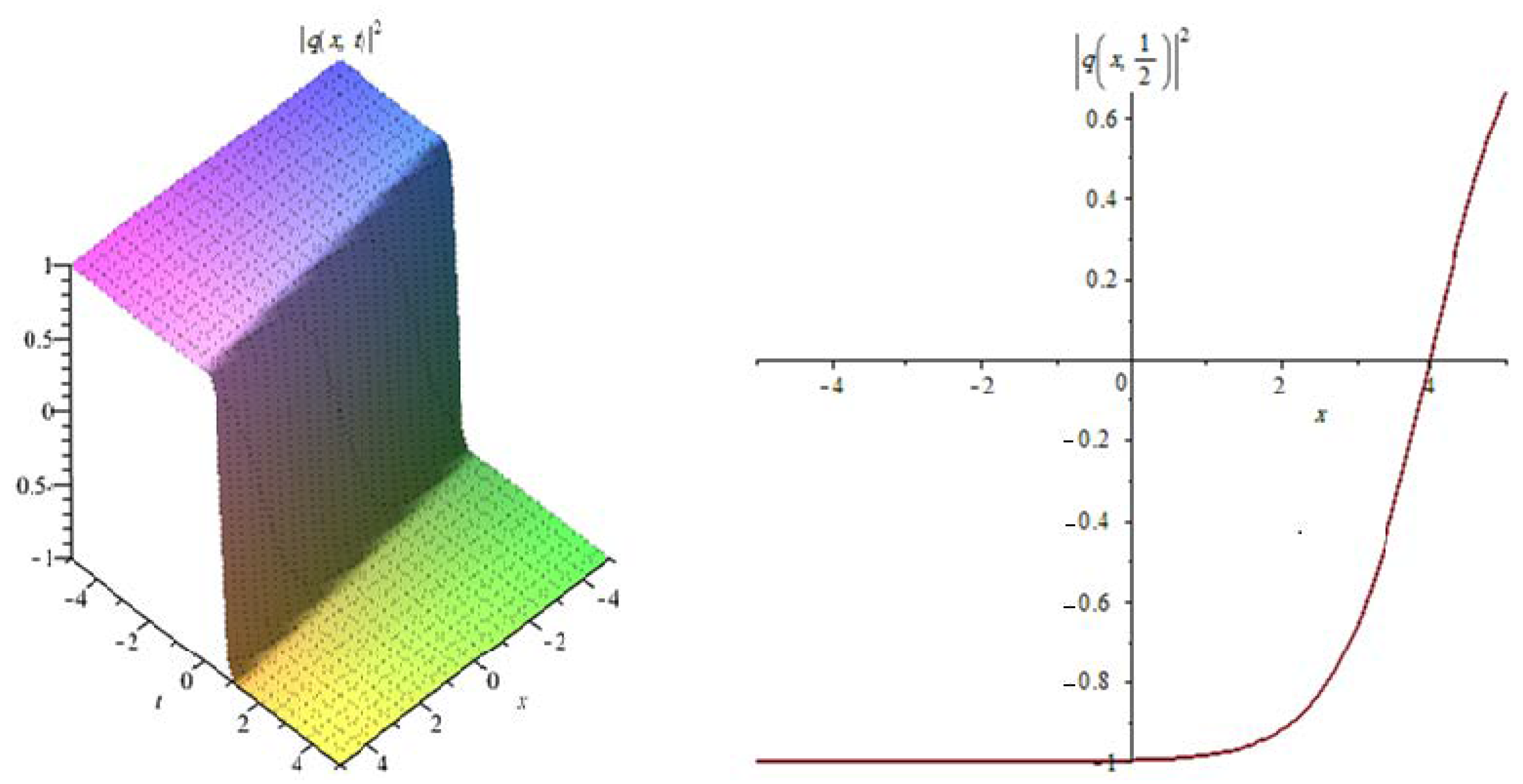
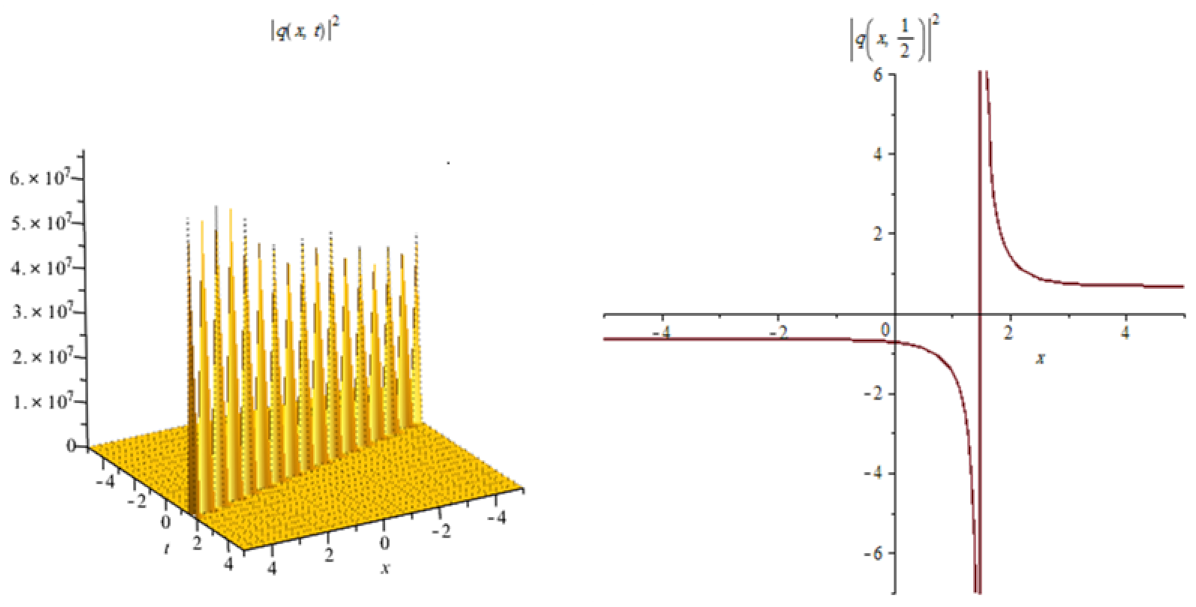
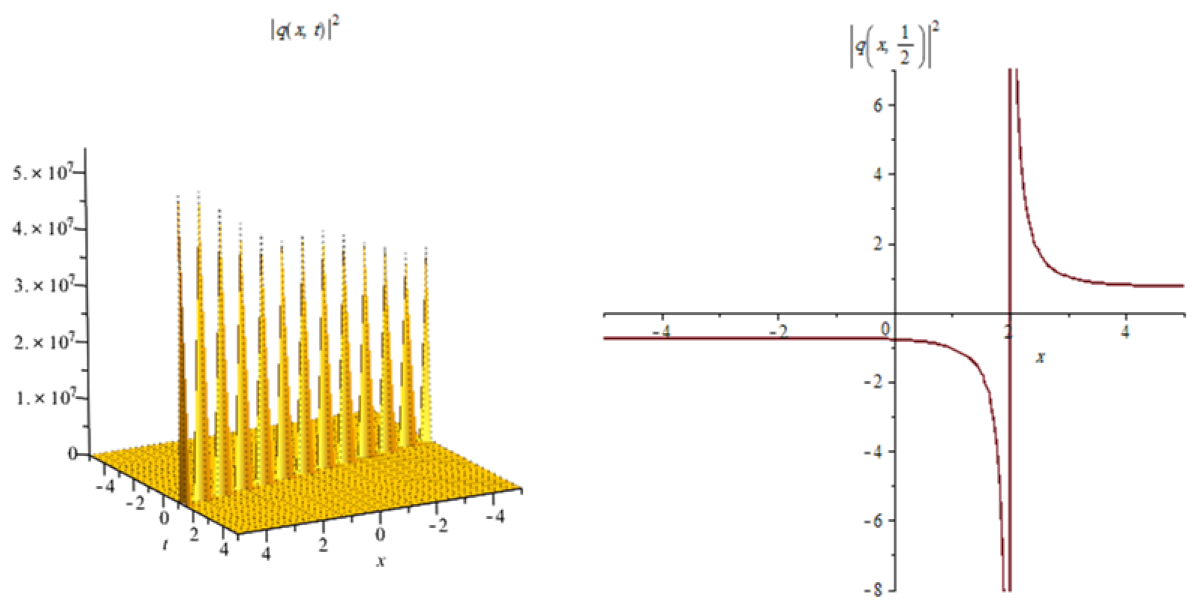
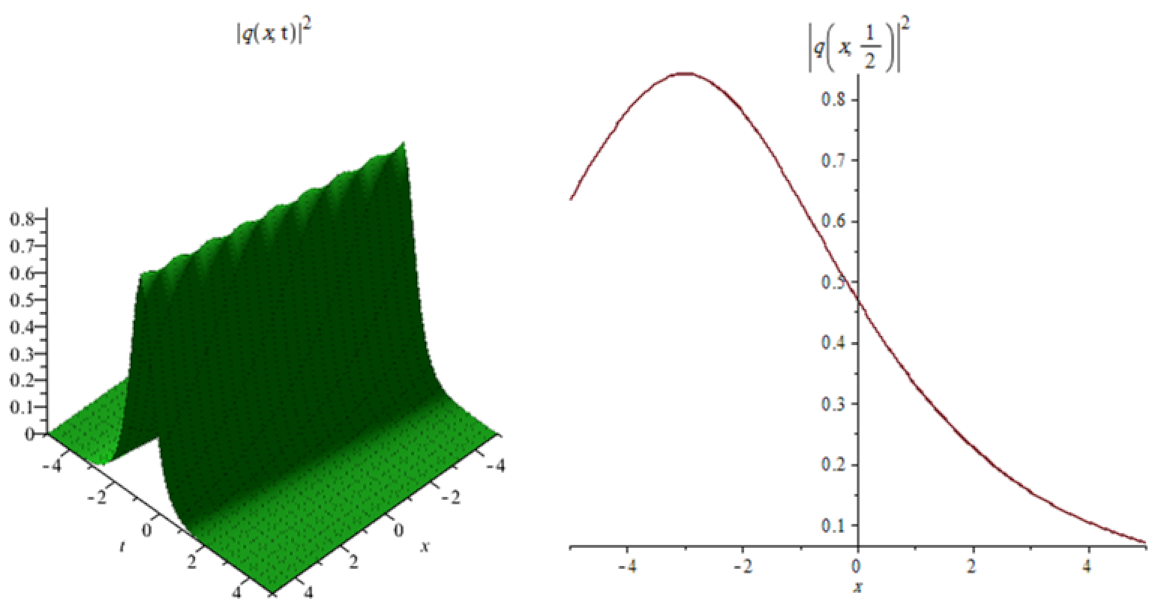
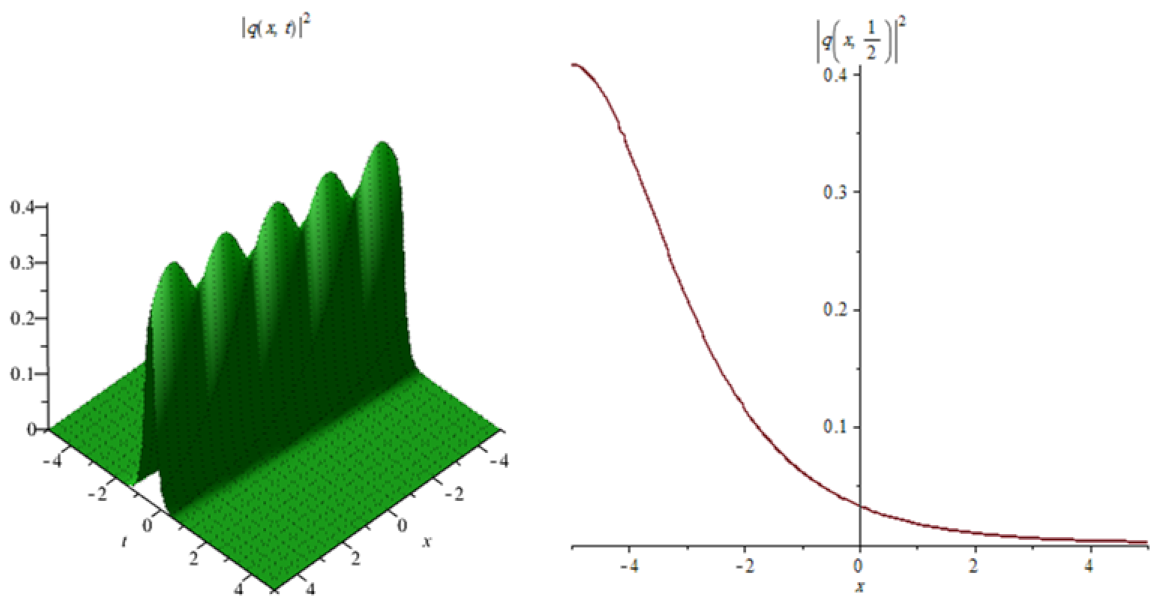
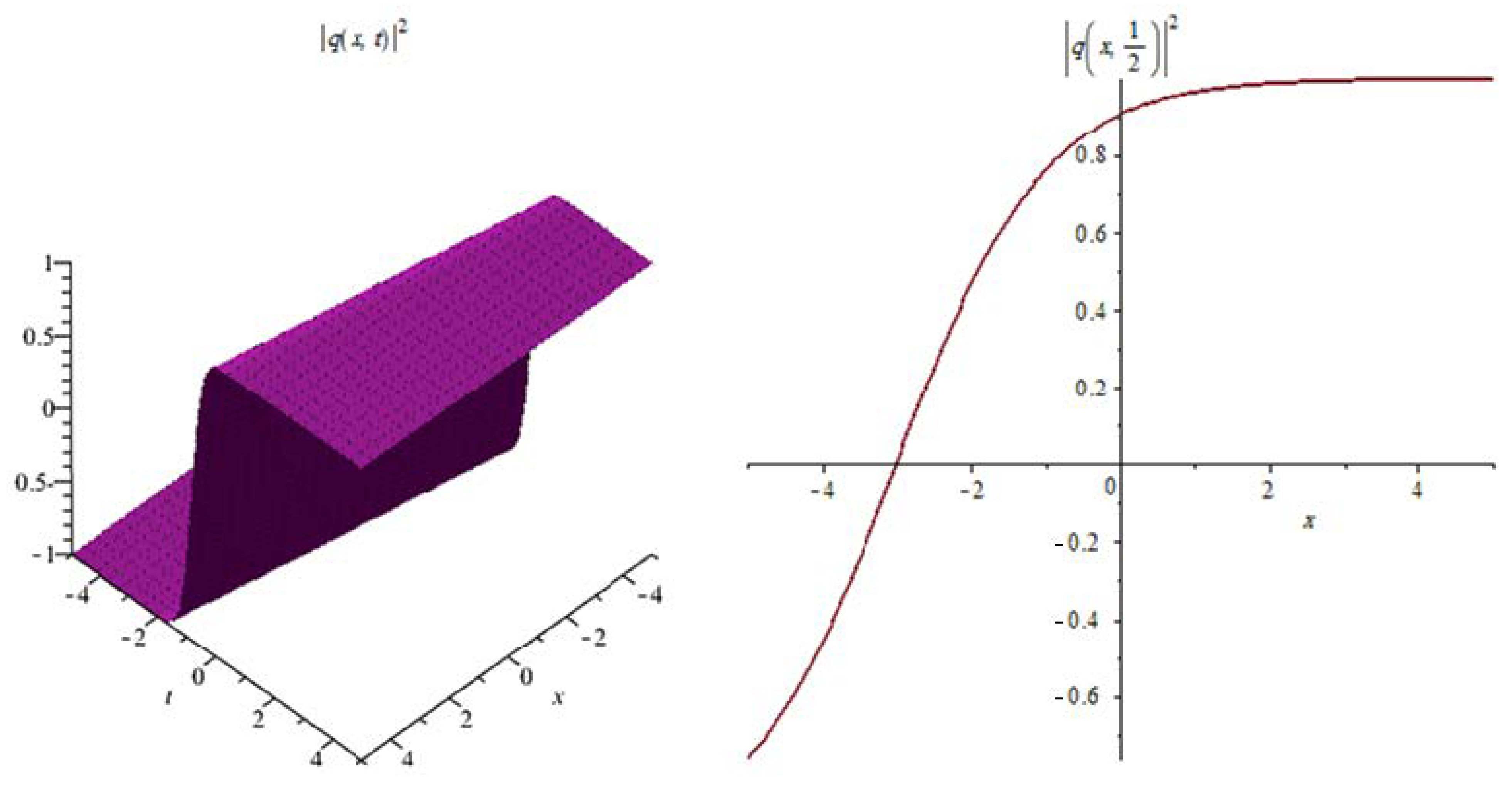
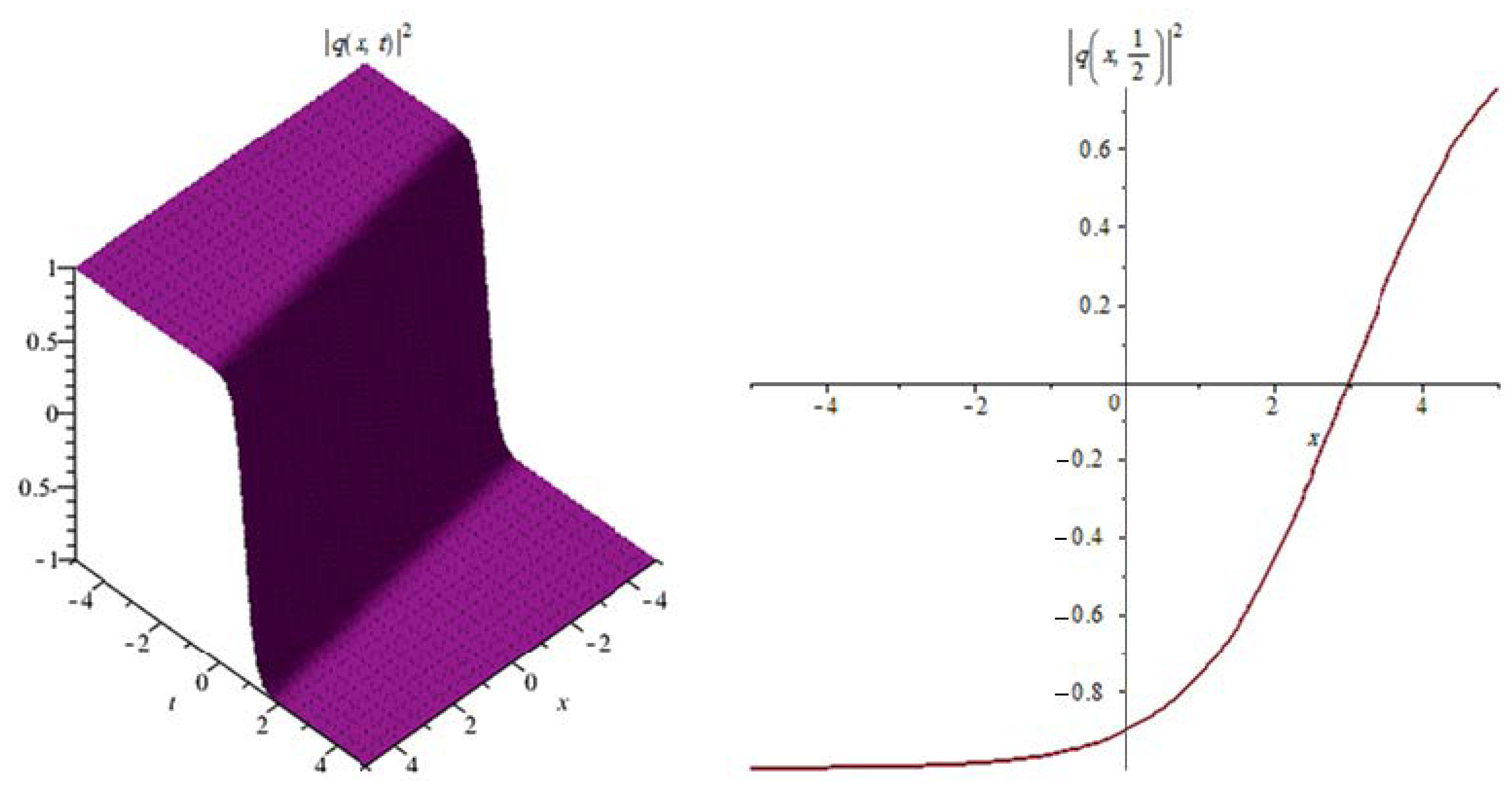
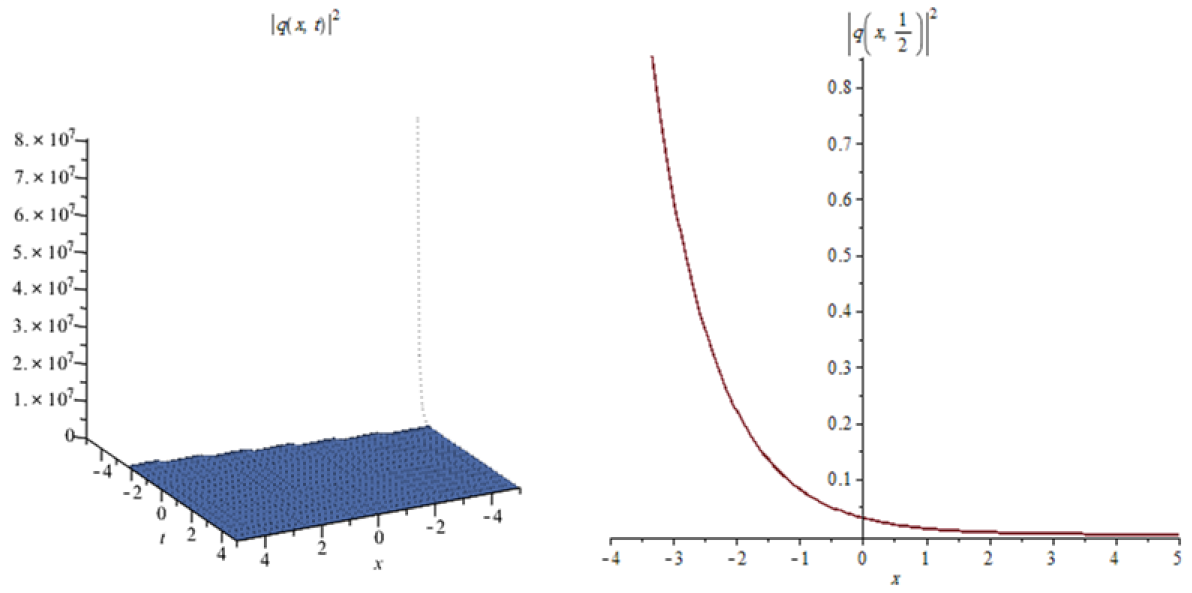
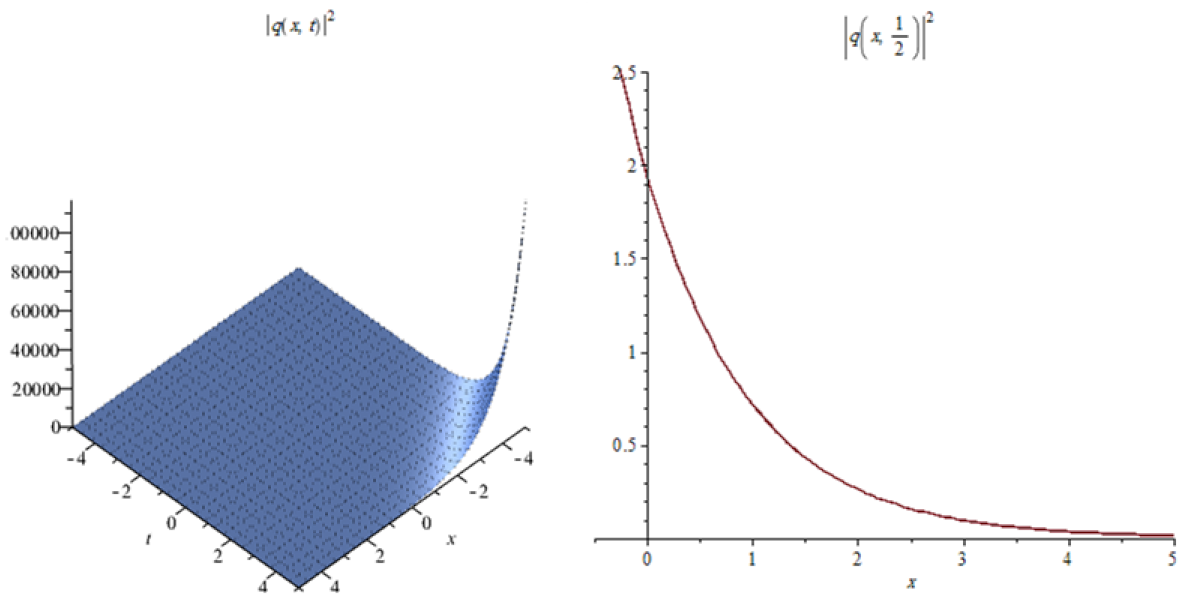
7. Numerical Simulations
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Biswas, A.; Yakup, Y.; Yaşar, E.; Zhou, Q.; Moshokoa, S.P.; Belic, M. Optical soliton solutions to Fokas-lenells equation using some different methods. Optik 2018, 173, 21–31. [Google Scholar] [CrossRef]
- Biswas, A. Chirp-free bright optical soliton perturbation with Fokas–Lenells equation by traveling wave hypothesis and semi-inverse variational principle. Optik 2018, 170, 431–435. [Google Scholar] [CrossRef]
- Albosaily, S.; Mohammed, W.W.; Aiyashi, M.A.; Abdelrahman, A.A.E. Excat solutions of the (2+1)-dimensional stochastic chiral nonlinear Schrödinger’s Equation. Symmetry 2020, 12, 1874. [Google Scholar] [CrossRef]
- Mohammed, W.W.; El-Morshedy, M. The influence of multiplicative noise on the stochastic exact solutions of the Nizhnik-Novikov-Veselov system. Math. Comput. Simul. 2021, 190, 192–202. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Albosaily, S.; Iqbal, N.; El-Morshedy, M. The effect of multiplicative noise on the exact solutions of the stochastic Burger equation. Wave Random Complex Media 2021, 1–13. [Google Scholar] [CrossRef]
- Abdelrahman, A.A.E.; Mohammed, W.W.; Alesemi, M.; Albosaily, S. The effect of multiplicative noise on the exact solutions of Nonlinear Schrödinger’s Equation. AIMS Math. 2021, 6, 2970–2980. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Ahmad, H.; Boulares, H.; Khelifi, F.; El-Morshedy, M. Exact solution of Hirota-Maccari system forced by multiplicative noise in the Itô sense. J. Low FrEquation Noise Vib Act. Control. 2021, 41, 74–84. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alngar, M.E.M.; Shohib, R.M.A.; Biswas, A.; Yildirim, Y.; Alshomrani, A.S.; Alshehri, H.M. Optical solitons having Kudryashov’s self-phase modulation with multiplicative white noise via Itô Calculus using new mapping approach. Optik 2022, 264, 169369. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Shohib, R.M.A.; Alngar, M.E.M.; Biswas, A.; Moraru, L.; Khan, S.; Yildirim, Y.; Alshehri, H.M.; Belic, M.R. Dispersive optical solitons with Schrödinger-Hirota model having multiplicative white noise via Itô calculus. Phys. Lett. A 2022, 445, 128268. [Google Scholar] [CrossRef]
- Fokas, A.S. On a class of physically important integrable equations. Phys. D Nonlinear Phenom. 1995, 87, 145–150. [Google Scholar] [CrossRef]
- Jonatan, L. Exactly Solvable Model for Nonlinear Pulse Propagation in Optical Fibers. Stud. Appl. Math. 2009, 123, 215–232. [Google Scholar]
- Jonatan, L.; Fokas, A.S. On a Novel Integrable Generalization of the Nonlinear Schrödinger Equation. Nonlinearity 2009, 22, 11–27. [Google Scholar]
- Jonatan, L. Dressing for a Novel Integrable Generalization of the Nonlinear Schrödinger Equation. J. Nonlinear Sci. 2010, 20, 709–722. [Google Scholar]
- Kundu, A. Two-fold Integrable Hierarchy of Nonholonomic Deformation of the Derivative Nonlinear Schrödinger and the Lenells-Fokas Equation. J. Math. Phys. 2010, 51, 1–17. [Google Scholar] [CrossRef]
- Zayed, E.M.E. A note on the modified simple equation method applied to Sharma-Tasso-Olver equation. Appl. Math. Comput. 2011, 218, 3962–3964. [Google Scholar] [CrossRef]
- Jawad, A.J.M.; Petkovic, M.D.; Biswas, A. Modified simple equation method for nonlinear evolution equations. Appl. Math. Comput. 2010, 217, 869–877. [Google Scholar]
- Zayed, E.M.E.; Al-Nowehy, A.G. The modified simple equation method, the exp-function method and the method of soliton ansatz for solving the long-short wave resonance equations. Z. Naturforsch. 2016, 71, 103–112. [Google Scholar] [CrossRef]
- El-Borai, M.; El-Owaidy, H.; Arnous, A.H.; Moshokoa, S.; Biswas, A.; Belic, M. Dark and singular optical solitons with spatio-temporal dispersion using modified simple equation method. Optik 2017, 130, 324–331. [Google Scholar] [CrossRef]
- Arnous, A.H.; Ullah, M.Z.; Moshokoa, S.P.; Zhou, Q.; Triki, H.; Mirzazadeh, M.; Biswas, A. Optical solitons in birefringent fibers with modified simple equation method. Optik 2017, 130, 996–1003. [Google Scholar] [CrossRef]
- Arnous, A.H.; Ullah, M.Z.; Asma, M.; Moshokoa, S.P.; Zhou, Q.; Mirzazadeh, M.; Biswas, A.; Belic, M. Dark and singular dispersive optical solitons of Schrödinger-Hirota equation by modified simple equation method. Optik 2017, 136, 445–450. [Google Scholar] [CrossRef]
- Biswas, A. The tanh and the sine-cosine methods for the complex modified KdV and the generalized KdV equations. Comput. Math. Appl. 2005, 49, 1101–1112. [Google Scholar]
- Biswas, A. A sine–cosine method for handing nonlinear wave equations. Math. Comput. Model. 2004, 40, 499–509. [Google Scholar]
- Zayed, E.M.E.; Al-Nowehy, A.G. Solitons and other solutions for the generalized KdV-mKdV equation with higher-order nonlinear terms. J. Part. Diff. Equ. 2016, 29, 218–245. [Google Scholar]
- Yusufuglu, E.; Bekir, A.; Alp, M. Periodic and Solitary wave solutions of Kawahara and modifed Kawahara equations by using sine-cosine method. Chaos Solitons Fract. 2008, 37, 1193–1197. [Google Scholar] [CrossRef]
- Tascan, F.; Bekir, A. Analytical solutions of the (2 + 1)-dimensional nonlinear equations using the sine–cosine method. Appl. Math. Comput. 2009, 215, 3134–3139. [Google Scholar]
- Zayed, E.M.E.; Amer, Y.A.; Shohib, R.M.A. The Jacobi elliptic function expansion method and its applications for solving the higher order dispersive nonlinear Schrödinger’s equation. Sci. J. Math. Res. 2014, 4, 53–72. [Google Scholar]
- Liu, S.; Fu, Z.; Liu, S.; Zhao, Q. Jacobi elliptic function expansion method and periodic wave solutions of nonlinear wave equations. Phys. Lett. A 2001, 289, 69–74. [Google Scholar] [CrossRef]
- Xiang, C. Jacobi elliptic function solutions for (2+1)-dimensional Boussinesq and Kadomtsev-Petviashvilli equation. Appl. Math. 2011, 2, 1313–1316. [Google Scholar] [CrossRef]
- Lu, D.; Shi, Q. New Jacobi elliptic functions solutions for the combined KdV-mKdV equation. Int. J. Nonlinear Sci. 2010, 10, 320–325. [Google Scholar]
- Zheng, B.; Feng, Q. The Jacobi elliptic equation method for solving fractional partial differential equations. Abs. Appl. Anal. 2014, 228, 249071–249080. [Google Scholar] [CrossRef]
- Wazwaz, A.-M. New solitons and kink solutions for the Gardner equation. Commun. Nonlinear Sci. Numer. 2007, 12, 1395–1404. [Google Scholar] [CrossRef]
- Palencia, J.L.D. Travelling waves approach in a parabolic coupled system for modeling the behaviour of substances in a fuel tank. App. Sci. 2021, 11, 5846. [Google Scholar] [CrossRef]
- Jiao, Y.; Yang, J.; Zhang, H. Traveling wave solutions to a cubic predator-prey diffusion model with stage structure for the prey. AIMS Math. 2022, 7, 16261–16277. [Google Scholar] [CrossRef]
- Hu, Y.; Liu, Q. On traveling wave solutions of a class of KdV-Burgers-Kuramoto type equations. AIMS Math. 2019, 4, 1450–1465. [Google Scholar] [CrossRef]
- Bracken, P. The quantum Hamilton–Jacobi formalism in complex space. Quantum Stud. Math. Found. 2022, 7, 389–403. [Google Scholar] [CrossRef]
- Palencia, J.L.D.; ur Rahman, S.; Redond, A.N. Regularity and reduction to a Hamilton-Jacobi equation for a MHD Eyring-Powell fluid. Alex. Eng. 2022, 61, 12283–12291. [Google Scholar] [CrossRef]
- Gomez, C.A.; Roshid, S.H.O.; Inc, M.; Akinyemi, L.; Rezazadeh, H. On soliton solutions for perturbed Fokas–Lenells equation. Opt. Quantum Electron. 2022, 54, 370. [Google Scholar] [CrossRef]
- Nandi, D.C.; Safi Ullah, M.; Roshid, H.O.; Ali, M.Z. Application of the unified method to solve the ion sound and Langmuir waves model. Heliyon 2022, 8, e10924. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zayed, E.M.E.; El-Horbaty, M.; Alngar, M.E.M.; El-Shater, M. Dispersive Optical Solitons for Stochastic Fokas-Lenells Equation With Multiplicative White Noise. Eng 2022, 3, 523-540. https://doi.org/10.3390/eng3040037
Zayed EME, El-Horbaty M, Alngar MEM, El-Shater M. Dispersive Optical Solitons for Stochastic Fokas-Lenells Equation With Multiplicative White Noise. Eng. 2022; 3(4):523-540. https://doi.org/10.3390/eng3040037
Chicago/Turabian StyleZayed, Elsayed M. E., Mahmoud El-Horbaty, Mohamed E. M. Alngar, and Mona El-Shater. 2022. "Dispersive Optical Solitons for Stochastic Fokas-Lenells Equation With Multiplicative White Noise" Eng 3, no. 4: 523-540. https://doi.org/10.3390/eng3040037
APA StyleZayed, E. M. E., El-Horbaty, M., Alngar, M. E. M., & El-Shater, M. (2022). Dispersive Optical Solitons for Stochastic Fokas-Lenells Equation With Multiplicative White Noise. Eng, 3(4), 523-540. https://doi.org/10.3390/eng3040037




