Lateral Deformation Capacity and Plastic Hinge Length of RC Columns Confined with Textile Reinforced Mortar Jackets
Abstract
:1. Introduction
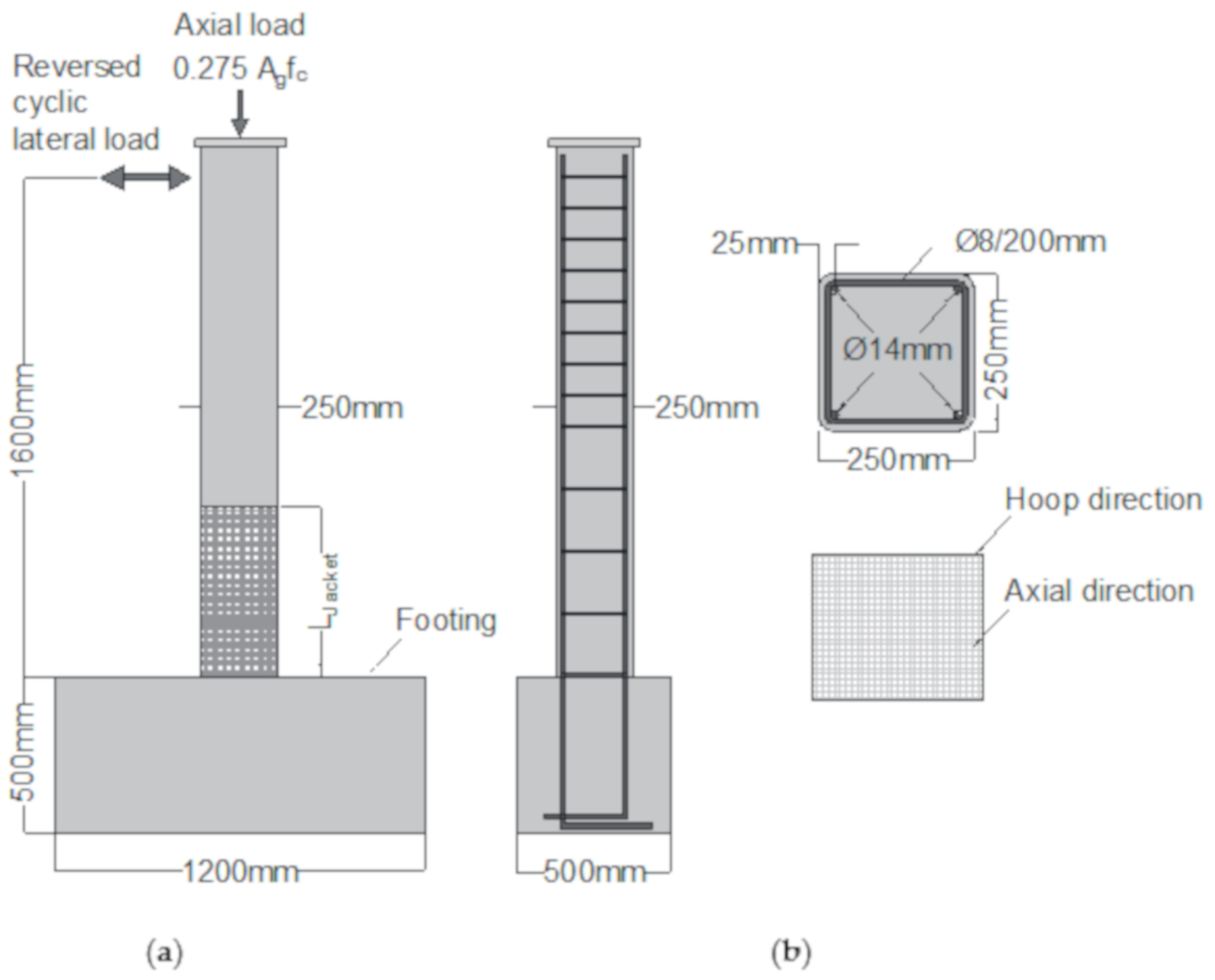
2. Experimental Tests Adopted for Validation of FEA Models
3. Finite Element Analysis Modelling of RC and Retrofitted Columns
3.1. Element Types
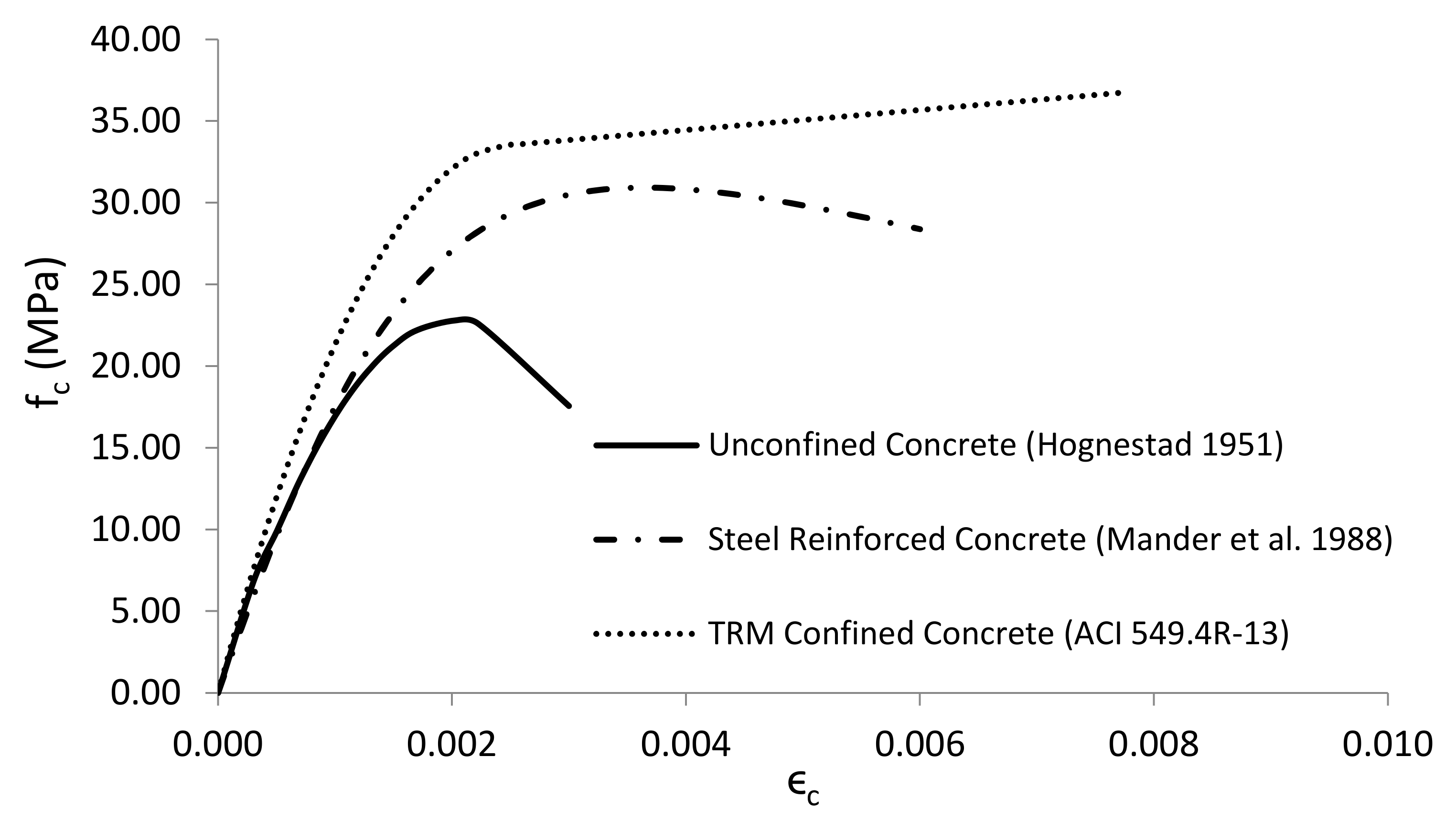
3.2. Material Models
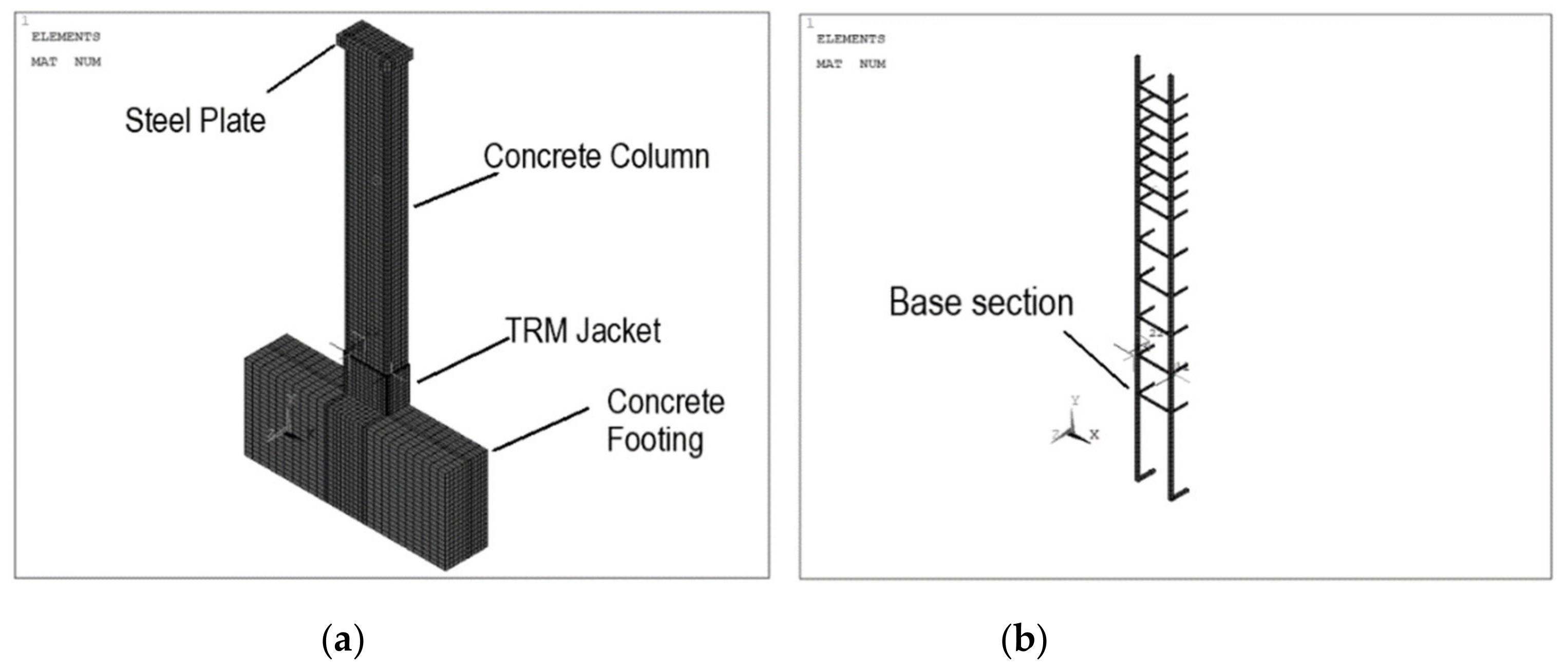
3.3. Mesh Generation and Boundary Conditions
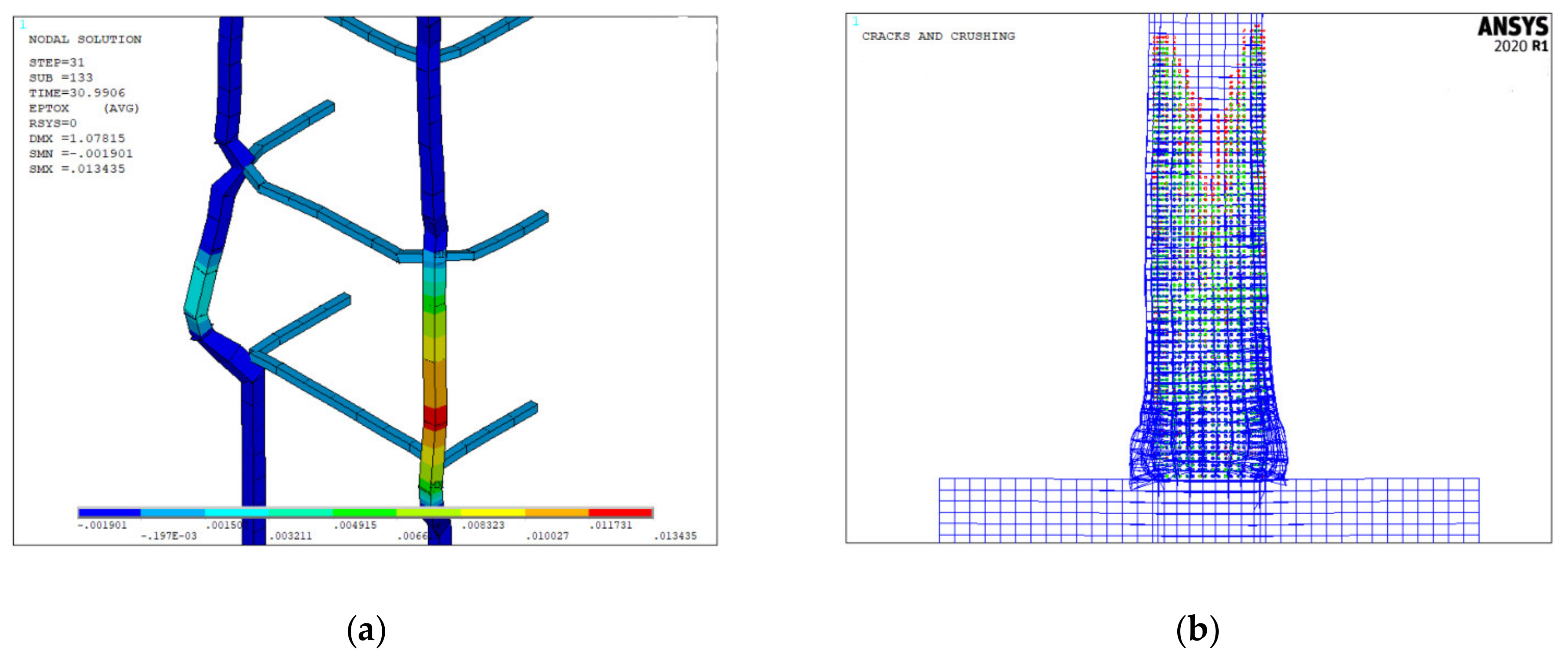
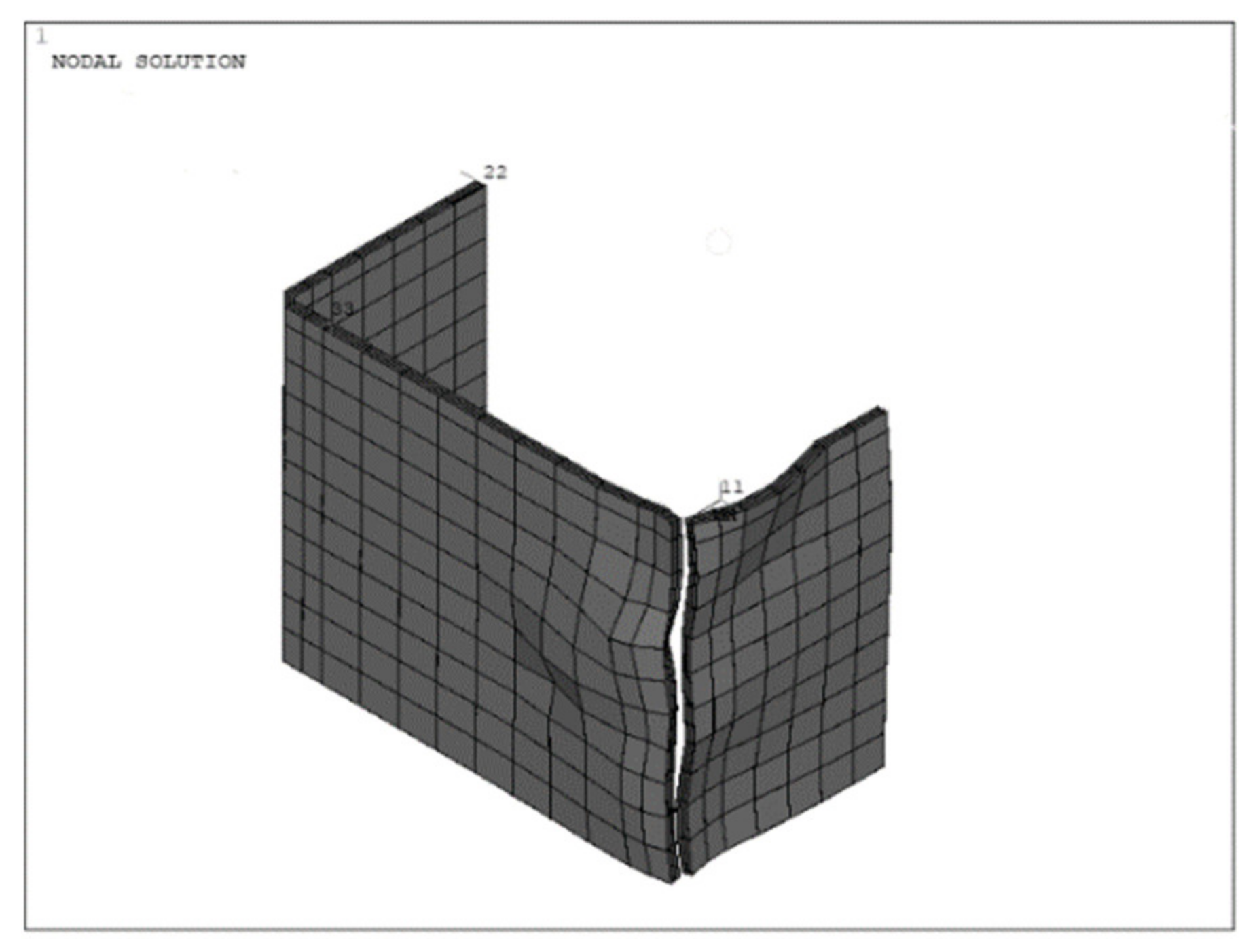
4. Validated Column Models Results
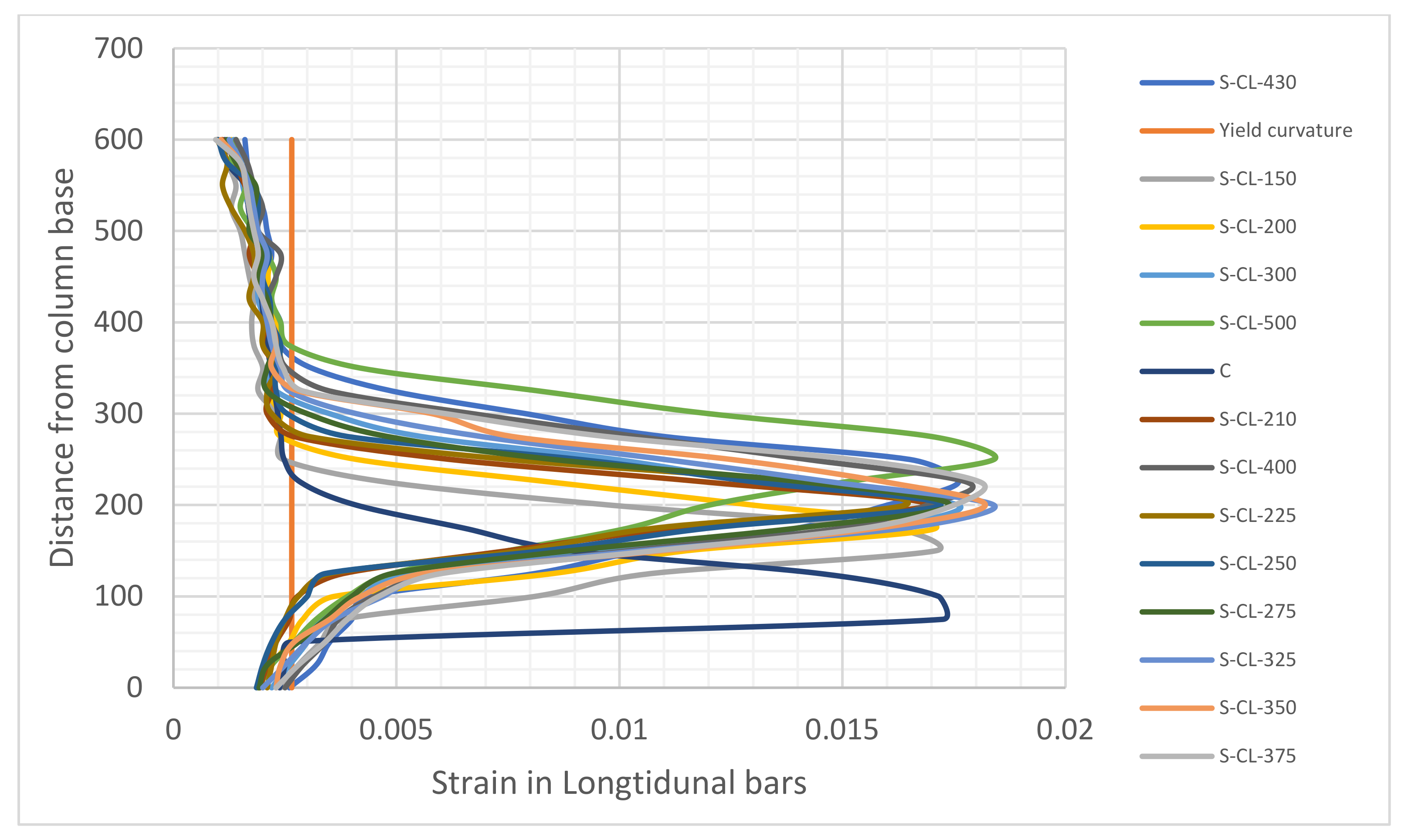
Strains in Longitudinal Bars at the Onset of Bar Buckling
5. Parametric Study
5.1. The Effect of TRM Jacket Length
5.2. The Effect of Mortar Strengths
6. Semiempirical Modeling
6.1. Proposed Model for the Plastic Hinge Length (LP)
6.1.1. Plastic Hinge Length from FEA Models
6.1.2. Equivalent Plastic Hinge Length
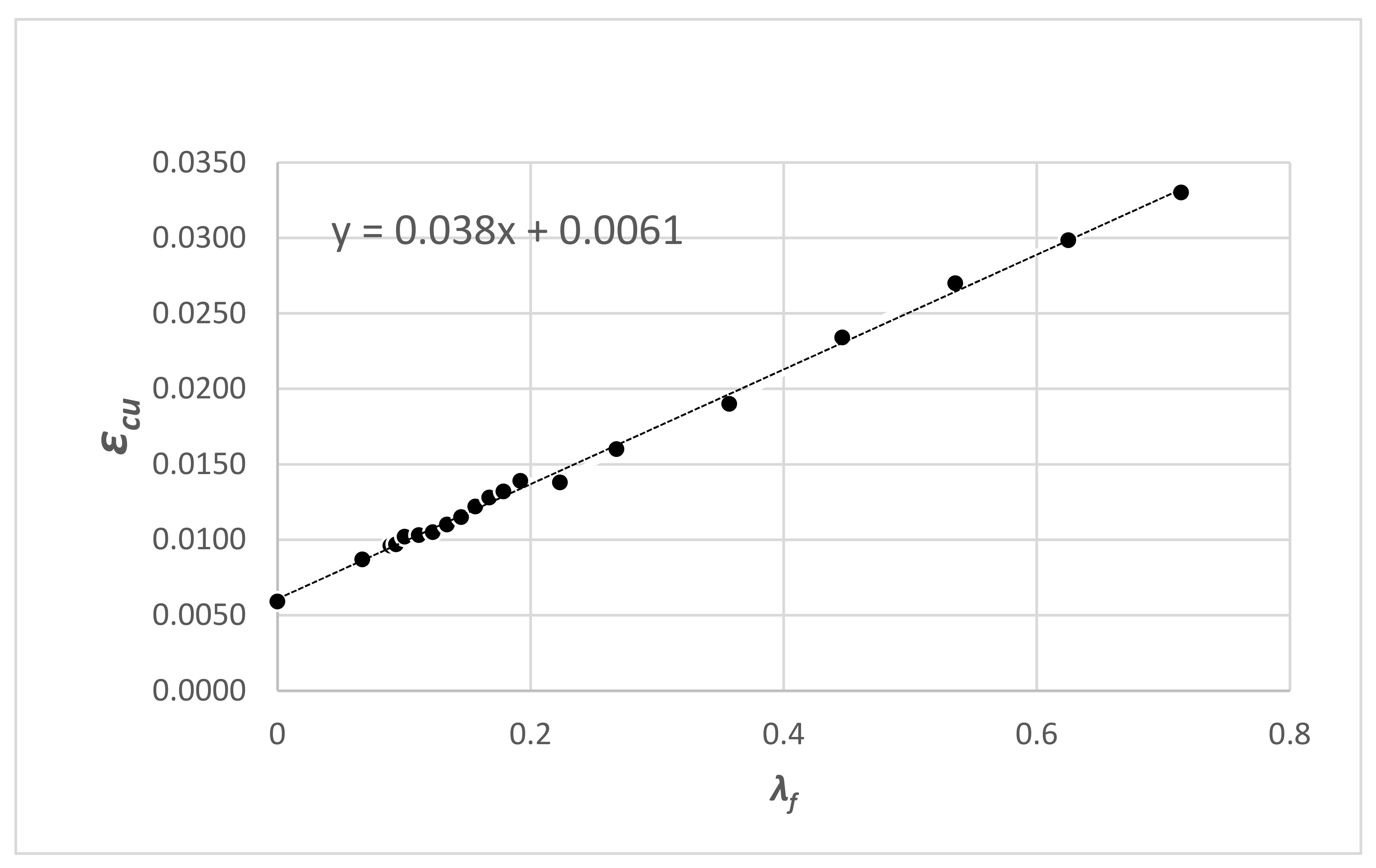
6.1.3. Proposed Equation for Lp
6.1.4. Regression Analysis
6.2. Proposed Model for Ultimate Drift Ratio
7. Conclusions
- The ultimate drift ratio and lateral load capacity increase as the length of the TRM jackets increase. A fully wrapped column model with a jacket length of 1600 mm yielded a 110 and 32.9% enhancement in the ultimate drift ratio and lateral load capacity, respectively, as compared to column model with only a 150-mm jacket length.
- The plastic hinge length enlarged with the increase in the length of the TRM jacket up to a confinement ratio of 0.2. Beyond this point, the plastic hinge length reduced.
- Mortars with higher flexural strengths resulted in an enhancement in the deformation capacity. On the other hand, the difference in the compressive strength of M2 and M3 had an insignificant effect on the ultimate lateral deformation capacity as model S-CL-M3 resulted in a 6% improvement as compared to model S-CL-M2.
- Mortar strengths have an insignificant impact on the plastic hinge length of the column. The maximum difference observed was 2.8%.
- The failure mechanism was independent of the tensile and compressive strengths of the mortar as all the columns failed due to the fracture of the fibers at the base section. However, increasing the jacket’s length shifted the failure from the buckling of the longitudinal bars to the rupture of the textiles.
- An equation for the plastic hinge length of TRM-confined RC columns, while considering the effect of the length of the jacket, was proposed. The results agreed well with the experimental and FEA models’ results with an average difference of 3.52%.
- A semiempirical model was developed for the ultimate drift capacity of RC columns confined with TRM or FRP. The results were compared with experimental and FEA results, and the average difference was 15.23%. On the other hand, the average error of the Yuan [26] model was 23.9% as compared to the data collected from the literature, indicating that the proposed model in this study yielded relatively accurate results.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xue, Q.; Chen, C. Performance-based seismic design of structures: A direct displacement-based approach. Eng. Struct. 2003, 25, 1803–1813. [Google Scholar] [CrossRef]
- Sheikh, S.A.; Khoury, S.S. A performance-based approach for the design of confining steel in tied columns. ACI Struct. J. 1997, 94, 421–431. [Google Scholar]
- Paultre, P.; Légeron, F. Confinement reinforcement design for reinforced concrete columns. J. Struct. Eng. 2008, 134, 738–749. [Google Scholar] [CrossRef]
- Priestley, M.; Park, R. Strength and ductility of concrete bridge columns under seismic loading. ACI Struct. J. 1987, 84, 61–76. [Google Scholar]
- Paulay, T.; Priestley, M.J.N. Seismic Design of Reinforced Concrete and Masonry Buildings; Wiley: New York, NY, USA, 1992. [Google Scholar]
- Sheikh, S.A.; Khoury, S.S. Confined concrete columns with stubs. ACI Struct. J. 1993, 90, 414–431. [Google Scholar]
- Panagiotakos, T.B.; Fardis, M.N. Deformations of reinforced concrete members at yielding and ultimate. ACI Struct. J. 2001, 98, 135–148. [Google Scholar]
- Berry, M.P. Performance Modeling Strategies for Modern Reinforced Concrete Bridge Columns. Ph.D. Thesis, University of Washington, Seattle, WA, USA, 2006. [Google Scholar]
- Biskinis, D.; Fardis, M.N. Models for FRP-wrapped rectangular RC columns with continuous or lap-spliced bars under cyclic lateral loading. Eng. Struct. 2013, 57, 199–212. [Google Scholar] [CrossRef]
- ElGawady, M.; Booker, A.; Dawood, H. Seismic behavior of posttensioned concrete-filled fiber tubes. J. Compos. Constr. 2010, 14, 616–628. [Google Scholar] [CrossRef]
- Ozbakkaloglu, T.; Idris, Y. Seismic behavior of FRP-high-strength concrete-steel double-skin tubular columns. J. Struct. Eng. 2014, 140, 4014–4019. [Google Scholar] [CrossRef]
- Youssf, O.; ElGawady, M.; Mills, J.; Ma, X. Finite element modelling and dilation of FRP-confined concrete columns. Eng. Struct. 2014, 79, 70–85. [Google Scholar] [CrossRef]
- Tetta, Z.; Koutas, L.; Bournas, D. Shear strengthening of concrete members with TRM jackets: Effect of shear span-to-depth ratio, material and amount of external reinforcement. Compos. Part B Eng. 2018, 137, 184–201. [Google Scholar] [CrossRef]
- Bournas, D.A.; Lontou, P.V.; Papanicolaou, C.G.; Triantafillou, T.C. Textile-reinforced mortar (TRM) versus FRP confinement in reinforced concrete columns. ACI Struct. J. 2007, 104, 740–748. [Google Scholar]
- Bournas, D.A.; Triantafillou, T.C.; Zygouris, K.; Stavropoulos, F. Textile-reinforced mortar (TRM) versus FRP jacketing in seismic retrofitting of RC columns with continuous or lap-spliced deformed bars. J. Compos. Constr. 2009, 13, 360–371. [Google Scholar] [CrossRef]
- Bournas, D.A.; Triantafillou, T.C. Innovative Seismic Retrofitting of RC Columns Using Advanced Composites, Advances in Performance-Based Earthquake Engineering; Springer: Amsterdam, The Netherlands, 2010. [Google Scholar]
- Bournas, D.A.; Triantafillou, T.C. Bar buckling in RC columns confined with composite materials. J. Compos. Constr. 2011, 15, 393–403. [Google Scholar] [CrossRef]
- Yin, S.P.; Peng, C.; Jin, Z.Y. Research on mechanical properties of axial-compressive concrete columns strengthened with TRC under a conventional and chloride wet-dry cycle environment. J. Compos. Constr. 2016, 21, 1–11. [Google Scholar] [CrossRef]
- Yao, L.; Shi-ping, Y.; Wen-jie, C. Seismic behavior of corrosion-damaged RC columns strengthened with TRC under a chloride environment. Constr. Build. Mater. 2019, 201, 736–745. [Google Scholar] [CrossRef]
- Dinh, N.H.; Park, S.H.; Choi, K.K. Seismic performance of reinforced concrete columns retrofitted by textile-reinforced mortar jackets. Struct. Infrastruct. Eng. 2020, 16, 1364–1381. [Google Scholar] [CrossRef]
- Ming, L.; Shi-Ping, Y.; Xi, C. Seismic behavior of textile-reinforced concrete–strengthened RC columns under different axial compression ratios. J. Eng. Fibers Fabr. 2019, 14, 1–11. [Google Scholar] [CrossRef]
- Yin, S.; Li, Y.; Li, S.; Yang, Y. Seismic performance of RC columns strengthened with textile-reinforced concrete in chloride environment. J. Compos. Constr. 2020, 24, 1–13. [Google Scholar] [CrossRef]
- Li, Y.; Yin, S.; Dai, J.; Liu, M. Numerical investigation on the influences of different factors on the seismic performance of TRC-strengthened RC columns. J. Build. Eng. 2020, 30, 101245. [Google Scholar] [CrossRef]
- Gu, D.; Wu, Y.; Wu, G.; Wu, Z. Plastic hinge analysis of FRP confined circular concrete columns. Constr. Build. Mater. 2012, 27, 223–233. [Google Scholar] [CrossRef]
- Youssf, O.; ElGawady, M.; Mills, J. Displacement and plastic hinge length of FRP-confined circular reinforced concrete columns. Eng. Struct. 2015, 101, 465–476. [Google Scholar] [CrossRef]
- Yuan, F.; Wu, Y.; Li, C. Modelling plastic hinge of FRP-confined RC columns. Eng. Struct. 2017, 131, 651–668. [Google Scholar] [CrossRef]
- Jiang, C.; Wu, Y.; Wu, G. Plastic Hinge Length of FRP-Confined Square RC Columns. J. Compos. Constr. 2014, 18, 04014003. [Google Scholar] [CrossRef]
- ANSYS® Academic Research Mechanical Release 17; Help System: ANSYS, Inc. 2017. Available online: http://www.ansys.com/ (accessed on 27 September 2017).
- ACI 318. Building Code Requirements for Structural Concrete (ACI 318–19) and Commentary (ACI 318R–19); American Concrete Institute (ACI): Farmington Hills, MI, USA, 2019. [Google Scholar]
- Mander, J.B.; Priestly, M.J.N.; Park, R. Theoretical stress-strain model for confined concrete. ASCE J. Struct. Eng. 1988, 114, 1804–1826. [Google Scholar] [CrossRef] [Green Version]
- American Concrete Institute. ACI 549.4R–13: Guide to Design and Construction of Externally Bonded Fabric-Reinforced Cementitious Matrix (FRCM) Systems for Repair and Strengthening Concrete and Masonry Structures; American Concrete Institute: Farmington Hills, MI, USA, 2013; p. 74. [Google Scholar]
- William, K.J.; Warnke, E.P. Constitutive Model for the Triaxial Behavior of Concrete; International Association for Bridge and Structural Engineering: Zürich, Switzerland; ISMES: Bergamo, Italy, 1975; Volume 19, p. 174. [Google Scholar]
- Alhaddad, M.; Siddiqui, N.; Abadel, A.; Alsayed, S.; Al-salloum, Y. Numerical investigations on the seismic behavior of FRP and TRM upgraded RC exterior beam-column joints. J. Compos. Constr. 2012, 16, 308–321. [Google Scholar] [CrossRef]
- Lu, X.; Teng, J.; Ye, L.; Jiang, J. Bond-slip models for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 920–937. [Google Scholar] [CrossRef]
- Murcia-Delso, J.; Shing, P.B. Bond-slip model for detailed finite-element analysis of reinforced concrete structures. J. Struct. Eng. 2014, 141, 04014125. [Google Scholar] [CrossRef]
- Triantafillou, T.C.; Papanicolaou, C.G.; Zissimopoulos, P.; Laourdekis, T. Concrete confinement with textile-reinforced mortar jackets. ACI Struct. J. 2006, 103, 28–37. [Google Scholar]
- Hines, E.M.; Restrepo, J.I.; Seible, F. Force-displacement characterization of well-confined bridge piers. ACI Struct. J. 2004, 101, 537–548. [Google Scholar]
- Liang, R.; Fang, B.; Wang, K.; Yuan, F. Numerical investigation on plastic hinge length of ultra-high performance concrete column under cyclic load. J. Earthq. Eng. 2020, 1–19. [Google Scholar] [CrossRef]
- Benzaid, R.; Mesbah, H. Strength model for square concrete columns confined by external CFRP sheets. Struct. Eng. Mech. 2013, 46, 111–135. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.; Cheng, L. Experimental study of seismic behavior of high-strength RC columns strengthened with CFRP subjected to cyclic loading. J. Struct. Eng. 2019, 145, 04018240. [Google Scholar] [CrossRef]
- Del Zoppo, M.; Di Ludovico, M.; Balsamo, A.; Prota, A. Comparative analysis of existing RC columns jacketed with CFRP or FRCC. Polymers 2018, 10, 361. [Google Scholar] [CrossRef] [Green Version]
- Ouyang, L.; Gao, W.; Zhen, B.; Lu, Z. Seismic retrofit of square reinforced concrete columns using basalt and carbon fiber-reinforced polymer sheets: A comparative study. Compos. Struct. 2017, 162, 294–307. [Google Scholar] [CrossRef]
- Wang, D.; Huang, L.; Yu, T.; Wang, Z. Seismic performance of CFRP-retrofitted large-scale square RC columns with high axial compression ratios. J. Compos. Constr. 2017, 21, 04017031. [Google Scholar] [CrossRef]
- Park, R.; Paulay, T. Reinforced Concrete Structures; John Wiley & Sons: Hoboken, NJ, USA, 1975. [Google Scholar]
- Biskinis, D.E. Deformations of Concrete Members at Yielding and Ultimate. Ph.D. Thesis, University of Patras, Patras, Greece, 2007. (In Greek). [Google Scholar]



| Drift at Failure (%) | Peak Force (KN) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Experiment | FEA | Difference (%) | Experiment | FEA | Difference (%) | ||||||||
| Experiment ID | FEA model ID | Push | Pull | Push | Pull | Push | Pull | Push | Pull | Push | Pull | Push | Pull |
| L0_C | C | 3.43 | 3.43 | 3.75 | 3.75 | 9.3 | 9.3 | 41.63 | −42.5 | 43.7 | −43.9 | 4.97 | 3.34 |
| L0_M4 | S-CL-430 | >7.8 | >7.8 | 9.38 | 9.1 | - | - | 45.77 | −49.2 | 48.1 | −48.4 | 5.1 | 1.6 |
| L0_M4G | S-G-430 | 7.5 | 6.9 | 8.1 | 7.5 | 8 | 8.7 | 48.82 | −45.3 | 49.5 | −49.3 | 1.4 | 8.9 |
| Compressive Axial Strain in Longitudinal Bars | ||||
|---|---|---|---|---|
| Column ID | Drift Ratio (%) | Experimental | Numerical (ANSYS) | Exp./Num. (%) |
| C | 3.1 | −0.0077 | −0.0074 | 3.9 |
| S-CL-430 | 4.3 | −0.0082 | −0.0081 | 1.22 |
| S-G-430 | 5.3 | −0.0063 | −0.00582 | 7.62 |
| Column ID | Jacket Length (mm) | Θu (%) | Difference of Strengthened and Control (%) | Lpr (mm) | Difference of Strengthened and Control (%) | Mode of Failure |
|---|---|---|---|---|---|---|
| C | - | 3.75 | - | 239 | - | Buckling of longitudinal bars at the base section |
| S-CL-150 | 150 | 5.94 | 58.4 | 248 | 3.77 | Buckling of longitudinal bars above the jacket |
| S-CL-200 | 200 | 6.56 | 74.9 | 271 | 13.4 | Buckling of longitudinal bars above the jacket |
| S-CL-210 | 210 | 6.56 | 74.9 | 277 | 15.9 | Buckling of longitudinal bars above the jacket |
| S-CL-225 | 225 | 6.87 | 83.2 | 287 | 20.1 | Buckling of longitudinal bars above the jacket |
| S-CL-250 | 250 | 7.18 | 91.5 | 296 | 23.8 | Buckling of longitudinal bars above the jacket |
| S-CL-275 | 275 | 7.18 | 91.5 | 302 | 26.4 | Buckling of longitudinal bars above the jacket |
| S-CL-300 | 300 | 7.5 | 100 | 306 | 28 | Rupture of the jacket |
| S-CL-325 | 325 | 7.81 | 108.3 | 314 | 31.4 | Rupture of the jacket |
| S-CL-350 | 350 | 8.13 | 116.8 | 319 | 33.5 | Rupture of the jacket |
| S-CL-375 | 375 | 8.43 | 124.8 | 328 | 37.2 | Rupture of the jacket |
| S-CL-400 | 400 | 8.75 | 133.3 | 335 | 40.2 | Rupture of the jacket |
| S-CL-430 | 430 | 9.38 | 150 | 341 | 42.7 | Rupture of the jacket |
| S-CL-500 | 500 | 10.0 | 166.7 | 332 | 38.9 | Rupture of the jacket |
| S-CL-600 | 600 | 10.6 | 182.7 | 314 | 31.4 | Rupture of the jacket |
| S-CL-800 | 800 | 10.9 | 190.7 | 291 | 21.8 | Rupture of the jacket |
| S-CL-1000 | 1000 | 11.25 | 200 | 271 | 13.4 | Rupture of the jacket |
| S-CL-1200 | 1200 | 11.25 | 200 | 258 | 7.9 | Rupture of the jacket |
| S-CL-1400 | 1400 | 11.56 | 208.7 | 246 | 2.9 | Rupture of the jacket |
| S-CL-1600 | 1600 | 12.5 | 233.3 | 235 | −1.7 | Rupture of the jacket |
| Mortar | Flexural Strength (MPa) | Compressive Strength (MPa) |
|---|---|---|
| M1 | 3.28 | 8.56 |
| M2 | 4.24 | 30.61 |
| M3 | 6.51 | 20.8 |
| Column Notation | θu (%) | Diff. between Strengthened and Control (%) | Lpr (mm) | Mode of Failure |
|---|---|---|---|---|
| C | 3.75 | - | 239 | Buckling of longitudinal bars at the base section |
| S-CL-M1 | 8.75 | 133 | 352 | Rupture of the jacket |
| S-CL-M2 | 9.1 | 142 | 363 | Rupture of the jacket |
| S-CL-M3 | 9.38 | 150 | 356 | Rupture of the jacket |
| Model | Lpr (mm) | Lp (mm) |
|---|---|---|
| C | 239 | 280 |
| S-CL-150 | 248 | 285 |
| S-CL-200 | 271 | 296 |
| S-CL-210 | 277 | 300 |
| S-CL-225 | 287 | 305 |
| S-CL-250 | 296 | 309 |
| S-CL-275 | 302 | 312 |
| S-CL-300 | 306 | 314 |
| S-CL-325 | 314 | 318 |
| S-CL-350 | 319 | 320 |
| S-CL-375 | 328 | 325 |
| S-CL-400 | 335 | 328 |
| S-CL-430 | 341 | 332 |
| S-CL-500 | 332 | 331 |
| S-CL-600 | 314 | 318 |
| S-CL-800 | 291 | 306 |
| S-CL-1000 | 271 | 296 |
| S-CL-1200 | 258 | 290 |
| S-CL-1400 | 246 | 284 |
| S-CL-1600 | 235 | 278 |
| Study | ID | Ljacket (mm) | Lc (mm) | dP (mm) | fy (Mpa) | fco (Mpa) | tf (mm) | fFRP (Mpa) | λf | Lp (mm) Experiments | Lp (mm)FEA | Lp (mm) Predicted | Difference (%) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wang [40] | LA2 | 500 | 650 | 16 | 362.8 | 50.4 | 0.222 | 3400 | 0.20 | 216 | 197 | 8.96 | |
| Zoppo [41] | CL_FRPa | 500 | 1500 | 18 | 525 | 14.9 | 0.33 | 2990 | 0.22 | 370 | 360 | 2.57 | |
| CL_FRPb | 500 | 1500 | 18 | 525 | 16 | 0.33 | 4788 | 0.32 | 330 | 343 | 4.01 | ||
| CM_FRPa | 500 | 1500 | 18 | 525 | 29.1 | 0.66 | 2990 | 0.22 | 367 | 360 | 1.96 | ||
| CM_FRPb | 500 | 1500 | 18 | 525 | 33.3 | 0.66 | 4788 | 0.31 | 333 | 347 | 3.94 | ||
| Ouyang [42] | C-B2 | 1000 | 1175 | 18 | 386 | 29.6 | 0.34 | 2100 | 0.20 | 285 | 272 | 4.63 | |
| C-B3 | 1000 | 1175 | 18 | 386 | 29.6 | 0.51 | 2100 | 0.30 | 253 | 263 | 3.95 | ||
| C-BC | 1000 | 1175 | 18 | 386 | 29.6 | 0.337 | 2100 | 0.20 | 286 | 272 | 4.87 | ||
| C-C2 | 1000 | 1175 | 18 | 386 | 29.6 | 0.334 | 3100 | 0.29 | 256 | 265 | 3.63 | ||
| C-C3 | 1000 | 1175 | 18 | 386 | 29.6 | 0.501 | 3100 | 0.44 | 230 | 215 | 6.59 | ||
| Wang [43] | N4C3A75 | 500 | 1400 | 20 | 437 | 27.4 | 0.501 | 4340 | 0.22 | 372 | 358 | 3.64 | |
| N4C4A45 | 500 | 1400 | 20 | 437 | 27.4 | 0.668 | 4340 | 0.29 | 323 | 343 | 6.2 | ||
| N3C2A55 | 450 | 1050 | 16 | 358 | 27.4 | 0.334 | 4340 | 0.23 | 252 | 250 | 1.08 | ||
| N3C3A45 | 450 | 1050 | 16 | 358 | 27.4 | 0.501 | 4340 | 0.35 | 203 | 216 | 6.62 | ||
| FEA | S-CL-150 | 150 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.07 | 285 | 290 | 2.12 | |
| S-CL-200 | 200 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.09 | 296 | 303 | 2.18 | ||
| S-CL-210 | 210 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.09 | 300 | 305 | 1.9 | ||
| S-CL-225 | 225 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.10 | 305 | 309 | 0.95 | ||
| S-CL-250 | 250 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.11 | 309 | 314 | 1.57 | ||
| S-CL-275 | 275 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.12 | 312 | 318 | 2 | ||
| S-CL-300 | 300 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.13 | 314 | 322 | 2.61 | ||
| S-CL-325 | 325 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.15 | 318 | 326 | 2.38 | ||
| S-CL-350 | 350 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.16 | 320 | 329 | 2.64 | ||
| S-CL-375 | 375 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.17 | 325 | 331 | 1.8 | ||
| S-CL-400 | 400 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.18 | 328 | 333 | 1.43 | ||
| S-CL-430 | 430 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.19 | 332 | 335 | 0.68 | ||
| S-CL-500 | 500 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.22 | 331 | 341 | 3.16 | ||
| S-CL-600 | 600 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.27 | 318 | 323 | 2.57 | ||
| S-CL-800 | 800 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.36 | 306 | 295 | 3.4 | ||
| S-CL-1000 | 1000 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.45 | 296 | 277 | 6.62 | ||
| S-CL-1200 | 1200 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.54 | 290 | 271 | 6.52 | ||
| S-CL-1400 | 1400 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.62 | 284 | 267 | 2.49 | ||
| S-CL-1600 | 1600 | 1600 | 14 | 523 | 24.3 | 0.38 | 3800 | 0.71 | 278 | 264 | 6.54 | ||
| Average | 3.52 |
| Study | ID | θu Experiment | Θu Proposed Equation (30) | Error (%) | Θu Yuan [26] | Error (%) |
|---|---|---|---|---|---|---|
| Wang | LA2 | 3.40 | 3.14 | 15.7 | 3.14 | 7.65 |
| Zoppo | CL_FRPa | 10.40 | 7.66 | 23.67 | 8.66 | 16.73 |
| CL_FRPb | 9.60 | 8.95 | 6.76 | 8.95 | 6.77 | |
| CM_FRPa | 9.60 | 7.72 | 15.96 | 7.72 | 19.58 | |
| CM_FRPb | 9.60 | 8.79 | 4.38 | 8.79 | 8.44 | |
| Ouyang | C-B2 | 3.15 | 3.02 | 2.75 | 3.02 | 4.13 |
| C-B3 | 3.11 | 3.67 | 34.69 | 3.67 | 18.01 | |
| C-BC | 3.68 | 3.01 | 17.27 | 3.01 | 18.21 | |
| C-C2 | 3.74 | 3.60 | 9.23 | 3.6 | 3.74 | |
| C-C3 | 3.07 | 4.83 | 68.5 | 4.83 | 57.33 | |
| Wang | N4C3A75 | 2.48 | 2.31 | 14 | 2.31 | 6.85 |
| N4C4A45 | 4.51 | 3.66 | 16.45 | 3.66 | 18.85 | |
| N3C2A55 | 3.84 | 2.81 | 30.7 | 2.81 | 26.82 | |
| N3C3A45 | 4.86 | 3.69 | 20.12 | 3.69 | 24.07 | |
| FEA | S-CL-150 | 5.94 | 2.39 | 16.97 | 3.39 | 42.93 |
| S-CL-200 | 6.56 | 3.43 | 7.8 | 4.43 | 32.47 | |
| S-CL-210 | 6.56 | 3.81 | 10.12 | 4.81 | 26.68 | |
| S-CL-225 | 6.87 | 3.89 | 11.36 | 4.69 | 31.73 | |
| S-CL-250 | 7.18 | 4.01 | 6.88 | 4.81 | 33.01 | |
| S-CL-275 | 7.18 | 4.22 | 6.45 | 5.02 | 30.08 | |
| S-CL-300 | 7.50 | 4.43 | 5.82 | 5.23 | 30.27 | |
| S-CL-325 | 7.81 | 4.65 | 5.25 | 5.44 | 30.35 | |
| S-CL-350 | 8.13 | 4.87 | 4.39 | 5.67 | 30.26 | |
| S-CL-375 | 8.43 | 5.09 | 3.71 | 5.99 | 28.94 | |
| S-CL-400 | 8.75 | 5.33 | 6.19 | 6.43 | 26.51 | |
| S-CL-430 | 9.38 | 5.57 | 7.38 | 6.47 | 31.02 | |
| S-CL-500 | 10.00 | 5.86 | 28.8 | 6.76 | 32.40 | |
| S-CL-600 | 10.60 | 6.34 | 24.9 | 7.43 | 29.91 | |
| S-CL-800 | 10.90 | 6.8 | 20.1 | 6.8 | 37.61 | |
| S-CL-1000 | 11.25 | 7.93 | 18.9 | 7.93 | 29.51 | |
| S-CL-1200 | 11.25 | 9.27 | 5.21 | 9.52 | 15.38 | |
| S-CL-1400 | 11.56 | 11.06 | 10.3 | 10.06 | 12.98 | |
| S-CL-1600 | 12.50 | 13.57 | 21.8 | 14.75 | 18.00 | |
| 15.23 | 23.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Parvin, A.; Alhusban, M. Lateral Deformation Capacity and Plastic Hinge Length of RC Columns Confined with Textile Reinforced Mortar Jackets. CivilEng 2021, 2, 670-691. https://doi.org/10.3390/civileng2030037
Parvin A, Alhusban M. Lateral Deformation Capacity and Plastic Hinge Length of RC Columns Confined with Textile Reinforced Mortar Jackets. CivilEng. 2021; 2(3):670-691. https://doi.org/10.3390/civileng2030037
Chicago/Turabian StyleParvin, Azadeh, and Mohannad Alhusban. 2021. "Lateral Deformation Capacity and Plastic Hinge Length of RC Columns Confined with Textile Reinforced Mortar Jackets" CivilEng 2, no. 3: 670-691. https://doi.org/10.3390/civileng2030037
APA StyleParvin, A., & Alhusban, M. (2021). Lateral Deformation Capacity and Plastic Hinge Length of RC Columns Confined with Textile Reinforced Mortar Jackets. CivilEng, 2(3), 670-691. https://doi.org/10.3390/civileng2030037






