Fiber Reinforced Polymer as Wood Roof-to-Wall Connections to Withstand Hurricane Wind Loads
Abstract
1. Introduction
2. Objectives
3. Experimental Program Adopted for Validation of FEA Model
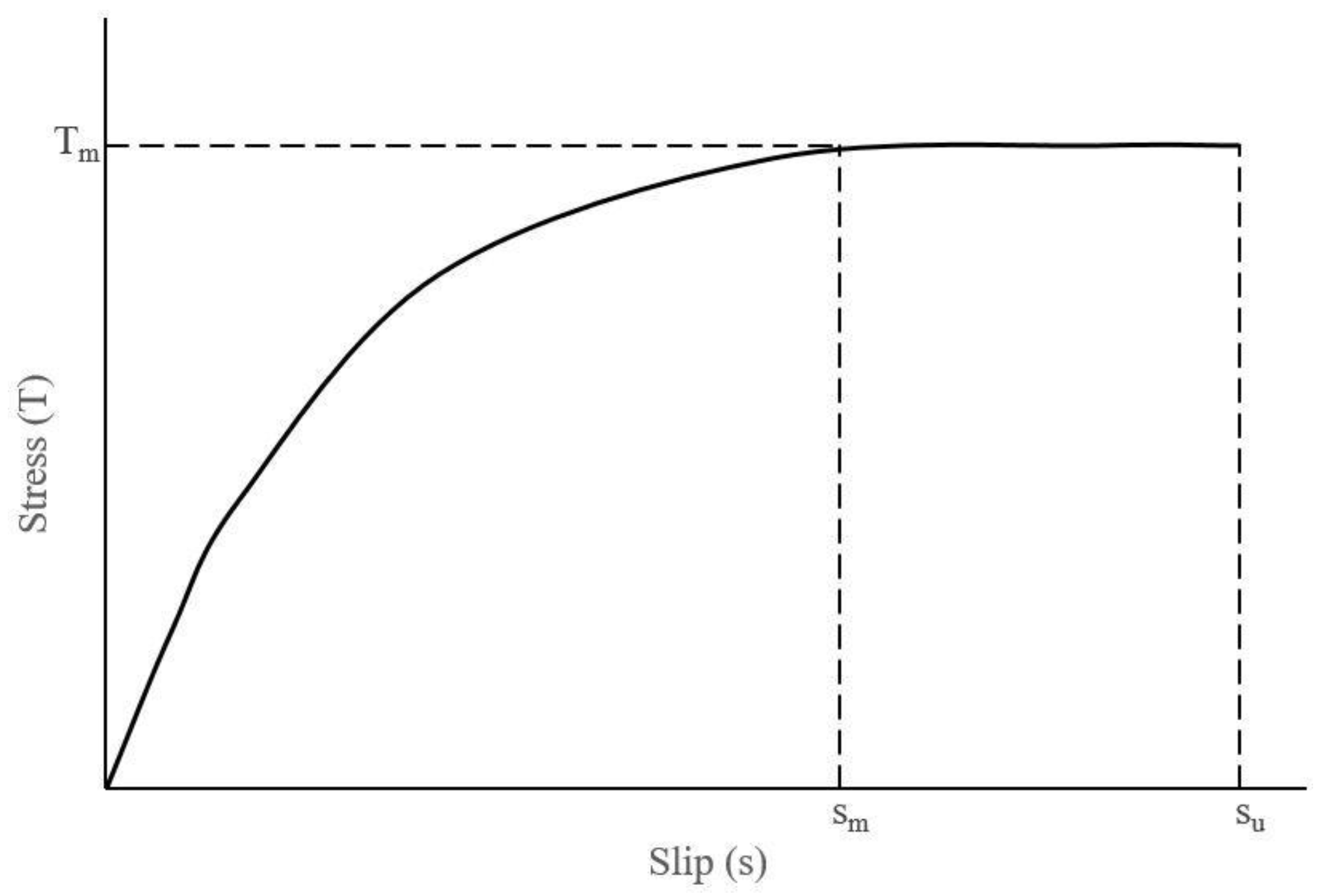
4. Finite Element Analysis Approach
5. Results
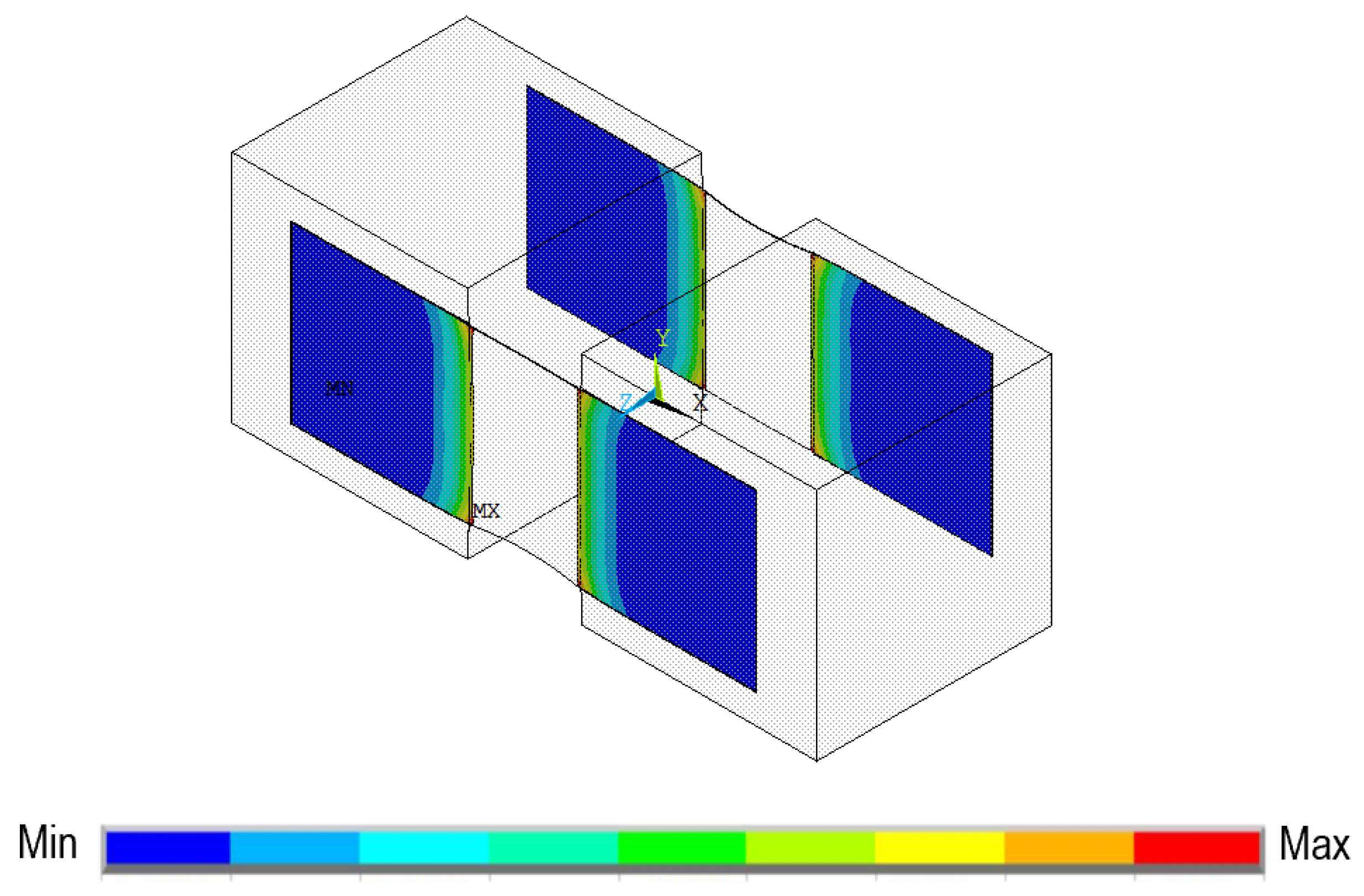
5.1. Comparison of Experiment and Finite Element Analysis Models Results
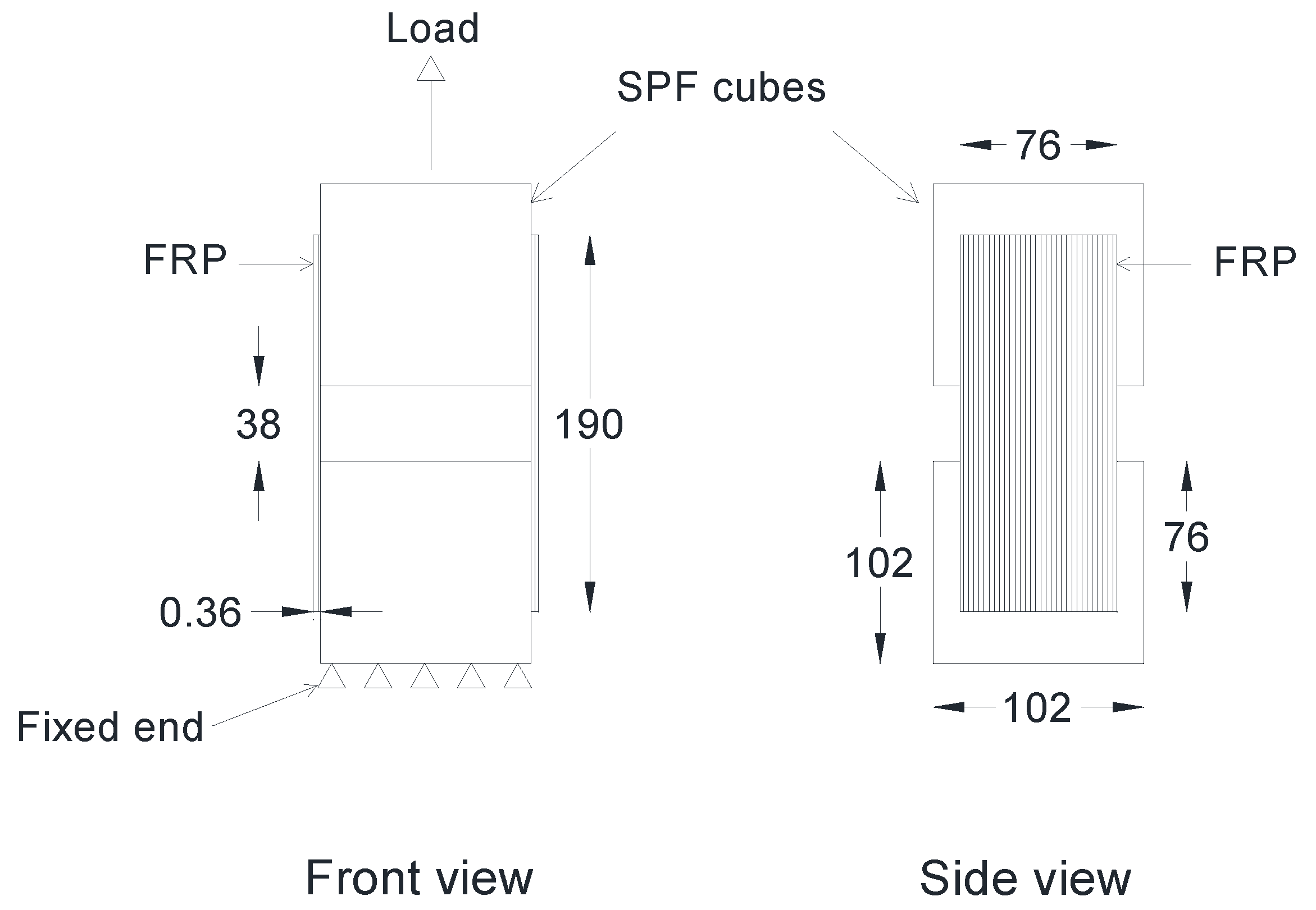
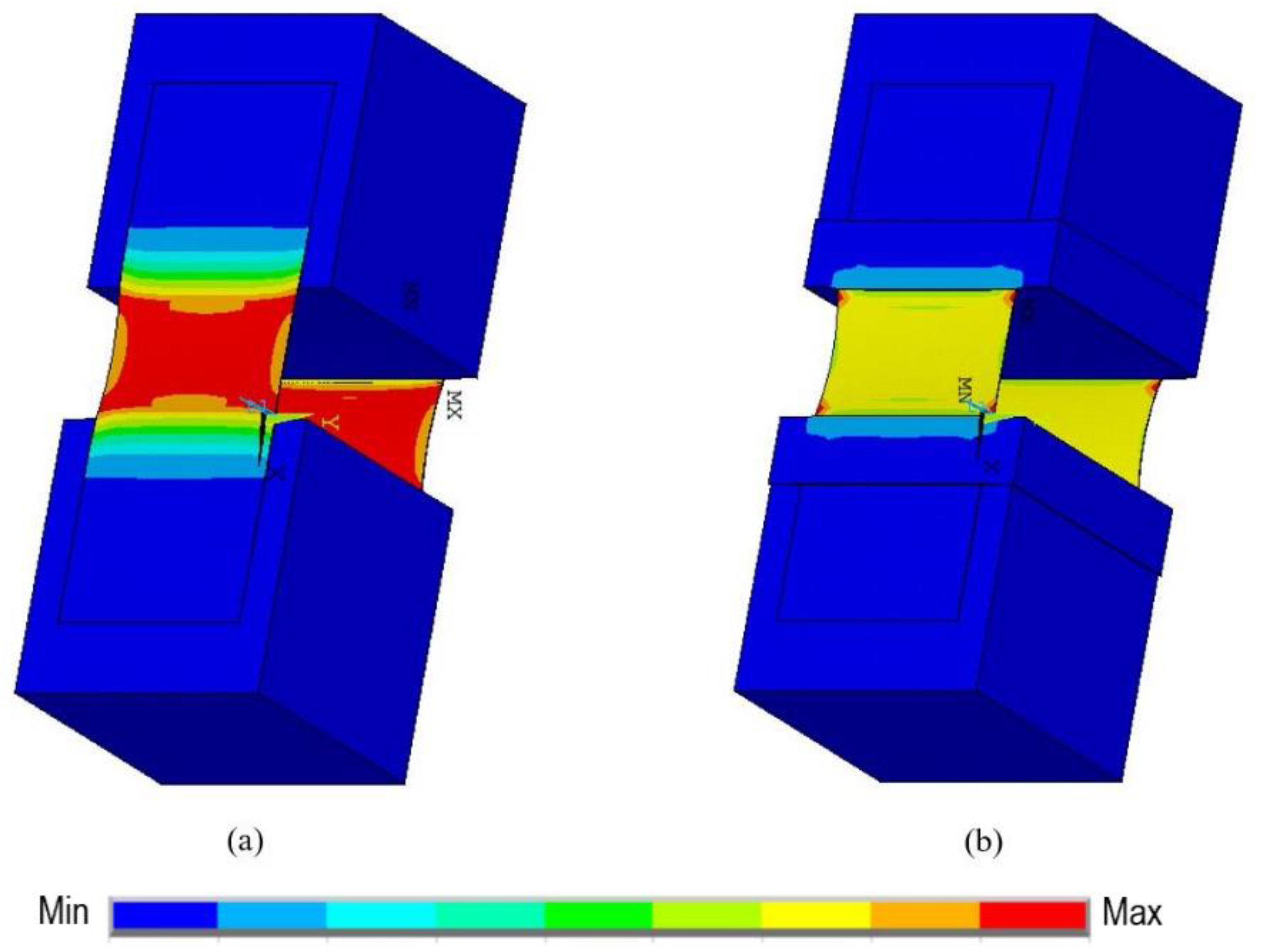
5.1.1. Component Level Double Shear Test
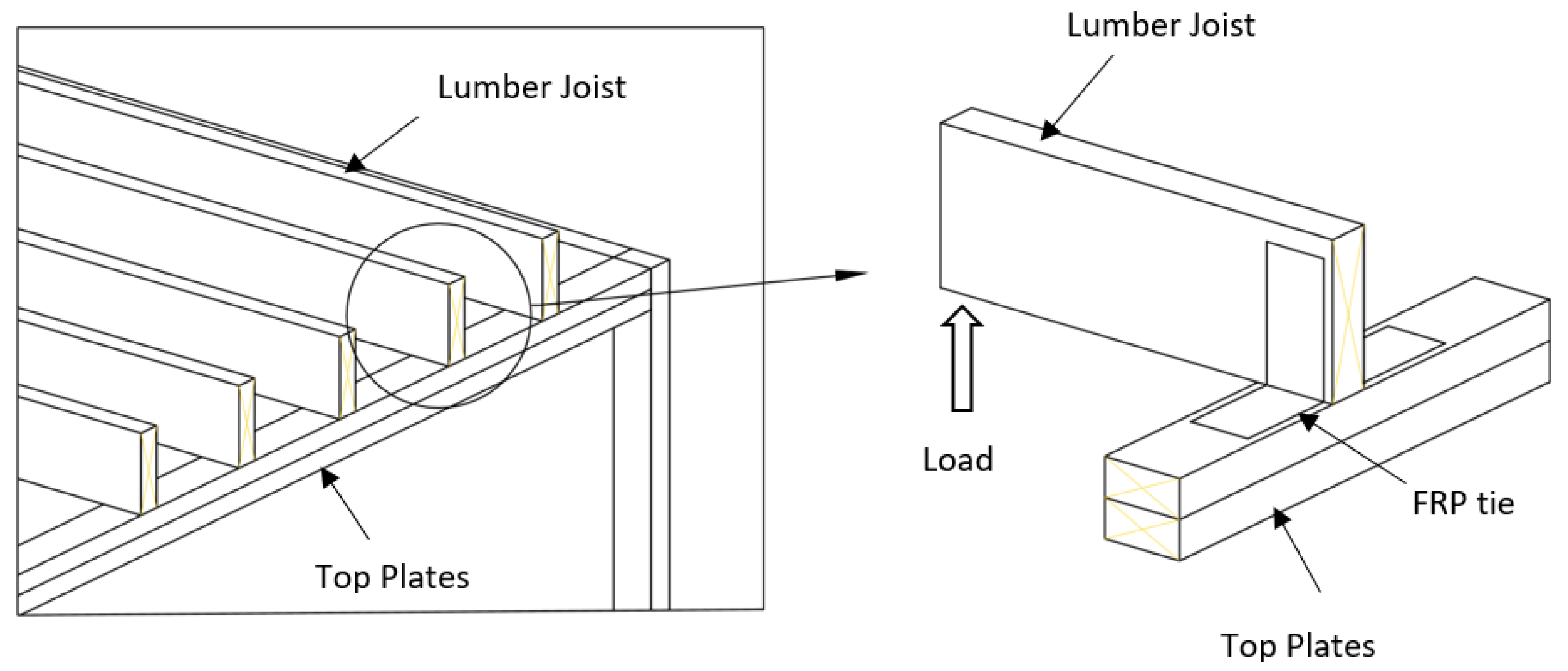
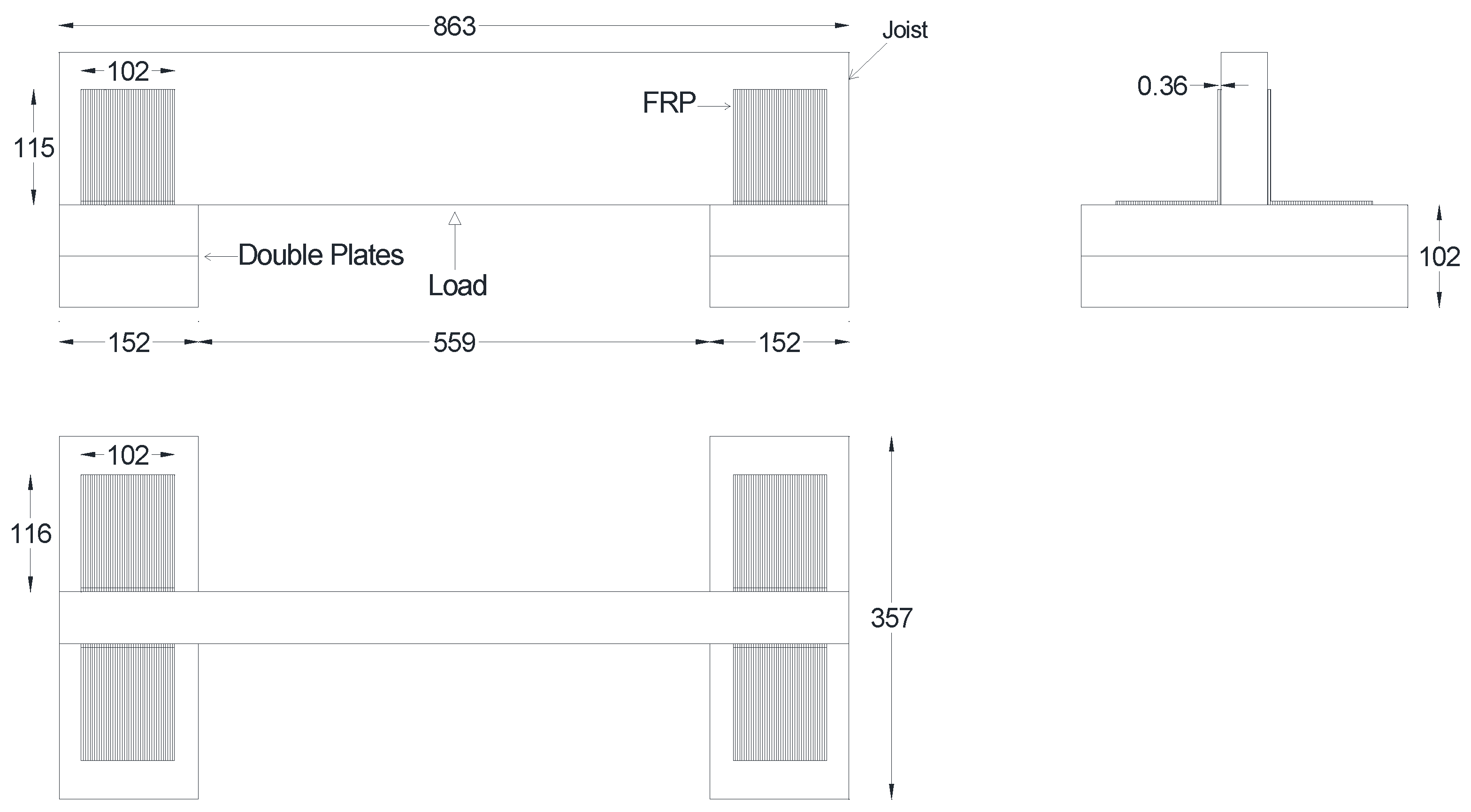
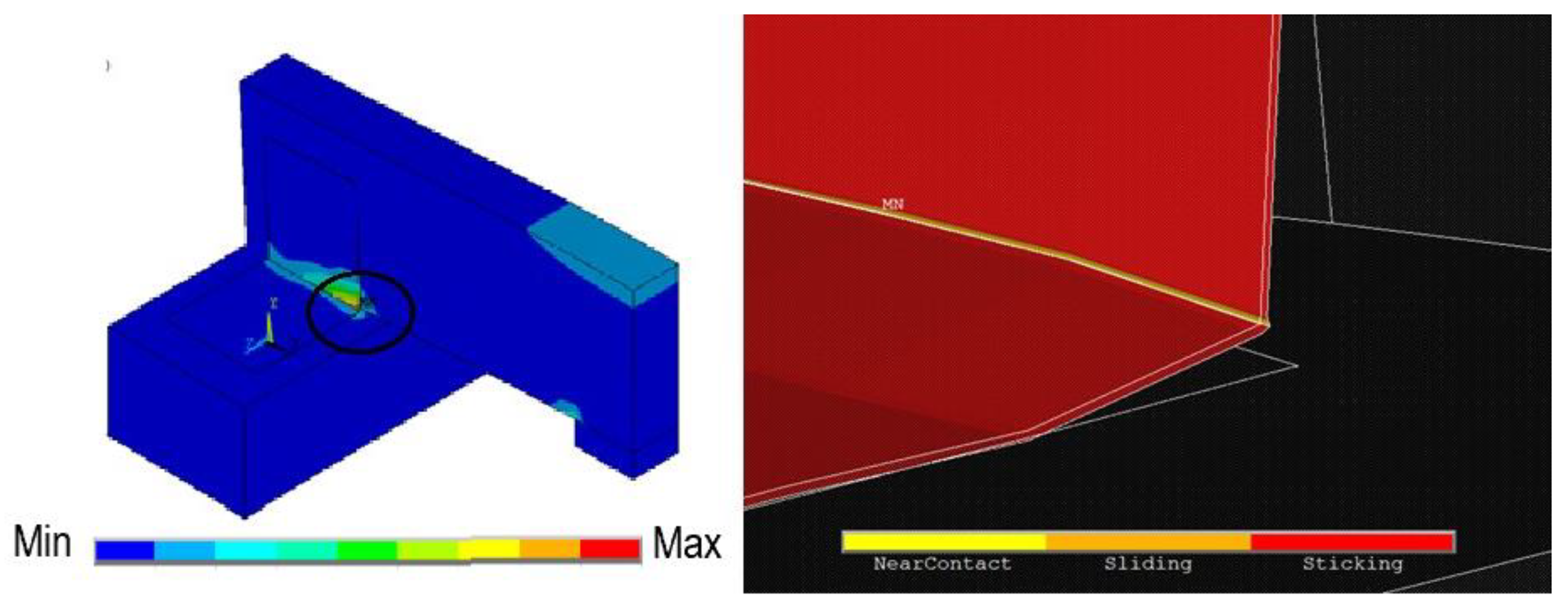
5.1.2. Roof-to-Wall Connection under Uplift Test
5.2. Parametric Study
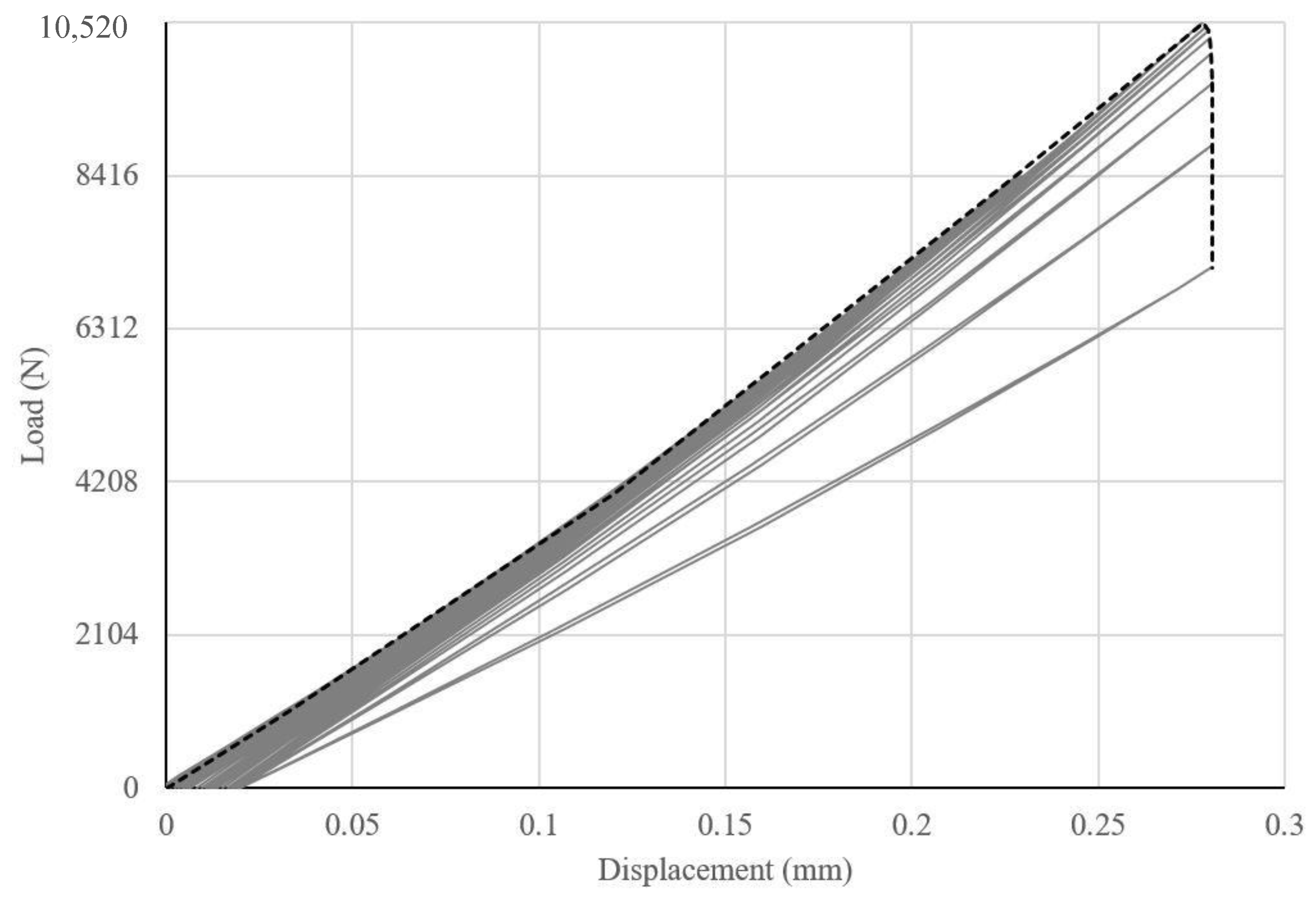
5.2.1. Behavior of Control Model under Cyclic Uniaxial Tensile Load
5.2.2. Effect of Various FRP Types on Roof-to-Wall Connection under Uniaxial Cyclic Load
5.2.3. Effect of Anchorage on the GFRP Ties in Double Shear Test Model
5.2.4. Effect of Anchorage on the GFRP Ties under Uplift Force
5.3. Design Considerations
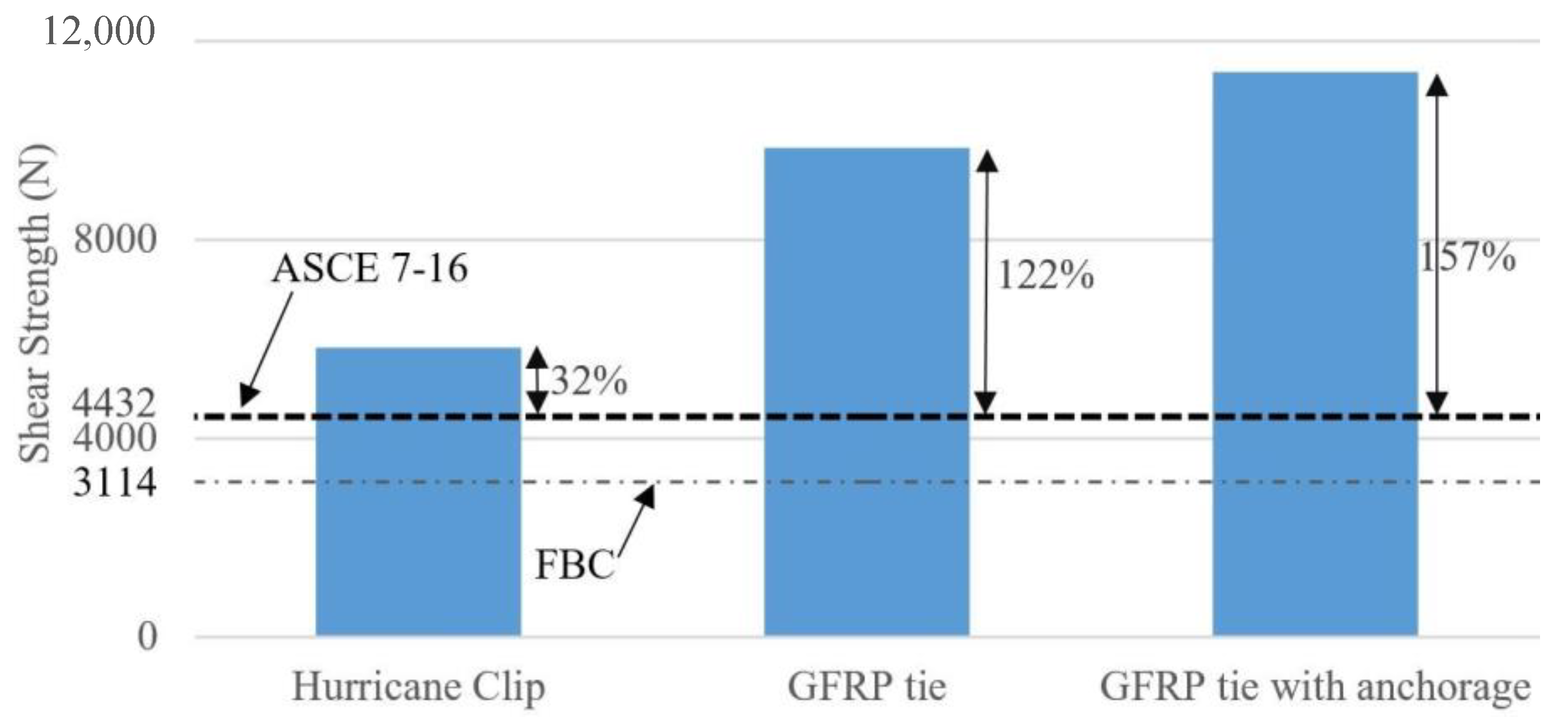
5.3.1. Comparison of Shear and Uplift Forces to ASCE Standards and Traditional Hurricane Clips
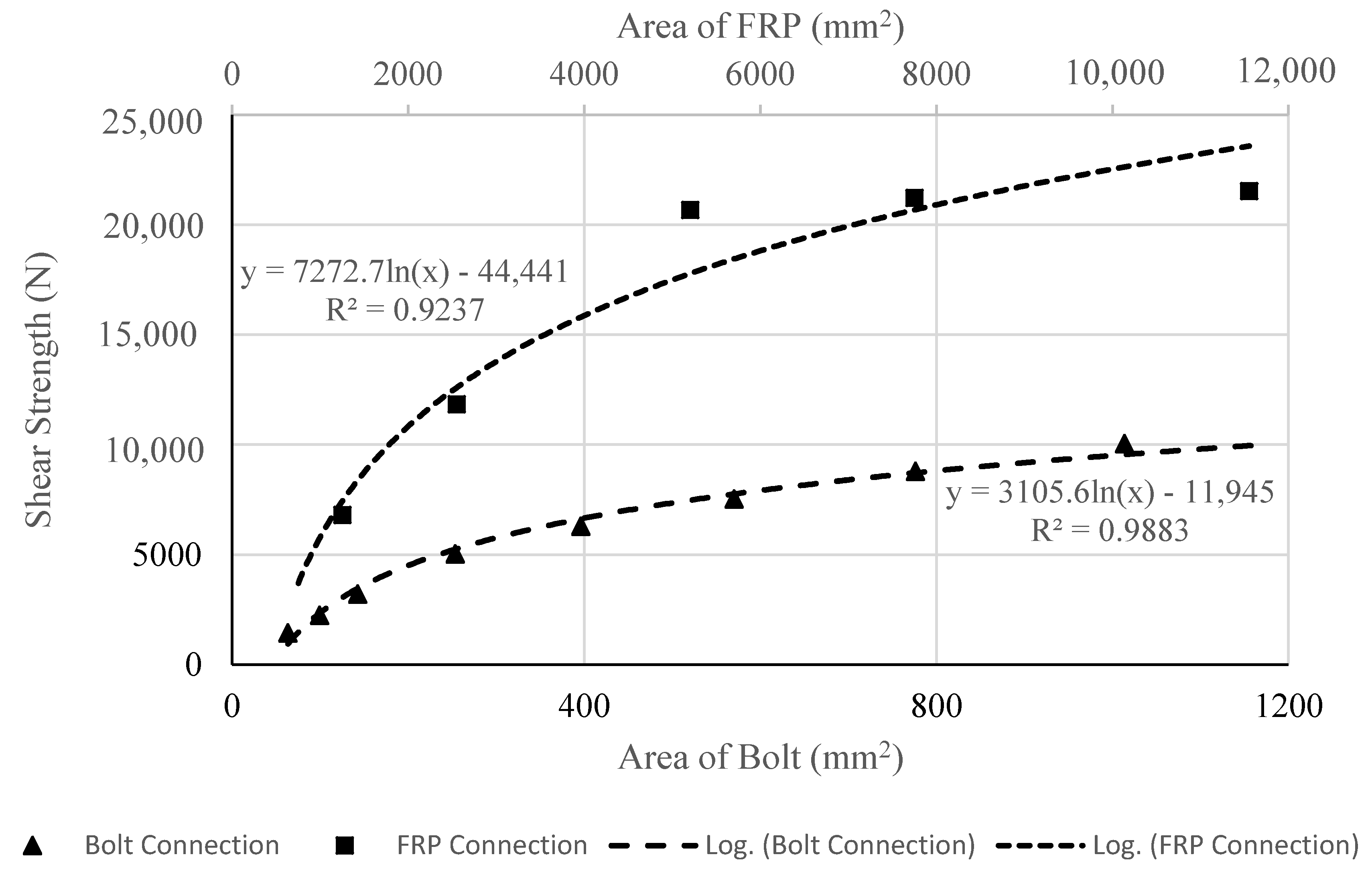
5.3.2. Analytical Model
6. Summary and Conclusions
- The results of TCLS and TCLU finite element control models of GFRP roof-to-wall connections and experimental study were in good agreement with less than 3% discrepancy and similar failure modes were observed under static loading condition.
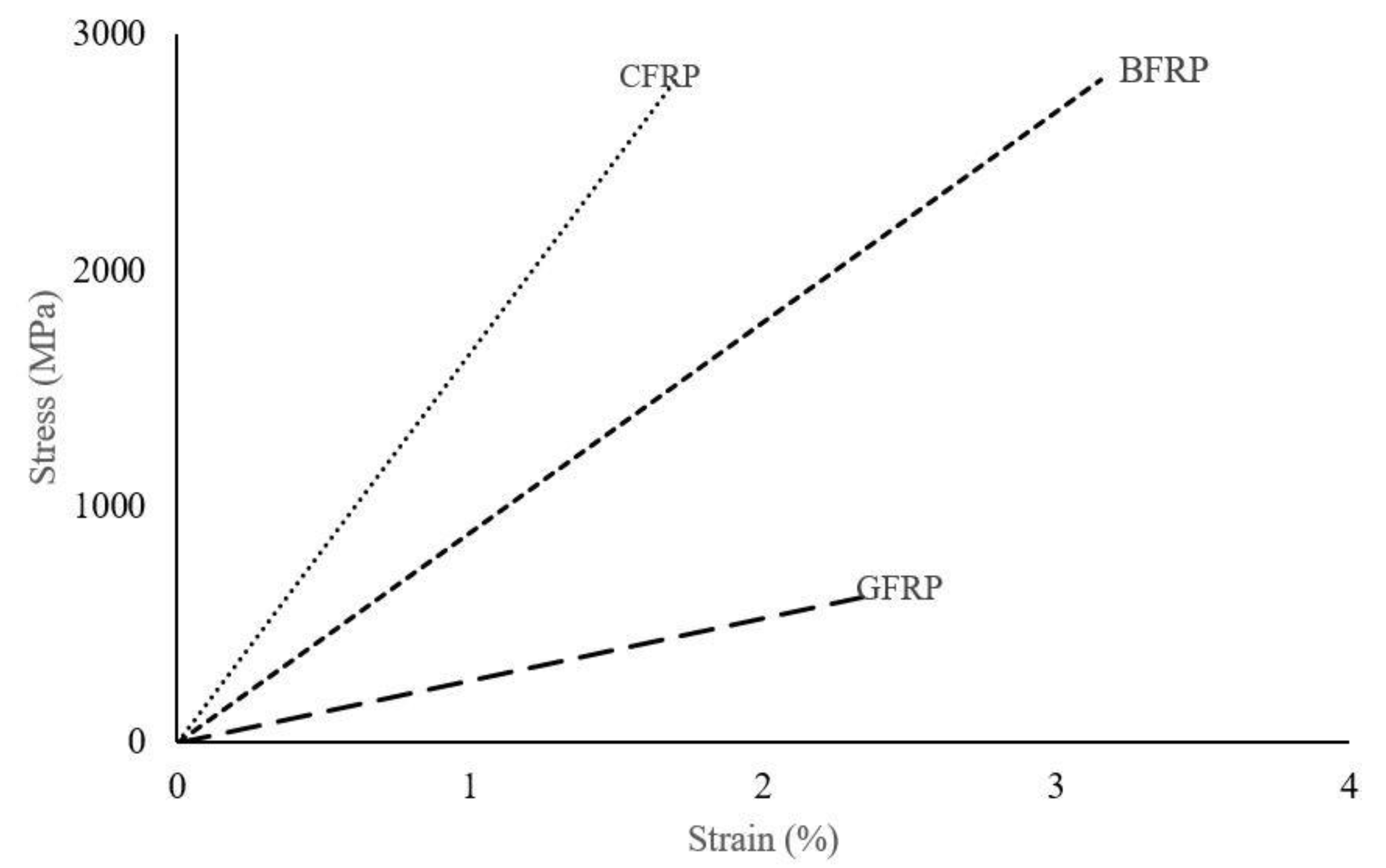
- From load versus displacement data of the roof-to-wall connection under cyclic uplift load (TCLU-CYC FE model) for various FRP types, stiffness and modulus of resilience of the system were calculated. BFRP and CFRP ties showed similar tensile strength. However, CFRP provided more stiffness to the system. In the design, if more energy absorption is desired, GFRP would be a better choice. However, BFRP could provide higher deformation capability.
- Although, the provided anchorages in the double shear model (TCLS-A) changed the model of failure from debonding to the rupture of the GFRP ties, the shear load capacity did not change.
- However, the anchorages provided in the uplift model (TCLU-A) exhibited substantial increase of 15.6% in its load carrying capacity. The mode of failure changed from peeling to debonding.
- The shear and uplift design strengths for the connection was calculated using ASCE design provisions and compared with the hurricane clips and GFRP ties. Shear strength of GFRP ties was 9.5 times the strength of hurricane clip; and GFRP ties withstood 1.7 times more uplift force than a typical hurricane clip. Thus, GFRP ties are much safer connections than typical hurricane clips.
- From the regression models of the GFRP tie and the bolted wood-to-metal connection, the relation between the bonded area of GFRP tie to the area of the bolt in bolted connection was established. Subsequently, shear strength of the GFRP tie connection, with an area equal to 10 times the bolt area, is equivalent to π/ts times the shear strength of the bolted connection calculated using NDS for wood construction.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ellingwood, B.R.; Rosowsky, D.V.; Li, Y.; Kim, J.H. Fragility assessment of light-frame wood construction subjected to wind and earthquake hazards. J. Struct. Eng. 2004, 130, 1921–1930. [Google Scholar] [CrossRef]
- Lin, T.J.; LaFave, J.M. Experimental structural behavior of wall-diaphragm connections for older masonry buildings. Constr. Build. Mater. 2012, 26, 180–189. [Google Scholar] [CrossRef]
- Van De Lindt, J.W.; Graettinger, A.; Gupta, R.; Skaggs, T.; Pryor, S.; Fridley, K.J. Performance of wood-frame structures during hurricane Katrina. J. Perform. Constr. Facil. 2007, 21, 108–116. [Google Scholar] [CrossRef]
- Morrison, M.J.; Henderson, D.J.; Kopp, G.A. The response of a wood-frame, gable roof to fluctuating wind loads. Eng. Struct. 2012, 41, 498–509. [Google Scholar] [CrossRef]
- Riley, M.A.; Sadek, F. Experimental Testing of Roof to Wall Connections in Wood Frame Houses; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2003.
- Cheng, J. Testing and analysis of the toe-nailed connection in the residential roof-to-wall system. For. Prod. J. 2004, 54, 58–65. [Google Scholar]
- Canbek, C. Development of a Hurricane-Resistant Roof-To-Wall Connection Using High-Performance Fiber Composites; Florida International University: Miami, FL, USA, 2009. [Google Scholar]
- National Association of Home Builders (NAHB). Roof Framing Connections in Conventional Residential Construction; Rep No. H-21172CA; National Association of Home Builders (NAHB): Washington, DC, USA, 2002; pp. 1–48. [Google Scholar]
- Reed, T.D.; Rosowsky, D.V.; Schiff, S.D. Uplift capacity of light-frame rafter to top plate connections. J. Archit. Eng. 1997, 3, 156–163. [Google Scholar] [CrossRef]
- Ku, H.; Wang, H.; Pattarachaiyakoop, N.; Trada, M. A review on the tensile properties of natural fiber reinforced polymer composites. Compos. Part B: Eng. 2011, 10, 213–222. [Google Scholar] [CrossRef]
- ACI (American Concrete Institute). Guide for the Design and Construction of Structural Concrete Reinforced with FRP Bars; ACI 440.1R-06; ACI: Farmington Hills, MI, USA, 2006. [Google Scholar]
- Korany, Y.; Drysdale, R. Rehabilitation of masonry walls using unobtrusive FRP techniques for enhanced out-of-plane seismic resistance. J. Compos. Constr. 2006, 10, 213–222. [Google Scholar] [CrossRef]
- Nanni, A. North american design guidelines for concrete reinforcement and strengthening using FRP: Principles, applications and unresolved issues. Constr. Build. Mater. 2003, 17, 439–446. [Google Scholar] [CrossRef]
- Parvin, A.; Altay, S.; Yalcin, C.; Kaya, O. CFRP rehabilitation of concrete frame joints with inadequate shear and anchorage details. J. Compos. Constr. 2010, 14, 72–82. [Google Scholar] [CrossRef]
- Parvin, A.; Jamwal, A.S. Performance of externally FRP reinforced columns for changes in angle and thickness of the wrap and concrete strength. Compos. Struct. 2006, 73, 451–457. [Google Scholar] [CrossRef]
- Parvin, A.; Shah, T.S. Fiber reinforced polymer strengthening of structures by near-surface mounting method. Polymers 2016, 8, 298. [Google Scholar] [CrossRef]
- Kim, Y.J.; Hossain, M.; Harries, K.A. CFRP strengthening of timber beams recovered from a 32 year old quonset: Element and system level tests. Eng. Struct. 2013, 57, 213–221. [Google Scholar] [CrossRef]
- Wang, B.; Bachtiar, E.V.; Yan, L.; Kasal, B.; Fiore, V. Strengthened wood beams: An experimental study. Polymers 2019, 11, 9–12. [Google Scholar] [CrossRef]
- Yang, J.-Q.; Smith, T.; Wu, Y.-F.; Feng, P. Strengthening single-bolt timber joints with externally bonded CFRP composites. In Structures; Elsevier: Amsterdam, The Netherlands, 2020; Volume 28, pp. 2671–2685. [Google Scholar]
- De Lorenzis, L.; Scialpi, V.; La Tegola, A. Analytical and experimental study on bonded-in FRP bars in glulam timber. Compos. Part B Eng. 2004, 36, 279–289. [Google Scholar] [CrossRef]
- Jia, J.; Davalos, J.F. An artificial neural network for the fatigue study of bonded FRP—Wood interfaces. Compos. Struct. 2006, 74, 106–114. [Google Scholar] [CrossRef]
- Canbek, C.; Mirmiran, A.; Chowdhury, A.G.; Suksawang, N. Development of fiber-reinforced polymer roof-to-wall connection. J. Compos. Constr. 2011, 15, 644–652. [Google Scholar] [CrossRef]
- Azzi, Z.; Elawady, A.; Chowdhury, A.G. Determining the Efficacy of a Retrofit Technique for Residential Buildings Using Holistic Testing. In Structures Congress 2020, St. Louis, MO, USA, 5–8 April, 2020; American Society of Civil Engineers: Reston, VA, USA, 2020. [Google Scholar]
- ASCE. Minimum Design Loads for Buildings and Other Structures; American Society of Civil Engineers Inc.: Chicago, IL, USA, 2016. [Google Scholar]
- Kim, Y.J.; Harries, K.A. Modeling of timber beams strengthened with various CFRP composites. Eng. Struct. 2010, 32, 3225–3234. [Google Scholar] [CrossRef]
- Fonseca, E.M.M.; Coelho, D.C.S.; Barreira, L.M.S. Structural safety in wooden beams under thermal and mechanical loading conditions. Int. J. Saf. Secur. Eng. 2012, 2, 242–255. [Google Scholar] [CrossRef][Green Version]
- Bagale, B.R.; Parvin, A. Fiber-Reinforced Polymer Strengthening of Steel Beams under Static and Fatigue Loading Practice Periodical on Structural Design and Construction. Pract. Period. Struct. Des. Constr. 2021, 26, 04020046. [Google Scholar] [CrossRef]
- ANSYS® Academic Research Mechanical Release 18; Help System; ANSYS, Inc.: Canonsburg, PA, USA, 2018; Available online: http://www.ansys.com/. (accessed on 27 September 2018).
- Molina, M.; Cruz, J.J.; Oller, S.; Barbat, A.H.; Gil, L. Behaviour of the interface between concrete and FRP using serial/parallel mixing theory. Ingeniería e Investigación 2013, 31, 26–39. [Google Scholar]
- Narmashiri, K.; Jumaat, M.Z. Reinforced steel I-beams: A comparison between 2D and 3D simulation. Simul. Model. Pract. Theory 2011, 19, 564–585. [Google Scholar] [CrossRef]
- Biscaia, H.C.; Chastre, C.; Borba, I.S. Experimental evaluation of bonding between CFRP laminates and different structural materials. J. Compos. Constr. 2016, 20, 04015070. [Google Scholar] [CrossRef]
- Fiedler, B.; Hojo, M.; Ochiai, S.; Schulte, K.; Ando, M. (2001) Failure behavior of an epoxy matrix under different kinds of static loading. Compos. Sci. Technol. 2001, 61, 1615–1624. [Google Scholar] [CrossRef]
- Parvin, A.; Wang, W. Concrete columns confined by fiber composite wraps under combined axial and cyclic lateral loads. Compos. Struct. 2002, 58, 539–549. [Google Scholar] [CrossRef]
- Ceroni, F. Experimental performances of RC beams strengthened with FRP materials. Constr. Build. Mater. 2010, 24, 1547–1559. [Google Scholar] [CrossRef]
- Sika Corporation Sika CarboDur Carbon Fiber Laminate for Structural Strengthening. 2011. Available online: www.sikausa.com (accessed on 11 January 2019).
- Singha, K. A short review on basalt fiber. Int. J. Text. Sci. 2012, 1, 19–28. [Google Scholar] [CrossRef]
- Grelle, S.V.; Sneed, L.H. Review of anchorage systems for externally bonded FRP laminates. Int. J. Concr. Struct. Mater. 2013, 7, 17–33. [Google Scholar] [CrossRef]
- Aiello, M.A.; Ombres, L. Moment redistribution in continuous fiber-reinforced polymer-strengthened reinforced concrete beams. ACI Struct. J. 2011, 108. [Google Scholar] [CrossRef]
- Kalfat, R.; Al-Mahaidi, R.; Smith, S.T. Anchorage devices used to improve the performance of reinforced concrete beams retrofitted with FRP composites: State-of-the-art review. J. Compos. Constr. 2013, 17, 14–33. [Google Scholar] [CrossRef]
- Chowdhury, A.G.; Mirmiran, A.; Canino, I. Development of Effective Roof-To Wall Connection for Low-Rise Buildings to Withstand Hurricane Wind Loads–Phase 1: Literature Review and Concept Development; Florida International University: Miami, FL, USA, 2007. [Google Scholar]
- Florida Building Code (FBC). Building, Wood; Florida Building Code: Tallahassee, FL, USA, 2007. [Google Scholar]
- National Forest Products Association. National Design Specification for Wood Construction: Recommended Practice for Structural Design; National Forest Products Association: Quincy, MA, USA, 2018. [Google Scholar]

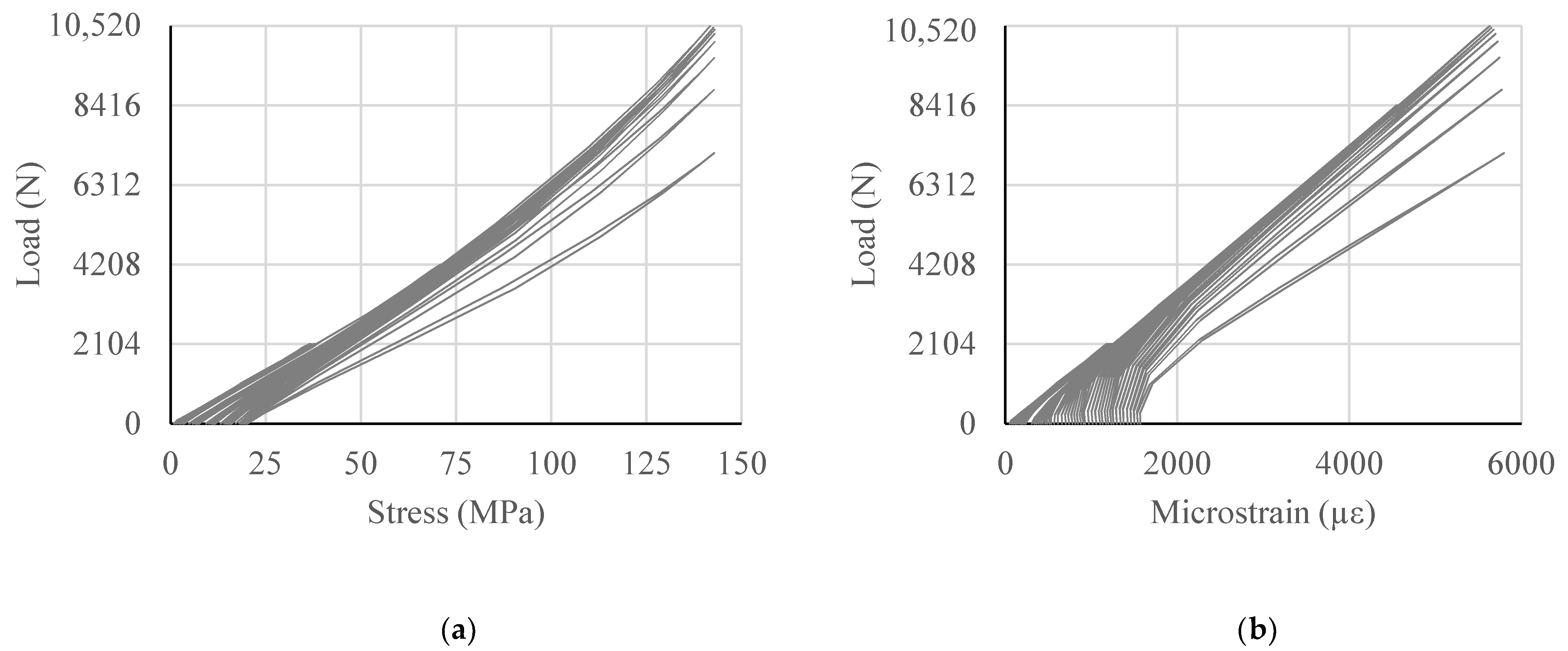

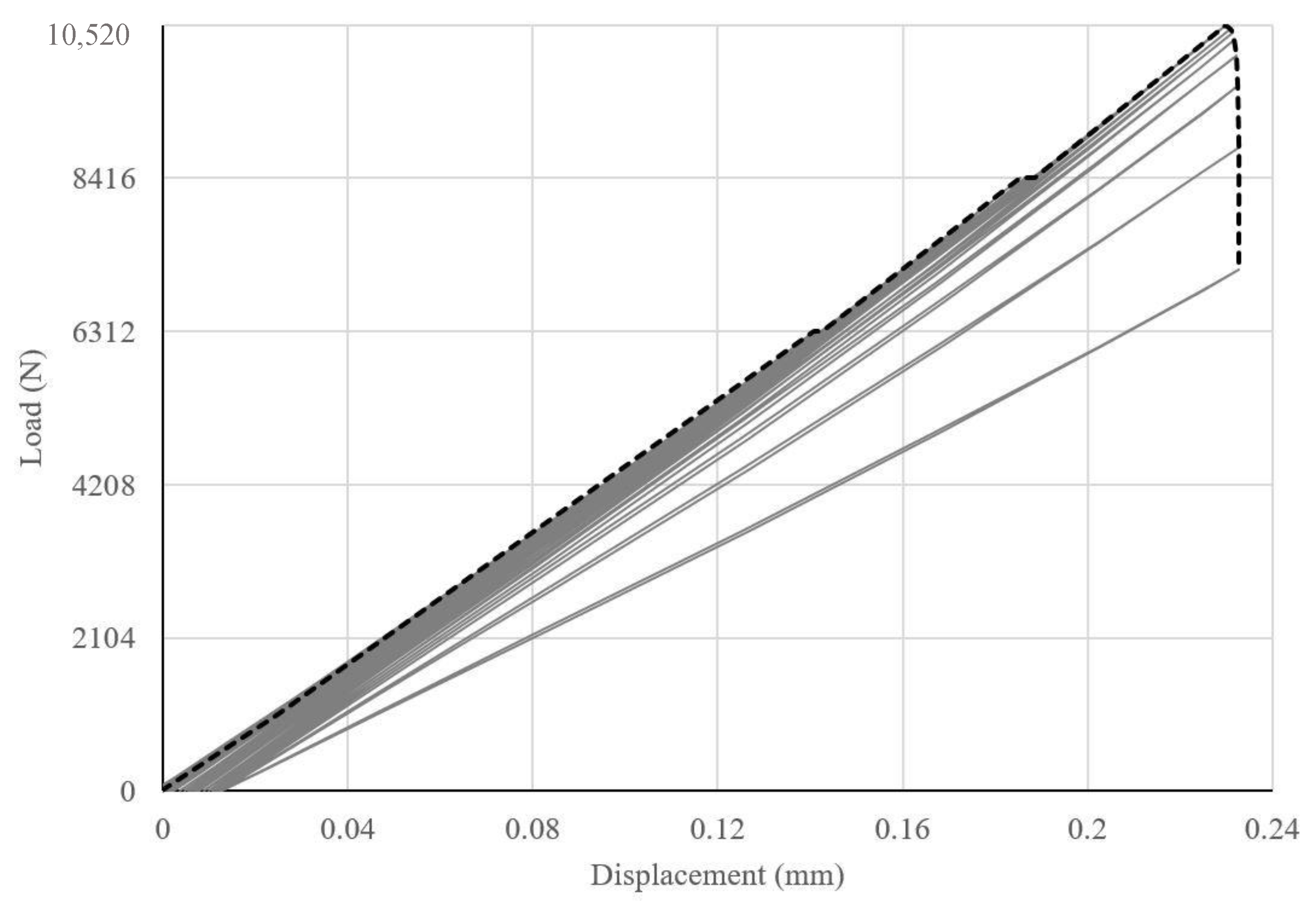
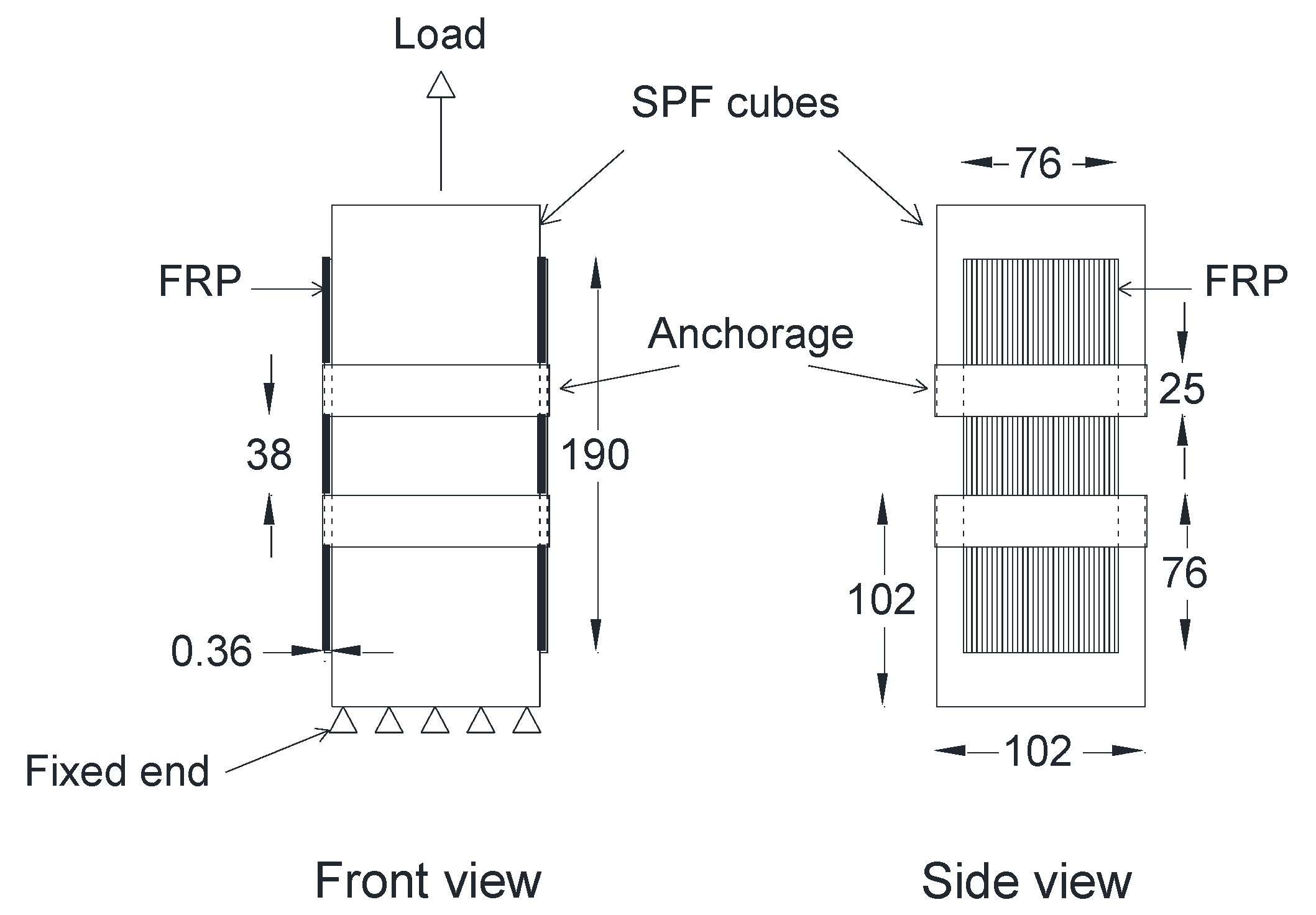
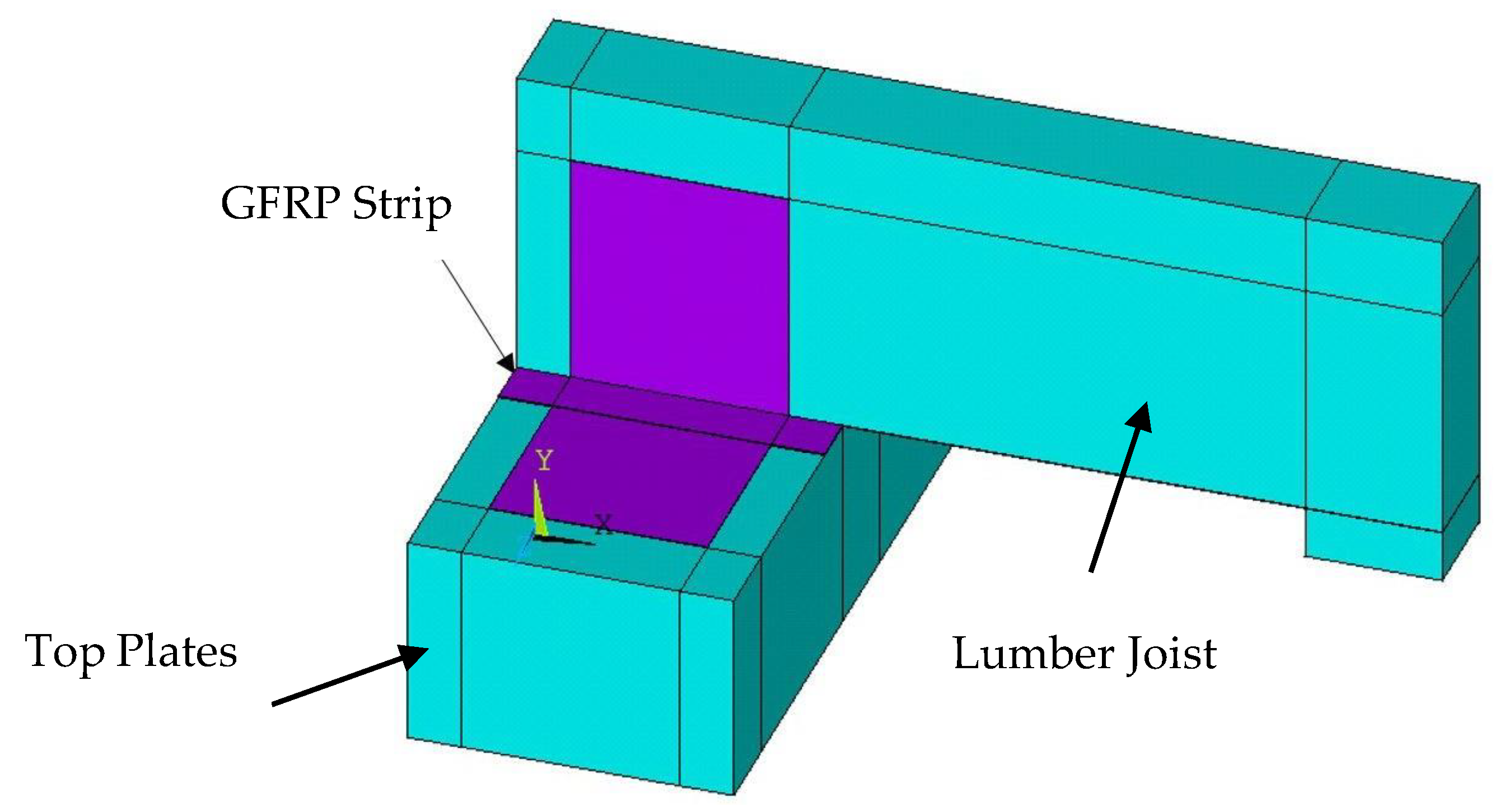


| Test | Failure Load (N) | Discrepancy (%) | Mode of Failure | |
|---|---|---|---|---|
| Experiment | FEA Model | |||
| Shear | 21,540 | 20,867.55 | 3.12 | Debonding |
| Uplift | 9606.67 | 9855.52 | 2.59 | Delamination |
| Model ID | Description | Objective |
|---|---|---|
| TCLU-CYC | FEA model of uplift test component under uniaxial cyclic load | To simulate wind load more realistically |
| TCLU-CYC-G, C, B | FEA model of uplift test component with glass, carbon, and basalt ties under uniaxial cyclic load | To observe the effect of FRP material types as ties |
| TCLS-A | FEA model of shear test component with anchorage | To observe the effect of anchorage in increasing shear load capacity |
| TCLU-A | FEA model of uplift test component with anchorage | To observe the effect of anchorage in increasing uplift load capacity |
| FRP Type | Elastic Modulus (GPa) | Tensile Strength (MPa) | Ultimate Strain (%) | Poisson’s Ratio |
|---|---|---|---|---|
| GFRP [22] | 26.12 | 612 | 2.34 | 0.3 |
| CFRP [22] | 165 | 2800 | 1.69 | 0.3 |
| BFRP [32] | 89 | 2804 | 3.15 | 0.3 |
| Model ID | Load (N) | Mode of Failure | Discrepancy |
|---|---|---|---|
| TCLS | 20,867.55 | Debonding | 3.25% |
| TCLS-A | 20,231.20 | Rupture of fiber |
| Model ID | Load (N) | Mode of Failure | Discrepancy |
|---|---|---|---|
| TCLU | 9855.52 | Delamination | 15.55% |
| TCLU-A | 11,387.68 | Debonding |
| Load Type | Strength Required (N) | Strength Capacity (N) | |||
|---|---|---|---|---|---|
| FBC | ASCE 7-16 | Hurricane clips | GFRP ties (FEA validated models) | GFRP ties with anchorage | |
| Shear | - | 2058 | 2202 | 10,434 | 10,116 |
| Uplift | 3114 | 4432 | 5832 | 9856 | 11,388 |
| Model ID | Area (mm2) | Shear Strength (N) |
|---|---|---|
| A’ (Validated FE model) | 5776 | 20,868 |
| A | 5776 | 21,540 |
| B | 3876 | 21,230 |
| C | 2601 | 20,680 |
| D | 1275 | 11,850 |
| E | 625 | 6800 |
| Metal Plate | Thickness, ts (mm) | Equation (7) | Regression | Discrepancy |
|---|---|---|---|---|
| 14 gauge | 1.63 | 1.927 | 1.944 | 0.83% |
| 16 gauge | 1.26 | 2.435 | 2.377 | 2.38% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dhakal, A.; Parvin, A. Fiber Reinforced Polymer as Wood Roof-to-Wall Connections to Withstand Hurricane Wind Loads. CivilEng 2021, 2, 652-669. https://doi.org/10.3390/civileng2030036
Dhakal A, Parvin A. Fiber Reinforced Polymer as Wood Roof-to-Wall Connections to Withstand Hurricane Wind Loads. CivilEng. 2021; 2(3):652-669. https://doi.org/10.3390/civileng2030036
Chicago/Turabian StyleDhakal, Aman, and Azadeh Parvin. 2021. "Fiber Reinforced Polymer as Wood Roof-to-Wall Connections to Withstand Hurricane Wind Loads" CivilEng 2, no. 3: 652-669. https://doi.org/10.3390/civileng2030036
APA StyleDhakal, A., & Parvin, A. (2021). Fiber Reinforced Polymer as Wood Roof-to-Wall Connections to Withstand Hurricane Wind Loads. CivilEng, 2(3), 652-669. https://doi.org/10.3390/civileng2030036