On the Free Vibration and the Buckling Analysis of Laminated Composite Beams Subjected to Axial Force and End Moment: A Dynamic Finite Element Analysis
Abstract
:1. Introduction
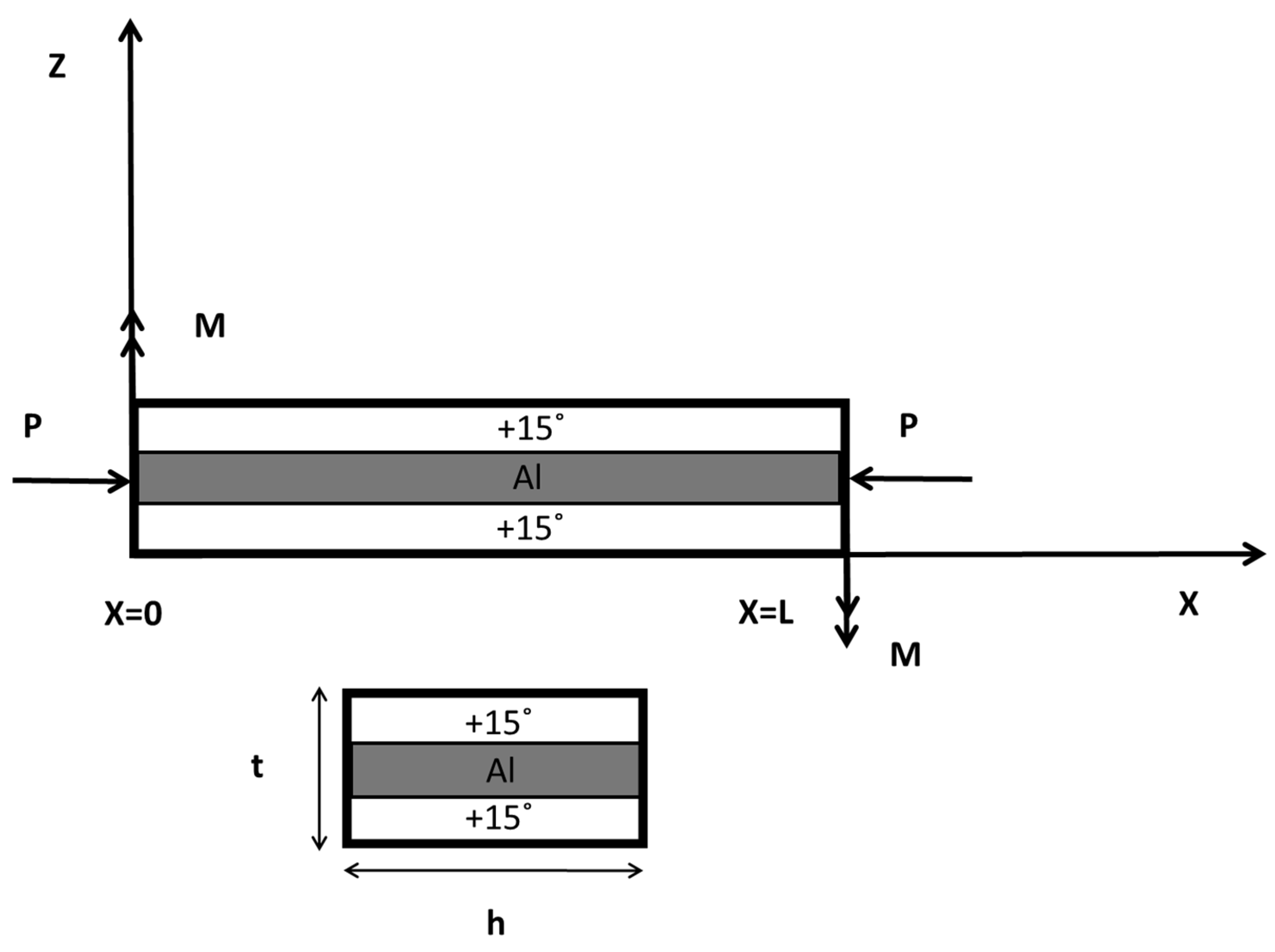
2. Materials and Methods
2.1. Finite Element Formulation (FEM)
2.2. Dynamic Finite Element (DFE) Formulation
3. Results and Discussion
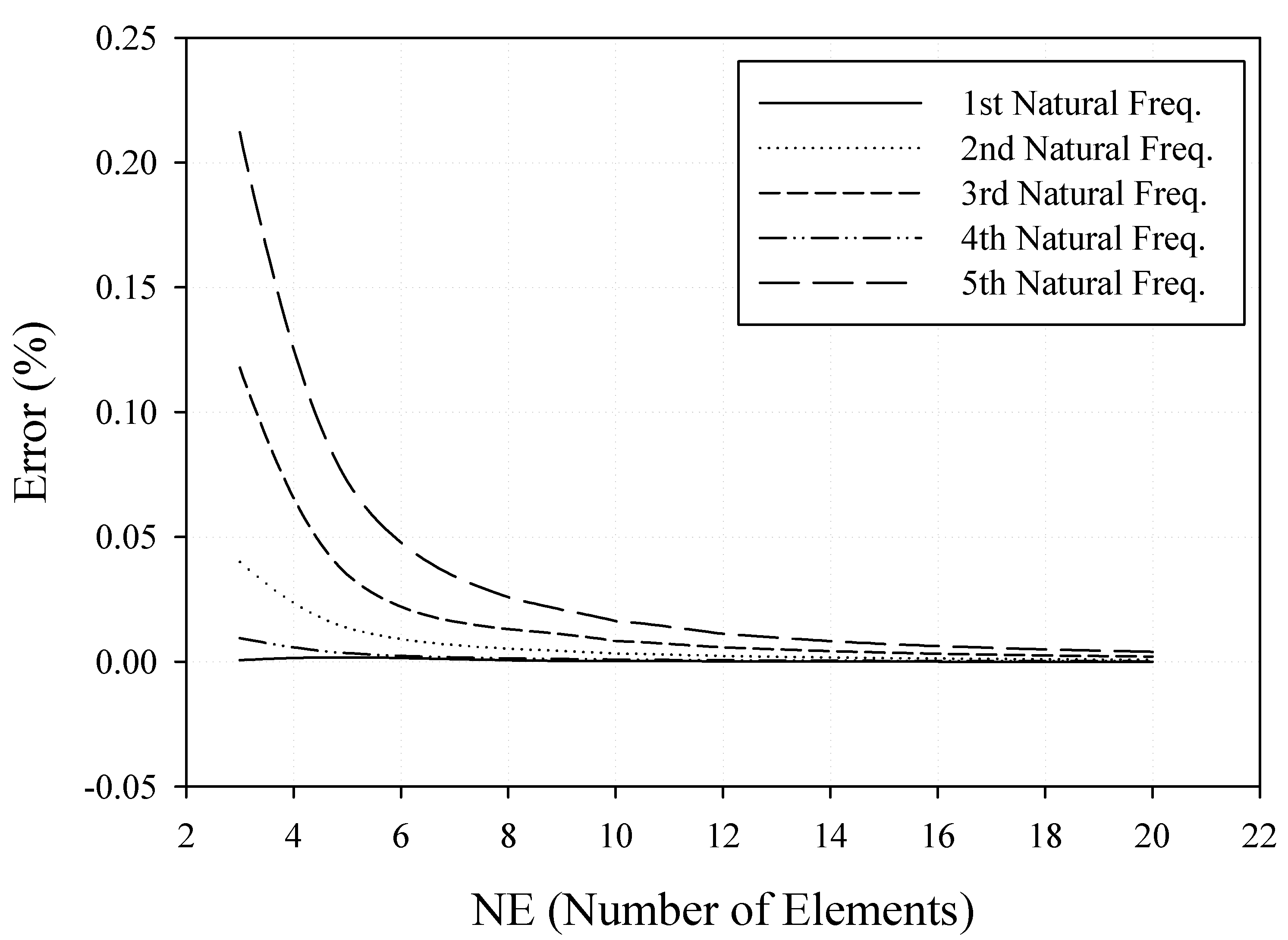
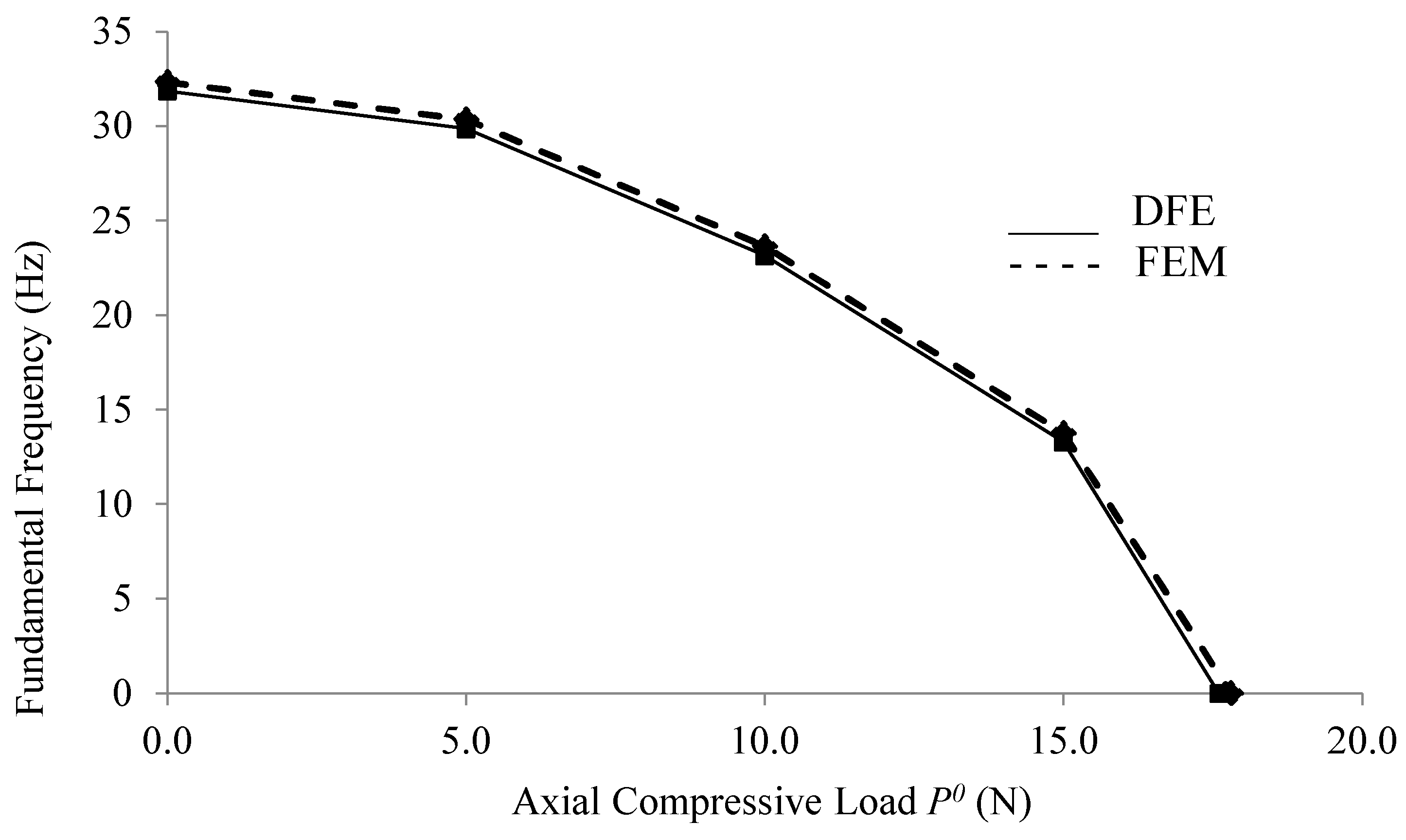
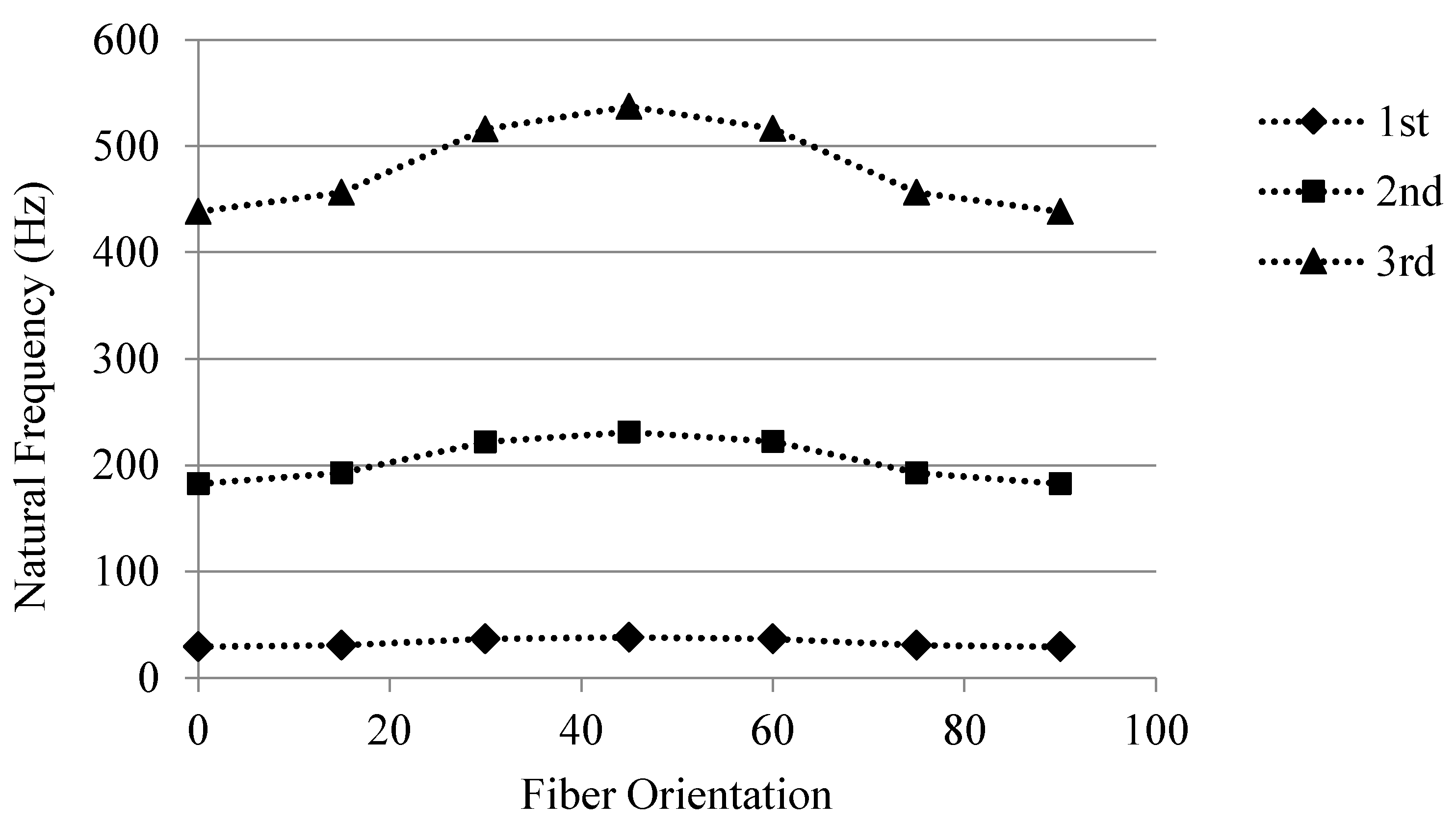
3.1. Single-Layer Glass/Epoxy Composite Beam
3.2. Three-Layer Fiber-Metal Laminated (FML) Beam
4. Discussion
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jones, R.M. Mechanics of Composite Materials, 2nd ed.; Taylor and Francis Inc.: London, UK, 1998. [Google Scholar]
- Berthelot, J.M. Composite Materials Mechanical Behaviour and Structural Analysis; Springer: New York, NY, USA, 1999. [Google Scholar]
- Hashemi, S.M.; Borneman, S. Doubly-Coupled Vibrations of Nonuniform Composite Wings: A Dynamic Finite Element. Math. Probl. Eng. Aerosp. Sci. 2011, 5, 141–152. [Google Scholar]
- Abramovich, H.; Livshits, A. Free Vibration of Non-Symmetric Cross-Ply Laminated Composite Beams. J. Sound Vib. 1994, 176, 597–612. [Google Scholar] [CrossRef]
- Jaehong, L.; Kim, S. Flexural-Torsional Coupled Composite Beams with Channel Sections. Comput. Struct. 2002, 80, 133–144. [Google Scholar]
- Chen, X.L.; Liu, G.R.; Lim, S.P. An Element Free Galerkin Method for the Free Vibration Analysis of Composite Laminates of Complicated Shape. Compos. Struct. 2003, 59, 279–289. [Google Scholar] [CrossRef]
- Jung, S.N.; Nagaraj, V.T.; Chopra, I. Refined Structural Dynamics Model for Composite Rotor Blades. AIAA J. 2001, 39, 339–348. [Google Scholar] [CrossRef]
- Bathe, K.-J. Finite Element Procedures in Engineering Analysis; Prentice Hall: Hoboken, NJ, USA, 1982. [Google Scholar]
- Kalousek, V. Dynamics in Engineering Structures; Butterworths: London, UK, 1973. [Google Scholar]
- Banerjee, J.R.; Williams, F.W. Free Vibration of Composite Beams—An Exact Method Using Symbolic Computation. J. Aircr. 1995, 32, 636–642. [Google Scholar] [CrossRef]
- Wittrick, W.; Williams, F. A General Algorithm for Computing Natural Frequencies of Elastic Structures. Q. J. Mech. Appl. Math. 1971, 24, 263–284. [Google Scholar] [CrossRef]
- Banerjee, J.R.; Williams, F.W. Exact Dynamic Stiffness Matrix for Composite Timoshenko Beams With Applications. J. Sound Vib. 1996, 194, 573–585. [Google Scholar] [CrossRef]
- Banerjee, J.R. Free Vibration of Axially Loaded Composite Timoshenko Beams Using the Dynamic Stiffness Matrix Method. Comput. Struct. 1998, 69, 197–208. [Google Scholar] [CrossRef]
- Hashemi, S.M.; Roach, A. Dynamic Finite Element Analysis of Extensional-Torsional Coupled Vibration in Nonuniform Composite Beams. Appl. Compos. Mater. 2011, 18, 521–538. [Google Scholar] [CrossRef]
- Kashani, M.; Jayasinghe, S.; Hashemi, S.M. On the Flexural-Torsional Vibration and Stability of Beams Subjected to Axial Load and End Moment. Shock. Vib. 2014, 2014, 153532. [Google Scholar] [CrossRef]
- Lottati, I. Flutter and Divergence Aeroelastic Characteristics for Composite Forward Swept Cantilevered Wing. J. Aircr. 1985, 22, 1001–1007. [Google Scholar] [CrossRef]
- Joshi, A. Vibration of Thin-Walled Tubular Structures in the Presence of Static Axial Stress Fields. Ph.D. Thesis, Department of Aeronautical Engineering, Indian Institute of Technology, Bombay, India, 1983. [Google Scholar]
- Banerjee, J.R.; Su, H.; Jayatunga, C. A dynamic stiffness element for free vibration analysis of composite beams and its application to aircraft wings. Comput. Struct. 2008, 86, 573–579. [Google Scholar] [CrossRef]

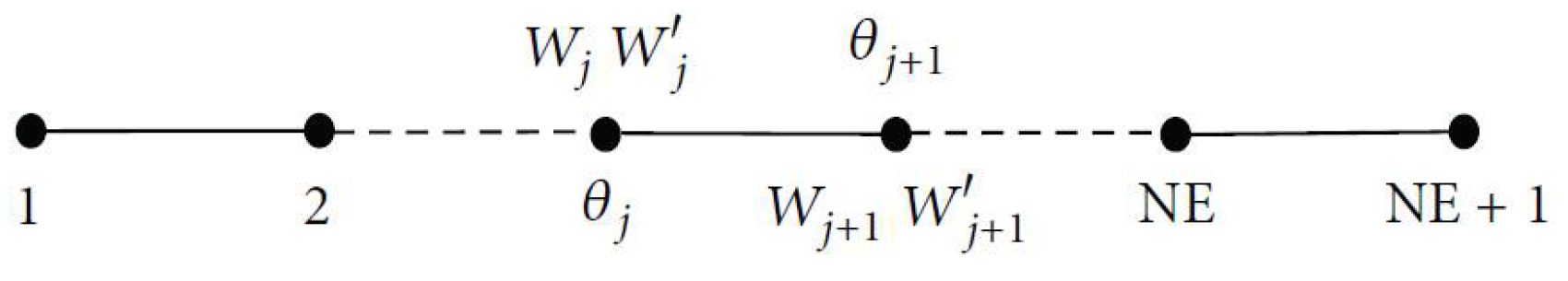
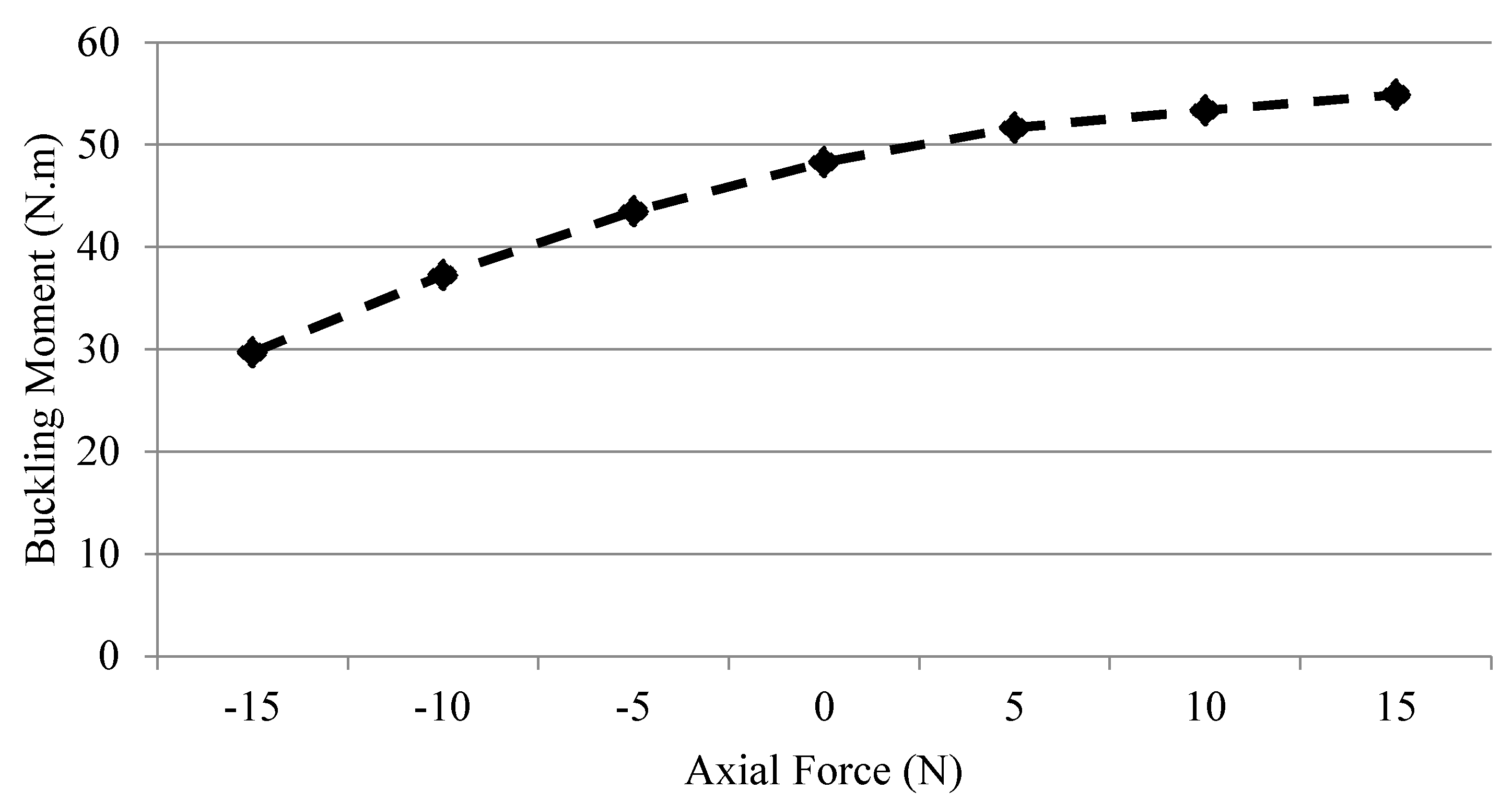


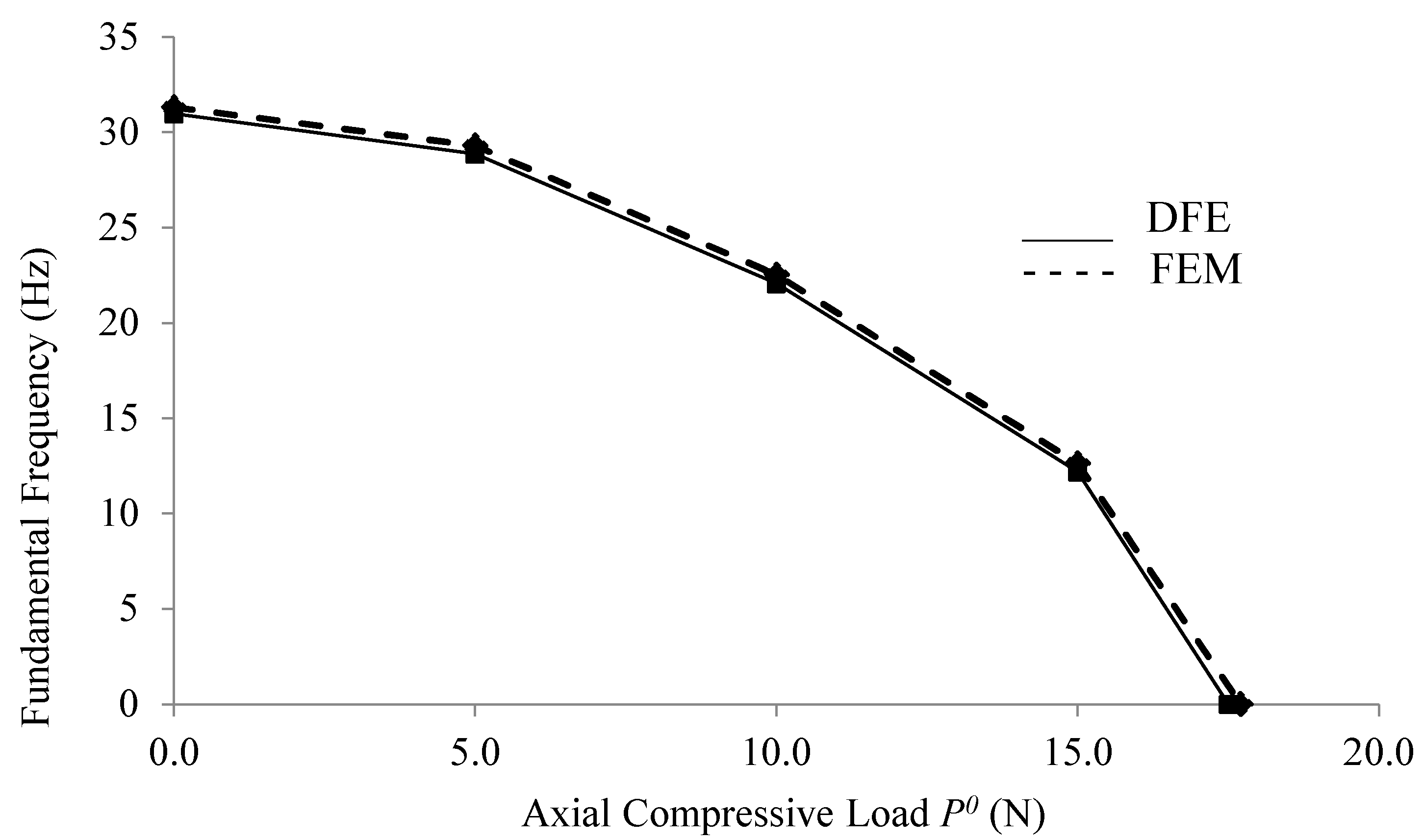
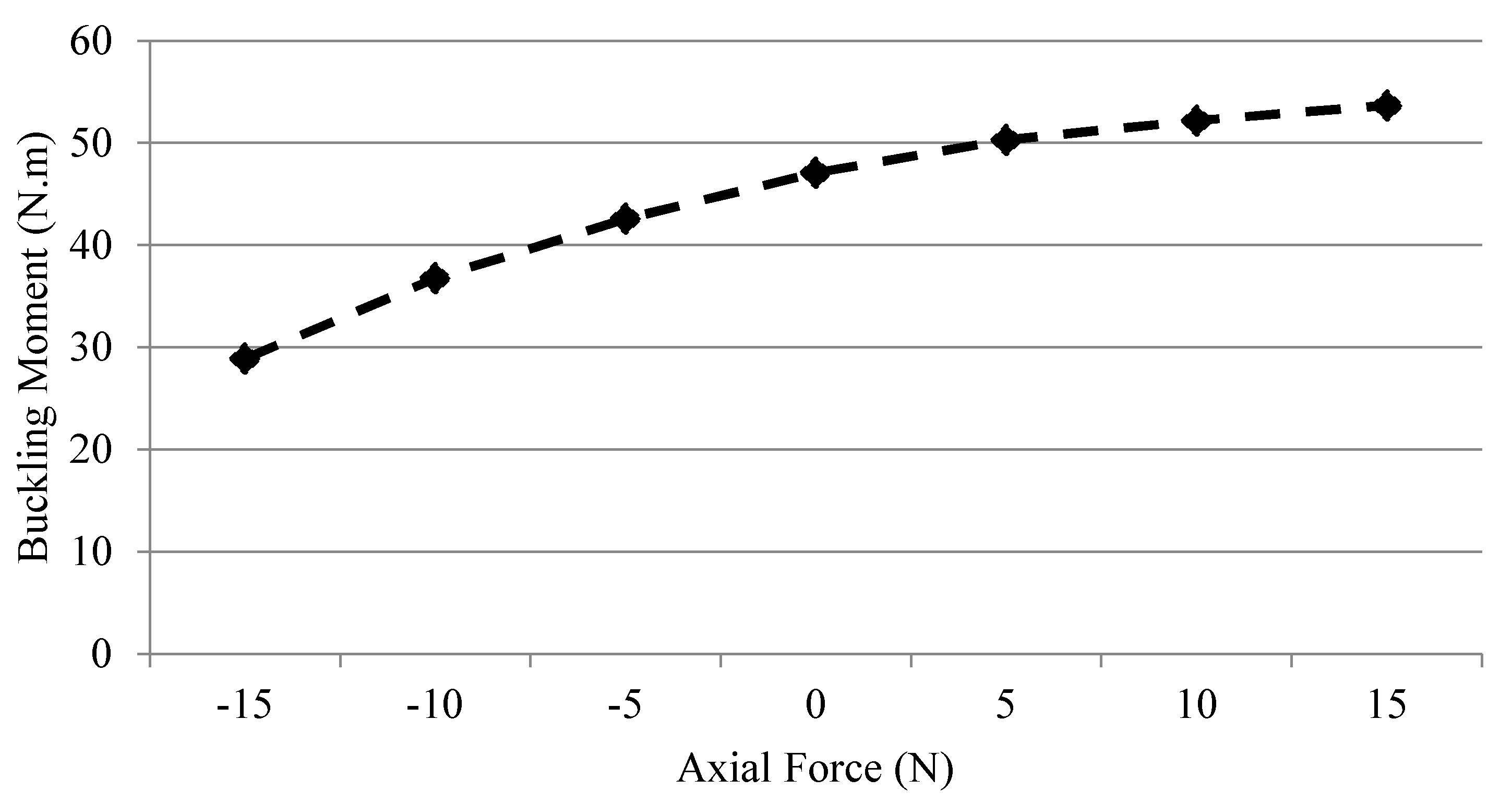
| Natural Frequency | Exact DSM [12] | 5 FEM Elements | 5 FEM |Error|% | 5 DFE Elements | 5 DFE |Error|% | 1 DFE Element | DFE |Error|% |
|---|---|---|---|---|---|---|---|
| 1st | 30.82 | 30.82 | 0.00% | 30.82 | 0.00% | 30.82 | 0.00% |
| 2nd | 192.72 | 192.87 | 0.08% | 192.72 | 0.00% | 192.72 | 0.00% |
| 3rd | 537.38 | 538.47 | 0.19% | 537.38 | 0.00% | 537.38 | 0.00% |
| 4th | 648.73 | 648.87 | 0.02% | 648.73 | 0.00% | 648.73 | 0.00% |
| 5th | 1049.73 | 1053.87 | 0.39% | 1049.73 | 0.00% | 1049.73 | 0.00% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kashani, M.; Hashemi, S.M. On the Free Vibration and the Buckling Analysis of Laminated Composite Beams Subjected to Axial Force and End Moment: A Dynamic Finite Element Analysis. Appl. Mech. 2022, 3, 210-226. https://doi.org/10.3390/applmech3010015
Kashani M, Hashemi SM. On the Free Vibration and the Buckling Analysis of Laminated Composite Beams Subjected to Axial Force and End Moment: A Dynamic Finite Element Analysis. Applied Mechanics. 2022; 3(1):210-226. https://doi.org/10.3390/applmech3010015
Chicago/Turabian StyleKashani, MirTahmaseb, and Seyed M. Hashemi. 2022. "On the Free Vibration and the Buckling Analysis of Laminated Composite Beams Subjected to Axial Force and End Moment: A Dynamic Finite Element Analysis" Applied Mechanics 3, no. 1: 210-226. https://doi.org/10.3390/applmech3010015
APA StyleKashani, M., & Hashemi, S. M. (2022). On the Free Vibration and the Buckling Analysis of Laminated Composite Beams Subjected to Axial Force and End Moment: A Dynamic Finite Element Analysis. Applied Mechanics, 3(1), 210-226. https://doi.org/10.3390/applmech3010015






