Investigation on Residual Compressive Distribution of High-Strength Steel for Bridges by Base Metal Hammer Impact Peening
Abstract
1. Introduction
2. Measurement of Residual Stress
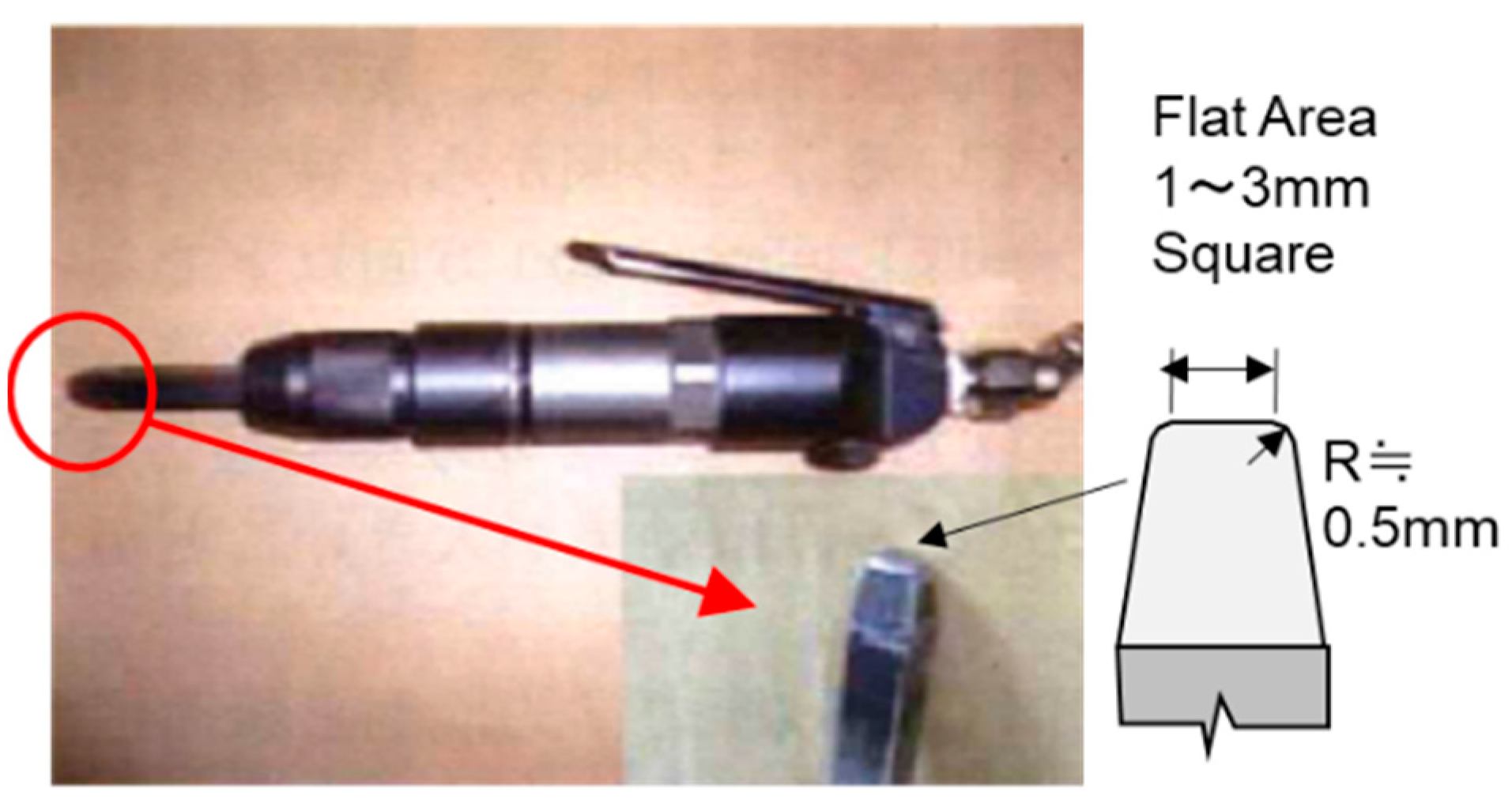
2.1. Specimens and Test Conditions
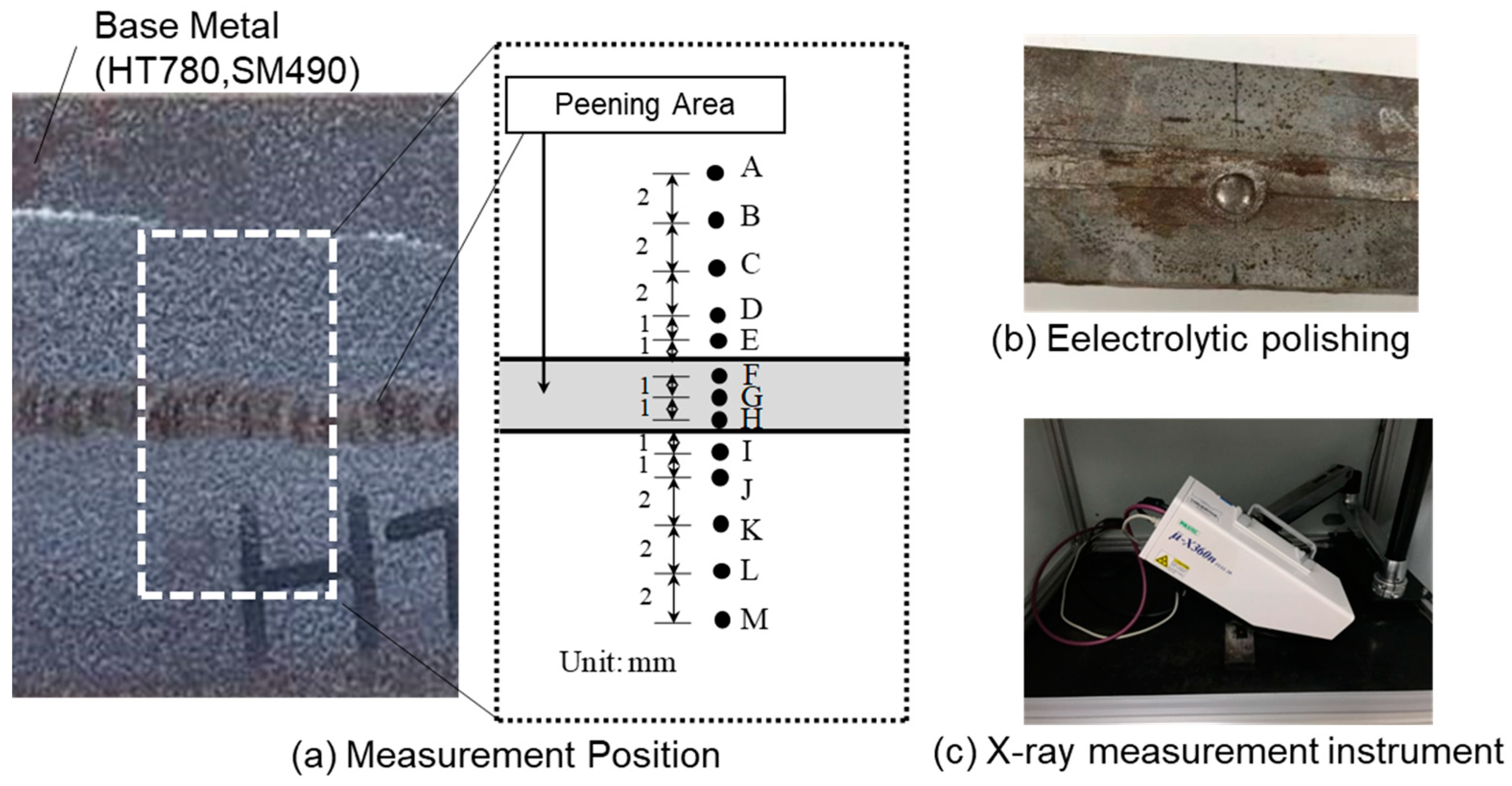
2.2. Method for Measuring Residual Stress
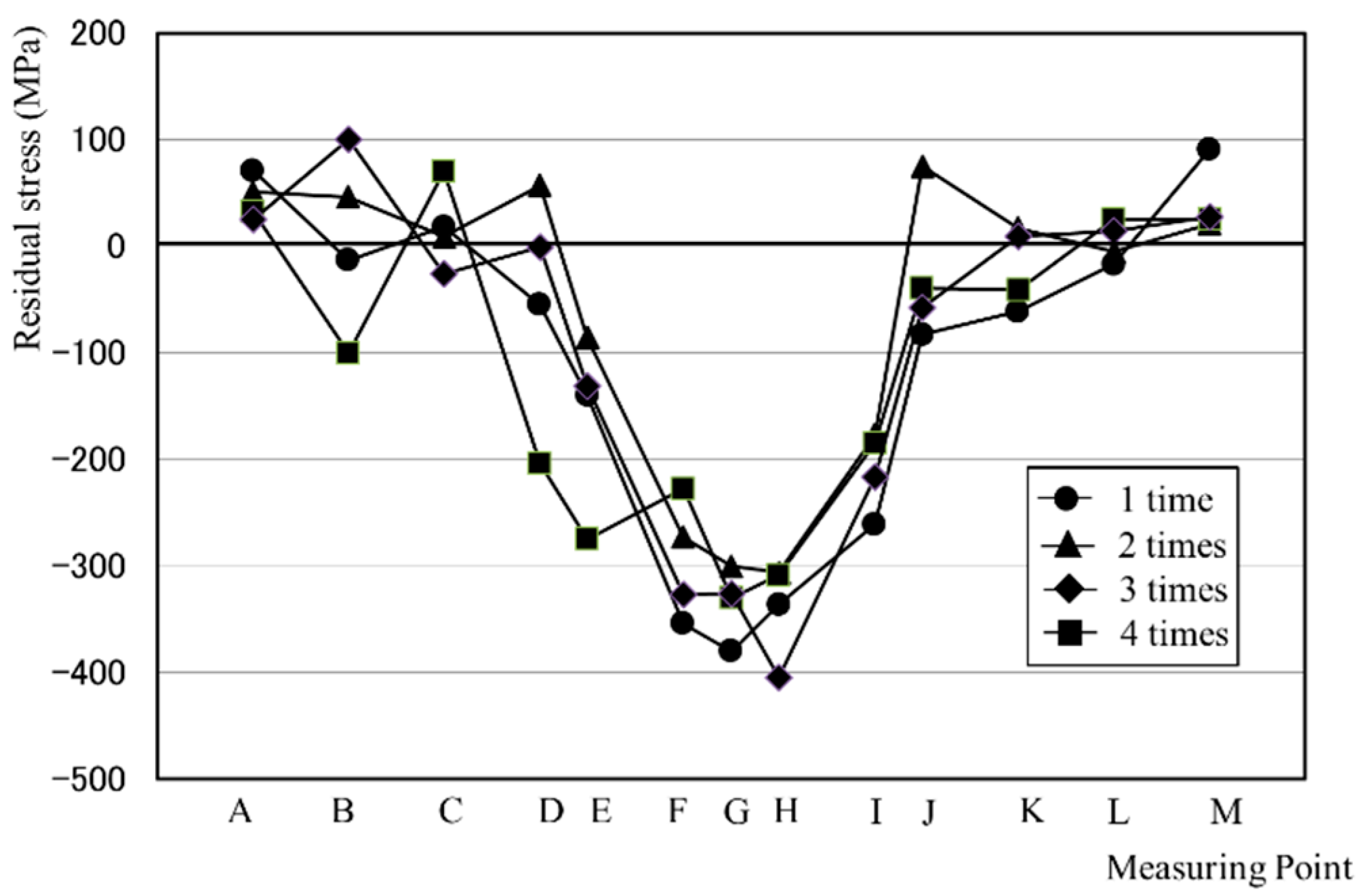
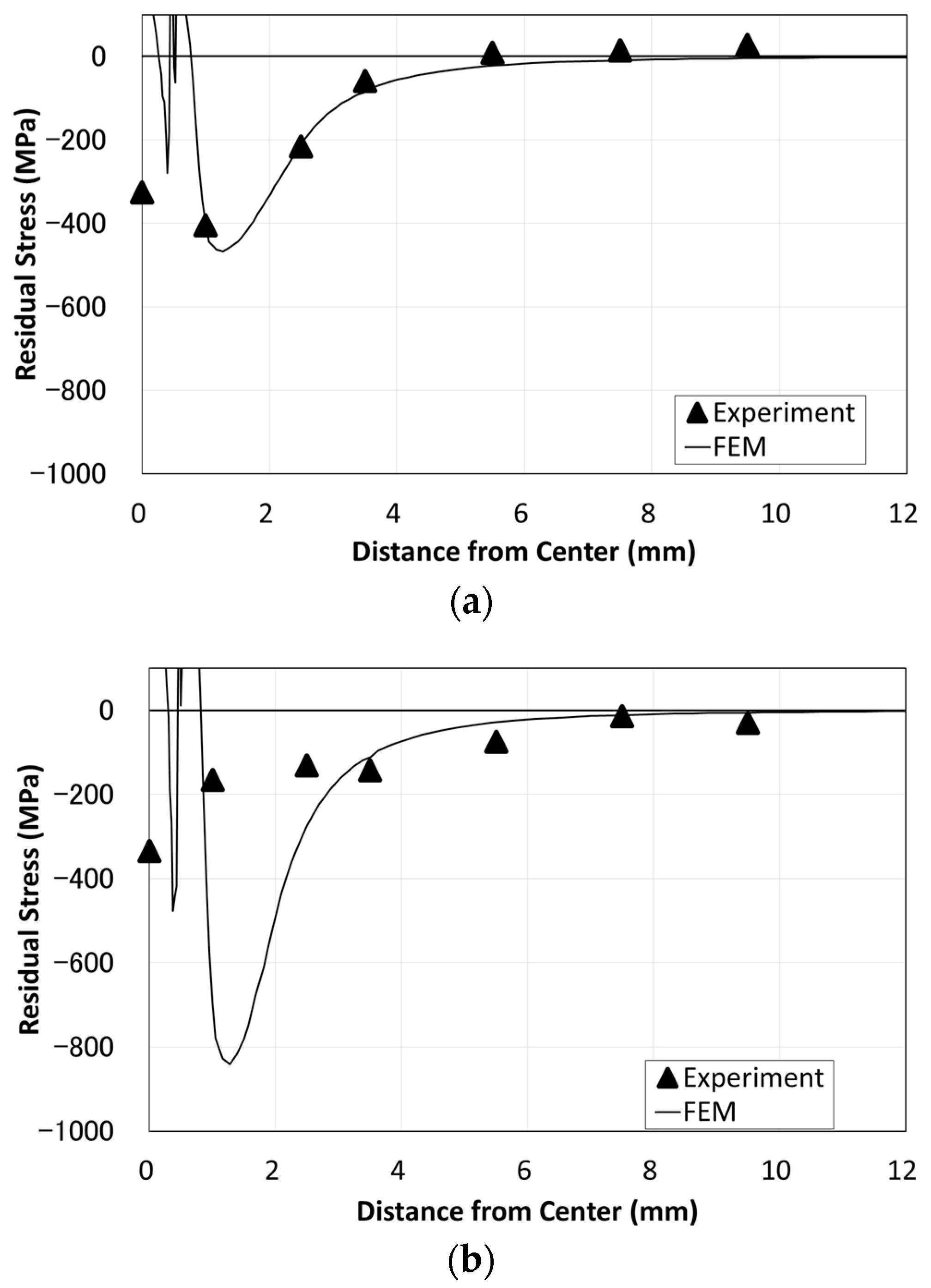
2.3. Surface Residual Stress Distribution
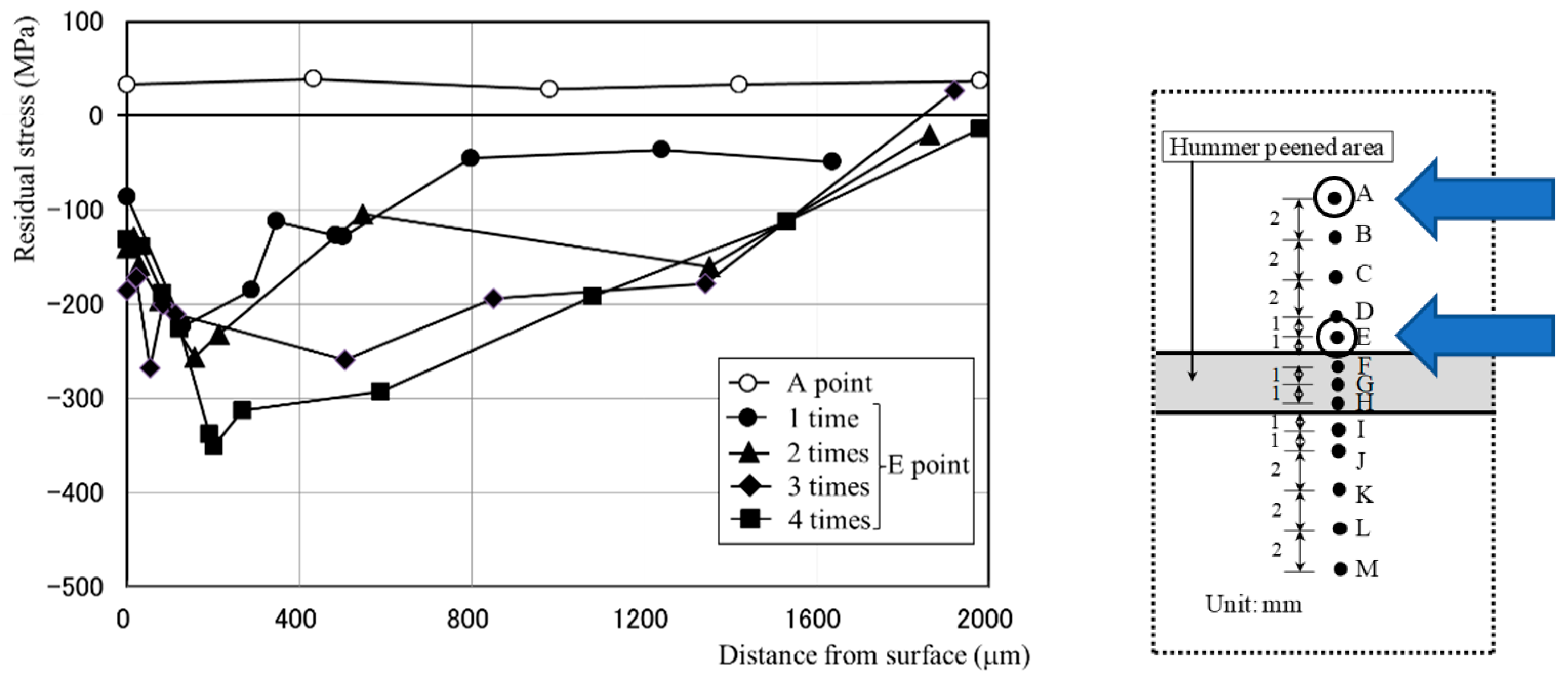
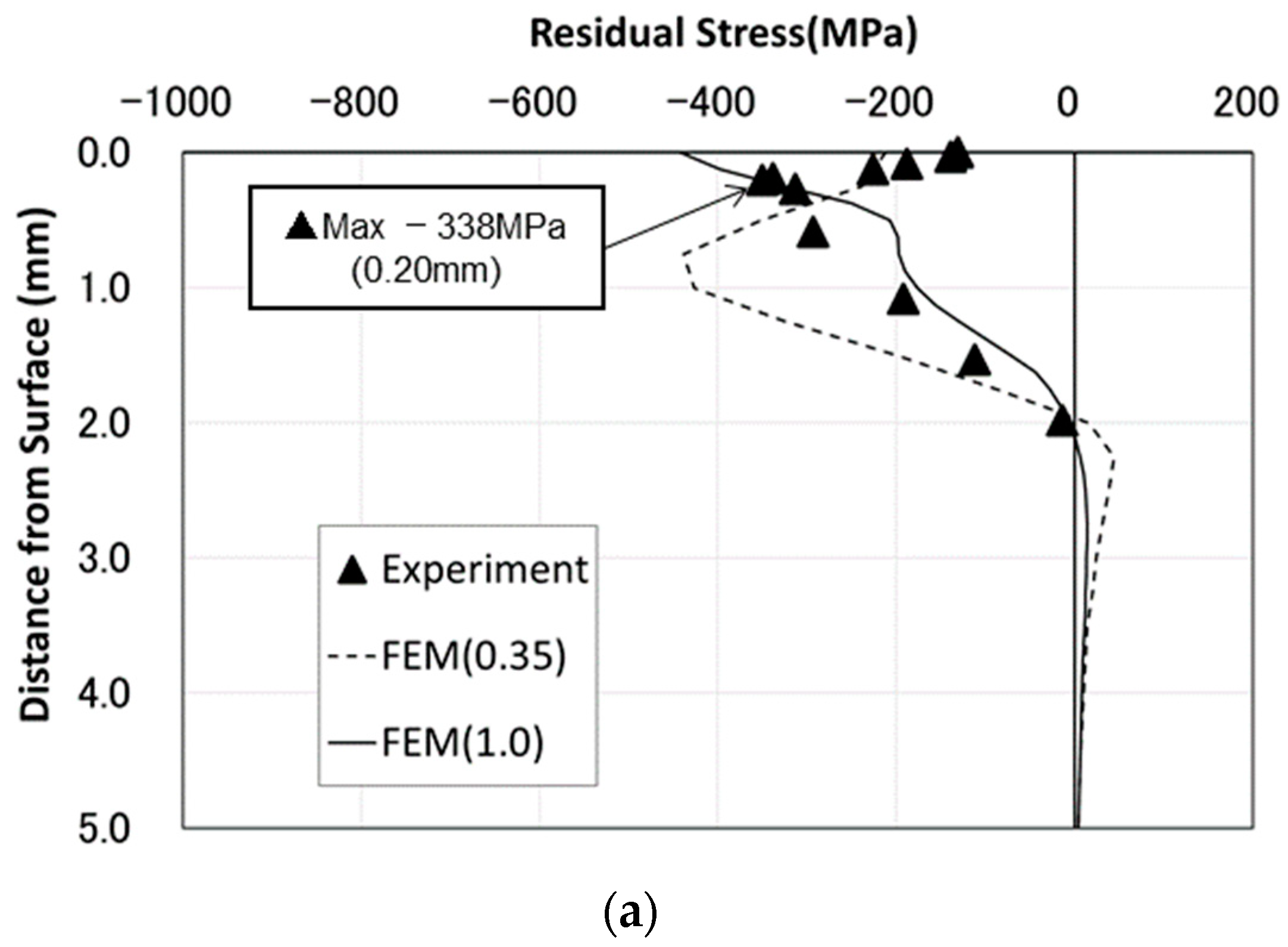
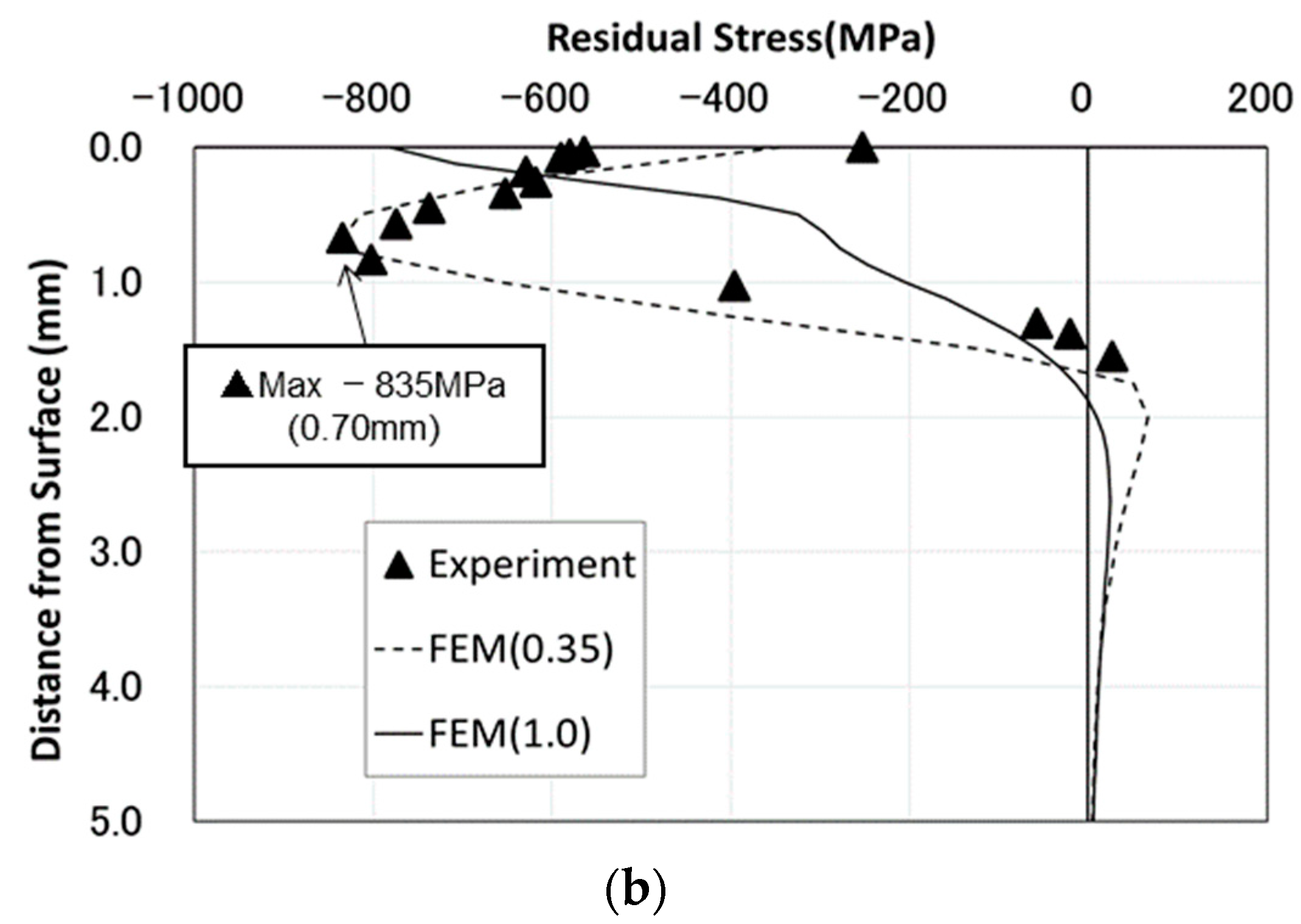
2.4. Distribution of Residual Stress in Depth
3. Elucidation of the Mechanism of Residual Stress Introduction by Analysis
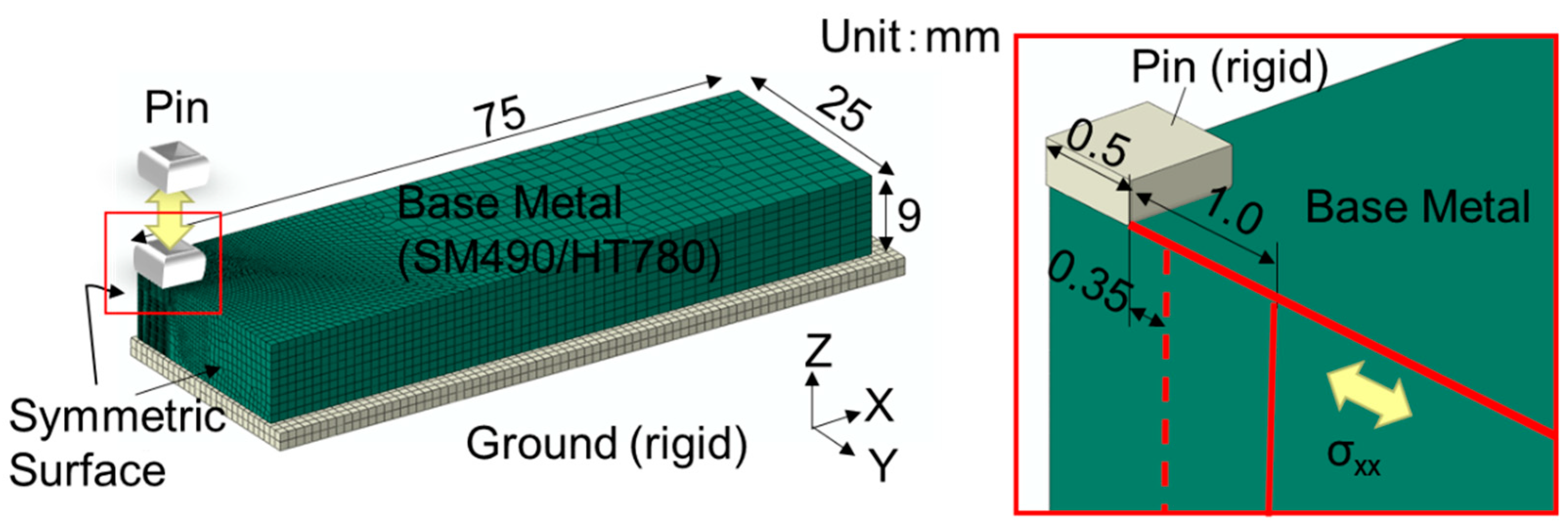
3.1. Analysis Conditions
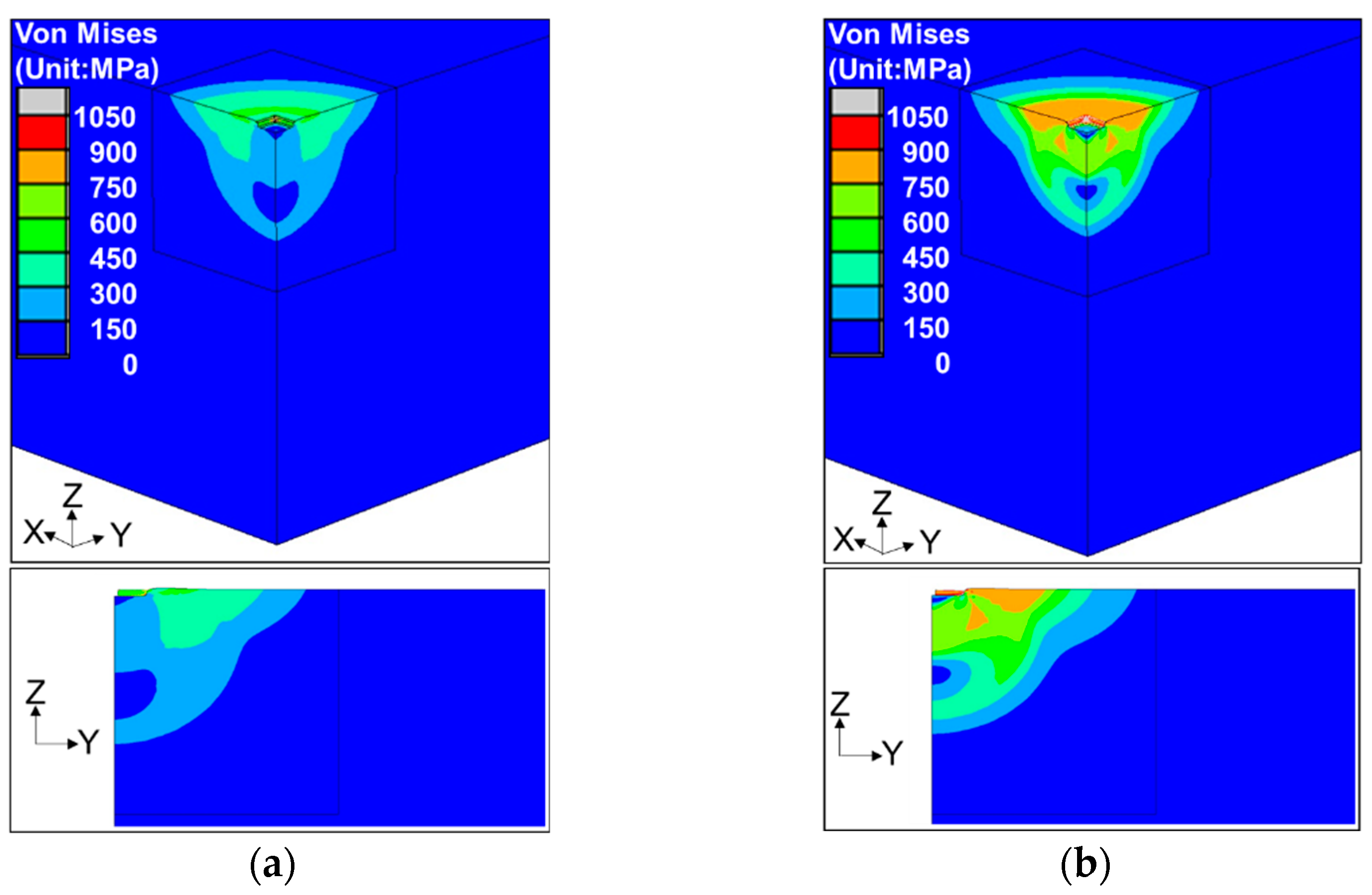
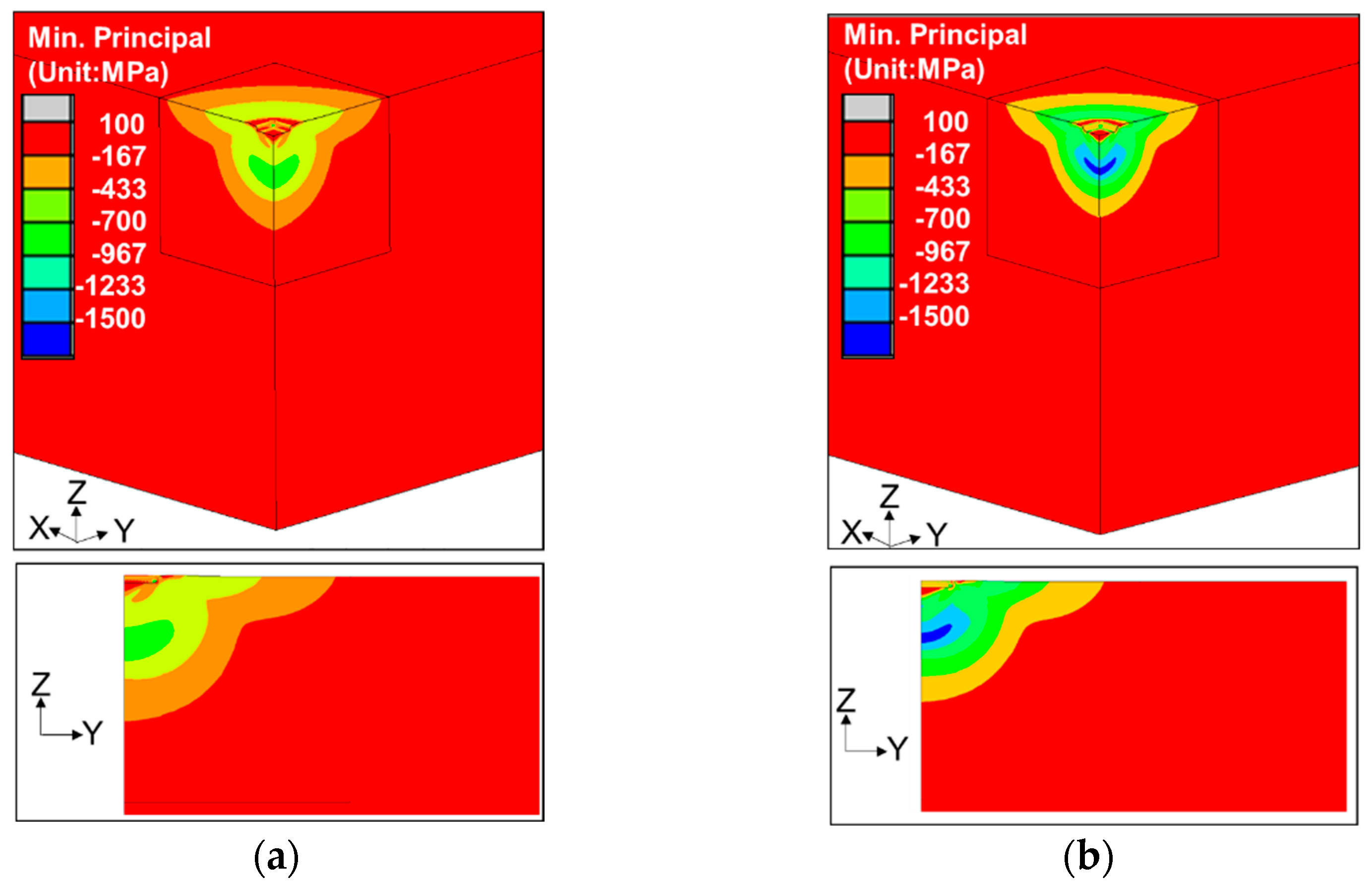
3.2. Analysis Results
4. Conclusions
- The surface depression of high-strength steel (HT780) caused by base metal hammer impact peening (BMHIP) was about half that of ordinary steel (SM490), but the maximum value of the residual stress distribution in the surface layer was about −400 MPa for both the high-strength steel and ordinary steel, and the distribution was similar. However, in the depth direction, the maximum value of −350 MPa was generated in the vicinity of 150 μm depth in the ordinary steel, and the maximum value of −835 MPa was generated in the vicinity of 800 μm depth in the high-tensile steel, and it was proven that the residual stress in proportion to the steel strength appeared inside.
- The number of times of BMHIP treatment greatly affected the distribution of residual stress generated by BMHIP in the depth direction, and as the number of times increased, the magnitude of the maximum compressive stress increased, and a large compressive residual stress was generated deep inside, but it reached the limit at about four times.
- It was shown that the residual stress condition after the BMHIP was carried out could be simulated by the elasto-plastic analysis in which the pin was pushed in.
- The peak value of the residual stress was proportional to the yield stress of the material. In addition, it was proven that in the vicinity of the impact by BMHIP, the value reached the maximum inside the plate thickness, and the maximum value tended to approach the surface as it separated from the impact part.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tateishi, K. Countermeasure Technologies for Fatigue in Steel Bridges. Steel Struct. Ser. 2013, 22, 87–128. (In Japanese) [Google Scholar]
- Hensel, J.; Eslami, H.; Nitschke-Pagel, T.; Thomas, D.; Dilger, K. Fatigue Strength Enhancement of Butt Welds by. Metals 2019, 9, 744. [Google Scholar] [CrossRef]
- Lago, J.; Trsko, L.; Jambor, M.; Novy, F.; Bokuvka, O.; Mician, M.; Pastorek, F. Fatigue Life Improvement of the High Strength Steel. Metals 2019, 9, 619. [Google Scholar] [CrossRef]
- Anami, K.; Miki, C.; Tani, H.; Yamamoto, H. Improving Fatigue Strength of Welded Joints by Hammer Peening and Tig-Dressing. J. Struct. Eng. Earthq. Eng. JSCE 2000, 17, 67–78. [Google Scholar] [CrossRef]
- Nakanishi, K.; Morikaga, Y.; Kawabata, A.; Tomo, H. Study on Improvement Method of Fatigue Strength of Weld Joints by Hammer Peening on Base Metal. J. Jpn Soc. Civil Eng. 2017, 73, 10–19. (In Japanese) [Google Scholar]
- Sakino, Y.; Kurihara, Y.; Tsutsumi, S. Application of hummer peening on base metal to high-strength steel. J. Jpn Weld. Soc. 2018, 36, 206–211. (In Japanese) [Google Scholar] [CrossRef]
- Sakino, Y.; Kurihara, Y.; Manabe, M.; Nakada, R. Application of hummer peening to box-welded joint of high-strength steel. Welded Structure Symposium. Jpn Weld. Soc. 2017, 36, 415–418. (In Japanese) [Google Scholar]
- Matsumoto, R.; Ishikawa, T.; Tsutsumi, S.; Kawano, H.; Yamada, K. Analytical vertification of residual stress distribution in steel plate after hammer peening. J. Struct. Eng. 2016, 62, 685–692. (In Japanese) [Google Scholar]
- Japanese Standards Association. JIS G3106 Rolled Steels for Weided Structure, G3128 High Yield Strength Steel Plates for Welded Structure; JIS: Tokyo, Japan, 2009. [Google Scholar]
- Akiniwa, Y.; Kojima, Y.; Kimura, H.; Maruko, K. Prediction of Residual Stress Distribution in Severe Surface Deformed Steel by Constant Penetration Depth Method. J. Soc. Mater. Sci. Jpn 2008, 57, 660–666. (In Japanese) [Google Scholar] [CrossRef][Green Version]



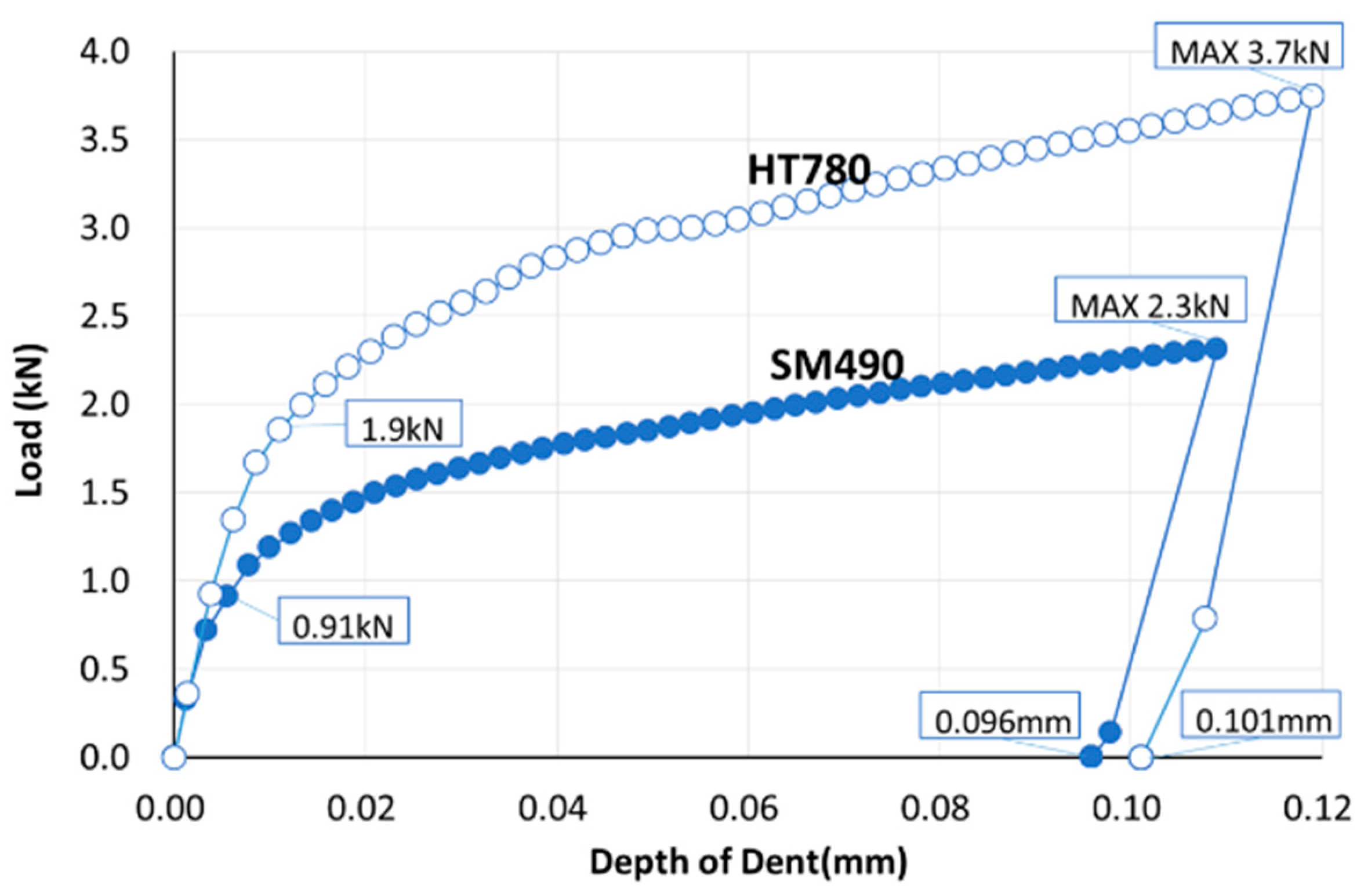
| Base Material | 1 Time | 2 Times | 3 Times | 4 Times |
|---|---|---|---|---|
| SM490 | 0.070 | 0.055 | 0.141 | 0.201 |
| HT780 | 0.017 | 0.042 | 0.063 | 0.093 |
| Case | Base Metal | Young’s Modulus (MPa) | Yield Stress (MPa) | Tension Stress (MPa) |
|---|---|---|---|---|
| Case 1 | SM490 | 205,000 | 371 | 533 |
| Case 2 | HT780 | 205,000 | 824 | 840 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurihara, Y.; Sakino, Y.; Kato, T. Investigation on Residual Compressive Distribution of High-Strength Steel for Bridges by Base Metal Hammer Impact Peening. Appl. Mech. 2021, 2, 932-941. https://doi.org/10.3390/applmech2040054
Kurihara Y, Sakino Y, Kato T. Investigation on Residual Compressive Distribution of High-Strength Steel for Bridges by Base Metal Hammer Impact Peening. Applied Mechanics. 2021; 2(4):932-941. https://doi.org/10.3390/applmech2040054
Chicago/Turabian StyleKurihara, Yasuyuki, Yoshihiro Sakino, and Tomoharu Kato. 2021. "Investigation on Residual Compressive Distribution of High-Strength Steel for Bridges by Base Metal Hammer Impact Peening" Applied Mechanics 2, no. 4: 932-941. https://doi.org/10.3390/applmech2040054
APA StyleKurihara, Y., Sakino, Y., & Kato, T. (2021). Investigation on Residual Compressive Distribution of High-Strength Steel for Bridges by Base Metal Hammer Impact Peening. Applied Mechanics, 2(4), 932-941. https://doi.org/10.3390/applmech2040054





