Nonuniform Deformation of Cell Structures Owing to Plastic Stress Wave Propagation
Abstract
1. Introduction
2. Specimen
2.1. Nickel Foam
2.2. Silicone Rubber-Filled Nickel Foam
2.3. Pure Nickel
2.4. Silicone Rubber
3. Compression Test
3.1. Quasi-Static Test
3.2. Dynamic/Impact Test
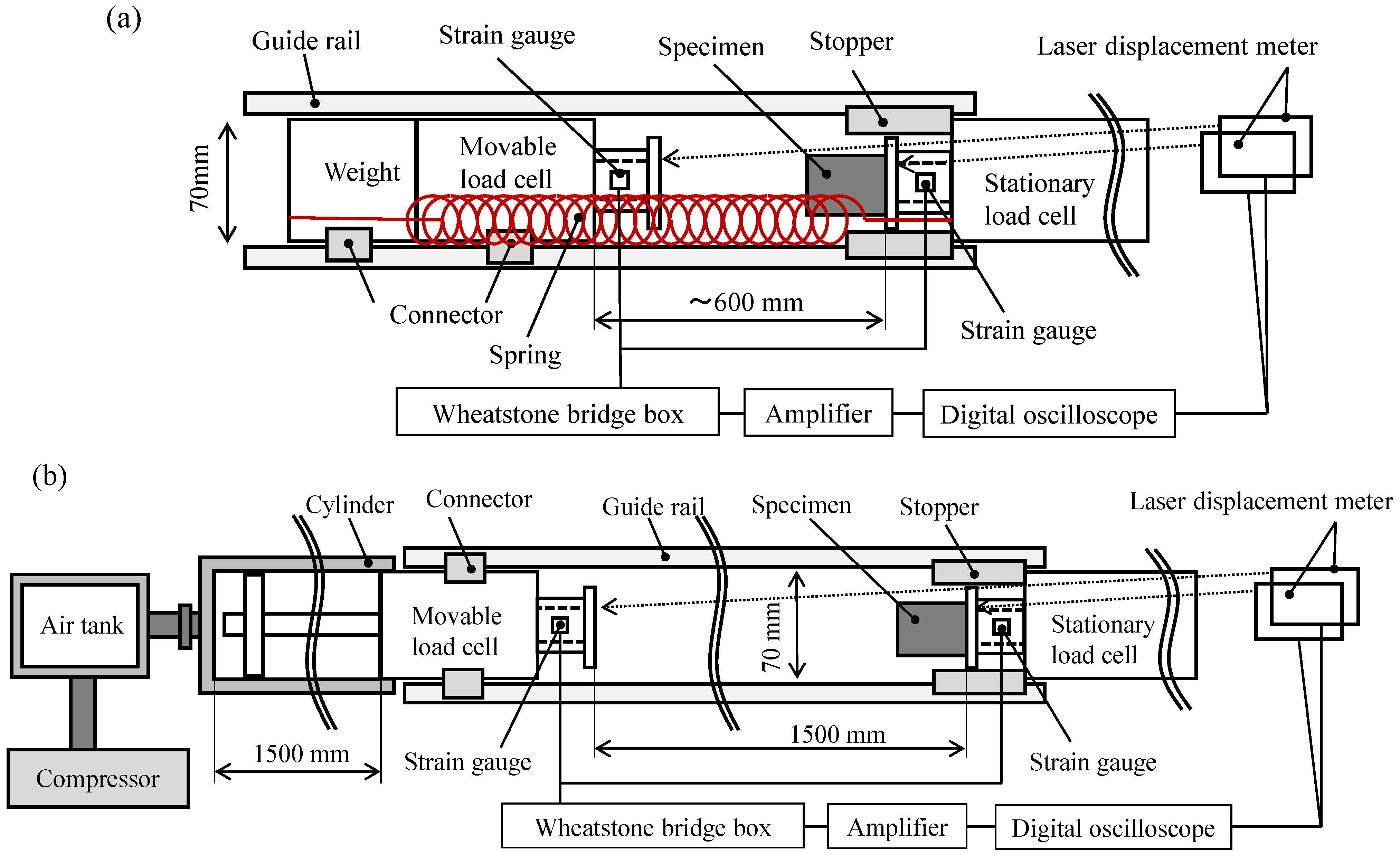
3.2.1. SHB Method
3.2.2. Dynamic and Impact Load-Measuring Apparatus with Opposite Load Cells
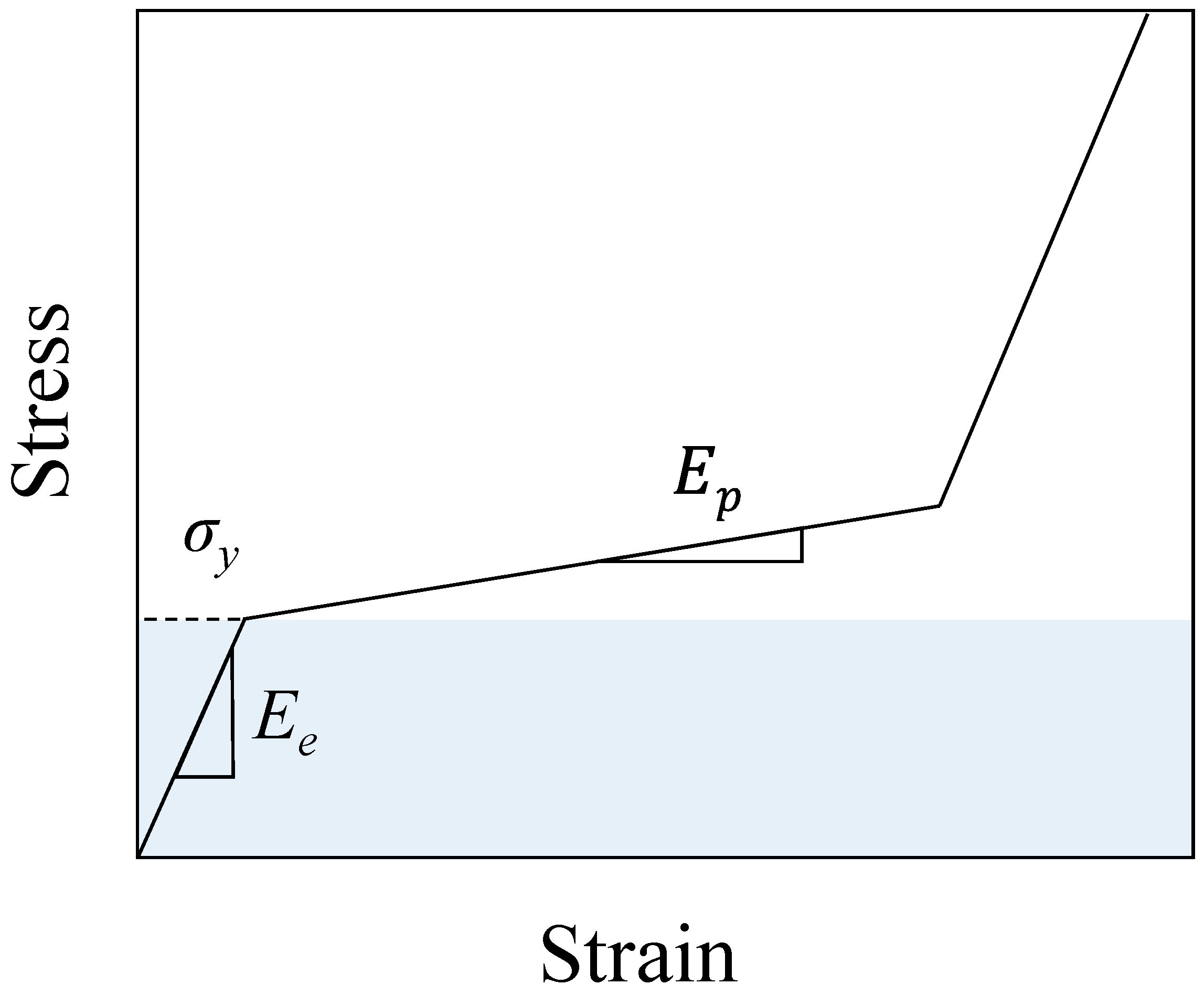
4. Results of Compression Tests of Constituent Materials
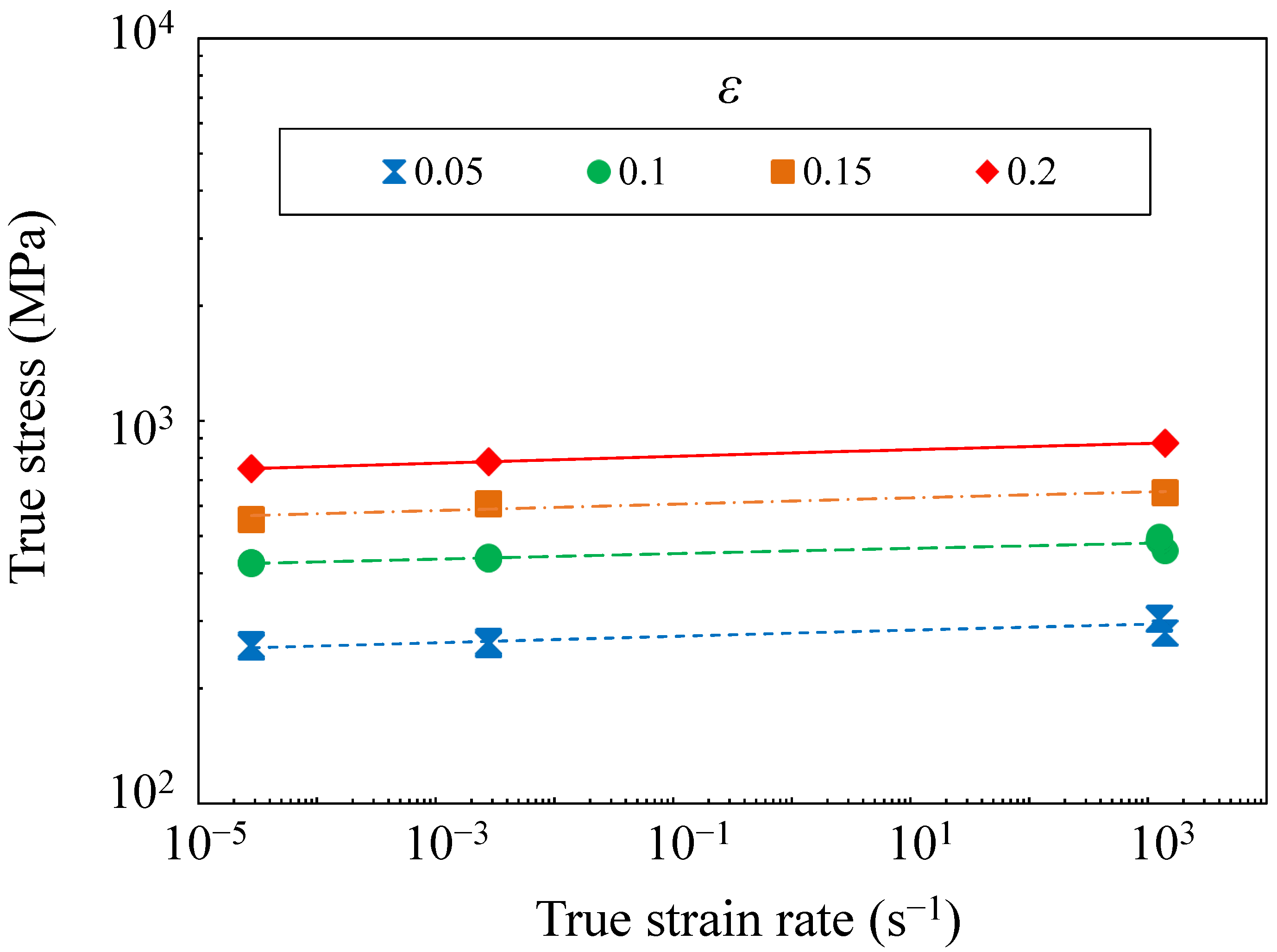
4.1. Pure Ni
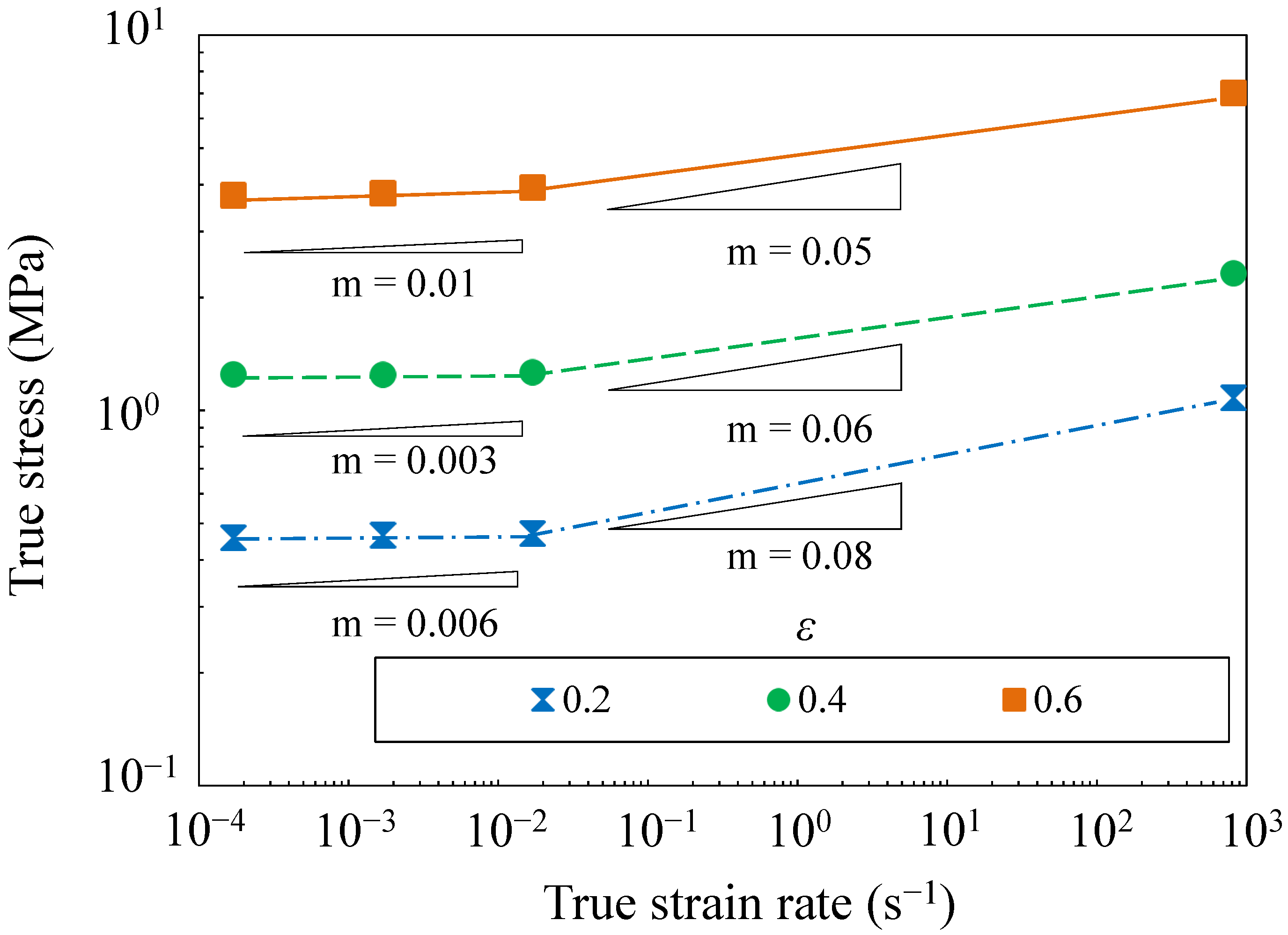
4.2. Silicone Rubber
5. Results of Compression Tests of Cell Structure
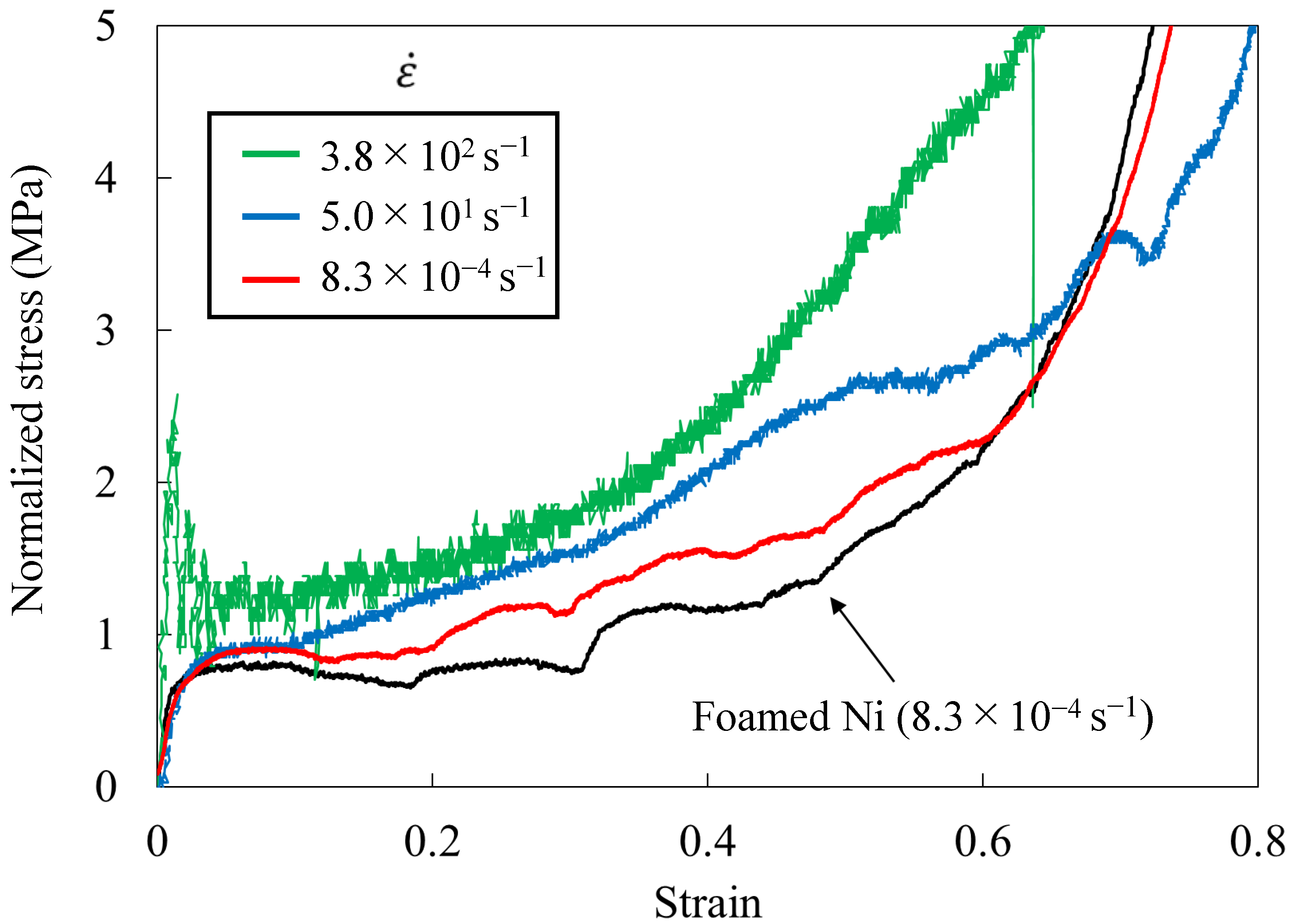
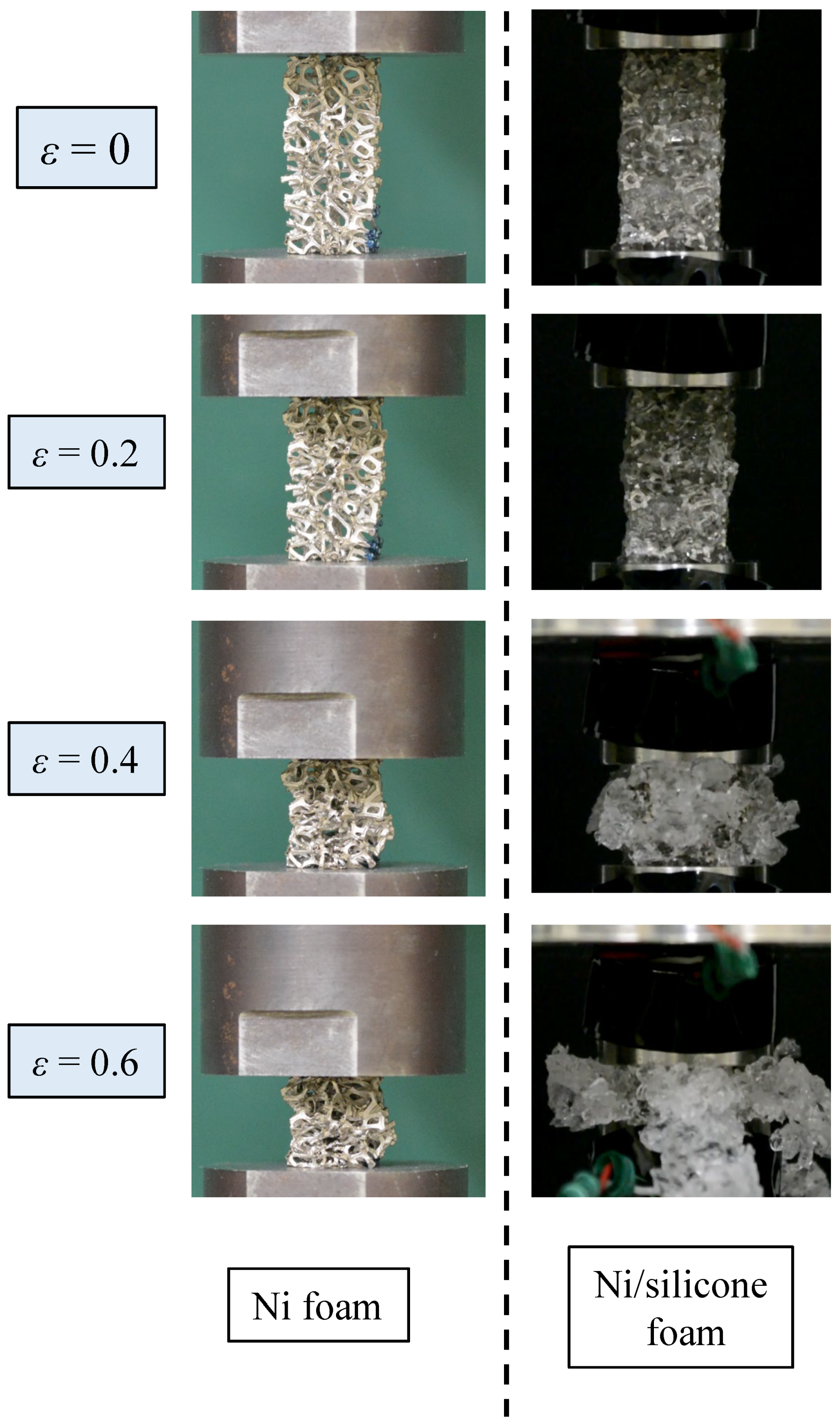
5.1. Compression Velocity of 20.0 m/s or Less
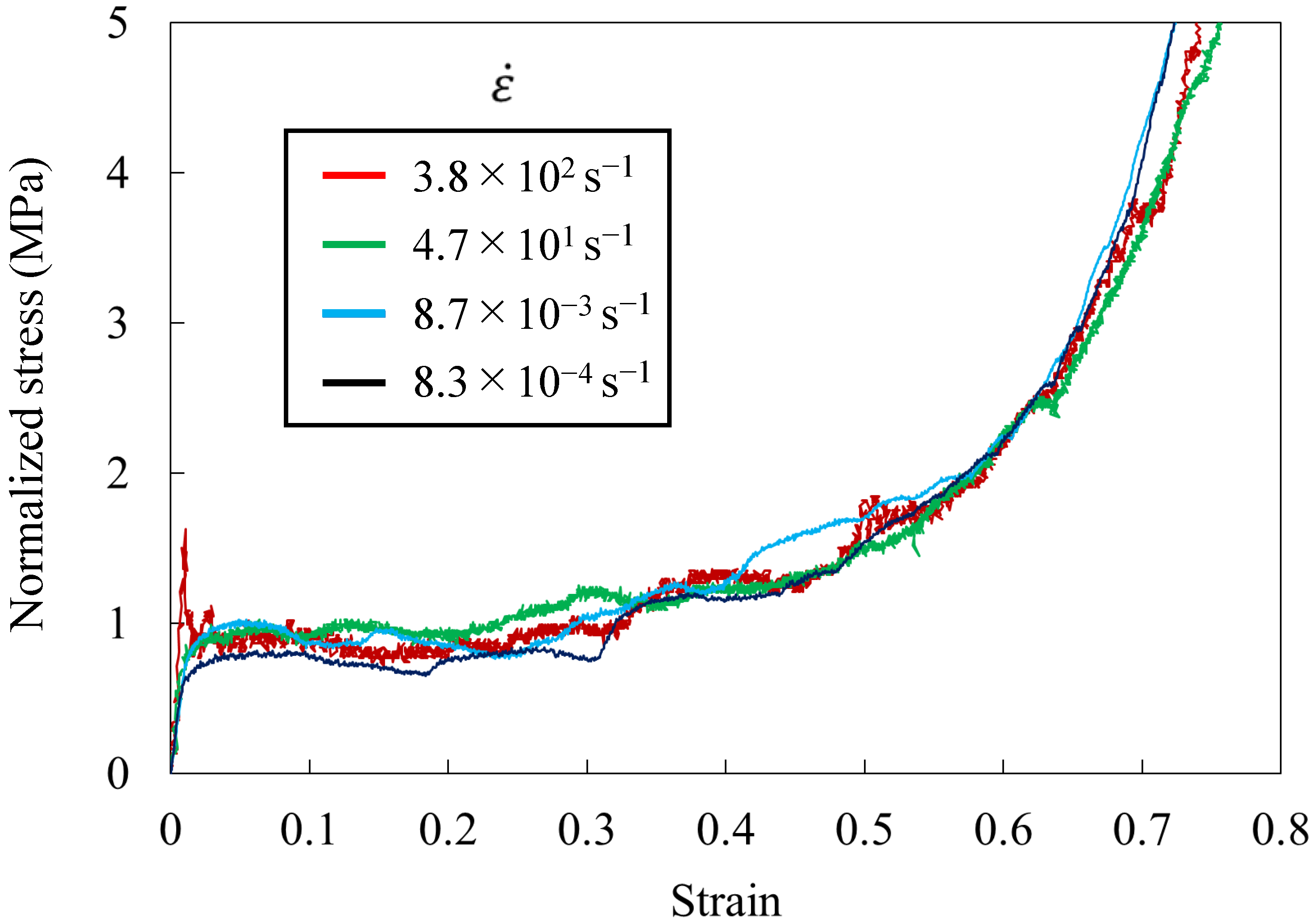
5.1.1. Ni Foam
5.1.2. Ni/Silicone Foam
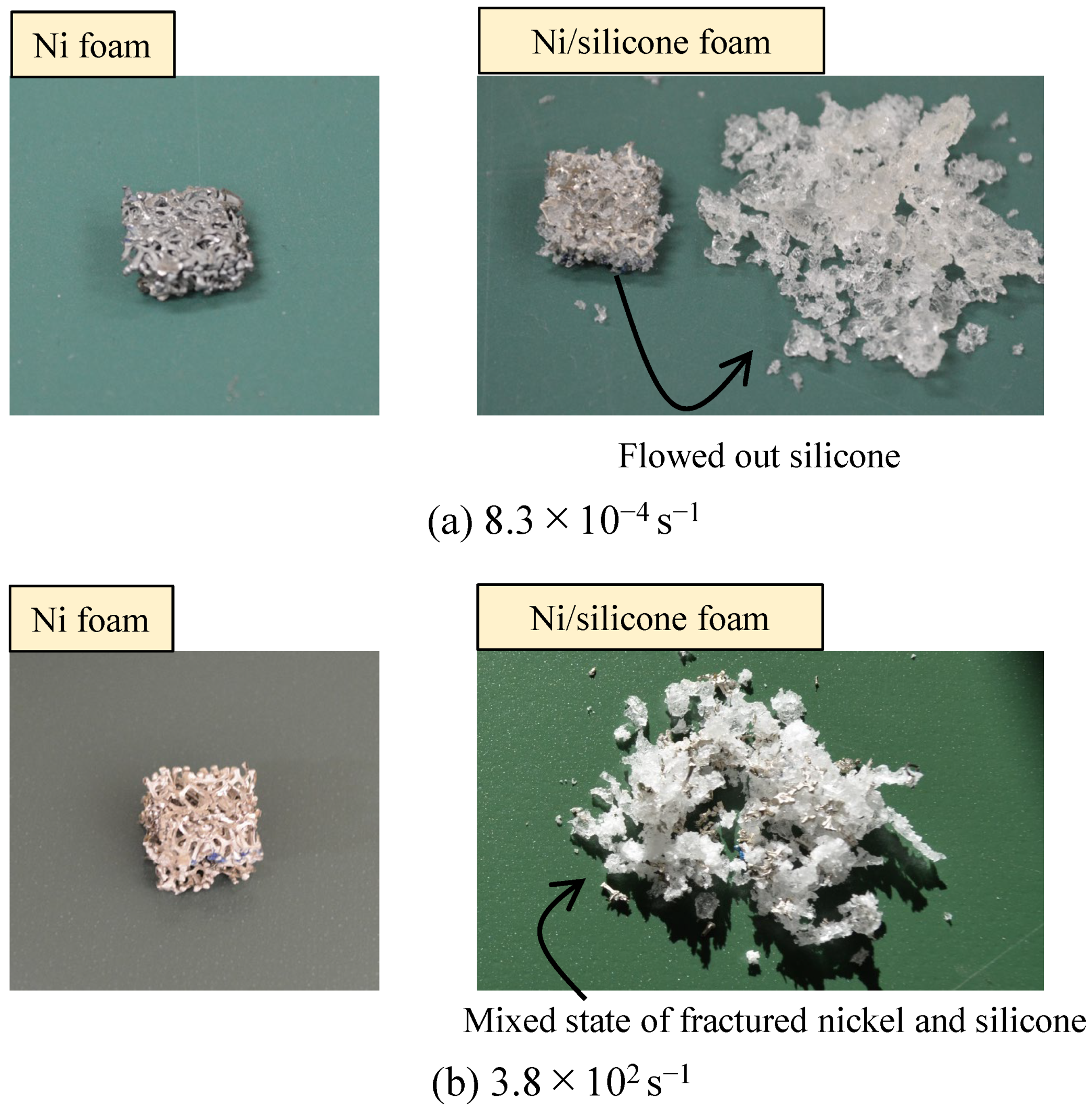
5.2. Compression Velocity of 20.0 m/s or More
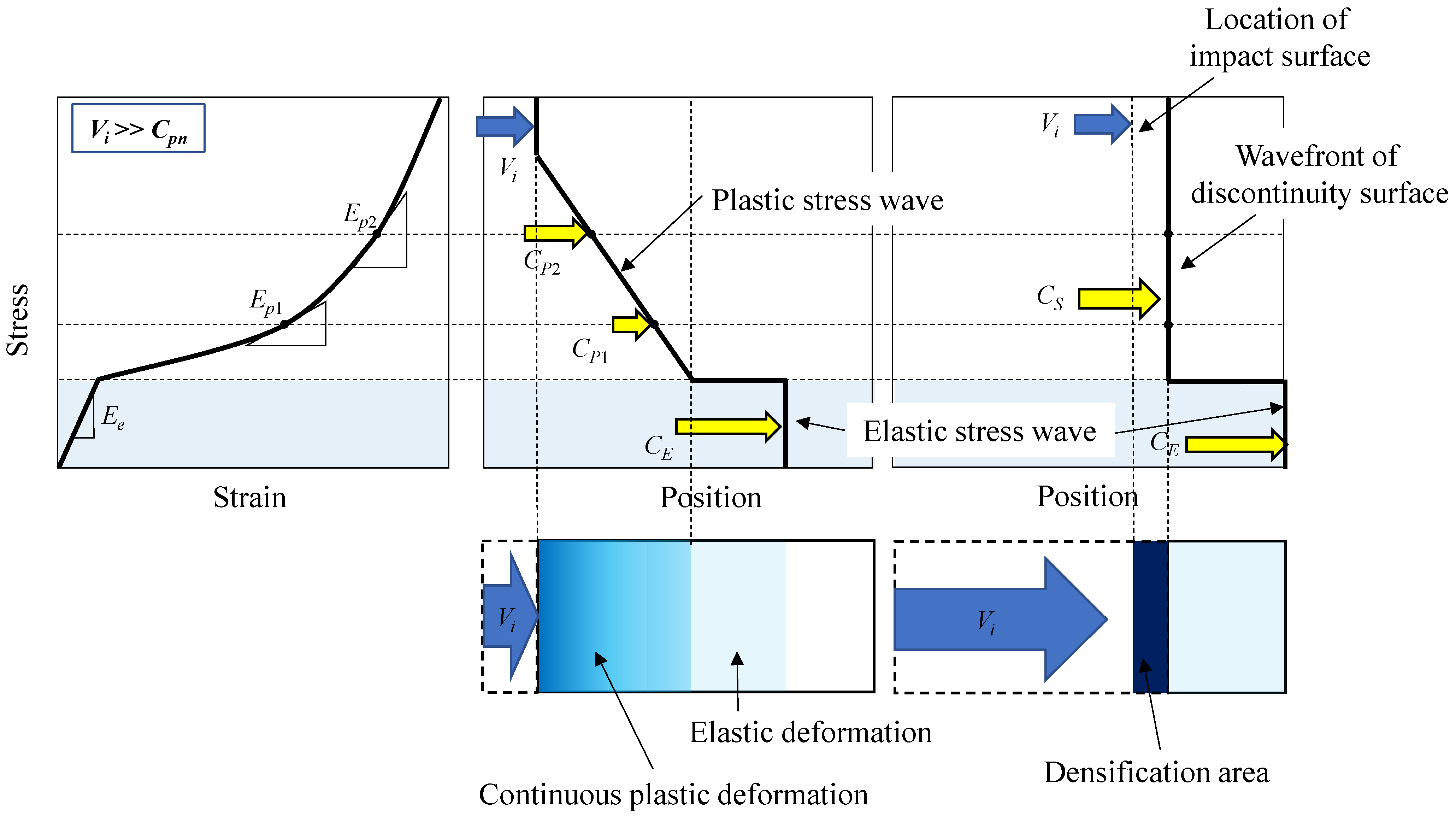
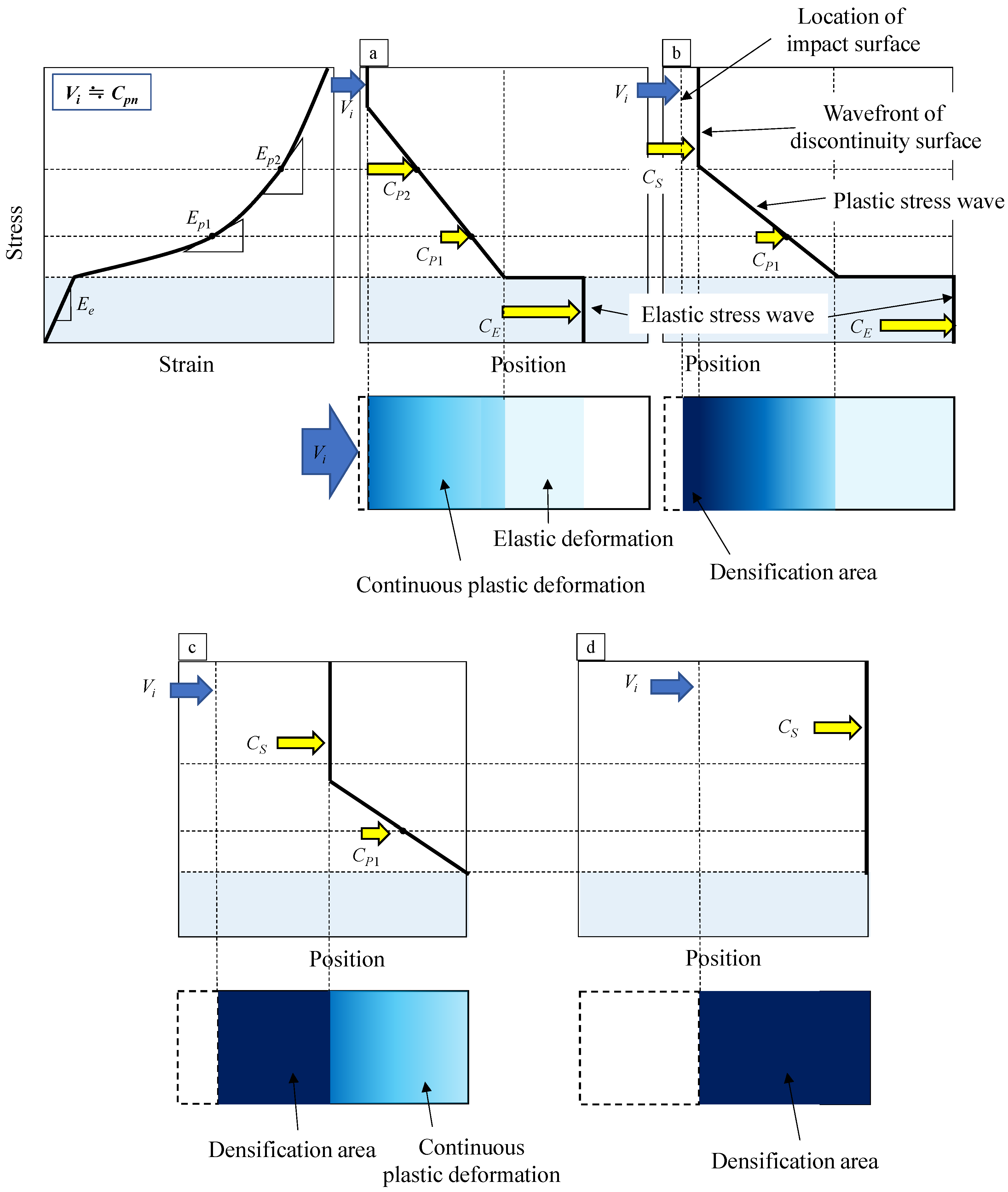
6. Discussion of Mechanism of Nonuniform Deformation
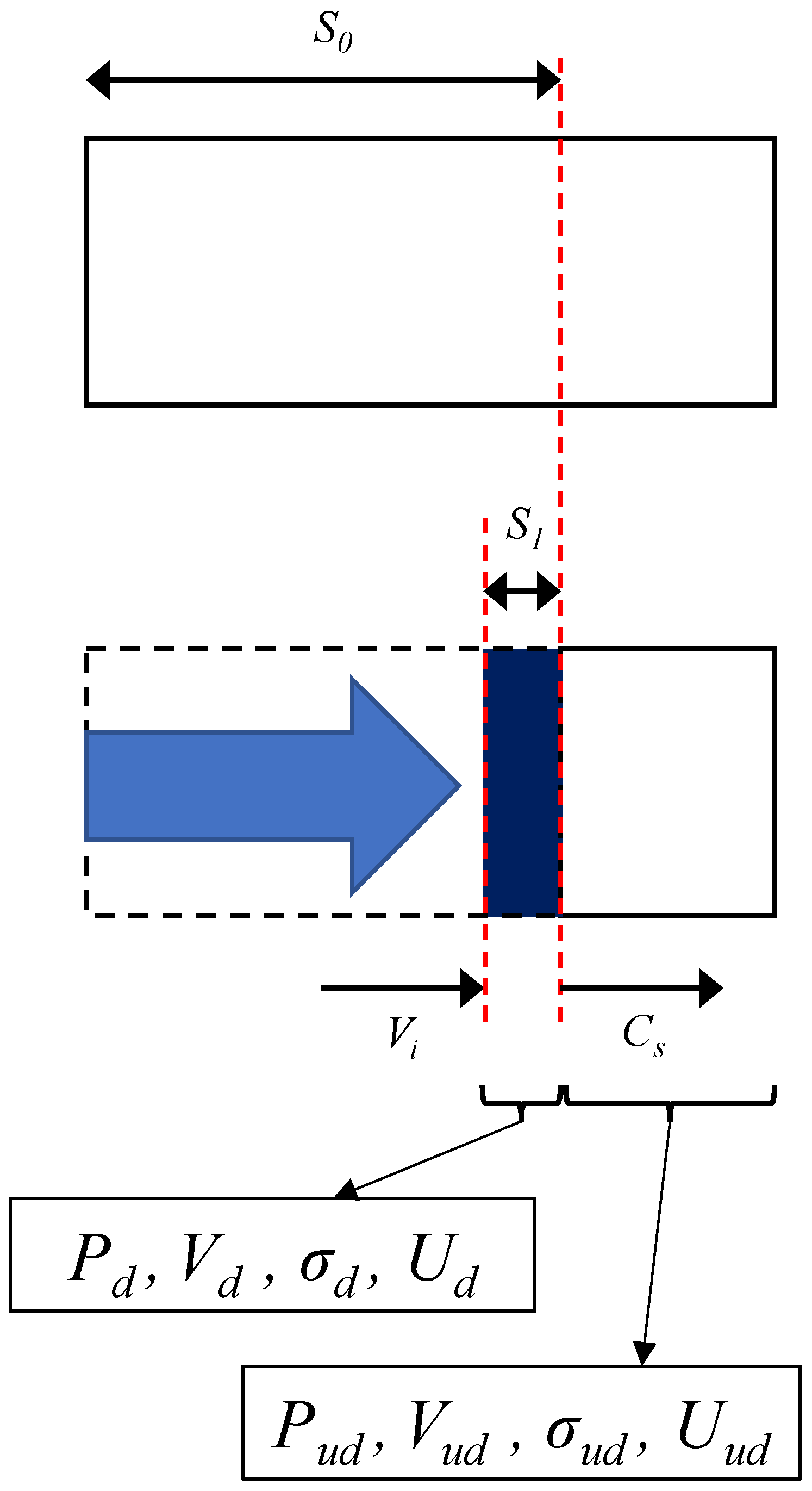
7. Conclusions
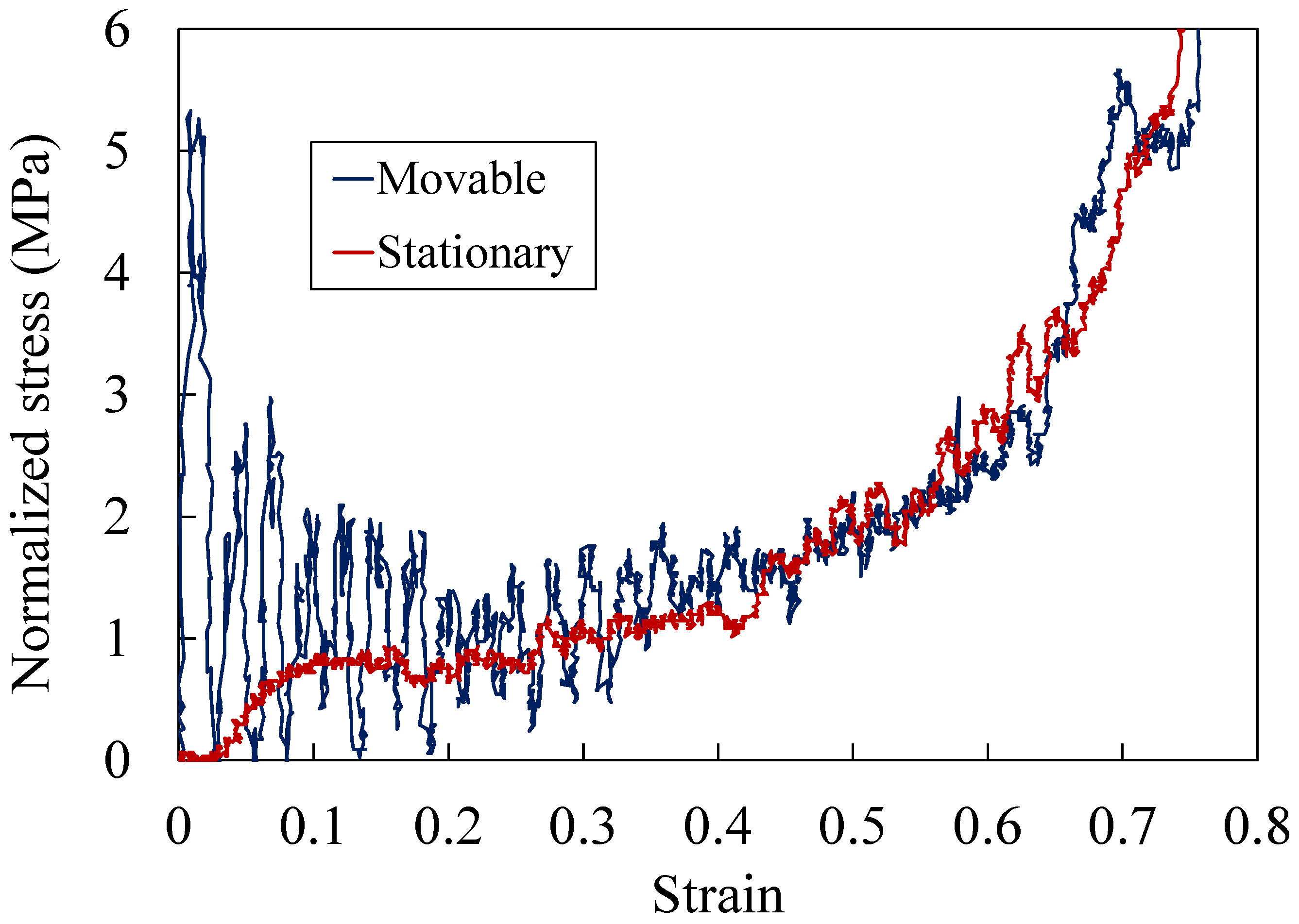
- At a compression velocity of 20.0 m/s or less (strain rate from 8.3 × 10−4 to 3.8 × 102 s−1), no nonuniform deformation was observed in either the Ni foam or the Ni/silicone foam, and the loads on the impact and fixed ends achieved force equilibrium.
- The flow stress of the Ni foam did not show any change with an increasing strain rate; by contrast, the flow stress of the Ni/silicone foam showed remarkable strain rate dependence. Perhaps this result was caused by the outflow resistance when silicone flows out from the inside and the pressure rises owing to the residual silicone.
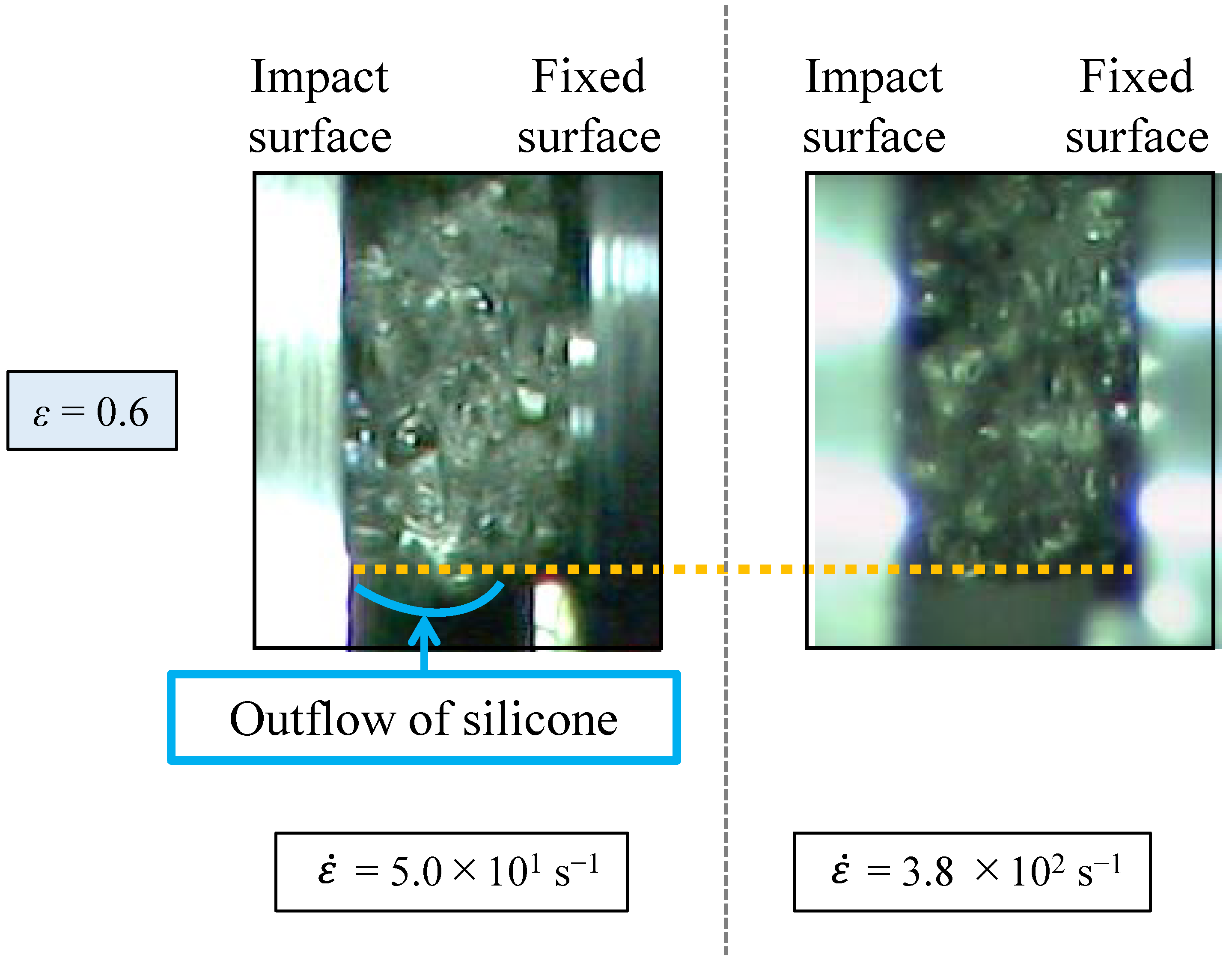
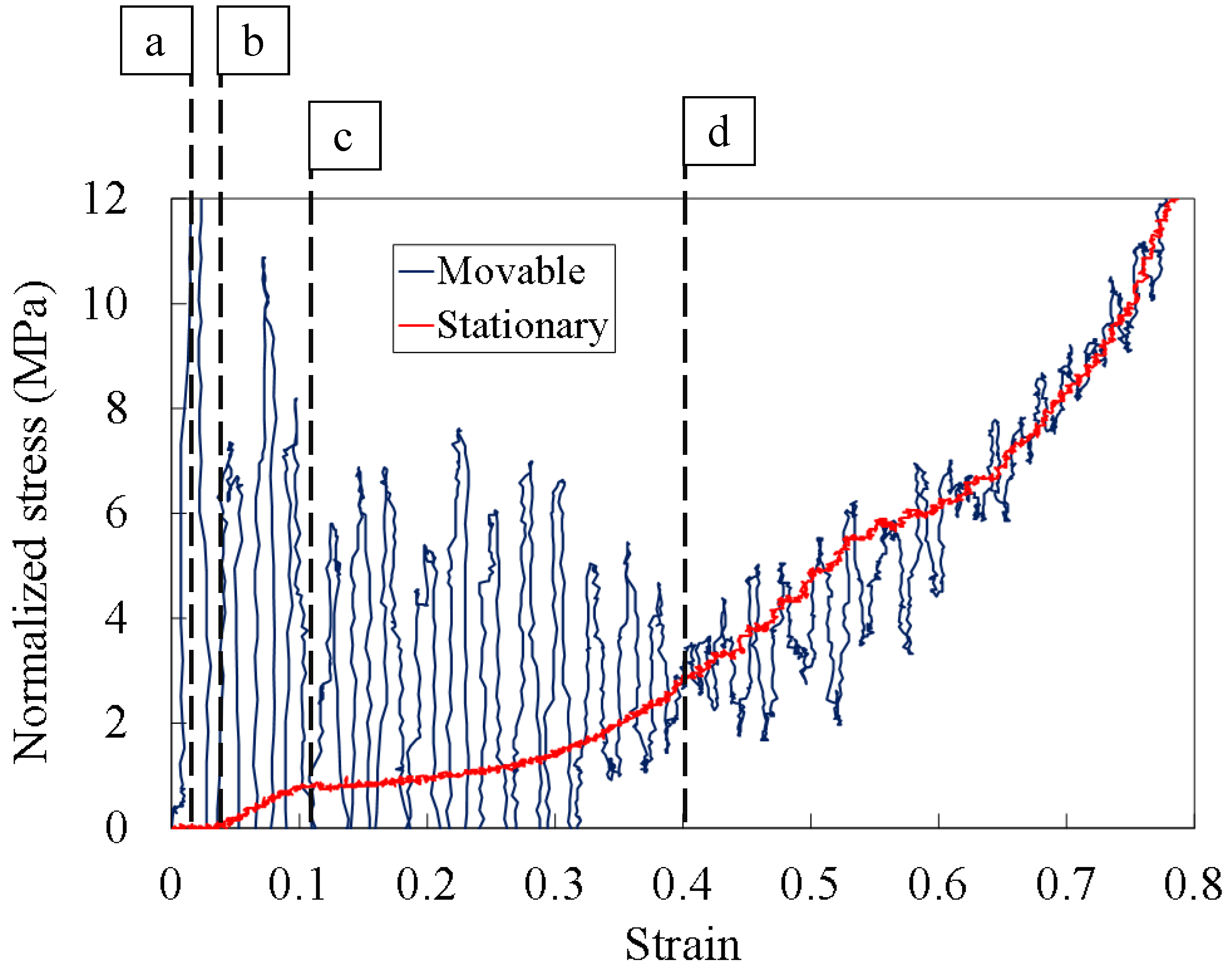
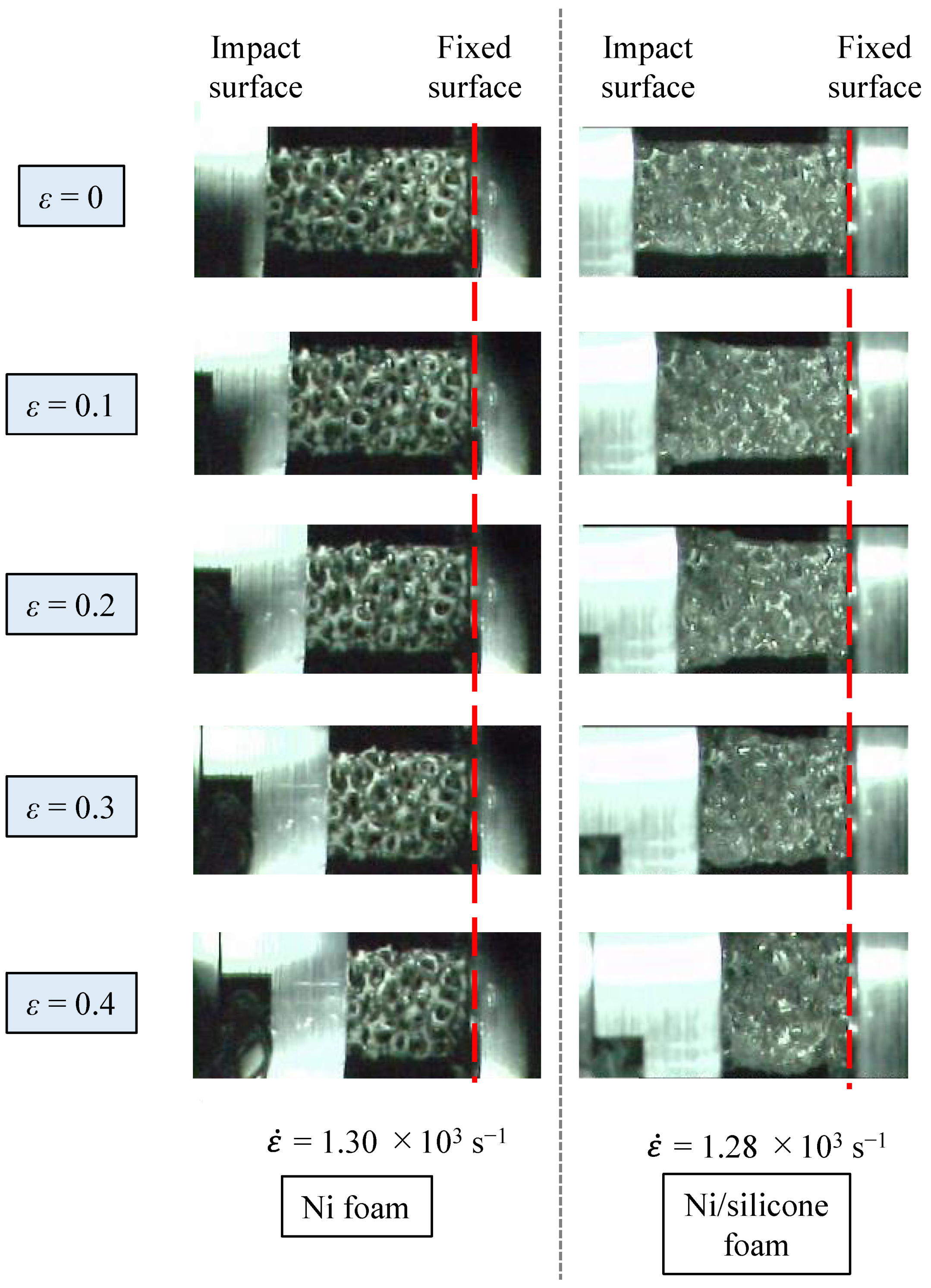
- At a compression velocity of approximately 26.0 m/s (strain rate: 1.3 × 103 s−1), the loads at both ends of the Ni/silicone foam differed, and we observed nonuniform deformation from the impact end. The plastic stress wave propagation speed in the Ni/silicone foam, derived from the slope of the plateau region, was 15.7 m/s, suggesting that nonuniform deformation occurs when the compression velocity becomes higher than the plastic stress wave propagation speed.
- Nonuniform deformation of the cell structure was probably caused by the accumulation of plastic stress waves. However, near the transition velocity, plastic deformation can occur even in front of the discontinuity surface owing to the accumulation of plastic stress waves. Therefore, deformation behavior cannot be considered a distinct discontinuity surface.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Avalle, M.; Belingardi, G.; Montanini, R. Characterization of Polymeric Structural Foams under Compressive Impact Loading by Means of Energy-Absorption Diagram. Int. J. Impact Eng. 2001, 25, 455–472. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 1988; ISBN 9781139878326. [Google Scholar]
- Saha, M.C.; Mahfuz, H.; Chakravarty, U.K.; Uddin, M.; Kabir, M.E.; Jeelani, S. Effect of Density, Microstructure, and Strain Rate on Compression Behavior of Polymeric Foams. Mater. Sci. Eng. A 2005, 406, 328–336. [Google Scholar] [CrossRef]
- Bouix, R.; Viot, P.; Lataillade, J.L. Polypropylene Foam Behaviour under Dynamic Loadings: Strain Rate, Density and Microstructure Effects. Int. J. Impact Eng. 2009, 36, 329–342. [Google Scholar] [CrossRef]
- Liao, S.; Zheng, Z.; Yu, J. On the Local Nature of the Strain Field Calculation Method for Measuring Heterogeneous Deformation of Cellular Materials. Int. J. Solids Struct. 2014, 51, 478–490. [Google Scholar] [CrossRef]
- Gasparetto, V.E.L.; ElSayed, M.S.A. Multiscale Modelling and Mechanical Anisotropy of Periodic Cellular Solids with Rigid-Jointed Truss-Like Microscopic Architecture. Appl. Mech. 2021, 2, 331–355. [Google Scholar] [CrossRef]
- Adachi, H.; Hasegawa, T. Effects of Cell Destruction on Compression Moduli of Closed-Celled Polyethylene Foams. Nihon Reoroji Gakkaishi 2003, 31, 105–108. [Google Scholar] [CrossRef][Green Version]
- Chakravarty, U.; Mahfuz, H.; Saha, M.; Jeelani, S. Strain Rate Effects on Sandwich Core Materials: An Experimental and Analytical Investigation. Acta Mater. 2003, 51, 1469–1479. [Google Scholar] [CrossRef]
- Ouellet, S.; Cronin, D.; Worswick, M. Compressive Response of Polymeric Foams under Quasi-Static, Medium and High Strain Rate Conditions. Polym. Test. 2006, 25, 731–743. [Google Scholar] [CrossRef]
- Song, B.; Chen, W.; Lu, W.Y. Compressive Mechanical Response of a Low-Density Epoxy Foam at Various Strain Rates. J. Mater. Sci. 2007, 42, 7502–7507. [Google Scholar] [CrossRef]
- Fowles, R.; Williams, R.F. Plane Stress Wave Propagation in Solids. J. Appl. Phys. 1970, 41, 360–363. [Google Scholar] [CrossRef]
- Meyers, M.A. Dynamic Behavior of Materials; Wiley: Hoboken, NJ, USA, 1994; ISBN 9780471582625. [Google Scholar]
- Elnasri, I.; Pattofatto, S.; Zhao, H.; Tsitsiris, H.; Hild, F.; Girard, Y. Shock Enhancement of Cellular Structures under Impact Loading: Part I Experiments. J. Mech. Phys. Solids 2007, 55, 2652–2671. [Google Scholar] [CrossRef]
- Pattofatto, S.; Elnasri, I.; Zhao, H.; Tsitsiris, H.; Hild, F.; Girard, Y. Shock Enhancement of Cellular Structures under Impact Loading: Part II Analysis. J. Mech. Phys. Solids 2007, 55, 2672–2686. [Google Scholar] [CrossRef]
- Wang, P.; Xu, S.; Li, Z.; Yang, J.; Zhang, C.; Zheng, H.; Hu, S. Experimental Investigation on the Strain-Rate Effect and Inertia Effect of Closed-Cell Aluminum Foam Subjected to Dynamic Loading. Mater. Sci. Eng. A 2015, 620, 253–261. [Google Scholar] [CrossRef]
- Gaitanaros, S.; Kyriakides, S. Dynamic Crushing of Aluminum Foams: Part II—Analysis. Int. J. Solids Struct. 2014, 51, 1646–1661. [Google Scholar] [CrossRef]
- Hopkinson, B.X. A Method of Measuring the Pressure Produced in the Detonation of High, Explosives or by the Impact of Bullets. Philos. Trans. R. Soc. Lond. Ser. Contain. Pap. Math. Phys. Character 1914, 213, 437–456. [Google Scholar] [CrossRef]
- Kolsky, H. An Investigation of the Mechanical Properties of Materials at Very High Rates of Loading. Proc. Phys. Soc. Sect. B 1949, 62, 676–700. [Google Scholar] [CrossRef]
- Tateyama, K.; Kakuno, C.; Ogasawara, N.; Yamada, H. Development of Universal Rate Range Load-Cell. J. Jpn. Soc. Exp. Mech. 2017, 17, 131–138. [Google Scholar] [CrossRef]
- Yamada, H.; Kakuno, C.; Tateyama, K.; Ogasawara, N. Development of Dynamic and Impact Load-Measuring Apparatus with Opposite Load-Cells. J. Jpn. Soc. Exp. Mech. 2017, 17, 117–123. [Google Scholar] [CrossRef]
- Tateyama, K.; Yamada, H.; Ogasawara, N. Effect of Strain Rate on Compressive Properties of Foamed Polyethylene Film. Polym. Test. 2016, 52, 54–62. [Google Scholar] [CrossRef]
- Barnes, A.T.; Ravi-Chandar, K.; Kyriakides, S.; Gaitanaros, S. Dynamic Crushing of Aluminum Foams: Part I—Experiments. Int. J. Solids Struct. 2014, 51, 1631–1645. [Google Scholar] [CrossRef]
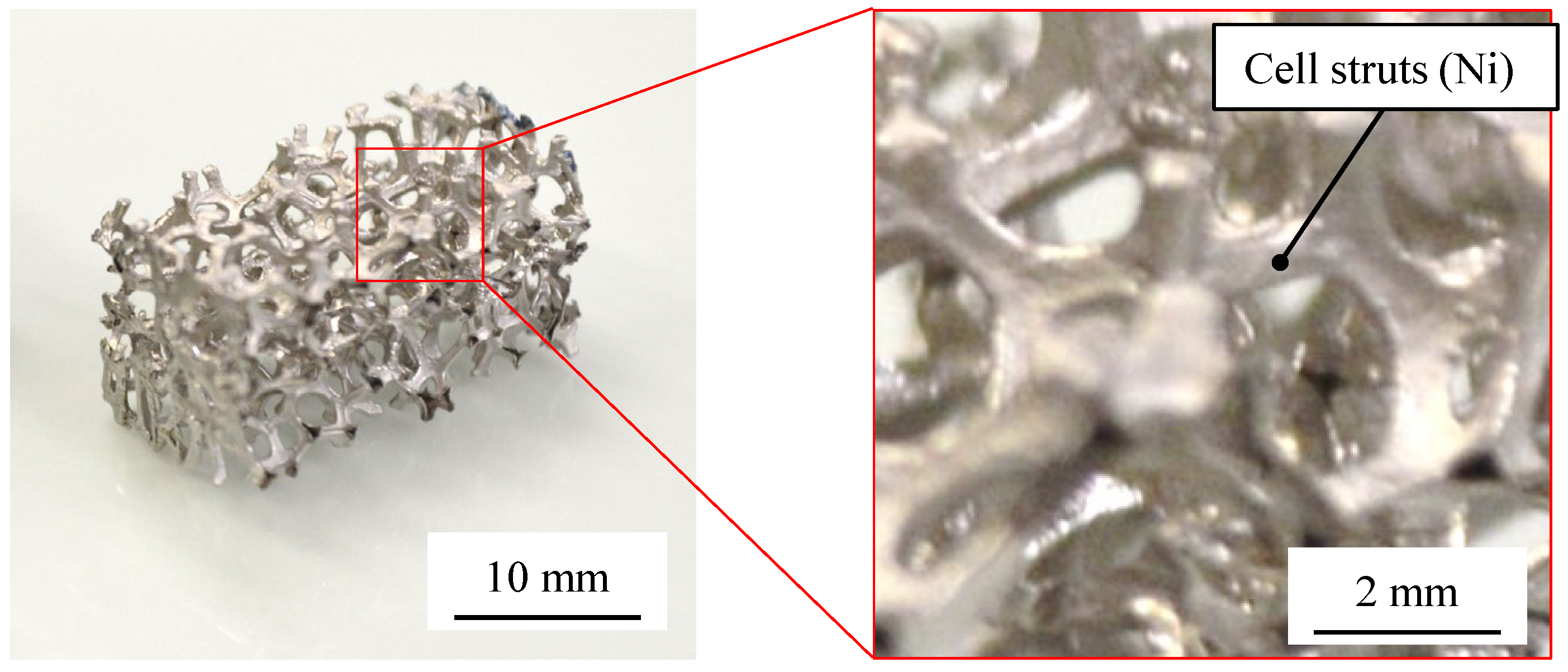
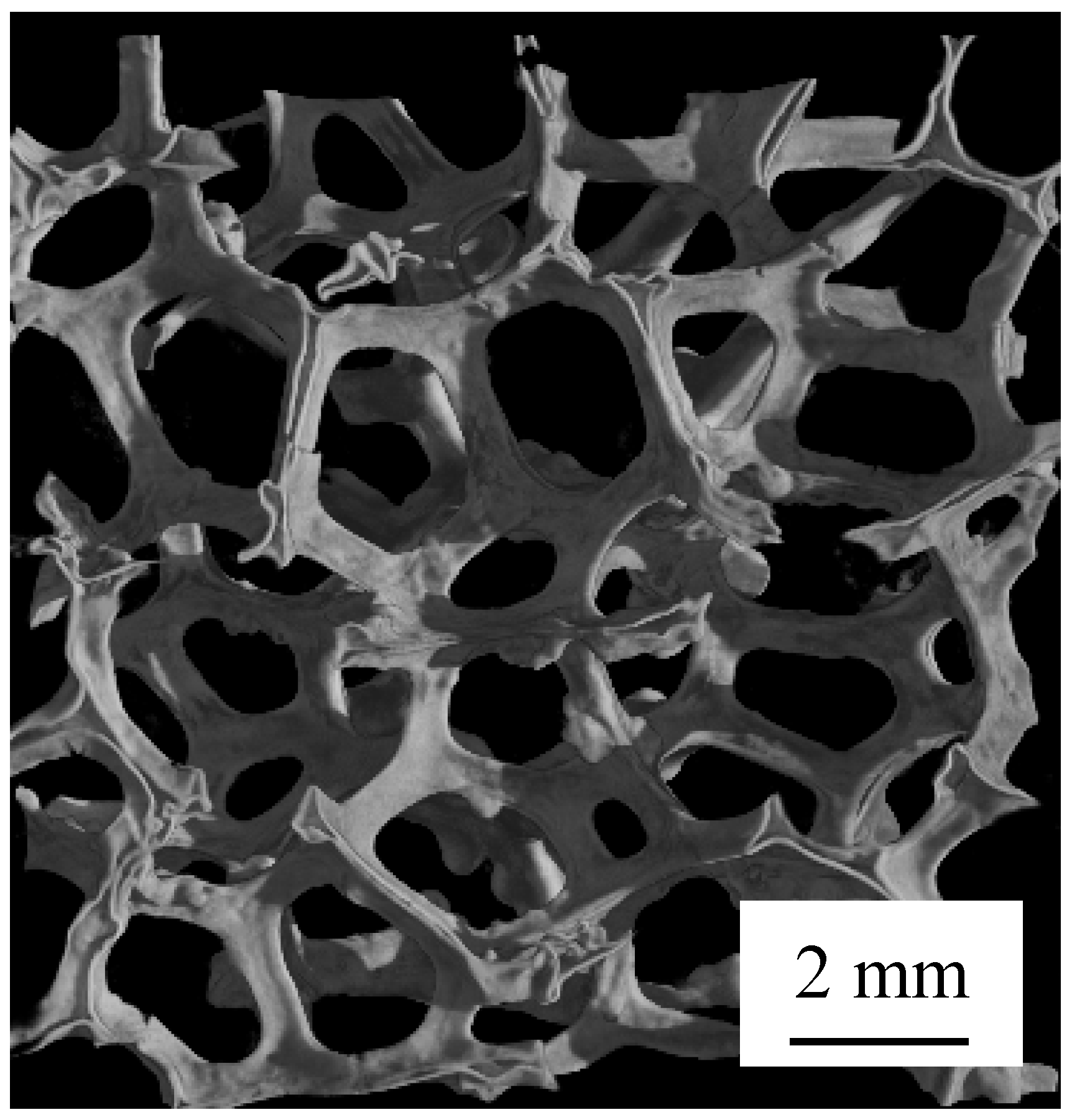
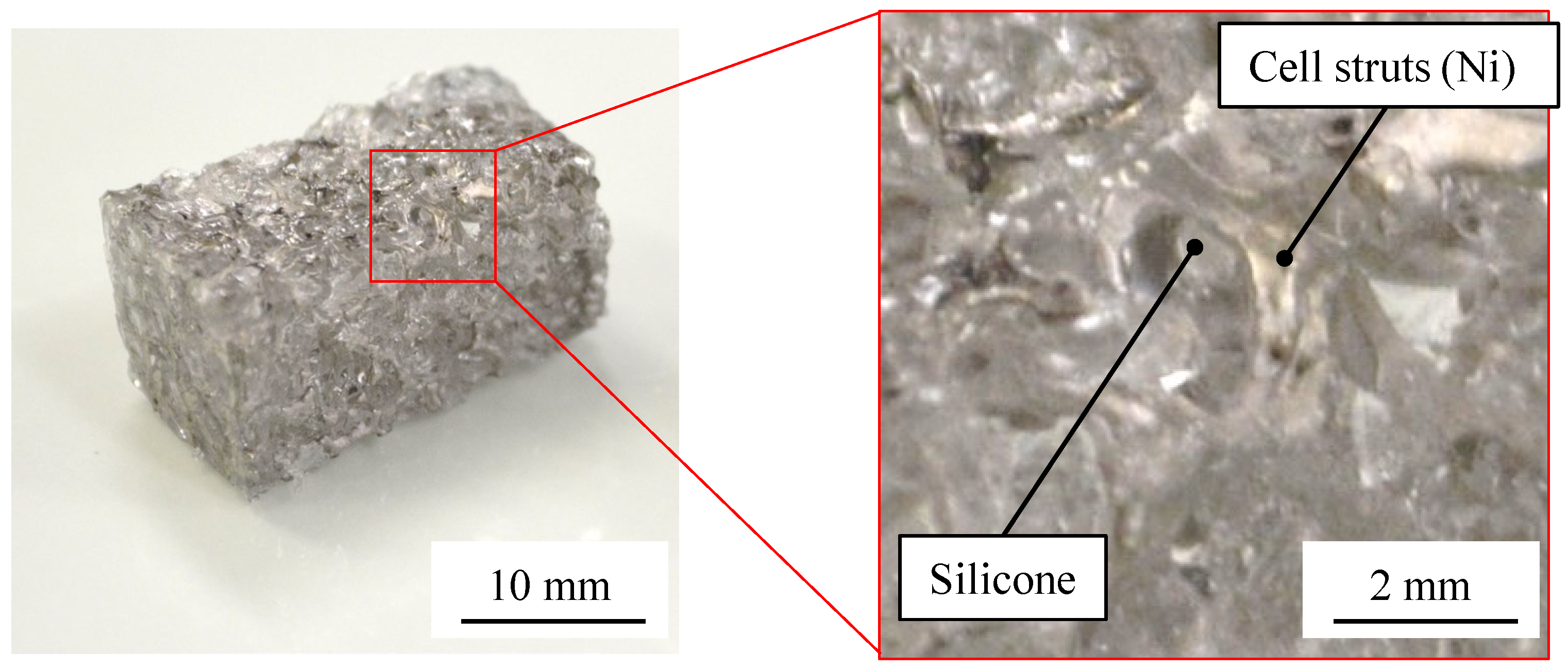

| Specimen | Ni Foam | Ni/Silicone Foam |
|---|---|---|
| Expansion ratio | Average value: 18.7 (Standard deviation: 1.91) | |
| Porosity | Average value: 95% (Standard deviation: 0.16) | |
| Dimensions | 20 × 10 × 10 mm3 | |
| Bulk density | 450 kg/m3 | 1300 kg/m3 |
| Filling in the pores | Air | Silicone rubber |
| Cell size | 3.2 mm | |
| Aperture length | 1.2 mm | |
| Cell struts thickness | 0.05 mm | |
| Cell shape | Dodecahedron | |
| Anisotropy | Isotropic | |
| Specimen | Dimensions | Density |
|---|---|---|
| Pure Ni | ϕ4 × 6 mm3 | 8900 kg/m3 |
| Silicone rubber | 10 × 10 × 10 mm3 | 1000 kg/m3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tateyama, K.; Yamada, H. Nonuniform Deformation of Cell Structures Owing to Plastic Stress Wave Propagation. Appl. Mech. 2021, 2, 911-931. https://doi.org/10.3390/applmech2040053
Tateyama K, Yamada H. Nonuniform Deformation of Cell Structures Owing to Plastic Stress Wave Propagation. Applied Mechanics. 2021; 2(4):911-931. https://doi.org/10.3390/applmech2040053
Chicago/Turabian StyleTateyama, Kohei, and Hiroyuki Yamada. 2021. "Nonuniform Deformation of Cell Structures Owing to Plastic Stress Wave Propagation" Applied Mechanics 2, no. 4: 911-931. https://doi.org/10.3390/applmech2040053
APA StyleTateyama, K., & Yamada, H. (2021). Nonuniform Deformation of Cell Structures Owing to Plastic Stress Wave Propagation. Applied Mechanics, 2(4), 911-931. https://doi.org/10.3390/applmech2040053





