Topological Properties of the 2D 2-Band System with Generalized W-Shaped Band Inversion
Abstract
:1. Introduction
2. Methods
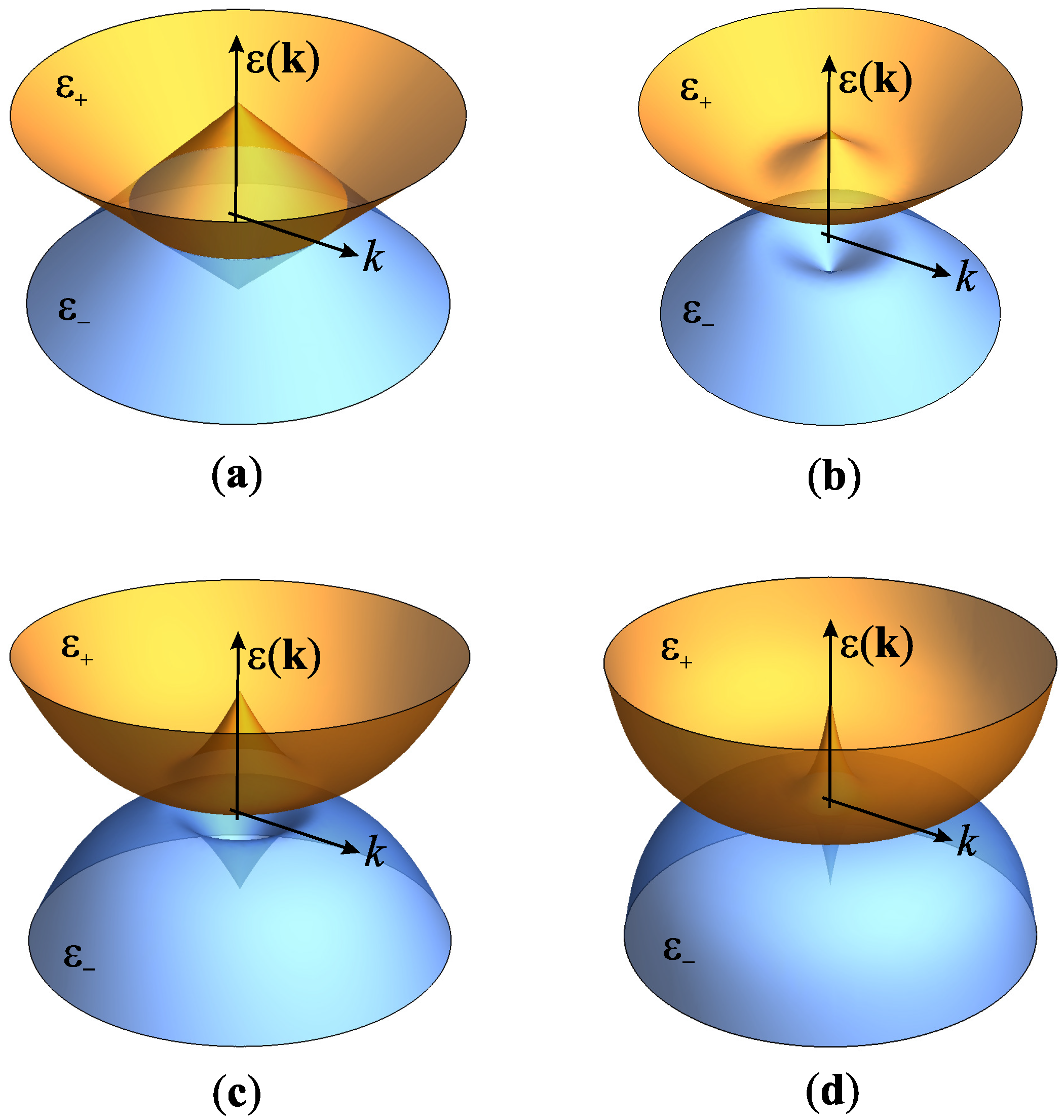
2.1. The Model
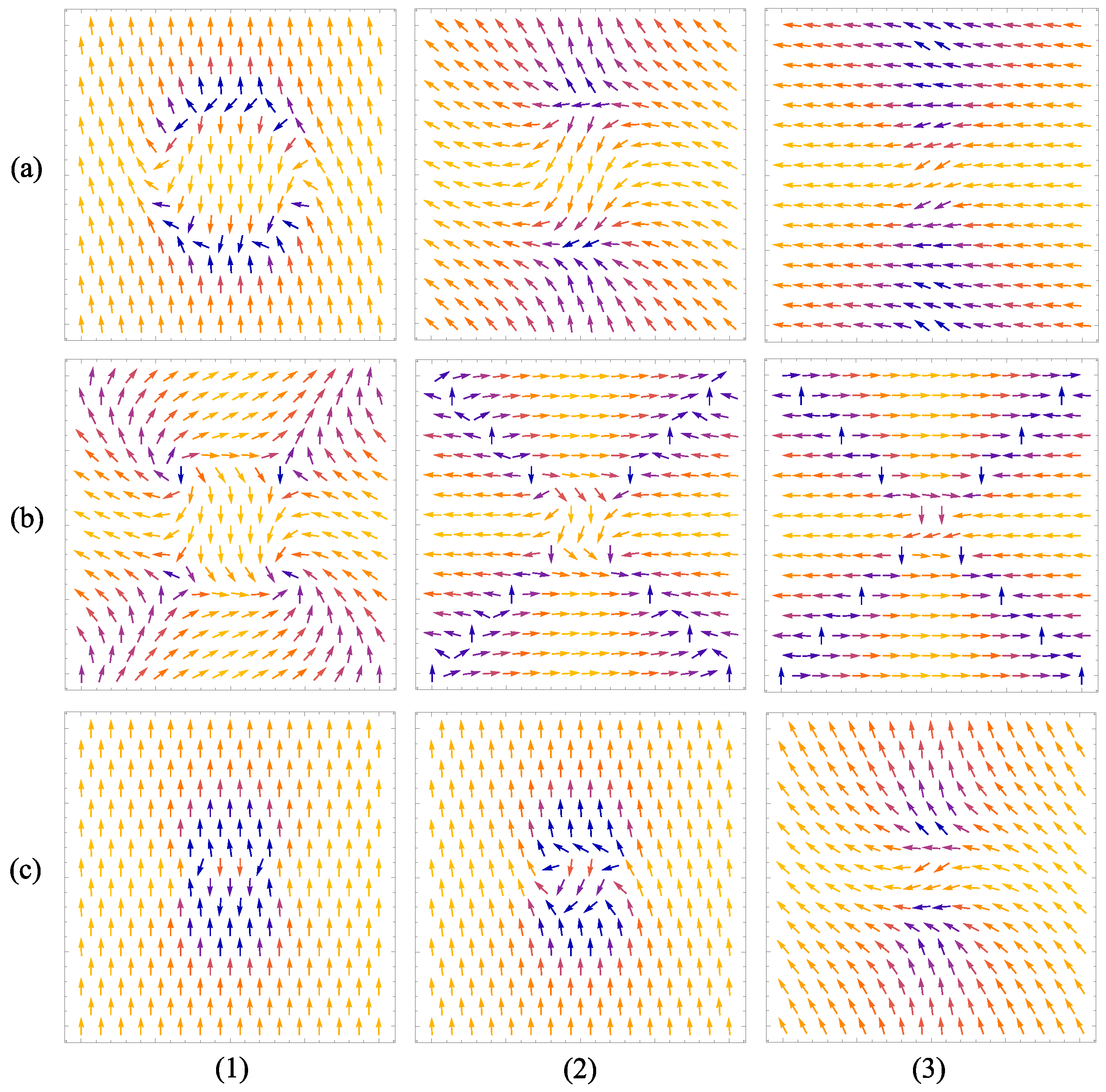
2.2. The Berry Phase
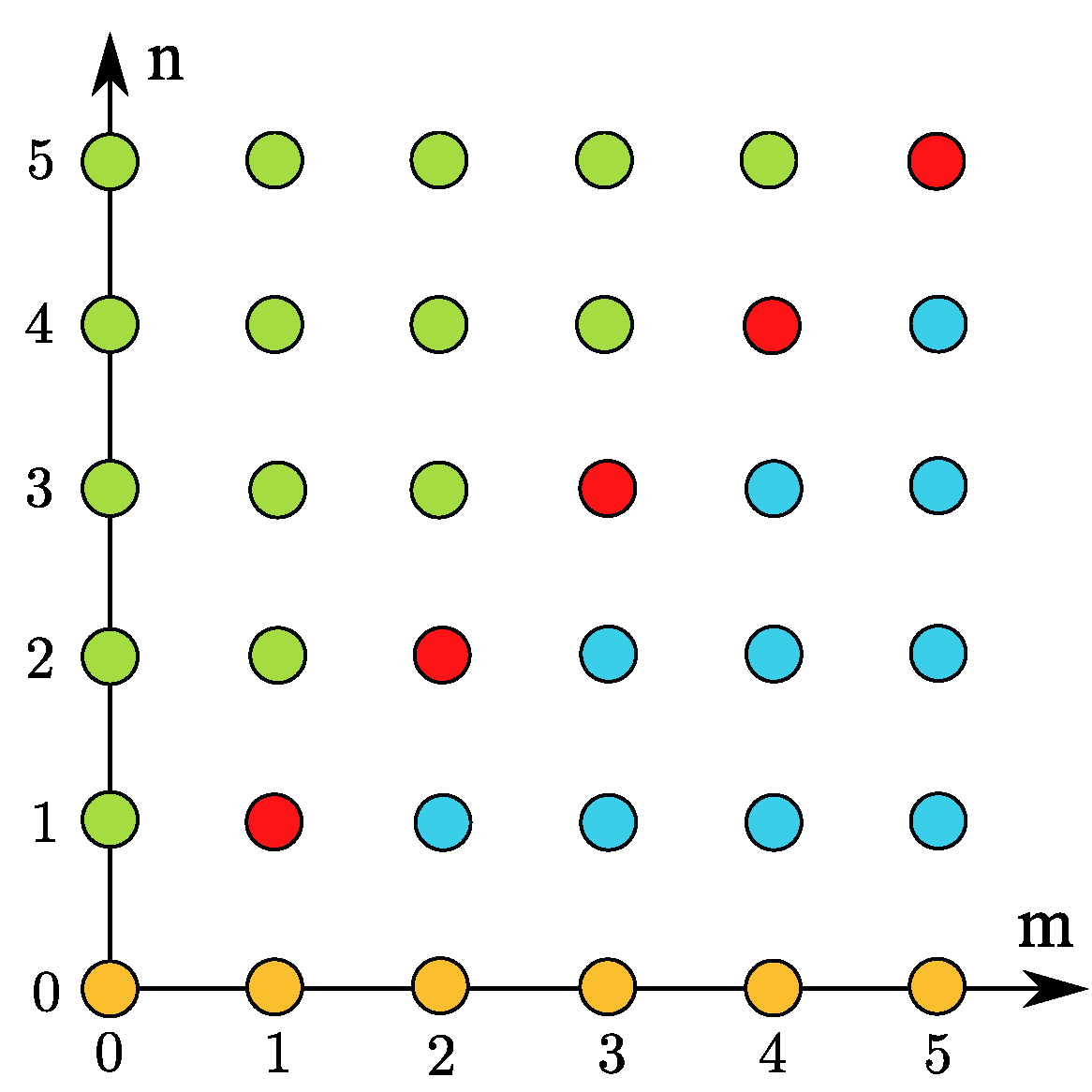
2.3. The Anomalous QHE
3. Results
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Berry, M.V. Quantal phase factor accompanying adiabatic changes. Proc. R. Soc. Lond. Ser. A 1984, 392, 45–57. [Google Scholar]
- Bohm, D. Quantm Theory; Prentice-Hall, Inc.: New York, NY, USA, 1951; Chapter 20, Section 1. [Google Scholar]
- Cohen, E.; Larocque, H.; Bouchard, F.; Nejadsattari, F.; Gefen, Y.; Karimi, E. Geometric phase from Aharonov–Bohm to Pancharatnam–Berry and beyond. Nat. Rev. Phys. 2019, 1, 437–449. [Google Scholar] [CrossRef] [Green Version]
- Moore, J. The birth of topological insulators. Nature 2010, 464, 194–198. [Google Scholar] [CrossRef] [PubMed]
- Klitzing, K.V.; Dorda, G.; Pepper, M. New Method for High-Accuracy Determination of the Fine-Structure Constant Based on Quantized Hall Resistance. Phys. Rev. Lett. 1980, 45, 494–497. [Google Scholar] [CrossRef] [Green Version]
- Klitzing, K.V. The quantized Hall effect. Rev. Mod. Phys. 1986, 58, 519–531. [Google Scholar] [CrossRef]
- Wilczek, F. Magnetic Flux, Angular Momentum, and Statistics. Phys. Rev. Lett. 1982, 48, 1144–1146. [Google Scholar] [CrossRef]
- Tsui, D.C.; Stormer, H.L.; Gossard, A.C. Two-Dimensional Magnetotransport in the Extreme Quantum Limit. Phys. Rev. Lett. 1982, 48, 1559–1562. [Google Scholar] [CrossRef] [Green Version]
- Laughlin, R.B. Anomalous Quantum Hall Effect: An Incompressible Quantum Fluid with Fractionally Charged Excitations. Phys. Rev. Lett. 1983, 50, 1395–1398. [Google Scholar] [CrossRef] [Green Version]
- Kitaev, A. Fault-tolerant quantum computation by anyons. Ann. Phys. 1997, 303, 2–30. [Google Scholar] [CrossRef] [Green Version]
- Todorić, M.; Jukić, D.; Radić, D.; Soljačić, M.; Buljan, H. Quantum Hall Effect with Composites of Magnetic Flux Tubes and Charged Particles. Phys. Rev. Lett. 2018, 120, 267201. [Google Scholar] [CrossRef] [Green Version]
- Larson, J.; Sjöquist, E.; Öhberg, P. Conical Intersections in Physics, an Introduction to Synthetic Gauge Theories; Springer Nature: Cham, Switzerland, 2020; Chapter 4; pp. 55–91. [Google Scholar]
- Chang, C.-Z.; Zhang, J.; Feng, X.; Shen, J.; Zhang, Z.; Guo, M.; Li, K.; Ou, Y.; Wei, P.; Wang, L.-L.; et al. Experimental observation of the quantum anomalous Hall effect in a magnetic topological insulator. Science 2013, 340, 167–170. [Google Scholar] [CrossRef] [PubMed]
- Haldane, F.D.M. Model for a quantum Hall Effect without Landau levels: Condensed-matter realization of the ‘parity’ anomaly. Phys. Rev. Lett. 1988, 61, 2015–2018. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jotzu, G.; Messer, M.; Desbuquois, R.; Lebrat, M.; Uehlinger, T.; Greif, D.; Esslinger, T. Experimental realization of the topological Haldane model with ultracold fermions. Nature 2014, 515, 237–240. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bernevig, B.A.; Hughes, T.L.; Zhang, S.-C. Quantum Spin Hall Effect and Topological Phase Transition in HgTe Quantum Wells. Science 2006, 314, 1757–1761. [Google Scholar] [CrossRef] [Green Version]
- Peres, N.M.R.; Santos, J.E. Strong light—Matter interaction in systems described by a modified Dirac equation. J. Phys. Condens. Matter 2013, 25, 305801. [Google Scholar] [CrossRef] [Green Version]
- Thouless, D.J.; Kohmoto, M.; Nightingale, M.P.; den Nijs, M. Quantized Hall conductance in a two-dimensional periodic potential. Phys. Rev. Lett. 1982, 49, 405–408. [Google Scholar] [CrossRef] [Green Version]
- Streda, P. Theory of quantised Hall conductivity in two dimensions. J. Phys. C Solid State Phys. 1982, 15, L717–L721. [Google Scholar] [CrossRef]
- Kohmoto, M. Topological invariant and the quantization of the Hall conductance. Ann. Phys. 1985, 160, 343–354. [Google Scholar] [CrossRef]
- Kohomoto, M. Zero modes and the quantized Hall conductance of the two-dimensional lattice in a magnetic field. Phys. Rev. B 1989, 39, 11943–11949. [Google Scholar] [CrossRef]
- Sinitsyn, N.A.; Hill, J.E.; Min, H.; Sinova, J.; MacDonald, A.H. Charge and Spin Hall Conductivity in Metallic Graphene. Phys. Rev. Lett. 2006, 97, 106804. [Google Scholar] [CrossRef] [Green Version]
- Kitaev, A. Periodic table for topological insulators and superconductors. AIP Conf. Proc. 2009, 1134, 22–30. [Google Scholar]
- Xiao, D.; Yao, W.; Niu, Q. Valley-Contrasting Physics in Graphene: Magnetic Moment and Topological Transport. Phys. Rev. Lett. 2007, 99, 236809. [Google Scholar] [CrossRef] [PubMed]
- Rukelj, Z.; Akrap, A. Carrier concentrations and optical conductivity of a band-inverted semimetal in two and three dimensions. Phys. Rev. B 2021, 104, 075108. [Google Scholar] [CrossRef]
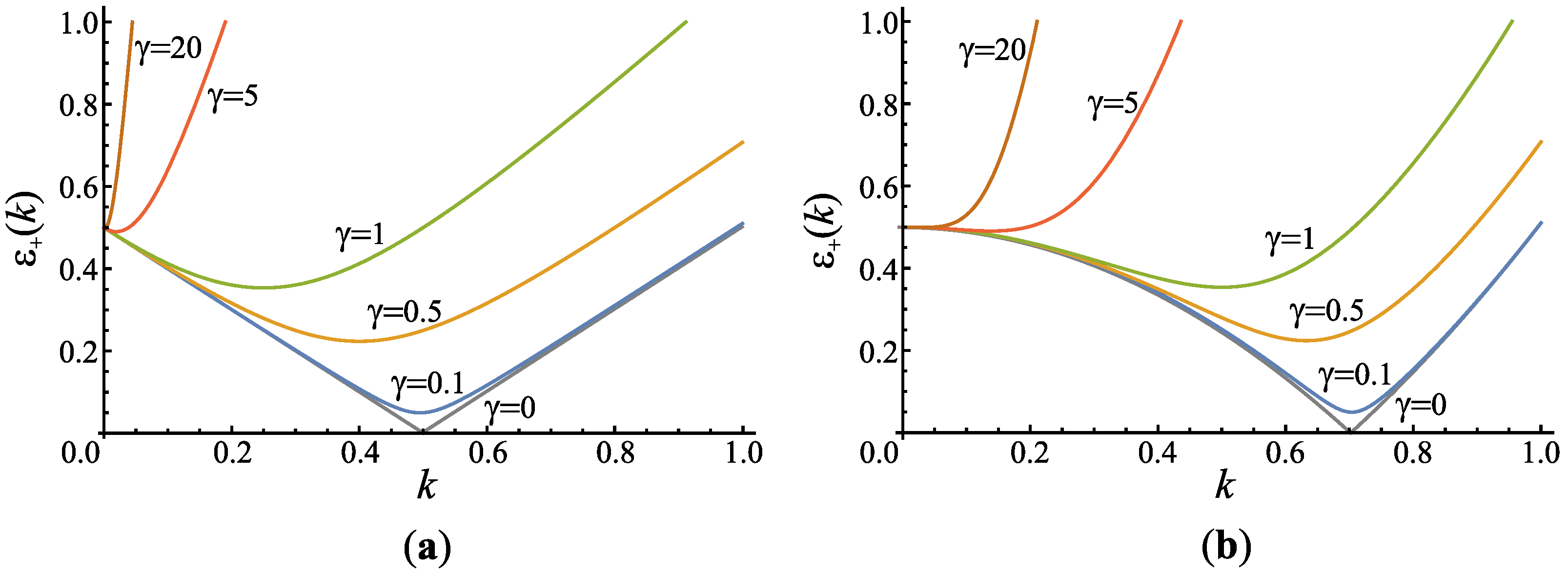

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rukelj, Z.; Radić, D. Topological Properties of the 2D 2-Band System with Generalized W-Shaped Band Inversion. Quantum Rep. 2022, 4, 476-485. https://doi.org/10.3390/quantum4040034
Rukelj Z, Radić D. Topological Properties of the 2D 2-Band System with Generalized W-Shaped Band Inversion. Quantum Reports. 2022; 4(4):476-485. https://doi.org/10.3390/quantum4040034
Chicago/Turabian StyleRukelj, Zoran, and Danko Radić. 2022. "Topological Properties of the 2D 2-Band System with Generalized W-Shaped Band Inversion" Quantum Reports 4, no. 4: 476-485. https://doi.org/10.3390/quantum4040034
APA StyleRukelj, Z., & Radić, D. (2022). Topological Properties of the 2D 2-Band System with Generalized W-Shaped Band Inversion. Quantum Reports, 4(4), 476-485. https://doi.org/10.3390/quantum4040034






