Casimir Effect between Superconducting Plates in the Mixed State
Abstract
:1. Introduction
2. Casimir Force between Parallel Plates
3. Permittivity of Superconductor in the Mixed State
3.1. Relationship between Conductivity and Permittivity
3.2. Permittivity along the Imaginary Axis
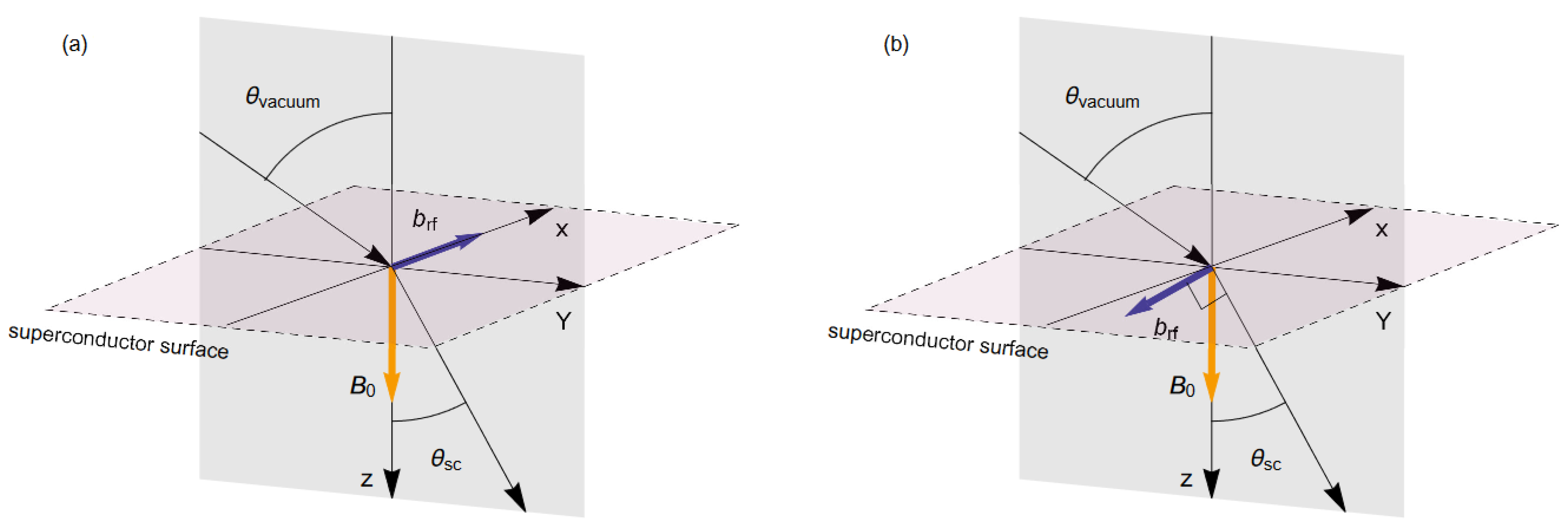
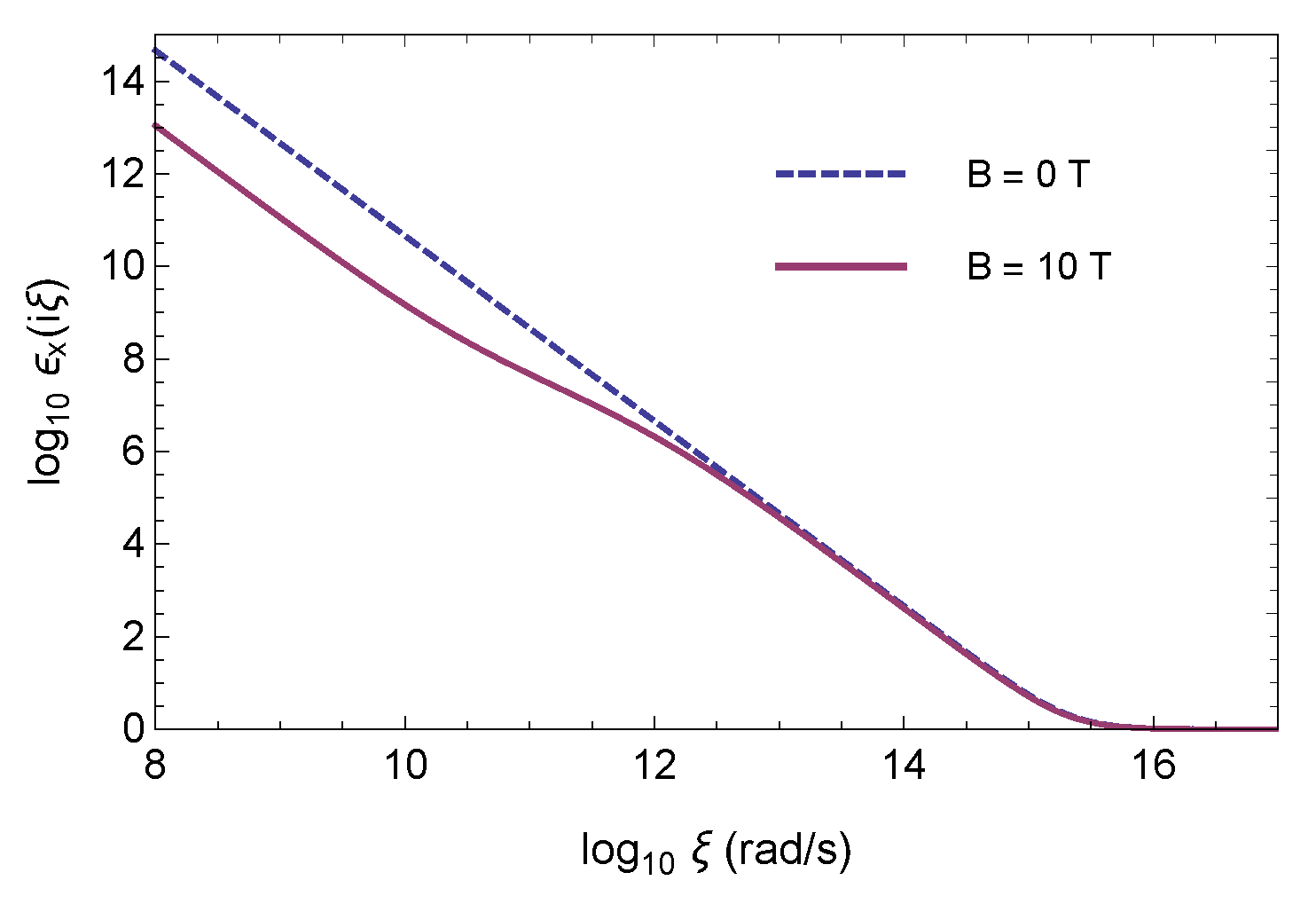
4. Effect of External Magnetic Field on Casimir Force between Superconductive Plates
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Derivation of the Penetration Depth
References
- Stange, A.; Campbell, D.K.; Bishop, D.J. Science and technology of the Casimir effect. Phys. Today 2021, 74, 42–48. [Google Scholar] [CrossRef]
- Lamoreaux, S.K. Demonstration of the Casimir force in the 0.6 to 6 μm range. Phys. Rev. Lett. 1997, 78, 5–8. [Google Scholar] [CrossRef] [Green Version]
- Milonni, P.W. The Quantum Vacuum; Academic Press: San Diego, CA, USA, 1994. [Google Scholar]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in the Casimir Effect; Oxford University Press: New York, NY, USA, 2009. [Google Scholar]
- Casimir, H.B.G. On the attraction between two perfectly counducting plates. Proc. Kon. Ned. Akad. Wet. 1948, 51, 793–795. [Google Scholar]
- Mohideen, U.; Roy, A. Precision Measurement of the Casimir Force from 0.1 to 0.9 μm. Phys. Rev. Lett. 1998, 81, 4549–4552. [Google Scholar] [CrossRef] [Green Version]
- Banishev, A.A.; Chang, C.C.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Measurement of the gradient of the Casimir force between a nonmagnetic gold sphere and a magnetic nickel plate. Phys. Rev. B 2012, 85, 195422. [Google Scholar] [CrossRef] [Green Version]
- Banishev, A.A.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Casimir interaction between two magnetic metals in comparison with nonmagnetic test bodies. Phys. Rev. B 2013, 88, 155410. [Google Scholar] [CrossRef] [Green Version]
- Rosa, F.S.S.; Dalvit, D.A.R.; Milonni, P.W. Casimir-Lifshitz theory and metamaterials. Phys. Rev. Lett. 2008, 100, 183602. [Google Scholar] [CrossRef] [Green Version]
- Bimonte, G.; Calloni, E.; Esposito, G.; Milano, L.; Rosa, L. Towards measuring variations of Casimir energy by a superconducting cavity. Phys. Rev. Lett. 2005, 94, 180402. [Google Scholar] [CrossRef] [Green Version]
- Bimonte, G.; Calloni, E.; Esposito, G.; Rosa, L. Variations of Casimir energy from a superconducting transition. Nuc. Phys. B 2005, 726, 441–463. [Google Scholar] [CrossRef] [Green Version]
- Bimonte, G. Casimir effect in a superconducting cavity and the thermal controversy. Phys. Rev. A 2008, 78, 062101. [Google Scholar] [CrossRef] [Green Version]
- Sushkov, A.O.; Kim, W.J.; Dalvit, D.A.R.; Lamoreaux, S.K. Observation of the thermal Casimir force. Nat. Phys. 2011, 7, 230–233. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Recent measurements of the Casimir force: Comparison between experiment and theory. Mod. Phys. Lett. 2020, 35, 2040007. [Google Scholar] [CrossRef] [Green Version]
- Perez-Morelo, D.; Stange, A.; Lally, R.W.; Barrett, L.K.; Imboden, M.; Som, A.; Campbell, D.K.; Aksyuk, V.A.; Bishop, D.J. A system for probing Casimir energy corrections to the condensation energy. Microsyst. Nanoeng. 2020, 6, 115. [Google Scholar] [CrossRef] [PubMed]
- Norte, R.A.; Forsch, M.; Wallucks, A.; Marinković, I.; Gröblacher, S. Platform for Measurements of the Casimir Force between Two Superconductors. Phys. Rev. Lett. 2018, 121, 030405. [Google Scholar] [CrossRef] [Green Version]
- Bimonte, G. Casimir effect between superconductors. Phys. Rev. A 2019, 99, 052507. [Google Scholar] [CrossRef] [Green Version]
- Quach, J.Q. Gravitational Casimir Effect. Phys. Rev. Lett. 2015, 114, 081104. [Google Scholar] [CrossRef]
- Golosovsky, M.; Tsindlekht, M.; Davidov, D. High-frequency vortex dynamics in YBa2Cu3O7. Supercond. Sci. Technol. 1996, 9, 1–7. [Google Scholar] [CrossRef]
- Brandt, E.H. Penetration of magnetic ac fields into type-II superconductors. Phys. Rev. Lett. 1991, 67, 2219–2222. [Google Scholar] [CrossRef]
- Kogan, V.G.; Nakagawa, N. Current distributions by moving vortices in superconductors. Phys. Rev. B 2021, 103, 134511. [Google Scholar] [CrossRef]
- Kogan, V.G.; Nakagawa, N. Moving vortices in anisotropic superconductors. Phys. Rev. B 2021, 104, 094523. [Google Scholar] [CrossRef]
- Villarreal, C.; Caballero-Benitez, S.F. Casimir forces and high-Tc superconductors. Phys. Rev. A 2019, 100, 042504. [Google Scholar] [CrossRef] [Green Version]
- Castillo-Lopez, S.G.; Villarreal, C.; Pirruccio, G.; Esquivel-Sirvent, R. Role of electronic relaxation rates in the Casimir force between high-Tc fuperconductors. Universe 2021, 7, 69. [Google Scholar] [CrossRef]
- Moser, E.K.; Tomasch, W.J.; McClorey, M.J.; Furdyna, J.K.; Coffey, N.W.; Pettiette-Hall, C.L.; Schwarzbek, S.M. Microwave properties of YBa2Cu307−x films at 35 GHz from magnetotransmission and magnetoreflection measurements. Phys. Rev. B 1994, 49, 4199–4208. [Google Scholar] [CrossRef] [PubMed]
- Coffey, M.W.; Clem, J.R. Magnetic field dependence of RF surface impedance. IEEE Trans. Mag. 1991, 27, 2136–2139. [Google Scholar] [CrossRef]
- Coffey, M.W.; Clem, J.R. Unified theory of effects of vortex pinning and flux creep upon the rf surface impedance of type-II superconductors. Phys. Rev. Lett. 1991, 67, 386–389. [Google Scholar] [CrossRef]
- Coffey, M.W.; Clem, J.R. Theory of microwave transmission and reflection in type-II superconductors in the mixed state. Phys. Rev. B 1993, 48, 342–350. [Google Scholar] [CrossRef]
- Lifshitz, E.M. The theory of molecular arrtactive forces between solids. Sov. Phys. JETP 1956, 2, 73–83. [Google Scholar]
- Greenaway, D.L.; Harbeke, G.; Bassani, F.; Tosatti, E. Anisotropy of the Optical Constants and the Band Structure of Graphite. Phys. Rev. 1969, 178, 1340–1348. [Google Scholar] [CrossRef]
- Tinkham, M. Energy gap interpretation of experiments on infrared transmission through superconducting films. Phys. Rev. 1956, 104, 845. [Google Scholar] [CrossRef]
- Kubo, R. Statistical-mechanical theory of irreversible processes. I. general theory and simple applications to magnetic and conduction problems. J. Phys. Soc. Jpn. 1957, 12, 570–586. [Google Scholar] [CrossRef]
- Wu, D.-H.; Sridhar, S. Pinning forces and lower critical fields in YBa2Cu3Oy crystals: Temperature dependence and anisotropy. Phys. Rev. Lett. 1990, 65, 2074–2077. [Google Scholar] [CrossRef]
- Bonn, D.A.; O‘Reilly, A.H.; Greedan, J.E.; Stager, C.V.; Timusk, T.; Kamaras, K.; Tanner, D.B. Far-infrared properties of ab-plane oriented YBa2Cu3O7. Phys. Rev. B 1988, 37, 1574–1579. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Coffey, M.W.; Clem, J.R. Theory of rf magnetic permeability of type-II superconductors in slab geometry with an oblique applied static magnetic field. Phys. Rev. B 1992, 45, 10527–10535. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Klimchitskaya, G.L.; Romero, C. Surface impedance and the Casimir force. Phys. Rev. A 2001, 65, 012111. [Google Scholar] [CrossRef] [Green Version]
- Gong, T.; Corrado, M.R.; Mahbub, A.R.; Shelden, C.; Munday, J.N. Recent progress in engineering the Casimir effect? Applications to nanophotonics, nanomechanics, and chemistry. Nanophotonics 2021, 10, 523–536. [Google Scholar] [CrossRef]
- Chen, F.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Control of the Casimir force by the modification of dielectric properties with light. Phys. Rev. B 2007, 76, 035338. [Google Scholar] [CrossRef] [Green Version]
- Iannuzzi, D.; Lisanti, M.; Capasso, F. Effect of hydrogen-switchable mirrors on the Casimir force. Proc. Natl. Acad. Sci. USA 2004, 101, 4019–4023. [Google Scholar] [CrossRef] [PubMed] [Green Version]




| 88.2 K | |
| 87 K | |
| 140 nm | |
| 112 T | |
| 2.1 × N/m | |
| 2.0 × 10 m | |
| 9.7 × 10 m/K |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Inui, N. Casimir Effect between Superconducting Plates in the Mixed State. Quantum Rep. 2021, 3, 731-745. https://doi.org/10.3390/quantum3040046
Inui N. Casimir Effect between Superconducting Plates in the Mixed State. Quantum Reports. 2021; 3(4):731-745. https://doi.org/10.3390/quantum3040046
Chicago/Turabian StyleInui, Norio. 2021. "Casimir Effect between Superconducting Plates in the Mixed State" Quantum Reports 3, no. 4: 731-745. https://doi.org/10.3390/quantum3040046
APA StyleInui, N. (2021). Casimir Effect between Superconducting Plates in the Mixed State. Quantum Reports, 3(4), 731-745. https://doi.org/10.3390/quantum3040046






