Gain-Assisted Optical Pulling Force on Plasmonic Graded Nano-Shell with Equivalent Medium Theory
Abstract
:1. Introduction
2. Models and Methods
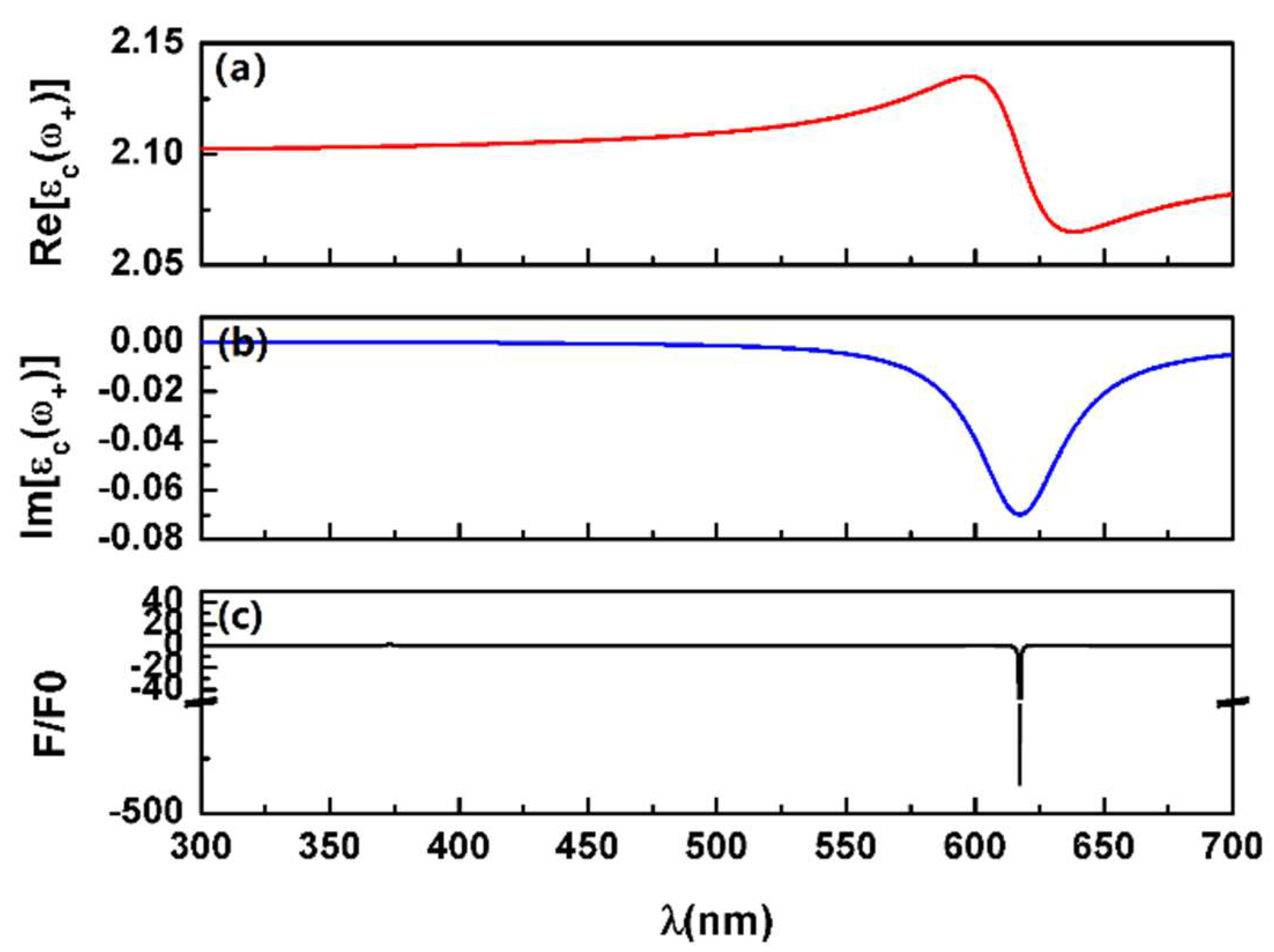
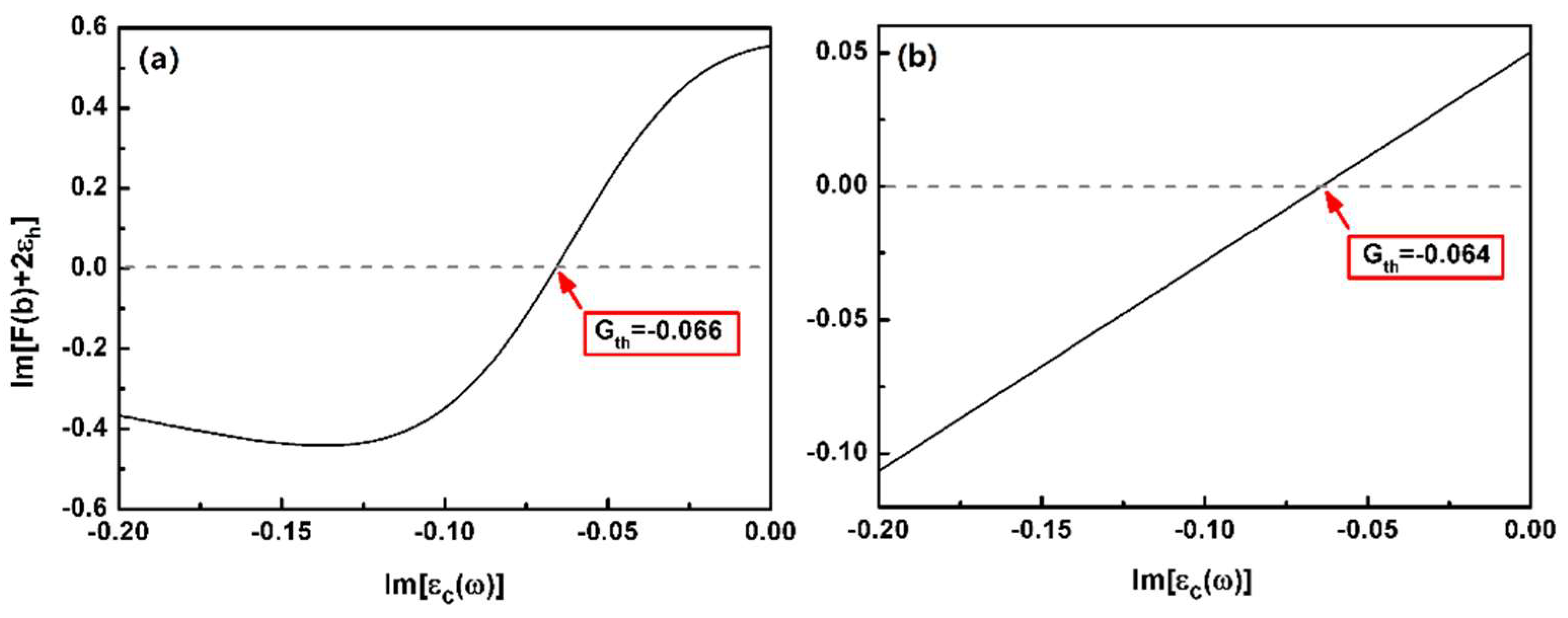
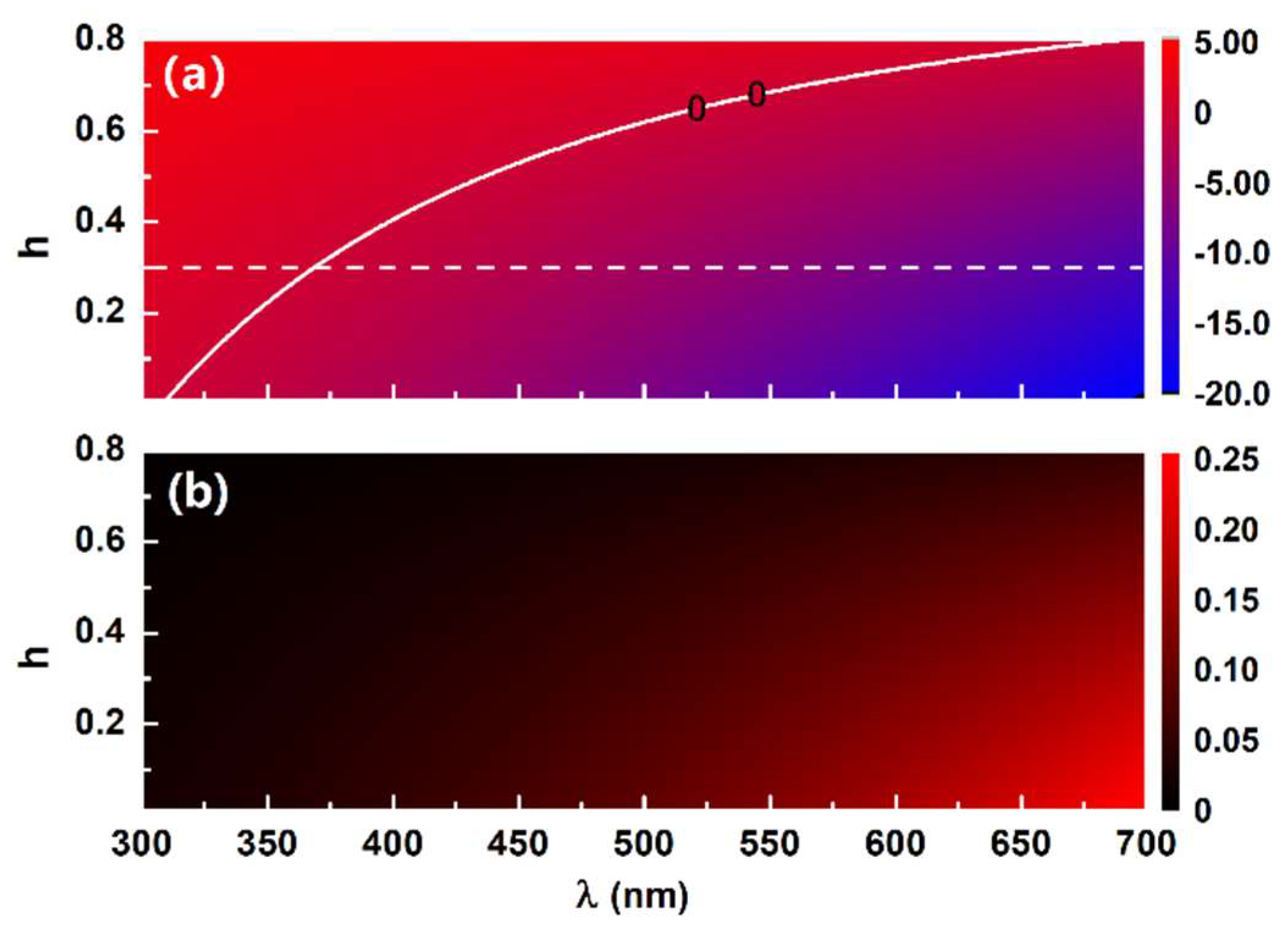
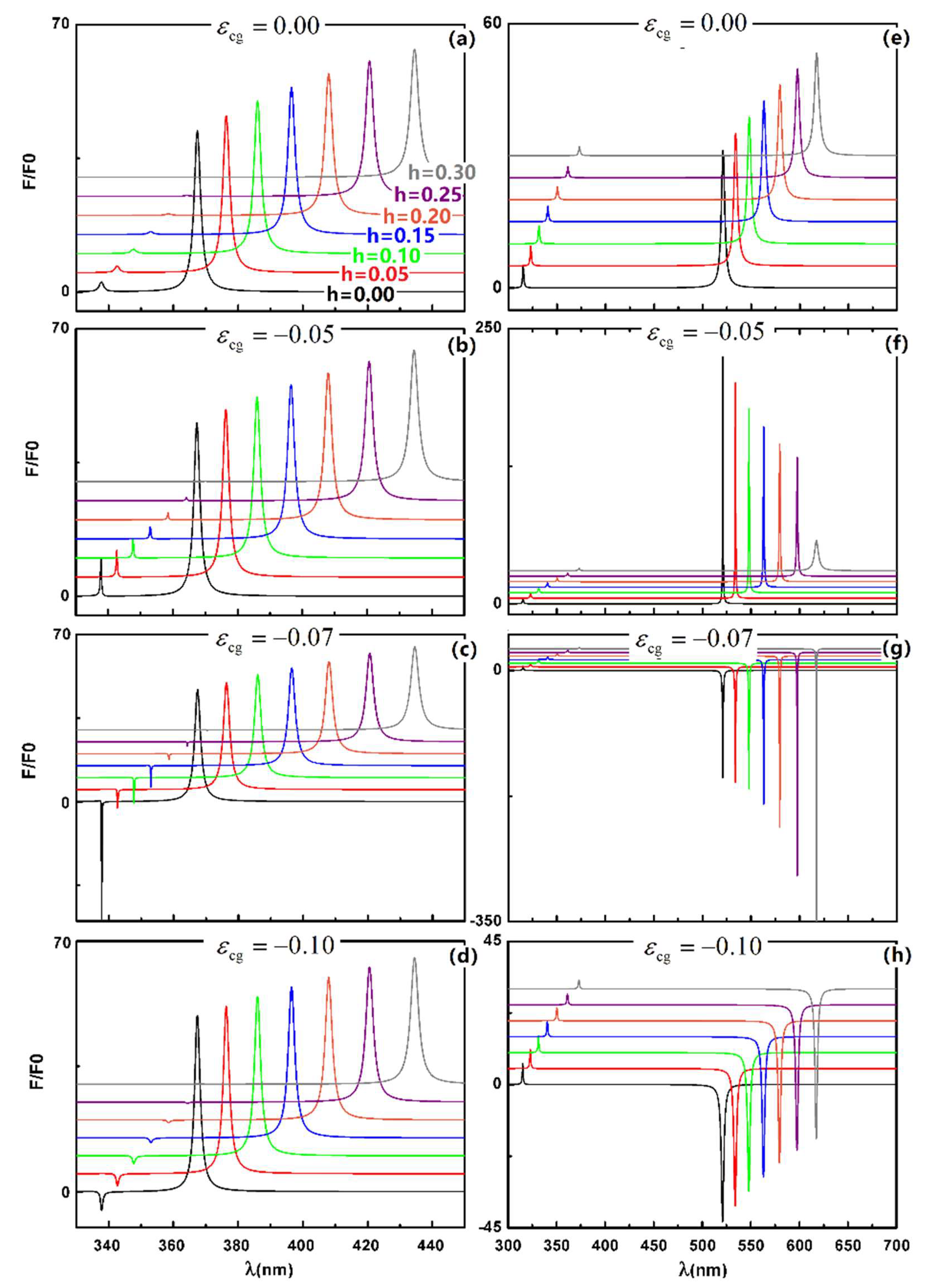
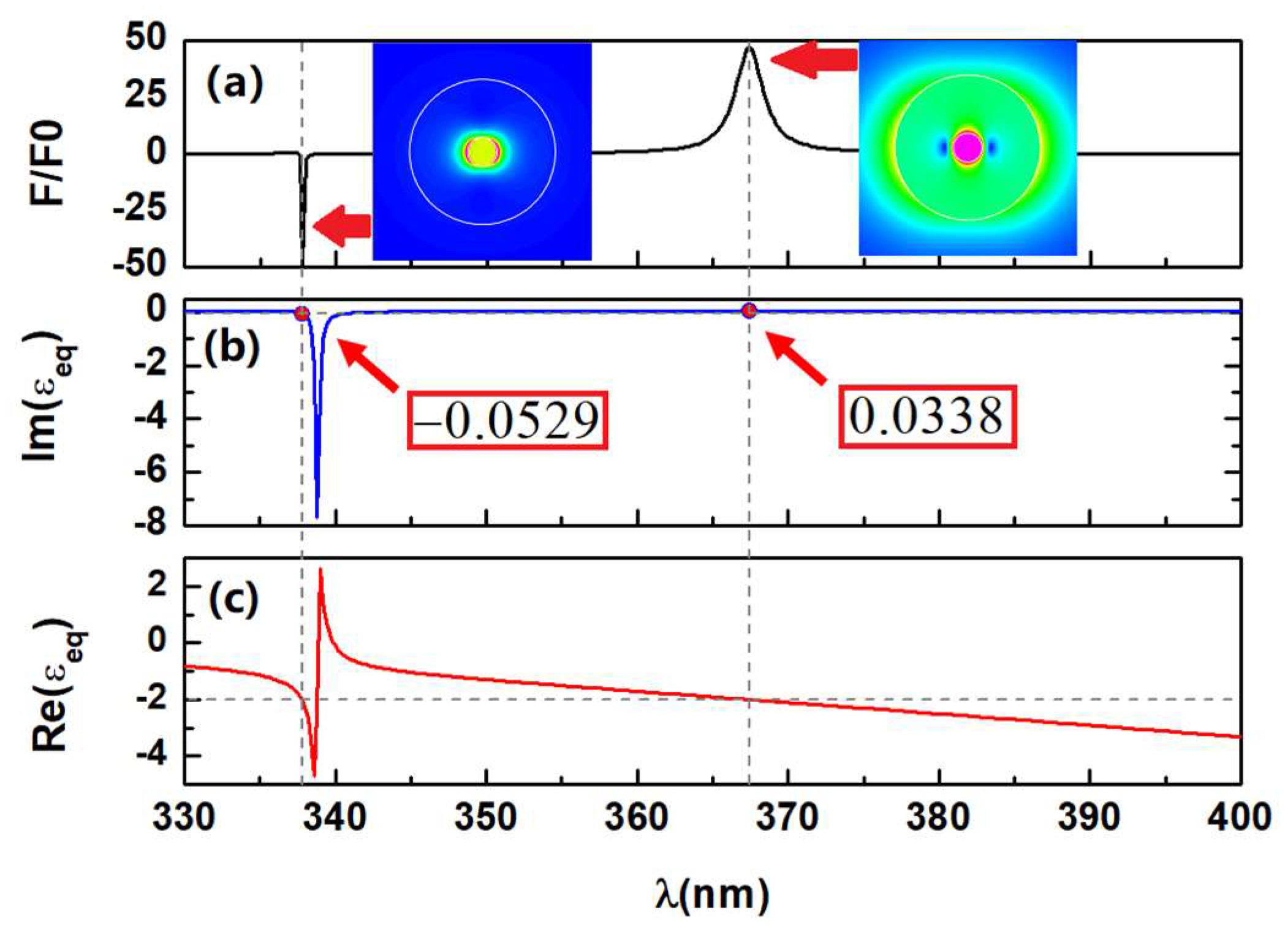
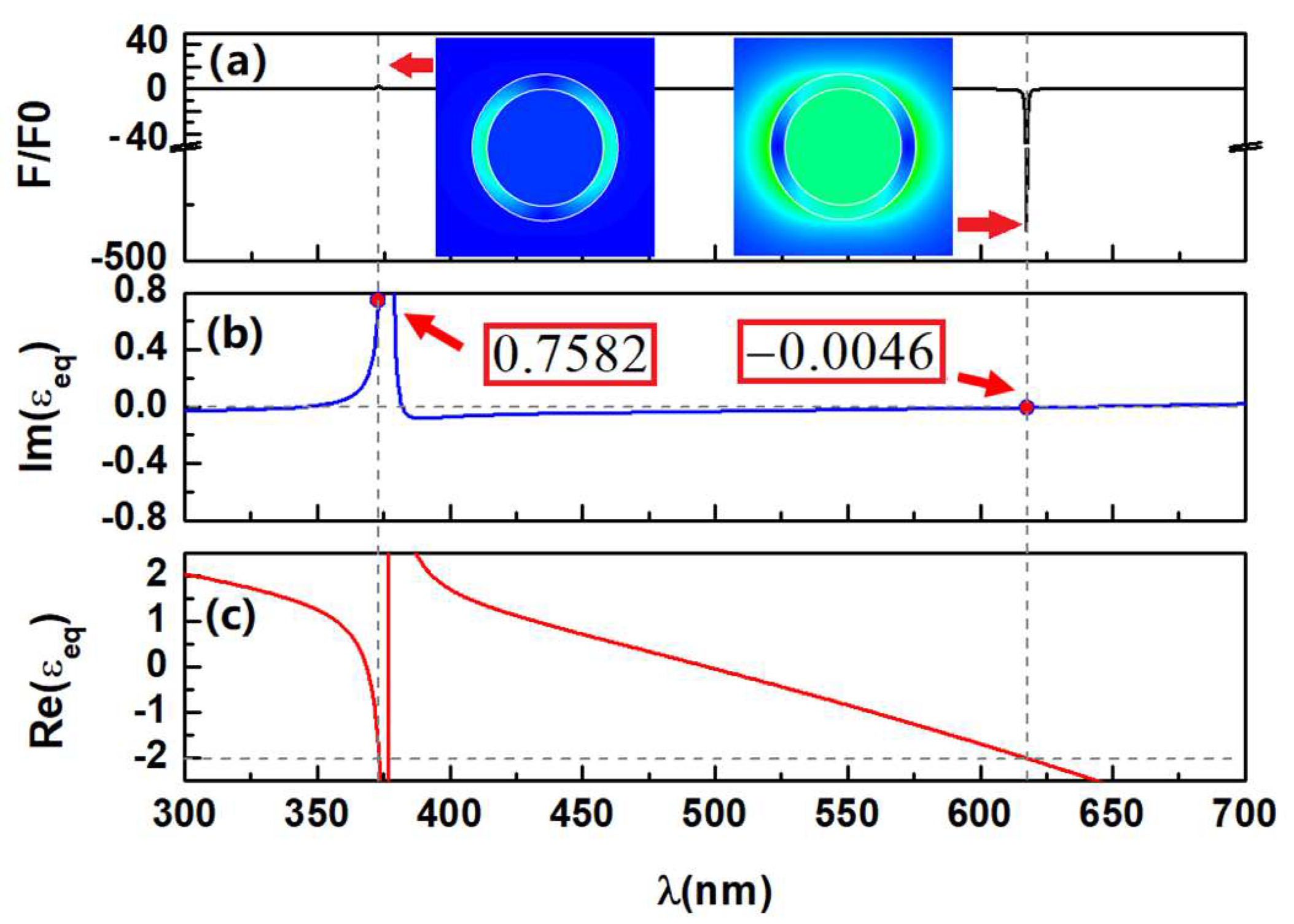
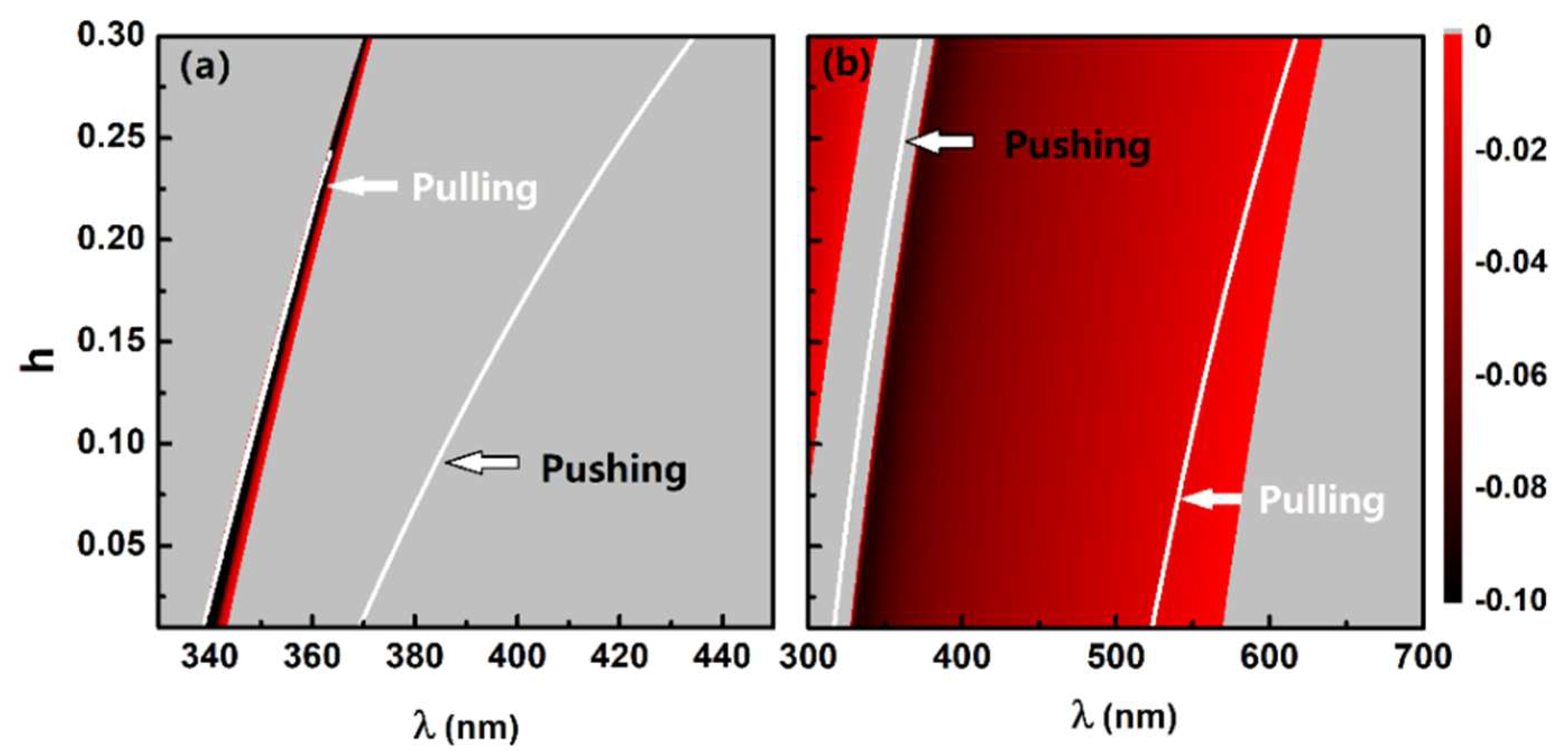
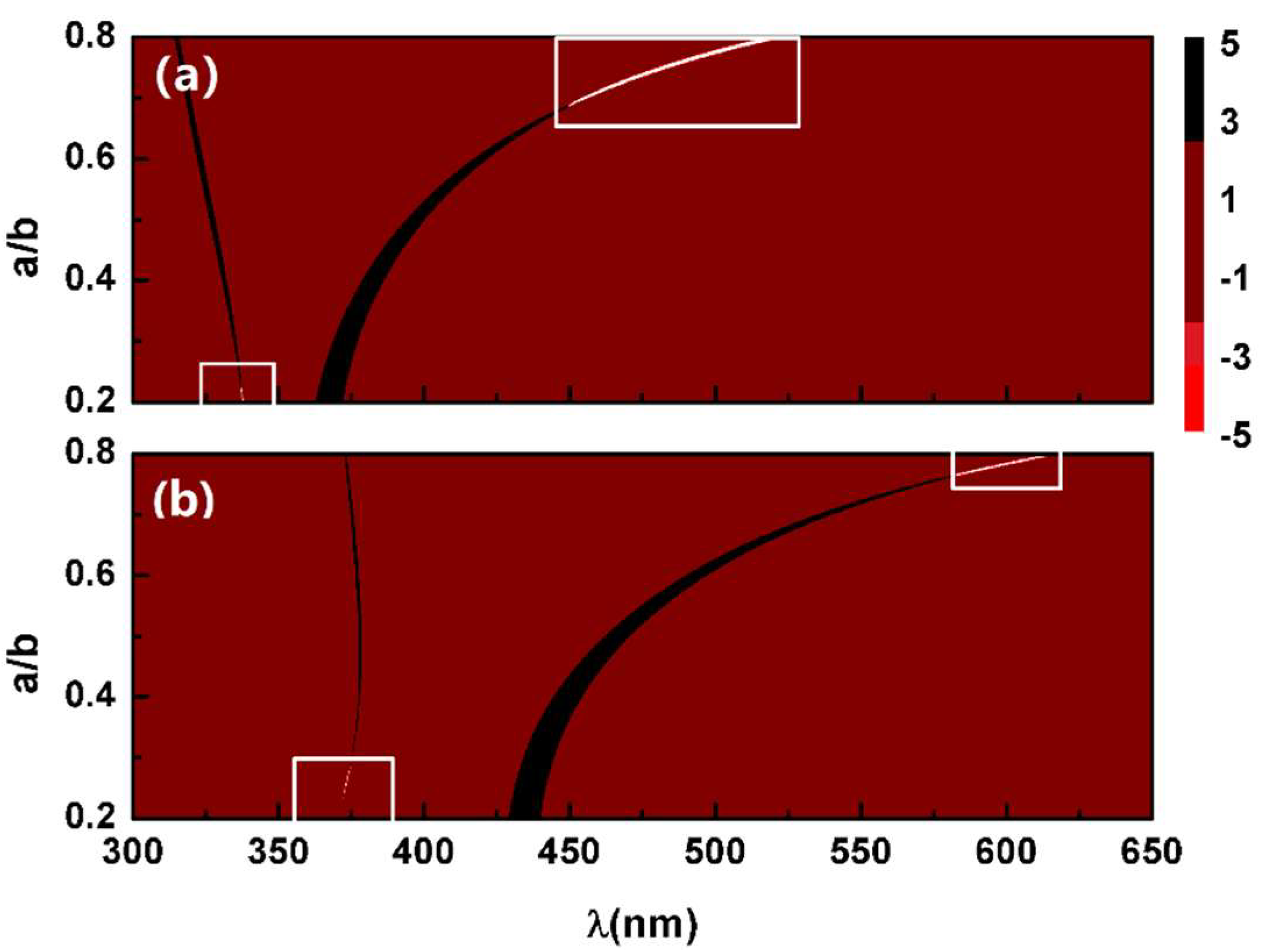
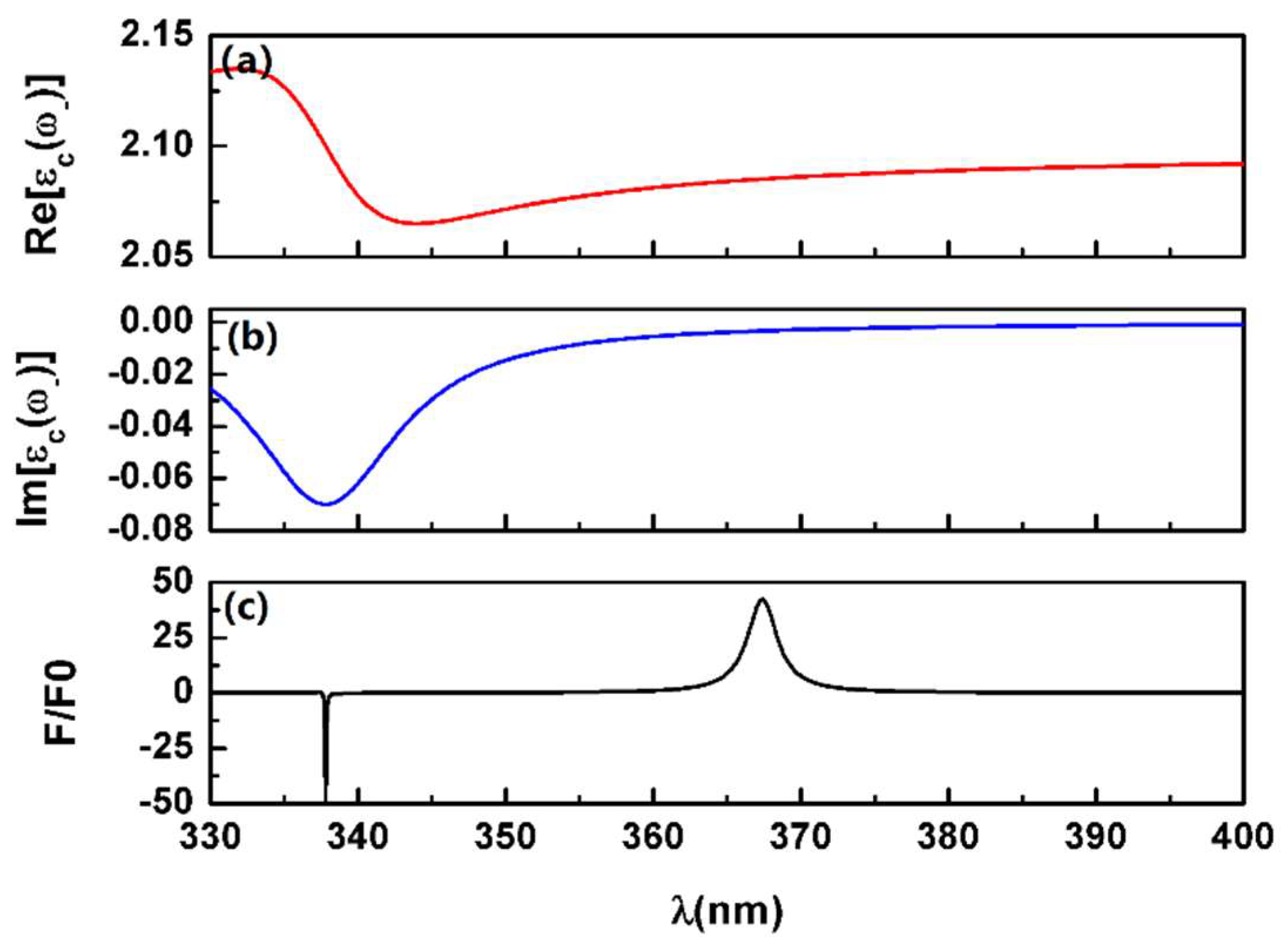
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xin, H.; Li, Y.; Liu, Y.; Zhang, Y.; Xiao, Y.; Li, B. Optical Forces: From Fundamental to Biological Applications. Adv. Mater. 2020, 32, 2001994. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Ng, J.; Lin, Z.; Chan, C.T. Optical Pulling Force. Nat. Photonics 2011, 5, 531–534. [Google Scholar] [CrossRef] [Green Version]
- Novitsky, A.; Qiu, C.-W.; Wang, H. Single Gradientless Light Beam Drags Particles as Tractor Beams. Phys. Rev. Lett. 2011, 107, 203601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dogariu, A.; Sukhov, S.; Sáenz, J. Optically Induced “Negative Forces”. Nat. Photonics 2013, 7, 24–27. [Google Scholar] [CrossRef]
- Gao, D.; Novitsky, A.; Zhang, T.; Cheong, F.C.; Gao, L.; Lim, C.T.; Luk’yanchuk, B.; Qiu, C.-W. Unveiling the Correlation between Non-Diffracting Tractor Beam and Its Singularity in Poynting Vector. Laser Photonics Rev. 2015, 9, 75–82. [Google Scholar] [CrossRef]
- Zhang, L.; Qiu, X.; Zeng, L.; Chen, L. Multiple Trapping Using a Focused Hybrid Vector Beam. Chin. Phys. B 2019, 28, 094202. [Google Scholar] [CrossRef]
- Ling, L.; Guo, H.-L.; Huang, L.; Qu, E.; Li, Z.-L.; Li, Z.-Y. The Measurement of Displacement and Optical Force in Multi-Optical Tweezers. Chin. Phys. Lett. 2012, 29, 014214. [Google Scholar] [CrossRef]
- Lepeshov, S.; Krasnok, A. Virtual Optical Pulling Force. Optica 2020, 7, 1024. [Google Scholar] [CrossRef]
- Guo, G.; Feng, T.; Xu, Y. Tunable Optical Pulling Force Mediated by Resonant Electromagnetic Coupling. Opt. Lett. 2018, 43, 4961. [Google Scholar] [CrossRef]
- Lee, E.; Huang, D.; Luo, T. Ballistic Supercavitating Nanoparticles Driven by Single Gaussian Beam Optical Pushing and Pulling Forces. Nat. Commun. 2020, 11, 2404. [Google Scholar] [CrossRef] [PubMed]
- Novitsky, A.; Qiu, C.-W. Pulling Extremely Anisotropic Lossy Particles Using Light without Intensity Gradient. Phys. Rev. A 2014, 90, 053815. [Google Scholar] [CrossRef] [Green Version]
- Ding, K.; Ng, J.; Zhou, L.; Chan, C.T. Realization of Optical Pulling Forces Using Chirality. Phys. Rev. A 2014, 89, 063825. [Google Scholar] [CrossRef] [Green Version]
- Li, G.; Wang, M.; Li, H.; Yu, M.; Dong, Y.; Xu, J. Wave Propagation and Lorentz Force Density in Gain Chiral Structures. Opt. Mater. Express 2016, 6, 388. [Google Scholar] [CrossRef]
- Wang, M.; Li, H.; Gao, D.; Gao, L.; Xu, J.; Qiu, C.-W. Radiation Pressure of Active Dispersive Chiral Slabs. Opt. Express 2015, 23, 16546. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shalin, A.S.; Sukhov, S.V.; Bogdanov, A.A.; Belov, P.A.; Ginzburg, P. Optical Pulling Forces in Hyperbolic Metamaterials. Phys. Rev. A 2015, 91, 063830. [Google Scholar] [CrossRef] [Green Version]
- Chen, H.; Gao, L.; Zhong, C.; Yuan, G.; Huang, Y.; Yu, Z.; Cao, M.; Wang, M. Optical Pulling Force on Nonlinear Nanoparticles with Gain. AIP Adv. 2020, 10, 015131. [Google Scholar] [CrossRef]
- Duan, X.-Y.; Wang, Z.-G. Fano Resonances in the Optical Scattering Force upon a High-Index Dielectric Nanoparticle. Phys. Rev. A 2017, 96, 053811. [Google Scholar] [CrossRef]
- Mizrahi, A.; Fainman, Y. Negative Radiation Pressure on Gain Medium Structures. Opt. Lett. 2010, 35, 3405. [Google Scholar] [CrossRef]
- Chen, H.; Ye, Q.; Zhang, Y.; Shi, L.; Liu, S.; Jian, Z.; Lin, Z. Reconfigurable Lateral Optical Force Achieved by Selectively Exciting Plasmonic Dark Modes near Fano Resonance. Phys. Rev. A 2017, 96, 023809. [Google Scholar] [CrossRef]
- Song, C.; Yang, S.; Li, X.M.; Li, X.; Feng, J.; Pan, A.; Wang, W.; Xu, Z.; Bai, X. Optically Manipulated Nanomechanics of Semiconductor Nanowires. Chin. Phys. B 2019, 28, 054204. [Google Scholar] [CrossRef]
- Wang, H.-C.; Li, Z.-P. Advances in Surface-Enhanced Optical Forces and Optical Manipulations. Acta Phys. Sin. 2019, 68, 144101. [Google Scholar] [CrossRef]
- Li, S.; Li, H.-Z.; Xu, J.-P.; Zhu, C.-J.; Yang, Y.-P. Squeezed Property of Optical Transistor Based on Cavity Optomechanical System. Acta Phys. Sin. 2019, 68, 174202. [Google Scholar] [CrossRef]
- Gu, K.-H.; Yan, D.; Zhang, M.-L.; Yin, J.-Z.; Fu, C.-B. Quantum Control of Fast/Slow Light in Atom-Assisted Optomechanical Cavity. Acta Phys. Sin. 2019, 68, 054201. [Google Scholar] [CrossRef]
- Zhang, X.-L.; Bao, Q.-Q.; Yang, M.-Z.; Tian, X.-S. Entanglement Characteristics of Output Optical Fields in Double-Cavity Optomechanics. Acta Phys. Sin. 2018, 67, 104203. [Google Scholar] [CrossRef]
- Gao, D.; Shi, R.; Huang, Y.; Gao, L. Fano-Enhanced Pulling and Pushing Optical Force on Active Plasmonic Nanoparticles. Phys. Rev. A 2017, 96, 043826. [Google Scholar] [CrossRef] [Green Version]
- Bian, X.; Gao, D.L.; Gao, L. Tailoring Optical Pulling Force on Gain Coated Nanoparticles with Nonlocal Effective Medium Theory. Opt. Express 2017, 25, 24566. [Google Scholar] [CrossRef]
- Chen, H.; Liu, S.; Zi, J.; Lin, Z. Fano Resonance-Induced Negative Optical Scattering Force on Plasmonic Nanoparticles. ACS Nano 2015, 9, 1926–1935. [Google Scholar] [CrossRef]
- Sukhov, S.; Dogariu, A. Negative Nonconservative Forces: Optical “Tractor Beams” for Arbitrary Objects. Phys. Rev. Lett. 2011, 107, 203602. [Google Scholar] [CrossRef]
- Novitsky, A.; Qiu, C.-W.; Lavrinenko, A. Material-Independent and Size-Independent Tractor Beams for Dipole Objects. Phys. Rev. Lett. 2012, 109, 023902. [Google Scholar] [CrossRef] [Green Version]
- Brzobohatý, O.; Karásek, V.; Šiler, M.; Chvátal, L.; Čižmár, T.; Zemánek, P. Experimental Demonstration of Optical Transport, Sorting and Self-Arrangement Using a ‘Tractor Beam’. Nat. Photonics 2013, 7, 123–127. [Google Scholar] [CrossRef] [Green Version]
- Salandrino, A.; Christodoulides, D.N. Reverse Optical Forces in Negative Index Dielectric Waveguide Arrays. Opt. Lett. 2011, 36, 3103. [Google Scholar] [CrossRef] [PubMed]
- Kajorndejnukul, V.; Ding, W.; Sukhov, S.; Qiu, C.-W.; Dogariu, A. Linear Momentum Increase and Negative Optical Forces at Dielectric Interface. Nat. Photonics 2013, 7, 787–790. [Google Scholar] [CrossRef] [Green Version]
- Petrov, M.I.; Sukhov, S.V.; Bogdanov, A.A.; Shalin, A.S.; Dogariu, A. Surface Plasmon Polariton Assisted Optical Pulling Force: Surface Plasmon Polariton Assisted Optical Pulling Force. Laser Photonics Rev. 2016, 10, 116–122. [Google Scholar] [CrossRef] [Green Version]
- Alaee, R.; Christensen, J.; Kadic, M. Optical Pulling and Pushing Forces in Bilayer PT-Symmetric Structures. Phys. Rev. Appl. 2018, 9, 014007. [Google Scholar] [CrossRef] [Green Version]
- Veltri, A.; Chipouline, A.; Aradian, A. Multipolar, Time-Dynamical Model for the Loss Compensation and Lasing of a Spherical Plasmonic Nanoparticle Spaser Immersed in an Active Gain Medium. Sci. Rep. 2016, 6, 33018. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Caligiuri, V.; Pezzi, L.; Veltri, A.; De Luca, A. Resonant Gain Singularities in 1D and 3D Metal/Dielectric Multilayered Nanostructures. ACS Nano 2017, 11, 1012–1025. [Google Scholar] [CrossRef]
- Pezzi, L.; Iatì, M.A.; Saija, R.; De Luca, A.; Maragò, O.M. Resonant Coupling and Gain Singularities in Metal/Dielectric Multishells: Quasi-Static Versus T-Matrix Calculations. J. Phys. Chem. C 2019, 123, 29291–29297. [Google Scholar] [CrossRef]
- Polimeno, P.; Patti, F.; Infusino, M.; Sánchez, J.; Iatì, M.A.; Saija, R.; Volpe, G.; Maragò, O.M.; Veltri, A. Gain-Assisted Optomechanical Position Locking of Metal/Dielectric Nanoshells in Optical Potentials. ACS Photonics 2020, 7, 1262–1270. [Google Scholar] [CrossRef]
- Huang, J.P.; Yu, K.W.; Gu, G.Q.; Karttunen, M. Electrorotation in Graded Colloidal Suspensions. Phys. Rev. E 2003, 67, 051405. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gao, L.; Huang, J.P.; Yu, K.W. Effective Nonlinear Optical Properties of Composite Media of Graded Spherical Particles. Phys. Rev. B 2004, 69, 075105. [Google Scholar] [CrossRef] [Green Version]
- Gao, L.; Yu, K.W. Second- and Third-Harmonic Generation in Random Composites of Graded Spherical Particles. Phys. Rev. B 2005, 72, 075111. [Google Scholar] [CrossRef]
- Huang, J.P.; Yu, K.W. Effective Nonlinear Optical Properties of Graded Metal-Dielectric Composite Films of Anisotropic Particles. J. Opt. Soc. Am. B 2005, 22, 1640. [Google Scholar] [CrossRef]
- Wei, E.-B.; Sun, L.; Yu, K.-W. Controlling Electric Field Distribution by Graded Spherical Core-Shell Metamaterials. Chin. Phys. B 2010, 19, 107802. [Google Scholar] [CrossRef]
- Huang, J.P.; Hui, P.M.; Yu, K.W. Second-Harmonic Generation in Graded Metal-Dielectric Films of Anisotropic Particles. Phys. Lett. A 2005, 342, 484–490. [Google Scholar] [CrossRef]
- Espinosa, D.H.G.; Oliveira, C.L.P.; Figueiredo Neto, A.M. Influence of an External Magnetic Field in the Two-Photon Absorption Coefficient of Magnetite Nanoparticles in Colloids and Thin Films. J. Opt. Soc. Am. B 2018, 35, 346. [Google Scholar] [CrossRef]
- Veltri, A.; Aradian, A. Optical Response of a Metallic Nanoparticle Immersed in a Medium with Optical Gain. Phys. Rev. B 2012, 85, 115429. [Google Scholar] [CrossRef]
- Huang, J.; Yu, K. Enhanced Nonlinear Optical Responses of Materials: Composite Effects. Phys. Rep. 2006, 431, 87–172. [Google Scholar] [CrossRef] [Green Version]
- Strangi, G.; De Luca, A.; Ravaine, S.; Ferrie, M.; Bartolino, R. Gain Induced Optical Transparency in Metamaterials. Appl. Phys. Lett. 2011, 98, 251912. [Google Scholar] [CrossRef]
- Gao, L.; Wan, J.T.K.; Yu, K.W.; Li, Z.Y. Effects of Highly Conducting Interface and Particle Size Distribution on Optical Nonlinearity in Granular Composites. J. Appl. Phys. 2000, 88, 1893–1899. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, J.J.; Gao, L. Antiboding and Bonding Lasing Modes with Low Gain Threshold in Nonlocal Metallic Nanoshell. Opt. Express 2015, 23, 8818–8828. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Huang, Y.; Ma, P.; Gao, L. Gain-Assisted Optical Pulling Force on Plasmonic Graded Nano-Shell with Equivalent Medium Theory. Physics 2021, 3, 955-967. https://doi.org/10.3390/physics3040060
Wu Y, Huang Y, Ma P, Gao L. Gain-Assisted Optical Pulling Force on Plasmonic Graded Nano-Shell with Equivalent Medium Theory. Physics. 2021; 3(4):955-967. https://doi.org/10.3390/physics3040060
Chicago/Turabian StyleWu, Yamin, Yang Huang, Pujuan Ma, and Lei Gao. 2021. "Gain-Assisted Optical Pulling Force on Plasmonic Graded Nano-Shell with Equivalent Medium Theory" Physics 3, no. 4: 955-967. https://doi.org/10.3390/physics3040060
APA StyleWu, Y., Huang, Y., Ma, P., & Gao, L. (2021). Gain-Assisted Optical Pulling Force on Plasmonic Graded Nano-Shell with Equivalent Medium Theory. Physics, 3(4), 955-967. https://doi.org/10.3390/physics3040060