Efficient Forced Response Computations of Acoustical Systems with a State-Space Approach
Abstract
:1. Introduction
2. Methods
2.1. State-Space Descriptions
2.2. Generalized ERA
2.3. Forced Response Computations
3. Example
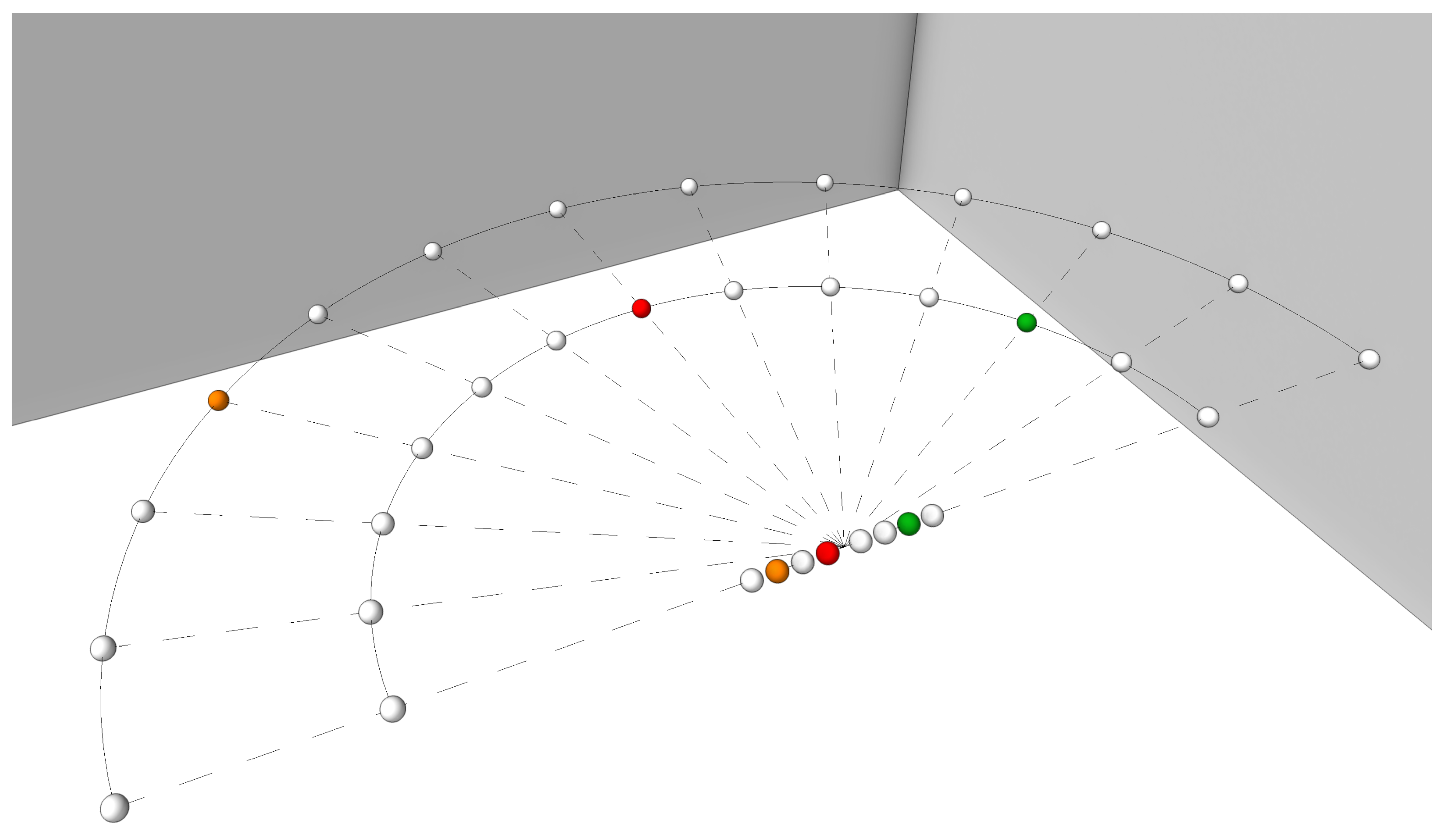
3.1. Database
3.2. Preprocessing
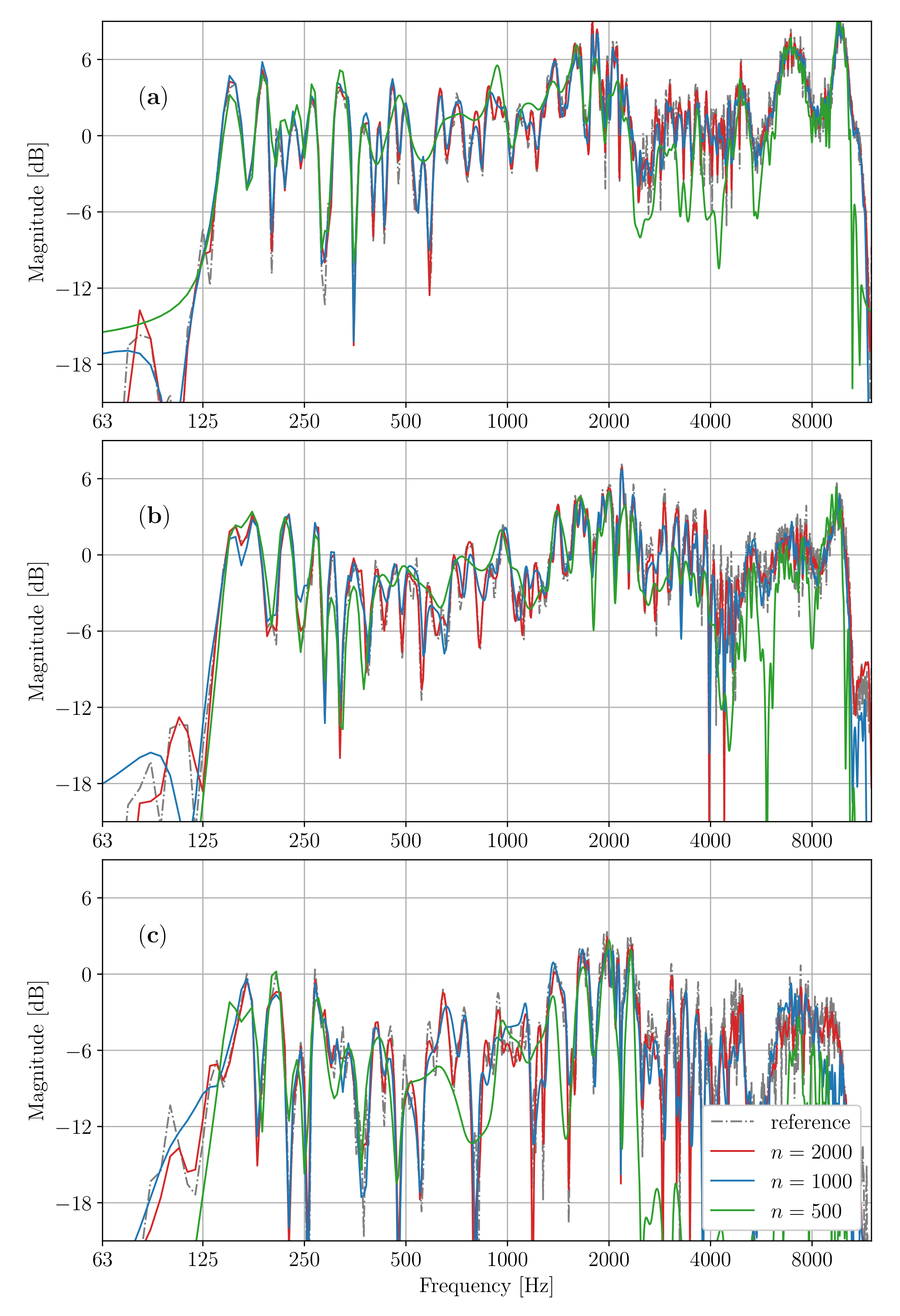
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| DFT | Discrete Fourier Transform |
| ERA | Eigensystem Realization Algorithm |
| FEM | Finite Element Method |
| FFT | Fast Fourier Transform |
| HRTF | Head-related Transfer Function |
| LTI | Linear Time-invariant |
| MOR | Model Order Reduction |
| RIR | Room Impulse Response |
| SSID | Subspace System Identification |
| SVD | Singular Value Decomposition |
References
- Antoulas, A.C. Approximation of Large-Scale Dynamical Systems; Advances in Design and Control; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2005. [Google Scholar]
- Gugercin, S.; Antoulas, A.C. A Survey of Model Reduction by Balanced Truncation and Some New Results. Int. J. Control 2004, 77, 748–766. [Google Scholar] [CrossRef]
- Zhou, K.; Doyle, J.C.; Glover, K. Robust and Optimal Control; Prentice Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Van Ophem, S.; Atak, O.; Deckers, E.; Desmet, W. Application of a Time-Stable Model Order Reduction Scheme to an Exterior Vibro-Acoustic Finite Element Model. In Proceedings of the ISMA 2016, Leuven, Belgium, 19–21 September 2016; p. 14. [Google Scholar]
- Van de Walle, A.; Shiozawa, Y.; Matsuda, H.; Desmet, W. Model Order Reduction for the Transient Vibro-Acoustic Simulation of Acoustic Guitars. In Proceedings of the ISMA 2016, Leuven, Belgium, 19–21 September 2016; p. 12. [Google Scholar]
- Van de Walle, A.; Naets, F.; Deckers, E.; Desmet, W. Stability-Preserving Model Order Reduction for Time-Domain Simulation of Vibro-Acoustic FE Models. Int. J. Numer. Meth. Eng. 2017, 109, 889–912. [Google Scholar] [CrossRef]
- Van Ophem, S.; Deckers, E.; Desmet, W. Parametric Model Order Reduction without a Priori Sampling for Low Rank Changes in Vibro-Acoustic Systems. Mech. Syst. Signal Process. 2019, 130, 597–609. [Google Scholar] [CrossRef]
- Brunton, S.L.; Dawson, S.T.; Rowley, C.W. State-Space Model Identification and Feedback Control of Unsteady Aerodynamic Forces. J. Fluids Struct. 2014, 50, 253–270. [Google Scholar] [CrossRef] [Green Version]
- Ma, Z.; Ahuja, S.; Rowley, C.W. Reduced-Order Models for Control of Fluids Using the Eigensystem Realization Algorithm. Theor. Comput. Fluid Dyn. 2011, 25, 233–247. [Google Scholar] [CrossRef] [Green Version]
- Mangesius, H.; Polifke, W. A Discrete-Time, State-Space Approach for the Investigation of Non-Normal Effects in Thermoacoustic Systems. Int. J. Spray Combust. Dyn. 2011, 3, 331–350. [Google Scholar] [CrossRef]
- Illingworth, S.J. Acoustic State-Models Using a Wave Based Approach. In Proceedings of the 21st International Congress on Sound and Vibration 2014, ICSV 2014, Beijing, China, 13–17 July 2014; p. 8. [Google Scholar]
- Meindl, M.; Emmert, T.; Polifke, W. Efficient Calculation of Thermoacoustic Modes Utilizing State-Space Models. In Proceedings of the 23rd International Congress on Sound and Vibration, ICSV23, Athens, Greece, 10–14 July 2016; p. 9. [Google Scholar]
- De Oliveira, L.P.; Varoto, P.S.; Sas, P.; Desmet, W. A State-Space Modeling Approach for Active Structural Acoustic Control. Shock Vib. 2009, 16, 607–621. [Google Scholar] [CrossRef]
- Hull, A.J.; Radcliffe, C.J.; Southward, S.C. Global Active Noise Control of a One-Dimensional Acoustic Duct Using a Feedback Controller. J. Dyn. Syst. Meas. Control 1993, 115, 488–494. [Google Scholar] [CrossRef]
- Hong, J.; Akers, J.; Venugopal, R.; Lee, M.-N.; Sparks, A.; Washabaugh, P.; Bernstein, D. Modeling, Identification, and Feedback Control of Noise in an Acoustic Duct. IEEE Trans. Contr. Syst. Technol. 1996, 4, 283–291. [Google Scholar] [CrossRef] [Green Version]
- Petersen, C.D.; Fraanje, R.; Cazzolato, B.S.; Zander, A.C.; Hansen, C.H. A Kalman Filter Approach to Virtual Sensing for Active Noise Control. Mech. Syst. Signal Process. 2008, 22, 490–508. [Google Scholar] [CrossRef] [Green Version]
- Nijsse, G.; Verhaegen, M.; Schutter, B.D.; Westwick, D.; Doelman, N. State Space Modeling in Multichannel Active Control Systems. In Proceedings of the 1999 International Symposium on Active Control of Sound and Vibration, Ft. Lauderdale, FL, USA, 2–4 December 1999; Institute of Noise Control Engineering of the USA: Saddle River, NJ, USA, 1999; pp. 909–920. [Google Scholar]
- Georgiou, P.; Kyriakakis, C. Modeling of Head Related Transfer Functions for Immersive Audio Using a State-Space Approach. In Proceedings of the Conference Record of the Thirty-Third Asilomar Conference on Signals, Systems, and Computers (Cat. No.CH37020), Pacific Grove, CA, USA, 24–27 October 1999; Volume 1, pp. 720–724. [Google Scholar] [CrossRef] [Green Version]
- Georgiou, P.; Kyriakakis, C. A Multiple Input Single Output Model for Rendering Virtual Sound Sources in Real Time. In Proceedings of the 2000 IEEE International Conference on Multimedia and Expo, ICME2000, New York, NY, USA, 30 July–2 August 2000; Proceedings. Latest Advances in the Fast Changing World of Multimedia (Cat. No.00TH8532). Volume 1, pp. 253–256. [Google Scholar] [CrossRef]
- Adams, N.; Wakefield, G. Efficient Binaural Display Using MIMO State-Space Systems. In Proceedings of the 2007 IEEE International Conference on Acoustics, Speech and Signal Processing, ICASSP’07, Honolulu, HI, USA, 15–20 April 2007; Volume 1, pp. I-169–I-172. [Google Scholar] [CrossRef]
- Adams, N.H.; Wakefield, G.H. State-Space Synthesis of Virtual Auditory Space. IEEE Trans. Audio Speech Lang. Process. 2008, 16, 881–890. [Google Scholar] [CrossRef]
- Juang, J.N.; Pappa, R.S. An Eigensystem Realization Algorithm for Modal Parameter Identification and Model Reduction. J. Guid. Control Dyn. 1985, 8, 620–627. [Google Scholar] [CrossRef]
- Kung, S. A New Identification and Model Reduction Algorithm via Singular Value Decomposition. In Proceedings of the 12th Asilomar Conference on Circuits, Systems and Computers, Pacific Grove, CA, USA, 6–8 November 1978; pp. 705–714. [Google Scholar]
- Verhaegen, M.; Dewilde, P. Subspace Model Identification Part 1. The Output-Error State-Space Model Identification Class of Algorithms. Int. J. Control 1992, 56, 1187–1210. [Google Scholar] [CrossRef]
- Van Overschee, P.; de Moor, B. N4SID: Numerical Algorithms for State Space Subspace System Identification. IFAC Proc. Vol. 1993, 26, 55–58. [Google Scholar] [CrossRef]
- Golub, G.H.; Van Loan, C.F. Matrix Computations, 4th ed.; Johns Hopkins Studies in the Mathematical Sciences; The Johns Hopkins University Press: Baltimore, MD, USA, 2013. [Google Scholar]
- Kramer, B.; Gorodetsky, A.A. System Identification via CUR-Factored Hankel Approximation. SIAM J. Sci. Comput. 2018, 40, A848–A866. [Google Scholar] [CrossRef]
- Minster, R.; Saibaba, A.K.; Kar, J.; Chakrabortty, A. Efficient Algorithms for Eigensystem Realization Using Randomized SVD. SIAM J. Matrix Anal. Appl. 2021, 42, 1045–1072. [Google Scholar] [CrossRef]
- Friedland, S.; Mehrmann, V.; Miedlar, A.; Nkengla, M. Fast Low Rank Approximations of Matrices and Tensors. Electron. J. Linear Algebra 2011, 22, 1031–1048. [Google Scholar] [CrossRef] [Green Version]
- Halko, N.; Martinsson, P.G.; Tropp, J.A. Finding Structure with Randomness: Probabilistic Algorithms for Constructing Approximate Matrix Decompositions. SIAM Rev. 2011, 53, 217–288. [Google Scholar] [CrossRef]
- Lu, L.; Xu, W.; Qiao, S. A Fast SVD for Multilevel Block Hankel Matrices with Minimal Memory Storage. Numer. Algorithms 2015, 69, 875–891. [Google Scholar] [CrossRef]
- Van Loan, C. Computational Frameworks for the Fast Fourier Transform; Number Vol. 10 in Frontiers in Applied Mathematics; Society for Industrial and Applied Mathematics (SIAM): Philadelphia, PA, USA, 1992. [Google Scholar]
- Wefers, F. Partitioned Convolution Algorithms for Real-Time Auralization; Number Band 20 in Aachener Beiträge Zur Technischen Akustik; Logos Verlag Berlin GmbH: Berlin, Germany, 2015. [Google Scholar]
- Hadad, E.; Heese, F.; Vary, P.; Gannot, S. Multichannel Audio Database in Various Acoustic Environments. In Proceedings of the 2014 14th International Workshop on Acoustic Signal Enhancement (IWAENC), Juan-les-Pins, France, 8–11 September 2014; pp. 313–317. Available online: https://www.iks.rwth-aachen.de/en/research/tools-downloads/databases/multi-channel-impulse-response-database (accessed on 11 August 2021). [CrossRef]
- Gawronski, W.; Juang, J.N. Model Reduction in Limited Time and Frequency Intervals. Int. J. Syst. Sci. 1990, 21, 349–376. [Google Scholar] [CrossRef]
- Barry, D.; Danalis, A.; Jagode, H. Effortless Monitoring of Arithmetic Intensity with PAPI’s Counter Analysis Toolkit. In Tools for High Performance Computing 2018/2019; Mix, H., Niethammer, C., Zhou, H., Nagel, W.E., Resch, M.M., Eds.; Springer International Publishing: Cham, Switzerland, 2021; pp. 195–218. [Google Scholar] [CrossRef]
- SciPy 1.0 Contributors; Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; et al. SciPy 1.0: Fundamental Algorithms for Scientific Computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benner, P.; Werner, S.W. Frequency- and Time-Limited Balanced Truncation for Large-Scale Second-Order Systems. Linear Algebra Appl. 2021, 623, 68–103. [Google Scholar] [CrossRef]
- Akinola, R.O.; Freitag, M.A.; Spence, A. The Calculation of the Distance to a Nearby Defective Matrix. Numer. Linear Algebra Appl. 2014, 21, 403–414. [Google Scholar] [CrossRef]
- Kramer, B.; Gugercin, S. Tangential Interpolation-Based Eigensystem Realization Algorithm for MIMO Systems. Math. Comput. Model. Dyn. Syst. 2016, 22, 282–306. [Google Scholar] [CrossRef]

| Method | Computational Cost | Storage Cost |
|---|---|---|
| Convolution, time domain | ||
| Convolution, frequency domain | ||
| State-space, dense | ||
| State-space, Hessenberg | ||
| State-space, diagonal |
| Model Order | Frequency-Limited | ||
|---|---|---|---|
| 2000 | no | 1.99 | 1.50 |
| 1000 | no | 3.46 | 2.59 |
| 500 | no | 7.45 | 5.16 |
| 120 | no | 12.74 | 8.52 |
| 120 | yes | 14.09 | 2.07 |
| Method | n | C/[Mflops] | F/[Mflops] | |
|---|---|---|---|---|
| Convolution, time domain | 43,616 | 38442 | - | |
| Convolution, frequency domain | 932 | 760 | - | |
| State-space, dense | 226,387 | 227,223 | - | |
| State-space, Hessenberg | 2000 | 115,197 | 129,121 | 1 |
| State-space, quasi-diagonal | 4008 | 4567 | 1838.5 | |
| State-space, dense | 57,515 | 57,934 | - | |
| State-space, Hessenberg | 1000 | 29,760 | 33,244 | 1 |
| State-space, quasi-diagonal | 2004 | 2284 | 855 | |
| State-space, dense | 14,838 | 15,048 | - | |
| State-space, Hessenberg | 500 | 7920 | 8794 | 1 |
| State-space, quasi-diagonal | 1002 | 1143 | 100 | |
| State-space, dense | 1022 | 1074 | - | |
| State-space, Hessenberg | 120 | 631 | 684 | 1 |
| State-space, quasi-diagonal | 240 | 275 | (17.7) 12.5 | |
| , l = 24,000, , | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pelling, A.J.R.; Sarradj, E. Efficient Forced Response Computations of Acoustical Systems with a State-Space Approach. Acoustics 2021, 3, 581-593. https://doi.org/10.3390/acoustics3030037
Pelling AJR, Sarradj E. Efficient Forced Response Computations of Acoustical Systems with a State-Space Approach. Acoustics. 2021; 3(3):581-593. https://doi.org/10.3390/acoustics3030037
Chicago/Turabian StylePelling, Art J. R., and Ennes Sarradj. 2021. "Efficient Forced Response Computations of Acoustical Systems with a State-Space Approach" Acoustics 3, no. 3: 581-593. https://doi.org/10.3390/acoustics3030037
APA StylePelling, A. J. R., & Sarradj, E. (2021). Efficient Forced Response Computations of Acoustical Systems with a State-Space Approach. Acoustics, 3(3), 581-593. https://doi.org/10.3390/acoustics3030037






