Applicability of Aeroacoustic Scaling Laws of Leading Edge Serrations for Rotating Applications
Abstract
:1. Introduction
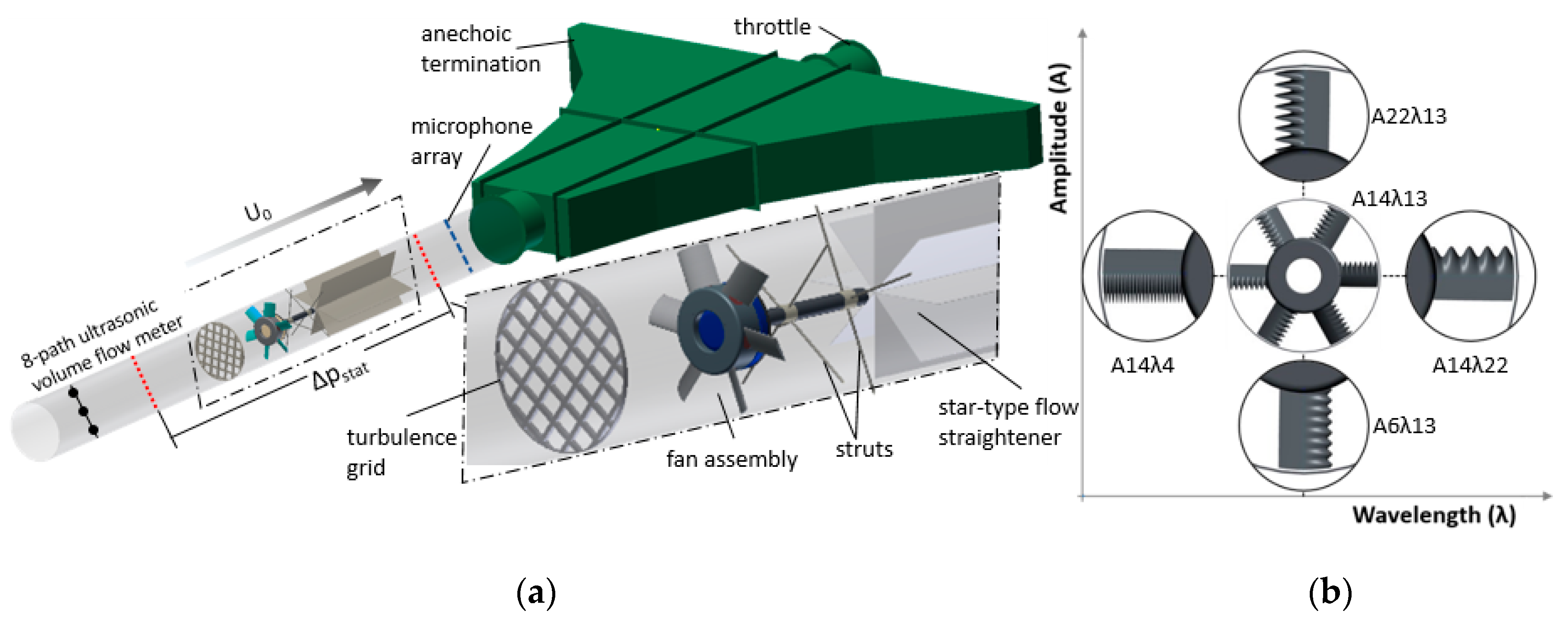
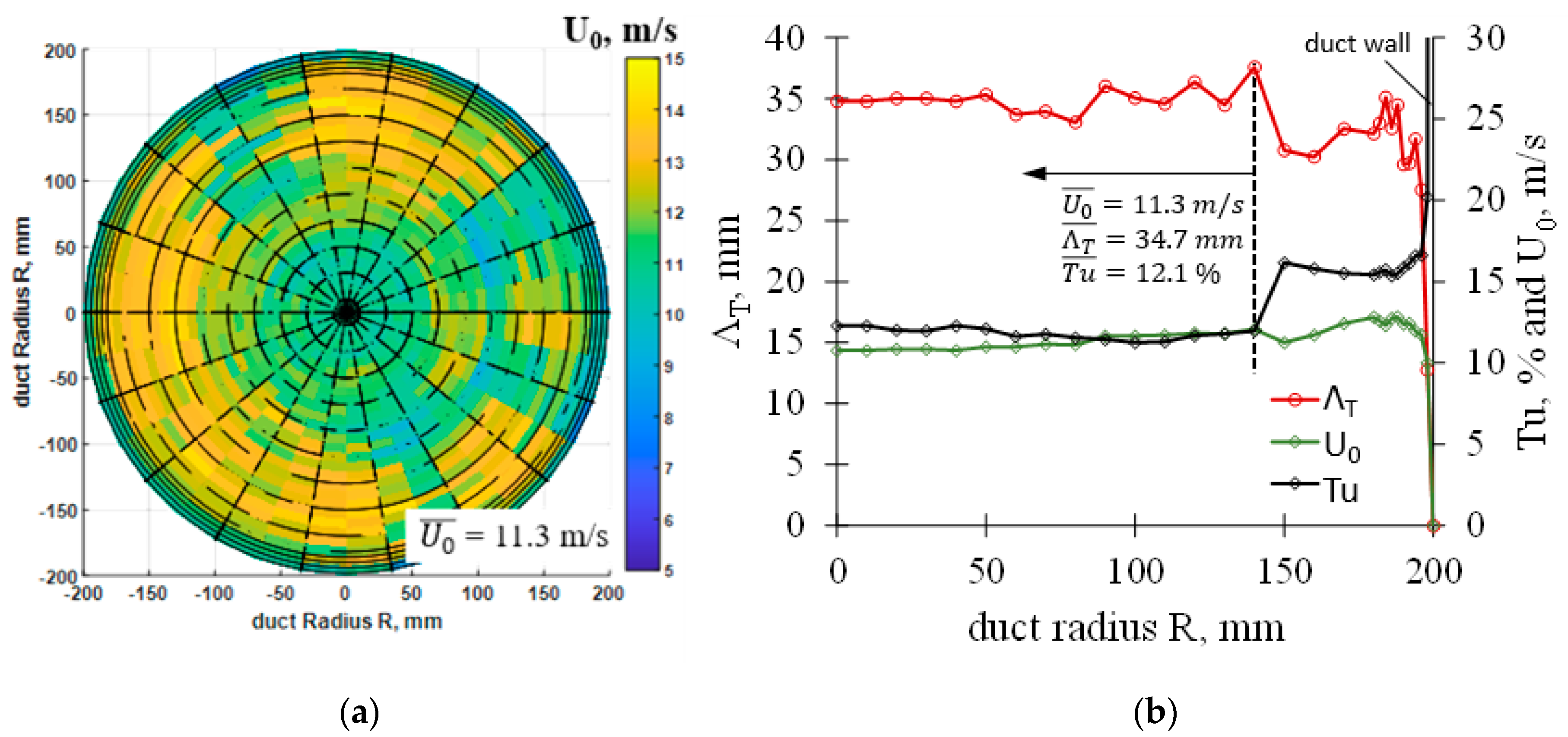
2. Materials and Methods
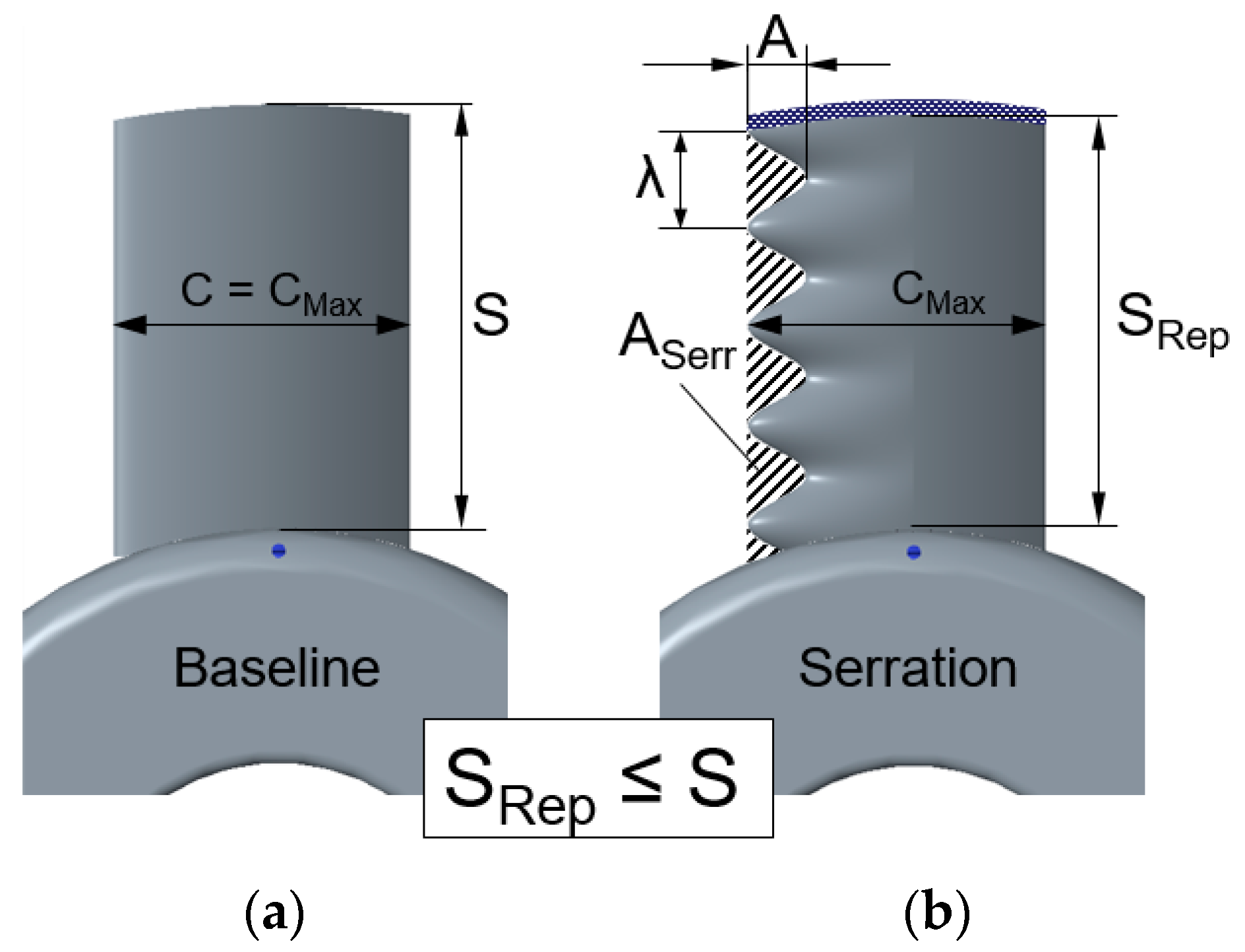
- A constant maximum blade chord results in only one baseline reference case for comparison as well as in a constant blade thickness for different serration geometries.
- Keeping constant maximum solidity σS prevents amplitude-dependent interaction effects of successive blades at solidities σS ≥ 0.7.
- Pursuing a conservative approach that remains close to practical applications, in which serrations might be included as a substituting technology at limited installation space by simply replacing previously mounted straight blades.
3. Results
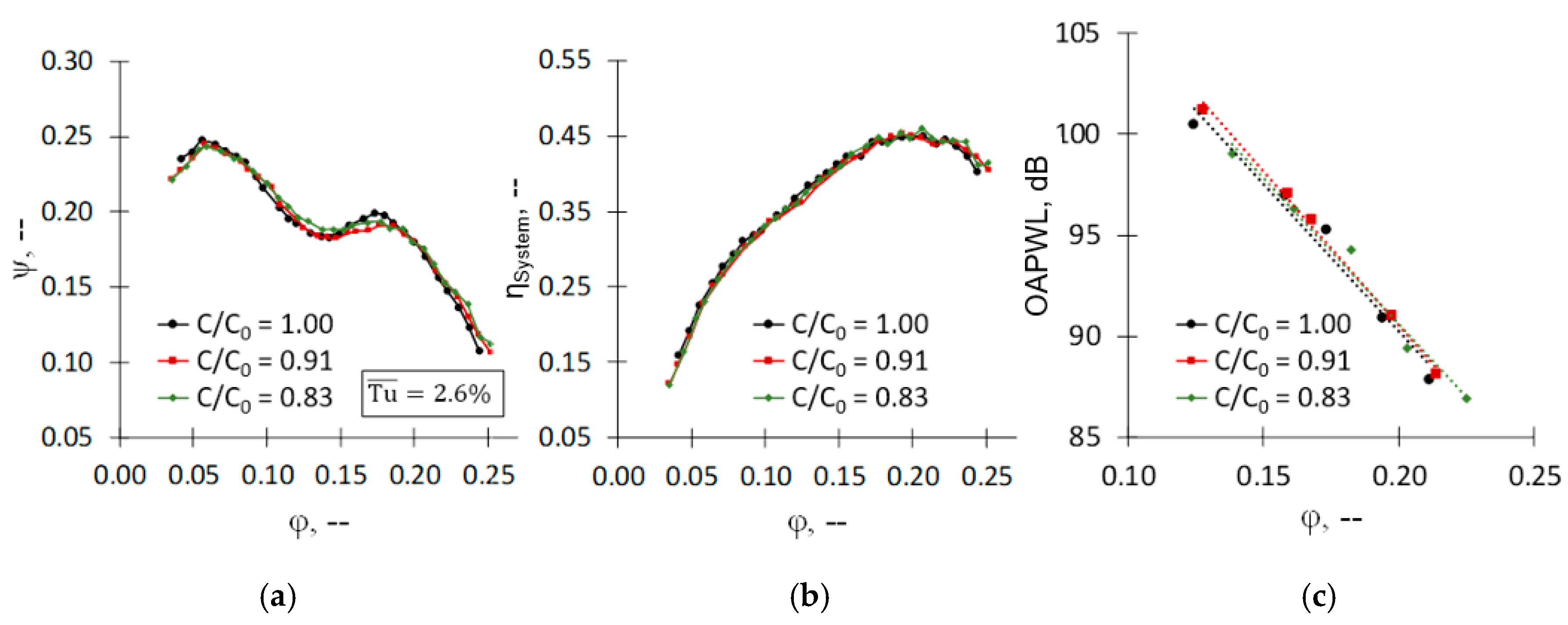
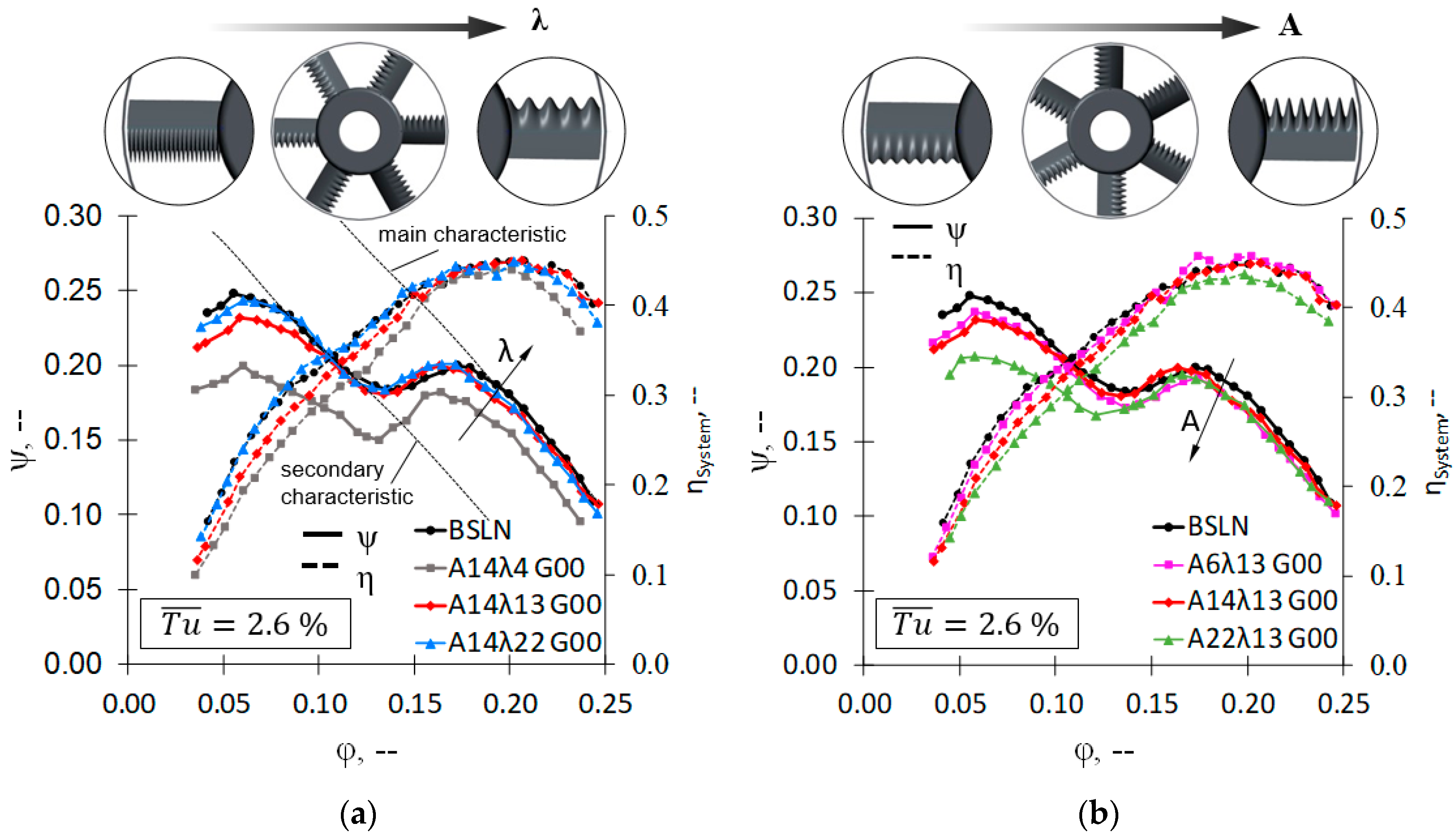
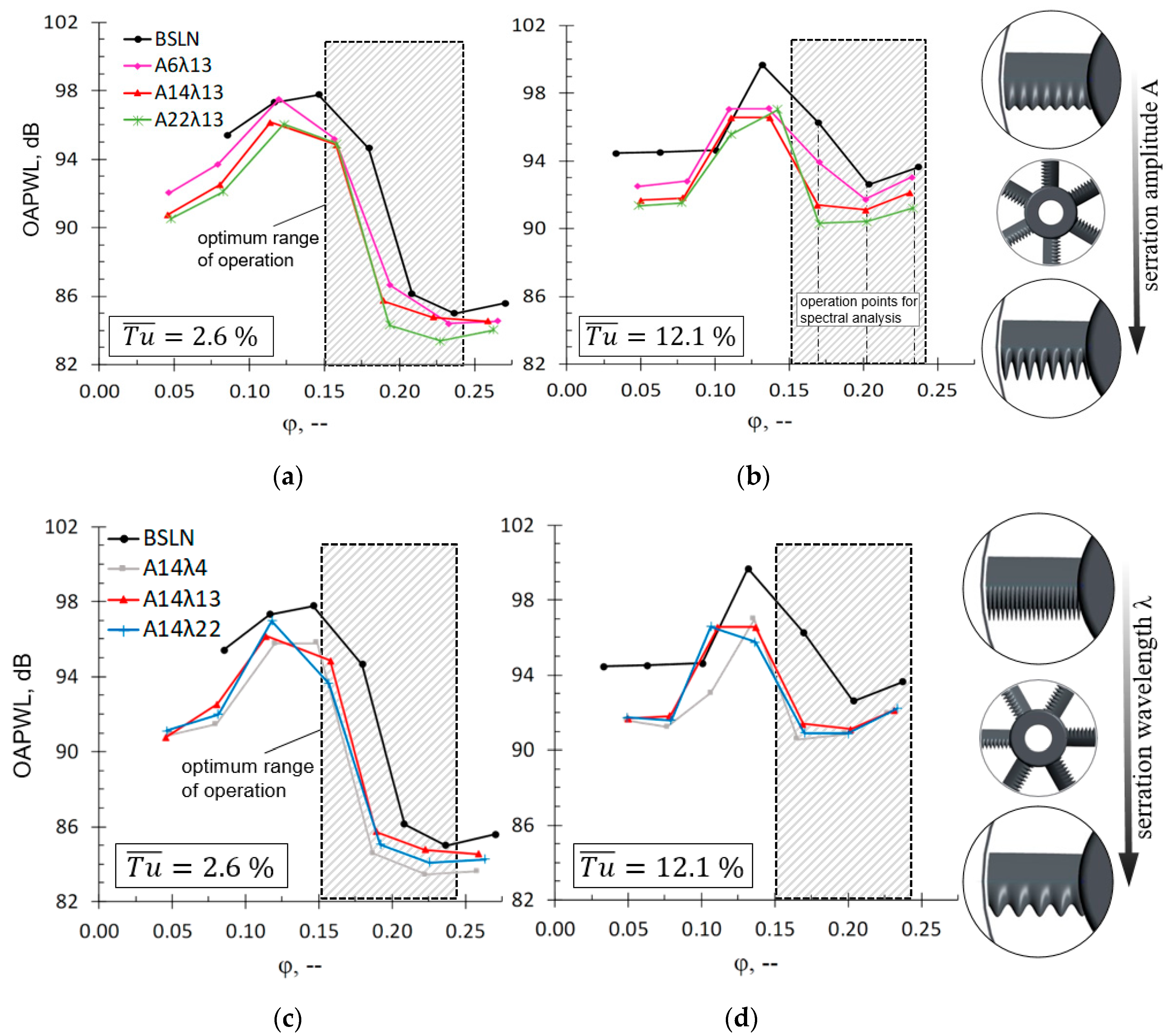
3.1. Low-Pressure Axial Fans: Aerodynamic Performance
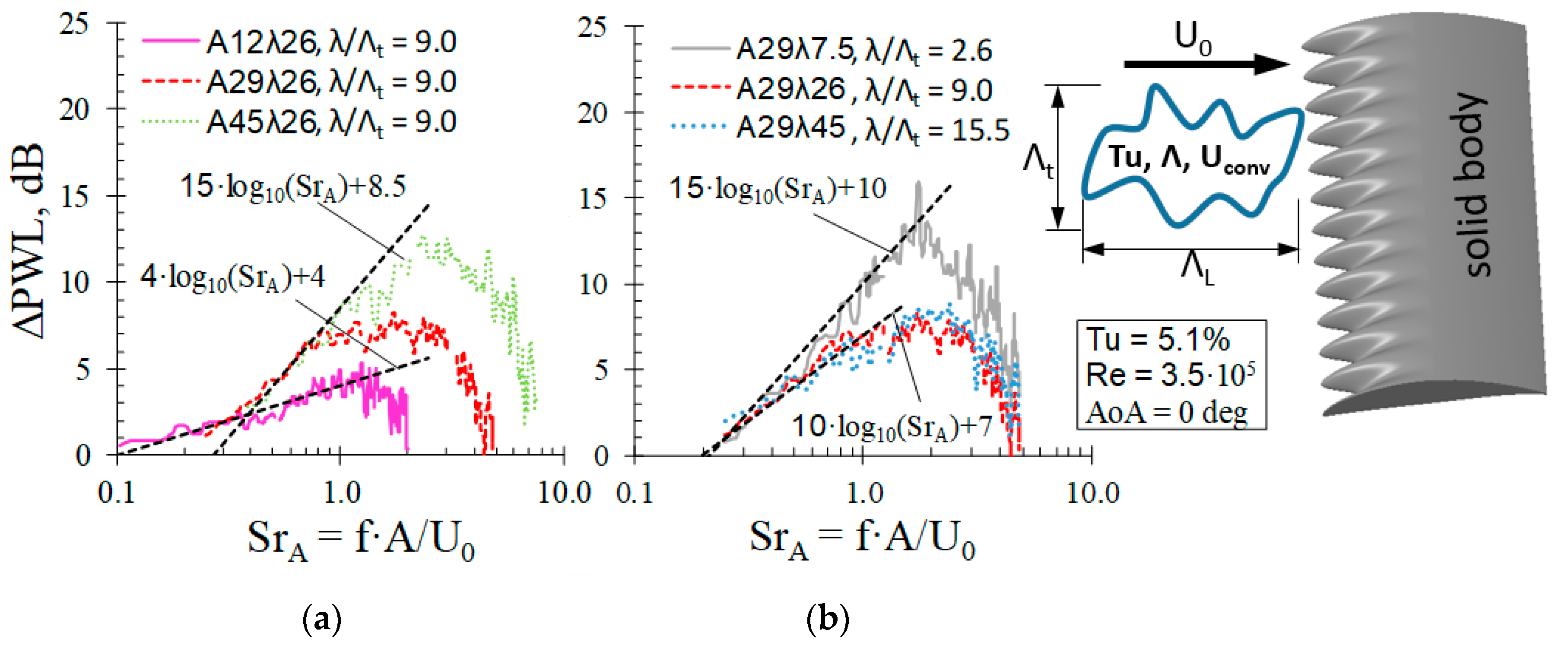
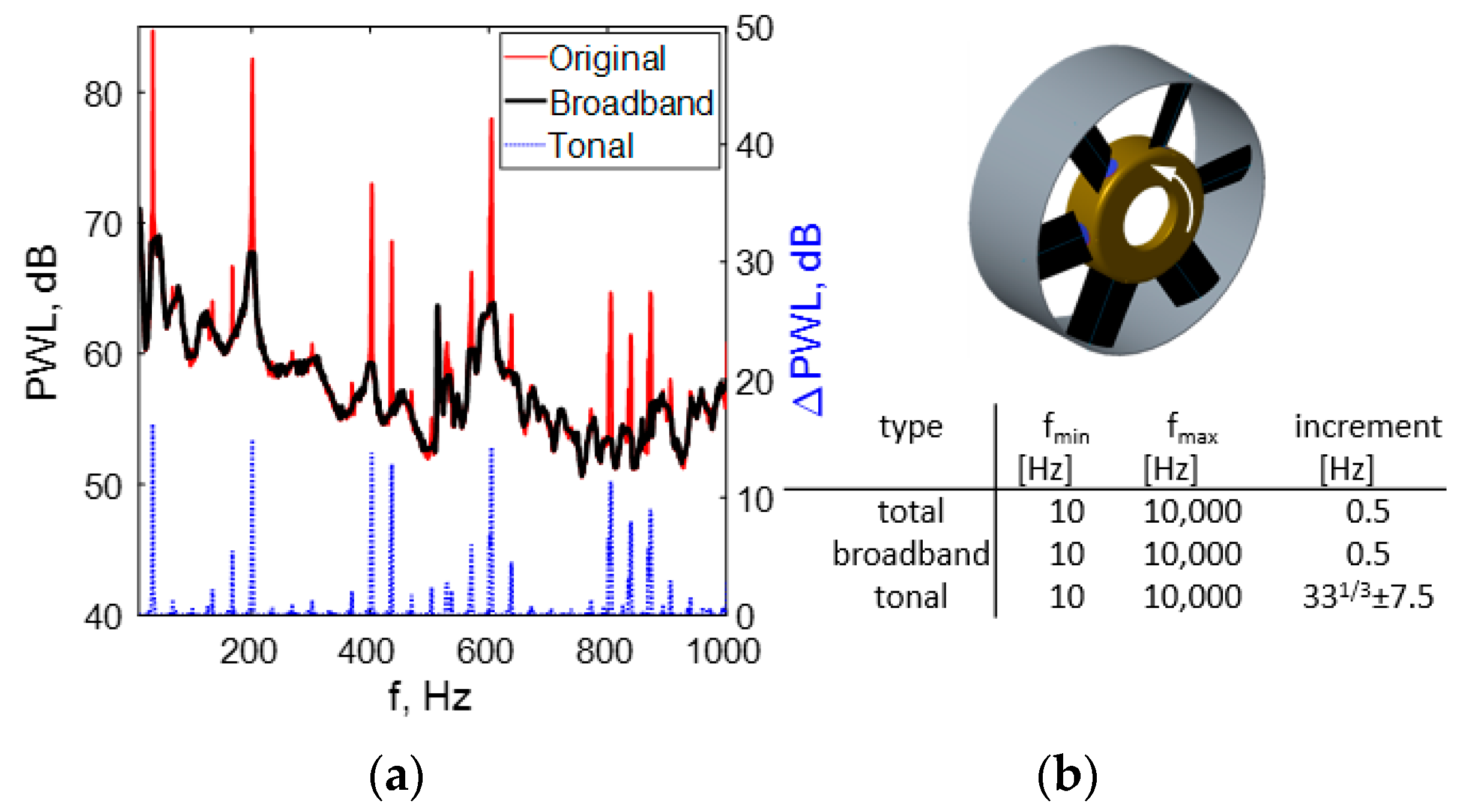
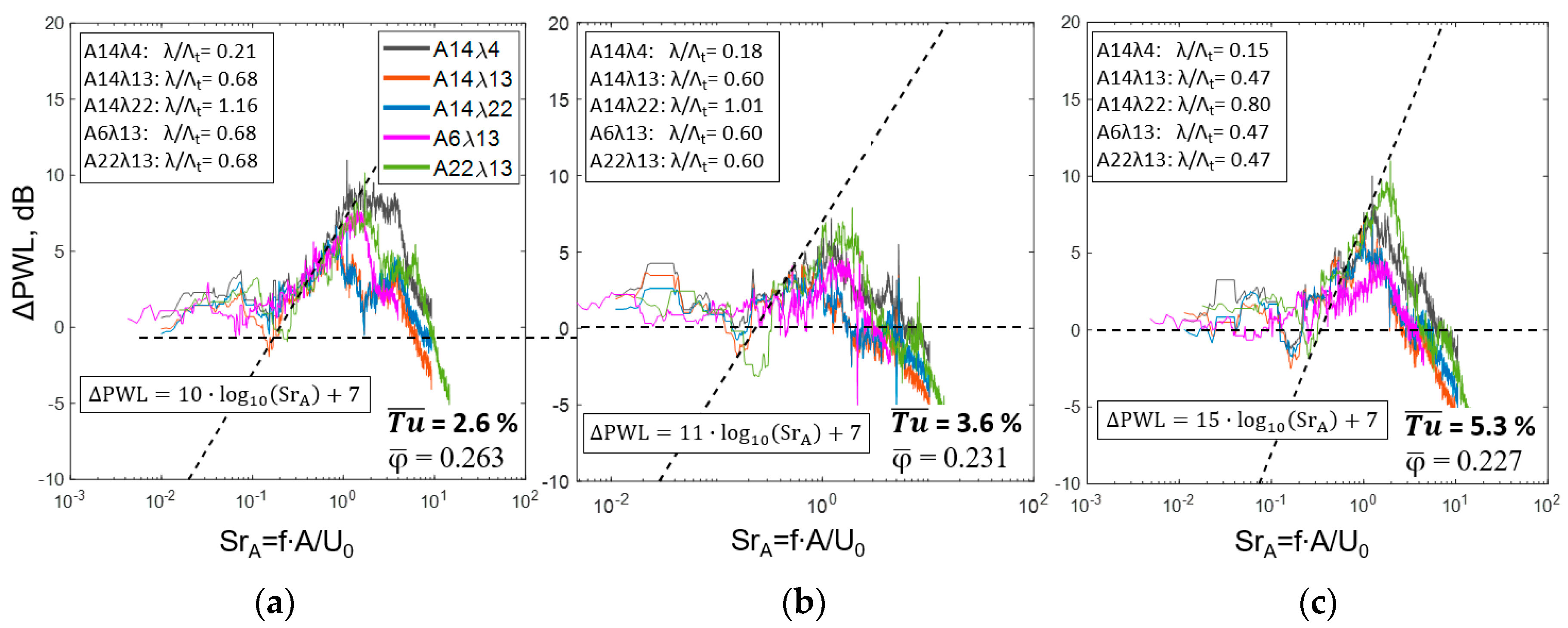
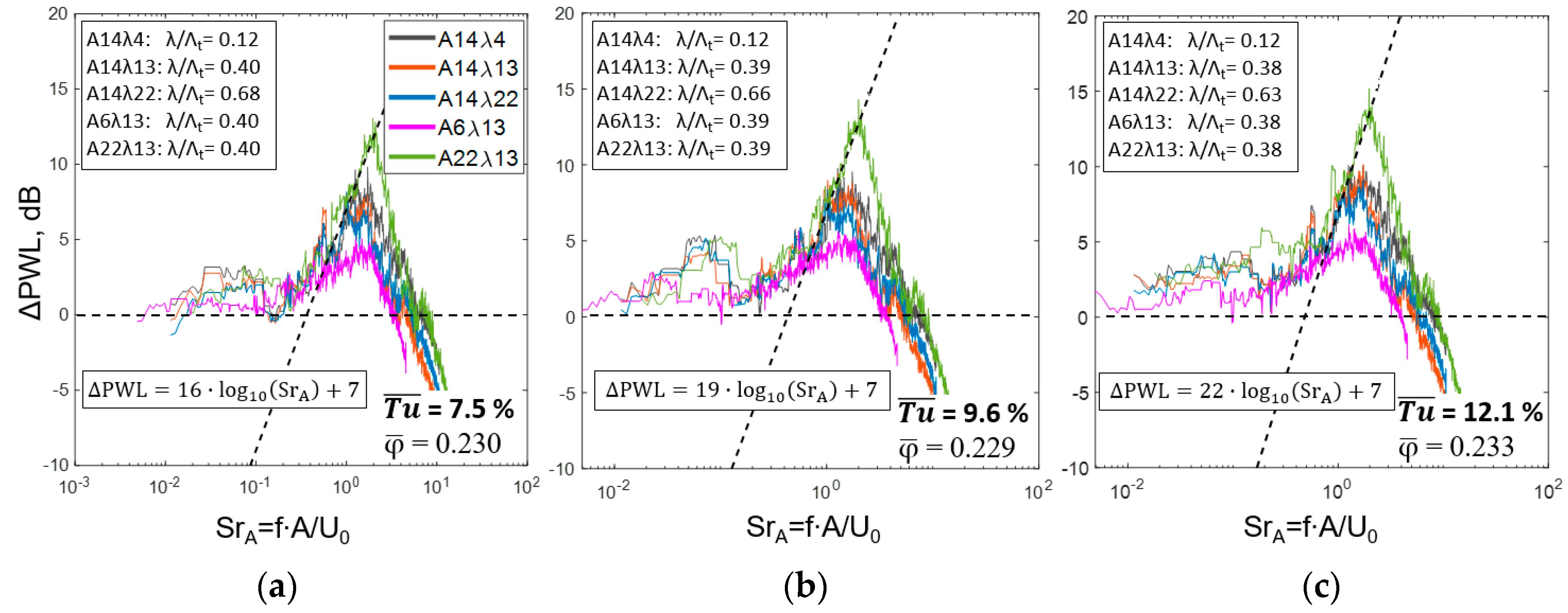
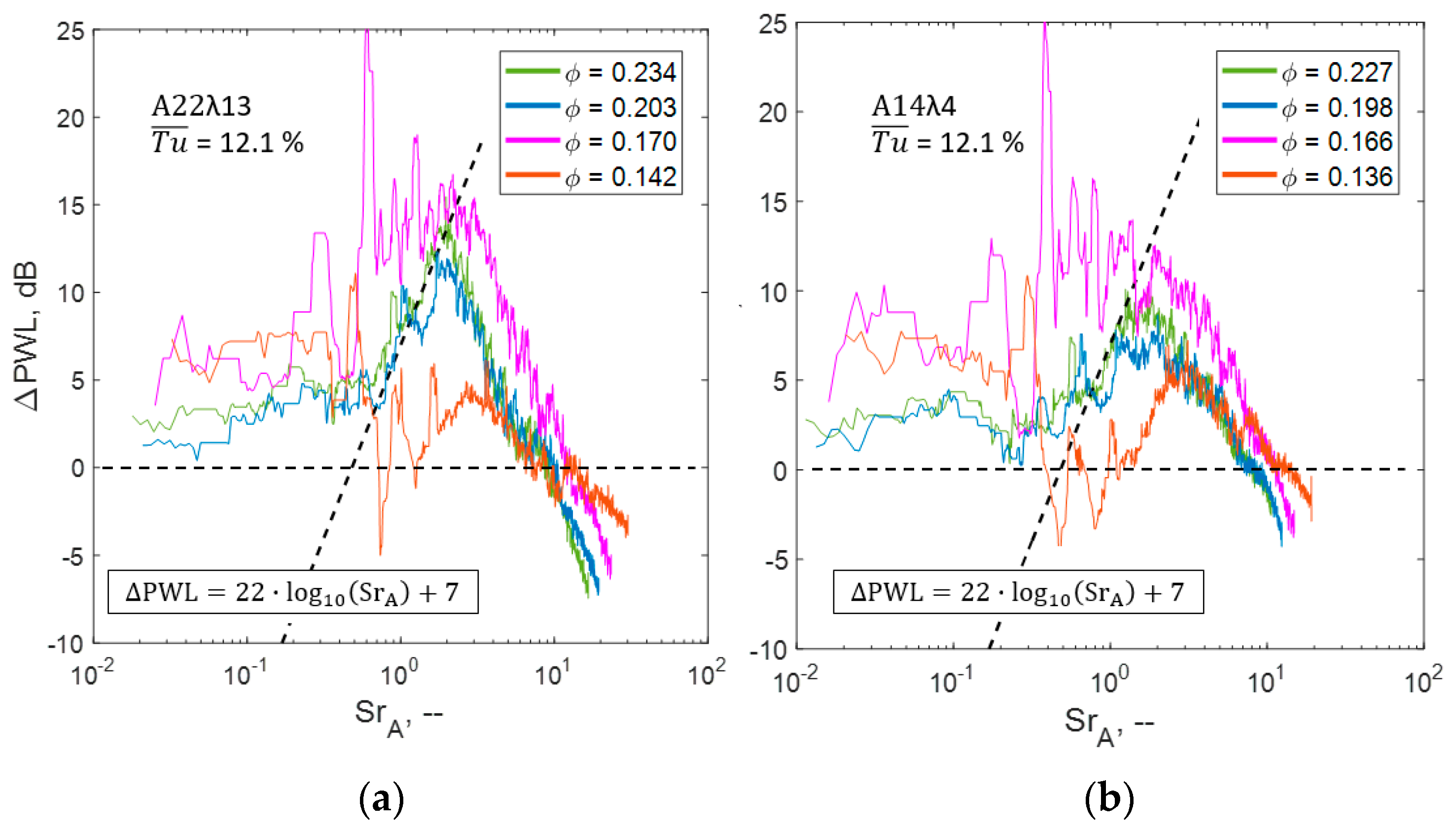
3.2. Low-Pressure Axial Fans: Spectral Broadband Noise Reduction
3.3. Low-Pressure Axial Fans: Overall Acoustic Performance
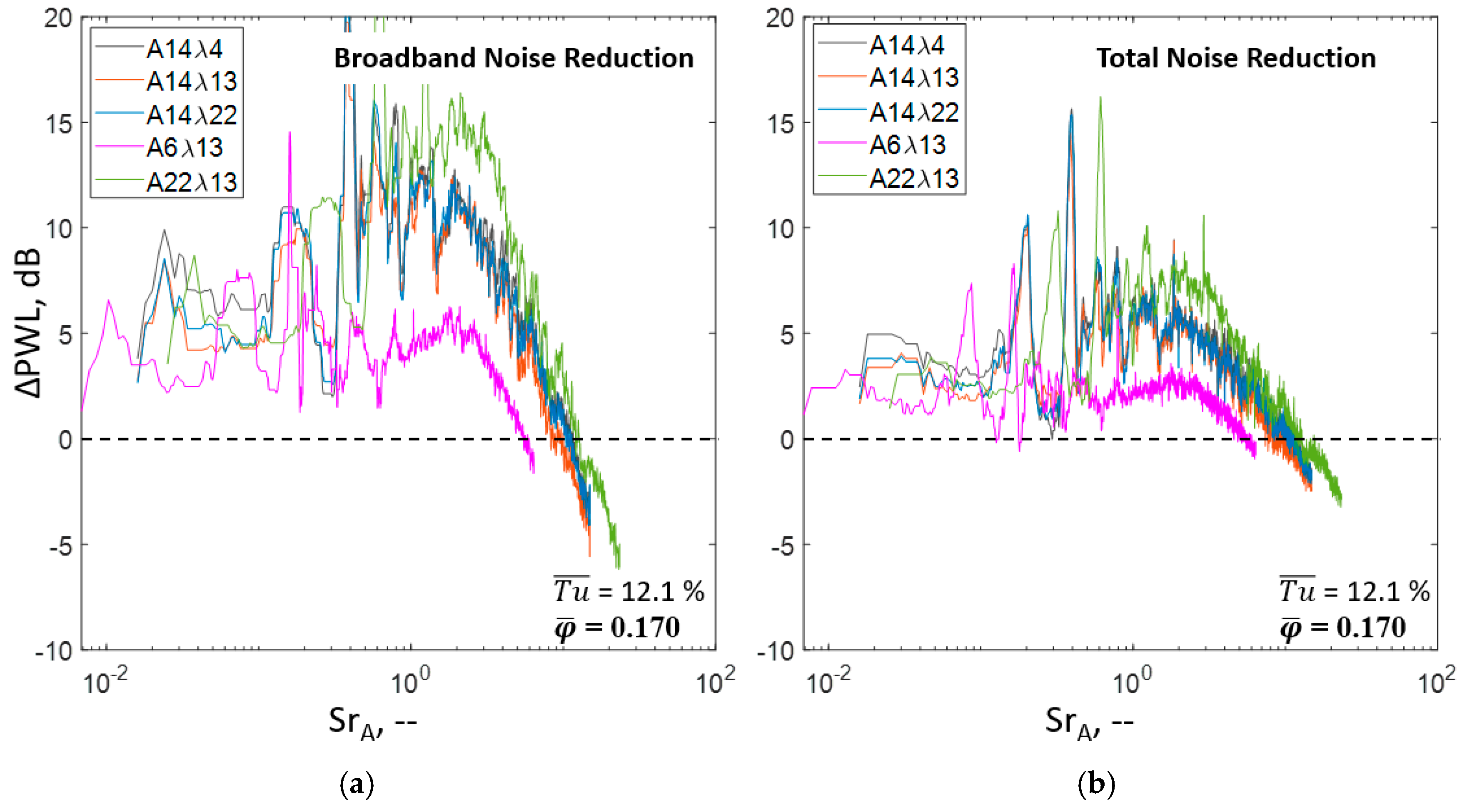
3.4. Low-Pressure Axial Fans: Broadband vs. Total Noise Reduction
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Devenport, W.J.; Staubs, J.K.; Glegg, S.A. Sound radiation from real airfoils in turbulence. J. Sound Vib. 2010, 329, 3470–3483. [Google Scholar] [CrossRef]
- Staubs, J.K. Real Airfoil Effects on Leading Edge Noise. Ph.D. Dissertation, Virginia State University, Blacksburg, WV, USA, 2008. [Google Scholar]
- Paruchuri, C.; Subramanian, N.; Joseph, P.; Vanderwel, C.; Kim, J.W.; Ganapathisubramani, B. Broadband noise reduction through leading edge serrations on realistic aerofoils. In Proceedings of the 21st AIAA/CEAS Aeroacoustics Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef]
- Chong, T.P.; Vathylakis, A.; McEwen, A.; Kemsley, F.; Muhammad, C.; Siddiqi, S. Aeroacoustic and Aerodynamic Performances of an Aerofoil Subjected to Sinusoidal Leading Edges. In Proceedings of the 21st AIAA/CEAS Aeroacoustics Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef] [Green Version]
- Narayanan, S.; Chaitanya, P.; Haeri, S.; Joseph, P.; Kim, J.W.; Polacsek, C. Airfoil noise reductions through leading edge serrations. Phys. Fluids 2015, 27, 25109. [Google Scholar] [CrossRef]
- Chaitanya, P.; Joseph, P.; Narayanan, S.; Vanderwel, C.; Turner, J.; Kim, J.W.; Ganapathisubramani, B. Performance and mechanism of sinusoidal leading edge serrations for the reduction of turbulence-aerofoil interaction noise. J. Fluid Mech. 2017, 818, 435–464. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.W.; Haeri, S.; Joseph, P.F. On the reduction of aerofoil–turbulence interaction noise associated with wavy leading edges. J. Fluid Mech. 2016, 792, 526–552. [Google Scholar] [CrossRef] [Green Version]
- Turner, J.; Kim, J.W. Towards Understanding Aerofoils with Wavy Leading Edges Interacting with Vortical Disturbances. In Proceedings of the 22st AIAA/CEAS Aeroacoustics Conference, Lyon, France, 30 May–1 June 2016. [Google Scholar] [CrossRef]
- Turner, J.M.; Kim, J.W. Aeroacoustic source mechanisms of a wavy leading edge undergoing vortical disturbances. J. Fluid Mech. 2017, 811, 582–611. [Google Scholar] [CrossRef] [Green Version]
- Lau, A.S.; Haeri, S.; Kim, J.W. The effect of wavy leading edges on aerofoil–gust interaction noise. J. Sound Vib. 2013, 332, 6234–6253. [Google Scholar] [CrossRef] [Green Version]
- Lyu, B.; Azarpeyvand, M. On the noise prediction for serrated leading edges. J. Fluid Mech. 2017, 826, 205–234. [Google Scholar] [CrossRef] [Green Version]
- Chaitanya, P.; Joseph, P.; Narayanan, S.; Kim, J.W. Aerofoil broadband noise reductions through double-wavelength leading-edge serrations: A new control concept. J. Fluid Mech. 2018, 855, 131–151. [Google Scholar] [CrossRef] [Green Version]
- Corsini, A.; Delibra, G.; Rispoli, F.; Sheard, A.G. Aeroacoustic Assessment of Leading Edge Bumps in Industrial Fans. In Proceedings of the Fan 2015 Conference, Lyon, France, 15–17 April 2015. [Google Scholar]
- Arndt, R.E.; Nagel, R.T. Effect of leading edge serrations on noise radiation from a model rotor. In Proceedings of the AIAA 5th Fluid and Plasma Dynamics Conference, Boston, MA, USA, 26–28 June 1972. [Google Scholar] [CrossRef]
- Krömer, F.; Becker, S. Experimental investigation of the sound reduction by leading edge serrations on a flat-plate axial fan. In Proceedings of the 24th AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar] [CrossRef]
- Biedermann, T.M.; Kameier, F.; Paschereit, C.O. Successive Aeroacoustic Transfer of Leading Edge Serrations from Single Airfoil to Low-Pressure Fan Application. ASME J. Eng. Gas Turbines Power 2019, 2019. [Google Scholar] [CrossRef]
- Biedermann, T.M.; Chong, T.P.; Kameier, F.; Paschereit, C.O. Statistical-Empirical Modelling of Airfoil Noise Subjected to Leading Edge Serrations. AIAA J. 2017, 55, 3128–3142. [Google Scholar] [CrossRef]
- Biedermann, T.M.; Czeckay, P.; Geyer, T.F.; Kameier, F.; Paschereit, C.O. Effect of Inflow Conditions on the Noise Reduction through Leading Edge Serrations. AIAA J. 2019, 57, 4104–4109. [Google Scholar] [CrossRef]
- Bampanis, G.; Roger, M.; Ragni, D.; Avallone, F.; Teruna, C. Airfoil-Turbulence Interaction Noise Source Identification and its Reduction by Means of Leading Edge Serrations. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019. [Google Scholar] [CrossRef]
- ISO. Acoustics—Determination of Sound Power Radiated into a Duct by Fans and Other Air-Moving Devices—In-Duct Method (ISO 5136:2003); International Organization for Standardization: Geneva, Switzerland, 2009. [Google Scholar]
- Carolus, T.H.; Starzmann, R. An Aerodynamic Design Methodology for Low Pressure Axial Fans with Integrated Airfoil Polar Prediction. In Proceedings of the 2011 ASME Turbo Expo, Vancouver, BC, Canada, 6–10 June 2011. [Google Scholar] [CrossRef]
- Biedermann, T.M.; Kameier, F.; Paschereit, C.O. Optimised Test Rig for Measurement of Aerodynamic and Aeroacoustic Performance of Leading Edge Serrations in Low-Speed Fan Application. In Proceedings of the 2018 ASME Turbo Expo, Oslo, Norway, 11–15 June 2018. [Google Scholar] [CrossRef] [Green Version]
- Biedermann, T.M. Aeroacoustic Transfer of Leading Edge Serrations from Single Aerofoils to Low-Pressure Fan Applications. Ph.D. Thesis, Technical University Berlin, Berlin, Germany, 2019. [Google Scholar] [CrossRef]
- Laws, E.M.; Livesey, J.L. Flow through Screens. Annu. Rev. Fluid Mech. 1978, 10, 247–266. [Google Scholar] [CrossRef]
- Nakano, T. A theory of homogeneous, isotropic turbulence of incompressible fluids. Ann. Phys. 1972, 73, 326–371. [Google Scholar] [CrossRef]
- Neise, W. Lärm und Lärmbekämpfung Bei Ventilatoren—Eine Bestandsaufnahme; DFVLR Forschungsbericht 80-16: Berlin, Germany, 1980. [Google Scholar]
- Hansen, K.L.; Rostamzadeh, N.; Kelso, R.M.; Dally, B.B. Evolution of the streamwise vortices generated between leading edge tubercles. J. Fluid Mech. 2016, 788, 730–766. [Google Scholar] [CrossRef]
- Chong, T.P.; Biedermann, T.; Koster, O.; Hasheminejad, S.M. On the Effect of Leading Edge Serrations on Aerofoil Noise Production. In Proceedings of the 24th AIAA/CEAS Aeroacoustics Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar] [CrossRef] [Green Version]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Biedermann, T.M.; Czeckay, P.; Hintzen, N.; Kameier, F.; Paschereit, C.O. Applicability of Aeroacoustic Scaling Laws of Leading Edge Serrations for Rotating Applications. Acoustics 2020, 2, 579-594. https://doi.org/10.3390/acoustics2030030
Biedermann TM, Czeckay P, Hintzen N, Kameier F, Paschereit CO. Applicability of Aeroacoustic Scaling Laws of Leading Edge Serrations for Rotating Applications. Acoustics. 2020; 2(3):579-594. https://doi.org/10.3390/acoustics2030030
Chicago/Turabian StyleBiedermann, Till M., Pasquale Czeckay, Nils Hintzen, Frank Kameier, and C. O. Paschereit. 2020. "Applicability of Aeroacoustic Scaling Laws of Leading Edge Serrations for Rotating Applications" Acoustics 2, no. 3: 579-594. https://doi.org/10.3390/acoustics2030030
APA StyleBiedermann, T. M., Czeckay, P., Hintzen, N., Kameier, F., & Paschereit, C. O. (2020). Applicability of Aeroacoustic Scaling Laws of Leading Edge Serrations for Rotating Applications. Acoustics, 2(3), 579-594. https://doi.org/10.3390/acoustics2030030




