Noncontact and Full-Field Measurement of Residual and Thermal Stress in Film/Substrate Structures
Abstract
1. Introduction
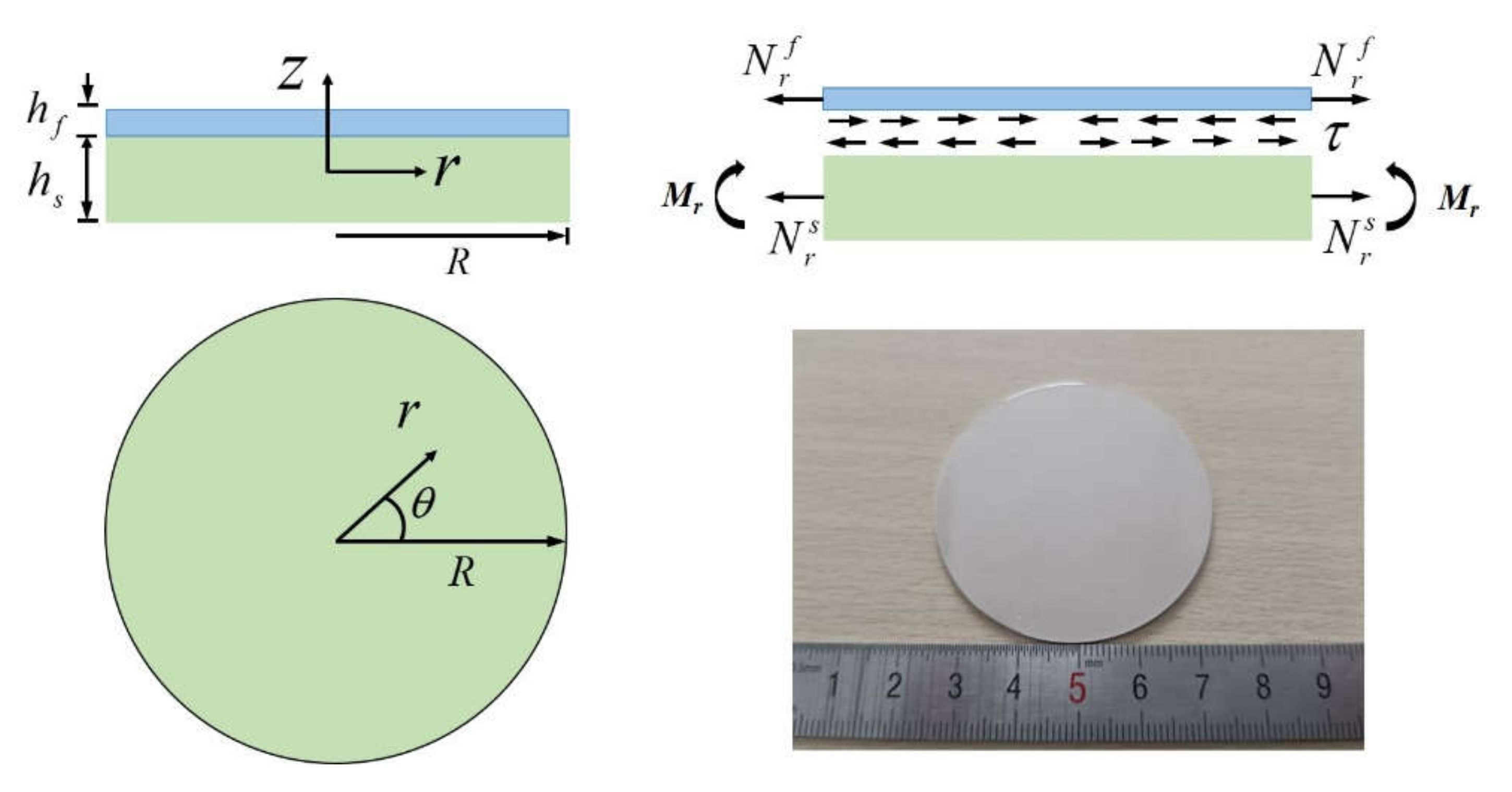
2. Theoretical Equation
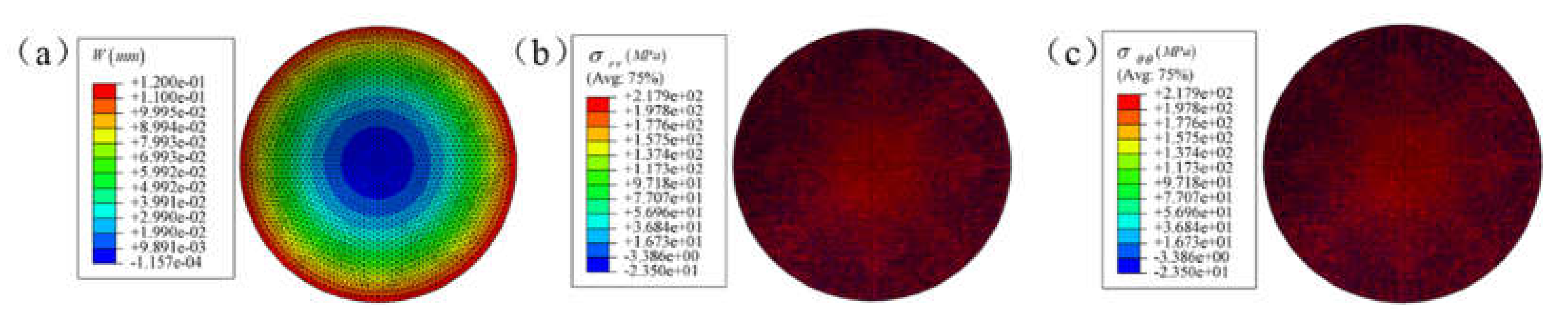
3. Numerical Simulation
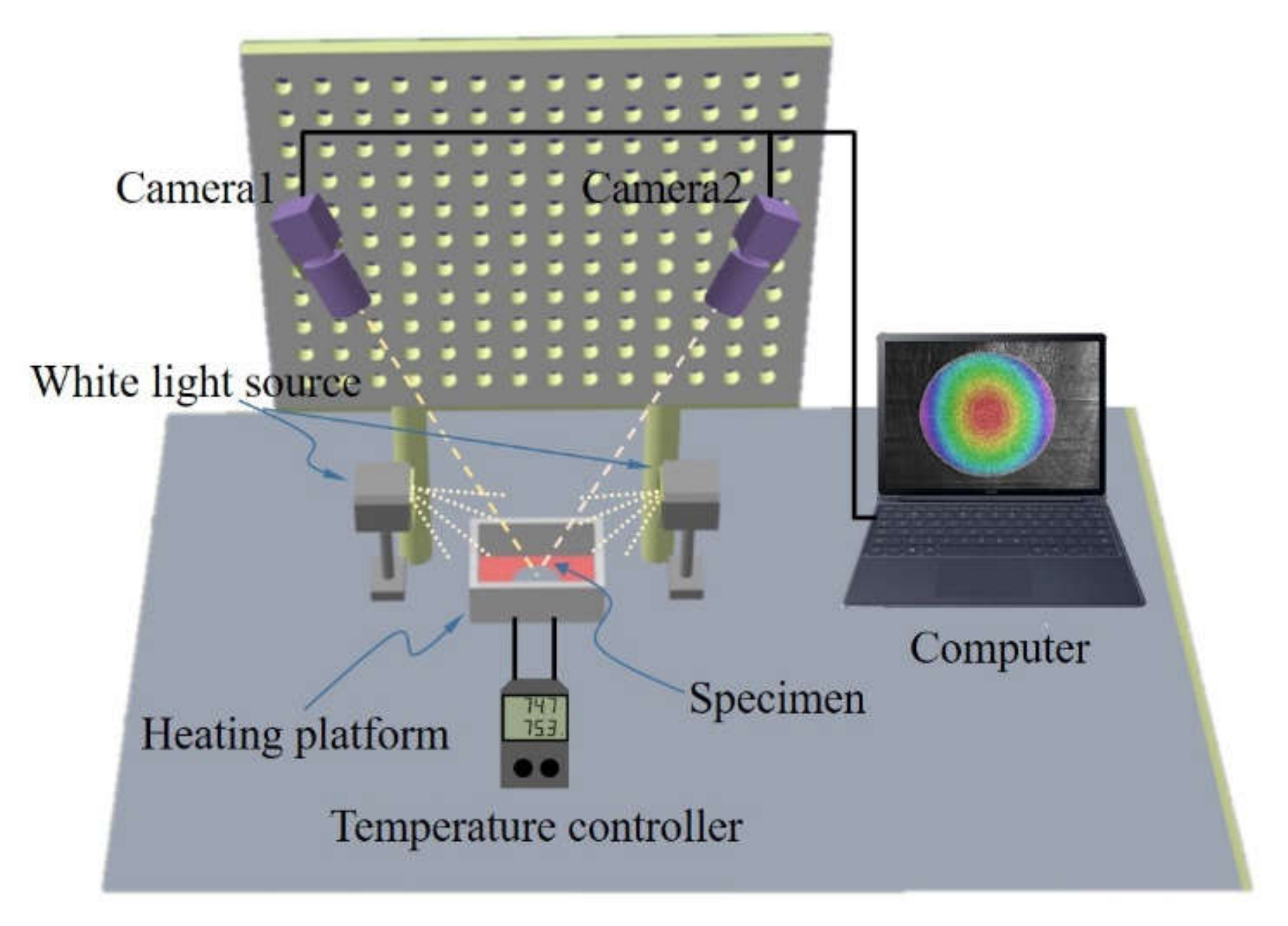
4. Experiment
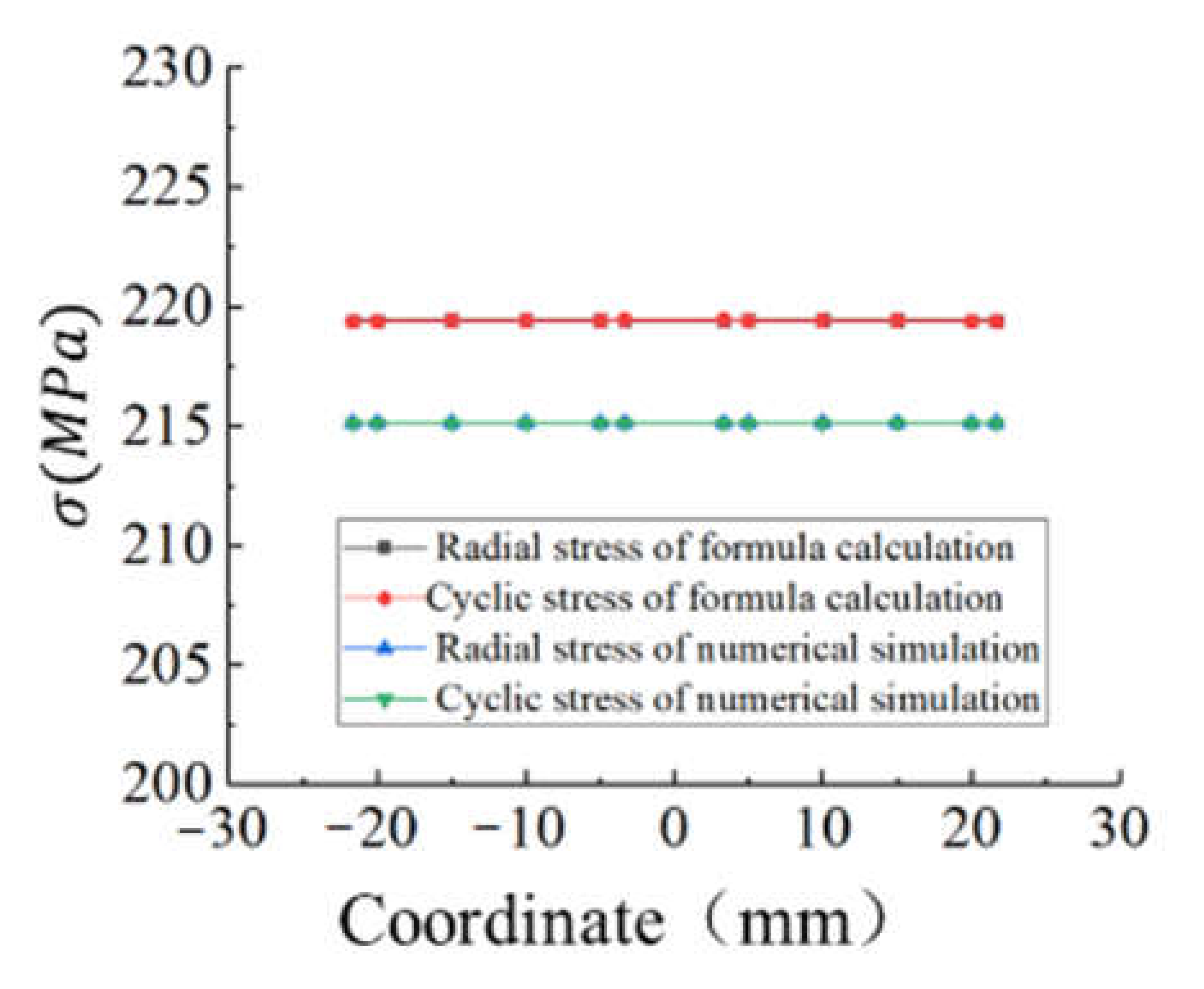
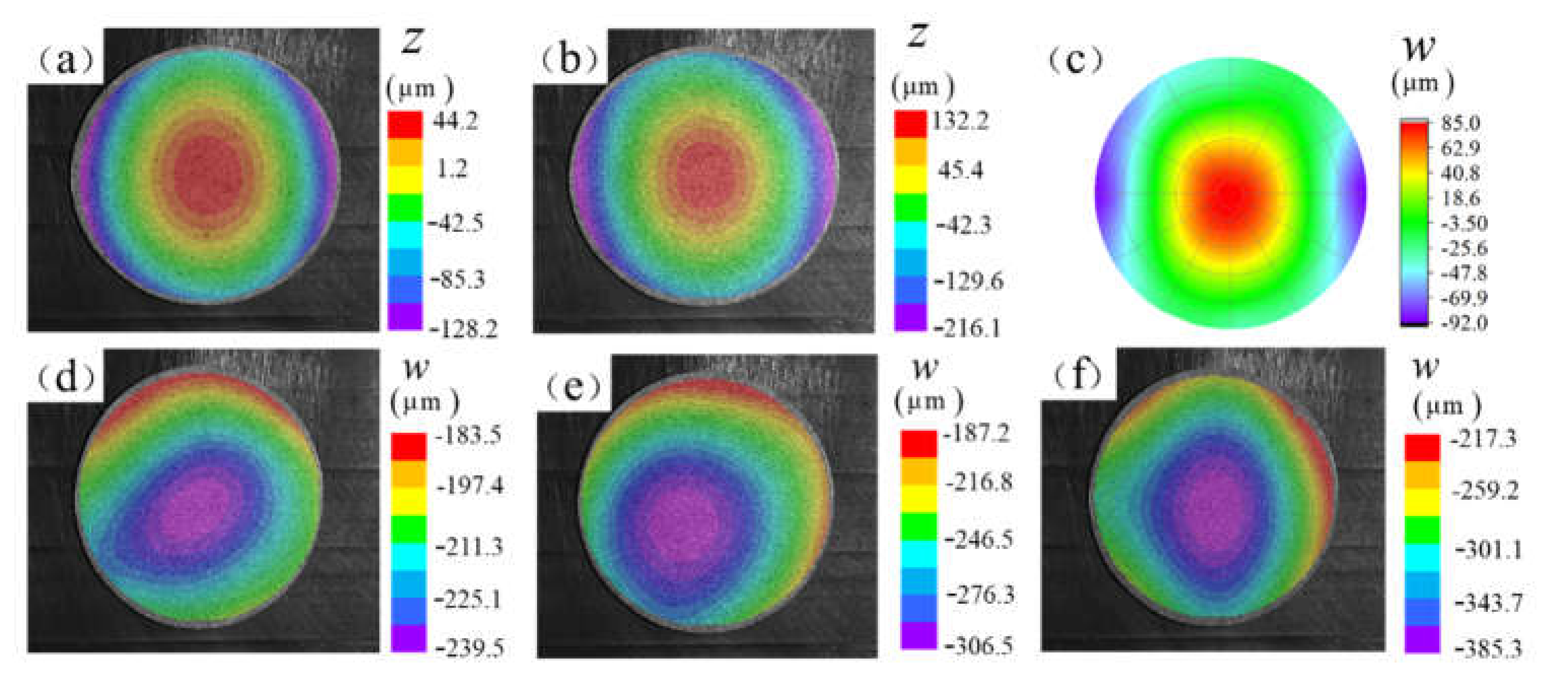
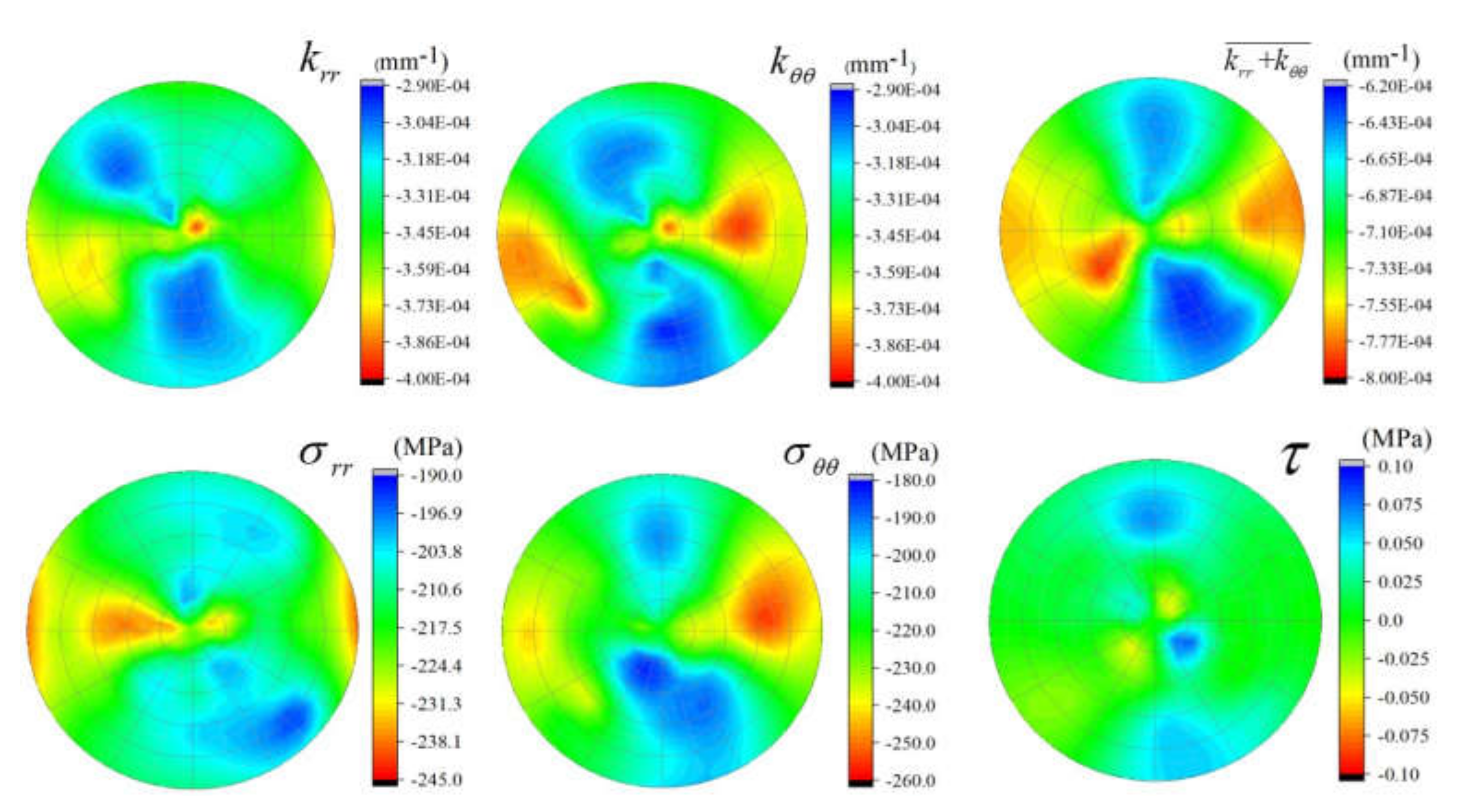
5. Results and Discussion
5.1. Residual Stress
5.2. Thermal Stress
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abadias, G.; Chason, E.; Keckes, J.; Sebastiani, M.; Thompson, G.B.; Barthel, E.; Doll, G.; Murray, C.; Stoessel, C.H.; Martinu, L. Review Article: Stress in thin films and coatings: Current status, challenges, and prospects. J. Vac. Sci. Technol. A 2018, 36, 020801. [Google Scholar] [CrossRef]
- Chason, E.; Guduru, P.R. Tutorial: Understanding residual stress in polycrystalline thin films through real-time measure-ments and physical models. J. Appl. Phys. 2016, 119, 1032–1114. [Google Scholar] [CrossRef]
- Yamamoto, T.; Nagayama, T.; Nakamura, T. Thermal Expansion and Thermal Stress Behavior of Electroless-Plated Fe–Ni–B Alloy Thin Film for High-Density Packaging. J. Electrochem. Soc. 2018, 166, D3238–D3245. [Google Scholar] [CrossRef]
- Zhang, X.; Meng, H.; Wang, H.; Guo, F. Effect of thermal misfit stress on steam-driven delamination in electronic packages. Eng. Fract. Mech. 2018, 194, 61–72. [Google Scholar] [CrossRef]
- Zhu, J.G.; Xie, H.M.; Li, Y.J.; Hu, Z.X.; Luo, Q.; Gu, C.Z. Interfacial Residual Stress Analysis of Thermal Spray Coatings by Miniature Ring-Core Cutting Combined with DIC Method. Exp. Mech. 2014, 54, 127–136. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Z.; Xie, H. A real time deformation evaluation method for surface and interface of thermal barrier coatings during 1100 °C thermal shock. Meas. Sci. Technol. 2012, 23, 105604. [Google Scholar] [CrossRef]
- Zhu, J.G.; Chen, W.; Xie, H.M. Simulation of residual stresses and their effects on thermal barrier coating systems using finite element method. Sci. China Phys. Mech. Astron. 2015, 58, 1–10. [Google Scholar] [CrossRef]
- Stoney, G. The tension of metallic films deposited by electrolysis. J. R. Soc. Lond. Ser. A 1909, 82, 172–175. [Google Scholar]
- Yu, H.Z.; Thompson, C.V. Effects of oblique-angle deposition on intrinsic stress evolution during polycrystalline film growth. Acta Mater. 2014, 77, 284–293. [Google Scholar] [CrossRef]
- Yu, H.Z.; Thompson, C.V. Grain growth and complex stress evolution during Volmer–Weber growth of polycrystalline thin films. Acta Mater. 2014, 67, 189–198. [Google Scholar] [CrossRef]
- Al-Masha’Al, A.; Bunting, A.; Cheung, R. Evaluation of residual stress in sputtered tantalum thin-film. Appl. Surf. Sci. 2016, 371, 571–575. [Google Scholar] [CrossRef]
- Dong, X.; Feng, X.; Hwang, K.-C.; Ma, S.; Ma, Q. Full-field measurement of nonuniform stresses of thin films at high temperature. Opt. Express 2011, 19, 13201–13208. [Google Scholar] [CrossRef] [PubMed]
- Dong, X.L.; Feng, X.; Hwang, K.C. Non-uniform stress distribution and deformation bifurcation of thin film/substrate sys-tem subjected to gradient temperature. Thin Solid Films 2011, 519, 2464–2469. [Google Scholar] [CrossRef]
- Feng, X.; Huang, Y.; Rosakis, A.J. Stresses in a Multilayer Thin Film/Substrate System Subjected to Nonuniform Temperature. J. Appl. Mech. 2008, 75, 021022. [Google Scholar] [CrossRef]
- Zhang, C.; Dong, X.; Feng, X.; Hwang, K.-C. Multiwavelength shearing interferometry for measuring the slopes, curvatures, and shapes of thin films/substrate systems. Opt. Lett. 2013, 38, 5446–5449. [Google Scholar] [CrossRef] [PubMed]
- Tippur, H.V. Simultaneous and real-time measurement of slope and curvature fringes in thin structures using shearing in-terferometery. Opt. Eng. 2004, 43, 3014–3020. [Google Scholar] [CrossRef]
- Ma, K.; Xie, H.; Zhu, J. Two modified coherent gradient sensing methods for slope measurement of reflective surfaces. Meas. Sci. Technol. 2014, 25, 125202. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, C.; Feng, X.; Duan, Z. Analysis and improvement of accuracy, sensitivity, and resolution of the coherent gradient sensing method. Appl. Opt. 2016, 55, 4752–4758. [Google Scholar] [CrossRef]
- Colin, J.J.; Abadias, G.; Michel, A.; Jaouen, C. On the origin of the metastable beta-Ta phase stabilization in tantalum sputtered thin films. Acta Mater. 2017, 126, 481–493. [Google Scholar] [CrossRef]
- Pan, B. Digital image correlation for surface deformation measurement: Historical developments, recent advances and future goals. Meas. Sci. Technol. 2018, 29, 082001. [Google Scholar] [CrossRef]
- Pelleg, J. Mechanical properties of materials. Solid Mech. Appl. 2013, 190, 1–634. [Google Scholar]
- Touloukian, Y.S.; Kirby, R.K.; Taylor, R.E.; Desai, P.D. Thermophysical Properties of Matters. Thermal Expansion of Metallic Elements and Alloys; Plenum: New York, NY, USA, 1976; Volume 12. [Google Scholar]
- Sutton, M.A.; Orteu, J.J.; Schreier, H. Image Correlation for Shape, Motion and Deformation Measurements; Springer: New York, NY, USA, 2009. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, D.; Zhang, X.; Zhu, J. Noncontact and Full-Field Measurement of Residual and Thermal Stress in Film/Substrate Structures. Surfaces 2021, 4, 89-96. https://doi.org/10.3390/surfaces4010011
Yang D, Zhang X, Zhu J. Noncontact and Full-Field Measurement of Residual and Thermal Stress in Film/Substrate Structures. Surfaces. 2021; 4(1):89-96. https://doi.org/10.3390/surfaces4010011
Chicago/Turabian StyleYang, Dong, Xumeng Zhang, and Jianguo Zhu. 2021. "Noncontact and Full-Field Measurement of Residual and Thermal Stress in Film/Substrate Structures" Surfaces 4, no. 1: 89-96. https://doi.org/10.3390/surfaces4010011
APA StyleYang, D., Zhang, X., & Zhu, J. (2021). Noncontact and Full-Field Measurement of Residual and Thermal Stress in Film/Substrate Structures. Surfaces, 4(1), 89-96. https://doi.org/10.3390/surfaces4010011





