Controllable CaF2 Nanosized Stripe Arrays on Si(001) Studied by X-ray and Electron Diffraction
Abstract
:1. Introduction
2. Materials and Methods
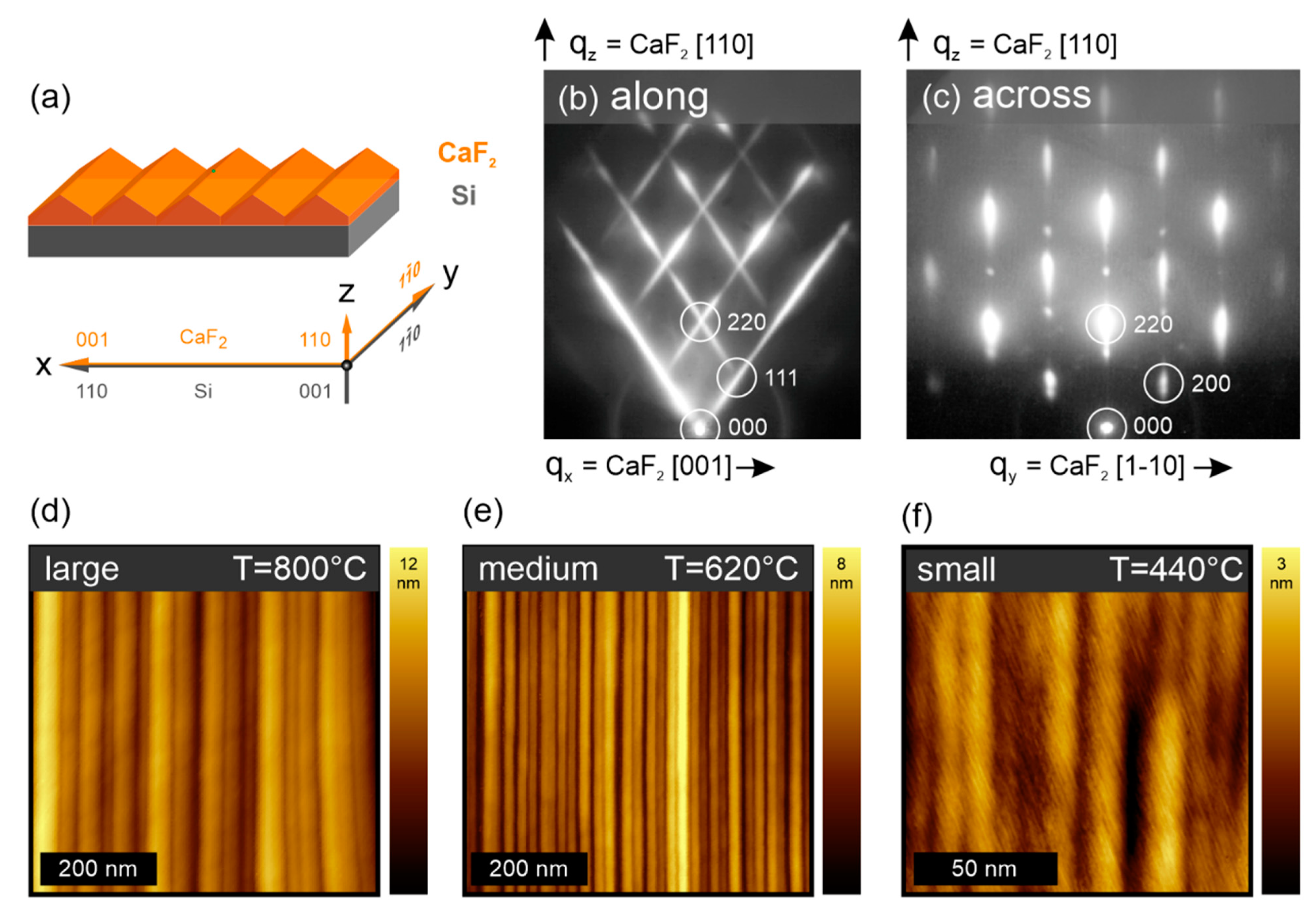
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tromp, R.M.; Reuter, M.C. Structure of the Si(111)-CaF2 Interface. Phys. Rev. Lett. 1988, 61, 1756–1759. [Google Scholar] [CrossRef] [PubMed]
- Zegenhagen, J.; Patel, J.R. CaF2/Si heteroepitaxy: Importance of stoichiometry, interface bonding, and lattice mismatch. Phys. Rev. B 1990, 41, 5315–5318. [Google Scholar] [CrossRef] [PubMed]
- Rieger, D.; Himpsel, F.J.; Karlsson, U.O.; McFeely, F.R.; Morar, J.F.; Yarmoff, J.A. Electronic structure of the CaF2/Si(111) interface. Phys. Rev. B 1986, 34, 7295–7306. [Google Scholar] [CrossRef] [PubMed]
- Sugiyama, M.; Oshima, M. MBE growth of fluorides. Microelectron. J. 1996, 27, 361–382. [Google Scholar] [CrossRef]
- Schowalter, L.J.; Fathauer, R.W. Growth and characterization of single crystal insulators on silicon. Crit. Rev. Solid State Mater. Sci. 1989, 15, 367–421. [Google Scholar] [CrossRef]
- Sokolov, N.S.; Suturin, S.M.; Krichevtsov, B.B.; Dubrovskii, V.G.; Gastev, S.V.; Sibirev, N.V.; Baranov, D.A.; Fedorov, V.V.; Sitnikova, A.A.; Nashchekin, A.V.; et al. Cobalt epitaxial nanoparticles on CaF2/Si(111): Growth process, morphology, crystal structure, and magnetic properties. Phys. Rev. B 2013, 87, 125407. [Google Scholar] [CrossRef]
- Baranov, D.A.; Krichevtsov, B.B.; Gastev, S.V.; Banschikov, A.G.; Fedorov, V.V.; Koshmak, K.V.; Suturin, S.M.; Sokolov, N.S. Magnetic anisotropy of cobalt nanoparticle 2D arrays grown on corrugated MnF2(110) and CaF2(110) surfaces. Appl. Surf. Sci. 2013, 267, 196–199. [Google Scholar] [CrossRef]
- Suturin, S.M.; Fedorov, V.V.; Korovin, A.M.; Sokolov, N.S.; Nashchekin, A.V.; Tabuchi, M. Epitaxial Ni nanoparticles on CaF2 (001), (110) and (111) surfaces studied by three-dimensional RHEED, GIXD and GISAXS reciprocal-space mapping techniques. J. Appl. Crystallogr. 2017, 50, 830–839. [Google Scholar] [CrossRef]
- Suturin, S.M.; Korovin, A.M.; Fedorov, V.V.; Valkovsky, G.A.; Tabuchi, M.; Sokolov, N.S. An advanced three-dimensional RHEED mapping approach to the diffraction study of Co/MnF2/CaF2/Si(001) epitaxial heterostructures. J. Appl. Crystallogr. 2016, 49, 1532–1543. [Google Scholar] [CrossRef]
- Suturin, S.M.; Fedorov, V.V.; Banshchikov, A.G.; Baranov, D.A.; Koshmak, K.V.; Torelli, P.; Fujii, J.; Panaccione, G.; Amemiya, K.; Sakamaki, M.; et al. Proximity effects and exchange bias in Co/MnF2(111) heterostructures studied by X-ray magnetic circular dichroism. J. Phys. Condens. Matter 2013, 25, 046002. [Google Scholar] [CrossRef]
- Banshchikov, A.G.; Golosovskii, I.V.; Krupin, A.V.; Koshmak, K.V.; Sokolov, N.S.; Chernenkov, Y.P.; Yagovkina, M.A.; Ulin, V.P.; Tabuchi, M. Epitaxial layers of nickel fluoride on Si(111): Growth and stabilization of the orthorhombic phase. Phys. Solid State 2015, 57, 1647–1652. [Google Scholar] [CrossRef]
- Rothman, A.; Dubrovskii, V.G.; Joselevich, E. Kinetics and mechanism of planar nanowire growth. Proc. Natl. Acad. Sci. USA 2020, 117, 152–160. [Google Scholar] [CrossRef]
- Weiss, N.O.; Duan, X. A guide for nanowire growth. Proc. Natl. Acad. Sci. USA 2013, 110, 15171–15172. [Google Scholar] [CrossRef] [Green Version]
- Ito, K.; Nakano, H.; Nakamura, Y. Formation of Silicon Quantum Dots Sheet on a Nonmetallic CaF2 Surface. Adv. Mater. Interfaces 2020, 7, 2001295. [Google Scholar] [CrossRef]
- Illarionov, Y.Y.; Vexler, M.I.; Fedorov, V.V.; Suturin, S.M.; Sokolov, N.S. Electrical and optical characterization of Au/CaF2/p-Si(111) tunnel-injection diodes. J. Appl. Phys. 2014, 115, 223706. [Google Scholar] [CrossRef] [Green Version]
- Vexler, M.I.; Sokolov, N.S.; Suturin, S.M.; Banshchikov, A.G.; Tyaginov, S.E.; Grasser, T. Electrical characterization and modeling of the Au/CaF2/nSi(111) structures with high-quality tunnel-thin fluoride layer. J. Appl. Phys. 2009, 105, 083716. [Google Scholar] [CrossRef]
- Illarionov, Y.Y.; Banshchikov, A.G.; Polyushkin, D.K.; Wachter, S.; Knobloch, T.; Thesberg, M.; Mennel, L.; Paur, M.; Stöger-Pollach, M.; Steiger-Thirsfeld, A.; et al. Ultrathin calcium fluoride insulators for two-dimensional field-effect transistors. Nat. Electron. 2019, 2, 230–235. [Google Scholar] [CrossRef]
- Sumiya, T.; Miura, T.; Fujinuma, H.; Tanaka, S. Surface reconstruction in CaF2/Si(001) investigated by scanning tunneling microscopy. Surf. Sci. 1997, 376, 192–204. [Google Scholar] [CrossRef]
- Loretto, D.; Ross, F.M.; Lucas, C.A. Quasi-one-dimensional CaF2 islands formed on Si(001) by molecular beam epitaxy. Appl. Phys. Lett. 1996, 68, 2363–2365. [Google Scholar] [CrossRef]
- Pasquali, L.; D’Addato, S.; Selvaggi, G.; Nannarone, S.; Sokolov, N.S.; Suturin, S.M.; Zogg, H. Formation of CaF2 nanostructures on Si(001). Nanotechnology 2001, 12, 403–408. [Google Scholar] [CrossRef]
- Sokolov, N.; Suturin, S.; Ulin, V.; Pasquali, L.; Selvaggi, G.; Nannarone, S. Initial stages of MBE growth and formation of CaF2/Si(001) high-temperature interface. Appl. Surf. Sci. 2004, 234, 480–486. [Google Scholar] [CrossRef]
- Pasquali, L.; Suturin, S.; Ulin, V.; Sokolov, N.; Selvaggi, G.; Giglia, A.; Mahne, N.; Pedio, M.; Nannarone, S. Calcium fluoride on Si(001): Adsorption mechanisms and epitaxial growth modes. Phys. Rev. B 2005, 72, 045448. [Google Scholar] [CrossRef]
- Suturin, S.M.; Sokolov, N.S.; Roy, J.; Zegenhagen, J. STM and LEED studies of CaF2 submonolayer coverage on Si(001). Surf. Sci. 2011, 605, 153–157. [Google Scholar] [CrossRef]
- Suturin, S.M.; Sokolov, N.S.; Banshchikov, A.G.; Kyutt, R.N.; Sakata, O.; Shimura, T.; Harada, J.; Tabuchi, M.; Takeda, Y. Initial Stages of High-Temperature CaF2/Si(001) Epitaxial Growth Studied by Surface X-Ray Diffraction. J. Nanosci. Nanotechnol. 2011, 11, 2990–2996. [Google Scholar] [CrossRef] [PubMed]
- Alzahrani, A.Z.; Usanmaz, D. Progressive changes in surface structure and electronic properties on Si(001) surface by CaF2 adsorption. J. Appl. Phys. 2011, 109, 123708. [Google Scholar] [CrossRef]
- Baskurt, M.; Kang, J.; Sahin, H. Octahedrally coordinated single layered CaF2: Robust insulating behaviour. Phys. Chem. Chem. Phys. 2020, 22, 2949–2954. [Google Scholar] [CrossRef]
- Suturin, S.M.; Fedorov, V.V.; Korovin, A.M.; Valkovskiy, G.A.; Konnikov, S.G.; Tabuchi, M.; Sokolov, N.S. A look inside epitaxial cobalt-on-fluorite nanoparticles with three-dimensional reciprocal space mapping using GIXD, RHEED and GISAXS. J. Appl. Crystallogr. 2013, 46, 874–881. [Google Scholar] [CrossRef] [Green Version]
- Chiaravalloti, F.; Dujardin, G.; Riedel, D. Atomic scale control of hexaphenyl molecules manipulation along functionalized ultra-thin insulating layer on the Si(100) surface at low temperature (9 K). J. Phys. Condens. Matter 2015, 27, 054006. [Google Scholar] [CrossRef]
- Duverger, E.; Boyer, A.-G.; Sauriat-Dorizon, H.; Sonnet, P.; Stephan, R.; Hanf, M.-C.; Riedel, D. Two-Dimensional Functionalized Ultrathin Semi-Insulating CaF2 Layer on the Si(100) Surface at a Low Temperature for Molecular Electronic Decoupling. ACS Appl. Mater. Interfaces 2020, 12. [Google Scholar] [CrossRef]
- Ramos, P.; Mankarious, M.; Pavanello, M.; Riedel, D. Probing charge transfer dynamics in a single iron tetraphenylporphyrin dyad adsorbed on an insulating surface. Nanoscale 2018, 10, 17603–17616. [Google Scholar] [CrossRef] [Green Version]
- Ishizaka, A.; Shiraki, Y. Low Temperature Surface Cleaning of Silicon and Its Application to Silicon MBE. J. Electrochem. Soc. 1986, 133, 666–671. [Google Scholar] [CrossRef]
- Rueda, D.R.; Martín-Fabiani, I.; Soccio, M.; Alayo, N.; Pérez-Murano, F.; Rebollar, E.; García-Gutiérrez, M.C.; Castillejo, M.; Ezquerra, T.A. Grazing-incidence small-angle X-ray scattering of soft and hard nanofabricated gratings. J. Appl. Crystallogr. 2012, 45, 1038–1045. [Google Scholar] [CrossRef]
- Wernecke, J.; Scholze, F.; Krumrey, M. Direct structural characterisation of line gratings with grazing incidence small-angle x-ray scattering. Rev. Sci. Instrum. 2012, 83, 103906. [Google Scholar] [CrossRef] [PubMed]
- Lazzari, R. IsGISAXS: A program for grazing-incidence small-angle X-ray scattering analysis of supported islands. J. Appl. Crystallogr. 2002, 35, 406–421. [Google Scholar] [CrossRef] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suturin, S.M.; Fedorov, V.V.; Korovin, A.M.; Valkovskiy, G.A.; Tabuchi, M.; Sokolov, N.S. Controllable CaF2 Nanosized Stripe Arrays on Si(001) Studied by X-ray and Electron Diffraction. Surfaces 2021, 4, 97-105. https://doi.org/10.3390/surfaces4020012
Suturin SM, Fedorov VV, Korovin AM, Valkovskiy GA, Tabuchi M, Sokolov NS. Controllable CaF2 Nanosized Stripe Arrays on Si(001) Studied by X-ray and Electron Diffraction. Surfaces. 2021; 4(2):97-105. https://doi.org/10.3390/surfaces4020012
Chicago/Turabian StyleSuturin, Sergey M., Vladimir V. Fedorov, Alexander M. Korovin, Gleb A. Valkovskiy, Masao Tabuchi, and Nikolai S. Sokolov. 2021. "Controllable CaF2 Nanosized Stripe Arrays on Si(001) Studied by X-ray and Electron Diffraction" Surfaces 4, no. 2: 97-105. https://doi.org/10.3390/surfaces4020012
APA StyleSuturin, S. M., Fedorov, V. V., Korovin, A. M., Valkovskiy, G. A., Tabuchi, M., & Sokolov, N. S. (2021). Controllable CaF2 Nanosized Stripe Arrays on Si(001) Studied by X-ray and Electron Diffraction. Surfaces, 4(2), 97-105. https://doi.org/10.3390/surfaces4020012





