Production Optimization of Exotic Hypernuclei via Heavy-Ion Beams at GSI-FAIR
Abstract
1. Introduction
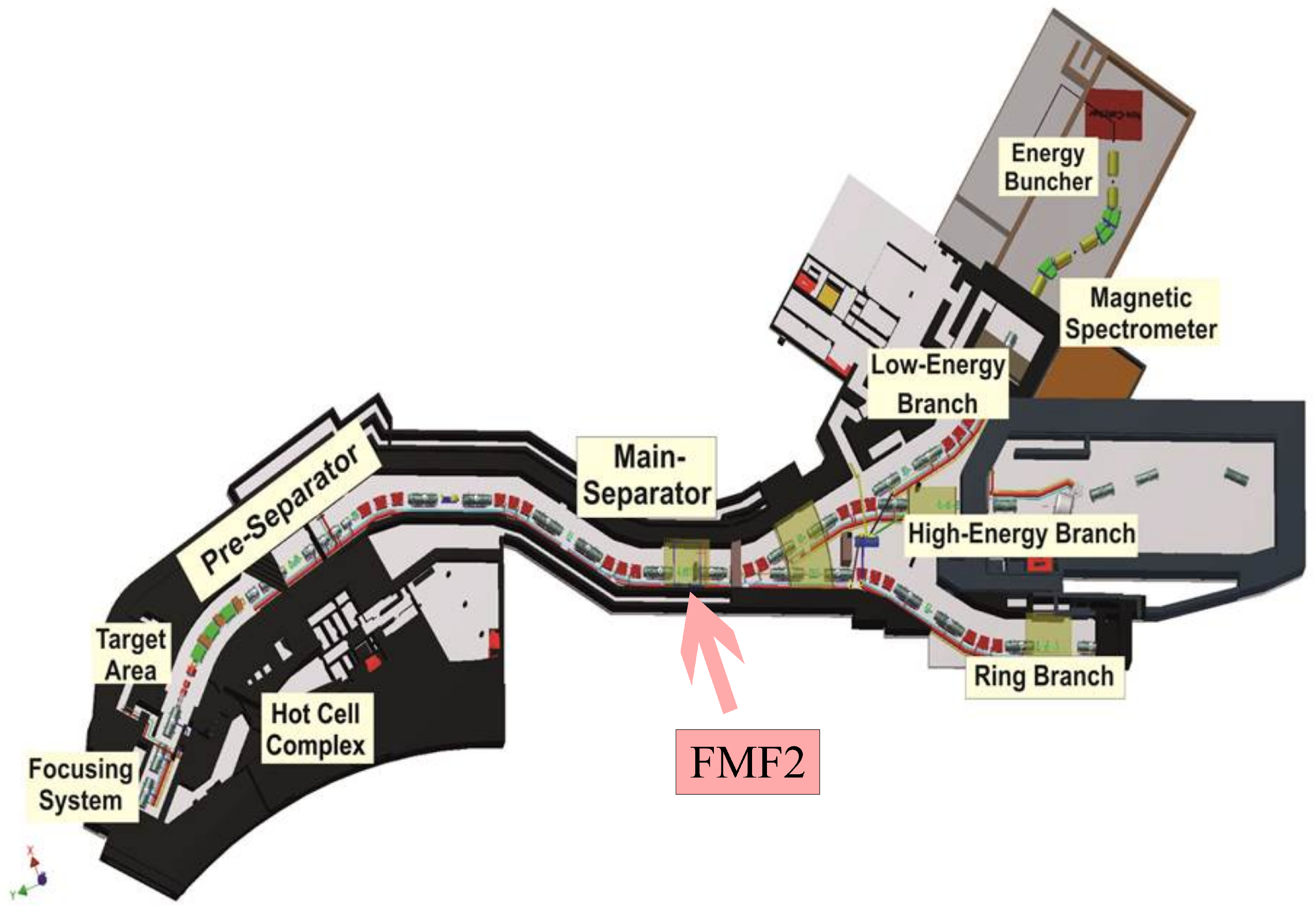
2. Materials and Methods
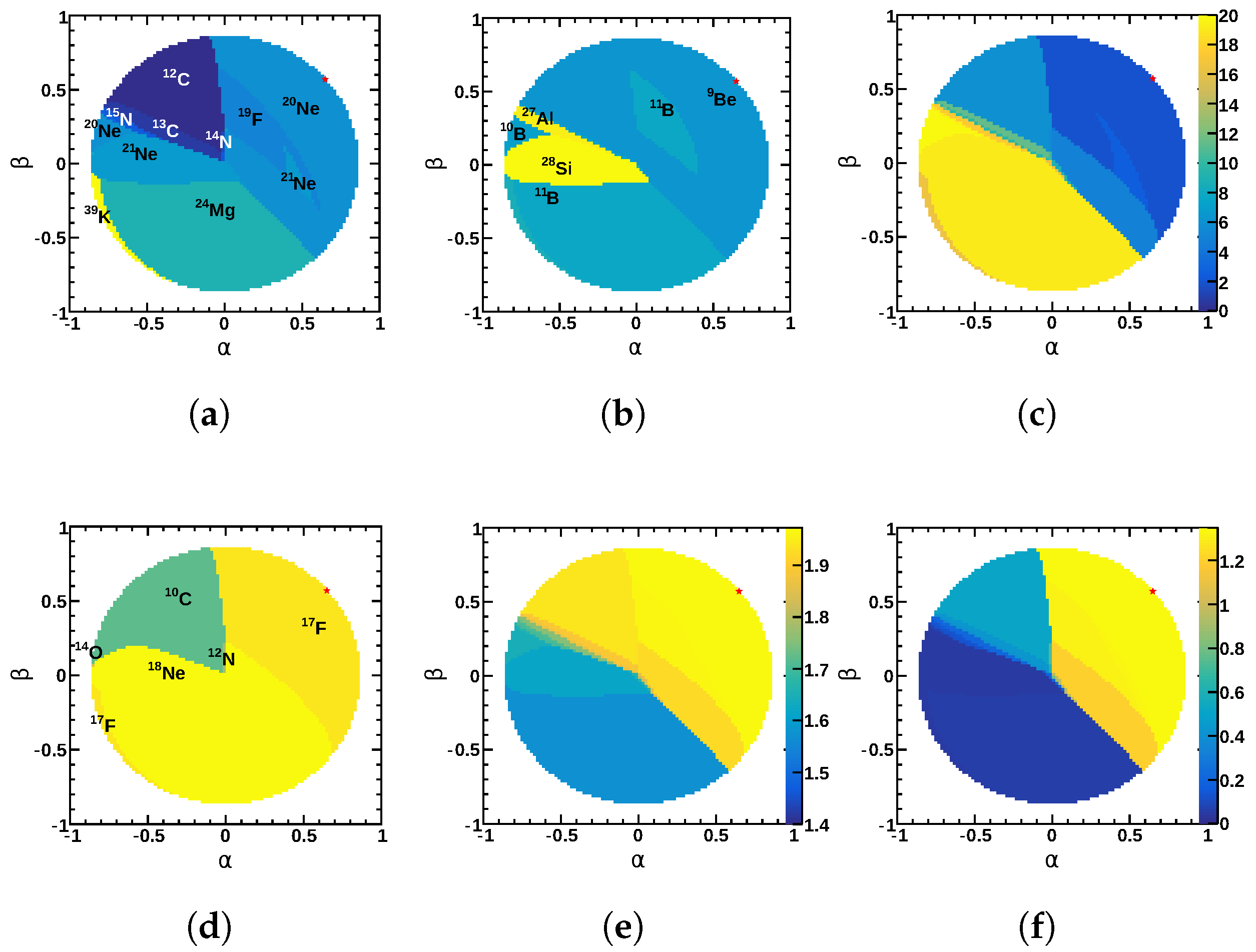
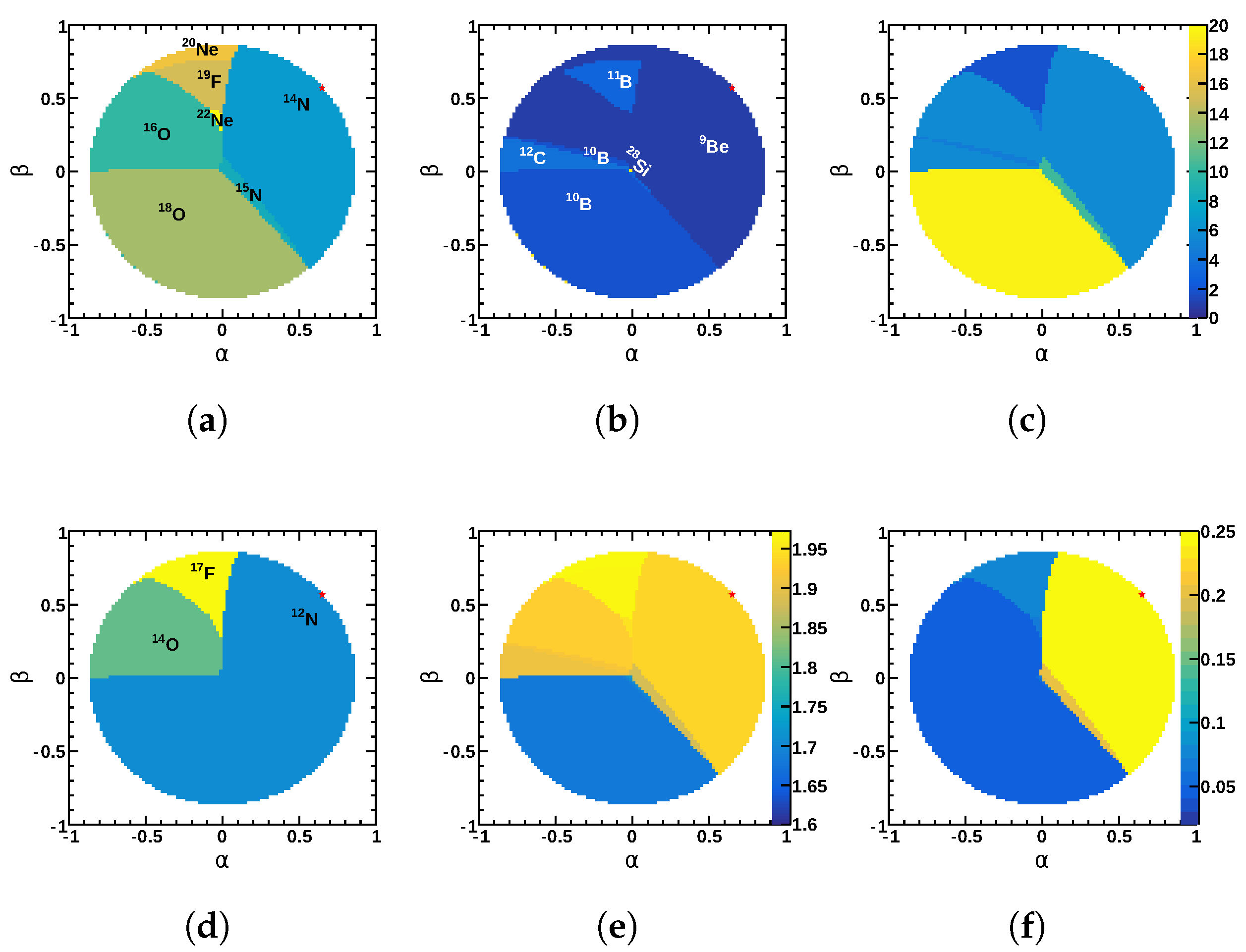
3. Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koch, P.; Müller, B.; Rafelski, J. Strangeness in relativistic heavy ion collisions. Phys. Rep. 1986, 142, 167–262. [Google Scholar] [CrossRef]
- Margetis, S.; Safarík, K.; Villalobos Baillie, O. Strangeness Production in Heavy-Ion Collisions. Annu. Rev. Nucl. Part. Sci. 2000, 50, 299–342. [Google Scholar] [CrossRef]
- Tamura, H. Baryon-baryon interactions with strangeness studied from hypernuclei. Nucl. Phys. A 2005, 752, 155–164. [Google Scholar] [CrossRef]
- GSI Helmholtz Centre for Heavy Ion Research. Available online: https://www.gsi.de (accessed on 1 February 2025).
- FAIR Facility for Antiproton and Ion Research in Europe. Available online: https://fair-center.eu (accessed on 1 February 2025).
- Blasche, K.; Bohne, D.; Franzke, B.; Prange, H. The SIS Heavy Ion Synchrotron Project. IEEE Trans. Nucl. Sci. 1985, 32, 2657–2661. [Google Scholar] [CrossRef]
- Blasche, K.; Prange, H.; Eickhoff, H.; Steiner, R.; Böhne, D.; Franczak, B.; Franzke, B. Status of the SIS/ESR-facility at Gsi Darmstadt. Part. Accel. 1990, 32, 83–90. [Google Scholar]
- Armbruster, P.; Clerc, H.; Dufour, J.P.; Franczak, B.; Geissel, H.; Hanelt, E.; Klepper, O.; Langenbeck, B.; Münzenberg, G.; Nickel, F.; et al. The projectile-fragment separator at the Darmstadt SIS/ESR facility. AIP Conf. Proc. 1987, 164, 839–844. [Google Scholar] [CrossRef]
- Geissel, H.; Armbruster, P.; Behr, K.; Brünle, A.; Burkard, K.; Chen, M.; Folger, H.; Franczak, B.; Keller, H.; Klepper, O.; et al. The GSI projectile fragment separator (FRS): A versatile magnetic system for relativistic heavy ions. Nucl. Instrum. Meth. B 1992, 70, 286–297. [Google Scholar] [CrossRef]
- Geissel, H.; Weick, H.; Winkler, M.; Münzenberg, G.; Chichkine, V.; Yavor, M.; Aumann, T.; Behr, K.; Böhmer, M.; Brünle, A.; et al. The Super-FRS project at GSI. Nucl. Instrum. Meth. B 2003, 204, 71–85. [Google Scholar] [CrossRef]
- Hashimoto, O.; Tamura, H. Spectroscopy of Λ hypernuclei. Prog. Part. Nucl. Phys. 2006, 57, 564–653. [Google Scholar] [CrossRef]
- Botta, E.; Bressani, T.; Garbarino, G. Strangeness nuclear physics: A critical review on selected topics. Eur. Phys. J. A 2012, 48, 41. [Google Scholar] [CrossRef]
- Feliciello, A.; Nagae, T. Experimental review of hypernuclear physics: Recent achievements and future perspectives. Rep. Prog. Phys. 2015, 78, 096301. [Google Scholar] [CrossRef] [PubMed]
- Nield, K.J.; Bowen, T.; Cable, G.D.; DeLise, D.A.; Jenkins, E.W.; Kalbach, R.M.; Noggle, R.C.; Pifer, A.E. Production of hypernuclei in a 2.1 GeV/nucleon oxygen beam. Phys. Rev. C 1976, 13, 1263–1266. [Google Scholar] [CrossRef]
- Abdurakhimov, A.; Aksinenko, V.; Anikina, M.; Avramenko, S.; Bannik, B.; Butenko, V.; Glagoleva, N.; Golokhvastov, A.; Grachev, A.; Dementiev, E.; et al. Experimental study of relativistic hypernuclei using the HYBS-spectrometer. Nuov. Cim. A 1989, 102, 645–652. [Google Scholar] [CrossRef]
- Avramenko, S.; Aksinenko, V.; Anikina, M.; Bannik, B.; Belikov, Y.; Butenko, V.; Drozdov, V.; Gajevski, K.; Galperin, A.; Glagoleva, N.; et al. A study of the production and lifetime of the lightest relativistic hypernuclei. Nucl. Phys. A 1992, 547, 95–100. [Google Scholar] [CrossRef]
- Rappold, C.; Kim, E.; Nakajima, D.; Saito, T.; Bertini, O.; Bianchin, S.; Bozkurt, V.; Kavatsyuk, M.; Ma, Y.; Maas, F.; et al. Hypernuclear spectroscopy of products from 6Li projectiles on a carbon target at 2 AGeV. Nucl. Phys. A 2013, 913, 170–184. [Google Scholar] [CrossRef]
- Rappold, C.; Kim, E.; Saito, T.R.; Bertini, O.; Bianchin, S.; Bozkurt, V.; Kavatsyuk, M.; Ma, Y.; Maas, F.; Minami, S.; et al. Search for evidence of by observing d+π− and t+π− final states in the reaction of 6Li+12C at 2A GeV. Phys. Rev. C 2013, 88, 041001. [Google Scholar] [CrossRef]
- Rappold, C.; Saito, T.; Bertini, O.; Bianchin, S.; Bozkurt, V.; Kim, E.; Kavatsyuk, M.; Ma, Y.; Maas, F.; Minami, S.; et al. Hypernuclear production cross-section in the reaction of 6Li + 12C at 2 A GeV. Phys. Lett. B 2015, 747, 129–134. [Google Scholar] [CrossRef]
- Miyagawa, K.; Kamada, H.; Glöckle, W.; Stoks, V. Properties of the bound Λ(Σ)NN system and hyperon-nucleon interactions. Phys. Rev. C 1995, 51, 2905–2913. [Google Scholar] [CrossRef]
- Gal, A.; Garcilazo, H. Is there a bound nΛ3? Phys. Lett. B 2014, 736, 93–97. [Google Scholar] [CrossRef]
- Hiyama, E.; Ohnishi, S.; Gibson, B.F.; Rijken, T.A. Three-body structure of the nnΛ system with ΛN − ΣN coupling. Phys. Rev. C 2014, 89, 061302. [Google Scholar] [CrossRef]
- Ando, S.I.; Raha, U.; Oh, Y. Investigation of the nnΛ bound state in pionless effective theory. Phys. Rev. C 2015, 92, 024325. [Google Scholar] [CrossRef]
- Richard, J.M.; Wang, Q.; Zhao, Q. Lightest neutral hypernuclei with strangeness −1 and −2. Phys. Rev. C 2015, 91, 014003. [Google Scholar] [CrossRef]
- Saito, T.R.; Dou, W.; Drozd, V.; Ekawa, H.; Escrig, S.; He, Y.; Kalantar-Nayestanaki, N.; Kasagi, A.; Kavatsyuk, M.; Liu, E.; et al. New directions in hypernuclear physics. Nat. Rev. Phys. 2021, 3, 803–813. [Google Scholar] [CrossRef]
- Escrig, S. Status of the WASA-FRS HypHI Experiment: Study of Light Hypernuclei at GSI-FAIR. Acta Phys. Pol. B Proc. Suppl. 2024, 17, 3-A19. [Google Scholar] [CrossRef]
- Saito, T.; Achenbach, P.; Alfaki, H.A.; Amjad, F.; Armstrong, M.; Behr, K.H.; Benlliure, J.; Brencic, Z.; Dickel, T.; Drozd, V.; et al. The WASA-FRS project at GSI and its perspective. Nucl. Instrum. Meth. B 2023, 542, 22–25. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adler, A.; Aglieri Rinella, G.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahmad, S.; Ahn, S.U.; Ahuja, I.; et al. Measurement of the Lifetime and Λ Separation Energy of . Phys. Rev. Lett. 2023, 131, 102302. [Google Scholar] [CrossRef]
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Anderson, D.M.; Aparin, A.; et al. Measurement of the mass difference and the binding energy of the hypertriton and antihypertriton. Nat. Phys. 2020, 16, 409–412. [Google Scholar] [CrossRef]
- Bargholtz, C.; Bashkanov, M.; Berłowski, M.; Bondar, A.; Bogoslawsky, D.; Brodowski, W.; Brudvik, J.; Calén, H.; Capellaro, F.; Chilingarov, A.; et al. The WASA detector facility at CELSIUS. Nucl. Instrum. Meth. A 2008, 594, 339–350. [Google Scholar] [CrossRef]
- Rappold, C.; López-Fidalgo, J. Examination of experimental conditions for the production of proton-rich and neutron-rich hypernuclei. Phys. Rev. C 2016, 94, 044616. [Google Scholar] [CrossRef]
- Sümmerer, K.; Brüchle, W.; Morrissey, D.J.; Schädel, M.; Szweryn, B.; Weifan, Y. Target fragmentation of Au and Th by 2.6 GeV protons. Phys. Rev. C 1990, 42, 2546–2561. [Google Scholar] [CrossRef]
- Sümmerer, K.; Blank, B. Modified empirical parametrization of fragmentation cross sections. Phys. Rev. C 2000, 61, 034607. [Google Scholar] [CrossRef]
- Sümmerer, K. Improved empirical parametrization of fragmentation cross sections. Phys. Rev. C 2012, 86, 014601. [Google Scholar] [CrossRef]
- Iwasa, N.; Weick, H.; Geissel, H. New features of the Monte-Carlo code MOCADI. Nucl. Instrum. Meth. B 2011, 269, 752–758. [Google Scholar] [CrossRef]
- Iwasa, N.; Geissel, H.; Münzenberg, G.; Scheidenberger, C.; Schwab, T.; Wollnik, H. MOCADI, a universal Monte Carlo code for the transport of heavy ions through matter within ion-optical systems. Nucl. Instrum. Meth. B 1997, 126, 284–289. [Google Scholar] [CrossRef]
- Buyukcizmeci, N.; Botvina, A.S.; Pochodzalla, J.; Bleicher, M. Mechanisms for the production of hypernuclei beyond the neutron and proton drip lines. Phys. Rev. C 2013, 88, 014611. [Google Scholar] [CrossRef]
- Botvina, A.S.; Gudima, K.K.; Steinheimer, J.; Bleicher, M.; Mishustin, I.N. Production of spectator hypermatter in relativistic heavy-ion collisions. Phys. Rev. C 2011, 84, 064904. [Google Scholar] [CrossRef]
- Botvina, A.S.; Pochodzalla, J. Production of hypernuclei in multifragmentation of nuclear spectator matter. Phys. Rev. C 2007, 76, 024909. [Google Scholar] [CrossRef]
- Fäldt, G.; Wilkin, C. Comparison of the near-threshold production of η-and K-mesons in proton-proton collisions. Z. Phys. A 1997, 357, 241–243. [Google Scholar] [CrossRef]
- The COSY-TOF Collaboration; Abdel-Bary, M.; Abdel-Samad, S.; Brinkmann, K.T.; Clement, H.; Dietrich, J.; Doroshkevich, E.; Dshemuchadse, S.; Ehrhardt, K.; Erhardt, A.; et al. Production of and hyperons in proton-proton collisions. Eur. Phys. J. A 2010, 46, 27–44. [Google Scholar] [CrossRef]

| Reaction | Target (cm) | 2nd Beam | (AGeV) | I (/s) | Yield (/s) | |
|---|---|---|---|---|---|---|
| 27Al + 10B | 9 | 19O | 1.82 | 5.2 | 0.06 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.71 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.36 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.10 | |
| 23Na + 11B | 15.5 | 12B | 1.79 | 11 | 0.06 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.49 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.29 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.00 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.50 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.18 | |
| 23Na + 11B | 15.5 | 12B | 1.79 | 11 | 0.08 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.58 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.24 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.56 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.35 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.01 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.50 | |
| 23Na + 11B | 15.5 | 12B | 1.79 | 11 | 0.25 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.39 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.74 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.29 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.49 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.26 | |
| 16O + 9Be | 5.5 | 14O | 1.93 | 5.5 | 0.74 | |
| 23Na + 11B | 15.5 | 12B | 1.79 | 11 | 0.34 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.20 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.61 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 1.24 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.25 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 1.29 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.92 | |
| 20Ne + 9Be | 5 | 18Ne | 1.92 | 5.0 | 0.16 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.22 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.88 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 1.12 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.82 | |
| 20Ne + 9Be | 2 | 17F | 1.97 | 5.7 | 0.44 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.07 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.25 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.49 | |
| 14N + 9Be | 5.5 | 12N | 1.94 | 5.1 | 0.29 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Escrig, S.; Rappold, C. Production Optimization of Exotic Hypernuclei via Heavy-Ion Beams at GSI-FAIR. Particles 2025, 8, 54. https://doi.org/10.3390/particles8020054
Escrig S, Rappold C. Production Optimization of Exotic Hypernuclei via Heavy-Ion Beams at GSI-FAIR. Particles. 2025; 8(2):54. https://doi.org/10.3390/particles8020054
Chicago/Turabian StyleEscrig, Samuel, and Christophe Rappold. 2025. "Production Optimization of Exotic Hypernuclei via Heavy-Ion Beams at GSI-FAIR" Particles 8, no. 2: 54. https://doi.org/10.3390/particles8020054
APA StyleEscrig, S., & Rappold, C. (2025). Production Optimization of Exotic Hypernuclei via Heavy-Ion Beams at GSI-FAIR. Particles, 8(2), 54. https://doi.org/10.3390/particles8020054







