Enhancement of Nuclear Fusion in Plasma Oscillation Systems
Abstract
:1. Introduction
2. Methods
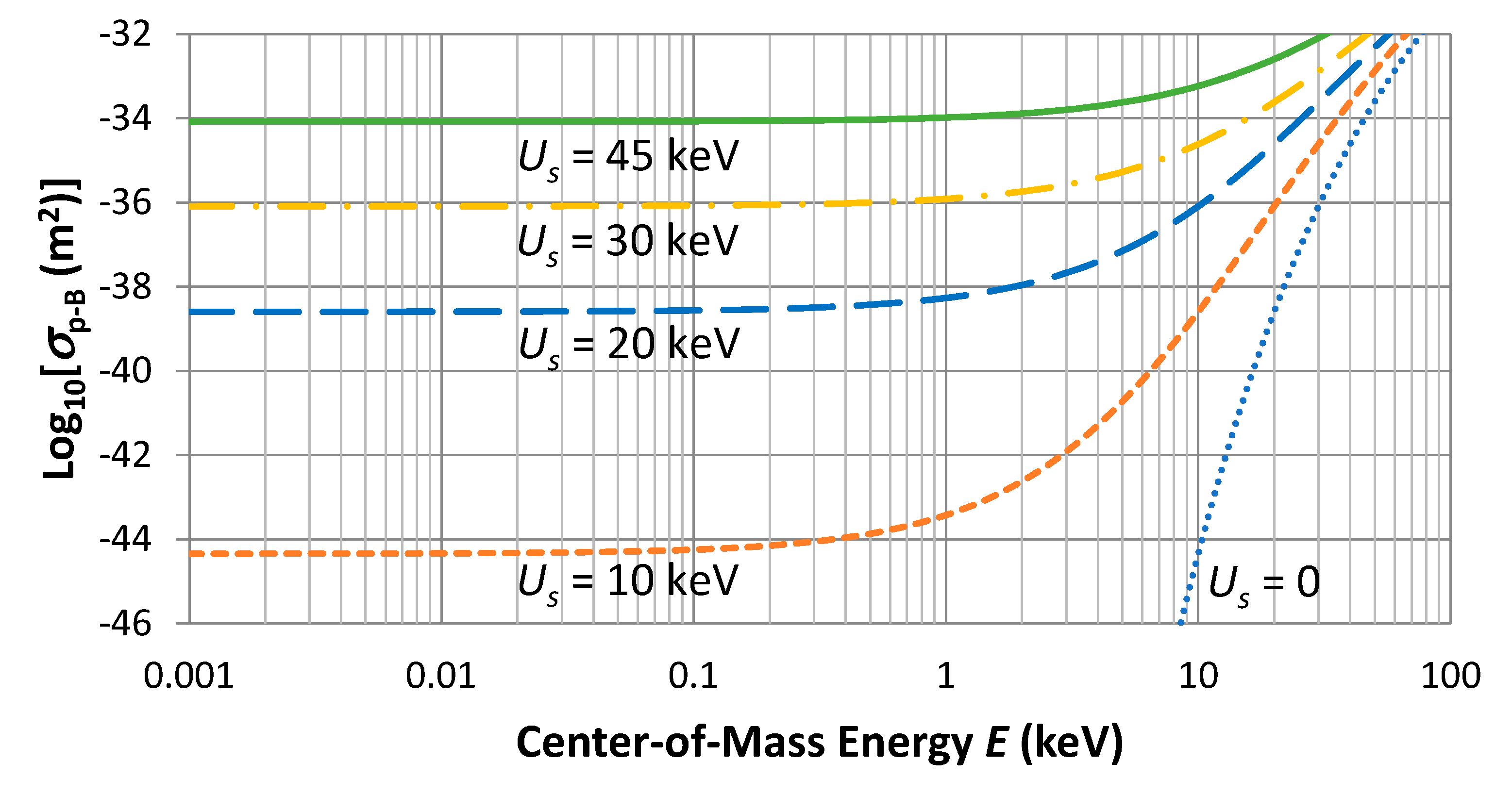
2.1. Effects of Electric Potential on Fusion Cross Section
2.2. Plasma Rotation and Negative Ions
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lawson, J.D. Some criteria for a power producing thermonuclear reactor. Proc. Phys. Soc. Sect. B 1956, 70, 6–10. [Google Scholar] [CrossRef]
- Glasstone, S. Controlled Nuclear Fusion; US Atomic Energy Commission: Washington, DC, USA, 1974. [Google Scholar]
- Labaune, C.; Baccou, C.; Depierreux, S.; Goyon, C.; Loisel, G.; Yahia, V.; Rafelski, J. Fusion reactions initiated by laser-accelerated particle beams in a laser-produced plasma. Nat. Commun. 2013, 4, 2506. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schiff, L.I. Quantum Mechanics; McGraw-Hill: New York, NY, USA, 1968; Section 21. [Google Scholar]
- Ichimaru, S.; Kitamura, H. Pycnonuclear reactions in dense astrophysical and fusion plasmas. Phys. Plasmas 1999, 6, 2649. [Google Scholar] [CrossRef]
- Becker, H.W.; Rolfs, C.; Trautvetter, H.P. Low-Energy Cross Sections for 11B(p, 3α). Z. Phys. 1987, A 327, 341. [Google Scholar]
- Lee, K.H.; Lee, L.C.; Wong, A.Y. Acceleration of ions and neutrals by a traveling electrostatic wave. Phys. Plasmas 2018, 25, 023113. [Google Scholar] [CrossRef]
- Rau, A.R.P. The negative ion of hydrogen. J. Astrophys. Astron. 1996, 17, 113. [Google Scholar] [CrossRef]
- Wong, A.Y.; Mamas, D.L.; Arnush, D. Negative ion plasmas. Phys. Fluids 1975, 18, 1489. [Google Scholar] [CrossRef]
- Alvarez, L.W.; Bradner, H.; Crawford, F.S.; Crawford, J.A.; Falk-Vairant, P.; Good, M.L.; Gow, J.D.; Rosenfeld, A.H.; Solmitz, F.; Stevenson, M.L.; et al. Catalysis of nuclear reactions by µ mesons. Phys. Rev. 1957, 105, 1127. [Google Scholar] [CrossRef]
- Jackson, J.D. Catalysis of nuclear reactions between hydrogen isotopes by µ-mesons. Phys. Rev. 1957, 106, 330. [Google Scholar] [CrossRef]
- Stix, T.H. The Theory of Plasma Waves; McGraw-Hill: New York, NY, USA, 1962. [Google Scholar]
- Wong, A.Y.; Shih, C.C. Approach to Nuclear Fusion Utilizing Dynamics of High-Density Electrons and Neutrals. arXiv 2019, arXiv:1908.11068. [Google Scholar]
- Wong, A.Y.; Gunn, A.; Chen, A.X.; Shih, C.C.; Guffey, M.J. Enhanced D-D Fusion Rates when the Coulomb Barrier Is Lowered by Electrons. arXiv 2021, arXiv:2106.12988. [Google Scholar]
- Guffey, M.J.; Wong, A.Y. Ponderomotive Screening of Nuclear Fusion Reactions Based on Localized Surface Plasmon Resonance. arXiv 2020, arXiv:2106.08127. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wong, A.Y.; Shih, C.-C. Enhancement of Nuclear Fusion in Plasma Oscillation Systems. Plasma 2022, 5, 176-183. https://doi.org/10.3390/plasma5010013
Wong AY, Shih C-C. Enhancement of Nuclear Fusion in Plasma Oscillation Systems. Plasma. 2022; 5(1):176-183. https://doi.org/10.3390/plasma5010013
Chicago/Turabian StyleWong, Alfred YiuFai, and Chun-Ching Shih. 2022. "Enhancement of Nuclear Fusion in Plasma Oscillation Systems" Plasma 5, no. 1: 176-183. https://doi.org/10.3390/plasma5010013
APA StyleWong, A. Y., & Shih, C.-C. (2022). Enhancement of Nuclear Fusion in Plasma Oscillation Systems. Plasma, 5(1), 176-183. https://doi.org/10.3390/plasma5010013




