Electrostatic Ion-Acoustic Shock Waves in a Magnetized Degenerate Quantum Plasma
Abstract
:1. Introduction
2. Model Equations
3. Derivation of the Burgers Equation
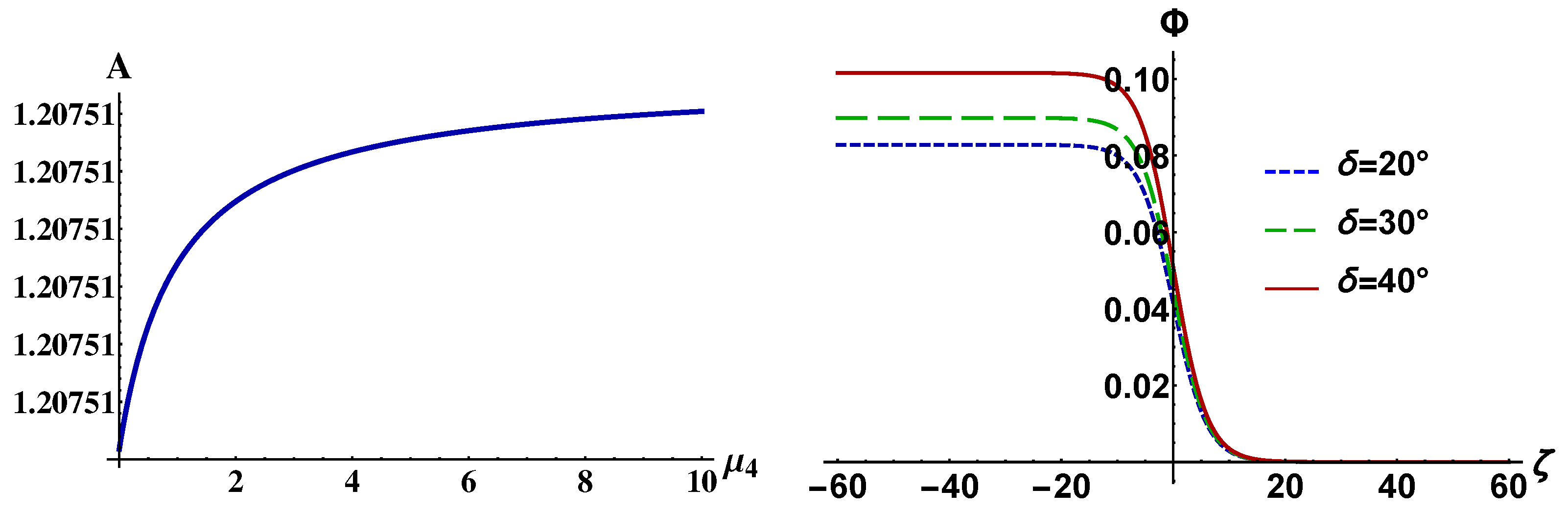
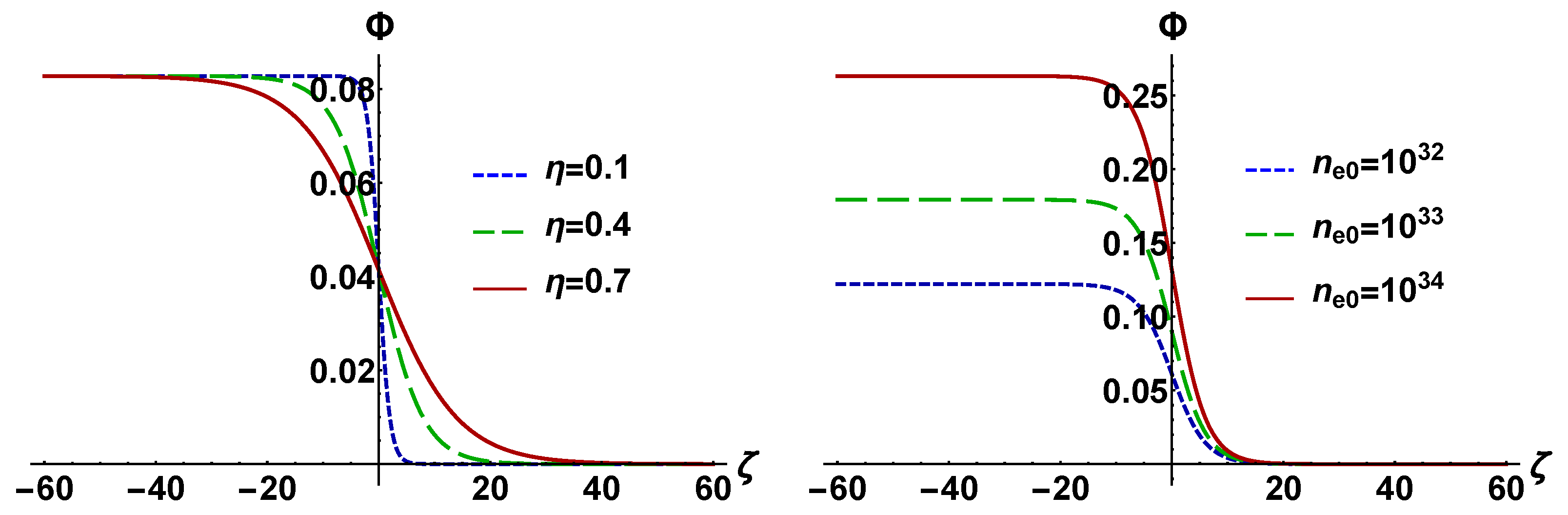
4. Results and Discussion
5. Conclusions
- The plasma model only supports positive shock potential under the consideration of both non-relativistic positively charged heavy and light ions (i.e., ), and ultra-relativistically degenerate electrons (i.e., );
- The increasing number density of ultra-relativistic electrons enhances the amplitude of the IASHWs;
- The increasing charge state and number density of the non-relativistic heavy and light ion species enhance the amplitude of the IASHWs associated with (i.e., );
- The steepness of the shock profile is decreased with the increasing kinematic viscosity () of ions;
- The amplitude of the shock profile is found to increase as the oblique angle increases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. First-Order and Second-Order Perturbation Terms
References
- Chandrasekhar, S. The density of white dwarf stars. Philos. Mag. 1982, 11, 592. [Google Scholar] [CrossRef]
- Chandrasekhar, S. The Maximum Mass of Ideal White Dwarfs. Astrophys. J. 1931, 74, 81. [Google Scholar] [CrossRef]
- Van Horn, H.M. Dense Astrophysical Plasmas. Science 1991, 252, 384. [Google Scholar] [CrossRef] [PubMed]
- Vanderburg, A.; Johnson, J.A.; Rappaport, S.; Bieryla, A.; Irwin, J.; Lewis, J.A.; Kipping, D.; Brown, W.R.; Dufour, P.; Ciardi, D.R.; et al. A disintegrating minor planet transiting a white dwarf. Nature 2015, 526, 546. [Google Scholar] [CrossRef] [Green Version]
- Witze, A. Space-station science ramps up. Nature 2014, 510, 196. [Google Scholar] [CrossRef] [Green Version]
- Fletcher, R.S.; Zhang, X.L.; Rolston, S.L. Observation of Collective Modes of Ultracold Plasmas. Phys. Rev. Lett. 2006, 96, 105003. [Google Scholar] [CrossRef] [Green Version]
- Killian, T.C. Plasma physics: Cool vibes. Nature 2006, 441, 297. [Google Scholar] [CrossRef]
- Sultana, S.; Schlickeiser, R. Fully nonlinear heavy ion-acoustic solitary waves in astrophysical degenerate relativistic quantum plasmas. Astrophys Space Sci. 2018, 363, 1. [Google Scholar] [CrossRef]
- Koester, D.; Chanmugam, G. Physics of white dwarf stars. Rep. Prog. Phys. 1990, 53, 837. [Google Scholar] [CrossRef]
- Koester, D. White dwarfs: Recent developments. Astron Astrophys. Rev. 2002, 11, 33. [Google Scholar] [CrossRef]
- Mamun, A.A. Degenerate pressure driven self-gravito-acoustic solitary waves in a self-gravitating degenerate quantum plasma system. Phys. Plasmas 2018, 25, 022307. [Google Scholar] [CrossRef]
- Mamun, A.A. Self-gravito-acoustic waves and their instabilities in a self-gravitating degenerate quantum plasma system. Contrib. Plasma Phys. 2019, 60, e201900080. [Google Scholar] [CrossRef]
- Islam, S.; Sultana, S.; Mamun, A.A. Ultra-low frequency shock dynamics in degenerate relativistic plasmas. Phys. Plasmas 2017, 24, 092308. [Google Scholar] [CrossRef]
- Islam, S.; Sultana, S.; Mamun, A.A. Envelope solitons in three-component degenerate relativistic quantum plasmas. Phys. Plasmas 2017, 24, 092115. [Google Scholar] [CrossRef]
- Mannan, A. 3-D Cylindrical waves in a self-gravitating degenerate quantum plasma. IEEE Trans. Plasma Sci. 2020, 48, 3791. [Google Scholar] [CrossRef]
- Atteya, A.; Behery, E.E.; El-Taibany, W.F. Ion acoustic shock waves in a degenerate relativistic plasma with nuclei of heavy elements. Eur. Phys. J. Plus 2017, 132, 109. [Google Scholar] [CrossRef]
- Abdelwahed, H.G.; El-Shewy, E.K.; Mahmoud, A.A. Time fractional effect on ion acoustic shock waves in ion-pair plasma. J. Exp. Theor. Phys. 2016, 122, 1111. [Google Scholar] [CrossRef]
- Tantawy, S.A. Effect of ion viscosity on dust ion-acoustic shock waves in a nonextensive magnetoplasma. Astrophys. Space Sci. 2016, 361, 249. [Google Scholar] [CrossRef]
- Dev, A.N.; Deka, M.K. Effect of non-thermality of electron and negative ion on the polarity of shock waves in a relativistic plasma. Phys. Plasmas 2018, 25, 072117. [Google Scholar] [CrossRef]
- Bains, A.S.; Tribeche, M. Oblique shock dynamics in nonextensive magnetized plasma. Astrophys. Space Sci. 2014, 351, 191. [Google Scholar] [CrossRef]
- Bains, A.S.; Panwar, A.; Ryu, C.M. Oblique shock waves in a two electron temperature superthermally magnetized plasma. Astrophys. Space Sci. 2015, 360, 17. [Google Scholar] [CrossRef]
- Hossen, M.M.; Nahar, L.; Alam, M.S.; Sultana, S.; Mamun, A.A. Ion-acoustic shock waves in a magnetized plasma featuring super-thermal distribution. High Energy Density Phys. 2017, 24, 9. [Google Scholar] [CrossRef]
- Shaukat, M.I. Obliquely propagating ion acoustic solitary structures in the presence of quantized magnetic field. Phys. Plasmas 2017, 24, 102301. [Google Scholar] [CrossRef]
- Ashraf, S.; Yasmin, S.; Asaduzzaman, M.; Mamun, A.A. Nonlinear propagation of dust-acoustic waves in a magnetized nonextensive dusty plasma. Astrophys. Space Sci. 2013, 348, 495. [Google Scholar] [CrossRef]
- Karpman, V.I. Nonlinear Waves in Dispersive Media; Pergamon Press: Oxford, UK, 1975. [Google Scholar]
- Hasegawa, A. Plasma Instabilities and Nonlinear Effects; Springer: Berlin, Germany, 1975. [Google Scholar]
- Sikta, J.N.; Chowdhury, N.A.; Mannan, A.; Sultana, S.; Mamun, A.A. Electrostatic Dust-Acoustic Rogue Waves in an Electron Depleted Dusty Plasma. Plasma 2021, 4. [Google Scholar] [CrossRef]
- Rahman, M.H.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Dust-Acoustic Rogue Waves in an Electron-Positron-Ion-Dust Plasma Medium. Galaxies 2021, 9, 31. [Google Scholar] [CrossRef]
- Jahan, S.; Haque, M.N.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Ion-Acoustic Rogue Waves in Double Pair Plasma Having Non-Extensive Particles. Universe 2021, 7, 63. [Google Scholar] [CrossRef]
- Noman, A.A.; Islam, M.K.; Hassan, M.; Banik, S.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Dust-Ion-Acoustic Rogue Waves in a Dusty Plasma Having Super-Thermal Electrons. Gases 2021, 1. [Google Scholar] [CrossRef]
- Akter, J.; Chowdhury, N.A.; Mannan, A.; Mamun, A.A. Dust–Acoustic Envelope Solitons in an Electron-Depleted Plasma. Plasma Phys Rep. 2021, 47, 725. [Google Scholar] [CrossRef]
- Zamanian, J.; Marklund, M.; Brodin, G. Exchange effects in plasmas: The case of low-frequency dynamics. Phys. Rev. E 2013, 88, 063105. [Google Scholar] [CrossRef] [Green Version]
- Ourabah, K.; Tribeche, M. Quantum ion-acoustic solitary waves: The effect of exchange correlation. Phys. Rev. E 2013, 88, 045101. [Google Scholar] [CrossRef] [PubMed]
- Shukla, P.K.; Stenflo, L.; Bingham, B. Shielding of a slowly moving test charge in a quantum plasma. Phys. Lett. A 2006, 359, 218. [Google Scholar] [CrossRef]
- Pines, D. Classical and quantum plasmas. J. Nucl. Energy Part C Plasma Phys. 1961, 2, 5. [Google Scholar] [CrossRef]
- Stenflo, L.; Shukla, P.K. Wave-wave interactions in plasmas. AIP Conf. Proc. 2009, 1177, 4. [Google Scholar]
- El-Labany, S.K.; El-Taibany, W.F.; Behery, E.E.; Abd-Elbaki, R. Oblique collision of ion acoustic solitons in a relativistic degenerate plasma. Sci. Rep. 2020, 10, 16152. [Google Scholar] [CrossRef]
- Washimi, H.; Tanuti, T. Propagation of Ion-Acoustic Solitary Waves of Small Amplitude. Phys. Rev. Lett. 1966, 17, 996. [Google Scholar] [CrossRef]
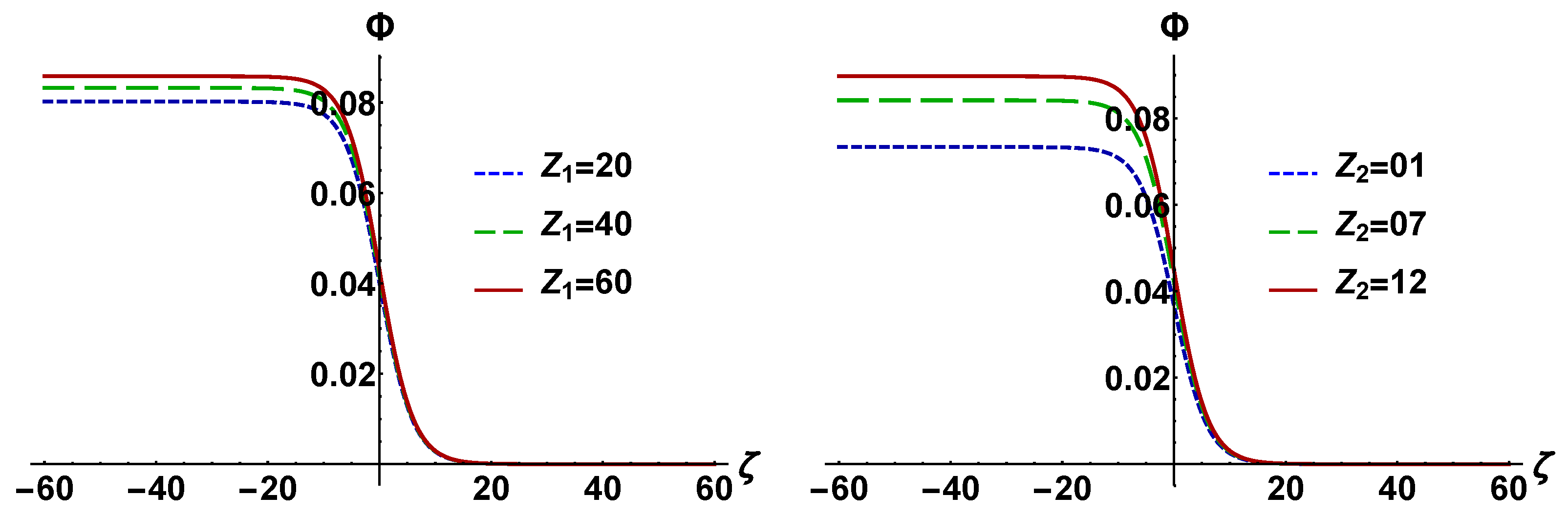

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jahan, S.; Sharmin, B.E.; Chowdhury, N.A.; Mannan, A.; Roy, T.S.; Mamun, A.A. Electrostatic Ion-Acoustic Shock Waves in a Magnetized Degenerate Quantum Plasma. Plasma 2021, 4, 426-434. https://doi.org/10.3390/plasma4030031
Jahan S, Sharmin BE, Chowdhury NA, Mannan A, Roy TS, Mamun AA. Electrostatic Ion-Acoustic Shock Waves in a Magnetized Degenerate Quantum Plasma. Plasma. 2021; 4(3):426-434. https://doi.org/10.3390/plasma4030031
Chicago/Turabian StyleJahan, Sharmin, Booshrat E. Sharmin, Nure Alam Chowdhury, Abdul Mannan, Tanu Shree Roy, and A A Mamun. 2021. "Electrostatic Ion-Acoustic Shock Waves in a Magnetized Degenerate Quantum Plasma" Plasma 4, no. 3: 426-434. https://doi.org/10.3390/plasma4030031
APA StyleJahan, S., Sharmin, B. E., Chowdhury, N. A., Mannan, A., Roy, T. S., & Mamun, A. A. (2021). Electrostatic Ion-Acoustic Shock Waves in a Magnetized Degenerate Quantum Plasma. Plasma, 4(3), 426-434. https://doi.org/10.3390/plasma4030031







