Proposed Methods for Testing and Comparing the Emulsifying Properties of Proteins from Animal, Plant, and Alternative Sources
Abstract
:1. Introduction
2. Physicochemical Principles of Emulsifier Performance
2.1. Formation of Emulsions
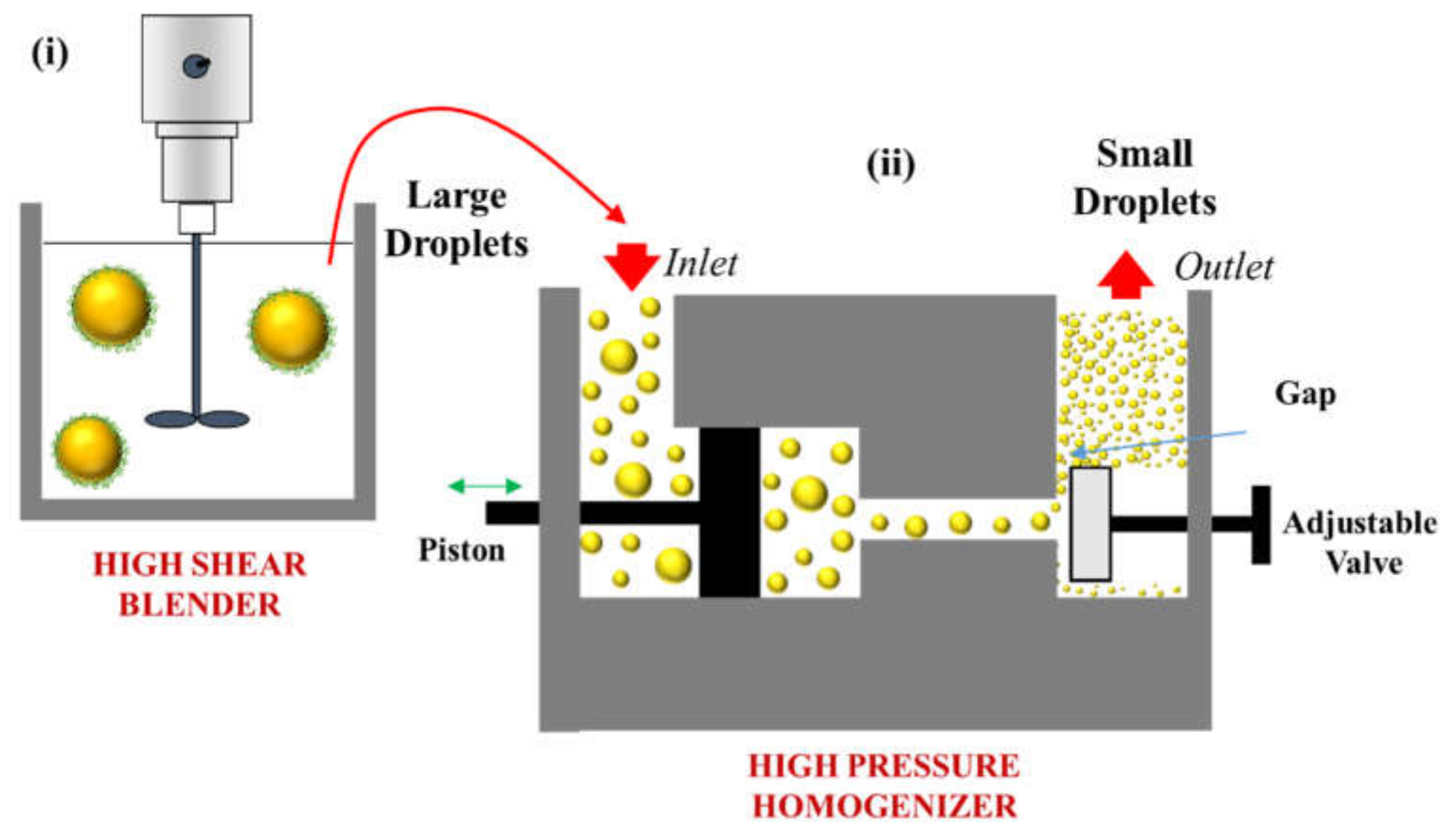
2.1.1. Principles of Homogenization
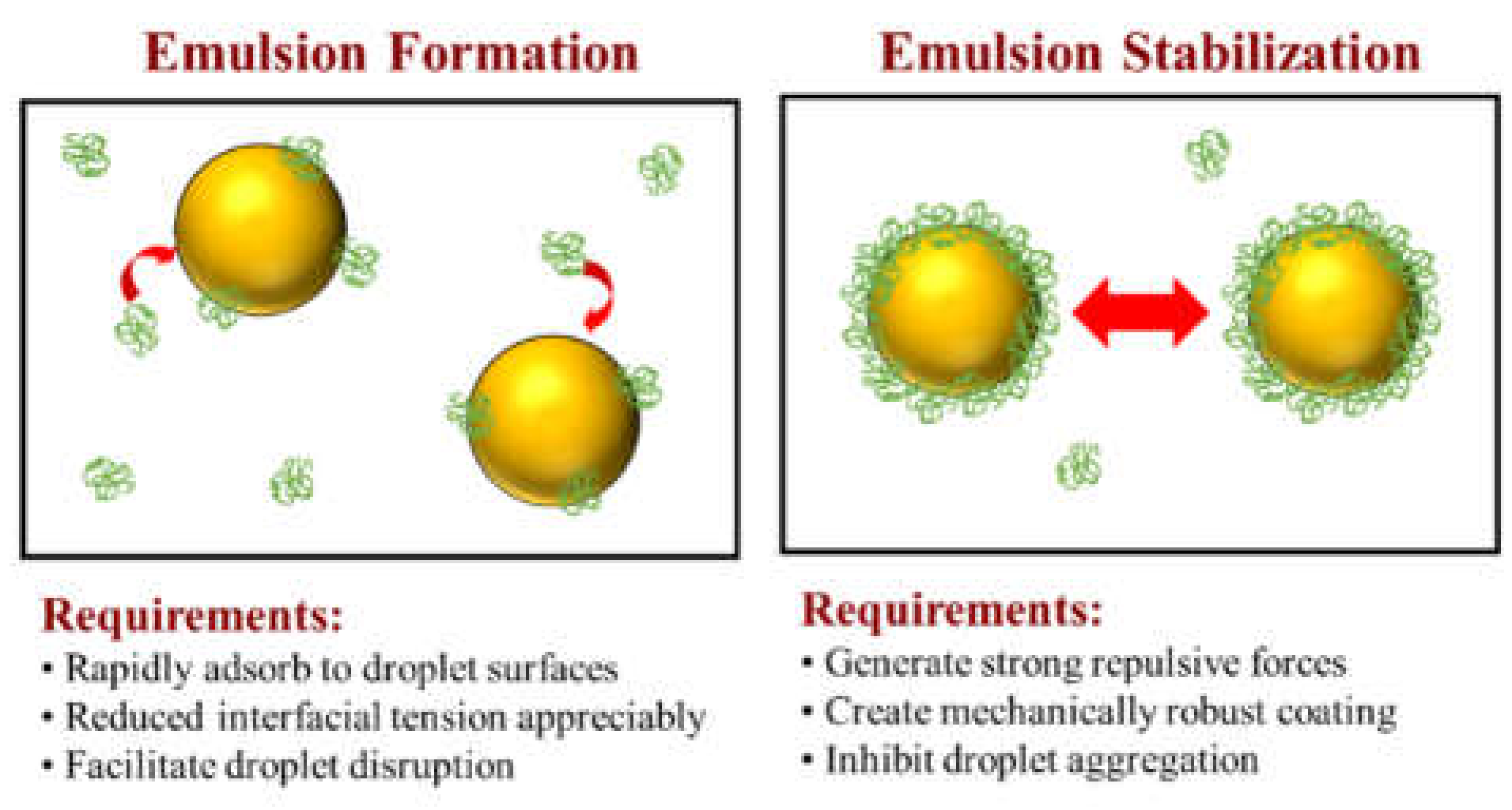
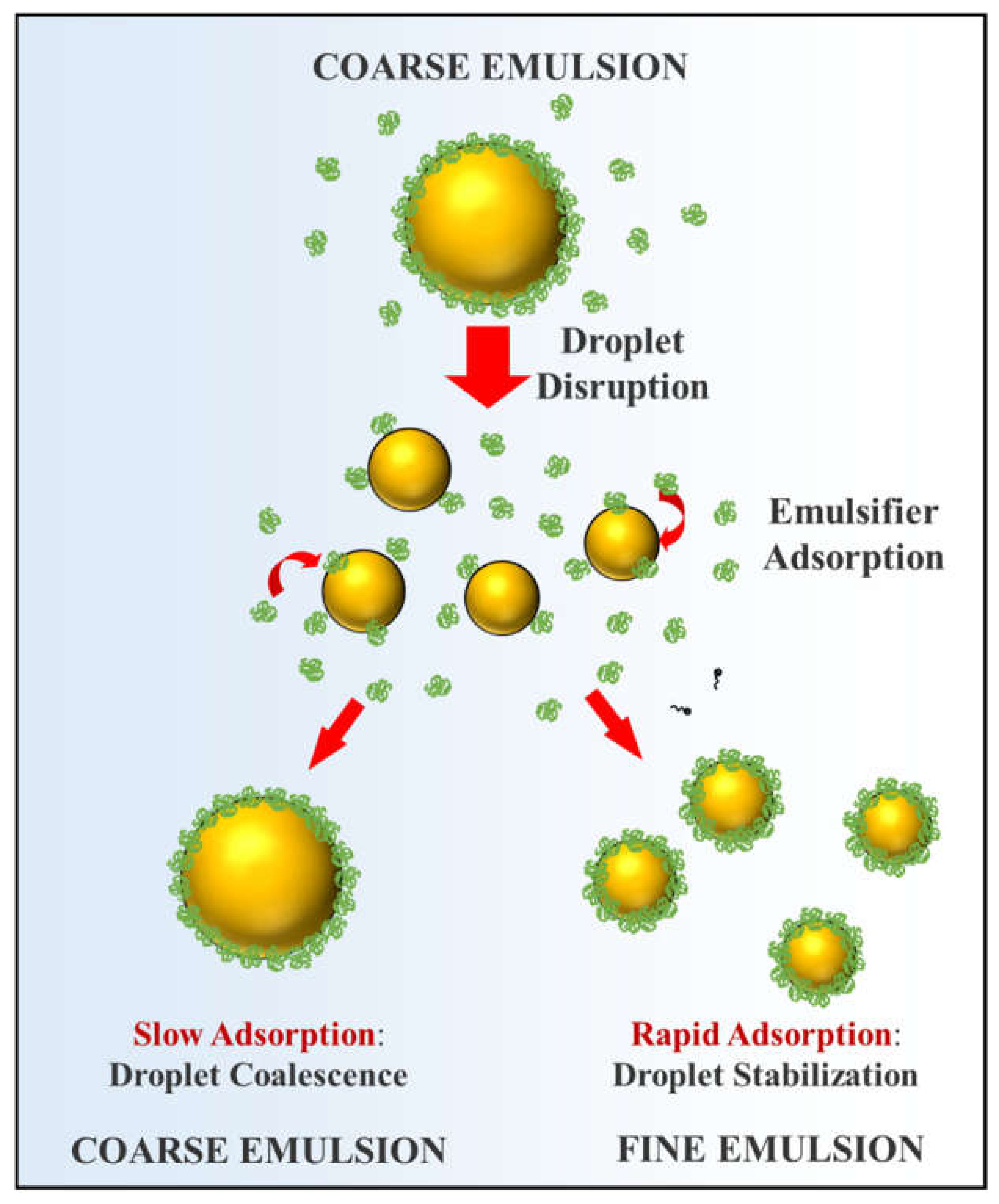
2.1.2. Role of Emulsifier in Emulsion Formation
Promotion of Droplet Disruption
Inhibition of Droplet Coalescence
Factors Affecting Emulsifier Performance
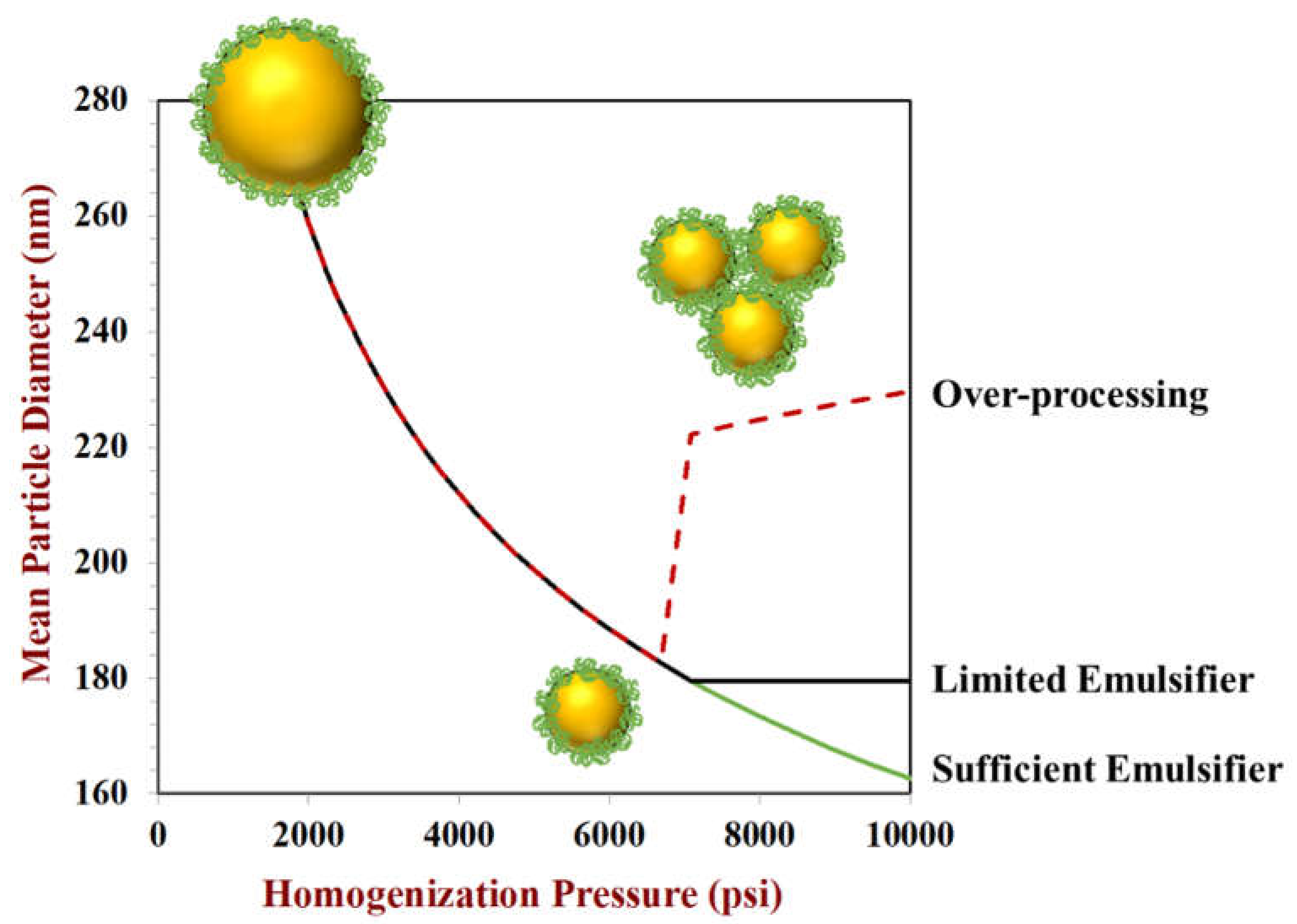
2.1.3. Role of Homogenization Conditions
2.2. Stabilization of Emulsions
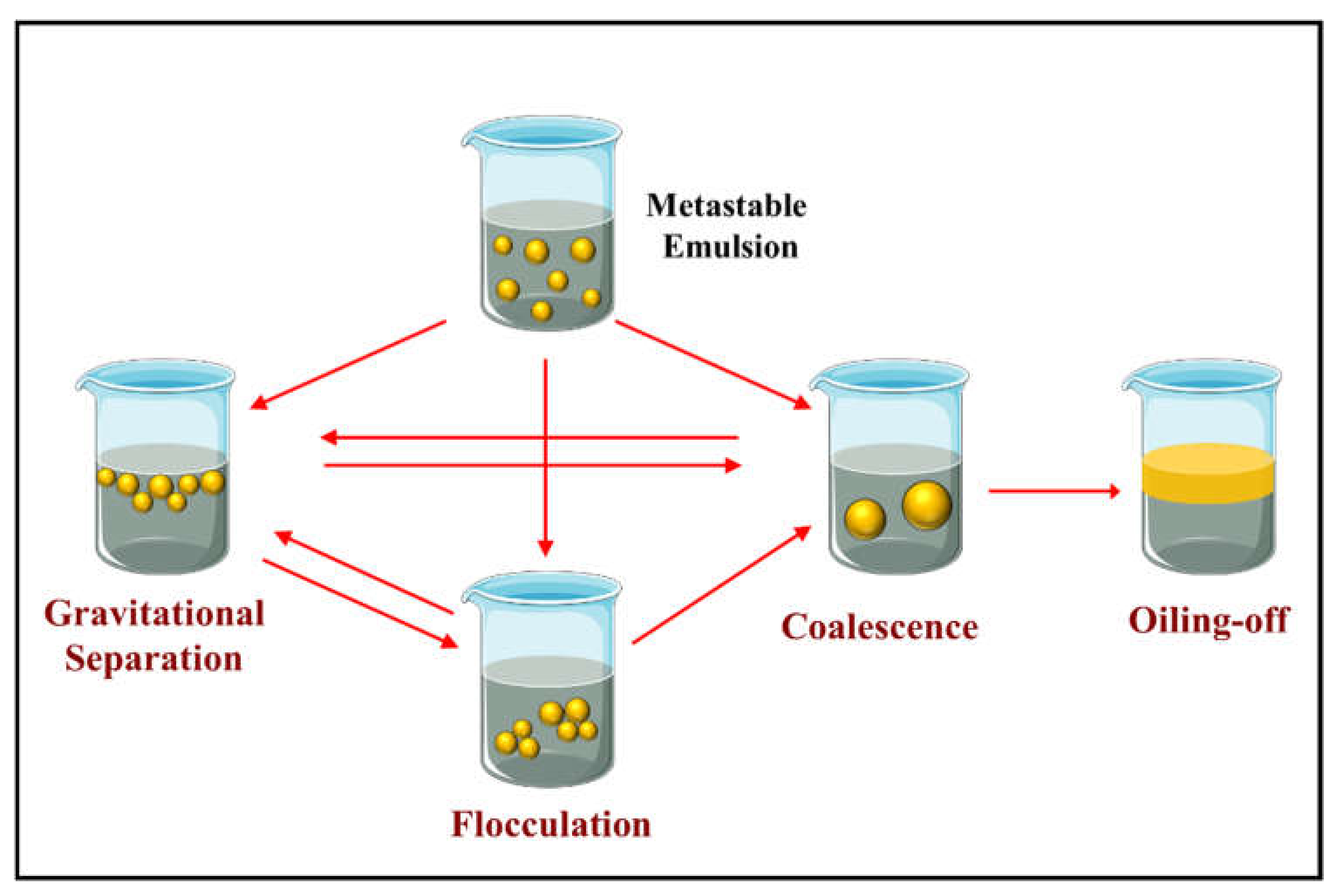
2.2.1. Gravitational Separation
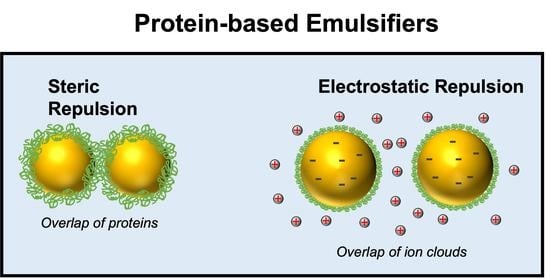
2.2.2. Droplet Aggregation
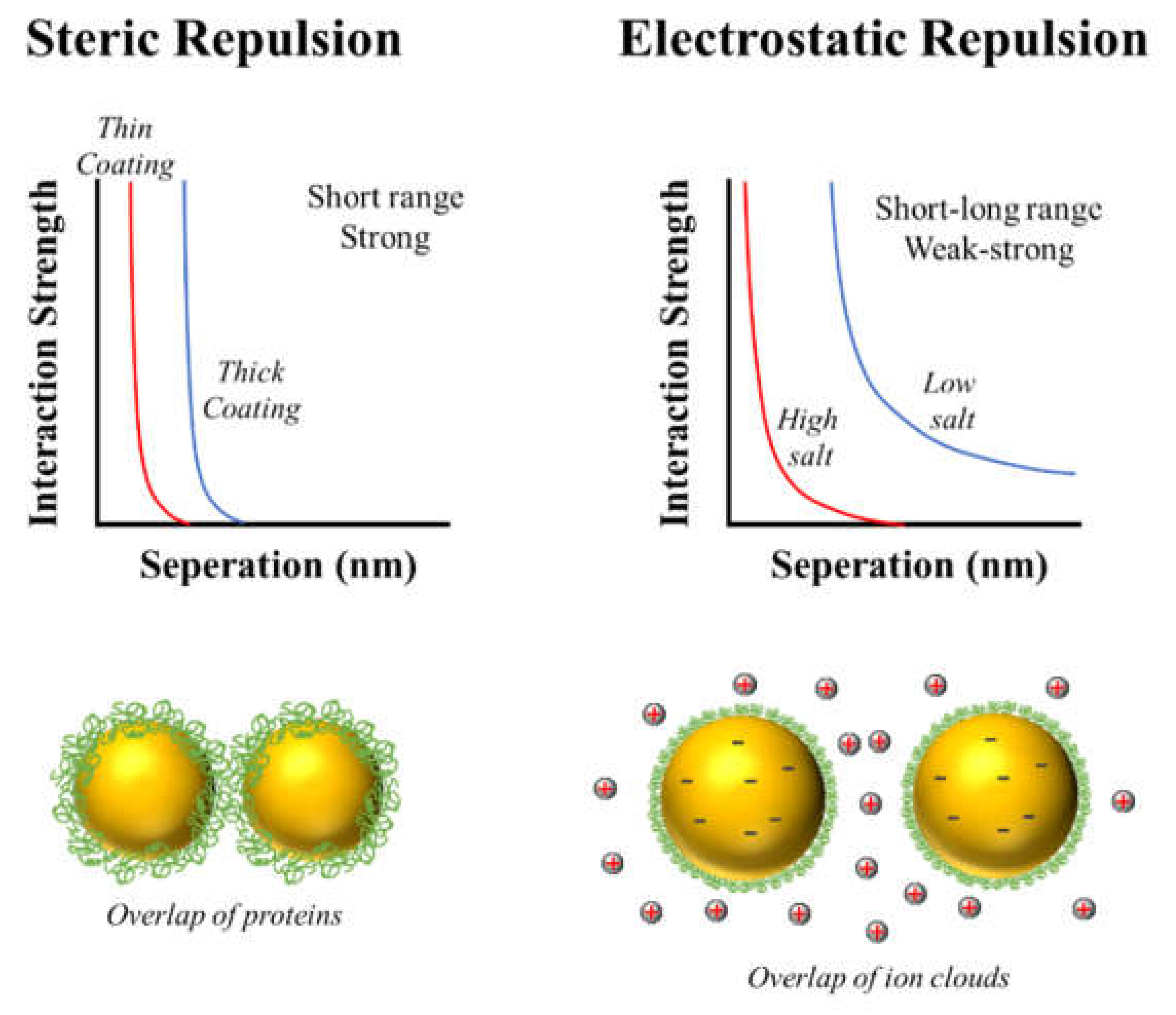
Electrostatic Interactions
Steric Repulsion
Hydrophobic Interactions
Covalent Interactions
2.2.3. Overall Interactions
2.2.4. Ostwald Ripening
2.3. Desirable Attributes of Protein-Based Emulsifiers
3. Recommended Protocols for Testing and Comparing Emulsifier Performance
3.1. Initial Ingredient Properties
3.1.1. Proximate Analysis
3.1.2. Protein Composition
3.1.3. Protein Solubility
3.1.4. Protein Aggregation State
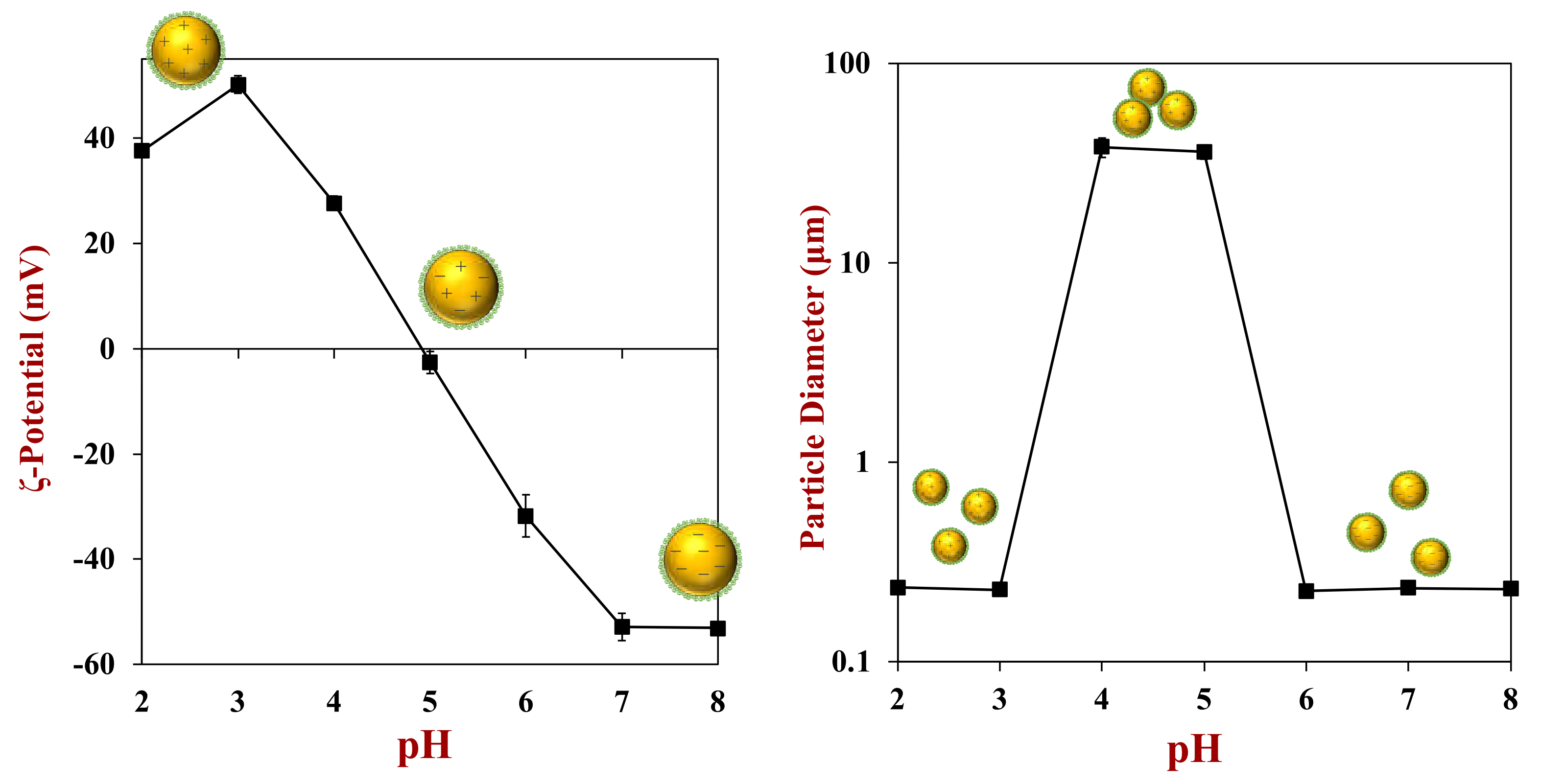
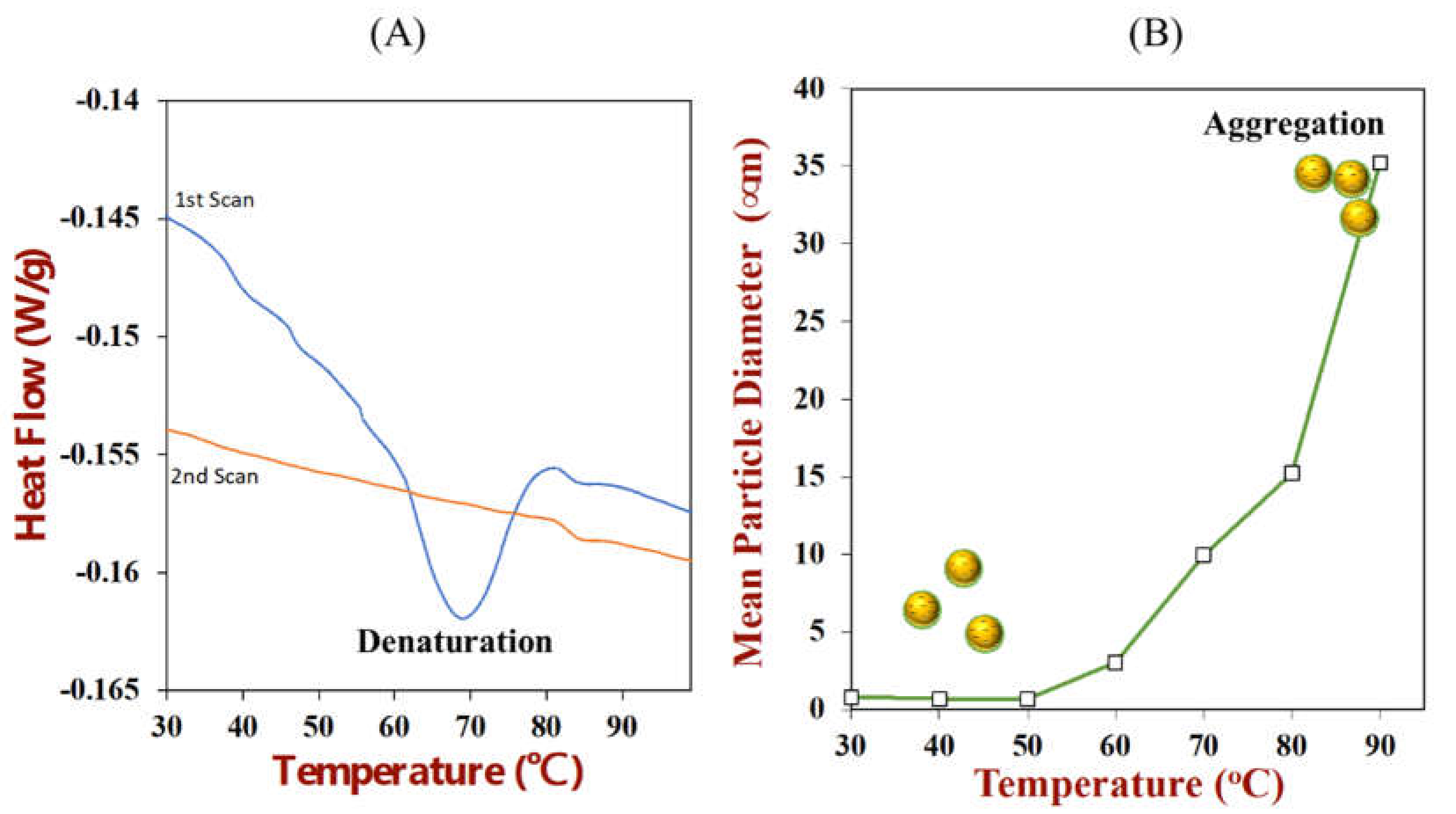
3.1.5. Protein Denaturation
3.2. Impact of Emulsifier on Emulsion Formation
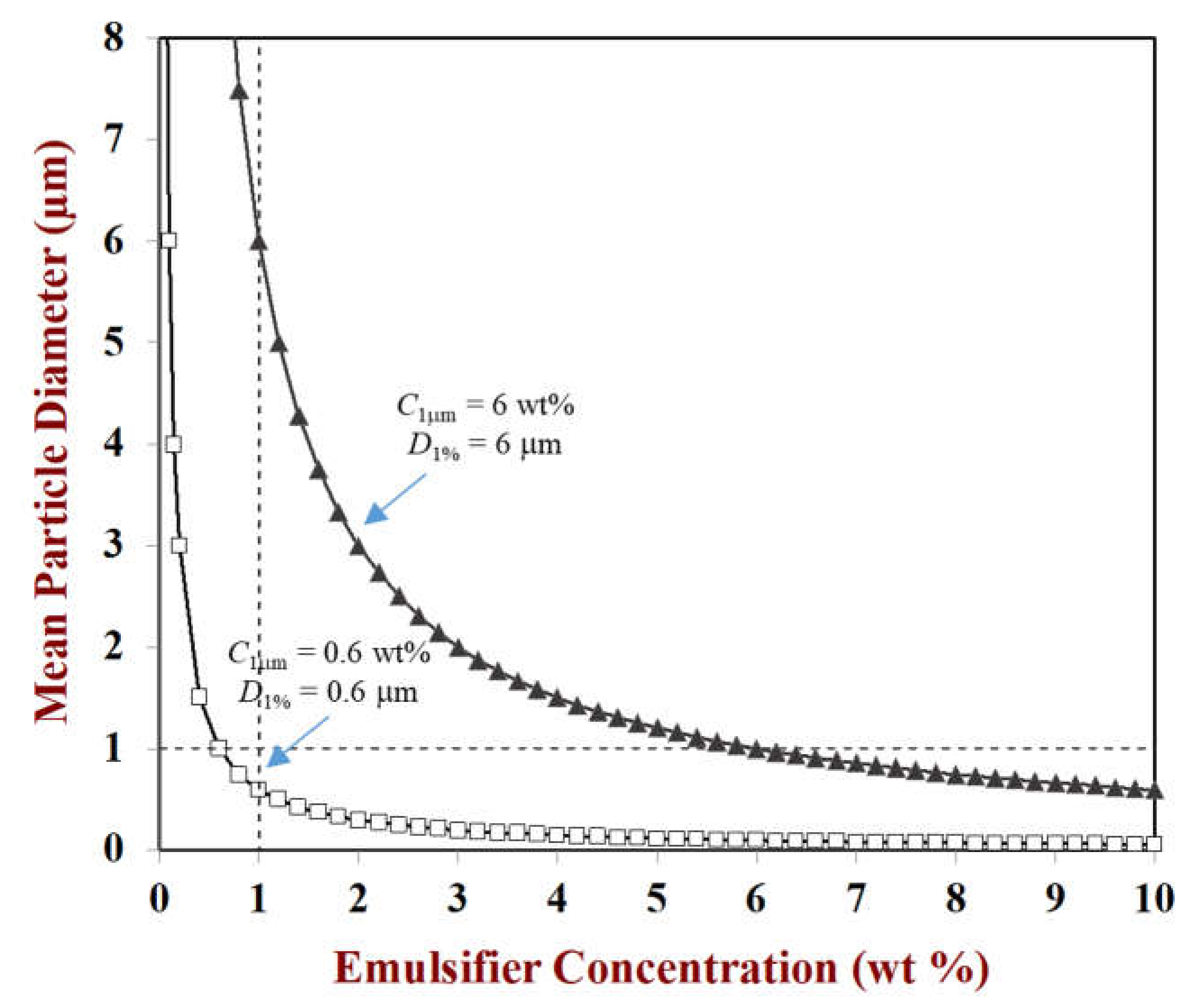
3.2.1. Emulsifying Capacity
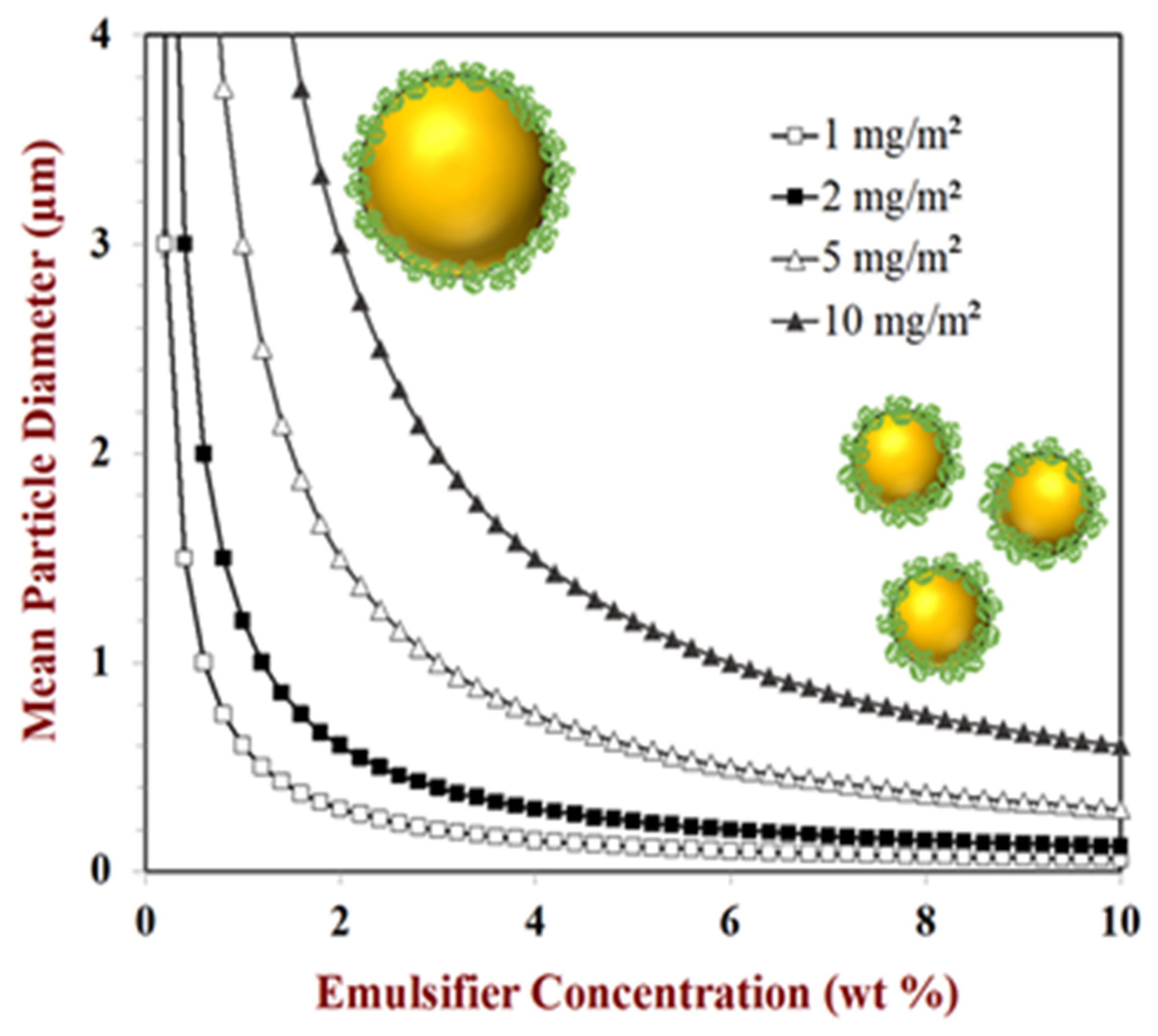
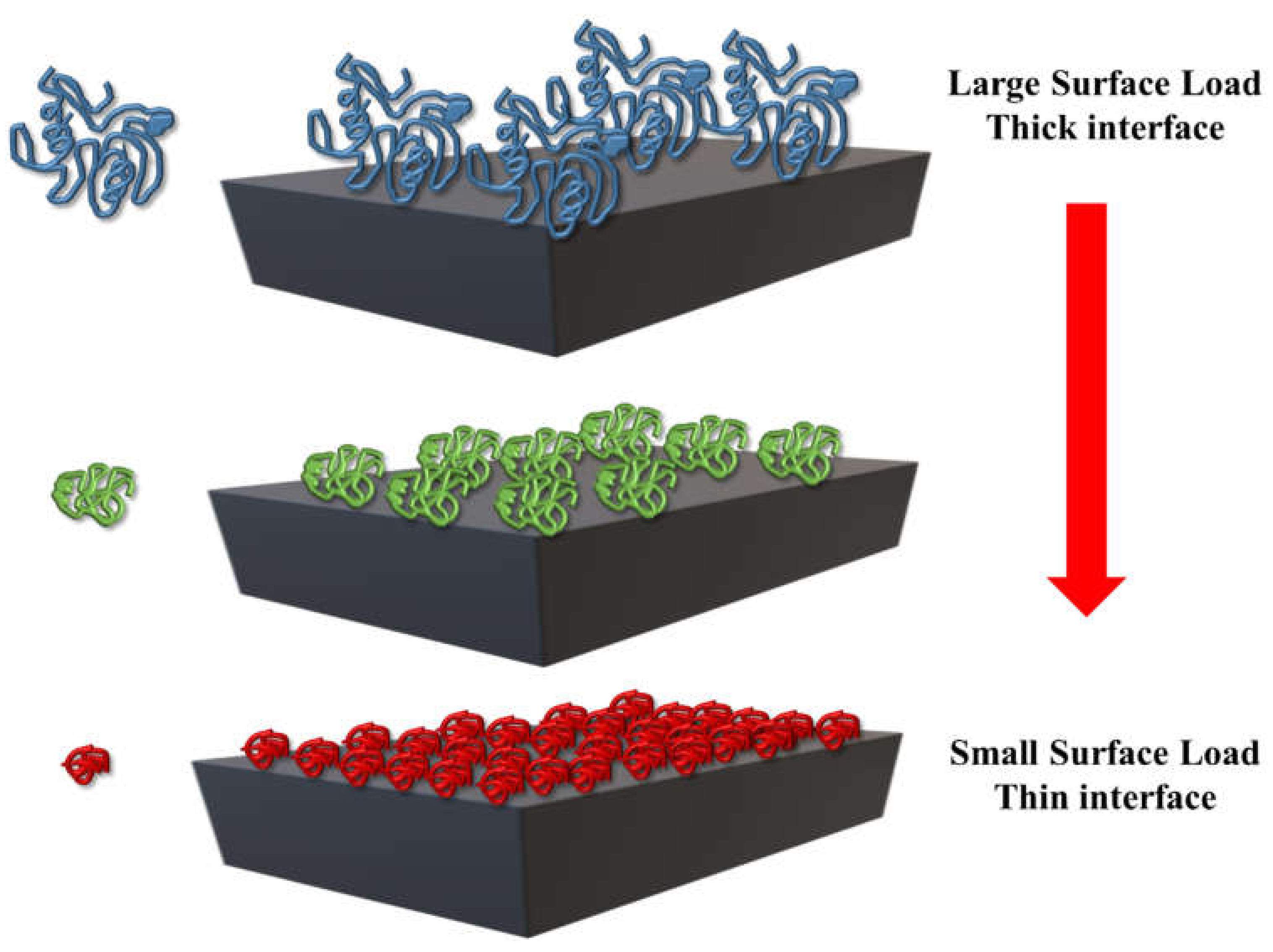
3.2.2. Surface Load
Definition
Theoretical Calculations
Experimental Determination
Importance of Surface Load
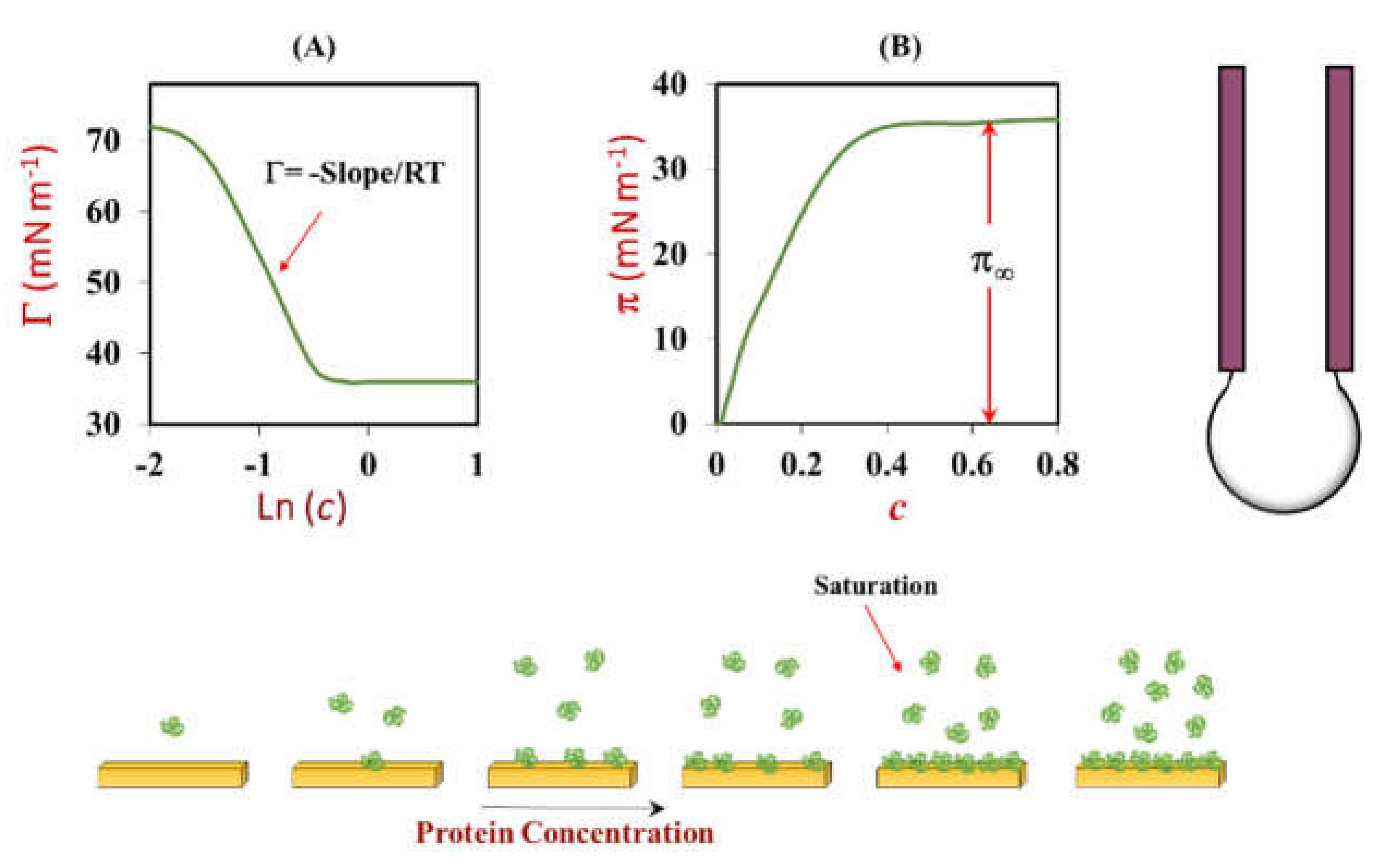
3.2.3. Saturation Surface Pressure
3.2.4. Surface Activity
3.2.5. Adsorption Kinetics
3.3. Impact of Emulsifier on Emulsion Stability
3.3.1. Analytical Instruments Providing Information Relevant to Colloidal Interactions
Interfacial Layer Thickness
Interfacial Layer Charge
Interfacial Rheology
3.3.2. Analytical Instruments for Characterizing Emulsion Stability
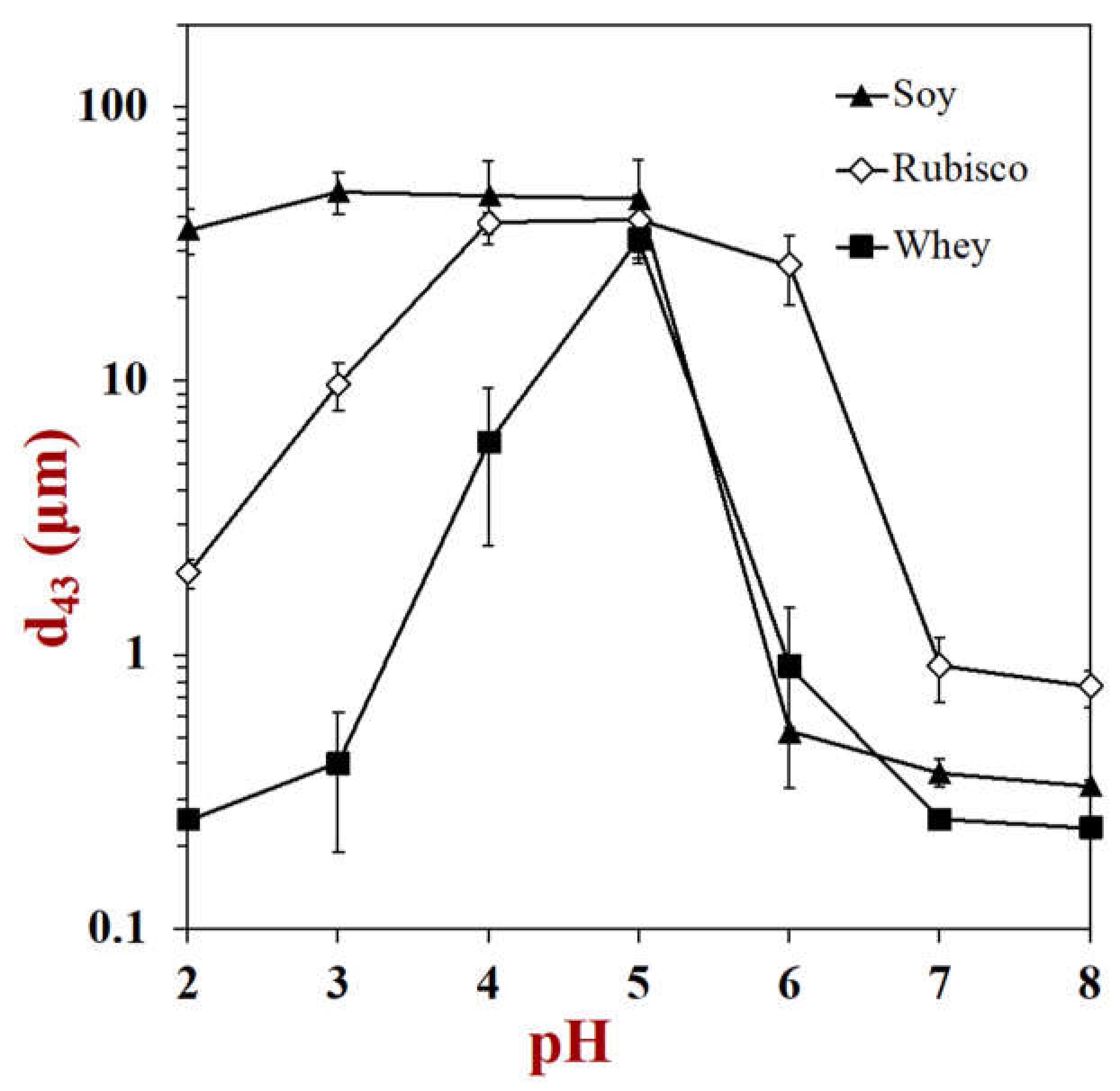
Particle Size and Aggregation State
- Aggregation type: It is difficult to establish whether an observed increase in particle size in an emulsion is due to flocculation or coalescence. The instrument reports a particle size distribution and mean particle diameter, but it is not possible to ascertain the origin of droplet aggregation from this data alone. In some cases, however, insights can be obtained about the aggregation type. For instance, the particle size distribution of a protein-stabilized emulsion can be measured, and then a small molecule surfactant (e.g., 1% SDS or Tween 20) is added. The emulsion is then incubated for a few hours and the particle size distribution is measured again. During incubation, the surfactant adsorbs to the fat droplet surfaces and displaces the original emulsifiers, thereby disrupting any flocs. If the particle size distribution does not change after the surfactant is added, then it is assumed that droplet aggregation is due to coalescence. Conversely, if the size of the particles decreases appreciably after the surfactant is added, then it can be assumed that droplet aggregation is due to flocculation.
- Actual particle size: The instrument software assumes that the objects that scatter light are isolated homogeneous spheres with well-defined refractive indices, which is not the case for flocculated emulsions. Flocculated emulsions contain heterogeneous irregular particles that do not have a well-defined refractive index. Consequently, the results reported by the instrument do not reflect the true size of the particles within the emulsion. In this case, the results should only be used to provide information about whether aggregation is occurring or not.
- Dilution effects: Typically, emulsions must be diluted prior to analysis by light scattering to obtain a sufficiently strong signal while avoiding multiple scattering. As mentioned earlier, it is critical to carefully dilute the emulsions so as not to alter their aggregation state. If the droplets in flocs are only held together by weak attractive forces (such as depletion forces), then they may be disrupted when they are diluted and stirred. As a result, the particle size measured for the analyzed emulsion may be smaller than that in the actual emulsion. For protein-stabilized emulsions, the pH and ionic strength of the aqueous phase used to dilute the samples should be similar to those found in the original sample to minimize any changes in the colloidal interactions between the droplets.
Gravitational Separation
3.3.3. Emulsion Stability Testing Protocols
Emulsion Stability Index
- The particle size of an emulsion may increase due to various physicochemical mechanisms including flocculation, coalescence, and Ostwald ripening. The ESI or EII value does not provide insights into which of these mechanisms is dominant, which can be important for developing effective strategies to improve emulsion stability.
- Typically, the mean particle diameter does not increase linearly with time. Instead, the particle size may increase during the initial stages of storage and then reach a constant value. As a result, the ESI or EII value depends on the time when the particle size is measured. For this reason, it is useful to stipulate a fixed storage time when comparing the effectiveness of different emulsifiers to stabilize emulsions, e.g., 24 h, 1 week or 1 month. Based on our practical experience with protein-stabilized emulsions, we recommend an incubation time of 24 h. This is usually sufficient to observe increases in droplet aggregation, without causing concerns with microbial growth.
- The particle size distribution of an emulsion often changes from mono-modal (single-peaked) to multi-modal (multi-peaked) during storage, depending on the nature of the instability mechanism. We recommend that the time-dependence of the particle size distribution should be measured to provide insights into the origins of emulsion instability.
- The values of the ESI and EII parameters depend on the type of mean particle diameter used in the calculations, such as d10, d32 or d43. Consequently, it is important to use the same mean particle diameter when comparing different emulsifiers. We recommend using the d43 value as this is most sensitive to particle aggregation.
- The rate of increase in the particle size over time depends on the initial droplet size, droplet concentration, and continuous phase rheology in the emulsion being tested. These factors should therefore be standardized when comparing different emulsifiers. We recommend using an initial droplet diameter of 100–500 nm (to avoid creaming during storage), a droplet concentration of 10%, and pure water or buffer solution as the aqueous phase.
- 0 < EII < 0.05: Highly stable
- 0.05 < EII < 0.5: Moderately stable
- 0.5 < EII < 5.0: Moderately unstable
- EII > 5.0: Highly unstable
Environmental Stress Tests
4. Application of Proposed Standardized Tests
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Petersen, K.S.; Flock, M.R.; Richter, C.K.; Mukherjea, R.; Slavin, J.L.; Kris-Etherton, P.M. Healthy Dietary Patterns for Preventing Cardiometabolic Disease: The Role of Plant-Based Foods and Animal Products. Curr. Dev. Nutr. 2017, 1, 12. [Google Scholar] [CrossRef] [Green Version]
- Singh, M.; Trivedi, N.; Enamala, M.K.; Kuppam, C.; Parikh, P.; Nikolova, M.P.; Chavali, M. Plant-based meat analogue (PBMA) as a sustainable food: A concise review. Eur. Food Res. Technol. 2021, 247, 2499–2526. [Google Scholar] [CrossRef]
- McClements, D.J.; Grossmann, L. The science of plant-based foods: Constructing next-generation meat, fish, milk, and egg analogs. Compr. Rev. Food Sci. Food Saf. 2021, 20, 4049–4100. [Google Scholar] [CrossRef] [PubMed]
- Grossmann, L.; Weiss, J. Alternative Protein Sources as Technofunctional Food Ingredients. Annu. Rev. Food Sci. Technol. 2021, 12, 93–117. [Google Scholar] [PubMed]
- Onwezen, M.C.; Bouwman, E.P.; Reinders, M.J.; Dagevos, H. A systematic review on consumer acceptance of alternative proteins: Pulses, algae, insects, plant-based meat alternatives, and cultured meat. Appetite 2021, 159, 1–5. [Google Scholar] [CrossRef]
- Tso, R.; Lim, A.J.; Forde, C.G. A Critical Appraisal of the Evidence Supporting Consumer Motivations for Alternative Proteins. Foods 2021, 10, 24. [Google Scholar] [CrossRef]
- Loveday, S.M. Food Proteins: Technological, Nutritional, and Sustainability Attributes of Traditional and Emerging Proteins. Annu. Rev. Food Sci. Technol. 2019, 10, 311–339. [Google Scholar]
- Craddock, J.C.; Genoni, A.; Strutt, E.F.; Goldman, D.M. Limitations with the Digestible Indispensable Amino Acid Score (DIAAS) with Special Attention to Plant-Based Diets: A Review. Curr. Nutr. Rep. 2021, 10, 93–98. [Google Scholar] [CrossRef]
- McClements, D.J.; Grossmann, L. A brief review of the science behind the design of healthy and sustainable plant-based foods. Npj Sci. Food 2021, 5, 1. [Google Scholar] [CrossRef]
- Comunian, T.A.; Drusch, S.; Brodkorb, A. Advances of plant-based structured food delivery systems on the in vitro digestibility of bioactive compounds. Crit. Rev. Food Sci. 2021, 1–21. [Google Scholar] [CrossRef]
- Tan, Y.B.; McClements, D.J. Plant-Based Colloidal Delivery Systems for Bioactives. Molecules 2021, 26, 6895. [Google Scholar] [CrossRef] [PubMed]
- McClements, D.J.; Bai, L.; Chung, C. Recent Advances in the Utilization of Natural Emulsifiers to Form and Stabilize Emulsions. Annu Rev. Food Sci. Technol. 2017, 28, 205–236. [Google Scholar]
- McClements, D.J.; Gumus, C.E. Natural emulsifiers—Biosurfactants, phospholipids, biopolymers, and colloidal particles: Molecular and physicochemical basis of functional performance. Adv. Colloid Interface Sci. 2016, 234, 3–26. [Google Scholar] [CrossRef] [Green Version]
- McClements, D.J.; Rao, J. Food-Grade Nanoemulsions: Formulation, Fabrication, Properties, Performance, Biological Fate, and Potential Toxicity. Crit. Rev. Food Sci. Nutr. 2011, 51, 285–330. [Google Scholar] [CrossRef] [PubMed]
- Santana, R.C.; Perrechil, F.A.; Cunha, R.L. High-and Low-Energy Emulsifications for Food Applications: A Focus on Process Parameters. Food Eng. Rev. 2013, 5, 107–122. [Google Scholar] [CrossRef]
- Hakansson, A. Emulsion Formation by Homogenization: Current Understanding and Future Perspectives. Annu. Rev. Food Sci. Technol. 2019, 10, 239–258. [Google Scholar] [PubMed]
- Hakansson, A.; Rayner, M. General Principles of Nanoemulsion Formation by High-Energy Mechanical Methods. In Nanoemulsions; Academic Press: Cambridge, MA, USA, 2018; pp. 103–139. [Google Scholar]
- McClements, D.J. Food Emulsions: Principles, Practices, and Techniques, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- O’Sullivan, J.; Murray, B.; Flynn, C.; Norton, I. The effect of ultrasound treatment on the structural, physical and emulsifying properties of animal and vegetable proteins. Food Hydrocoll. 2016, 53, 141–154. [Google Scholar] [CrossRef] [Green Version]
- Paez-Hernandez, G.; Mondragon-Cortez, P.; Espinosa-Andrews, H. Developing curcumin nanoemulsions by high-intensity methods: Impact of ultrasonication and microfluidization parameters. Lwt Food Sci. Technol. 2019, 111, 291–300. [Google Scholar] [CrossRef]
- Rampon, V.; Riaublanc, A.; Anton, M.; Genot, C.; McClements, D.J. Evidence that homogenization of BSA-stabilized hexadecane-in-water emulsions induces structure modification of the nonadsorbed protein. J. Agric. Food Chem. 2003, 51, 5900–5905. [Google Scholar] [CrossRef]
- Miriani, M.; Keerati-u-rai, M.; Corredig, M.; Iametti, S.; Bonomi, F. Denaturation of soy proteins in solution and at the oil-water interface: A fluorescence study. Food Hydrocoll. 2011, 25, 620–626. [Google Scholar] [CrossRef]
- Hinderink, E.B.A.; Berton-Carabin, C.C.; Schroen, K.; Riaublanc, A.; Houinsou-Houssou, B.; Boire, A.; Genot, C. Conformational Changes of Whey and Pea Proteins upon Emulsification Approached by Front-Surface Fluorescence. J. Agric. Food Chem. 2021, 69, 6601–6612. [Google Scholar] [CrossRef] [PubMed]
- Zhai, J.L.; Day, L.; Aguilar, M.I.; Wooster, T.J. Protein folding at emulsion oil/water interfaces. Curr. Opin. Colloid Interface Sci. 2013, 18, 257–271. [Google Scholar] [CrossRef]
- Gumus, C.E.; Decker, E.A.; McClements, D.J. Impact of legume protein type and location on lipid oxidation in fish oil-in-water emulsions: Lentil, pea, and faba bean proteins. Food Res. Int. 2017, 100, 175–185. [Google Scholar] [CrossRef] [PubMed]
- Hossain, K.M.Z.; Deeming, L.; Edler, K.J. Recent progress in Pickering emulsions stabilised by bioderived particles. Rsc Adv. 2021, 11, 39027–39044. [Google Scholar] [CrossRef]
- Tang, C.H. Emulsifying properties of soy proteins: A critical review with emphasis on the role of conformational flexibility. Crit. Rev. Food Sci. Nutr. 2017, 57, 2636–2679. [Google Scholar] [CrossRef]
- Liu, F.; Tang, C.H. Soy Protein Nanoparticle Aggregates as Pickering Stabilizers for Oil-in-Water Emulsions. J. Agric. Food Chem. 2013, 61, 8888–8898. [Google Scholar] [CrossRef]
- Zhu, X.F.; Zhang, N.; Lin, W.F.; Tang, C.H. Freeze-thaw stability of pickering emulsions stabilized by soy and whey protein particles. Food Hydrocoll. 2017, 69, 173–184. [Google Scholar] [CrossRef]
- Dumay, E.; Chevalier-Lucia, D.; Picart-Palmade, L.; Benzaria, A.; Gracia-Julia, A.; Blayo, C. Technological aspects and potential applications of (ultra) high-pressure homogenisation. Trends Food Sci. Technol. 2013, 31, 13–26. [Google Scholar] [CrossRef]
- Hakansson, A.; Innings, F.; Tragardh, C.; Bergenstahl, B. A high-pressure homogenization emulsification model-Improved emulsifier transport and hydrodynamic coupling. Chem. Eng. Sci. 2013, 91, 44–53. [Google Scholar] [CrossRef]
- Raikar, N.B.; Bhatia, S.R.; Malone, M.F.; McClerments, D.J.; Henson, M.A. Predicting the Effect of the Homogenization Pressure on Emulsion Drop-Size Distributions. Ind. Eng. Chem. Res. 2011, 50, 6089–6100. [Google Scholar] [CrossRef]
- McClements, D.J. Reduced-Fat Foods: The Complex Science of Developing Diet-Based Strategies for Tackling Overweight and Obesity. Adv. Nutr. 2015, 6, 338S–352S. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hakansson, A.; Hounslow, M.J. Simultaneous determination of fragmentation and coalescence rates during pilot-scale high-pressure homogenization. J. Food Eng. 2013, 116, 7–13. [Google Scholar] [CrossRef]
- Hakansson, A.; Tragardh, C.; Bergenstahl, B. A method for estimating effective coalescence rates during emulsification from oil transfer experiments. J. Colloid Interface Sci. 2012, 374, 25–33. [Google Scholar] [CrossRef] [PubMed]
- Lee, L.L.; Niknafs, N.; Hancocks, R.D.; Norton, I.T. Emulsification: Mechanistic understanding. Trends Food Sci. Technol. 2013, 31, 72–78. [Google Scholar] [CrossRef]
- Tcholakova, S.; Denkov, N.D.; Ivanov, I.B.; Campbell, B. Coalescence stability of emulsions containing globular milk proteins. Adv. Colloid Interface Sci. 2006, 123, 259–293. [Google Scholar] [CrossRef] [PubMed]
- Maindarkar, S.N.; Hoogland, H.; Henson, M.A. Predicting the combined effects of oil and surfactant concentrations on the drop size distributions of homogenized emulsions. Colloids Surf. A-Physicochem. Eng. Asp. 2015, 467, 18–30. [Google Scholar] [CrossRef]
- Chang, C.; Tu, S.; Ghosh, S.; Nickerson, M.T. Effect of pH on the inter-relationships between the physicochemical, interfacial and emulsifying properties for pea, soy, lentil and canola protein isolates. Food Res. Int. 2015, 77, 360–367. [Google Scholar]
- Chen, M.S.; Xu, F.F.; Nsor-Atindana, J.; Chen, X.M.; Liu, F.; Wu, J.L.; Zhong, F. High protein and high oil emulsions: Phase diagram, stability and interfacial adsorption. Lwt-Food Sci. Technol. 2022, 153, 112464. [Google Scholar] [CrossRef]
- Ye, A.Q.; Zhu, X.Q.; Singh, H. Oil-in-Water Emulsion System Stabilized by Protein-Coated Nanoemulsion Droplets. Langmuir 2013, 29, 14403–14410. [Google Scholar] [CrossRef]
- Qian, C.; McClements, D.J. Formation of nanoemulsions stabilized by model food-grade emulsifiers using high-pressure homogenization: Factors affecting particle size. Food Hydrocoll. 2011, 25, 1000–1008. [Google Scholar] [CrossRef]
- Wooster, T.J.; Golding, M.; Sanguansri, P. Impact of Oil Type on Nanoemulsion Formation and Ostwald Ripening Stability. Langmuir 2008, 24, 12758–12765. [Google Scholar] [CrossRef] [PubMed]
- Ozturk, B.; Argin, S.; Ozilgen, M.; McClements, D.J. Formation and stabilization of nanoemulsion-based vitamin E delivery systems using natural surfactants: Quillaja saponin and lecithin. J. Food Eng. 2014, 142, 57–63. [Google Scholar] [CrossRef]
- Ozturk, B.; Argin, S.; Ozilgen, M.; McClements, D.J. Formation and stabilization of nanoemulsion-based vitamin E delivery systems using natural biopolymers: Whey protein isolate and gum arabic. Food Chem. 2015, 188, 256–263. [Google Scholar] [CrossRef] [Green Version]
- Jafari, S.M.; Assadpoor, E.; He, Y.H.; Bhandari, B. Re-coalescence of emulsion droplets during high-energy emulsification. Food Hydrocoll. 2008, 22, 1191–1202. [Google Scholar] [CrossRef]
- Schroen, K.; Berton-Carabin, C.; Renard, D.; Marquis, M.; Boire, A.; Cochereau, R.; Amine, C.; Marze, S. Droplet Microfluidics for Food and Nutrition Applications. Micromachines 2021, 12, 863. [Google Scholar] [CrossRef] [PubMed]
- Schroën, K.; de Ruiter, J.; Berton-Carabin, C. The Importance of Interfacial Tension in Emulsification: Connecting Scaling Relations Used in Large Scale Preparation with Microfluidic Measurement Methods. ChemEngineering 2020, 4, 63. [Google Scholar]
- Dickinson, E. Flocculation of protein-stabilized oil-in-water emulsions. Colloids Surf. B-Biointerfaces 2010, 81, 130–140. [Google Scholar] [CrossRef]
- Dickinson, E. Strategies to control and inhibit the flocculation of protein-stabilized oil-in-water emulsions. Food Hydrocoll. 2019, 96, 209–223. [Google Scholar] [CrossRef]
- McClements, D.J. Edible nanoemulsions: Fabrication, properties, and functional performance. Soft Matter 2011, 7, 2297–2316. [Google Scholar] [CrossRef] [Green Version]
- Dickinson, E. Hydrocolloids at interfaces and the influence on the properties of dispersed systems. Food Hydrocoll. 2003, 17, 25–39. [Google Scholar]
- Fredrick, E.; Walstra, P.; Dewettinck, K. Factors governing partial coalescence in oil-in-water emulsions. Adv. Colloid Interface Sci. 2010, 153, 30–42. [Google Scholar] [CrossRef] [PubMed]
- Langevin, D. Coalescence in foams and emulsions: Similarities and differences. Curr. Opin. Colloid Interface Sci. 2019, 44, 23–31. [Google Scholar] [CrossRef]
- Pawar, A.B.; Caggioni, M.; Hartel, R.W.; Spicer, P.T. Arrested coalescence of viscoelastic droplets with internal microstructure. Faraday Discuss. 2012, 158, 341–350. [Google Scholar] [CrossRef] [PubMed]
- Israelachvili, J. Intermolecular and Surface Forces, 3rd ed.; Academic Press: London, UK, 2011. [Google Scholar]
- Kim, H.J.; Decker, E.A.; McClements, D.J. Impact of protein surface denaturation on droplet flocculation in hexadecane oil-in-water emulsions stabilized by beta-lactoglobulin. J. Agric. Food Chem. 2002, 50, 7131–7137. [Google Scholar] [CrossRef]
- Kim, H.J.; Decker, E.A.; McClements, D.J. Role of postadsorption conformation changes of beta-lactoglobulin on its ability to stabilize oil droplets against flocculation during heating at neutral pH. Langmuir 2002, 18, 7577–7583. [Google Scholar] [CrossRef]
- Thanasukarn, P.; Pongsawatmanit, R.; McClements, D.J. Influence of emulsifier type on freeze-thaw stability of hydrogenated palm oil-in-water emulsions. Food Hydrocoll. 2004, 18, 1033–1043. [Google Scholar] [CrossRef]
- Zhai, J.; Hoffmann, S.V.; Day, L.; Lee, T.-H.; Augustin, M.A.; Aguilar, M.-I.; Wooster, T.J. Conformational Changes of alpha-Lactalbumin Adsorbed at Oil-Water Interfaces: Interplay between Protein Structure and Emulsion Stability. Langmuir 2012, 28, 2357–2367. [Google Scholar] [CrossRef]
- Zhai, J.L.; Wooster, T.J.; Hoffmann, S.V.; Lee, T.H.; Augustin, M.A.; Aguilar, M.I. Structural Rearrangement of beta-Lactoglobulin at Different Oil-Water Interfaces and Its Effect on Emulsion Stability. Langmuir 2011, 27, 9227–9236. [Google Scholar] [CrossRef]
- Brady, J.W. Introductory Food Chemistry; Cornell University Press: Ithaca, NY, USA, 2013. [Google Scholar]
- McClements, D.J.; Monahan, F.J.; Kinsella, J.E. Disulfide bond formation affects stability of whey-protein isolate emulsions. J. Food Sci. 1993, 58, 1036–1039. [Google Scholar] [CrossRef]
- Monahan, F.J.; McClements, D.J.; German, J.B. Disulfide-mediated polymerization reactions and physical properties of heated WPI-stabilized emulsions. J. Food Sci. 1996, 61, 504–509. [Google Scholar] [CrossRef]
- Yi, J.H.; Qiu, M.Y.; Liu, N.; Tian, L.; Zhu, X.P.; Decker, E.A.; McClements, D.J. Inhibition of Lipid and Protein Oxidation in Whey-Protein-Stabilized Emulsions Using a Natural Antioxidant: Black Rice Anthocyanins. J. Agric. Food Chem. 2020, 68, 10149–10156. [Google Scholar] [CrossRef] [PubMed]
- Taylor, P. Ostwald ripening in emulsions. Adv. Colloid Interface Sci. 1998, 75, 107–163. [Google Scholar] [CrossRef]
- Kabalnov, A. Ostwald ripening and related phenomena. J. Dispers. Sci. Technol. 2001, 22, 1–12. [Google Scholar] [CrossRef]
- Koroleva, M.Y.; Yurtov, E.V. Ostwald ripening in macro- and nanoemulsions. Russ. Chem. Rev. 2021, 90, 293–323. [Google Scholar] [CrossRef]
- Chang, Y.; McLandsborough, L.; McClements, D.J. Physical Properties and Antimicrobial Efficacy of Thyme Oil Nanoemulsions: Influence of Ripening Inhibitors. J. Agric. Food Chem. 2012, 60, 12056–12063. [Google Scholar] [CrossRef]
- Mun, S.H.; McClements, D.J. Influence of interfacial characteristics on Ostwald ripening in hydrocarbon oil-in-water emulsions. Langmuir 2006, 22, 1551–1554. [Google Scholar] [CrossRef]
- Kabalnov, A.S.; Shchukin, E.D. Ostwald ripening theory—Applications to fluorocarbon emulsion stability. Adv. Colloid Interface Sci. 1992, 38, 69–97. [Google Scholar] [CrossRef]
- Erni, P.; Jerri, H.A.; Wong, K.; Parker, A. Interfacial viscoelasticity controls buckling, wrinkling and arrest in emulsion drops undergoing mass transfer. Soft Matter 2012, 8, 6958–6967. [Google Scholar] [CrossRef]
- Nielsen, S.S. Food Analysis, 5th ed.; Springer: New York, NY, USA, 2017. [Google Scholar]
- McClements, D.J.; Weiss, J.; Kinchla, A.J.; Nolden, A.A.; Grossmann, L. Methods for Testing the Quality Attributes of Plant-Based Foods: Meat- and Processed-Meat Analogs. Foods 2021, 10, 260. [Google Scholar] [CrossRef]
- Cozzolino, D. The Ability of Near Infrared (NIR) Spectroscopy to Predict Functional Properties in Foods: Challenges and Opportunities. Molecules 2021, 26, 6981. [Google Scholar] [CrossRef]
- Hong, P.; Koza, S.; Bouvier, E.S.P. A review size-exclusion chromatography for the analysis of protein biotherapeutics and their aggregates. J. Liq. Chromatogr. Relat. Technol. 2012, 35, 2923–2950. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fekete, S.; Beck, A.; Veuthey, J.L.; Guillarme, D. Theory and practice of size exclusion chromatography for the analysis of protein aggregates. J. Pharm. Biomed. Anal. 2014, 101, 161–173. [Google Scholar] [CrossRef] [PubMed]
- Singh, S.M.; Furman, R.; Singh, R.K.; Balakrishnan, G.; Chennamsetty, N.; Tao, L.; Li, Z.J. Size exclusion chromatography for the characterization and quality control of biologics. J. Liq. Chromatogr. Relat. Technol. 2021, 44, 265–278. [Google Scholar] [CrossRef]
- Phillips, G.O.; Williams, P.A. Handbook of Hydrocolloids, 3rd ed.; Woodhead Publishing: Cambridge, UK, 2021. [Google Scholar]
- Zhang, Y.; Sharan, S.; Rinnan, Å.; Orlien, V. Survey on Methods for Investigating Protein Functionality and Related Molecular Characteristics. Foods 2021, 10, 2848. [Google Scholar]
- Grossmann, L.; Hinrichs, J.; Weiss, J. Solubility of extracted proteins from Chlorella sorokiniana, Phaeodactylum tricornutum, and Nannochloropsis oceanica: Impact of pH-value. LWT 2019, 105, 408–416. [Google Scholar] [CrossRef]
- Morr, C.V.; German, B.; Kinsella, J.E.; Regenstein, J.M.; Vanburen, J.P.; Kilara, A.; Lewis, B.A.; Mangino, M.E. A collaborative study to develop a standardized food protein solubility procedure. J. Food Sci. 1985, 50, 1715–1718. [Google Scholar] [CrossRef]
- Walker, J.M. Nondenaturing Polyacrylamide Gel Electrophoresis of Proteins. In The Protein Protocols Handbook; Walker, J.M., Ed.; Humana Press: New York, NY, USA, 2002; pp. 57–60. [Google Scholar]
- Mahler, H.C.; Friess, W.; Grauschopf, U.; Kiese, S. Protein Aggregation: Pathways, Induction Factors and Analysis. J. Pharm. Sci. 2009, 98, 2909–2934. [Google Scholar] [CrossRef]
- Greenfield, N.J. Using circular dichroism collected as a function of temperature to determine the thermodynamics of protein unfolding and binding interactions. Nat. Protoc. 2006, 1, 2527–2535. [Google Scholar] [CrossRef]
- Eftink, M.R. The use of fluorescence methods to monitor unfolding transitions in proteins. Biophys J. 1994, 66, 482–501. [Google Scholar] [CrossRef] [Green Version]
- Usoltsev, D.; Sitnikova, V.; Kajava, A.; Uspenskaya, M. Systematic FTIR Spectroscopy Study of the Secondary Structure Changes in Human Serum Albumin under Various Denaturation Conditions. Biomolecules 2019, 9, 359. [Google Scholar] [CrossRef] [Green Version]
- Webb, N.B.; Ivey, F.J.; Craig, H.B.; Jones, V.A.; Monroe, R.J. Measurement of emulsifying capacity by electrical resistance. J. Food Sci. 1970, 35, 501. [Google Scholar] [CrossRef]
- Carpenter, J.A.; Saffle, R.L. Simple method of estimating emulsifying capacity of various sausage meats. J. Food Sci. 1964, 29, 774. [Google Scholar] [CrossRef]
- Gumus, C.E.; Decker, E.A.; McClements, D.J. Formation and Stability of omega-3 Oil Emulsion-Based Delivery Systems Using Plant Proteins as Emulsifiers: Lentil, Pea, and Faba Bean Proteins. Food Biophys. 2017, 12, 186–197. [Google Scholar] [CrossRef]
- Hinderink, E.B.A.; Munch, K.; Sagis, L.; Schroen, K.; Berton-Carabin, C.C. Synergistic stabilisation of emulsions by blends of dairy and soluble pea proteins: Contribution of the interfacial composition. Food Hydrocoll. 2019, 97, 105206. [Google Scholar] [CrossRef]
- Benjamin, O.; Silcock, P.; Beauchamp, J.; Buettner, A.; Everett, D.W. Emulsifying Properties of Legume Proteins Compared to beta-Lactoglobulin and Tween 20 and the Volatile Release from Oil-in-Water Emulsions. J. Food Sci. 2014, 79, E2014–E2022. [Google Scholar] [CrossRef]
- Schestkowa, H.; Drusch, S.; Wagemans, A.M. FTIR analysis of beta-lactoglobulin at the oil/water-interface. Food Chem. 2020, 302, 125349. [Google Scholar] [CrossRef]
- Relkin, P.; Launay, B.; Liu, T.X. Heat- and cold-setting gels of beta-lactoglobulin solutions. A DSC and TEM study. Thermochim. Acta 1998, 308, 69–74. [Google Scholar] [CrossRef]
- Qian, C.; Decker, E.A.; Xiao, H.; McClements, D.J. Comparison of Biopolymer Emulsifier Performance in Formation and Stabilization of Orange Oil-in-Water Emulsions. J. Am. Oil Chem. Soc. 2011, 88, 47–55. [Google Scholar] [CrossRef]
- Schroder, A.; Berton-Carabin, C.; Venema, P.; Cornacchia, L. Interfacial properties of whey protein and whey protein hydrolysates and their influence on O/W emulsion stability. Food Hydrocoll. 2017, 73, 129–140. [Google Scholar] [CrossRef]
- Bai, L.; Huan, S.Q.; Gu, J.Y.; McClements, D.J. Fabrication of oil-in-water nanoemulsions by dual-channel microfluidization using natural emulsifiers: Saponins, phospholipids, proteins, and polysaccharides. Food Hydrocoll. 2016, 61, 703–711. [Google Scholar] [CrossRef] [Green Version]
- Amine, C.; Dreher, J.; Helgason, T.; Tadros, T. Investigation of emulsifying properties and emulsion stability of plant and milk proteins using interfacial tension and interfacial elasticity. Food Hydrocoll. 2014, 39, 180–186. [Google Scholar] [CrossRef]
- Kharat, M.; Zhang, G.D.; McClements, D.J. Stability of curcumin in oil-in-water emulsions: Impact of emulsifier type and concentration on chemical degradation. Food Res. Int. 2018, 111, 178–186. [Google Scholar] [CrossRef] [PubMed]
- Hu, M.; McClements, D.J.; Decker, E.A. Lipid oxidation in corn oil-in-water emulsions stabilized by casein, whey protein isolate, and soy protein isolate. J. Agric. Food Chem. 2003, 51, 1696–1700. [Google Scholar] [CrossRef] [PubMed]
- Romero, A.; Beaumal, V.; David-Briand, E.; Cordobes, F.; Guerrero, A.; Anton, M. Interfacial and Oil/Water Emulsions Characterization of Potato Protein Isolates. J. Agric. Food Chem. 2011, 59, 9466–9474. [Google Scholar] [CrossRef]
- van Koningsveld, G.A.; Walstra, P.; Voragen, A.G.J.; Kuijpers, I.J.; Van Boekel, M.; Gruppen, H. Effects of protein composition and enzymatic activity on formation and properties of potato protein stabilized emulsions. J. Agric. Food Chem. 2006, 54, 6419–6427. [Google Scholar] [CrossRef]
- van Koningsveld, G.A.; Gruppen, H.; de Jongh, H.H.J.; Wijngaards, G.; van Boekel, M.A.J.S.; Walstra, P.; Voragen, A.G.J. Effects of pH and Heat Treatments on the Structure and Solubility of Potato Proteins in Different Preparations. J. Agric. Food Chem. 2001, 49, 4889–4897. [Google Scholar] [CrossRef]
- Oliete, B.; Potin, F.; Cases, E.; Saurel, R. Microfluidization as Homogenization Technique in Pea Globulin-Based Emulsions. Food Bioprocess. Technol. 2019, 12, 877–882. [Google Scholar] [CrossRef]
- Keivaninahra, F.; Gadkaria, P.; Benisb, K.Z.; Tulbekc, M.; Ghosh, S. Prediction of emulsification behaviour of pea and faba bean protein concentrates and isolates from structure–functionality analysis. Rsc Adv. 2021, 11, 12117–12135. [Google Scholar]
- Felix, M.; Romero, A.; Carrera-Sanchez, C.; Guerrero, A. Assessment of interfacial viscoelastic properties of Faba bean (Vicia faba) protein-adsorbed O/W layers as a function of pH. Food Hydrocoll. 2019, 90, 353–359. [Google Scholar] [CrossRef]
- Morales, E.; Burgos-Díaz, C.; Zúñiga, R.N.; Jorkowski, J.; Quilaqueo, M.; Rubilar, M. Effect of Interfacial Ionic Layers on the Food-Grade O/W Emulsion Physical Stability and Astaxanthin Retention during Spray-Drying. Foods 2021, 10, 312. [Google Scholar]
- Keerati-u-rai, M. Emulsifying Properties of Soy Protein Isolates and Their Effect of Bulk Properties of the Oil-in-Water Emulsion. Ph.D. Thesis, The University of Guelph, Guelph, ON, Canada, 2011. [Google Scholar]
- Li, Y.; Jin, H.; Sun, X.; Sun, J.; Liu, C.; Liu, C.; Xu, J. Physicochemical Properties and Storage Stability of Food Protein-Stabilized Nanoemulsions. Nanomaterials 2019, 9, 25. [Google Scholar]
- Tan, Y.B.; Lee, P.W.; Martens, T.D.; McClements, D.J. Comparison of emulsifying properties of plant and animal proteins in oil-in-water emulsions: Whey, soy, and RuBisCo proteins. Food Res. Int. 2022. submitted. [Google Scholar]
- Zhou, B.; Tobin, J.T.; Drusch, S.; Hogan, S.A. Interfacial properties of milk proteins: A review. Adv. Colloid Interface Sci. 2021, 295, 1–15. [Google Scholar]
- Miller, R.; Joos, P.; Fainerman, V.B. Dynamic surface and interfacial-tensions of surfactant and polymer-solutions. Adv. Colloid Interface Sci. 1994, 49, 249–302. [Google Scholar] [CrossRef]
- McClements, D.J. Critical review of techniques and methodologies for characterization of emulsion stability. Crit. Rev. Food Sci. Nutr. 2007, 47, 611–649. [Google Scholar] [CrossRef]
- Tcholakova, S.; Denkov, N.D.; Sidzhakova, D.; Ivanov, I.B.; Campbell, B. Interrelation between drop size and protein adsorption at various emulsification conditions. Langmuir 2003, 19, 5640–5649. [Google Scholar] [CrossRef]
- Keddie, J.L. Structural analysis of organic interfacial layers by ellipsometry. Curr. Opin. Colloid Interface Sci. 2001, 6, 102–110. [Google Scholar] [CrossRef]
- Atkinson, P.J.; Dickinson, E.; Horne, D.S.; Leermakers, F.A.M.; Richardson, R.M. Theoretical and experimental investigations of adsorbed protein structure at a fluid interface. Ber. Bunsen-Ges. Phys. Chem. Chem. Phys. 1996, 100, 994–998. [Google Scholar] [CrossRef]
- Atkinson, P.J.; Dickinson, E.; Horne, D.S.; Richardson, R.M. Neutron Reflectivity of Adsorbed Beta-Casein And Beta-Lactoglobulin At The Air/Water Interface. J. Chem. Soc. Faraday Trans. 1995, 91, 2847–2854. [Google Scholar] [CrossRef]
- Wooster, T.J.; Augustin, M.A. β-Lactoglobulin–dextran Maillard conjugates: Their effect on interfacial thickness and emulsion stability. J. Colloid Interface Sci. 2006, 303, 564–572. [Google Scholar] [CrossRef]
- Zielbauer, B.I.; Jackson, A.J.; Maurer, S.; Waschatko, G.; Ghebremedhin, M.; Rogers, S.E.; Heenan, R.K.; Porcar, L.; Vilgis, T.A. Soybean oleosomes studied by small angle neutron scattering (SANS). J. Colloid Interface Sci. 2018, 529, 197–204. [Google Scholar] [CrossRef] [PubMed]
- Karbaschi, M.; Lotfi, M.; Kragel, J.; Javadi, A.; Bastani, D.; Miller, R. Rheology of interfacial layers. Curr. Opin. Colloid Interface Sci. 2014, 19, 514–519. [Google Scholar] [CrossRef]
- van Aken, G.A.; van Vliet, T. Flow-induced coalescence in protein-stabilized highly concentrated emulsions: Role of shear-resisting connections between the droplets. Langmuir 2002, 18, 7364–7370. [Google Scholar] [CrossRef]
- Pearce, K.N.; Kinsella, J.E. Emulsifying Properties of Proteins—Evaluation of a Turbidimetric Technique. J. Agric. Food Chem. 1978, 26, 716–723. [Google Scholar]
- Xu, X.F.; Zhong, J.Z.; Chen, J.; Liu, C.M.; Luo, L.P.; Luo, S.J.; Wu, L.X.; McClements, D.J. Effectiveness of partially hydrolyzed rice glutelin as a food emulsifier: Comparison to whey protein. Food Chem. 2016, 213, 700–707. [Google Scholar] [CrossRef] [Green Version]
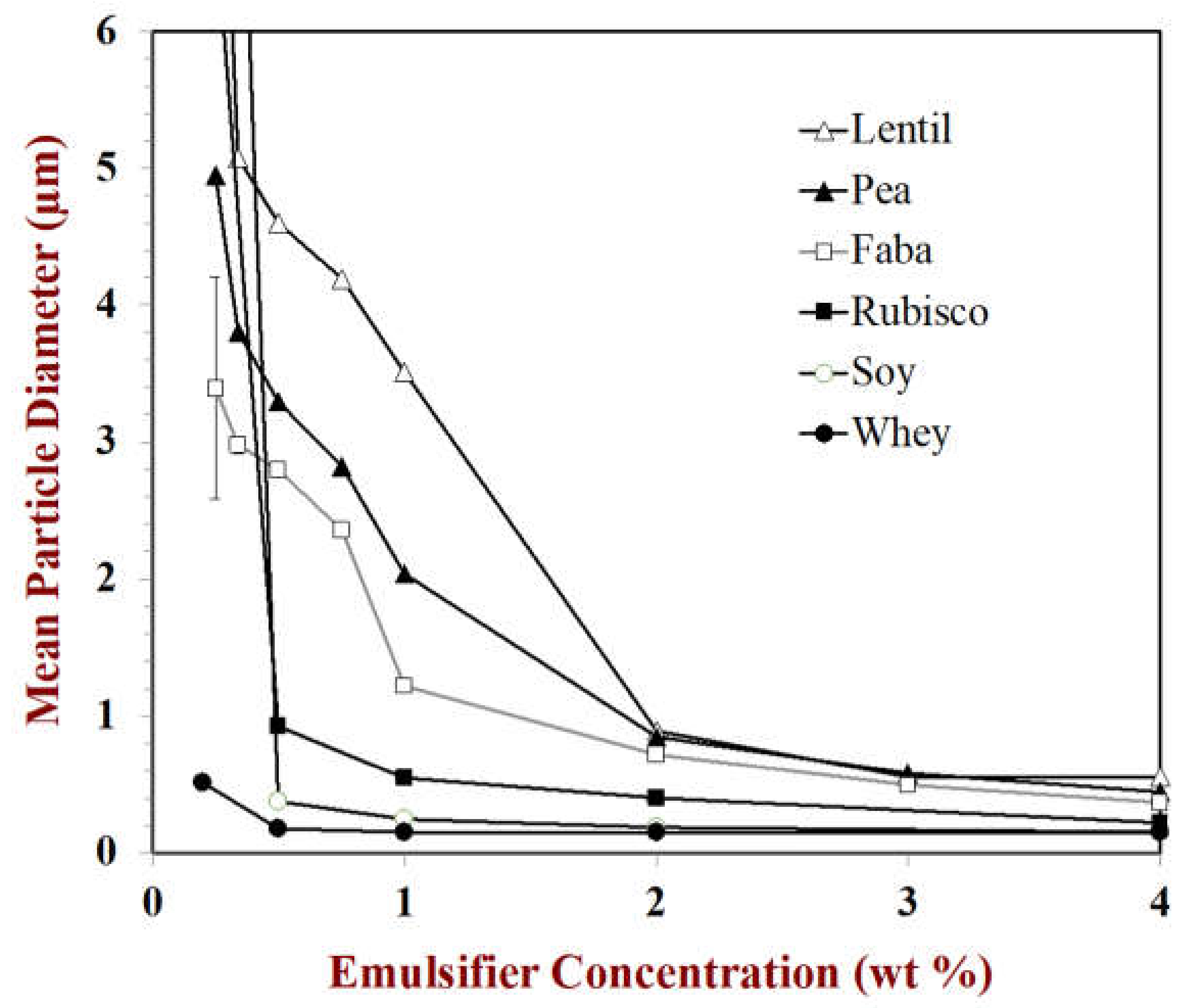


| Protein Type | ΓSat (mg/m2) | γOW (mN/m) | SA (wt%−1) | D0.1 (μm) | C1μm (g/g) | pI | Tm (oC) | Ref |
|---|---|---|---|---|---|---|---|---|
| β-lactoglobulin | 1.8−2.7 | 12.6 | 8.3 | 0.17 | 0.2 | 4.7 | 75 | [93,94,95] |
| Whey | 1.83 | 13.5 | 10 | 0.11 | 0.2 | 5.0 | 72 | [3,45,96,97] |
| Sodium Caseinate | 1.5 | 1.8 | 4 | 0.18 | <0.2 | 4.6 | N/A | [98,99,100] |
| Potato | 1.8 | 4.1 | 4 | 0.23 | 0.2 | 4.9 | 60 | [98,101,102,103] |
| Pea | 2.8−5.9 | 3.8 | 5 | 0.36 | 0.7 | 4.5 | 77−79 | [3,90,98,104] |
| Faba | 2.4 | 8.0 | 1.3 | 0.71 | 0.58 | 4.8 | [90,105,106] | |
| Lentil | 5.1 | 9.5 | >2 | 0.87 | 0.91 | 5.0 | 120 | [39,90,107] |
| Soy | 2−6 | 10.0 | 1.2 | 0.25 | 0.4 | 4.5 | 80−93 | [3,39,108,109,110] |
| Protein Type | Property | pH Value | Comments | Reference | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Charge Size Creaming | 2 | 3 | 4 | 5 | 6 | 7 | 8 | Oil content and type Protein-to-oil ratio (P:O) Homogenizer; Particle sizer | ||
| Whey | ζ (mV) | 24.1 | 14.3 | −7.2 | −20.4 | −25.2 | 2.5% corn oil P:O = 0.2:1 Sonicator; DLS | Our Lab (Cheryl Chung) | ||
| d/d0 | 1.87 | 12.7 | 22.6 | 0.99 | 1.00 | |||||
| CI (%) | - | - | - | - | - | |||||
| Whey | ζ (mV) | 37.7 | 50.2 | 27.8 | −2.6 | −31.8 | −52.8 | −53.1 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.02 | 1.61 | 23.7 | 132 | 3.62 | 1.00 | 0.93 | |||
| CI (%) | - | - | - | - | - | - | - | |||
| Whey | ζ (mV) | 38.9 | 47.7 | 31.7 | 0.0 | −30.5 | −47.7 | −45.1 | 10% orange oil: vitamin A P:O = 0.2:1 Microfluidizer, SLS | [45] |
| d/d0 | 1.00 | 1.00 | 1.01 | 121 | 1.00 | 1.00 | 0.99 | |||
| CI (%) | 0 | 0 | 0 | 27% | 0 | 0 | 0 | |||
| Caseinate | ζ (mV) | 18.8 | 11.9 | −23.0 | −37.1 | −41.6 | 2.5% corn oil P:O = 0.2:1 Sonicator; DLS | Our Lab (Chung) | ||
| d/d0 | 30.3 | 53.2 | 1.72 | 0.98 | 1.00 | |||||
| CI (%) | - | - | - | - | - | |||||
| Faba | ζ (mV) | 23.6 | 26.2 | 14.3 | −4.1 | −14.0 | −18.3 | −19.5 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.88 | 20.13 | 14.19 | 11.7 | 3.30 | 1.00 | 0.83 | |||
| CI (%) | 86 | 76 | 74 | 74 | 65 | 0 | 0 | |||
| Lentil | ζ (mV) | 24.5 | 29.4 | 19.4 | −0.7 | −18.4 | −20.8 | −21.2 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 0.96 | 1.08 | 0.93 | 16.3 | 0.83 | 1.00 | 0.91 | |||
| CI (%) | 0 | 0 | 59 | 47 | 48 | 0 | 0 | |||
| Pea | ζ (mV) | 22.6 | 26.8 | 15.9 | −4.1 | −16.4 | −17.2 | −17.6 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 0.82 | 15.3 | 24.2 | 23.8 | 4.03 | 1.00 | 0.70 | |||
| CI (%) | 0 | 76 | 78 | 81 | 78 | 0 | 0 | |||
| Soy | ζ (mV) | 26.9 | 31.2 | 18.0 | −6.1 | −19.1 | −35.9 | −42.6 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 95.9 | 132 | 128 | 124 | 1.41 | 1.00 | 0.89 | |||
| CI (%) | - | - | - | - | - | - | - | |||
| Rubisco | ζ (mV) | 22.3 | 30.1 | 18.4 | −7.7 | −27.0 | −35.0 | −31.6 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 2.18 | 10.7 | 41.5 | 42.3 | 29.0 | 1.00 | 0.84 | |||
| CI (%) | - | - | - | - | - | - | - | |||
| Hydrolyzed rice glutelin | ζ (mV) | 26.9 | 25.7 | 5.1 | −11.3 | −21.9 | −32.8 | −40.7 | 10 wt% corn oil P:O = 0.3:1 Microfluidizer, SLS | [123] |
| d/d0 | 71.5 | 78.9 | 89.9 | 73.7 | 36.7 | 1.00 | 0.98 | |||
| CI (%) | 10 | 14 | 13 | 16 | 0 | 0 | 0 | |||
| Protein Type | Property | Salt (NaCl) Concentration (mM) | Comments | Reference | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Charge Size Creaming | 0 | 50 | 100 | 200 | 300 | 400 | 500 | Oil content and type Protein-to-oil ratio (P:O) Homogenizer; Particle sizer | ||
| Whey protein | ζ (mV) | −52.8 | - | −52.9 | −49.9 | −48.9 | −48.8 | −47.4 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | - | 0.96 | 0.99 | 1.04 | 1.08 | 1.10 | |||
| CI (%) | S | - | S | S | S | S | S | |||
| Rubisco | ζ (mV) | −35.9 | - | −39.7 | −38.7 | −37.9 | −37.4 | −36.9 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | - | 4.25 | 7.08 | 12.5 | 12.9 | 14.6 | |||
| CI (%) | S | U | U | U | U | U | ||||
| Soy | ζ (mV) | −35.0 | - | −33.7 | −33.5 | −33.1 | −33.1 | −32.7 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | - | 8.75 | 7.56 | 43.5 | 111 | 236 | |||
| CI (%) | S | S | S | S | S | S | ||||
| Faba | ζ (mV) | −18.3 | - | −17.8 | −11.6 | −8.6 | −7.2 | −8.9 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | - | 28.74 | 1.42 | 0.97 | 0.88 | 1.00 | |||
| CI (%) | S | U | U | U | S | S | ||||
| Lentil | ζ (mV) | −20.8 | - | −16.6 | −13.2 | −11.9 | −9.0 | −20.8 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | - | 0.82 | 0.62 | 0.61 | 0.60 | 0.67 | |||
| CI (%) | S | S | S | S | S | S | ||||
| Pea | ζ (mV) | −17.2 | - | −14.4 | −12.1 | −11.9 | −11.0 | −8.4 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | - | 26.0 | 8.72 | 1.75 | 1.46 | 1.35 | |||
| CI (%) | S | U | U | S | S | S | ||||
| Hydrolyzed Rice Protein | ζ (mV) | −36.3 | −17.3 | −13.4 | −10.4 | −9.3 | - | −8.1 | 10 wt% corn oil P:O = 0.3:1 Microfluidizer, SLS | [123] |
| d/d0 | 1.00 | 1.53 | 1.52 | 1.53 | 21.5 | - | 22.2 | |||
| CI (%) | - | - | - | - | - | - | - | |||
| Protein Type | Property | Temperature (oC) | Comments | Reference | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Charge Size Creaming | 30 | 40 | 50 | 60 | 70 | 80 | 90 | Oil content, oil type, buffer, temperature | ||
| Whey protein | ζ (mV) | −47.6 | −53.2 | −53.0 | −53.2 | −51.1 | −51.4 | −45.0 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | 0.99 | 1.01 | 0.99 | 0.99 | 0.99 | 0.99 | |||
| CI (%) | S | S | S | S | S | S | S | |||
| Rubisco | ζ (mV) | −39.3 | −37.8 | −37.8 | −35.9 | −34.9 | −34.5 | −34.8 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | 0.85 | 0.86 | 3.70 | 12.1 | 18.6 | 42.8 | |||
| CI (%) | ||||||||||
| Soy | ζ (mV) | −33.9 | −35.4 | −35.6 | −35.4 | −35.7 | −33.8 | −32.8 | 10% soy oil P:O = 0.1:1 Microfluidizer; SLS | [110] |
| d/d0 | 1.00 | 0.88 | 0.97 | 0.86 | 0.92 | 0.98 | 1.00 | |||
| CI (%) | ||||||||||
| Faba | ζ (mV) | −36.7 | −35.0 | −45.3 | −18.1 | −18.7 | −18.9 | −19.5 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | 0.87 | 0.84 | 1.23 | 1.20 | 1.15 | 1.15 | |||
| CI (%) | S | S | S | S | S | S | S | |||
| Lentil | ζ (mV) | −44.9 | −47.3 | −24.4 | −22.7 | −21.8 | −21.8 | −22.1 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | 0.98 | 1.09 | 1.06 | 1.49 | 1.43 | 1.41 | |||
| CI (%) | S | S | S | S | S | S | S | |||
| Pea | ζ (mV) | −30.0 | −42.4 | −36.7 | −19.2 | −17.5 | −17.5 | −18.3 | 10 wt% algae oil P:O = 0.27:1 Microfluidizer, SLS | [90] |
| d/d0 | 1.00 | 1.06 | 1.03 | 0.91 | 1.22 | 1.19 | 1.17 | |||
| CI (%) | S | S | S | S | S | S | S | |||
| Hydrolyzed Rice Protein | ζ (mV) | −10.7 | −10.0 | −10.7 | −9.3 | −8.8 | −9.4 | −9.4 | 10 wt% corn oil P:O = 0.3:1 Microfluidizer, SLS | [123] |
| d/d0 | 1.00 | 1.15 | 1.23 | 23.2 | 30.8 | 36.0 | 37.4 | |||
| CI (%) | S | S | S | U | U | U | U | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
McClements, D.J.; Lu, J.; Grossmann, L. Proposed Methods for Testing and Comparing the Emulsifying Properties of Proteins from Animal, Plant, and Alternative Sources. Colloids Interfaces 2022, 6, 19. https://doi.org/10.3390/colloids6020019
McClements DJ, Lu J, Grossmann L. Proposed Methods for Testing and Comparing the Emulsifying Properties of Proteins from Animal, Plant, and Alternative Sources. Colloids and Interfaces. 2022; 6(2):19. https://doi.org/10.3390/colloids6020019
Chicago/Turabian StyleMcClements, David Julian, Jiakai Lu, and Lutz Grossmann. 2022. "Proposed Methods for Testing and Comparing the Emulsifying Properties of Proteins from Animal, Plant, and Alternative Sources" Colloids and Interfaces 6, no. 2: 19. https://doi.org/10.3390/colloids6020019
APA StyleMcClements, D. J., Lu, J., & Grossmann, L. (2022). Proposed Methods for Testing and Comparing the Emulsifying Properties of Proteins from Animal, Plant, and Alternative Sources. Colloids and Interfaces, 6(2), 19. https://doi.org/10.3390/colloids6020019