Diffusiophoresis of a Soft Particle as a Model for Biological Cells
Abstract
:1. Introduction
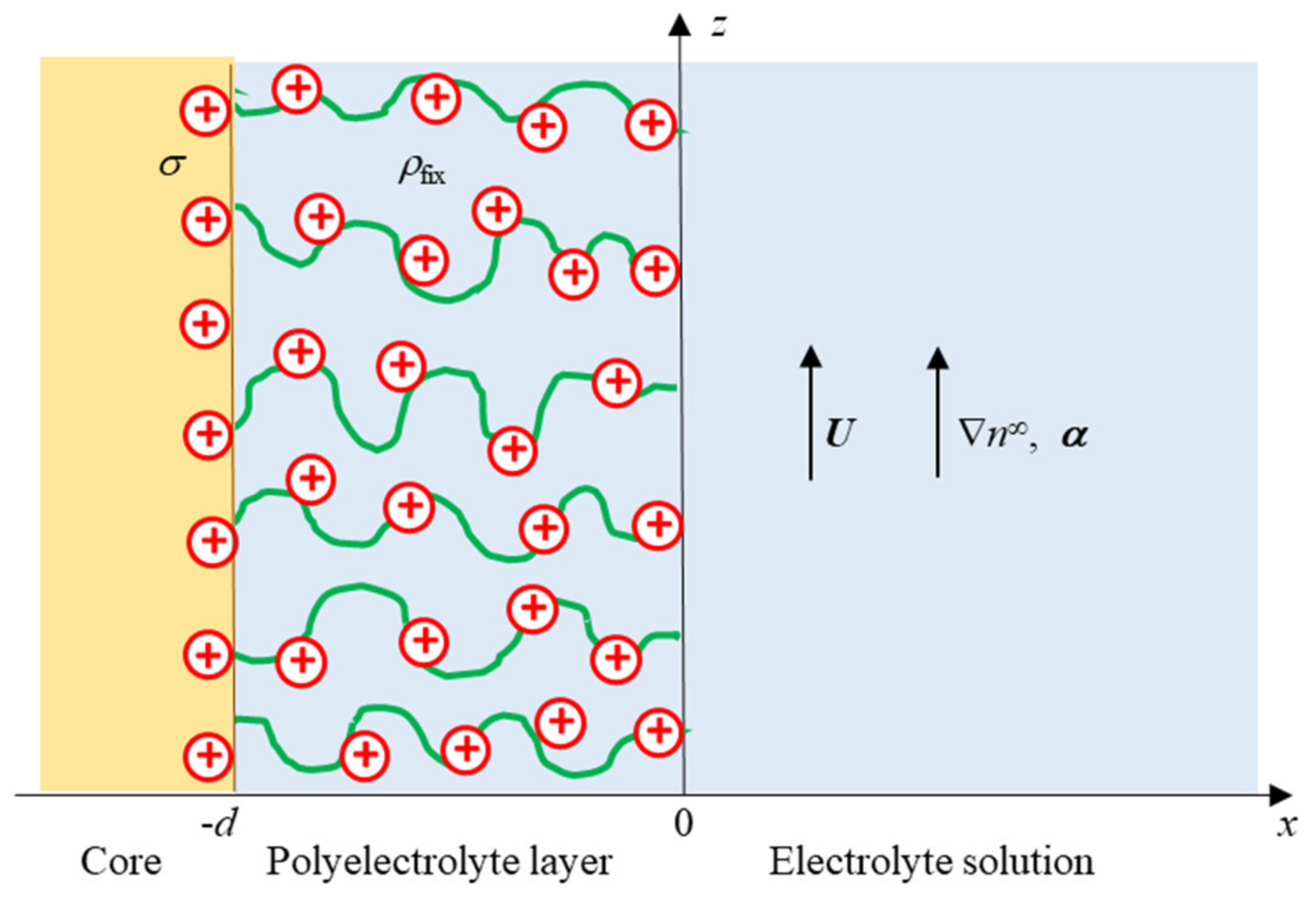
2. Theory
3. Results and Discussion
3.1. Weakly Charged Soft Particle
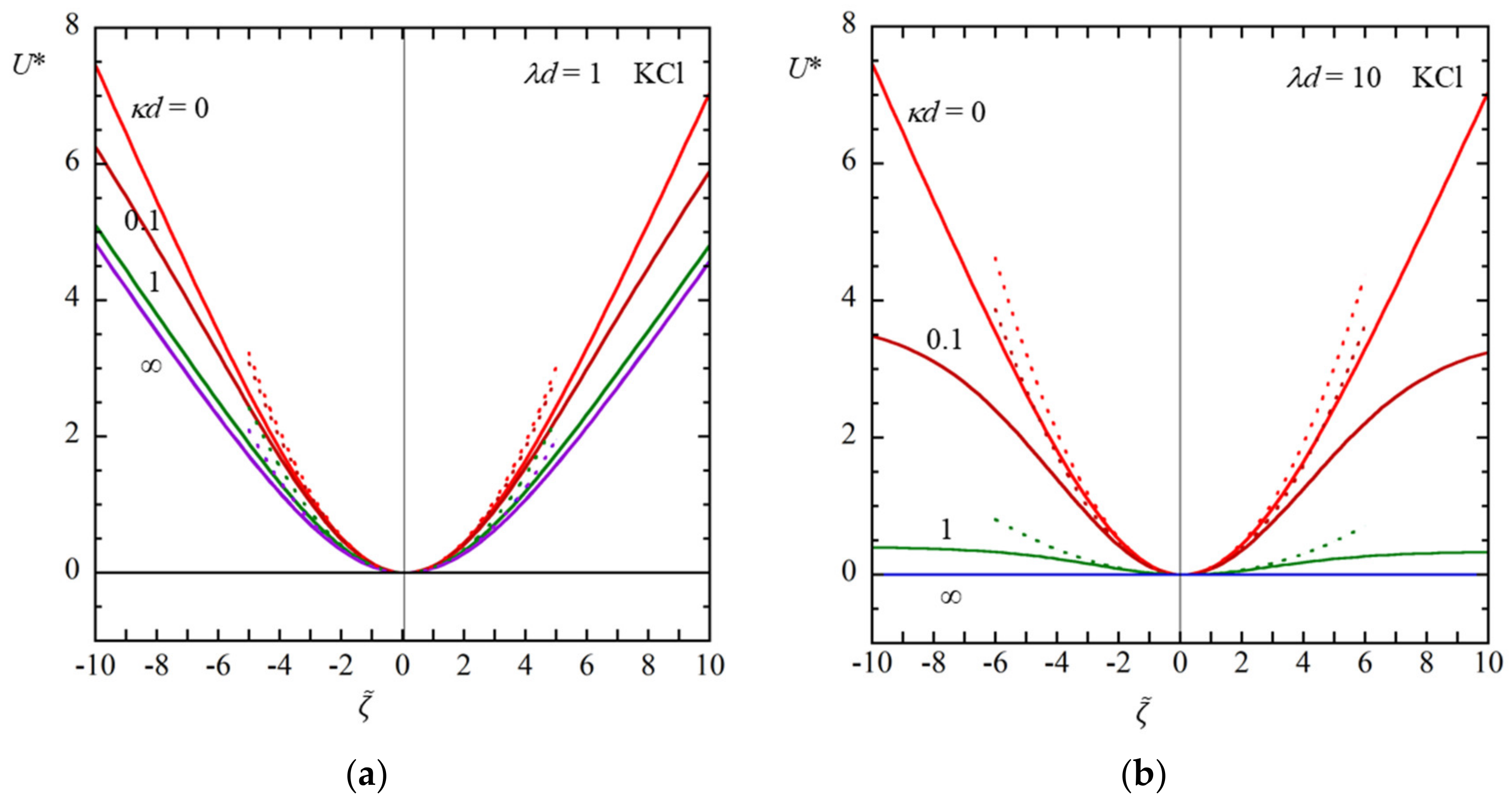
3.2. Soft Particle with a Thick Polyelectrolyte Layer
3.3. Soft Particle with an Uncharged Polymer Layer
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Derjaguin, B.V.; Dukhin, S.S.; Korotkova, A.A. Diffusiophoresis in electrolyte solutions and its role in the mechanism of film formation of cationic latex by ionic deposition. Kolloidyni Zh. 1961, 23, 53–58. [Google Scholar]
- Prieve, D.C. Migration of a colloidal particle in a gradient of electrolyte concentration. Adv. Colloid Interface Sci. 1982, 16, 321–335. [Google Scholar] [CrossRef]
- Prieve, D.C.; Anderson, J.L.; Ebel, J.P.; Lowell, M.E. Motion of a particle generated by chemical gradients. Part 2. Electrolytes. J. Fluid Mech. 1984, 148, 247–269. [Google Scholar] [CrossRef]
- Prieve, D.C.; Roman, R. Diffusiophoresis of a rigid sphere through a viscous electrolyte solution. J. Chem. Soc. Faraday Trans. II 1987, 83, 1287–1306. [Google Scholar] [CrossRef]
- Anderson, J.L. Colloid transport by interfacial forces. Ann. Rev. Fluid Mech. 1989, 21, 61–99. [Google Scholar] [CrossRef]
- Pawar, Y.; Solomentsev, Y.E.; Anderson, J.L. Polarization effects on diffusiophoresis in electrolyte gradients. J. Colloid Interface Sci. 1993, 155, 488–498. [Google Scholar] [CrossRef]
- Keh, H.J.; Chen, S.B. Diffusiophoresis and electrophoresis of colloidal cylinders. Langmuir 1993, 9, 1142–1149. [Google Scholar] [CrossRef]
- Keh, H.J.; Wei, Y.K. Diffusiophoretic mobility of spherical particles at low potential and arbitrary double-layer thickness. Langmuir 2000, 16, 5289–5294. [Google Scholar] [CrossRef]
- Hoshyargar, V.; Ashrafizadeh, S.N.; Sadeghi, A. Drastic alteration of diffusioosmosis due to steric effects. Phys. Chem. Chem. Phys. 2015, 17, 29193. [Google Scholar] [CrossRef]
- Keh, H.J. Diffusiophoresis of charged particles and diffusioosmosis of electrolyte solutions. Curr. Opin. Colloid Interface Sci. 2016, 24, 13–22. [Google Scholar] [CrossRef]
- Gupta, A.; Rallabandi, B.; Howard, A.; Stone, H.A. Diffusiophoretic and diffusioosmotic velocities for mixtures of valence-asymmetric electrolytes. Phys. Rev. Fluids 2019, 4, 043702. [Google Scholar] [CrossRef]
- Gupta, A.; Shim, S.; Stone, H.A. Diffusiophoresis: From dilute to concentrated electrolytes. Soft Matter. 2020, 16, 6975–6984. [Google Scholar] [CrossRef]
- Wilson, J.L.; Shim, S.; Yu, Y.E.; Gupta, A.; Stone, H.A. Diffusiophoresis in multivalent electrolytes. Langmuir 2020, 36, 7014–7020. [Google Scholar] [CrossRef]
- Ohshima, H. Approximate analytic expressions for the diffusiophoretic velocity of a spherical colloidal particle. Electrophoresis 2022, 43, 752–756. [Google Scholar] [CrossRef]
- Ohshima, H. Diffusiophoretic velocity of a large spherical colloidal particle in a solution of general electrolytes. Colloid Polym. Sci. 2021, 299, 1877–1884. [Google Scholar] [CrossRef]
- Ohshima, H. Ion-size effect on the diffusiophoretic mobility of a large colloidal particle. Colloid Polym. Sci. 2022, 1–6. [Google Scholar] [CrossRef]
- Lou, J.; Lee, E. Diffusiophoresis of concentrated suspensions of liquid drops. J. Phys. Chem. C 2008, 112, 12455–12462. [Google Scholar] [CrossRef]
- Yang, F.; Shin, S.; Stone, H.A. Diffusiophoresis of a charged drop. J. Fluid Mech. 2018, 852, 37–59. [Google Scholar] [CrossRef]
- Wu, Y.; Jian, E.; Fan, L.; Tseng, J.; Wan, R.; Lee, E. Diffusiophoresis of a highly charged dielectric fluid droplet. Phys. Fluids. 2021, 33, 122005. [Google Scholar] [CrossRef]
- Ohshima, H. Diffusiophoresis of a mercury drop. Colloid Polym Sci. 2022, 1–4. [Google Scholar] [CrossRef]
- Huang, P.Y.; Keh, H.J. Diffusiophoresis of a spherical soft particle in electrolyte gradients. J. Phys. Chem. B 2012, 116, 7575–7589. [Google Scholar] [CrossRef]
- Tseng, S.; Chung, Y.-C.; Hsu, J.-P. Diffusiophoresis of a soft, pH-regulated particle in a solution containing multiple ionic species. J. Colloid Interface Sci. 2015, 438, 196–293. [Google Scholar] [CrossRef] [PubMed]
- Majee, P.S.; Bhattacharyya, S. Impact of ion partitioning and double layer polarization on diffusiophoresis of a pH-regulated nanogel. Meccanica 2021, 56, 1989–2004. [Google Scholar] [CrossRef]
- Wu, Y.; Lee, Y.F.; Chang, W.C.; Fan, L.; Jian, E.; Tseng, J.; Lee, E. Diffusiophoresis of a highly charged soft particle in electrolyte solutions induced by diffusion potential. Phys. Fluids 2021, 33, 012014. [Google Scholar] [CrossRef]
- Lee, Y.F.; Chang, W.C.; Wu, Y.; Fan, L.; Lee, E. Diffusiophoresis of a highly charged soft particle in electrolyte solutions. Langmuir 2021, 37, 1480–1492. [Google Scholar] [CrossRef] [PubMed]
- Ohshima, H. Diffusiophoretic velocity of a spherical soft particle. Colloid Polym. Sci. 2022, 300, 153–157. [Google Scholar] [CrossRef]
- Ohshima, H. Electrophoretic mobility of soft particles. J. Colloid Interface Sci. 1994, 62, 474–483. [Google Scholar] [CrossRef]
- Ohshima, H. Electrophoresis of soft particles. Adv. Colloid Interface Sci. 1995, 62, 189–235. [Google Scholar] [CrossRef]
- Ohshima, H. On the general expression for the electrophoretic mobility of a soft particle. J. Colloid Interface Sci. 2000, 228, 190–193. [Google Scholar] [CrossRef]
- Ohshima, H. Theory of Colloid and Interfacial Electric Phenomena; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Ohshima, H. Approximate analytic expressions for the electrophoretic mobility of spherical soft particles. Electrophoresis 2021, 42, 2182–2188. [Google Scholar] [CrossRef]
- Hartman, S.V.; Božič, B.; Derganc, J. Migration of blood cells and phospholipid vesicles induced by concentration gradients in microcavities. New Biotech. 2018, 47, 60–69. [Google Scholar] [CrossRef]
- Shim, S.; Khodaparast, S.; Lai, C.-Y.; Yan, J.; Ault, J.T.; Rallabandi, B.; Shardt, O.; Stone, H.A. CO2-Driven diffusiophoresis for maintaining a bacteria-free surface. Soft Matter. 2021, 17, 2568–2576. [Google Scholar] [CrossRef]
- Shin, S. Diffusiophoretic separation of colloids in microfluidic flows. Phys. Fluids 2020, 32, 101302. [Google Scholar] [CrossRef]
- Brinkman, H.C. A calculation of the viscous force exerted by a flowing fluid on a dense swarm of particles. Appl. Sci. Res. 1947, 1, 27–34. [Google Scholar] [CrossRef]
- Debye, P.; Bueche, A.M. Intrinsic viscosity, diffusion, and sedimentation rate of polymers in solution. J. Chem. Phys. 1948, 16, 573–579. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ohshima, H. Diffusiophoresis of a Soft Particle as a Model for Biological Cells. Colloids Interfaces 2022, 6, 24. https://doi.org/10.3390/colloids6020024
Ohshima H. Diffusiophoresis of a Soft Particle as a Model for Biological Cells. Colloids and Interfaces. 2022; 6(2):24. https://doi.org/10.3390/colloids6020024
Chicago/Turabian StyleOhshima, Hiroyuki. 2022. "Diffusiophoresis of a Soft Particle as a Model for Biological Cells" Colloids and Interfaces 6, no. 2: 24. https://doi.org/10.3390/colloids6020024
APA StyleOhshima, H. (2022). Diffusiophoresis of a Soft Particle as a Model for Biological Cells. Colloids and Interfaces, 6(2), 24. https://doi.org/10.3390/colloids6020024





