Analysis of the Deformation Mechanisms of Fabrics Based on rCF Staple Fiber Yarns for Thermoset Composite Applications
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Experimental Characterization of Woven Fabrics
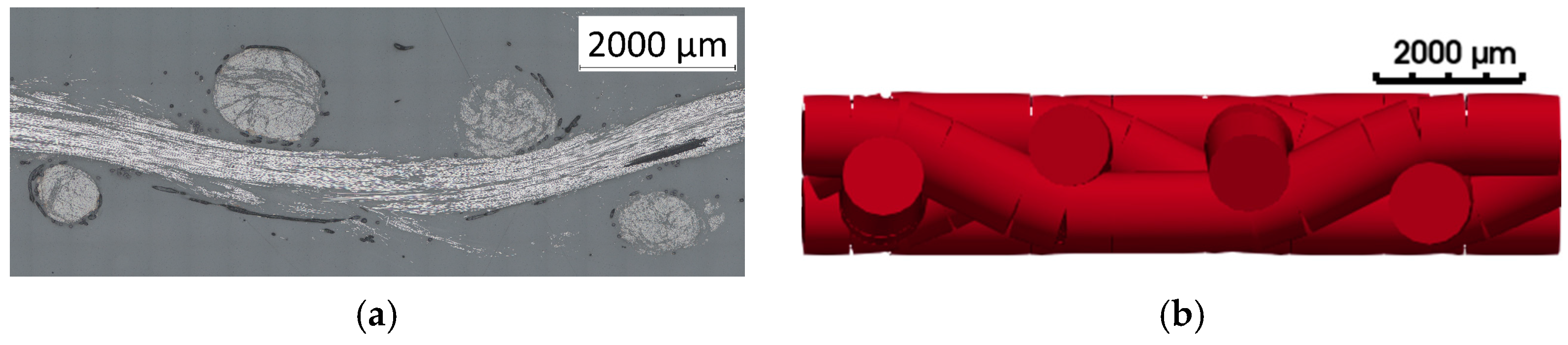
2.3. Meso-Scale Modeling of Textiles Made from Recycled Staple Fiber Yarns
3. Results
3.1. Fabric Characteristics
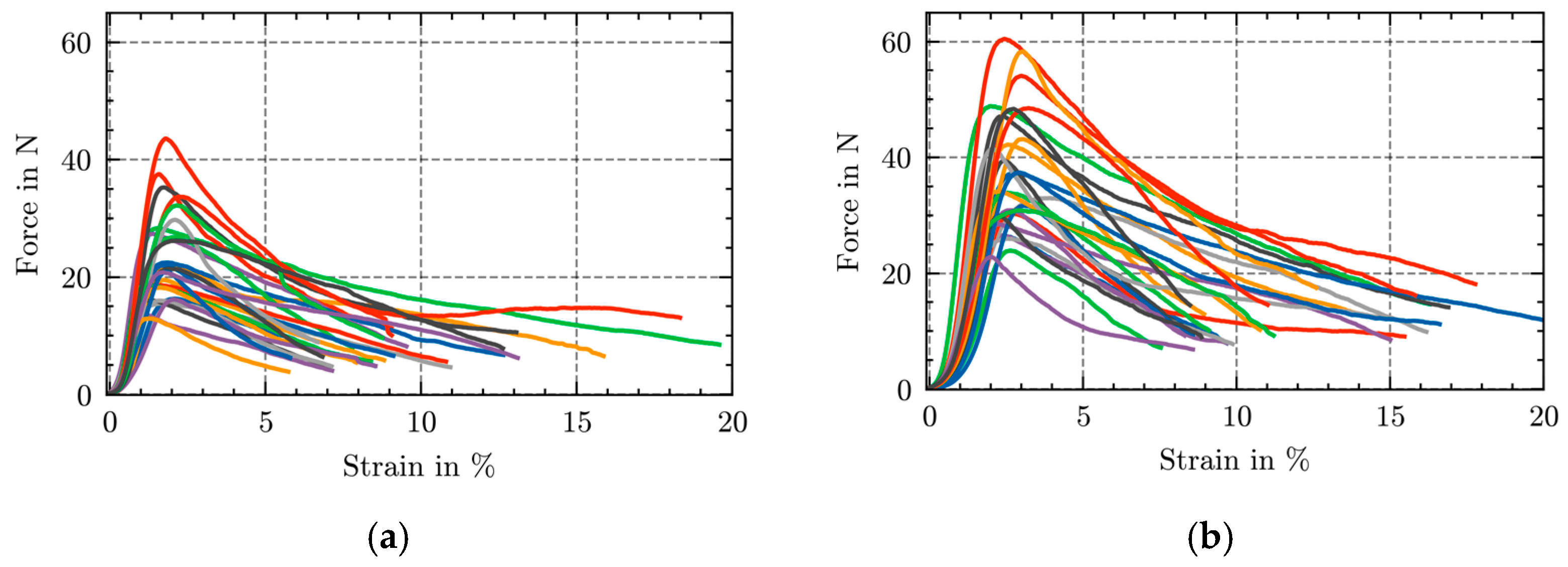
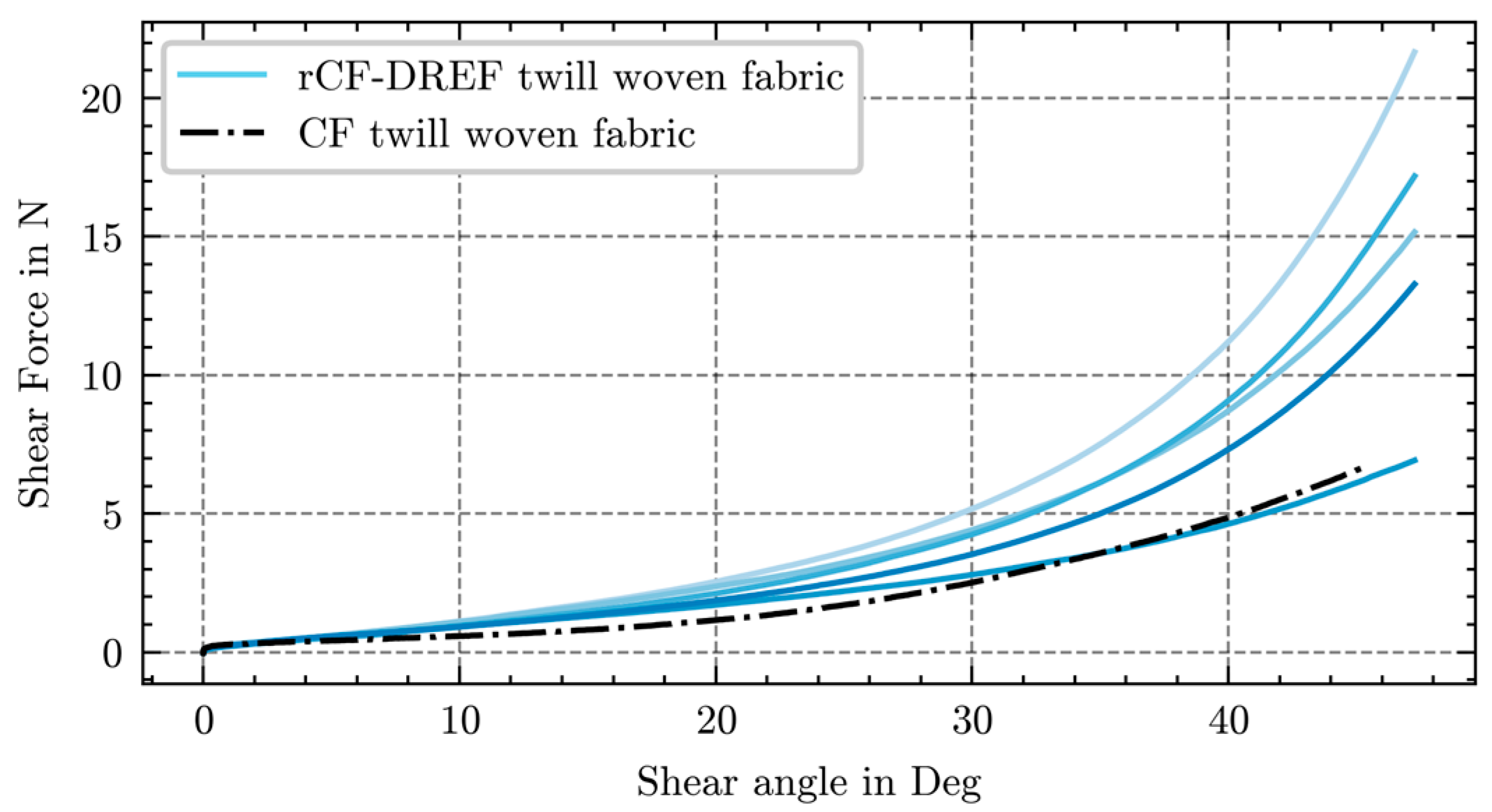
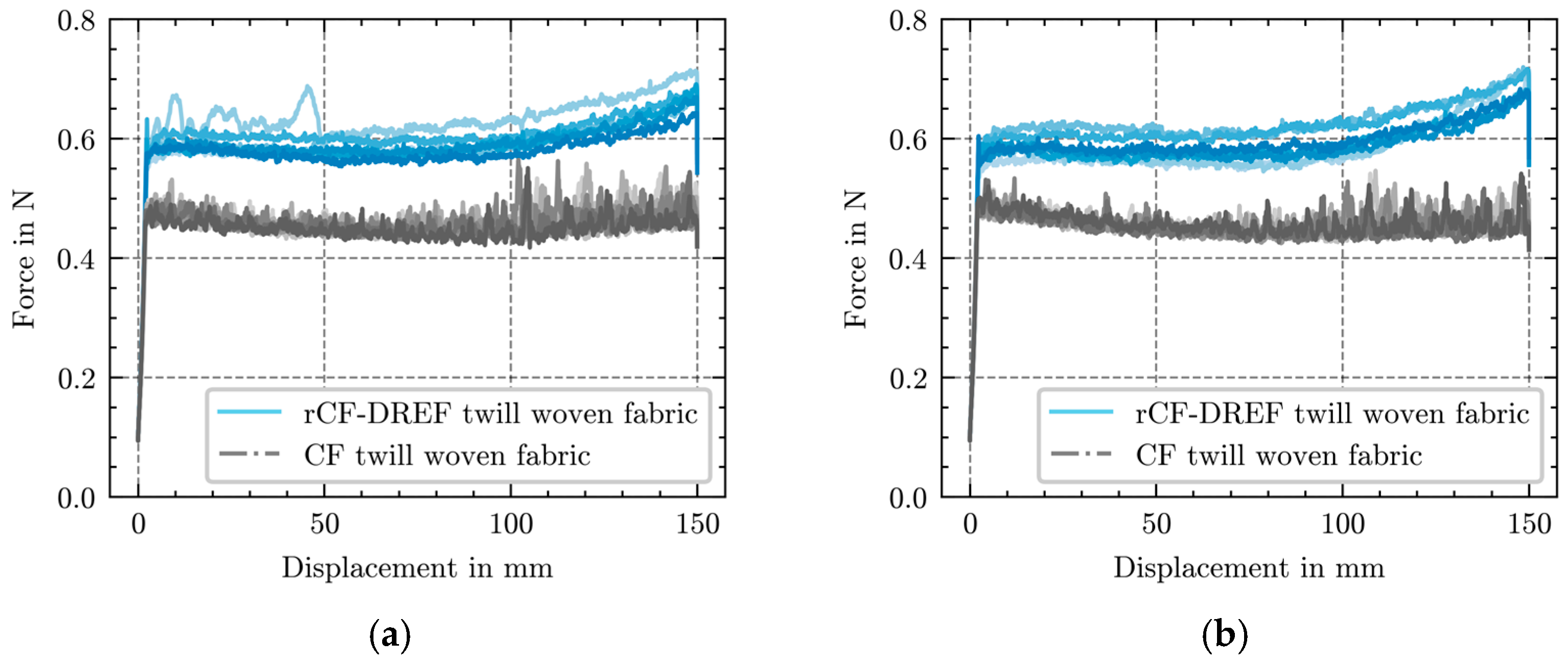
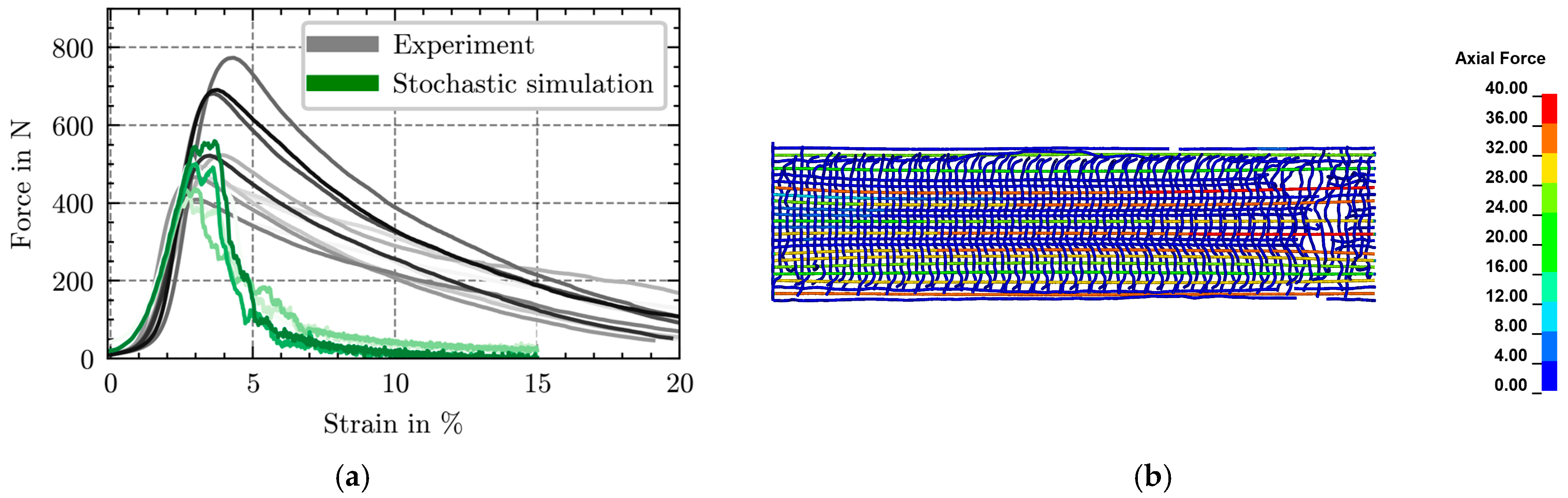
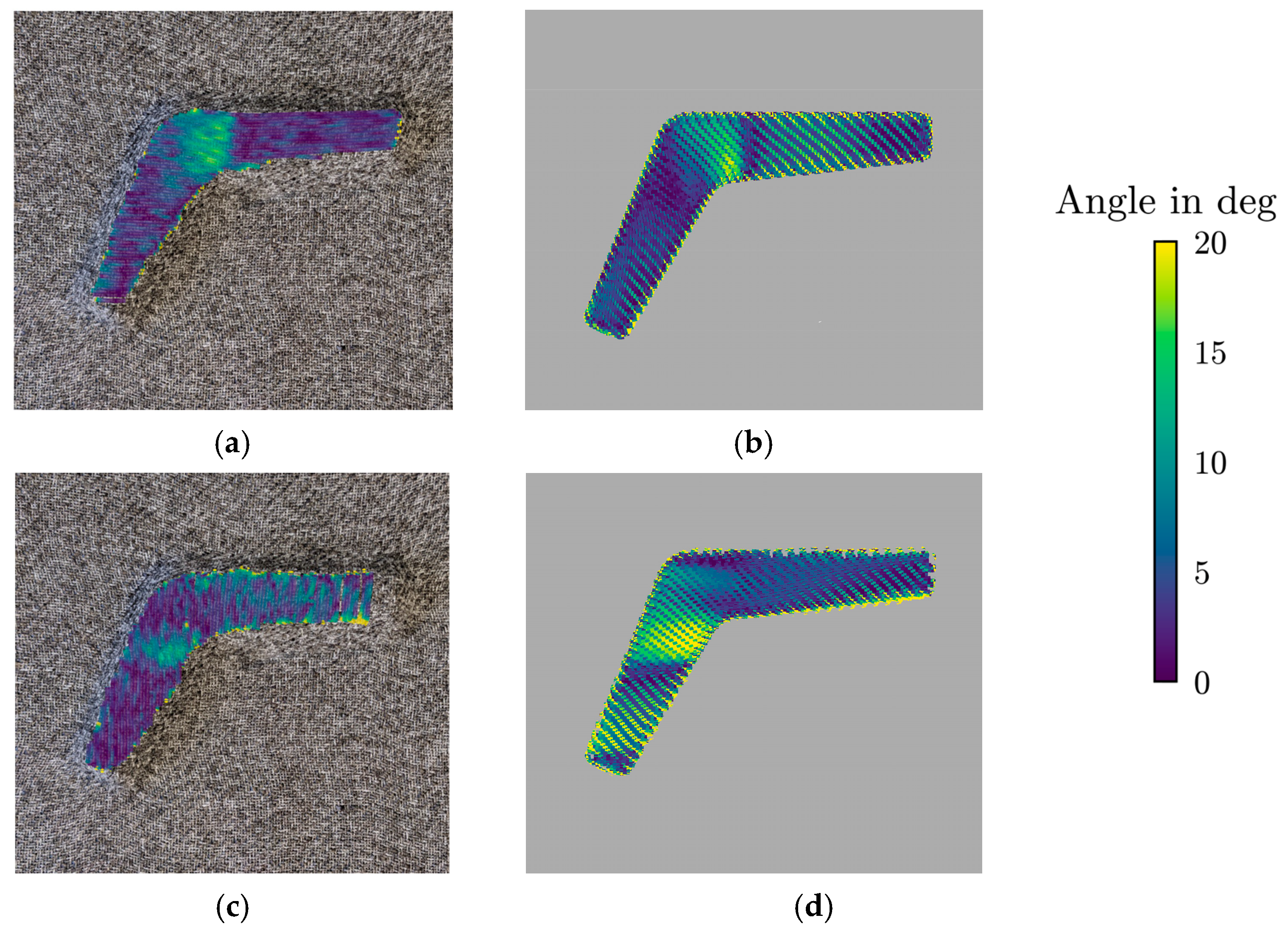
3.2. Simulation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lightfoot, J.S.; Wisnom, M.R.; Potter, K. Defects in woven preforms: Formation mechanisms and the effects of laminate design and layup protocol. Compos. Part A Appl. Sci. Manuf. 2013, 51, 99–107. [Google Scholar] [CrossRef]
- Kulkarni, P.; Mali, K.D.; Singh, S. An overview of the formation of fibre waviness and its effect on the mechanical performance of fibre reinforced polymer composites. Compos. Part A Appl. Sci. Manuf. 2020, 137, 106013. [Google Scholar] [CrossRef]
- Sauer, M. Market Report 2024. 2025. Available online: https://composites-united.com/en/composites-united-market-report-2024-published-overview-of-the-current-composites-market-environment/ (accessed on 21 March 2025).
- Das, S. Life cycle assessment of carbon fiber-reinforced polymer composites. Int. J. Life Cycle Assess. 2011, 16, 268–282. [Google Scholar] [CrossRef]
- Arnold, U.; Palmenaer, A.d.; Brück, T.; Kuse, K. Energy-Efficient Carbon Fiber Production with Concentrated Solar Power: Process Design and Techno-economic Analysis. Ind. Eng. Chem. Res. 2018, 57, 7934–7945. [Google Scholar] [CrossRef]
- Liddell, H.; Brueske, S.; Carpenter, A.; Cresko, J. Manufacturing Energy Intensity and Opportunity Analysis for Fiber-Reinforced Polymer Composites and Other Lightweight Materials. In Proceedings of the American Society for Composites: 31st Technical Conference, Williamsburg, VA, USA, 19–22 September 2016. [Google Scholar]
- Suzuki, T.; Takahashi, J. LCA of Lightweight Vehicles by Using CFRP for Mass-Produced Vehicles. In Proceedings of the Fifteenth International Conference on Composite Materials, Durban, South Africa, 27 June–1 July 2005. [Google Scholar]
- Liddell, H.; Dollinger, C.; Fisher, A.; Brueske, S. Bandwidth Study on Energy Use and Potential Energy Saving Opportunities in U.S. Carbon Fiber Reinforced Polymer Manufacturing, United States. 2017. Available online: https://www.osti.gov/biblio/1513860 (accessed on 21 March 2025).
- Spini, F.; Bettini, P. End-of-Life wind turbine blades: Review on recycling strategies. Compos. Part B Eng. 2024, 275, 111290. [Google Scholar] [CrossRef]
- Beauson, J.; Laurent, A.; Rudolph, D.P.; Pagh Jensen, J. The complex end-of-life of wind turbine blades: A review of the European context. Renew. Sustain. Energy Rev. 2022, 155, 111847. [Google Scholar] [CrossRef]
- Goergen, C.; Schommer, D.; Duhovic, M.; Mitschang, P. Deep drawing of organic sheets made of hybrid recycled carbon and thermoplastic polyamide 6 staple fiber yarns. J. Thermoplast. Compos. Mater. 2020, 33, 754–778. [Google Scholar] [CrossRef]
- Goergen, C.; Baz, S.; Mitschang, P.; Gresser, G.T. Recycled carbon fibers in complex structural parts—Organic sheets made of rCF staple fiber yarns. KEM 2017, 742, 602–609. [Google Scholar] [CrossRef]
- Connor, M.L. Characterization of Recycled Carbon Fibers and Their Formation of Composites Using Injection Molding. Master Thesis, North Carolina State University, Raleigh, NC, USA, 2008. [Google Scholar]
- Stoeffler, K.; Andjelic, S.; Legros, N.; Roberge, J.; Schougaard, S.B. Polyphenylene sulfide (PPS) composites reinforced with recycled carbon fiber. Compos. Sci. Technol. 2013, 84, 65–71. [Google Scholar] [CrossRef]
- Wölling, J.; Schmieg, M.; Manis, F.; Drechsler, K. Nonwovens from Recycled Carbon Fibres—Comparison of Processing Technologies. Procedia CIRP 2017, 66, 271–276. [Google Scholar] [CrossRef]
- Abdkader, A.; Khurshid, M.F.; Hasan, M.M.B.; Cherif, C. Recent developments in yarn formation technology for producing innovative hybrid yarn structures from staple carbon and thermoplastic fibers for high-performance composites. J. Compos. Mater. 2023, 57, 1343–1362. [Google Scholar] [CrossRef]
- Ishikawa, T.; Amaoka, K.; Masubuchi, Y.; Yamamoto, T.; Yamanaka, A.; Arai, M.; Takahashi, J. Overview of automotive structural composites technology developments in Japan. Compos. Sci. Technol. 2018, 155, 221–246. [Google Scholar] [CrossRef]
- Rimmel, O.; May, D.; Goergen, C.; Poeppel, A.; Mitschang, P. Development and validation of recycled carbon fiber-based binder tapes for automated tape laying processes. J. Compos. Mater. 2019, 53, 3257–3268. [Google Scholar] [CrossRef]
- Akonda, M.H.; Lawrence, C.A.; Weager, B.M. Recycled carbon fibre-reinforced polypropylene thermoplastic composites. Compos. Part A Appl. Sci. Manuf. 2012, 43, 79–86. [Google Scholar] [CrossRef]
- Akonda, M.H.; EL-Dessouky, H.M.; Lawrence, C.A.; Weager, B.M. A novel non-crimped thermoplastic fabric prepreg from waste carbon and polyester fibres. J. Compos. Mater. 2014, 48, 843–851. [Google Scholar] [CrossRef]
- Wellekötter, J.; Baz, S.; Schwingel, J.; Gresser, G.T.; Middendorf, P.; Bonten, C. Recycling of composites—A new approach minimizes downgrading. AIP Conf. Proc. 2019, 2055, 60009. [Google Scholar] [CrossRef]
- Abdkader, A.; Bachor, S.; Hasan, M.M.B.; Cherif, C. Development of yarns from recycled carbon fiber based on friction spinning technology with specific properties for thermoset composites. Text. Res. J. 2024, 94, 12–23. [Google Scholar] [CrossRef]
- Goergen, C.; Baz, S.; Reichert, O.; Mitschang, P.; Gresser, G.T. Tiefziehbare Organobleche aus recycelten Carbonfasern. Z. Kunststofftechnik 2019, 15, 54–94. [Google Scholar] [CrossRef]
- Shehab, E.; Meiirbekov, A.; Amantayeva, A.; Tokbolat, S. Cost Modelling for Recycling Fiber-Reinforced Composites: State-of-the-Art and Future Research. Polymers 2022, 15, 150. [Google Scholar] [CrossRef]
- Leon, A.d.; Sweat, R.D. Interfacial Engineering of CFRP Composites and Temperature Effects: A Review. Mech. Compos. Mater. 2023, 59, 419–440. [Google Scholar] [CrossRef]
- Yu, H.; Potter, K.D.; Wisnom, M.R. A novel manufacturing method for aligned discontinuous fibre composites (High Performance-Discontinuous Fibre method). Compos. Part A Appl. Sci. Manuf. 2014, 65, 175–185. [Google Scholar] [CrossRef]
- Hasan, M.M.; Bachor, S.; Abdkader, A.; Cherif, C. Tensile properties of thermoset composites based on yarn structures from recycled carbon fibre and low melting temperature Co-polyamide fibre. J. Compos. Mater. 2024, 58, 55–64. [Google Scholar] [CrossRef]
- Wang, J.; Tayari Akankwasa, N.; Zhang, Y.; Shi, Q.; Li, L. The three-dimensional model of staple yarn formation simulated from randomly assembled fibers. Text. Res. J. 2020, 90, 866–876. [Google Scholar] [CrossRef]
- Deng, W.; Wang, X.; Ke, W.; Wang, C.; Deng, Z. Parametric 3D simulations of spun yarns and fabrics. J. Text. Inst. 2024, 115, 1413–1422. [Google Scholar] [CrossRef]
- Zhang, H.; Jabbar, A.; Li, A.; Wang, X.; Yang, D.; Tausif, M. Image-based finite element modelling of fibre dynamics in polyester staple spun yarns. Compos. Sci. Technol. 2025, 261, 111036. [Google Scholar] [CrossRef]
- Lang, T.G.; Hasan, M.M.B.; Abdkader, A.; Cherif, C.; Gereke, T. Micro-scale model of rCF/PA6 spun yarn composite. J. Compos. Sci. 2023, 7, 66. [Google Scholar] [CrossRef]
- Lang, T.G.; Hasan, M.M.B.; Abdkader, A.; Cherif, C.; Gereke, T. Micromechanical Modelling of the Deformation Mechanisms of Friction-Spun Yarn from Recycled Carbon Fibres. MSF 2024, 1117, 47–53. [Google Scholar] [CrossRef]
- Gereke, T.; Cherif, C. A review of numerical models for 3D woven composite reinforcements. Compos. Struct. 2019, 209, 60–66. [Google Scholar] [CrossRef]
- Wielhorski, Y.; Mendoza, A.; Rubino, M.; Roux, S. Numerical modeling of 3D woven composite reinforcements: A review. Compos. Part A Appl. Sci. Manuf. 2022, 154, 106729. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Diestel, O.; Krzywinski, S.; Cherif, C. Decoupling the bending behavior and the membrane properties of finite shell elements for a correct description of the mechanical behavior of textiles with a laminate formulation. J. Ind. Text. 2013, 44, 70–84. [Google Scholar] [CrossRef]
- Boisse, P.; Colmars, J.; Hamila, N.; Naouar, N.; Steer, Q. Bending and wrinkling of composite fiber preforms and prepregs. A review and new developments in the draping simulations. Compos. Part B Eng. 2018, 141, 234–249. [Google Scholar] [CrossRef]
- Thompson, A.J.; Belnoue, J.P.-H.; Hallett, S.R. Modelling defect formation in textiles during the double diaphragm forming process. Compos. Part B Eng. 2020, 202, 108357. [Google Scholar] [CrossRef]
- Chen, B.; Colmars, J.; Naouar, N.; Boisse, P. A hypoelastic stress resultant shell approach for simulations of textile composite reinforcement forming. Compos. Part A Appl. Sci. Manuf. 2021, 149, 106558. [Google Scholar] [CrossRef]
- Boisse, P.; Akkerman, R.; Carlone, P.; Kärger, L.; Lomov, S.V.; Sherwood, J.A. Advances in composite forming through 25 years of ESAFORM. Int. J. Mater. Form. 2022, 15, 39. [Google Scholar] [CrossRef]
- Sun, X.; Belnoue, J.P.-H.; Thompson, A.; Said, B.E.; Hallett, S.R. Dry Textile Forming Simulations: A Benchmarking Exercise. Front. Mater. 2022, 9, 831820. [Google Scholar] [CrossRef]
- Boisse, P.; Hamila, N.; Madeo, A. Modelling the development of defects during composite reinforcements and prepreg forming. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 20150269. [Google Scholar] [CrossRef] [PubMed]
- Coutandin, S.; Brandt, D.; Heinemann, P.; Ruhland, P.; Fleischer, J. Influence of punch sequence and prediction of wrinkling in textile forming with a multi-punch tool. Prod. Eng. Res. Devel. 2018, 12, 779–788. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Cherif, C. Modeling the mechanical properties of textile-reinforced composites with a near micro-scale approach. Compos. Struct. 2016, 135, 1–7. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Hengstermann, M.; Cherif, C. Microscale finite element model of brittle multifilament yarn failure behavior. J. Ind. Text. 2018, 47, 870–882. [Google Scholar] [CrossRef]
- Daelemans, L.; Faes, J.; Allaoui, S.; Hivet, G.; Dierick, M.; van Hoorebeke, L.; van Paepegem, W. Finite element simulation of the woven geometry and mechanical behaviour of a 3D woven dry fabric under tensile and shear loading using the digital element method. Compos. Sci. Technol. 2016, 137, 177–187. [Google Scholar] [CrossRef]
- Teijin Limited. Tenax Filament Yarn: Product Data Sheet. 2024. Available online: https://dragonplate.com/images/uploaded/pdfs/fiberspecs/filament-product_programm__eu__v27_2018-06-27_en.pdf (accessed on 21 March 2025).
- Orawattanasrikul, S. Experimentelle Analyse der Scherdeformationen Biaxial Verstärkter Mehrlagengestricke. Ph.D. Thesis, TU Dresden, Dresden, Germany, 2006. [Google Scholar]
- Taha, I.; Abdin, Y.; Ebeid, S. Comparison of picture frame and Bias-Extension tests for the characterization of shear behaviour in natural fibre woven fabrics. Fibers Polym. 2013, 14, 338–344. [Google Scholar] [CrossRef]
- Nosrat Nezami, F.; Gereke, T.; Cherif, C. Analyses of interaction mechanisms during forming of multilayer carbon woven fabrics for composite applications. Compos. Part A Appl. Sci. Manuf. 2016, 84, 406–416. [Google Scholar] [CrossRef]
- Andrade, F.X.C.; Feucht, M.; Haufe, A.; Neukamm, F. An incremental stress state dependent damage model for ductile failure prediction. Int. J. Fract. 2016, 200, 127–150. [Google Scholar] [CrossRef]












| Type of Fiber | Fiber Length | Linear Density | Diameter | Tensile Strength | Young’s Modulus | Elongation at Break | |
|---|---|---|---|---|---|---|---|
| (mm) | (dtex) | (μm) | (cN/Tex) | (MPa) | (GPa) | (%) | |
| rCF | 60 (5) | 0.5 (0.1) | 5.8 (3.0) | 220.0 (55.6) | 3868 (1368) | 268.9 (59.6) | 1.6 (0.3) |
| Co-polyamide | 100 (2) | 11.2 (0.6) | 36.6 (8.8) | 25.1 (1.8) | 268 (20) | 0.5 (0.1) | 147.9 (13.9) |
| Type of Yarn | Fmax (N) | Elongation at Fmax (%) | Elongation at Break (%) |
|---|---|---|---|
| rCF-DREF 800 tex | 23.4 (7.9) | 1.74 (0.31) | 9.96 (3.58) |
| rCF-DREF 1600 tex | 37.6 (10.6) | 2.64 (0.43) | 12.26 (3.77) |
| CF roving 800 tex [46] | 2011 | 1.8 | 1.8 |
| CF roving 1600 tex [46] | 4022 | 1.8 | 1.8 |
| Parameter | a | k | Location | |
|---|---|---|---|---|
| Value | 4.878 | 0.934 | 5.549 | 7.692 |
| Type of Yarn | Test Direction | Fmax (N) | Elongation at Fmax (%) | Elongation at Break (%) |
|---|---|---|---|---|
| rCF-DREF 800 tex | Warp direction | 528.4 (120.9) | 3.40 (0.43) | 22.63 (4.17) |
| Weft direction | 558.2 (95.3) | 8.71 (1.55) | 27.02 (2.25) | |
| CF 800 tex | Warp direction | 10,278.8 (300.5) | 0.95 (0.10) | - |
| Weft direction | 9414.2 (745.6) | 1.04 (0.16) | - |
| Direction | Overhang Length (mm) | Bending Stiffness (mN cm) |
|---|---|---|
| Warp | 157.6 (11.3) | 291.4 (53.4) |
| Weft | 137.2 (4.5) | 188.4 (19.3) |
| Direction | Static Friction Coefficient | Dynamic Friction Coefficient |
|---|---|---|
| rCF Warp | 0.312 (0.015) | 0.306 (0.010) |
| rCF Weft | 0.305 (0.008) | 0.307 (0.010) |
| CF Warp | 0.255 (0.008) | 0.233 (0.003) |
| CF Weft | 0.261 (0.005) | 0.232 (0.001) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, T.G.; Hasan, M.M.B.; Abdkader, A.; Cherif, C.; Gereke, T. Analysis of the Deformation Mechanisms of Fabrics Based on rCF Staple Fiber Yarns for Thermoset Composite Applications. J. Compos. Sci. 2025, 9, 173. https://doi.org/10.3390/jcs9040173
Lang TG, Hasan MMB, Abdkader A, Cherif C, Gereke T. Analysis of the Deformation Mechanisms of Fabrics Based on rCF Staple Fiber Yarns for Thermoset Composite Applications. Journal of Composites Science. 2025; 9(4):173. https://doi.org/10.3390/jcs9040173
Chicago/Turabian StyleLang, Tobias Georg, Mir Mohammad Badrul Hasan, Anwar Abdkader, Chokri Cherif, and Thomas Gereke. 2025. "Analysis of the Deformation Mechanisms of Fabrics Based on rCF Staple Fiber Yarns for Thermoset Composite Applications" Journal of Composites Science 9, no. 4: 173. https://doi.org/10.3390/jcs9040173
APA StyleLang, T. G., Hasan, M. M. B., Abdkader, A., Cherif, C., & Gereke, T. (2025). Analysis of the Deformation Mechanisms of Fabrics Based on rCF Staple Fiber Yarns for Thermoset Composite Applications. Journal of Composites Science, 9(4), 173. https://doi.org/10.3390/jcs9040173







