Microstructural Analysis of the Transverse and Shear Behavior of Additively Manufactured CFRP Composite RVEs Based on the Phase-Field Fracture Theory
Abstract
:1. Introduction
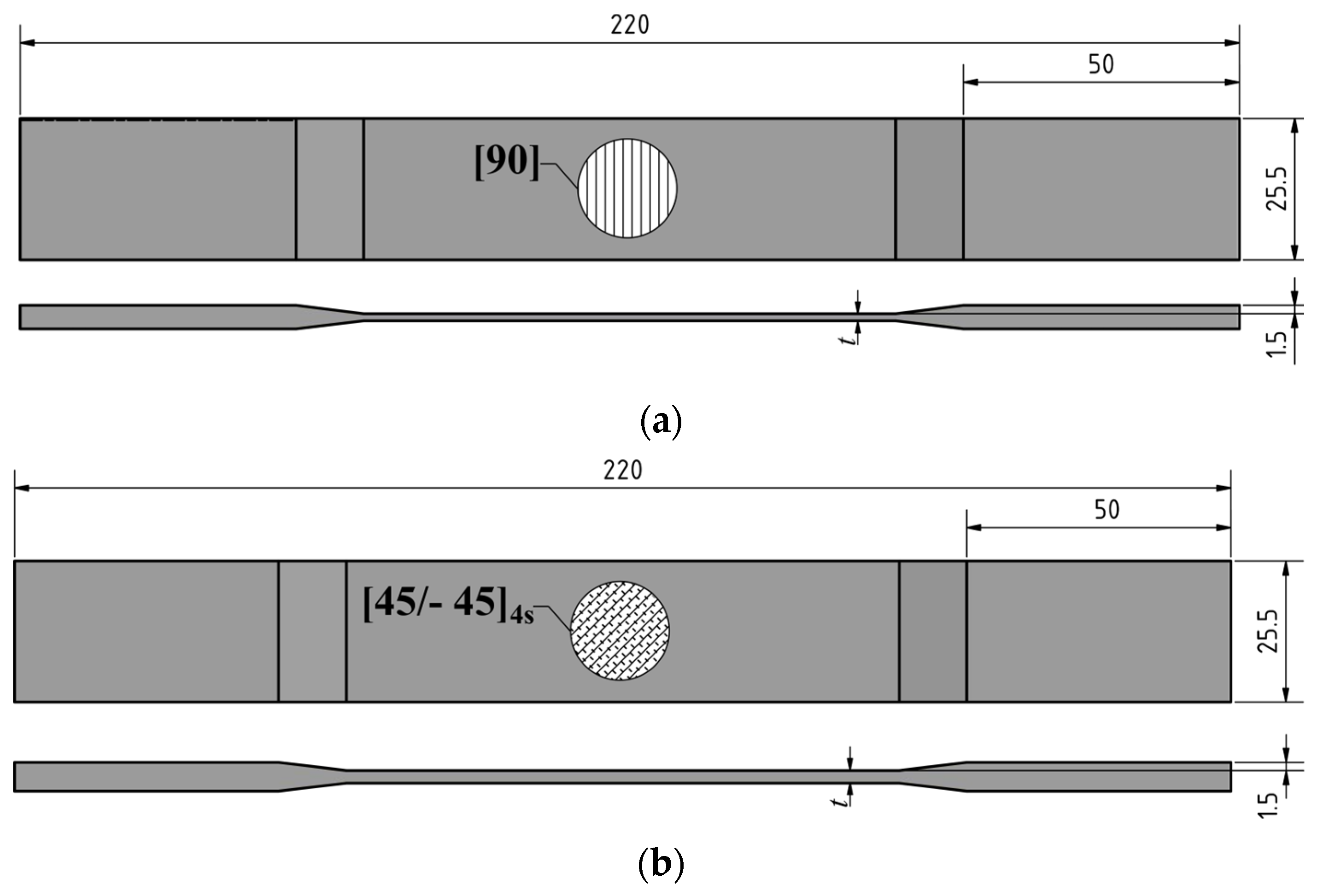
2. Materials and Methods
3. Results
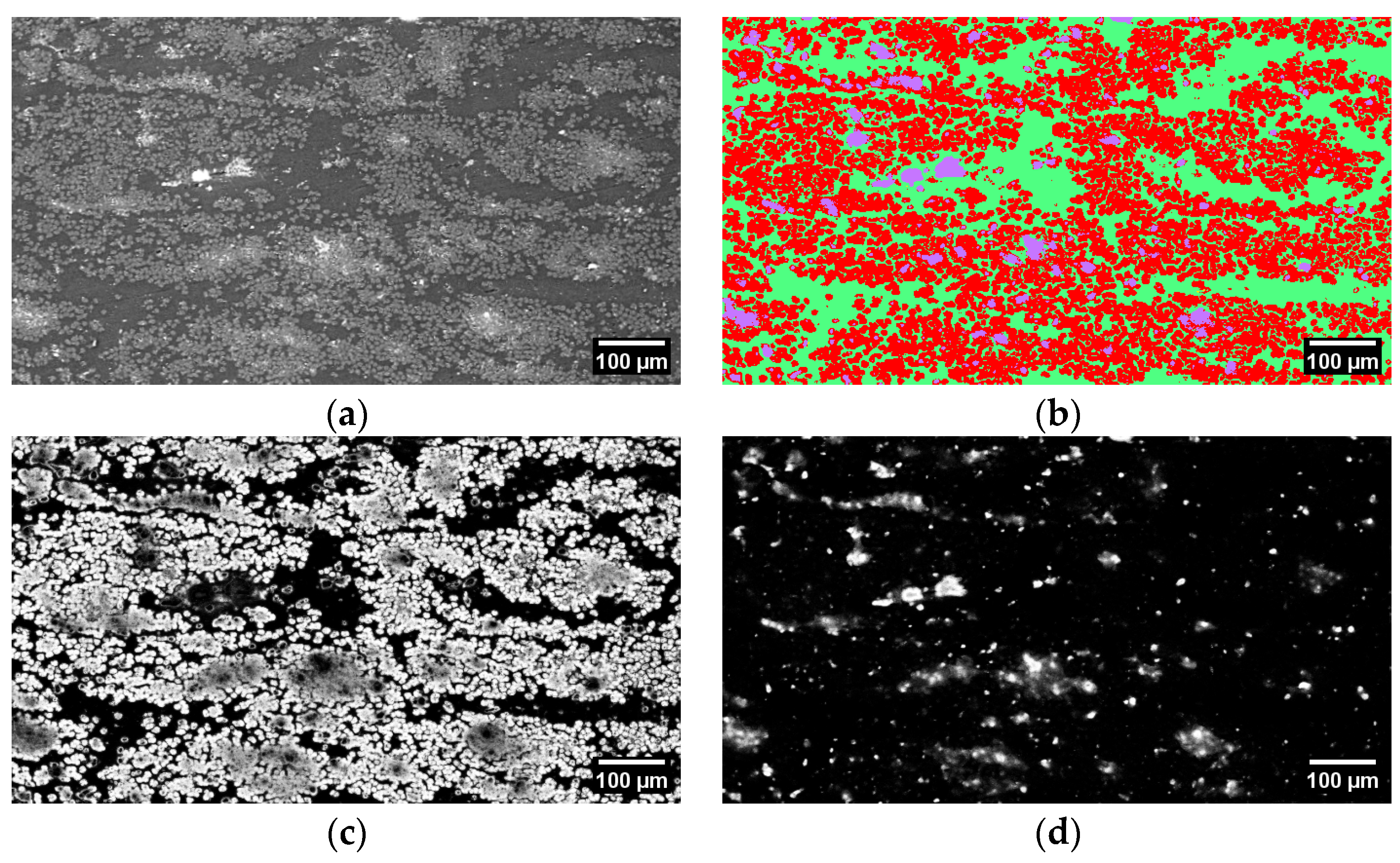
3.1. Microstructural Inspection
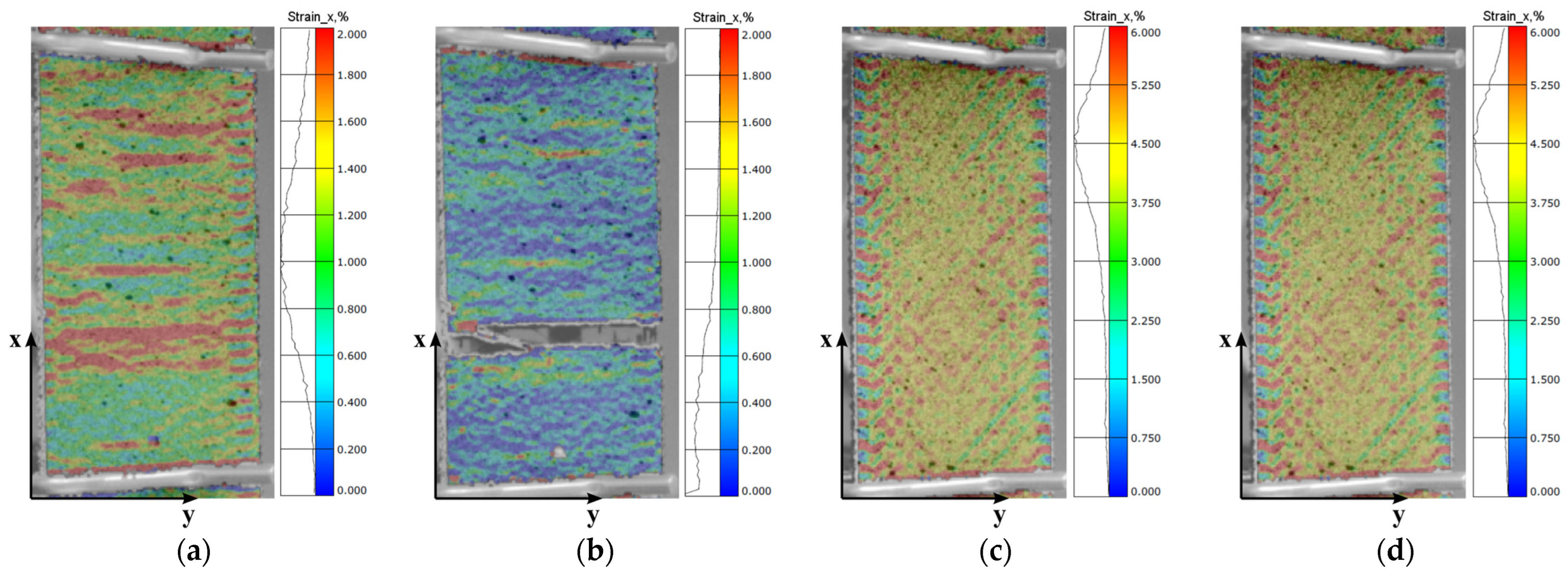
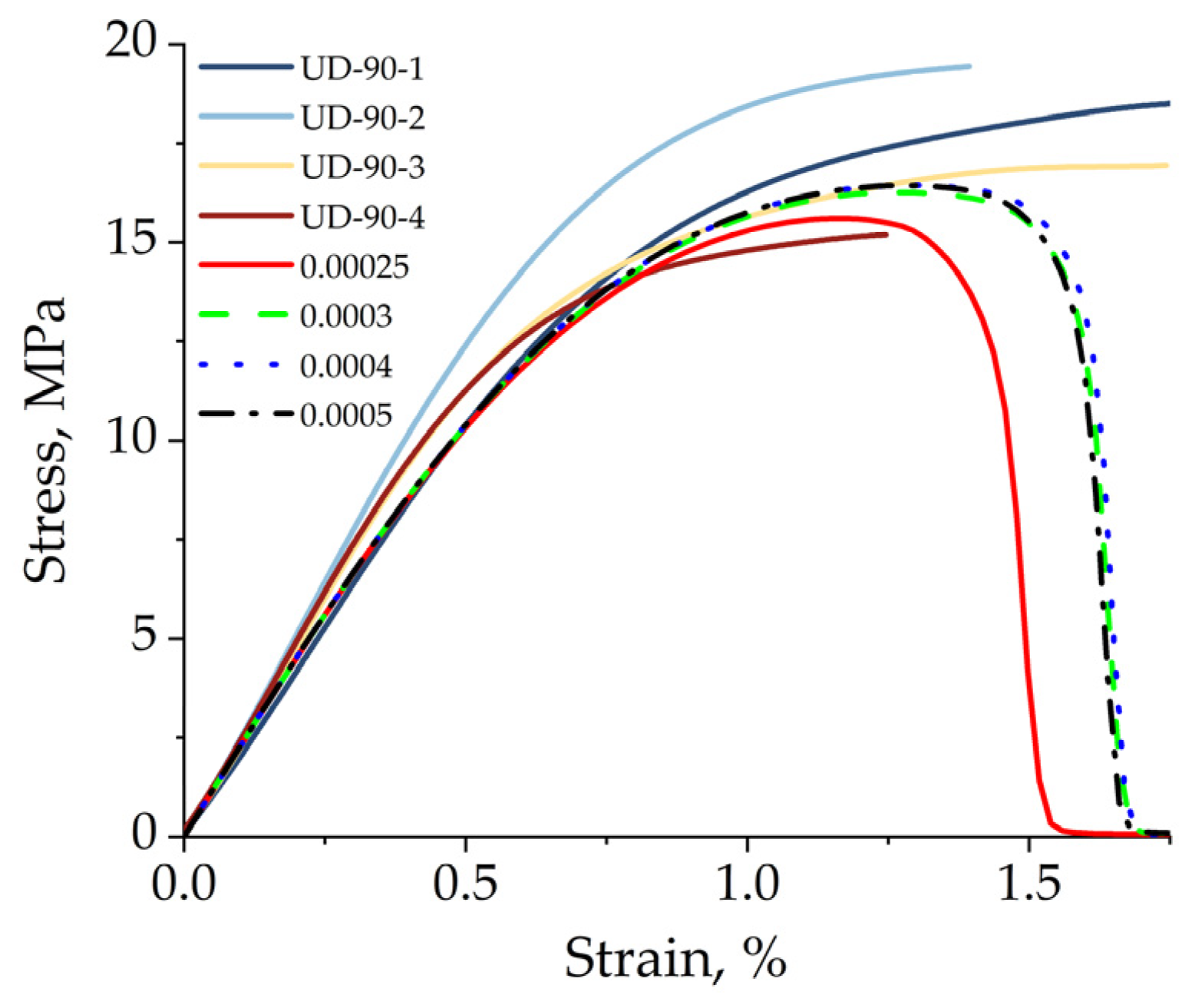
3.2. Experimental Acquisition of Lamina Properties
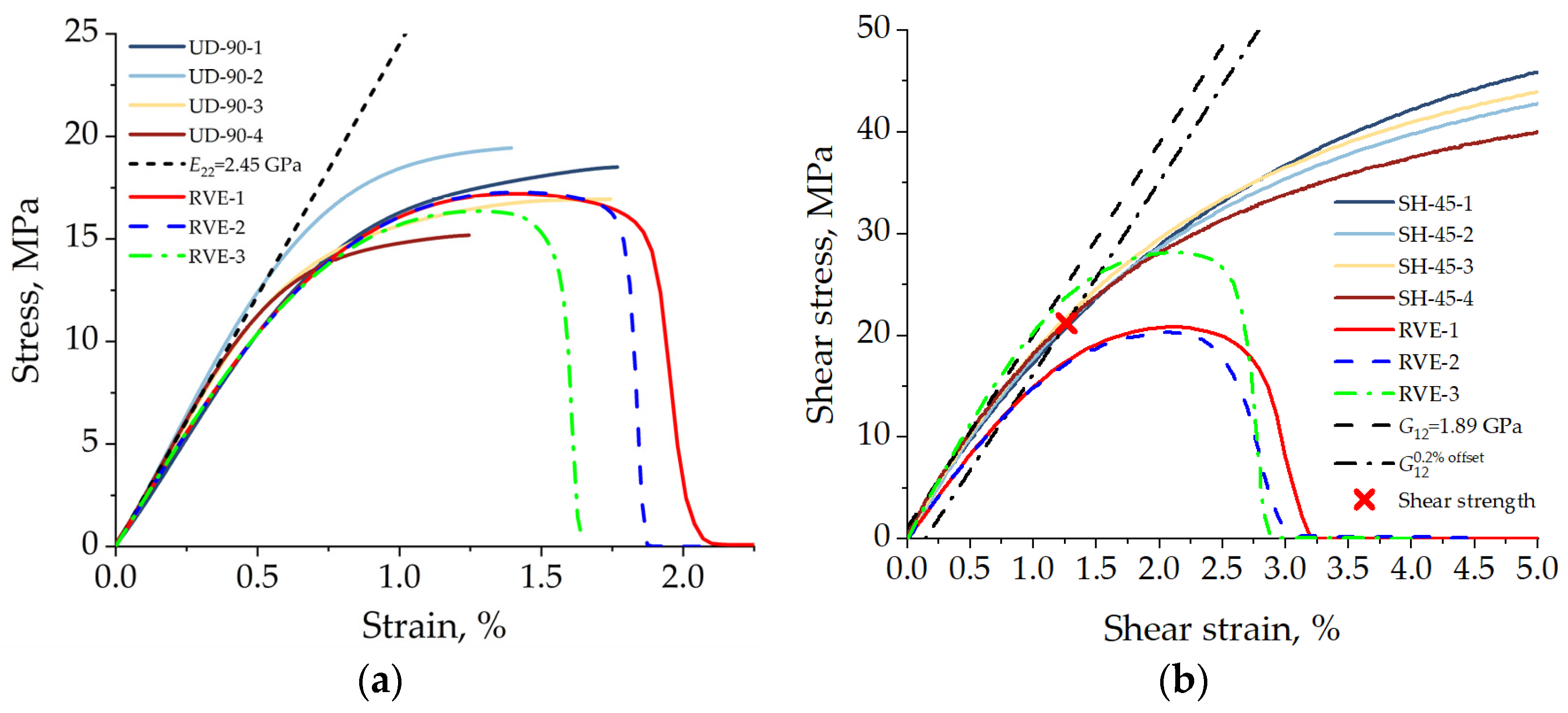
3.3. RVE Design
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Reprints
Conflicts of Interest
Appendix A
- Define the user material with five material properties including the Young’s modulus , Poisson’s ratio , phase field length scale , fracture toughness , and the tensile strength which is applicable for the phase field cohesive zone models, while otherwise neglected
- Set a solution-dependent state variable (SDV)
- Define the material conductivity equal to one
- State the analysis step as coupled temperature-displacement with steady-state or transient options, a constant increment size, a separated solution technique, and symmetric equation solver matrix storage
- Change the values of the solution controls parameters to 5000, to avoid convergence problems due to large number of iterations
- Define the initial temperature condition equal to zero to describe the undamaged material in the initial step
- Adopt the element type as coupled temperature–displacement
References
- Alghamdi, S.S.; John, S.; Choudhury, N.R.; Dutta, N.K. Additive Manufacturing of Polymer Materials: Progress, Promise and Challenges. Polymers 2021, 13, 753. [Google Scholar] [CrossRef]
- Ferreira, I.; Machado, M.; Alves, F.; Torres Marques, A. A Review on Fibre Reinforced Composite Printing via FFF. Rapid Prototyp. J. 2019, 25, 972–988. [Google Scholar] [CrossRef]
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive Manufacturing (3D Printing): A Review of Materials, Methods, Applications and Challenges. Compos. Part B 2018, 143, 172–196. [Google Scholar] [CrossRef]
- van de Werken, N.; Tekinalp, H.; Khanbolouki, P.; Ozcan, S.; Williams, A.; Tehrani, M. Additively Manufactured Carbon Fiber-Reinforced Composites: State of the Art and Perspective. Addit. Manuf. 2020, 31, 100962. [Google Scholar] [CrossRef]
- van de Werken, N. Additively Manufactured Continuous Carbon Fiber Thermoplastic Composites for High-Performance Applications. Doctoral Dissertation, The University of New Mexico, Albuquerque, NM, USA, 2019. [Google Scholar]
- Karaş, B.; Smith, P.J.; Fairclough, J.P.A.; Mumtaz, K. Additive Manufacturing of High Density Carbon Fibre Reinforced Polymer Composites. Addit. Manuf. 2022, 58, 103044. [Google Scholar] [CrossRef]
- Rengier, F.; Mehndiratta, A.; Von Tengg-Kobligk, H.; Zechmann, C.M.; Unterhinninghofen, R.; Kauczor, H.U.; Giesel, F.L. 3D Printing Based on Imaging Data: Review of Medical Applications. Int. J. Comput. Assist. Radiol. Surg. 2010, 5, 335–341. [Google Scholar] [CrossRef]
- Schubert, C.; Van Langeveld, M.C.; Donoso, L.A. Innovations in 3D Printing: A 3D Overview from Optics to Organs. Br. J. Ophthalmol. 2014, 98, 159–161. [Google Scholar] [CrossRef]
- Diegel, O.; Nordin, A.; Motte, D. Additive Manufacturing Technologies; Springer: Berlin/Heidelberg, Germany, 2019; ISBN 9781493921126. [Google Scholar]
- Abazari, S.; Shamsipur, A.; Bakhsheshi-Rad, H.R.; Ismail, A.F. Carbon Nanotubes (CNTs)-Reinforced Magnesium-Based Matrix Composites: A Comprehensive Review. J. Higher Educ. 2020, 13, 38. [Google Scholar] [CrossRef]
- Abazari, S.; Shamsipur, A.; Bakhsheshi-Rad, H.R.; Ramakrishna, S.; Berto, F. Graphene Family Nanomaterial Reinforced Magnesium-Based Matrix Composites for Biomedical Application: A Comprehensive Review. Metals 2020, 10, 1002. [Google Scholar] [CrossRef]
- Monfared, V.; Bakhsheshi-Rad, H.R.; Ramakrishna, S.; Razzaghi, M.; Berto, F. A Brief Review on Additive Manufacturing of Polymeric Composites and Nanocomposites. Micromachines 2021, 12, 24. [Google Scholar] [CrossRef]
- Gljušćić, M.; Franulović, M.; Lanc, D.; Žerovnik, A. Representative Volume Element for Microscale Analysis of Additively Manufactured Composites. Addit. Manuf. 2022, 56, 102902. [Google Scholar] [CrossRef]
- Gljušćić, M.; Franulović, M.; Žužek, B.; Žerovnik, A. Experimental Validation of Progressive Damage Modeling in Additively Manufactured Continuous Fiber Composites. Compos. Struct. 2022, 295, 115869. [Google Scholar] [CrossRef]
- Iragi, M.; Pascual-González, C.; Esnaola, A.; Lopes, C.S.; Aretxabaleta, L. Ply and Interlaminar Behaviours of 3D Printed Continuous Carbon Fibre-Reinforced Thermoplastic Laminates; Effects of Processing Conditions and Microstructure. Addit. Manuf. 2019, 30, 100884. [Google Scholar] [CrossRef] [Green Version]
- Carlota, V. Essentium’s Latest Survey: What Is the Future of Industrial 3D Printing? Available online: https://www.3dnatives.com/en/essentium-190320195/ (accessed on 15 October 2022).
- Gide, K.M.; Islam, S.; Bagheri, Z.S. Polymer-Based Materials Built with Additive Manufacturing Methods for Orthopedic Applications: A Review. J. Compos. Sci. 2022, 6, 262. [Google Scholar] [CrossRef]
- Deeba, F.; Shrivastava, K.; Bafna, M.; Jain, A. Tuning of Dielectric Properties of Polymers by Composite Formation: The Effect of Inorganic Fillers Addition. J. Compos. Sci. 2022, 6, 355. [Google Scholar] [CrossRef]
- Tran, C.C.; Nguyen, Q.K. An Efficient Method to Determine the Thermal Behavior of Composite Material with Loading High Thermal Conductivity Fillers. J. Compos. Sci. 2022, 6, 214. [Google Scholar] [CrossRef]
- Kabir, S.M.F.; Mathur, K.; Seyam, A.F.M. Maximizing the Performance of 3d Printed Fiber-Reinforced Composites. J. Compos. Sci. 2021, 5, 136. [Google Scholar] [CrossRef]
- Melenka, G.W.; Cheung, B.K.O.; Schofield, J.S.; Dawson, M.R.; Carey, J.P. Evaluation and Prediction of the Tensile Properties of Continuous Fiber-Reinforced 3D Printed Structures. Compos. Struct. 2016, 153, 866–875. [Google Scholar] [CrossRef]
- He, Q.; Wang, H.; Fu, K.; Ye, L. 3D Printed Continuous CF/PA6 Composites: Effect of Microscopic Voids on Mechanical Performance. Compos. Sci. Technol. 2020, 191, 108077. [Google Scholar] [CrossRef]
- Baechle-Clayton, M.; Loos, E.; Taheri, M.; Taheri, H. Failures and Flaws in Fused Deposition Modeling (FDM) Additively Manufactured Polymers and Composites. J. Compos. Sci. 2022, 6, 202. [Google Scholar] [CrossRef]
- Suquet, P. Continuum Micromechanics; Kaliszky, S., Sayir, M., Schneider, W., Bianchi, G., Tasso, C., Eds.; Springer: Berlin/Heidelberg, Germany, 1997; ISBN 9783211829028. [Google Scholar]
- Michel, J.C.; Moulinec, H.; Suquet, P. Effective Properties of Composite Materials with Periodic Microstructure: A Computational Approach. Comput. Methods Appl. Mech. Eng. 1999, 172, 109–143. [Google Scholar] [CrossRef]
- Rémond, Y.; Ahzi, S. Applied RVE Reconstruction and Homogenization of Heterogeneous Materials; John Wiley & Sons: Hoboken, NJ, USA, 2016; ISBN 9781848219014. [Google Scholar]
- Soutis, C.; Beaumont, P.W.R. Multi-Scale Modelling of Composite Material Systems: The Art of Predictive Damage Modelling; Woodhead Publishing Limited: Sawston, UK; CRC Press LLC: Boca Raton, FL, USA, 2005; Volume 3, ISBN 978-1-85573-936-9. [Google Scholar]
- Múgica, J.I.; Lopes, C.S.; Naya, F.; Herráez, M.; Martínez, V.; González, C. Multiscale Modelling of Thermoplastic Woven Fabric Composites: From Micromechanics to Mesomechanics. Compos. Struct. 2019, 228, 111340. [Google Scholar] [CrossRef] [Green Version]
- Riaño, L.; Joliff, Y. An ABAQUSTM Plug-in for the Geometry Generation of Representative Volume Elements with Randomly Distributed Fibers and Interphases. Compos. Struct. 2019, 209, 644–651. [Google Scholar] [CrossRef]
- Raju, B.; Hiremath, S.R.; Roy Mahapatra, D. A Review of Micromechanics Based Models for Effective Elastic Properties of Reinforced Polymer Matrix Composites. Compos. Struct. 2018, 204, 607–619. [Google Scholar] [CrossRef]
- Omairey, S.L.; Dunning, P.D.; Sriramula, S. Development of an ABAQUS Plugin Tool for Periodic RVE Homogenisation. Eng. Comput. 2019, 35, 567–577. [Google Scholar] [CrossRef] [Green Version]
- Akpoyomare, A.I.; Okereke, M.I.; Bingley, M.S. Virtual Testing of Composites: Imposing Periodic Boundary Conditions on General Finite Element Meshes. Compos. Struct. 2017, 160, 983–994. [Google Scholar] [CrossRef]
- Okereke, M.I.; Akpoyomare, A.I. A Virtual Framework for Prediction of Full-Field Elastic Response of Unidirectional Composites. Comput. Mater. Sci. 2013, 70, 82–99. [Google Scholar] [CrossRef]
- Kempesis, D.; Iannucci, L.; Ramesh, K.T.; Del Rosso, S.; Curtis, P.T.; Pope, D.; Duke, P.W. Micromechanical Analysis of High Fibre Volume Fraction Polymeric Laminates Using Micrograph-Based Representative Volume Element Models. Compos. Sci. Technol. 2022, 229, 109680. [Google Scholar] [CrossRef]
- Schindelin, J.; Arganda-Carreras, I.; Frise, E.; Kaynig, V.; Longair, M.; Pietzsch, T.; Preibisch, S.; Rueden, C.; Saalfeld, S.; Schmid, B.; et al. Fiji: An Open-Source Platform for Biological-Image Analysis. Nat. Methods 2012, 9, 676–682. [Google Scholar] [CrossRef] [Green Version]
- Breite, C.; Melnikov, A.; Turon, A.; de Morais, A.B.; Le Bourlot, C.; Maire, E.; Schöberl, E.; Otero, F.; Mesquita, F.; Sinclair, I.; et al. Detailed Experimental Validation and Benchmarking of Six Models for Longitudinal Tensile Failure of Unidirectional Composites. Compos. Struct. 2022, 279, 114828. [Google Scholar] [CrossRef]
- Liu, Y.; Straumit, I.; Vasiukov, D.; Lomov, S.V.; Panier, S. Multi-Scale Material Model for 3D Composite Using Micro CT Images Geometry Reconstruction. In Proceedings of the 7th European Conference on Composite Materials (ECCM), Munich, Germany, 26–30 June 2016. [Google Scholar]
- Straumit, I.; Lomov, S.V.; Wevers, M. Quantification of the Internal Structure and Automatic Generation of Voxel Models of Textile Composites from X-Ray Computed Tomography Data. Compos. Part A Appl. Sci. Manuf. 2015, 69, 150–158. [Google Scholar] [CrossRef]
- Dean, A.; Asur Vijaya Kumar, P.K.; Reinoso, J.; Gerendt, C.; Paggi, M.; Mahdi, E.; Rolfes, R. A Multi Phase-Field Fracture Model for Long Fiber Reinforced Composites Based on the Puck Theory of Failure. Compos. Struct. 2020, 251, 112446. [Google Scholar] [CrossRef]
- Guillén-Hernández, T.; García, I.G.; Reinoso, J.; Paggi, M. A Micromechanical Analysis of Inter-Fiber Failure in Long Reinforced Composites Based on the Phase Field Approach of Fracture Combined with the Cohesive Zone Model. Int. J. Fract. 2019, 220, 181–203. [Google Scholar] [CrossRef]
- Tan, W.; Martínez-Pañeda, E. Phase Field Fracture Predictions of Microscopic Bridging Behaviour of Composite Materials. Compos. Struct. 2022, 286, 115242. [Google Scholar] [CrossRef]
- Griffith, A.A., VI. The phenomena of rupture and flow in solids. Philos. Trans. R. Soc. Lond. 1921, 221, 163–198. [Google Scholar] [CrossRef] [Green Version]
- Biner, S.B. Programming Phase-Field Modeling; Springer: Cham, Switzerland, 2017; ISBN 9783319411965. [Google Scholar]
- Francfort, G.A.; Marigo, J.J. Revisiting Brittle Fracture as an Energy Minimization Problem. J. Mech. Phys. Solids 1998, 46, 1319–1342. [Google Scholar] [CrossRef]
- Bourdin, B.; Francfort, G.A.; Marigo, J. Numerical Experiments in Revisited Brittle Fracture. J. Mech. Phys. Solids 2000, 48, 797–826. [Google Scholar] [CrossRef]
- Navidtehrani, Y.; Betegón, C.; Martínez-Pañeda, E. A Unified Abaqus Implementation of the Phase Field Fracture Method Using Only a User Material Subroutine. Materials 2021, 14, 1913. [Google Scholar] [CrossRef]
- Tourret, D.; Liu, H.; LLorca, J. Phase-Field Modeling of Microstructure Evolution: Recent Applications, Perspectives and Challenges. Prog. Mater. Sci. 2022, 123, 100810. [Google Scholar] [CrossRef]
- Egger, A.; Pillai, U.; Agathos, K.; Kakouris, E.; Chatzi, E.; Aschroft, I.A.; Triantafyllou, S.P. Discrete and Phase Field Methods for Linear Elastic Fracture Mechanics: A Comparative Study and State-of-the-Art Review. Appl. Sci 2019, 9, 2436. [Google Scholar] [CrossRef]
- Wu, J.; Huang, Y. Comprehensive Implementations of Phase-Field Damage Models in Abaqus. Theor. Appl. Fract. Mech. 2020, 106, 102440. [Google Scholar] [CrossRef]
- Simoes, M.; Martínez-pañeda, E. Phase Field Modelling of Fracture and Fatigue in Shape Memory Alloys. Comput. Methods Appl. Mech. Eng. 2021, 373, 113504. [Google Scholar] [CrossRef]
- Natarajan, S.; Annabattula, R.K.; Martínez-pañeda, E. Phase Field Modelling of Crack Propagation in Functionally Graded Materials. Compos. Part B 2019, 169, 239–248. [Google Scholar] [CrossRef] [Green Version]
- Asur Vijaya Kumar, P.K.; Dean, A.; Reinoso, J.; Lenarda, P.; Paggi, M. Phase Field Modeling of Fracture in Functionally Graded Materials: Γ-Convergence and Mechanical Insight on the Effect of Grading. Thin-Walled Struct. 2021, 159, 107234. [Google Scholar] [CrossRef]
- Miehe, C.; Schänzel, L. Phase Field Modeling of Fracture in Rubbery Polymers. Part I: Finite Elasticity Coupled with Brittle Failure. J. Mech. Phys. Solids 2014, 65, 93–113. [Google Scholar] [CrossRef]
- Loew, P.J.; Peters, B.; Beex, L.A.A. Rate-Dependent Phase-Field Damage Modeling of Rubber and Its Experimental Parameter Identification. J. Mech. Phys. Solids 2019, 127, 266–294. [Google Scholar] [CrossRef] [Green Version]
- Zhou, S.; Zhuang, X.; Rabczuk, T. Phase Field Modeling of Brittle Compressive-Shear Fractures in Rock-like Materials: A New Driving Force and a Hybrid Formulation. Comput. Methods Appl. Mech. Eng. 2019, 355, 729–752. [Google Scholar] [CrossRef]
- Schuler, L.; Ilgen, A.G.; Newell, P. Chemo-Mechanical Phase-Field Modeling of Dissolution-Assisted Fracture. Comput. Methods Appl. Mech. Eng. 2020, 362, 112838. [Google Scholar] [CrossRef]
- Kristensen, P.K.; Martínez-pañeda, E.; Engineering, M.; Lyngby, D.-K. Phase Field Fracture Modelling Using Quasi-Newton Methods and a New Adaptive Step Scheme. Theor. Appl. Fract. Mech. 2020, 107, 102446. [Google Scholar] [CrossRef] [Green Version]
- Abdollahi, A.; Arias, I. Phase-Field Modeling of Crack Propagation in Piezoelectric and Ferroelectric Materials with Different Electromechanical Crack Conditions. J. Mech. Phys. Solids 2012, 60, 2100–2126. [Google Scholar] [CrossRef]
- Pillai, U. Damage Modelling in Fibre-Reinforced Composite Laminates Using Phase Field Approach. Doctoral Dissertation, University of Nottingham, Nottingham, UK, 2021. [Google Scholar]
- Quintanas-corominas, A.; Reinoso, J.; Casoni, E.; Turon, A.; Mayugo, J.A. A Phase Field Approach to Simulate Intralaminar and Translaminar Fracture in Long Fiber Composite Materials. Compos. Struct. 2019, 220, 899–911. [Google Scholar] [CrossRef]
- Ahmadi, M. A Hybrid Phase Field Model for Fracture Induced by Lithium Diffusion in Electrode Particles of Li-Ion Batteries. Comput. Mater. Sci. 2020, 184, 109879. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Yvonnet, J.; Zhu, Q.Z.; Bornert, M.; Chateau, C. A Phase Field Method to Simulate Crack Nucleation and Propagation in Strongly Heterogeneous Materials from Direct Imaging of Their Microstructure. Eng. Fract. Mech. 2015, 139, 18–39. [Google Scholar] [CrossRef]
- Goswami, S.; Anitescu, C.; Rabczuk, T. Adaptive Phase Field Analysis with Dual Hierarchical Meshes for Brittle Fracture. Eng. Fract. Mech. 2019, 218, 106608. [Google Scholar] [CrossRef]
- Goswami, S.; Anitescu, C.; Rabczuk, T. Adaptive Fourth-Order Phase Field Analysis Using Deep Energy Minimization. Theor. Appl. Fract. Mech. 2020, 107, 102527. [Google Scholar] [CrossRef]
- Huynh, G.D.; Zhuang, X.; Nguyen-Xuan, H. Implementation Aspects of a Phase-Field Approach for Brittle Fracture. Front. Struct. Civ. Eng. 2019, 13, 417–428. [Google Scholar] [CrossRef]
- Msekh, M.A.; Sargado, J.M.; Jamshidian, M.; Areias, P.M.; Rabczuk, T. Abaqus Implementation of Phase-Field Model for Brittle Fracture. Comput. Mater. Sci. 2015, 96, 472–484. [Google Scholar] [CrossRef]
- Liu, G.; Li, Q.; Msekh, M.A.; Zuo, Z. Abaqus Implementation of Monolithic and Staggered Schemes for Quasi-Static and Dynamic Fracture Phase-Field Model. Comput. Mater. Sci. 2016, 121, 35–47. [Google Scholar] [CrossRef]
- Molnár, G.; Gravouil, A. 2D and 3D Abaqus Implementation of a Robust Staggered Phase-Field Solution for Modeling Brittle Fracture. Finite Elem. Anal. Des. 2017, 130, 27–38. [Google Scholar] [CrossRef] [Green Version]
- Pillai, U.; Heider, Y.; Markert, B. A Diffusive Dynamic Brittle Fracture Model for Heterogeneous Solids and Porous Materials with Implementation Using a User-Element Subroutine. Comput. Mater. Sci. 2018, 153, 36–47. [Google Scholar] [CrossRef]
- Bhowmick, S.; Liu, G.R. A Phase-Field Modeling for Brittle Fracture and Crack Propagation Based on the Cell-Based Smoothed Finite Element Method. Eng. Fract. Mech. 2018, 204, 369–387. [Google Scholar] [CrossRef]
- Seleš, K.; Lesičar, T.; Tonković, Z.; Sorić, J. A Residual Control Staggered Solution Scheme for the Phase-Field Modeling of Brittle Fracture. Eng. Fract. Mech. 2019, 205, 370–386. [Google Scholar] [CrossRef]
- Zhou, S.; Rabczuk, T.; Zhuang, X. Phase Field Modeling of Quasi-Static and Dynamic Crack Propagation: COMSOL Implementation and Case Studies. Adv. Eng. Softw. 2018, 122, 31–49. [Google Scholar] [CrossRef] [Green Version]
- Pham, K.; Amor, H.; Marigo, J.J.; Maurini, C. Gradient Damage Models and Their Use to Approximate Brittle Fracture. Int. J. Damage Mech. 2011, 20, 618–652. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.Y. A Unified Phase-Field Theory for the Mechanics of Damage and Quasi-Brittle Failure. J. Mech. Phys. Solids 2017, 103, 72–99. [Google Scholar] [CrossRef]
- Wu, J.Y. A Geometrically Regularized Gradient-Damage Model with Energetic Equivalence. Comput. Methods Appl. Mech. Eng. 2018, 328, 612–637. [Google Scholar] [CrossRef]
- Wu, J.Y.; Nguyen, V.P. A Length Scale Insensitive Phase-Field Damage Model for Brittle Fracture. J. Mech. Phys. Solids 2018, 119, 20–42. [Google Scholar] [CrossRef]
- Zhang, P.; Hu, X.; Wang, X.; Yao, W. An Iteration Scheme for Phase Field Model for Cohesive Fracture and Its Implementation in Abaqus. Eng. Fract. Mech. 2018, 204, 268–287. [Google Scholar] [CrossRef]
- Miehe, C.; Hofacker, M.; Welschinger, F. A Phase Field Model for Rate-Independent Crack Propagation: Robust Algorithmic Implementation Based on Operator Splits. Comput. Methods Appl. Mech. Eng. 2010, 199, 2765–2778. [Google Scholar] [CrossRef]
- Gerasimov, T.; De Lorenzis, L. A Line Search Assisted Monolithic Approach for Phase-Field Computing of Brittle Fracture. Comput. Methods Appl. Mech. Eng. 2016, 312, 276–303. [Google Scholar] [CrossRef]
- Navidtehrani, Y.; Betegón, C.; Martínez-Pañeda, E. A Simple and Robust Abaqus Implementation of the Phase Field Fracture Method. Appl. Eng. Sci. 2021, 6, 100050. [Google Scholar] [CrossRef]
- Gljušćić, M. Multiscale Modelling of Additively Manufactured Composite Material Behaviour. Doctoral Dissertation, University of Rijeka, Rijeka, Croatia, 2022. [Google Scholar]
- Iragi, M.; Pascual-Gonzalez, C.; Esnaola, A.; Aurrekoetxea, J.; Lopes, C.S.; Aretxabaleta, L. Characterization of Elastic and Resistance Behaviours of 3D Printed Continuous Carbon Fibre Reinforced Thermoplastics. In Proceedings of the ECCM18—18th European Conference on Composite Materials, Athens, Greece, 24–28 June 2018; pp. 24–28. [Google Scholar]
- Carraro, P.A.; Quaresimin, M. A Stiffness Degradation Model for Cracked Multidirectional Laminates with Cracks in Multiple Layers. Int. J. Solids Struct. 2014, 58, 34–51. [Google Scholar] [CrossRef]
- Okereke, M.; Keates, S. Finite Element Applications: A Practical Guide to the FEM Process; Seung-Bok, C., Habinin, D., Fu, Y., Guardiola, C., Sun, J.-Q., Eds.; Springer International Publishing: Cham, Switzerland, 2018; ISBN 978-3-319-67124-6. [Google Scholar]
- Pascual-González, C.; Iragi, M.; Fernández, A.; Fernández-Blázquez, J.P.; Aretxabaleta, L.; Lopes, C.S. An Approach to Analyse the Factors behind the Micromechanical Response of 3D-Printed Composites. Compos. Part B Eng. 2020, 186, 107820. [Google Scholar] [CrossRef]
- VDI/VDE 2479; Materials for Precision Engineering; Polyamide Moulding Materials Unreinforced. VDI-Verlag GmbH: Dusseldorf, Germany, 1978; Volume 1.
- MarkForged. Material Datasheet Composites; Markforged: Watertown, MA, USA, 2018. [Google Scholar]
- Nabavi, A.; Goroshin, S.; Frost, D.L.; Barthelat, F. Mechanical Properties of Chromium–Chromium Sulfide Cermets Fabricated by Self-Propagating High-Temperature Synthesis. J. Mater. Sci. 2015, 50, 3434–3446. [Google Scholar] [CrossRef]
- Navidtehrani, Y.; Martinez-Paneda, E. A Simple yet General ABAQUS Phase Field Fracture Implementation Using a UMAT Subroutine. Eng. Sci. 2021, 6, 100050. [Google Scholar]
- Ambati, M.; Gerasimov, T.; De Lorenzis, L. A Review on Phase-Field Models of Brittle Fracture and a New Fast Hybrid Formulation. Comput. Mech. 2015, 55, 383–405. [Google Scholar] [CrossRef]
- Amor, H.; Marigo, J.J.; Maurini, C. Regularized Formulation of the Variational Brittle Fracture with Unilateral Contact: Numerical Experiments. J. Mech. Phys. Solids 2009, 57, 1209–1229. [Google Scholar] [CrossRef]
- ASTM D3039/D3039M-17; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2017.
- ASTM D3518/D3518M; 18 Standard Test Method for In-Plane Shear Response of Polymer Matrix Composite Materials by Tensile Test of a +/−45° Laminate. ASTM International: West Conshohocken, PA, USA, 2001; pp. 1–7.






| Fiber | Matrix | Interface | |
|---|---|---|---|
| Elastic modulus, E11 [MPa] | 191,000 | 3000 | 100 |
| Poisson ratio, v [/] | 0.2 | 0.3 | 0.3 |
| Toughness Gc, [kJ/m2] | 0.763 | 1 | 0.3 |
| Phase field length, l [mm] |
| Laminate [13] | Filament [85] | |
|---|---|---|
| Fiber volume ratio | 0.536 ± 0.026 | 0.34 ± 0.002 |
| Matrix volume ratio | 0.41 ± 0.02 | 0.66 ± 0.002 |
| Fiber local ratio | 0.568 ± 0.028 1 | 0.90 2 |
| 7.00 ± 0.41 | 7.2 ± 0.30 | |
| 138.22 ± 5.11 | / |
| Length (L), mm | Width (W), mm | Thickness (t), mm | |
|---|---|---|---|
| UD-90 | 220 | 26.903 ± 0.154 | 1.33 ± 0.017 |
| SH-45 | 220 | 27.250 ± 0.017 | 2.30 ± 0.017 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gljušćić, M.; Lanc, D.; Franulović, M.; Žerovnik, A. Microstructural Analysis of the Transverse and Shear Behavior of Additively Manufactured CFRP Composite RVEs Based on the Phase-Field Fracture Theory. J. Compos. Sci. 2023, 7, 38. https://doi.org/10.3390/jcs7010038
Gljušćić M, Lanc D, Franulović M, Žerovnik A. Microstructural Analysis of the Transverse and Shear Behavior of Additively Manufactured CFRP Composite RVEs Based on the Phase-Field Fracture Theory. Journal of Composites Science. 2023; 7(1):38. https://doi.org/10.3390/jcs7010038
Chicago/Turabian StyleGljušćić, Matej, Domagoj Lanc, Marina Franulović, and Andrej Žerovnik. 2023. "Microstructural Analysis of the Transverse and Shear Behavior of Additively Manufactured CFRP Composite RVEs Based on the Phase-Field Fracture Theory" Journal of Composites Science 7, no. 1: 38. https://doi.org/10.3390/jcs7010038
APA StyleGljušćić, M., Lanc, D., Franulović, M., & Žerovnik, A. (2023). Microstructural Analysis of the Transverse and Shear Behavior of Additively Manufactured CFRP Composite RVEs Based on the Phase-Field Fracture Theory. Journal of Composites Science, 7(1), 38. https://doi.org/10.3390/jcs7010038






