Moisture Absorption of Carbon/Epoxy Nanocomposites
Abstract
1. Introduction
2. Moisture Absorption Theory
3. Materials, Sample Preparation, and Moisture Absorption Experiments
3.1. Carbon Nanotube/Epoxy and Carbon Nanofiber/Epoxy Samples
3.2. Graphite Nanoplatelets/Epoxy and Carbon Black/Epoxy Samples
3.3. Determination of Absorption Parameters from Gravimetric Data
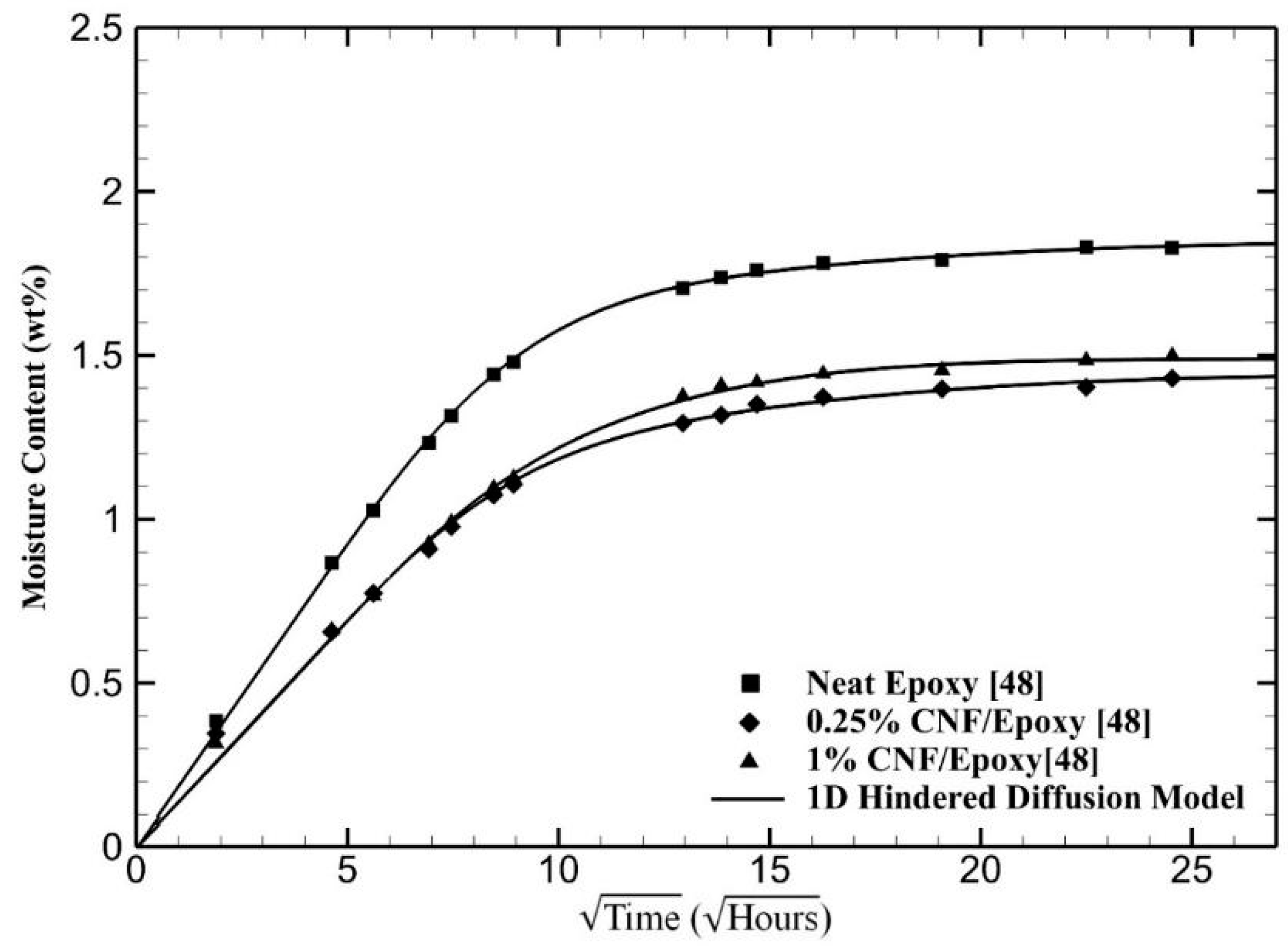
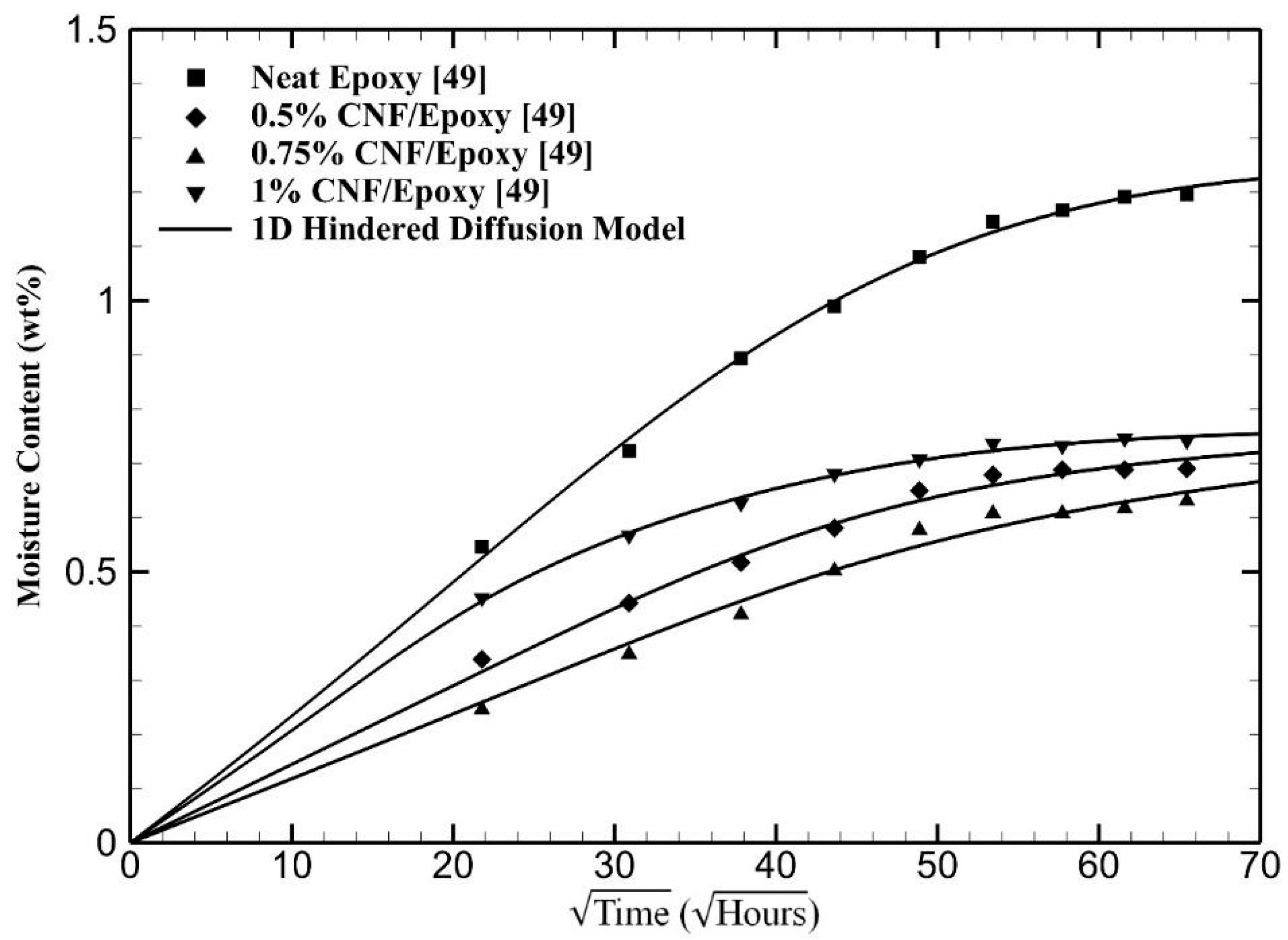
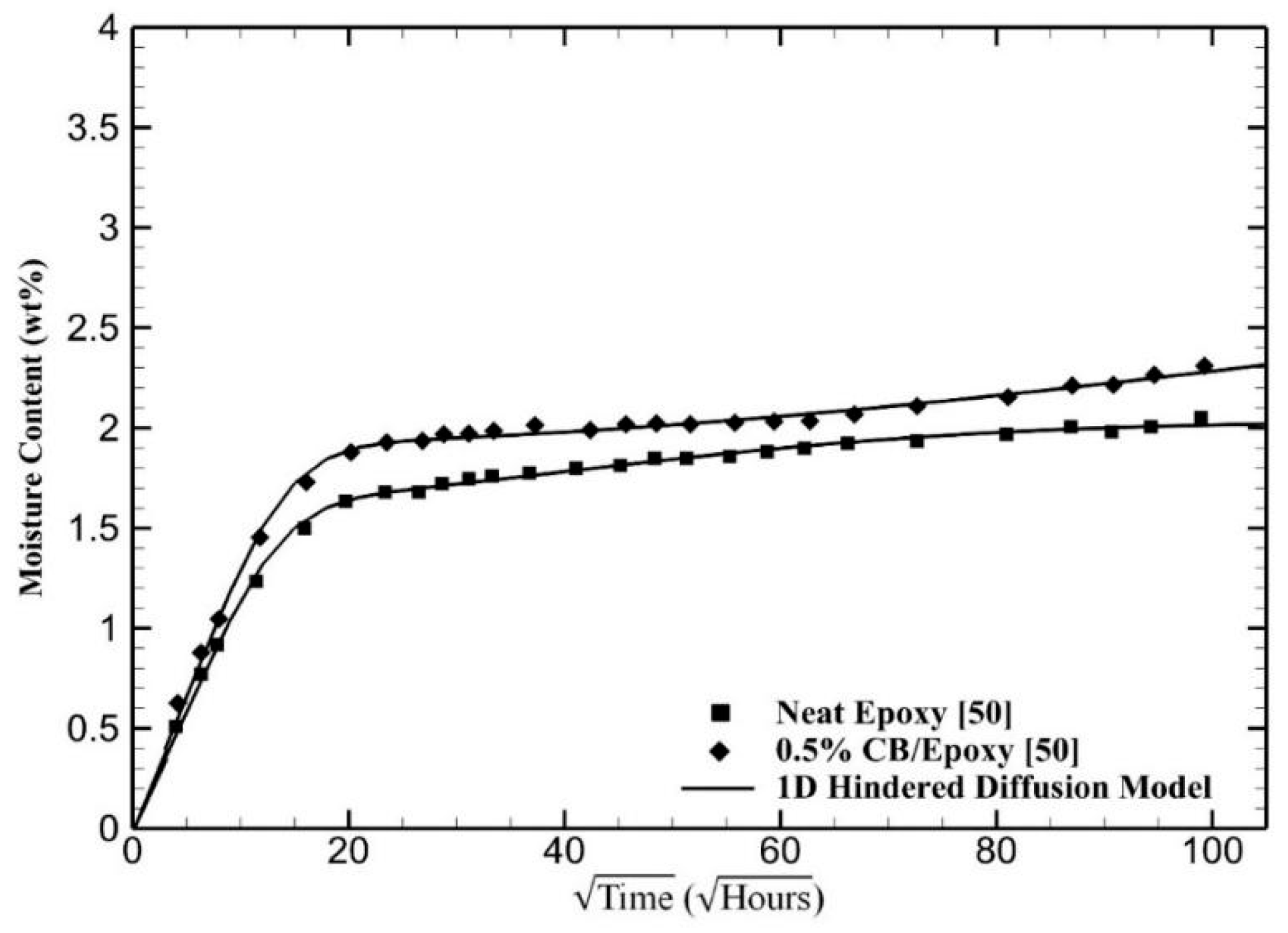
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Soares-Pozzi, A.C.; Dibbern-Brunelli, D. Study of the influence of saline solutions in carbon/epoxy composite by luminescence, Raman and UATR/FT-IR spectroscopy. J. Mater. Sci. 2016, 51, 9342–9355. [Google Scholar] [CrossRef]
- Sugita, Y.; Winkelmann, C.; La Saponara, V. Environmental and chemical degradation of carbon/epoxy lap joints for aerospace applications, and effects on their mechanical performance. Compos. Sci. Technol. 2010, 70, 829–839. [Google Scholar] [CrossRef]
- Kotrotsos, A.; Vavouliotis, A.; Tsantzalis, S.; Kostopoulos, V. Effect of CNT modified matrix of epoxy CFRPs on hydrothermal behaviour of material. Evaluation of water uptake using electrical resistance measurements. Plast. Rubber Compos. 2014, 43, 122–129. [Google Scholar] [CrossRef]
- Jefferson, G.D.; Farah, B.; Hempowicz, M.L.; Hsiao, K.T. Influence of hygrothermal aging on carbon nanofiber enhanced polyester material systems. Compos. Part B Eng. 2015, 78, 319–323. [Google Scholar] [CrossRef]
- Alzamora Guzman, V.; Brøndsted, P. Effects of moisture on glass fiber-reinforced polymer composites. J. Compos. Mater. 2015, 49, 911–920. [Google Scholar] [CrossRef]
- Barink, M.; Mavinkurve, A.; Janssen, J. Predicting non-Fickian moisture diffusion in EMCs for application in micro-electronic devices. Microelectron. Reliab. 2016, 62, 45–49. [Google Scholar] [CrossRef]
- Guermazi, N.; Haddar, N.; Elleuch, K.; Ayedi, F.H. Effect of filler addition and weathering conditions on the performance of PVC/CaCO3 composites. Polym. Compos. 2015, 37, 2171–2183. [Google Scholar] [CrossRef]
- Garg, M.; Sharma, S.; Mehta, R. Carbon nanotube-reinforced glass fiber epoxy composite laminates exposed to hygrothermal conditioning. J. Mater. Sci. 2016, 51, 8562–8578. [Google Scholar] [CrossRef]
- Starkova, O.; Buschhorn, S.T.; Mannov, E.; Schulte, K.; Aniskevich, A. Water transport in epoxy/MWCNT composites. Eur. Polym. J. 2013, 49, 2138–2148. [Google Scholar] [CrossRef]
- Korobkov, V.A.; Krylova, Y.E.; Kasatkina, T.B.; Levashov, A.S.; Gorokhov, R.V.; Bukov, N.N.; Startseva, L.T.; Krotov, A.S.; Startsev, O.V. Diffusion of moisture in an epoxy coating with a disperse mineral filler. Polym. Sci. Ser. D 2016, 9, 351–357. [Google Scholar] [CrossRef]
- Drozdov, A.D.; Christiansen, J.; Gupta, R.K.; Shah, A.P. Model for anomalous moisture diffusion through a polymer–Clay nanocomposite. J. Polym. Sci. Part B Polym. Phys. 2002, 476–492. [Google Scholar] [CrossRef]
- Kumar, A.; Roy, S. Modeling of anomalous moisture diffusion in nanographene reinforced thermoset polymers. Compos. Struct. 2015, 122, 1–7. [Google Scholar] [CrossRef]
- Al-Qadhi, M.; Merah, N.; Gasem, Z.M. Mechanical properties and water uptake of epoxy-clay nanocomposites containing different clay loadings. J. Mater. Sci. 2013, 48, 3798–3804. [Google Scholar] [CrossRef]
- Kim, D.H.; Kim, H.S. Waterproof characteristics of nanoclay/epoxy nanocomposite in adhesively bonded joints. Compos. Part B Eng. 2013, 55, 86–95. [Google Scholar] [CrossRef]
- Akil, H.M.; Santulli, C.; Sarasini, F.; Tirillò, J.; Valente, T. Environmental effects on the mechanical behaviour of pultruded jute/glass fibre-reinforced polyester hybrid composites. Compos. Sci. Technol. 2014, 94, 62–70. [Google Scholar] [CrossRef]
- Aktas, L.; Hamidi, Y.; Altan, M. Effect of moisture on the mechanical properties of resin transfer molded composites - Part I: Absorption. J. Mater. Process. Manuf. Sci. 2002, 10, 239–254. [Google Scholar] [CrossRef]
- Aktas, L.; Hamidi, Y.; Altan, M.C. Effect of moisture on the mechanical properties of resin transfer molded composites - Part II: Desorption. J. Mater. Process. Manuf. Sci. 2002, 10, 255–267. [Google Scholar] [CrossRef]
- Dhakal, H.N.; Zhang, Z.Y.; Richardson, M.O.W. Effect of water absorption on the mechanical properties of hemp fibre reinforced unsaturated polyester composites. Compos. Sci. Technol. 2007, 67, 1674–1683. [Google Scholar] [CrossRef]
- Agubra, V.A.; Mahesh, H.V. Environmental degradation of E-glass/nanocomposite under the combined effect of UV radiation, moisture, and rain. J. Polym. Sci. Part B Polym. Phys. 2014, 52, 1024–1029. [Google Scholar] [CrossRef]
- Sridhar, R.; Narasimha Murthy, H.N.; Karthik, B.; Vishnu Mahesh, K.R.; Krishna, M.; Ratna, P. Moisture diffusion through nanoclay/vinylester processed using twin-screw extrusion. J. Vinyl Addit. Technol. 2014, 20, 152–159. [Google Scholar] [CrossRef]
- Zulfli, N.M.; Bakar, A.A.; Chow, W.S. Mechanical and water absorption behaviors of carbon nanotube reinforced epoxy/glass fiber laminates. J. Reinf. Plast. Compos. 2013, 32, 1715–1721. [Google Scholar] [CrossRef]
- Liu, H.-K.; Wang, Y.-C.; Huang, T.-H. Moisture Effect on Mechanical Properties of Graphene/Epoxy Nanocomposites. J. Mech. 2016, 32, 673–682. [Google Scholar] [CrossRef]
- Khoramishad, H.; Alizadeh, O. Effects of silicon carbide nanoparticles and multi-walled carbon nanotubes on water uptake and resultant mechanical properties degradation of polymer nanocomposites immersed in hot water. Polym. Compos. 2018, 39, E883–E890. [Google Scholar] [CrossRef]
- Glaskova-Kuzmina, T.; Aniskevich, A.; Martone, A.; Giordano, M.; Zarrelli, M. Effect of moisture on elastic and viscoelastic properties of epoxy and epoxy-based carbon fibre reinforced plastic filled with multiwall carbon nanotubes. Compos. Part A Appl. Sci. Manuf. 2016, 90, 522–527. [Google Scholar] [CrossRef]
- Lee, B.L.; Yang, T.W.; Wilusz, E. Moisture effects on isobutylene-isoprene copolymer-based composite barrier. I: Moisture diffusion and detection. Polym. Eng. Sci. 1996, 36, 1217–1231. [Google Scholar] [CrossRef]
- Jana, S.; Zhong, W.-H. FTIR study of ageing epoxy resin reinforced by reactive graphitic nanofibers. J. Appl. Polym. Sci. 2007, 106, 3555–3563. [Google Scholar] [CrossRef]
- Fick, A. Ueber Difusion. Ann. Phys. 1855. [Google Scholar] [CrossRef]
- Aktas, L.; Hamidi, Y.K.; Altan, M.C. Combined edge and anisotropy effects on Fickian mass diffusion in polymer composites. J. Eng. Mater. Technol. 2004, 126, 427. [Google Scholar] [CrossRef]
- Berens, A.R.; Hopfenberg, H.B. Diffusion and relaxation in glassy polymer powders: 2. Separation of diffusion and relaxation parameters. Polymer 1978, 19, 489–496. [Google Scholar] [CrossRef]
- Jacobs, P.M.; Jones, E.R. Diffusion of moisture into two-phase polymers—Part 2 Styrenated polyester resins. J. Mater. Sci. 1989, 24, 2343–2347. [Google Scholar] [CrossRef]
- Roy, S.; Xu, W.X.; Park, S.J.; Liechti, K.M. Anomalous Moisture Diffusion in Viscoelastic Polymers: Modeling and Testing. J. Appl. Mech. 2000, 67, 391. [Google Scholar] [CrossRef]
- Carter, H.G.; Kibler, K.G. Langmuir-type model for anomalous moisture diffusion in composite resins. J. Compos. Mater. 1978, 12, 118–131. [Google Scholar] [CrossRef]
- Guloglu, G.E.; Hamidi, Y.K.; Altan, M.C. Fast recovery of non-fickian moisture absorption parameters for polymers and polymer composites. Polym. Eng. Sci. 2016, 57, 921–931. [Google Scholar] [CrossRef]
- Regazzi, A.; Léger, R.; Corn, S.; Ienny, P. Modeling of hydrothermal aging of short flax fiber reinforced composites. Compos. Part A Appl. Sci. Manuf. 2016, 90, 559–566. [Google Scholar] [CrossRef]
- Youssef, Z.; Jacquemin, F.; Gloaguen, D.; Guillén, R. A multiscale hygroviscoelastic approach to predicting the internal stresses in composite materials. Mech. Compos. Mater. 2010, 46, 201–210. [Google Scholar] [CrossRef]
- Peret, T.; Clement, A.; Freour, S.; Jacquemin, F. Numerical transient hygro-elastic analyses of reinforced Fickian and non-Fickian polymers. Compos. Struct. 2014, 116, 395–403. [Google Scholar] [CrossRef]
- Maggana, C.; Pissis, P. Water sorption and diffusion in an epoxy resin system. J. Polym. Sci. Part B Polym. Phys. 1999, 37, 1165–1182. [Google Scholar] [CrossRef]
- Grace, L.R.; Altan, M.C. Characterization of anisotropic moisture absorption in polymeric composites using hindered diffusion model. Compos. Part A Appl. Sci. Manuf. 2012, 43, 1187–1196. [Google Scholar] [CrossRef]
- Grace, L.R.; Altan, M.C. Non-Fickian three-dimensional hindered moisture absorption in polymeric composites: Model development and validation. Polym. Compos. 2013, 34, 1144–1157. [Google Scholar] [CrossRef]
- Glaskova, T.I.; Guedes, R.M.; Morais, J.J.; Aniskevich, A.N. A comparative analysis of moisture transport models as applied to an epoxy binder. Mech. Compos. Mater. 2007, 43, 377–388. [Google Scholar] [CrossRef]
- Scott, P.; Lees, J.M. Water, salt water, and alkaline solution uptake in epoxy thin films. J. Appl. Polym. Sci. 2013, 130, 1898–1908. [Google Scholar] [CrossRef]
- Joliff, Y.; Belec, L.; Chailan, J.F. Modified water diffusion kinetics in an unidirectional glass/fibre composite due to the interphase area: Experimental, analytical and numerical approach. Compos. Struct. 2013, 97, 296–303. [Google Scholar] [CrossRef]
- Popineau, S.; Rondeau-Mouro, C.; Sulpice-Gaillet, C.; Shanahan, M.E.R. Free/bound water absorption in an epoxy adhesive. Polymer 2005, 46, 10733–10740. [Google Scholar] [CrossRef]
- LaPlante, G.; Ouriadov, A.V.; Lee-Sullivan, P.; Balcom, B.J. Anomalous moisture diffusion in an epoxy adhesive detected by magnetic resonance imaging. J. Appl. Polym. Sci. 2008, 109, 1350–1359. [Google Scholar] [CrossRef]
- Grace, L.R.; Altan, M.C. Three-dimensional anisotropic moisture absorption in quartz-reinforced bismaleimide laminates. Polym. Eng. Sci. 2013, 54, 137–146. [Google Scholar] [CrossRef]
- Grace, L.R. Projecting long-term non-Fickian diffusion behavior in polymeric composites based on short-term data: A 5-year validation study. J. Mater. Sci. 2016, 51, 845–853. [Google Scholar] [CrossRef]
- Helbling, C.S.; Karbhari, V.M. Investigation of the sorption and tensile response of pultruded E-glass/vinylester composites subjected to hygrothermal exposure and sustained strain. J. Reinf. Plast. Compos. 2008, 27, 613–638. [Google Scholar] [CrossRef]
- Prolongo, S.G.; Gude, M.R.; Ureña, A. Water uptake of epoxy composites reinforced with carbon nanofillers. Compos. Part A Appl. Sci. Manuf. 2012, 43, 2169–2175. [Google Scholar] [CrossRef]
- Saha, S.; Bal, S. Long term hydrothermal effect on the mechanical and thermo-mechanical properties of carbon nanofiber doped epoxy composites. J. Polym. Eng. 2018, 38, 251. [Google Scholar] [CrossRef]
- Starkova, O.; Chandrasekaran, S.; Schnoor, T.; Sevcenko, J.; Schulte, K. Anomalous water diffusion in epoxy/carbon nanoparticle composites. Polym. Degrad. Stab. 2019, 164, 127–135. [Google Scholar] [CrossRef]
- Ramanathan, T.; Fisher, F.T.; Ruoff, R.S.; Brinson, L.C. Amino-Functionalized Carbon Nanotubes for Binding to Polymers and Biological Systems. Chem. Mater. 2005, 17, 1290–1295. [Google Scholar] [CrossRef]



| Materials | (10−3 h−1) | (10−4 h−1) | μ | |||
|---|---|---|---|---|---|---|
| Epoxy Resin | 0 | 5.32 | 4.51 | 4.51 | 0.100 | 1.85 |
| CNT/Epoxy | 0.1 | 4.19 | 4.51 | 6.47 | 0.143 | 1.42 |
| CNT/Epoxy | 0.25 | 3.70 | 4.73 | 5.82 | 0.123 | 1.69 |
| CNF/Epoxy | 0.25 | 5.52 | 5.21 | 8.97 | 0.172 | 1.44 |
| CNF/Epoxy | 1 | 5.69 | 11.8 | 29.5 | 0.250 | 1.49 |
| Materials | (10−3 h−1) | (10−4 h−1) | μ | |||
|---|---|---|---|---|---|---|
| Epoxy Resin | 0 | 2.15 | 52.5 | 0.762 | 0.145 | 1.25 |
| CNF/Epoxy | 0.5 | 2.10 | 0.577 | 0.161 | 0.279 | 0.895 |
| CNF/Epoxy | 0.75 | 1.79 | 1.45 | 0.497 | 0.342 | 0.829 |
| CNF/Epoxy | 1 | 7.83 | 9.76 | 4.81 | 0.493 | 0.761 |
| Materials | (10−3 h−1) | (10−3 h−1) | μ | |||
|---|---|---|---|---|---|---|
| Epoxy Resin | 0 | 6.37 | 3.77 | 0.965 | 0.256 | 2.78 |
| CNT/Epoxy | 0.5 | 7.55 | 8.70 | 9.99 | 1.15 | 3.43 |
| Materials | (10−5 h−1) | (10−5 h−1) | μ | |||
|---|---|---|---|---|---|---|
| Epoxy Resin | 0 | 4.77 | 31.6 | 7.60 | 0.240 | 2.03 |
| GnP/Epoxy | 0.5 | 1.47 | 2.41 | 0.89 | 0.369 | 3.17 |
| CB/Epoxy | 0.5 | 4.47 | 2.72 | 2.20 | 0.807 | 3.47 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guloglu, G.E.; Altan, M.C. Moisture Absorption of Carbon/Epoxy Nanocomposites. J. Compos. Sci. 2020, 4, 21. https://doi.org/10.3390/jcs4010021
Guloglu GE, Altan MC. Moisture Absorption of Carbon/Epoxy Nanocomposites. Journal of Composites Science. 2020; 4(1):21. https://doi.org/10.3390/jcs4010021
Chicago/Turabian StyleGuloglu, Gorkem E., and M. Cengiz Altan. 2020. "Moisture Absorption of Carbon/Epoxy Nanocomposites" Journal of Composites Science 4, no. 1: 21. https://doi.org/10.3390/jcs4010021
APA StyleGuloglu, G. E., & Altan, M. C. (2020). Moisture Absorption of Carbon/Epoxy Nanocomposites. Journal of Composites Science, 4(1), 21. https://doi.org/10.3390/jcs4010021




