Tube Joining by a Sheet Flange Connection
Abstract
1. Introduction
2. Materials and Methods
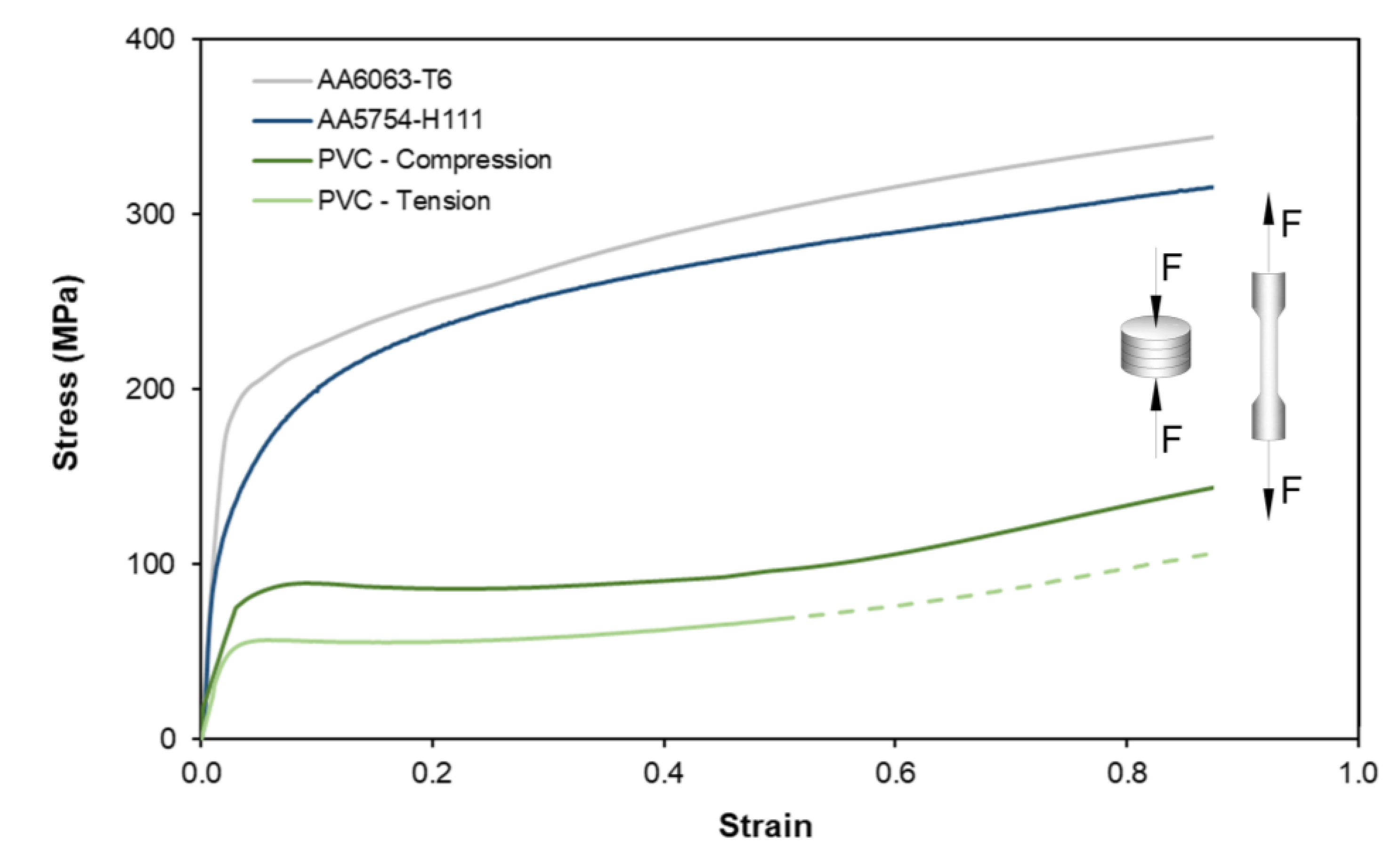
2.1. Material Properties
2.2. Numerical and Experimental Test Plan
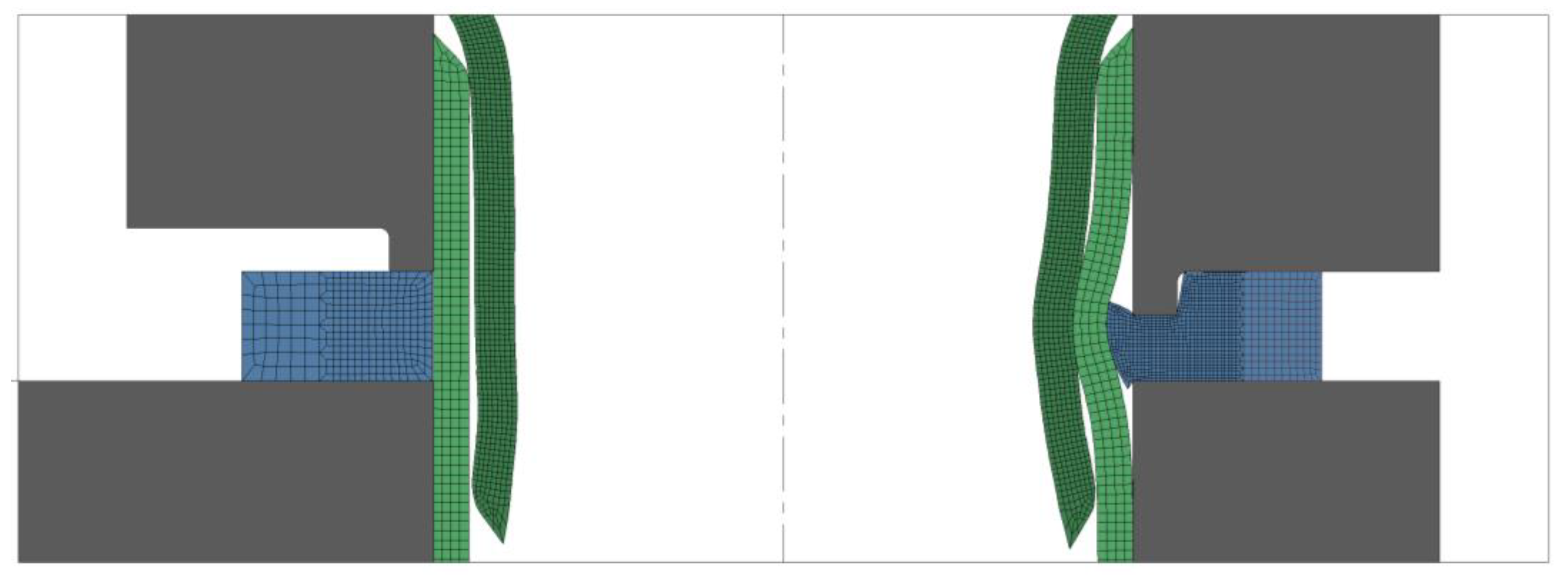
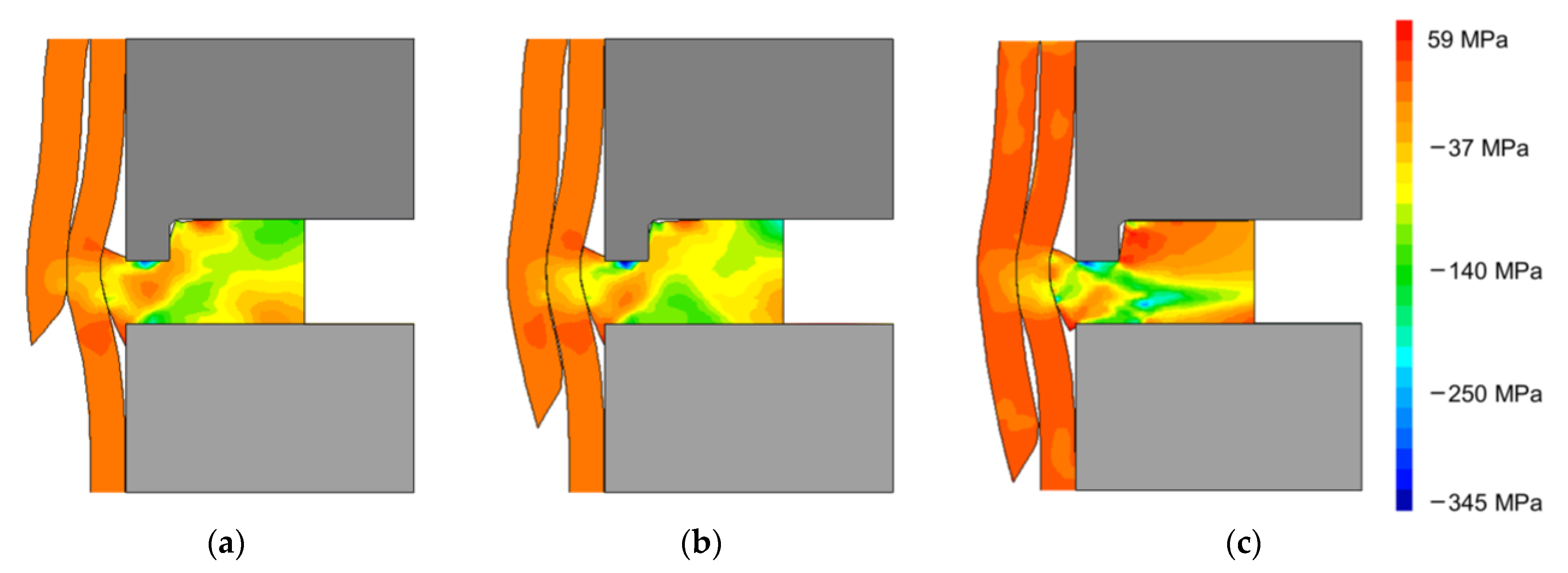
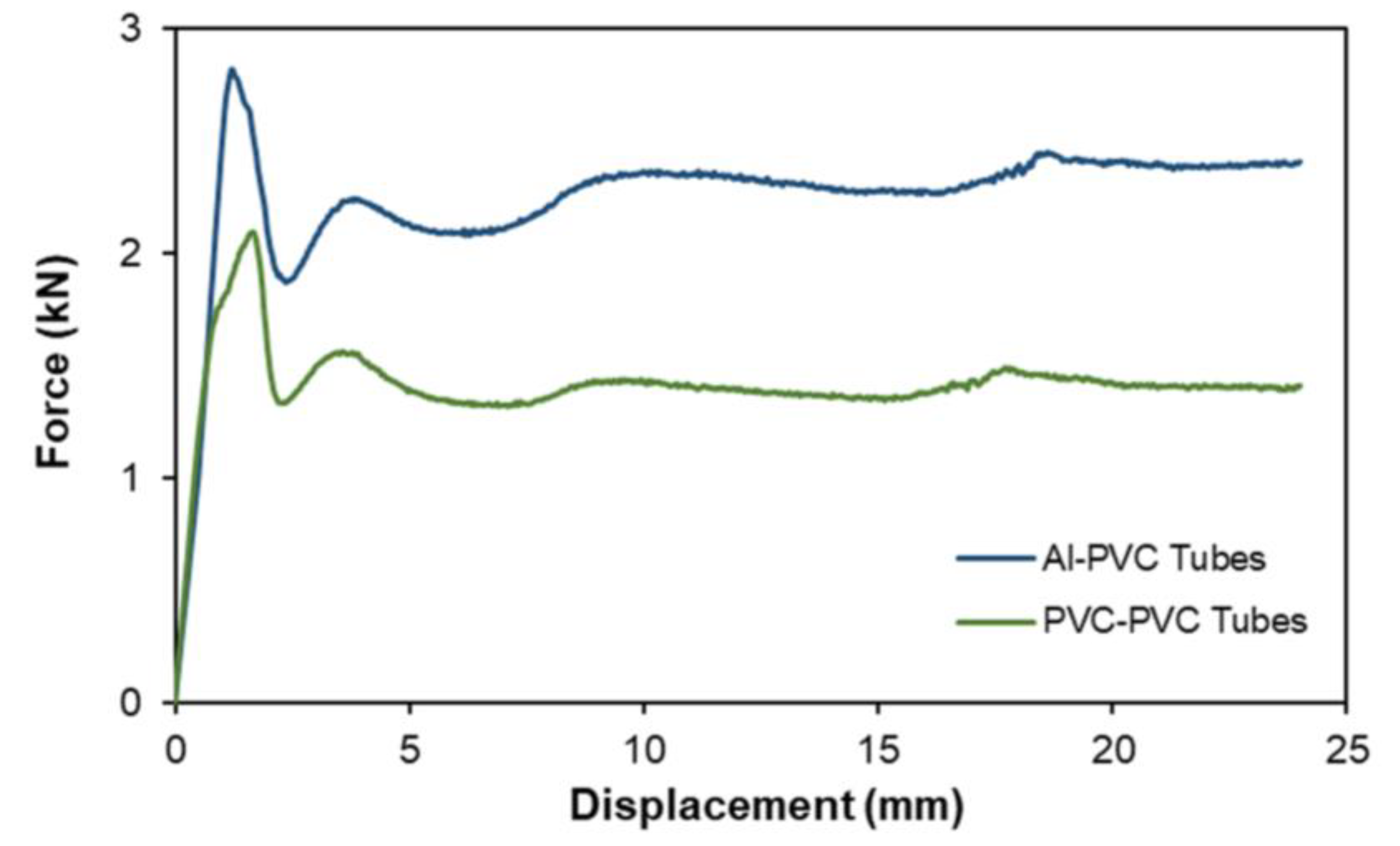
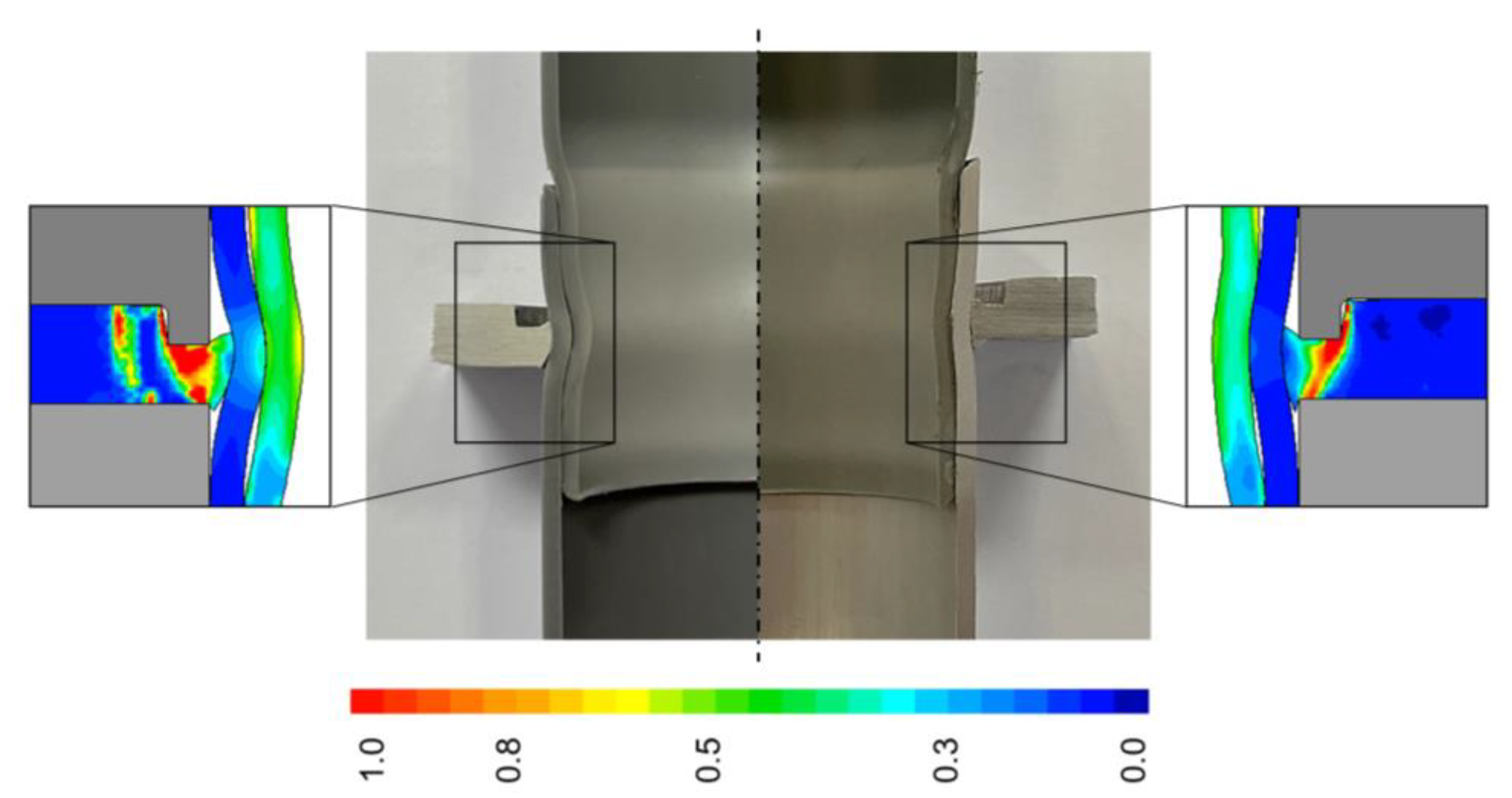
3. Results
Analysis of the Optimal Surface Contact between the Tubes
4. Discussion
Mechanisms behind the Joint and Their Influence on the Overall Performance
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kleiner, M.; Geiger, M.; Klaus, A. Manufacturing of lightweight components by metal forming. CIRP Ann. Manuf. Technol. 2003, 52, 521–542. [Google Scholar] [CrossRef]
- Mori, K.-I.; Bay, N.; Fratini, L.; Micari, F.; Tekkaya, A.E. Joining by plastic deformation. CIRP Ann. Manuf. Technol. 2013, 62, 673–694. [Google Scholar] [CrossRef]
- Groche, P.; Wohletz, S.; Brenneis, M.; Pabst, C.; Resch, F. Joining by forming—A review on joint mechanisms, applications and future trends. J. Mater. Process. Technol. 2014, 214, 1972–1994. [Google Scholar] [CrossRef]
- Hahn, M.; Gies, S.; Tekkaya, A.E. Light enough or go lighter? Mater. Des. 2019, 163, 107545. [Google Scholar] [CrossRef]
- Meschut, G.; Merklein, M.; Brosius, A.; Drummer, D.; Fratini, L.; Füssel, U.; Gude, M.; Homberg, W.; Martins, P.A.F.; Bobbert, M.; et al. Review on mechanical joining by plastic deformation. J. Adv. Join. Process. 2022, 5, 100113. [Google Scholar] [CrossRef]
- Buffa, G.; Fratini, L.; La Commare, U.; Römisch, D.; Wiesenmayer, S.; Wituschek, S.; Merklein, M. Joining by forming technologies: Current solutions and future trends. Int. J. Mater. Form. 2022, 15, 27. [Google Scholar] [CrossRef]
- Meschut, G.; Merklein, M.; Brosius, A.; Bobbert, M. Mechanical joining in versatile process chains. Prod. Eng. 2022, 16, 187–191. [Google Scholar] [CrossRef]
- Gonçalves, A.; Alves, L.M.; Martins, P.A.F. Tube joining by asymmetric plastic instability. J. Mater. Process. Technol. 2014, 214, 132–140. [Google Scholar] [CrossRef]
- Silva, C.M.A.; Alves, L.M.; Nielsen, C.V.; Martins, P.A.F. Environmentally friendly joining of tubes by their ends. J. Clean. Prod. 2015, 87, 777–786. [Google Scholar] [CrossRef]
- Zhang, Q.; Jin, K.; Mu, D. Tube/tube joining technology by using rotary swaging forming method. J. Mater. Process. Technol. 2014, 214, 2085–2094. [Google Scholar] [CrossRef]
- Alves, L.M.; Afonso, R.M.; Silva, C.M.A.; Martins, P.A.F. Joining sandwich composite panels to tubes. Proc. Inst. Mech. Eng. 2019, 233, 1472–1481. [Google Scholar] [CrossRef]
- Alves, L.M.; Reis, T.J.; Afonso, R.M.; Martins, P.A.F. Single-stroke attachment of sheets to tube ends made from dissimilar materials. Materials 2021, 14, 815. [Google Scholar] [CrossRef] [PubMed]
- Alves, L.M.; Afonso, R.M.; Martins, P.A.F. Joining by forming of polymer-metal sheet-tube connections. Proc. Inst. Mech. Eng. 2020, 234, 938–946. [Google Scholar] [CrossRef]
- Alves, L.M.; Afonso, R.M.; Silva, F.L.R.; Martins, P.A.F. Deformation-assisted joining of sheets to tubes by annular sheet squeezing. Materials 2019, 12, 3909. [Google Scholar] [CrossRef]
- Alves, L.M.; Afonso, R.M.; Silva, F.L.R.; Martins, P.A.F. Mechanical joining of sheets to tubes by squeeze-grooving. Proc. Inst. Mech. Eng. 2020, 234, 120–129. [Google Scholar] [CrossRef]
- Wang, P.-Y.; Jin, J.-G.; Wan, G.-H.; Zhang, C.-S.; Wang, Y.-C.; Xiang, N.; Zhao, X.-N.; Wang, Z.-J. An improved method for manufacturing sheet-tube connection structure by double-sided annular sheet squeezing. J. Adv. Manuf. Technol. 2022, 120, 829–842. [Google Scholar] [CrossRef]
- Nielsen, C.V.; Zhang, W.; Alves, L.M.; Bay, N.; Martins, P.A.F. Coupled Finite Element Flow Formulation. In Modeling of Thermo-Electro-Mechanical Manufacturing Processes; Springer: London, UK, 2013; pp. 11–36. [Google Scholar]
- Raghava, R.S.; Caddell, R.M. A macroscopic yield criterion for crystalline polymers. Int. J. Mech. Sci. 1973, 15, 967–974. [Google Scholar] [CrossRef]
- Caddell, R.M.; Raghava, R.S.; Atkins, A.G. Pressure dependent yield criteria for polymers. Mater. Sci. Eng. 1974, 13, 113–120. [Google Scholar] [CrossRef]
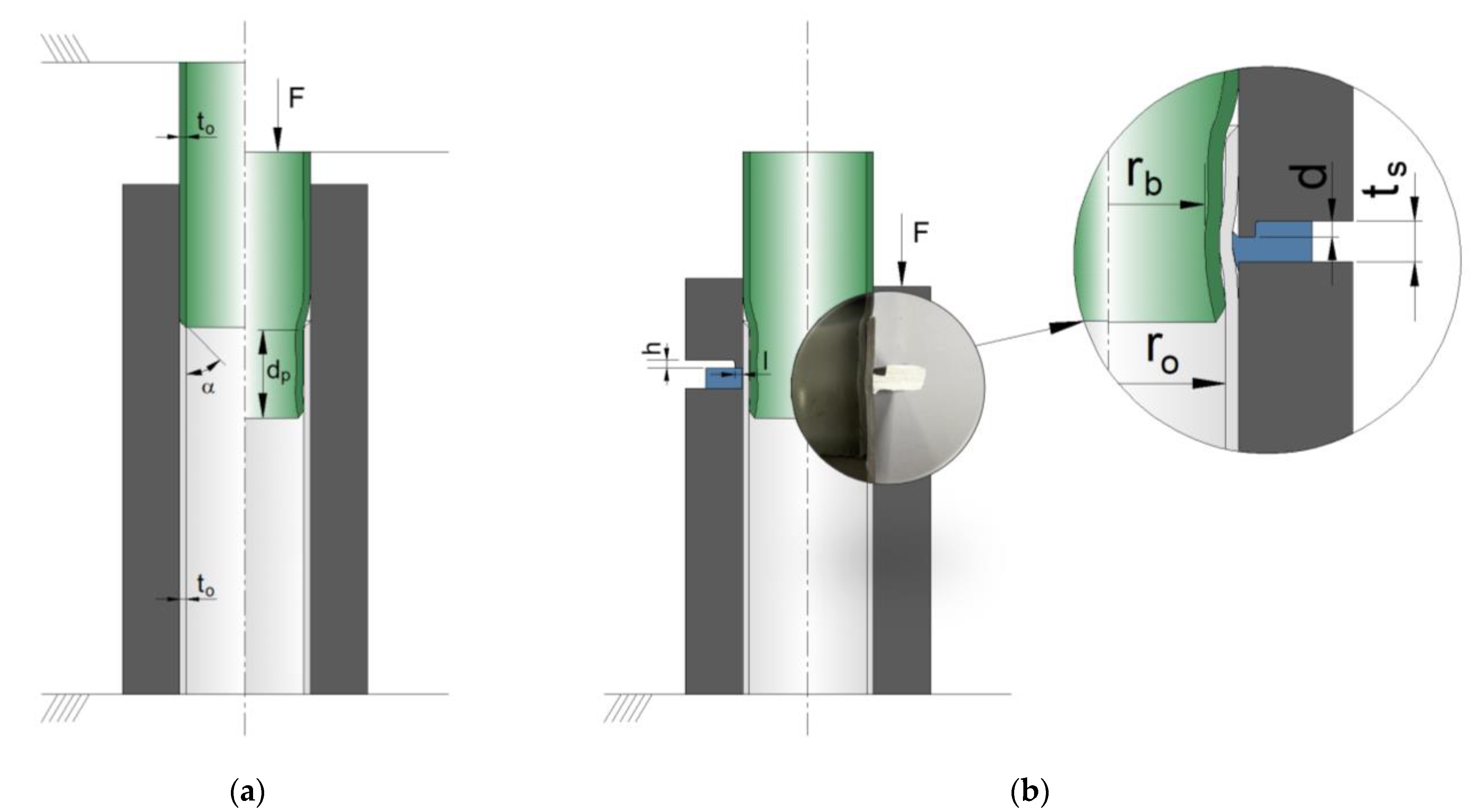






| Tubes | |||
| (mm) | (mm) | (°) | (mm) |
| 16 | 1.5 | 45 | 18, 22, 24 |
| Sheets | |||
| (mm) | (mm) | (mm) | h (mm) |
| 5 | 2 | 2 | 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Afonso, R.M.; Alves, L.M. Tube Joining by a Sheet Flange Connection. J. Manuf. Mater. Process. 2023, 7, 12. https://doi.org/10.3390/jmmp7010012
Afonso RM, Alves LM. Tube Joining by a Sheet Flange Connection. Journal of Manufacturing and Materials Processing. 2023; 7(1):12. https://doi.org/10.3390/jmmp7010012
Chicago/Turabian StyleAfonso, Rafael M., and Luís M. Alves. 2023. "Tube Joining by a Sheet Flange Connection" Journal of Manufacturing and Materials Processing 7, no. 1: 12. https://doi.org/10.3390/jmmp7010012
APA StyleAfonso, R. M., & Alves, L. M. (2023). Tube Joining by a Sheet Flange Connection. Journal of Manufacturing and Materials Processing, 7(1), 12. https://doi.org/10.3390/jmmp7010012









