Fundamental Investigation of Diamond Cutting of Micro V-Shaped Grooves on a Polycrystalline Soft-Brittle Material
Abstract
1. Introduction
- (1)
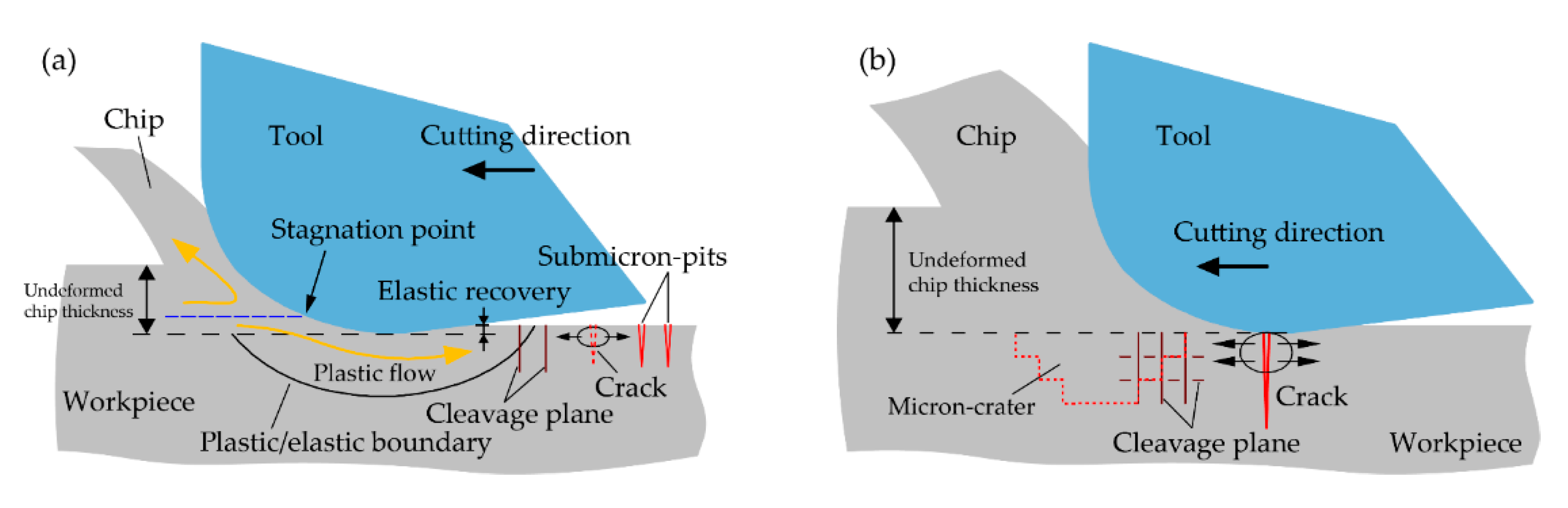
- The conventional ductile-regime machining model is not applicable to cutting of V-shaped grooves. It is well known that in cutting of flat surfaces, crack-free machined surface can be generated even if the cracks occur during cutting process, as long as median cracks do not propagate to below the machined surface plane [16], as illustrated in Figure 1a. However, when cutting V-shaped grooves, if cracks are produced, the cracks will remain in the machined surface without being removed, as illustrated in Figure 1b. Thus, to achieve crack-free machined surface, the initiation of cracks must be completely suppressed.
- (2)
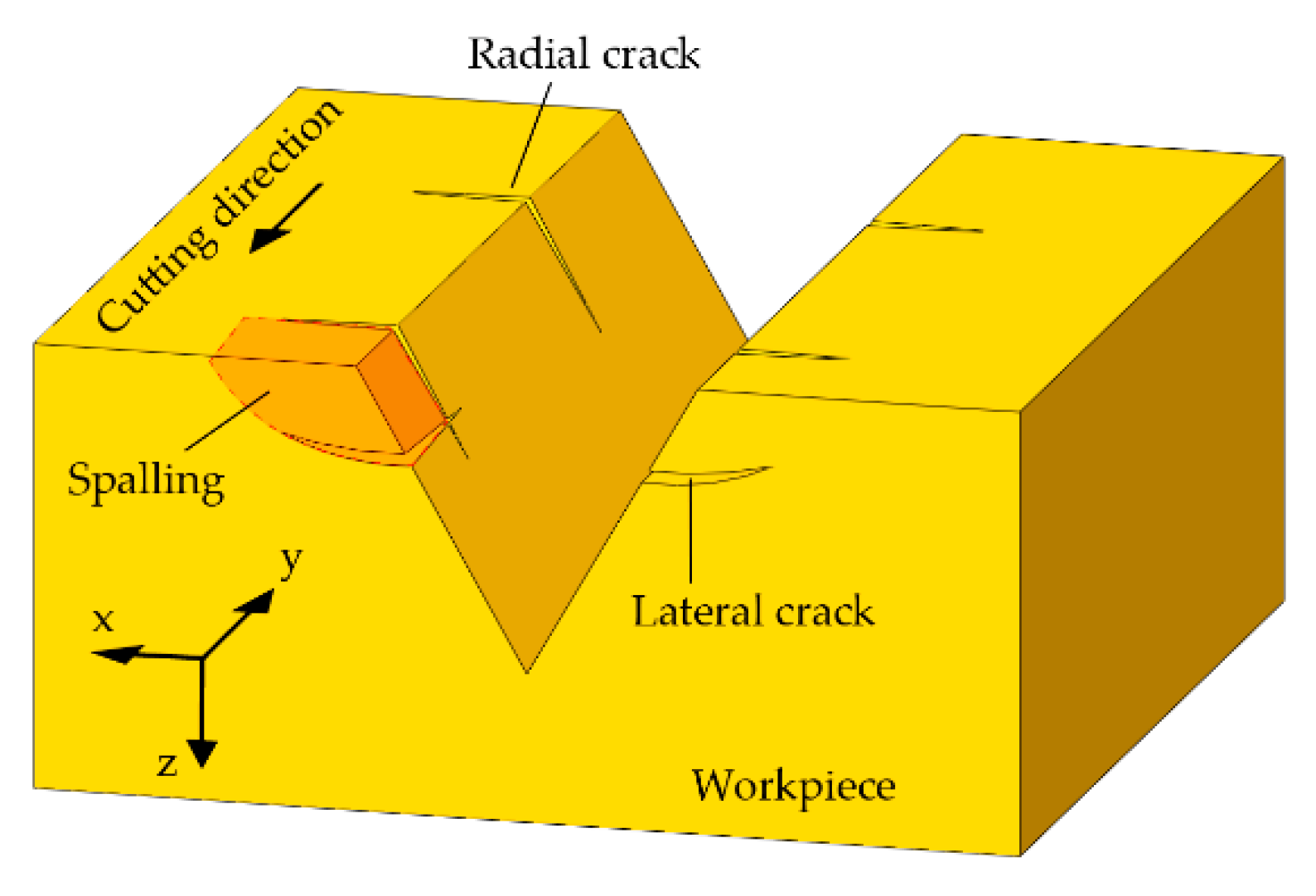
- High stress concentration occurs at the apex of groove edge. Existing research on machining V-shaped grooves has reported that high-stress region is located at the apex of groove edge [17,18]. Owing to the low fracture toughness of brittle materials, lateral cracks are likely to initiate at this region, causing material spalling, as illustrated in Figure 1b.
- (3)
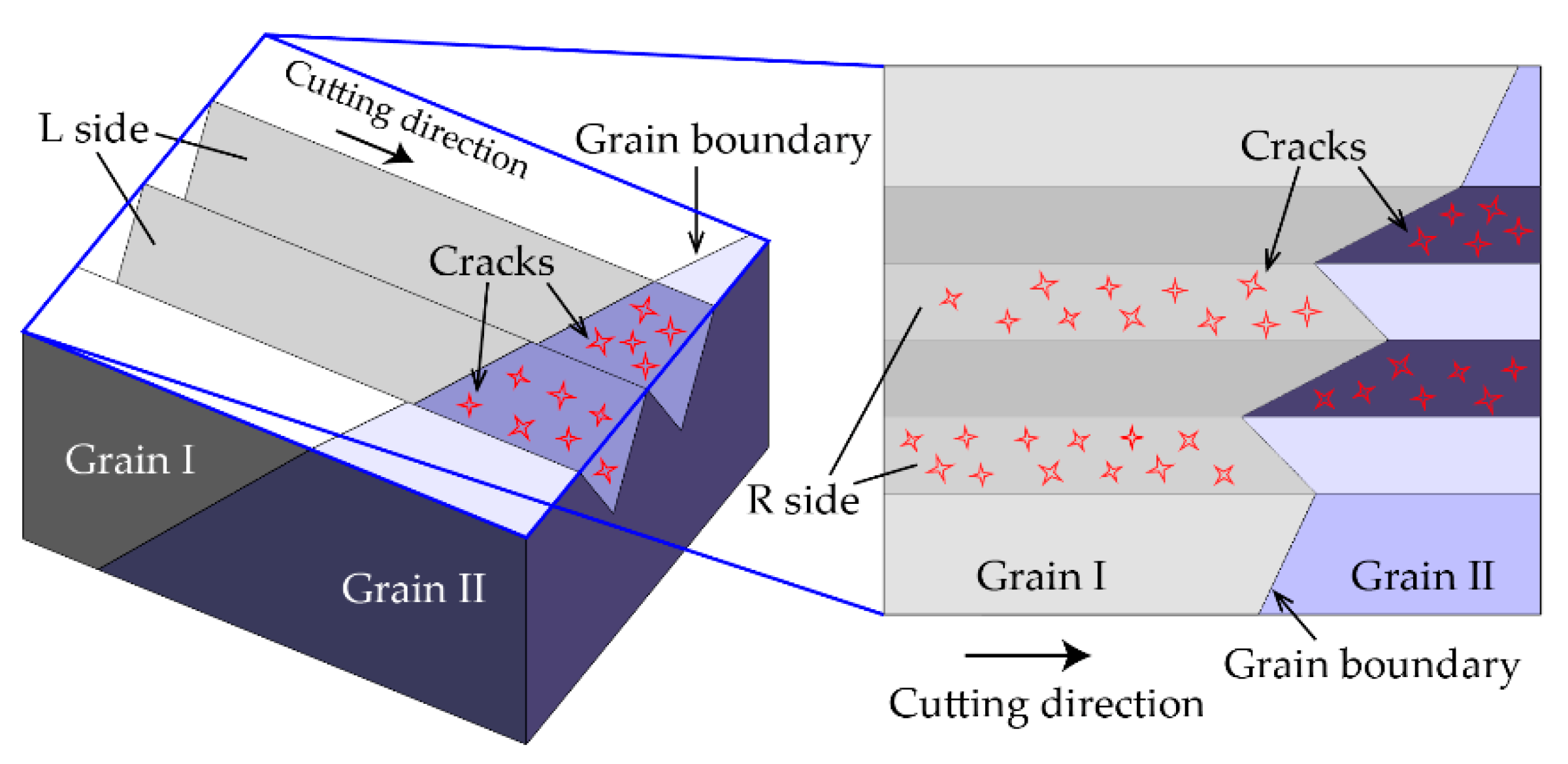
- The two side surfaces of a V-shaped groove are in different relative positions with respect to the cleavage planes and slip planes of crystal grains, as illustrated in Figure 1b. Therefore, the material removal mechanism of the two sides of a V-shaped groove is likely different.
2. Materials and Methods
3. Results and Discussion
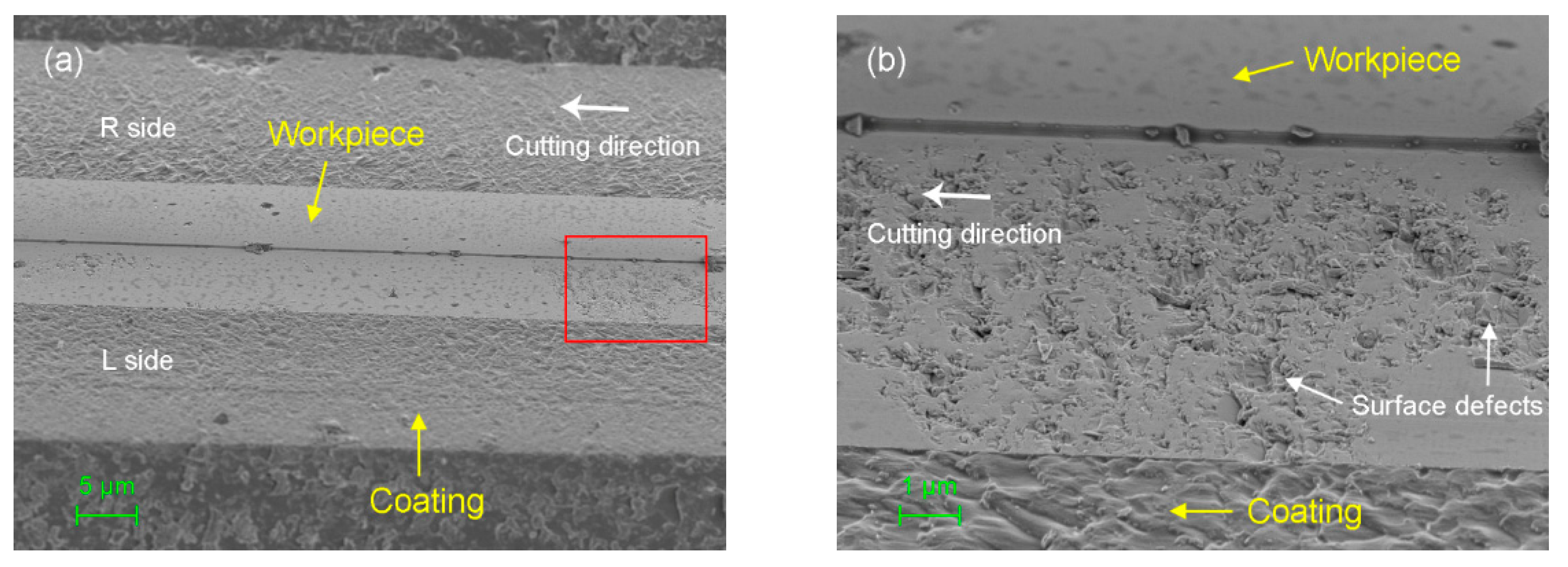
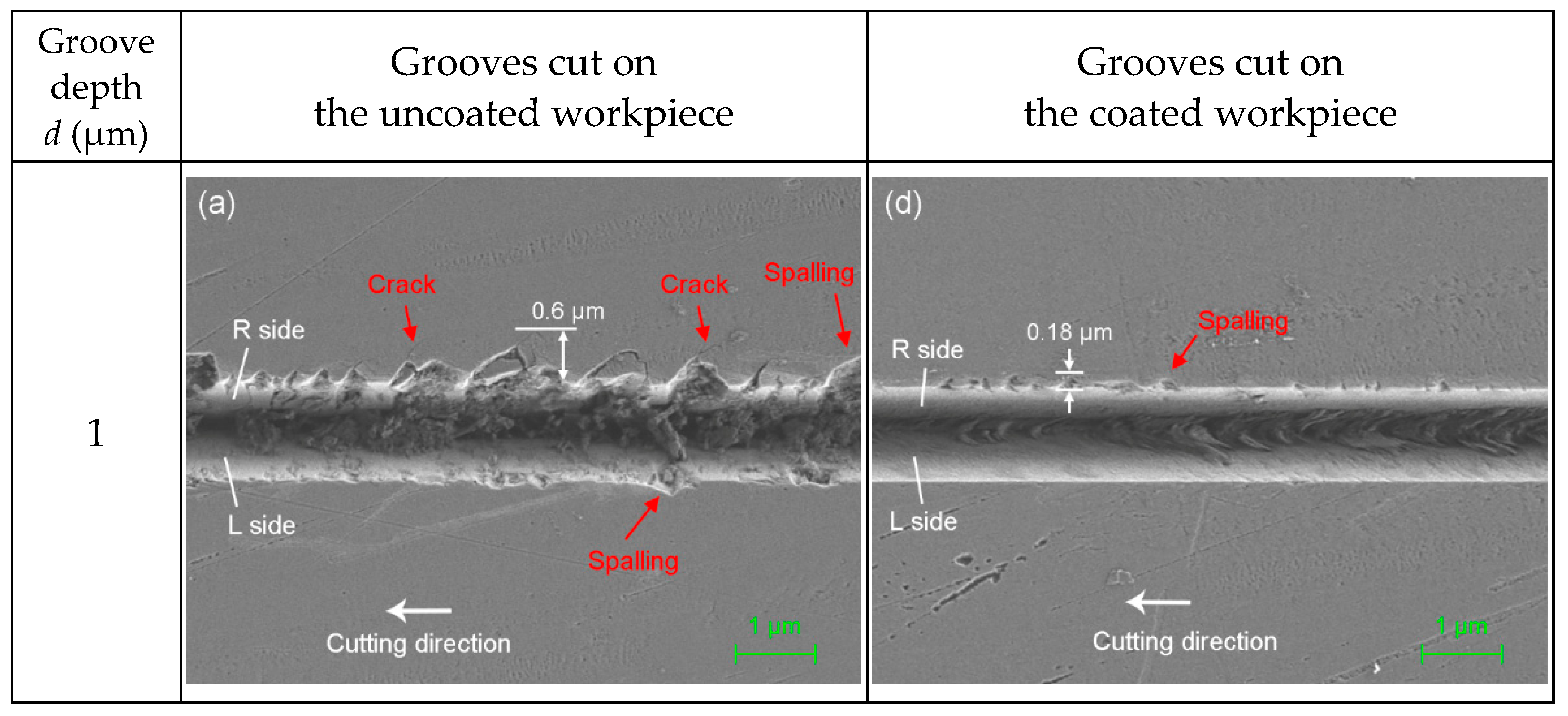
3.1. Effect of Resin Coating
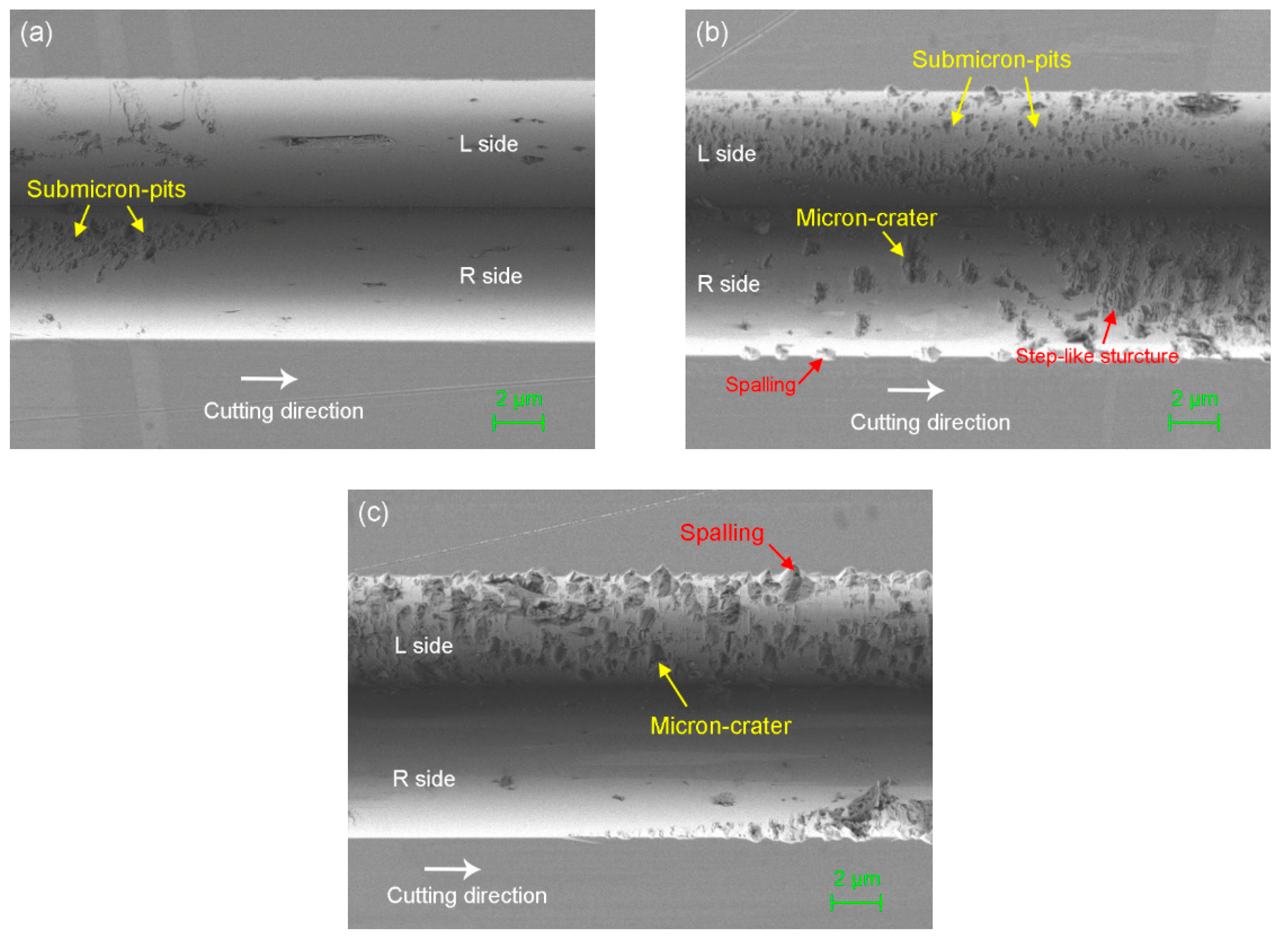
3.2. Effect of Tool Feed Rate
3.3. Formation Mechanism of Surface Defects
3.4. FEM Simulation of Stress Distribution
4. Conclusions
- (1)
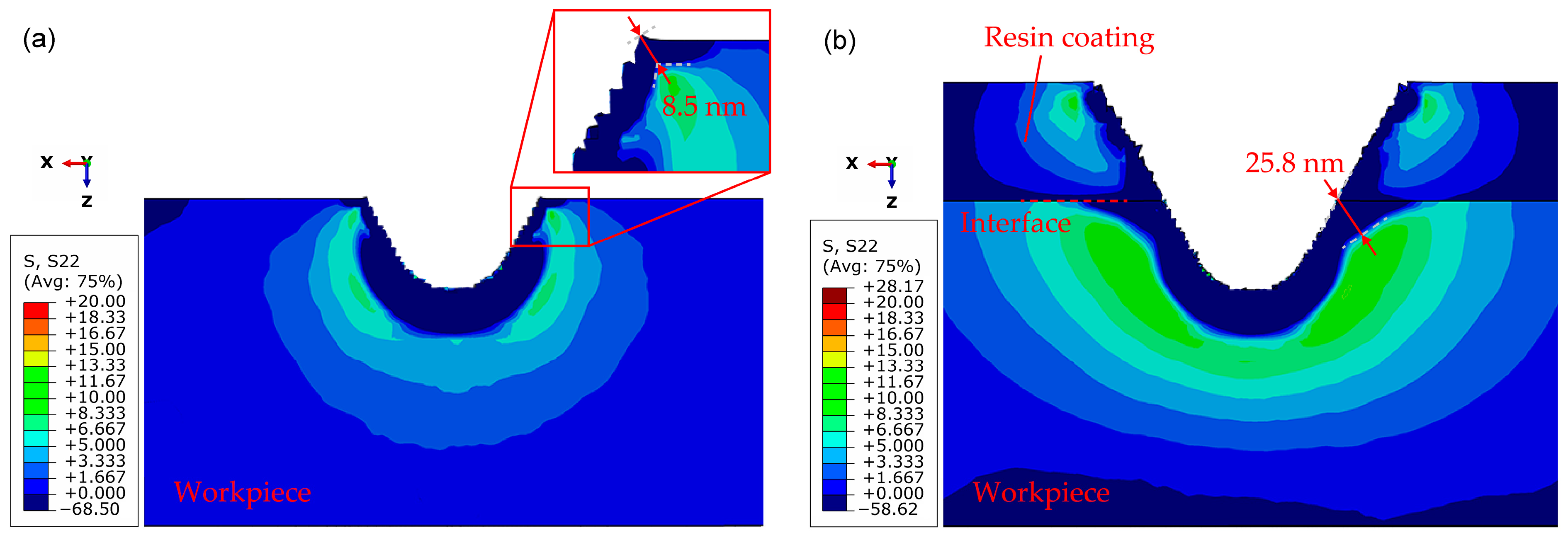
- Application of a solidified coating layer of cured photosensitive resin on the workpiece surface before machining significantly suppressed brittle fractures at the edge of the groove, which solves the edge chipping problem in brittle materials grooving, although the side surfaces of the groove are unaffected.
- (2)
- When the tool feed rate is small (<20 nm/rev), submicron-pits were observed on the groove surface. Further increasing the feed rate, not only submicron-pits but also micron-craters were observed on the groove surface.
- (3)
- The two side surfaces of the V-shaped groove show distinctly different morphologies. The formation of defects is strongly dependent on the angle of groove surface with respect to cleavage plane of grain.
- (4)
- FEM simulation results demonstrated that the resin coating was able to make the tensile stresses along the cutting direction and the direction normal to the workpiece surface distributed away from the edge of the groove and the workpiece surface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Liu, Q.; Liao, Z.; Axinte, D. Temperature effect on the material removal mechanism of soft-brittle crystals at nano/micron scale. Int. J. Mach. Tools Manuf. 2020, 159, 103620. [Google Scholar] [CrossRef]
- Yan, J.; Tamaki, J.I.; Syoji, K.; Kuriyagawa, T. Single-point diamond turning of CaF2 for nanometric surface. Int. J. Adv. Manuf. Technol. 2004, 24, 640–646. [Google Scholar] [CrossRef]
- Fang, F.Z.; Venkatesh, V.C.; Zhang, G.X. Diamond turning of soft semiconductors to obtain nanometric mirror surfaces. Int. J. Adv. Manuf. Technol. 2002, 19, 637–641. [Google Scholar] [CrossRef]
- Zhang, Z.; Yan, J.; Kuriyagawa, T. Manufacturing technologies toward extreme precision. Int. J. Extrem. Manuf. 2019, 1, 22001. [Google Scholar] [CrossRef]
- Mukaida, M.; Yan, J. Ductile machining of single-crystal silicon for microlens arrays by ultraprecision diamond turning using a slow tool servo. Int. J. Mach. Tools Manuf. 2017, 115, 2–14. [Google Scholar] [CrossRef]
- Zhang, L.; Zhou, L.; Zhou, W.; Zhang, S.; Yi, A.Y. Design, fabrication and testing of a compact large-field-of-view infrared compound eye imaging system by precision glass molding. Precis. Eng. 2020, 66, 87–98. [Google Scholar] [CrossRef]
- Zhang, S.; Zhou, Y.; Zhang, H.; Xiong, Z.; To, S. Advances in ultra-precision machining of micro-structured functional surfaces and their typical applications. Int. J. Mach. Tools Manuf. 2019, 142, 16–41. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Karpuschewski, B.; Yan, J.; Schönemann, L. Manufacturing of multiscale structured surfaces. CIRP Ann. 2020, 69, 717–739. [Google Scholar] [CrossRef]
- Tong, Z.; Zhong, W.; To, S.; Zeng, W. Fast-tool-servo micro-grooving freeform surfaces with embedded metrology. CIRP Ann. 2020, 69, 505–508. [Google Scholar] [CrossRef]
- Guo, J.; Zhang, J.; Pan, Y.; Kang, R.; Namba, Y.; Shore, P.; Yue, X.; Wang, B.; Guo, D. A critical review on the chemical wear and wear suppression of diamond tools in diamond cutting of ferrous metals. Int. J. Extrem. Manuf. 2020, 2, 12001. [Google Scholar]
- Mukaida, M.; Yan, J. Fabrication of hexagonal microlens arrays on single-crystal silicon using the tool-servo driven segment turning method. Micromachines 2017, 8, 323. [Google Scholar] [CrossRef]
- Zhu, Z.; Tong, Z.; To, S.; Jiang, X. Tuned diamond turning of micro-structured surfaces on brittle materials for the improvement of machining efficiency. CIRP Ann. 2019, 68, 559–562. [Google Scholar] [CrossRef]
- Jasinevicius, R.G.; Duduch, J.G.; Cirino, G.A.; Pizani, P.S. Diamond turning of small Fresnel lens array in single crystal InSb. J. Micromech. Microeng. 2013, 23, 55025. [Google Scholar] [CrossRef]
- Yan, J.; Maekawa, K.; Tamaki, J.; Kuriyagawa, T. Micro grooving on single-crystal germanium for infrared Fresnel lenses. J. Micromech. Microeng. 2005, 15, 1925–1931. [Google Scholar] [CrossRef]
- Huang, W.; Yan, J. Surface formation mechanism in ultraprecision diamond turning of coarse-grained polycrystalline ZnSe. Int. J. Mach. Tools Manuf. 2020, 153, 103554. [Google Scholar] [CrossRef]
- Blake, P.N.; Scattergood, R.O. Ductile-regime machining of germanium and silicon. J. Am. Ceram. Soc. 1990, 73, 949–957. [Google Scholar] [CrossRef]
- Yan, J.; Oowada, T.; Zhou, T.; Kuriyagawa, T. Precision machining of microstructures on electroless-plated NiP surface for molding glass components. J. Mater. Process. Technol. 2009, 209, 4802–4808. [Google Scholar] [CrossRef]
- Guo, J.; Zhang, J.; Wang, H.; Liu, K.; Kumar, A.S. Surface quality characterisation of diamond cut V-groove structures made of rapidly solidified aluminium RSA-905. Precis. Eng. 2018, 53, 120–133. [Google Scholar] [CrossRef]
- Heidari, M.; Yan, J. Ultraprecision surface flattening of porous silicon by diamond turning. Precis. Eng. 2017, 49, 262–277. [Google Scholar] [CrossRef]
- Lee, Y.J.; Chong, J.Y.; Chaudhari, A.; Wang, H. Enhancing ductile-mode cutting of calcium fluoride single crystals with solidified coating. Int. J. Precis. Eng. Manuf. Green Technol. 2019, 7, 1019–1029. [Google Scholar] [CrossRef]
- Kalkhoran, S.N.A.; Vahdati, M.; Zhang, Z.; Yan, J. Influence of Wax Lubrication on Cutting Performance of Single-Crystal Silicon in Ultraprecision Microgrooving. Int. J. Precis. Eng. Manuf. Green Technol. 2020. [Google Scholar] [CrossRef]
- Huang, W.; Yan, J. Chip-free surface patterning of toxic brittle polycrystalline materials through micro/nanoscale burnishing. Int. J. Mach. Tools Manuf. 2021, 162, 103688. [Google Scholar] [CrossRef]
- Carr, J.W.; Feger, C. Ultraprecision machining of polymers. Precis. Eng. 1993, 15, 221–237. [Google Scholar] [CrossRef]
- Yan, J. Ultraprecision cutting of photoresist/gold composite microstructures. CIRP Ann. 2011, 60, 133–136. [Google Scholar] [CrossRef]
- Yan, J.; Syoji, K.; Kuriyagawa, T.; Suzuki, H. Ductile regime turning at large tool feed. J. Mater. Process. Technol. 2002, 121, 363–372. [Google Scholar] [CrossRef]
- Sun, H.; Adhyaksa, G.W.P.; Garnett, E.C. The Application of Electron Backscatter Diffraction on Halide Perovskite Materials. Adv. Energy Mater. 2020, 10, 2000364. [Google Scholar] [CrossRef]
- Jung, J.; Yoon, J.I.; Kim, J.G.; Latypov, M.I.; Kim, J.Y.; Kim, H.S. Continuum understanding of twin formation near grain boundaries of FCC metals with low stacking fault energy. Npj Comput. Mater. 2017, 3, 21. [Google Scholar] [CrossRef]
- Sun, Y.; Sun, Z.; Gao, S.; Cheng, H.; Liu, Q.; Piao, J.; Yao, T.; Wu, C.; Hu, S.; Wei, S.; et al. Fabrication of flexible and freestanding zinc chalcogenide single layers. Nat. Commun. 2012, 3, 1057. [Google Scholar] [CrossRef] [PubMed]
- Huang, W.; Yu, D.; Zhang, M.; Cao, Q.; Yao, J. Predictive cutting force model for ductile-regime machining of brittle materials. Int. J. Adv. Manuf. Technol. 2018, 98, 781–790. [Google Scholar] [CrossRef]
- Material Properties of ZnSe. Available online: https://www.pveducation.org/pvcdrom/materials/znse (accessed on 20 December 2020).
- Jiang, C.; Zhao, G. A Preliminary Study of 3D Printing on Rock Mechanics. Rock Mech. Rock Eng. 2015, 48, 1041–1050. [Google Scholar] [CrossRef]
- Lawn, B.; Wilshaw, R. Indentation fracture: Principles and applications. J. Mater. Sci. 1975, 10, 1049–1081. [Google Scholar] [CrossRef]

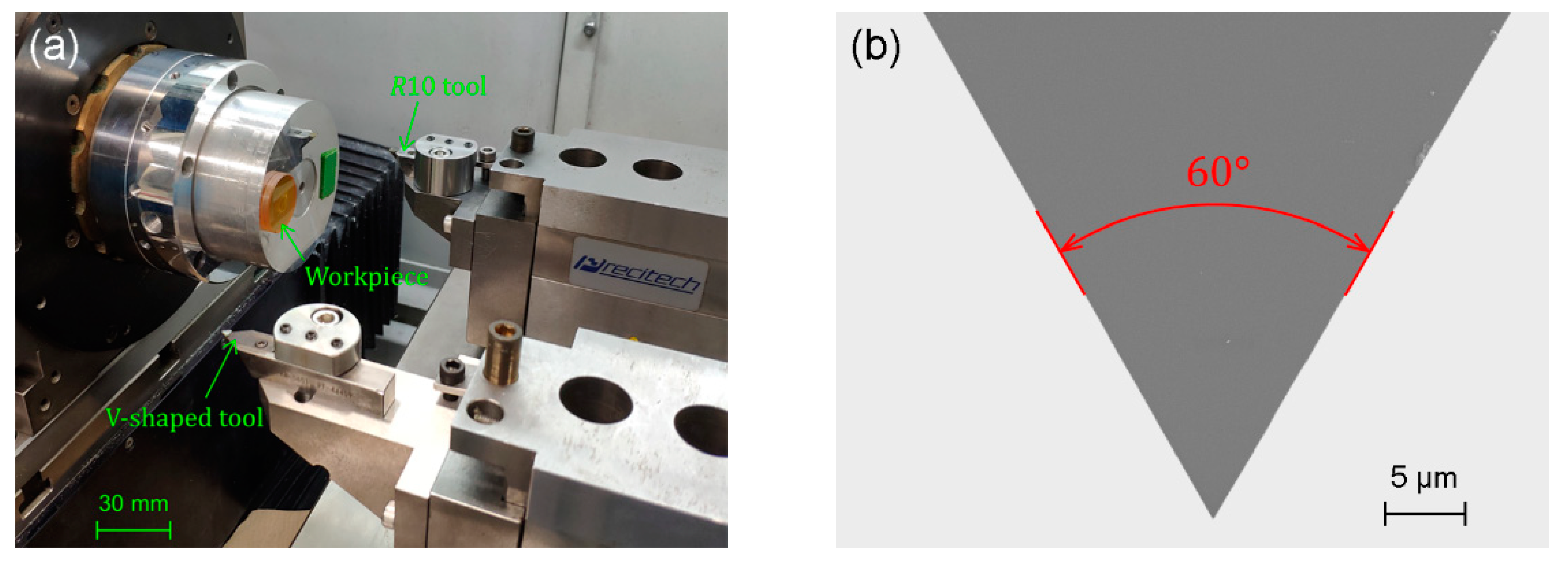






| Exp. No. | Condition | Spindle Rotation Rate S (rpm) | Groove Depth d (μm) | Feed Rate fz (nm/rev) |
|---|---|---|---|---|
| 1–3 | Uncoated workpiece | 50 | 1, 6, 9 | 10 |
| 4–6 | Coated workpiece | 1, 6, 9 | 10 | |
| 7–9 | Coated workpiece | 9 | 20, 40, 60 |
| Grain No. | Cleavage Plane | Groove Surface | Angle (Deg.) | ||
|---|---|---|---|---|---|
| Direction | Normal Vector in SCS | Normal Vector in CCS | |||
| A 1 | (1 0 −1) | Left | 86.5 | ||
| Right | 60.5 | ||||
| B 2 | Left | 44.8 | |||
| Right | 79.4 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, W.; Yan, J. Fundamental Investigation of Diamond Cutting of Micro V-Shaped Grooves on a Polycrystalline Soft-Brittle Material. J. Manuf. Mater. Process. 2021, 5, 17. https://doi.org/10.3390/jmmp5010017
Huang W, Yan J. Fundamental Investigation of Diamond Cutting of Micro V-Shaped Grooves on a Polycrystalline Soft-Brittle Material. Journal of Manufacturing and Materials Processing. 2021; 5(1):17. https://doi.org/10.3390/jmmp5010017
Chicago/Turabian StyleHuang, Weihai, and Jiwang Yan. 2021. "Fundamental Investigation of Diamond Cutting of Micro V-Shaped Grooves on a Polycrystalline Soft-Brittle Material" Journal of Manufacturing and Materials Processing 5, no. 1: 17. https://doi.org/10.3390/jmmp5010017
APA StyleHuang, W., & Yan, J. (2021). Fundamental Investigation of Diamond Cutting of Micro V-Shaped Grooves on a Polycrystalline Soft-Brittle Material. Journal of Manufacturing and Materials Processing, 5(1), 17. https://doi.org/10.3390/jmmp5010017






