An Analytical Method for Modelling Pull-In Effect during Anodic Bonding †
Abstract
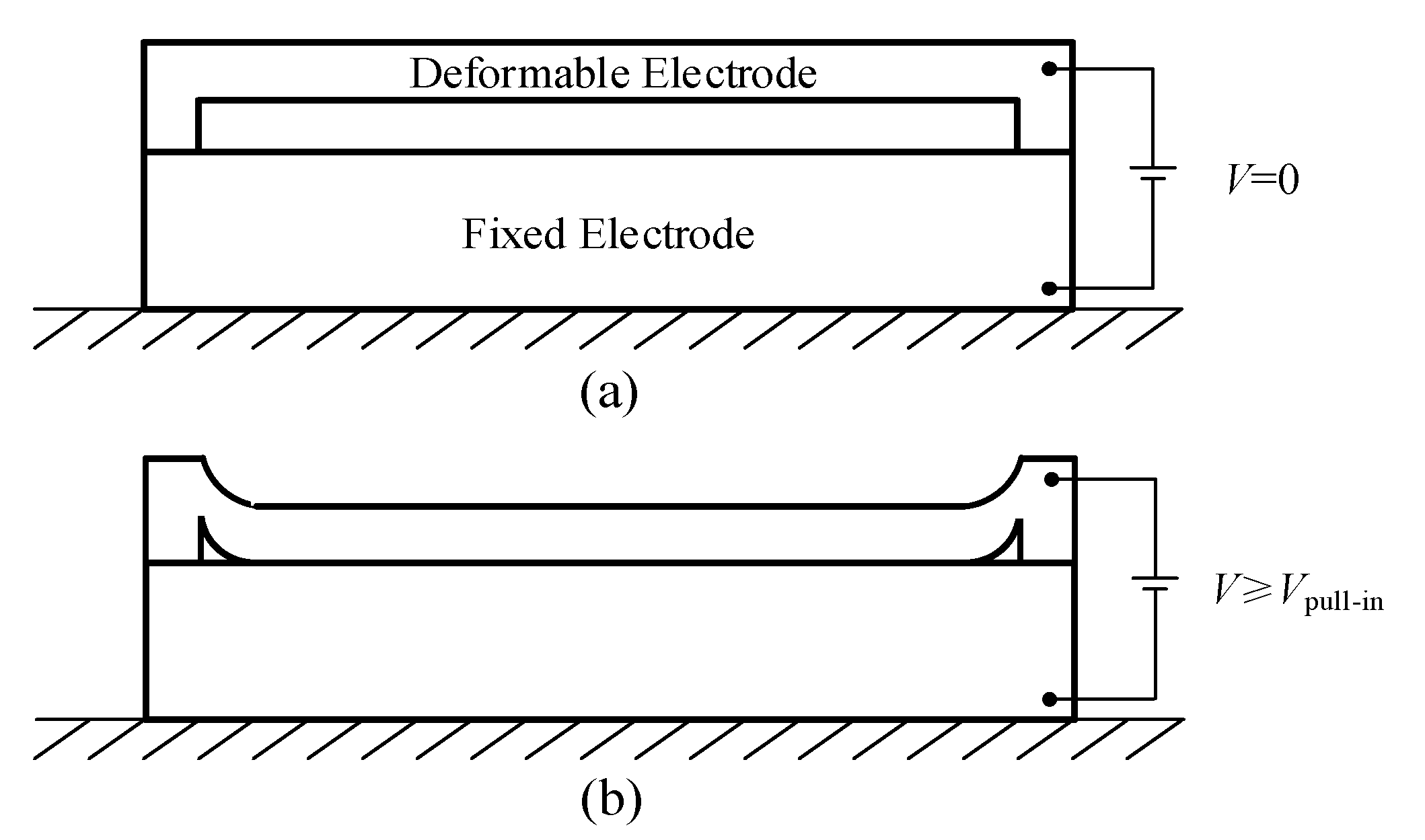
:1. Introduction
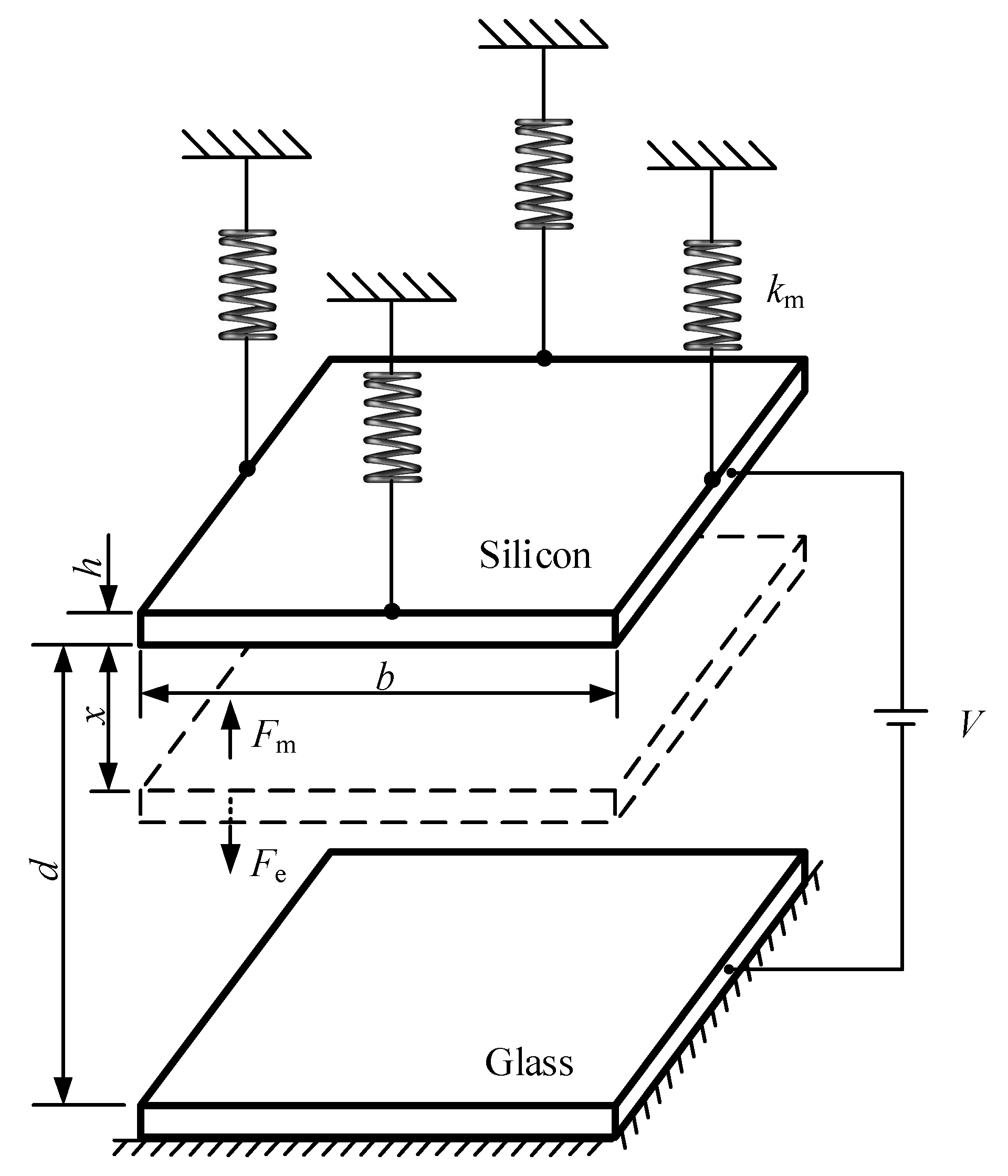
2. Methods
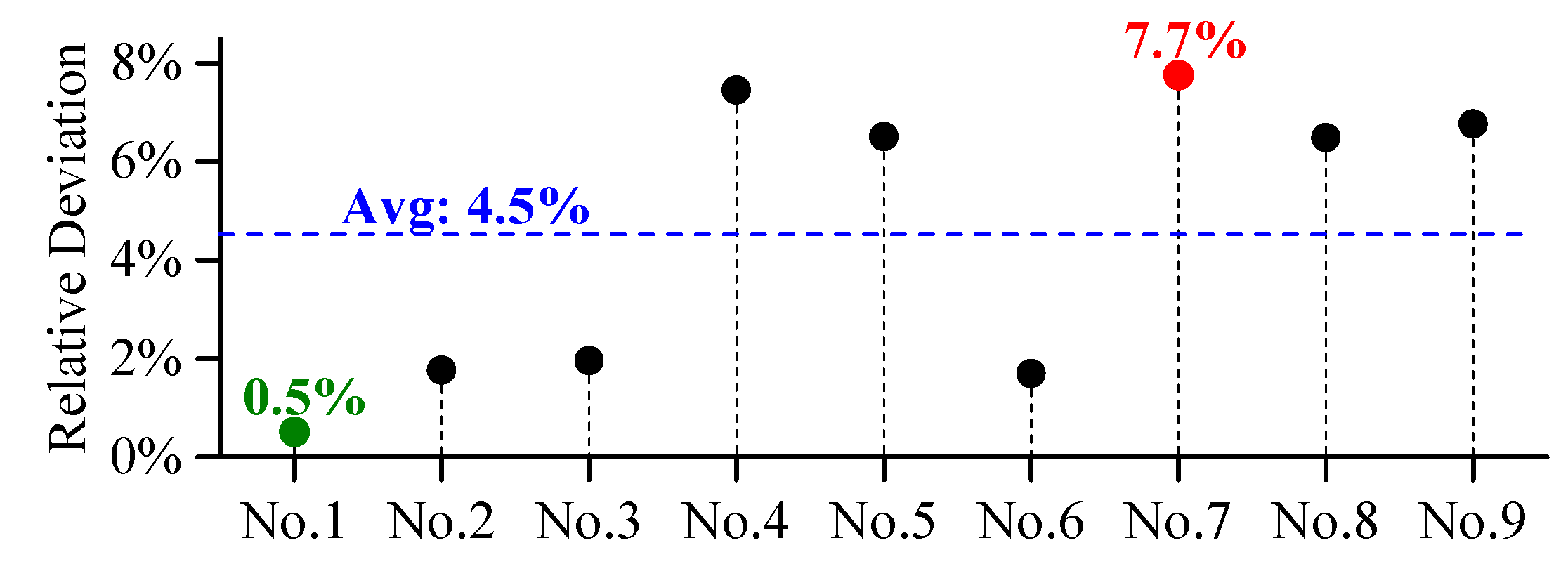
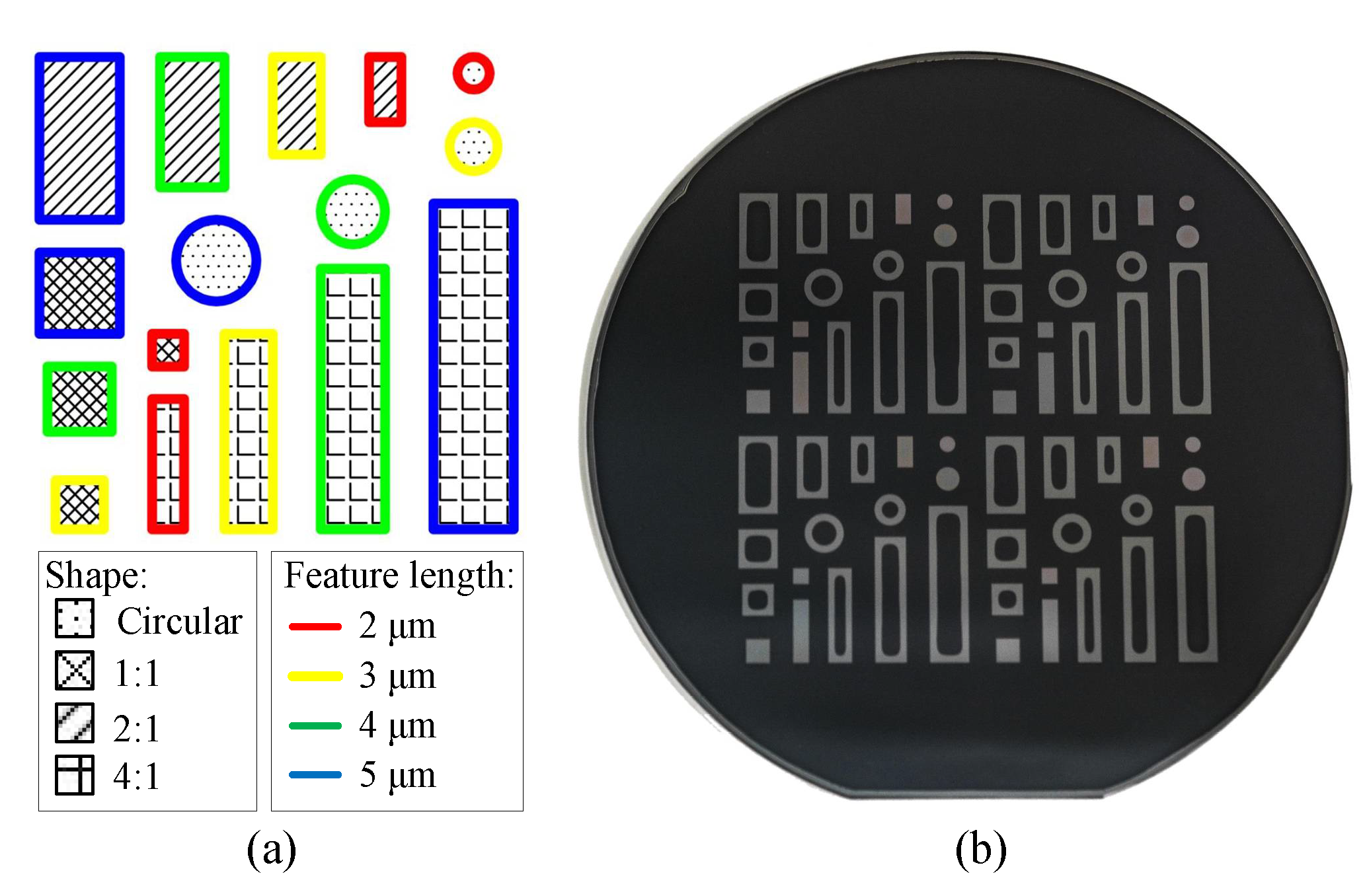
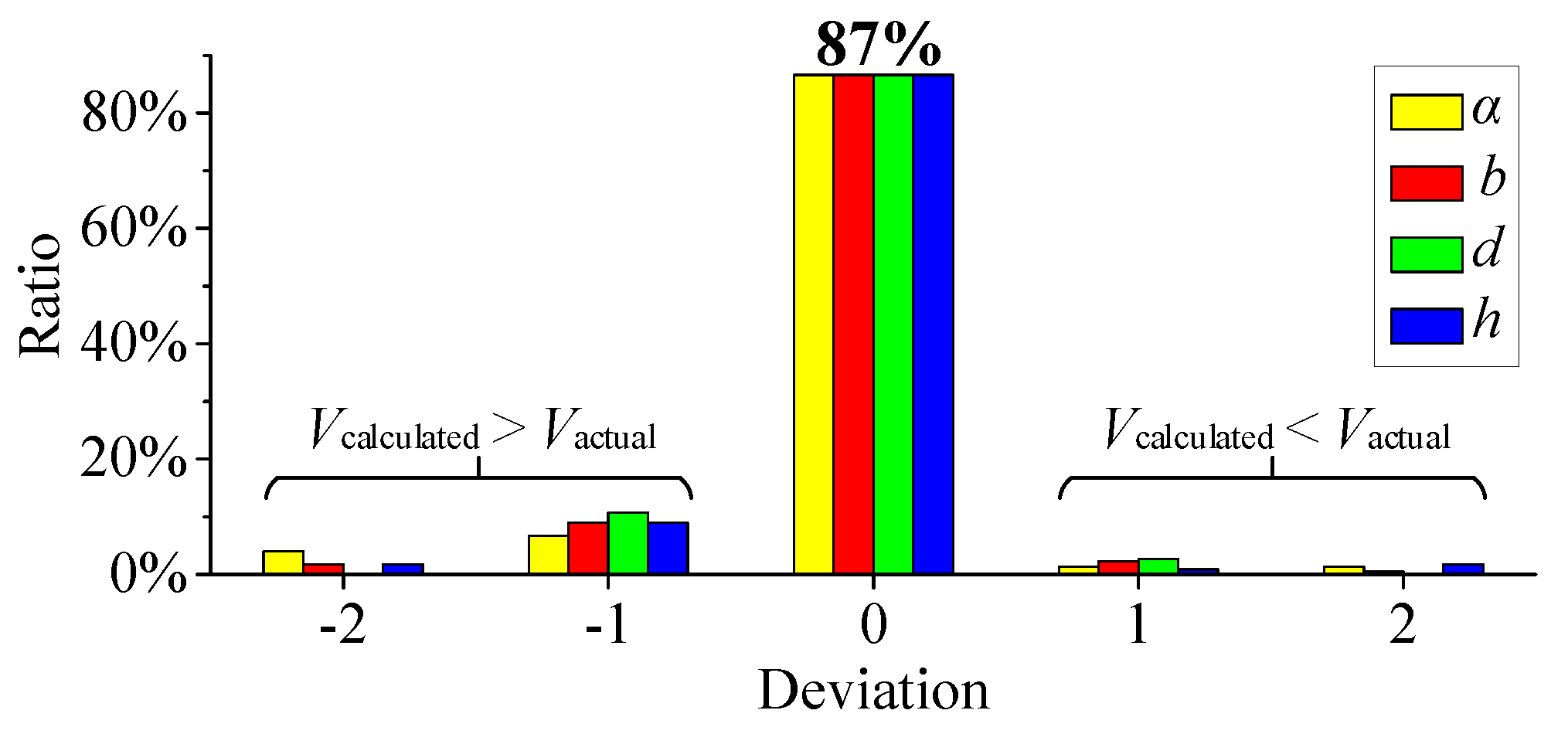
3. Results and Discussion
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Batra, R.C.; Porfiri, M.; Spinello, D. Review of modeling electrostatically actuated microelectromechanical systems. Smart Mater. Struct. 2007, 16, 23–31. [Google Scholar] [CrossRef]
- Yamaner, F.Y.; Zhang, X.; Oralkan, O. Fabrication of anodically bonded capacitive micromachined ultrasonic transducers with vacuum-sealed cavities. In Proceedings of the 2014 IEEE International Ultrasonics Symposium, Chicago, IL, USA, 3–6 September 2014; pp. 604–607. [Google Scholar]
- Lin, M. Micro focusing deformable mirror fabricated by combination with silicon based and nonsilicon based micromachining. In Proceedings of the 2009 4th International Microsystems, Packaging, Assembly and Circuits Technology Conference, Taipei, Taiwan, 21–23 October 2009; pp. 315–318. [Google Scholar]
- Zhang, X.; Yamaner, F.Y.; Oralkan, O. Fabrication of vacuum-sealed capacitive micromachined ultrasonic transducers with through-glass-via interconnects using anodic bonding. J. Microelectromech. Syst. 2017, 26, 226–234. [Google Scholar] [CrossRef]
- Sangwoo, L.; Jaeyoong, C.; Najafi, K. Fabrication of vertical comb electrodes using selective anodic bonding. In Proceedings of the 2007 20th IEEE International Conference on Micro Electro Mechanical Systems, Hyogo, Japan, 21–25 January 2007; pp. 349–352. [Google Scholar]

| Circular Diagram | Rectangle Diagram: a/b | ||||||
|---|---|---|---|---|---|---|---|
| 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | ∞ | |
| 0.0108 | 0.0138 | 0.0188 | 0.0226 | 0.0251 | 0.0267 | 0.0277 | 0.0284 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, Q.; Xie, B.; Lu, Y.; Chen, D.; Chen, J.; Wang, J. An Analytical Method for Modelling Pull-In Effect during Anodic Bonding. Proceedings 2018, 2, 969. https://doi.org/10.3390/proceedings2130969
Wei Q, Xie B, Lu Y, Chen D, Chen J, Wang J. An Analytical Method for Modelling Pull-In Effect during Anodic Bonding. Proceedings. 2018; 2(13):969. https://doi.org/10.3390/proceedings2130969
Chicago/Turabian StyleWei, Qiuxu, Bo Xie, Yulan Lu, Deyong Chen, Jian Chen, and Junbo Wang. 2018. "An Analytical Method for Modelling Pull-In Effect during Anodic Bonding" Proceedings 2, no. 13: 969. https://doi.org/10.3390/proceedings2130969
APA StyleWei, Q., Xie, B., Lu, Y., Chen, D., Chen, J., & Wang, J. (2018). An Analytical Method for Modelling Pull-In Effect during Anodic Bonding. Proceedings, 2(13), 969. https://doi.org/10.3390/proceedings2130969







