Tuneable Q-Factor of MEMS Cantilevers with Integrated Piezoelectric Thin Films †
Abstract
:1. Introduction
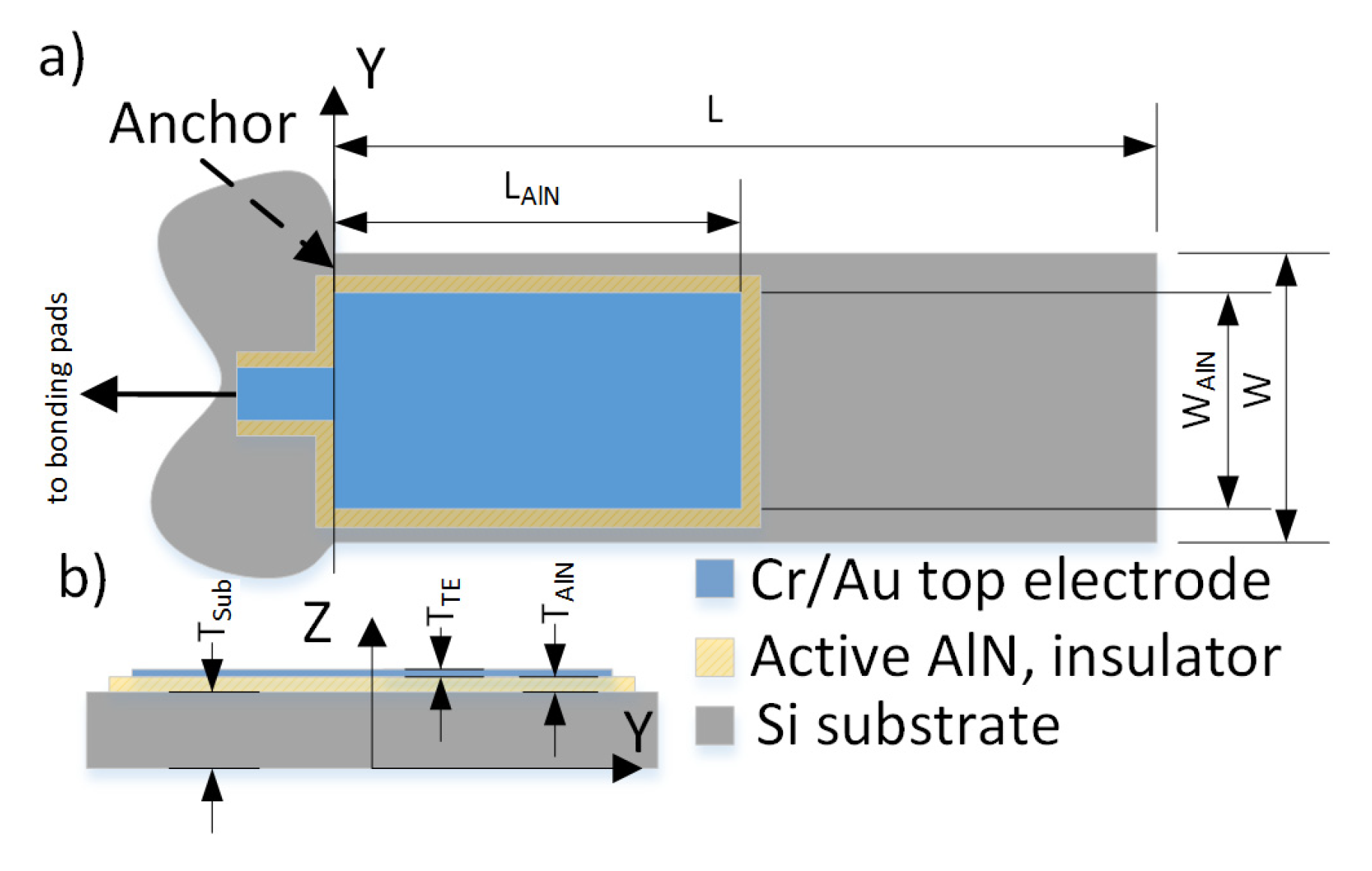
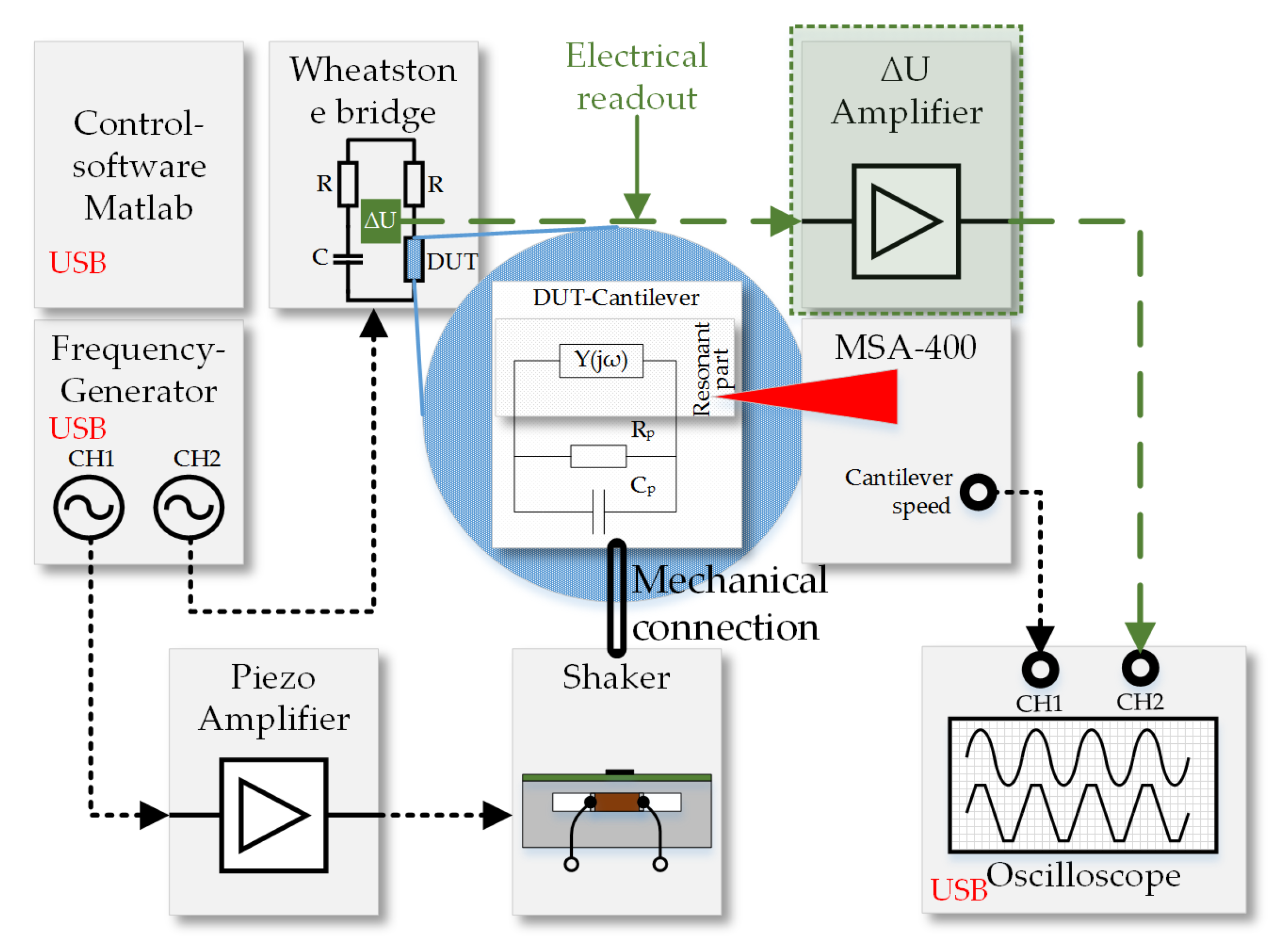
2. Experimental Details
3. Results
4. Summary
Acknowledgments
Conflicts of Interest
References
- Goeders, M.K.; Colton, J.S.; Bottomley, L.A. Microcantilevers: Sensing Chemical Interactions via Mechanical Motion. Chem. Rev. 2008, 108, 522–542. [Google Scholar] [CrossRef] [PubMed]
- Dionne, R.E.; Toader, V.; Badia, A. Microcantilevers Bend to the Pressure of Clustered Redox Centers. Langmuir 2014, 30, 742–752. [Google Scholar] [CrossRef] [PubMed]
- Tadigadapa, S.; Mateti, K. Piezoelectric MEMS sensors: State-of-the-art and perspectives. Meas. Sci. Technol. 2009, 20, 092001. [Google Scholar] [CrossRef]
- Kucera, M.; Wistrela, E.; Pfusterschmied, G.; Ruiz-Díez, V.; Manzaneque, T.; Hernando-García, J.; Sánchez-Rojas, J.L.; Jachimowicz, A.; Schalko, J.; Bittner, A.; et al. Design-dependent performance of self-actuated and self-sensing piezoelectric-AlN cantilevers in liquid media oscillating in the fundamental in-plane bending mode. Sens. Actuators B Chem. 2014, 200, 235–244. [Google Scholar] [CrossRef]
- Michels, T.; Rangelow, I.W. Review of scanning probe micromachining and its applications within nanoscience. Microelectron. Eng. 2014, 126, 191–203. [Google Scholar] [CrossRef]
- Boisen, A.; Dohn, S.; Keller, S.S.; Schmid, S.; Tenje, M. Cantilever-like micromechanical sensors. Rep. Prog. Phys. 2011, 74, 036101. [Google Scholar] [CrossRef]
- Ababneh, A.; Schmid, U.; Hernando, J.; Sánchez-Rojas, J.L.; Seidel, H. The influence of sputter deposition parameters on piezoelectric and mechanical properties of AlN thin films. Mater. Sci. Eng. B 2010, 172, 253–258. [Google Scholar] [CrossRef]
- Pérez-Campos, A.; Iriarte, G.F.; Hernando-Garcia, J.; Calle, F. Post-CMOS compatible high-throughput fabrication of AlN-based piezoelectric microcantilevers. J. Micromech. Microeng. 2015, 25, 025003. [Google Scholar] [CrossRef]
- Ambacher, O. Growth and applications of Group III-nitrides. J. Phys. D Appl. Phys. 1998, 31, 2653–2710. [Google Scholar] [CrossRef]
- Kucera, M.; Hofbauer, F.; Wistrela, E.; Manzaneque, T.; Ruiz-Díez, V.; Sánchez-Rojas, J.L.; Bittner, A.; Schmid, U. Lock-in amplifier powered analogue Q-control circuit for self-actuated self-sensing piezoelectric MEMS resonators. Microsyst. Technol. 2014, 20, 615–625. [Google Scholar] [CrossRef]
- Hemon, S.; Akjouj, A.; Soltani, A.; Pennec, Y.; El Hassouani, Y.; Talbi, A.; Mortet, V.; Djafari-Rouhani, B. Hypersonic band gap in an AlN-TiN bilayer phononic crystal slab. Appl. Phys. Lett. 2014, 104, 063101. [Google Scholar] [CrossRef]
- De Lima, M.M., Jr.; Paulo, V.S. Modulation of photonic structures by surface acoustic waves. Rep. Prog. Phys. 2005, 68, 1639. [Google Scholar] [CrossRef]
- Steven, A.R.; Henry, A.S. A review of power harvesting using piezoelectric materials (2003–2006). Smart Mater. Struct. 2007, 16, R1. [Google Scholar] [CrossRef]
- Fairbairn, W.M.; Moheimani, S.O.R.; Fleming, A.J. Control of an Atomic Force Microscope Microcantilever: A Sensorless Approach. J. Microelectromech. Syst. 2011, 20, 1372–1381. [Google Scholar] [CrossRef]


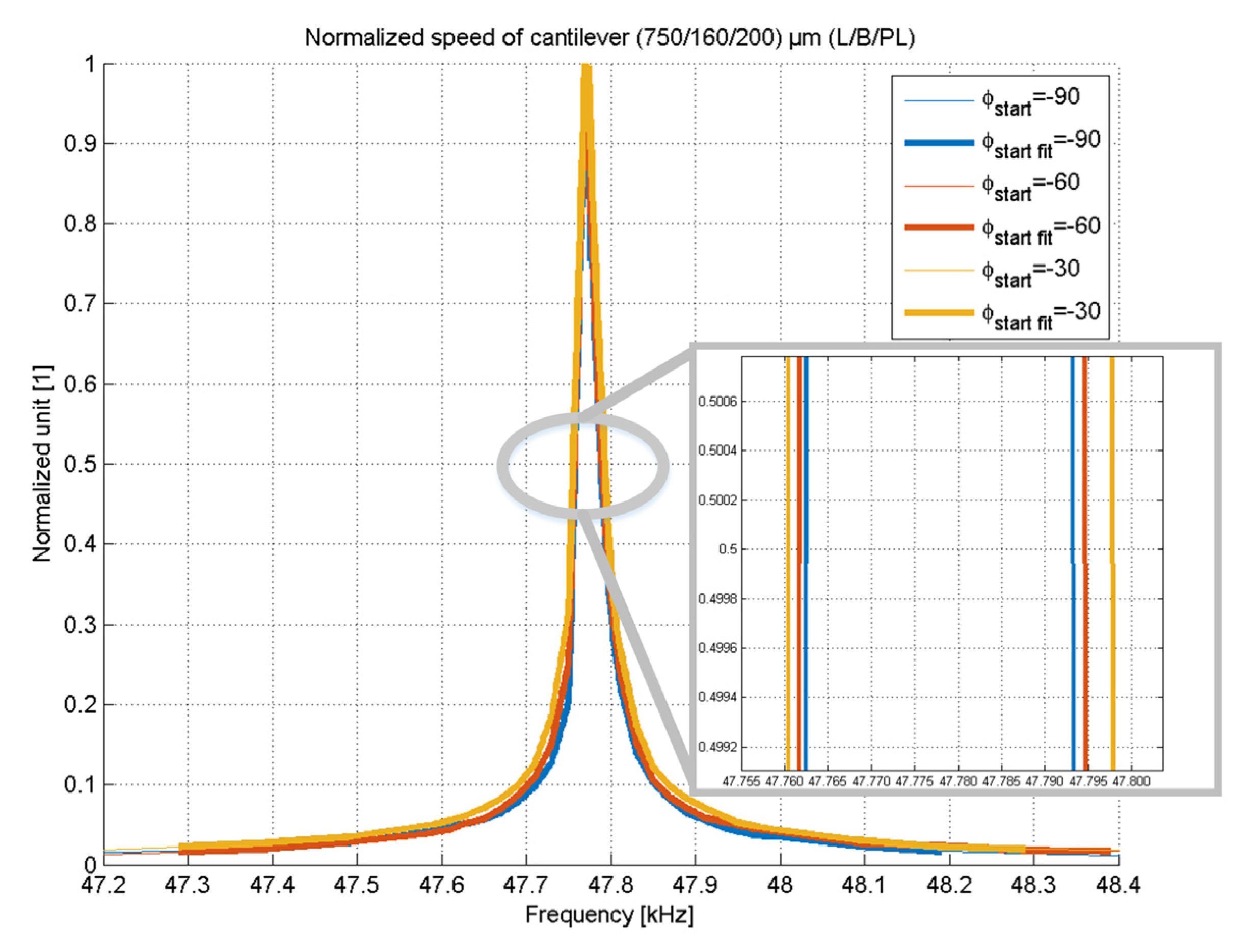
| φstart | Qmech |
|---|---|
| 0 (ϕconstant) | 1080 |
| −60 | 724 |
| −30 | 639 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fischeneder, M.; Oposich, M.; Schneider, M.; Schmid, U. Tuneable Q-Factor of MEMS Cantilevers with Integrated Piezoelectric Thin Films. Proceedings 2017, 1, 380. https://doi.org/10.3390/proceedings1040380
Fischeneder M, Oposich M, Schneider M, Schmid U. Tuneable Q-Factor of MEMS Cantilevers with Integrated Piezoelectric Thin Films. Proceedings. 2017; 1(4):380. https://doi.org/10.3390/proceedings1040380
Chicago/Turabian StyleFischeneder, Martin, Martin Oposich, Michael Schneider, and Ulrich Schmid. 2017. "Tuneable Q-Factor of MEMS Cantilevers with Integrated Piezoelectric Thin Films" Proceedings 1, no. 4: 380. https://doi.org/10.3390/proceedings1040380
APA StyleFischeneder, M., Oposich, M., Schneider, M., & Schmid, U. (2017). Tuneable Q-Factor of MEMS Cantilevers with Integrated Piezoelectric Thin Films. Proceedings, 1(4), 380. https://doi.org/10.3390/proceedings1040380






