Fractal Dimension Analysis of Pore Throat Structure in Tight Sandstone Reservoirs of Huagang Formation: Jiaxing Area of East China Sea Basin
Abstract
1. Introduction
2. Materials and Methods
2.1. Geological Setting and Samples
2.2. Experiment Methods
2.2.1. Thin Section
2.2.2. Scanning Electron Microscopy
2.2.3. X-ray Diffraction Analysis
2.2.4. Scanning Electron Microscopy Mineral Quantitative Evaluation
2.2.5. High Pressure Mercury Injection Experiment
2.3. Fractal Methodology
3. Results
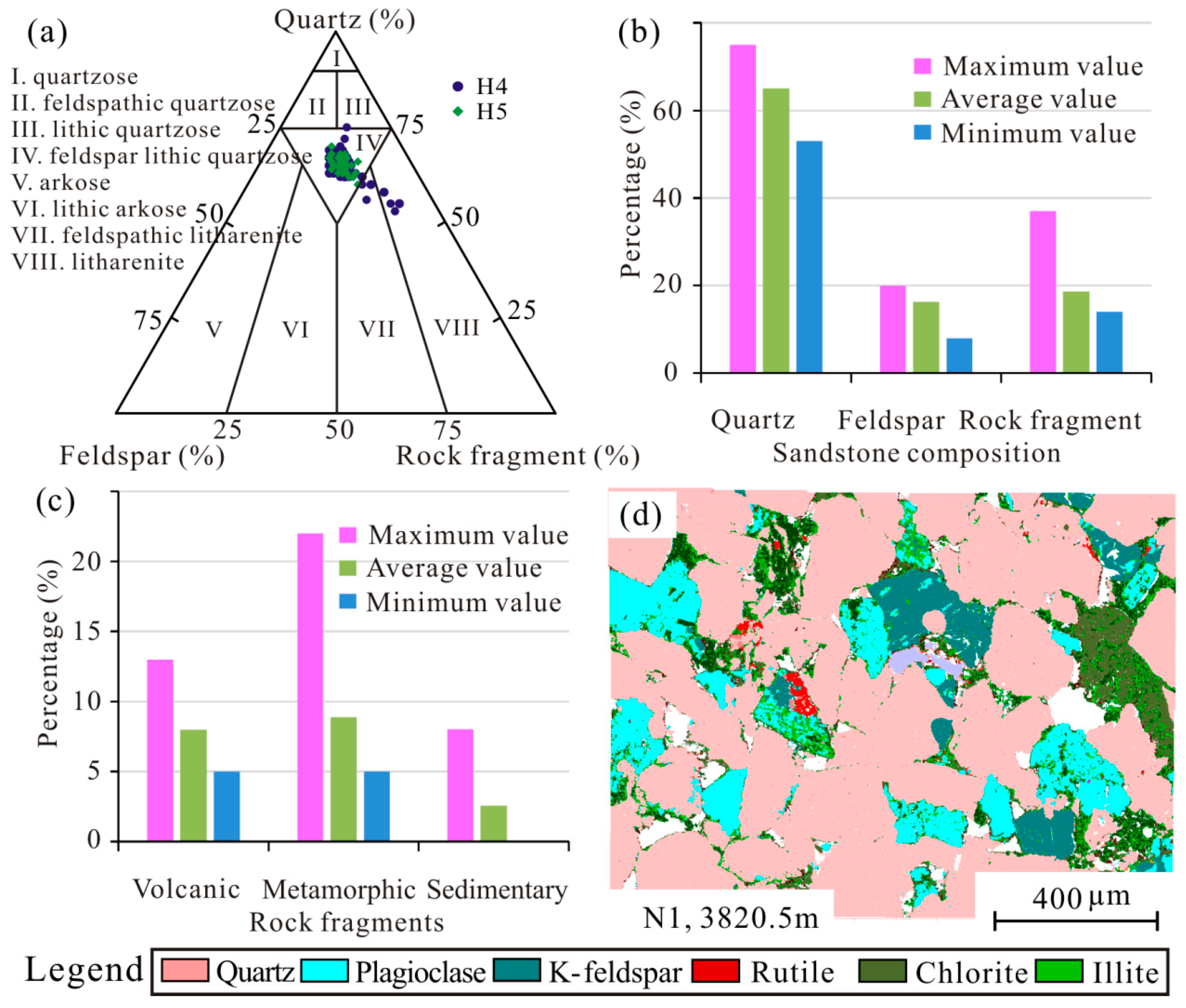
3.1. Reservoir Characteristics
3.2. Diagenesis
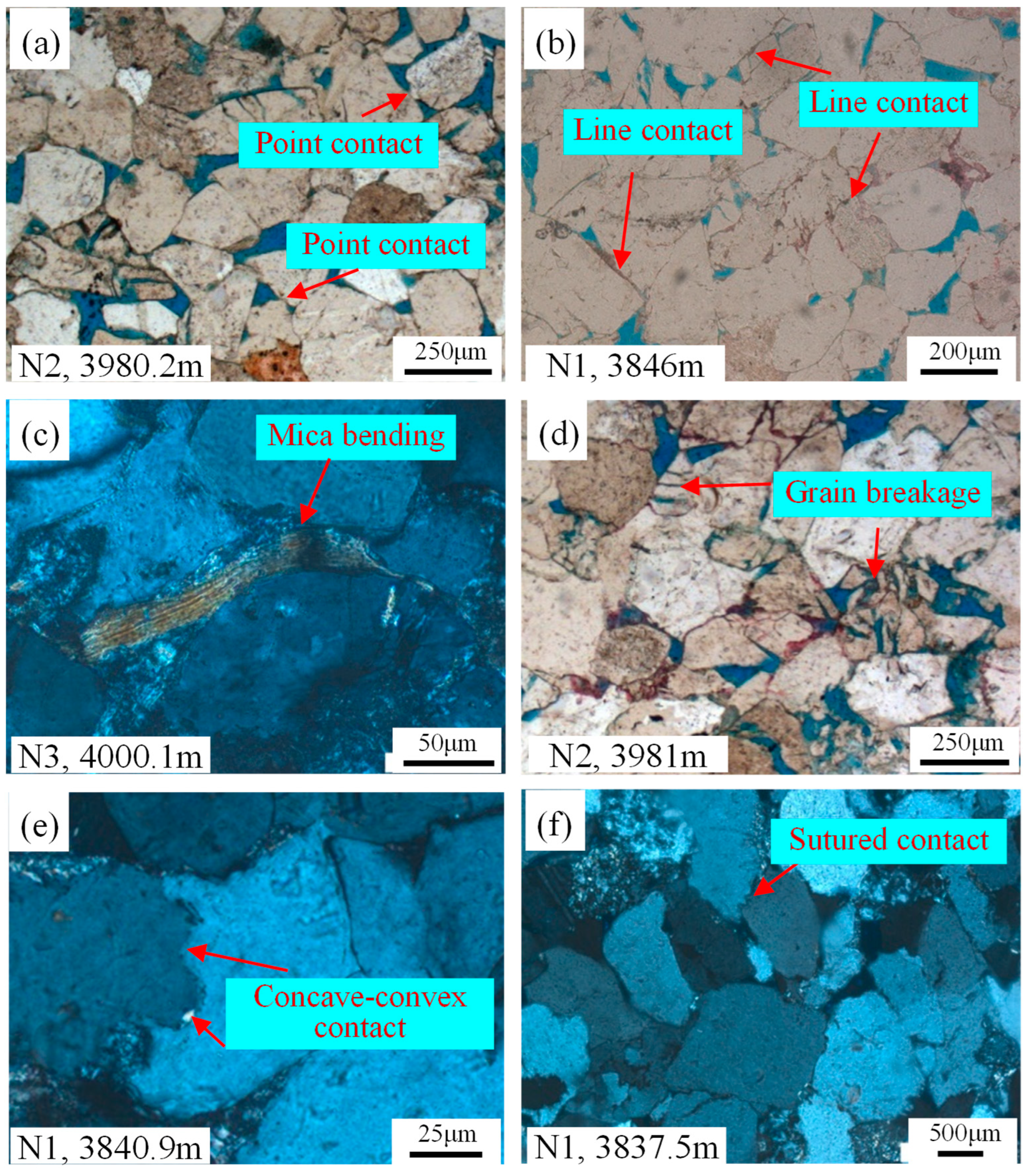
3.2.1. Compaction
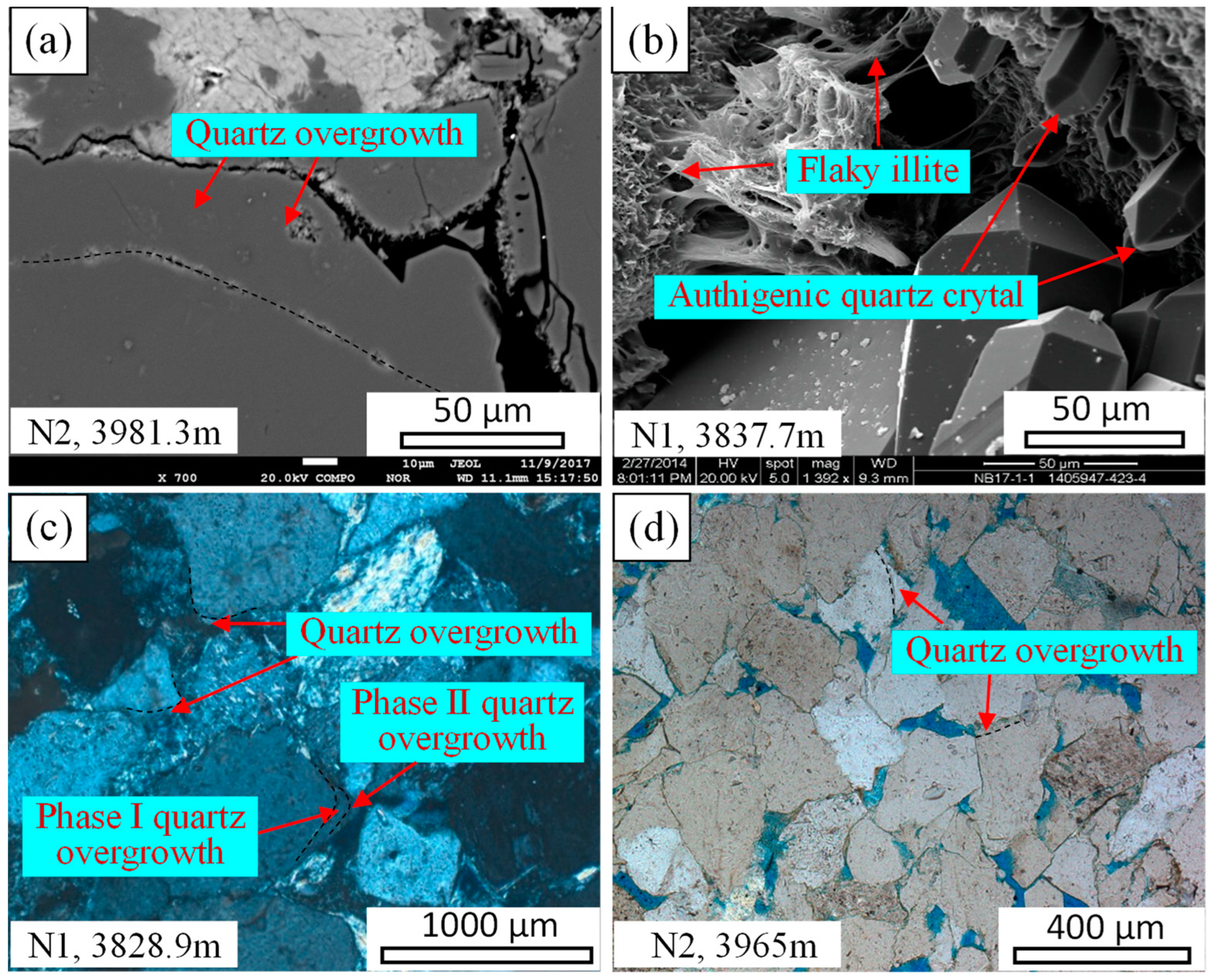
3.2.2. Quartz Cement
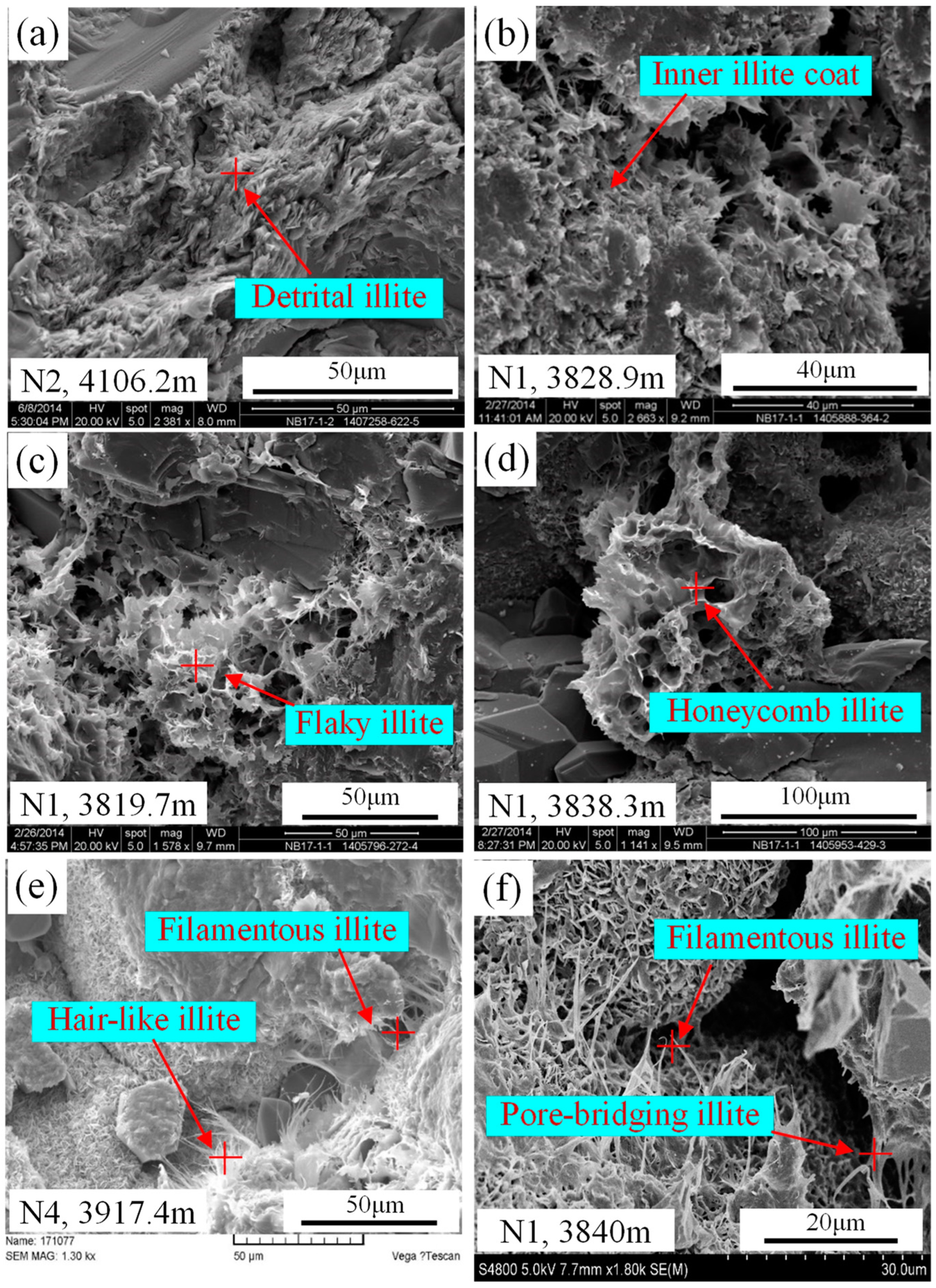
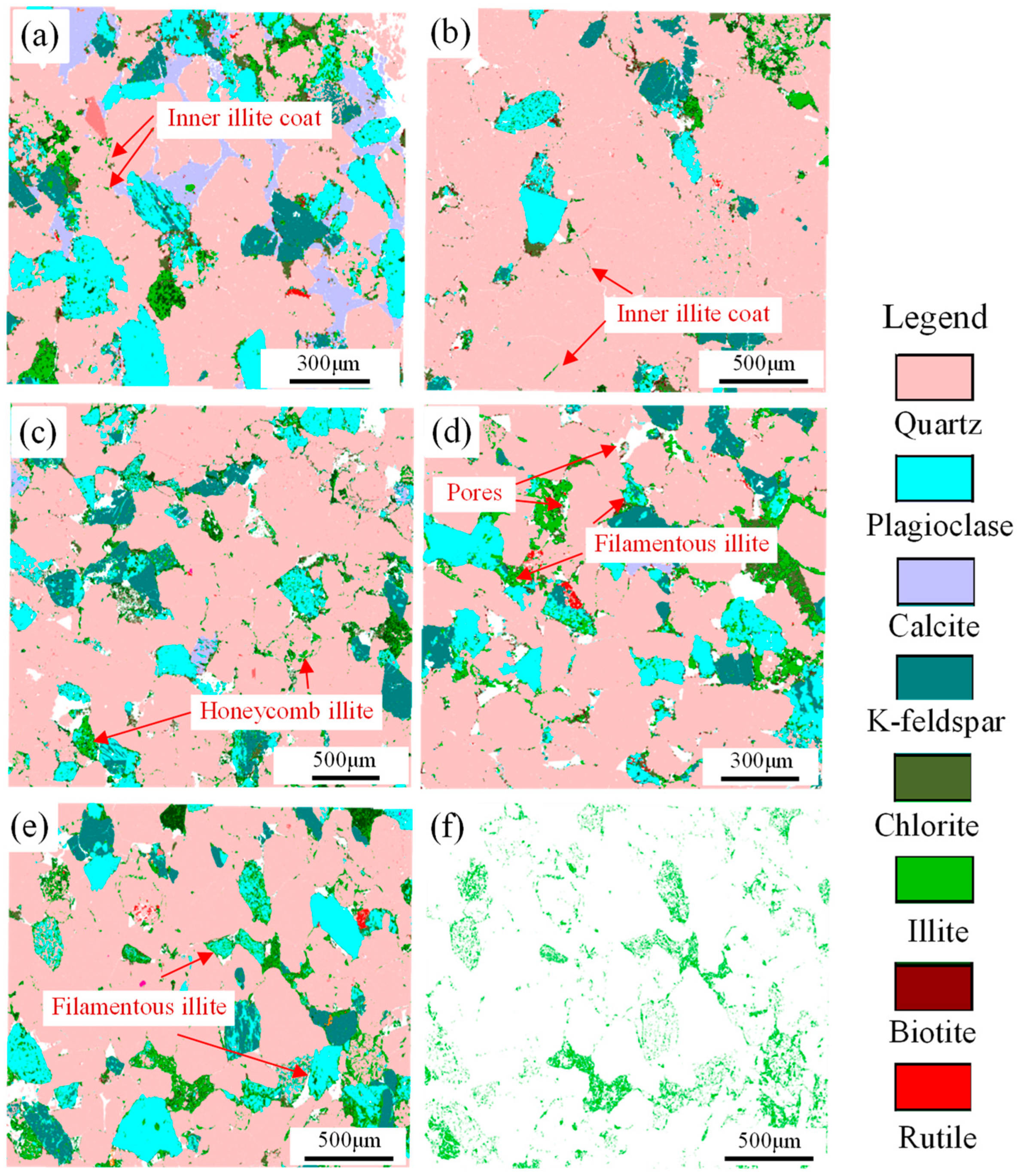
3.2.3. Illite
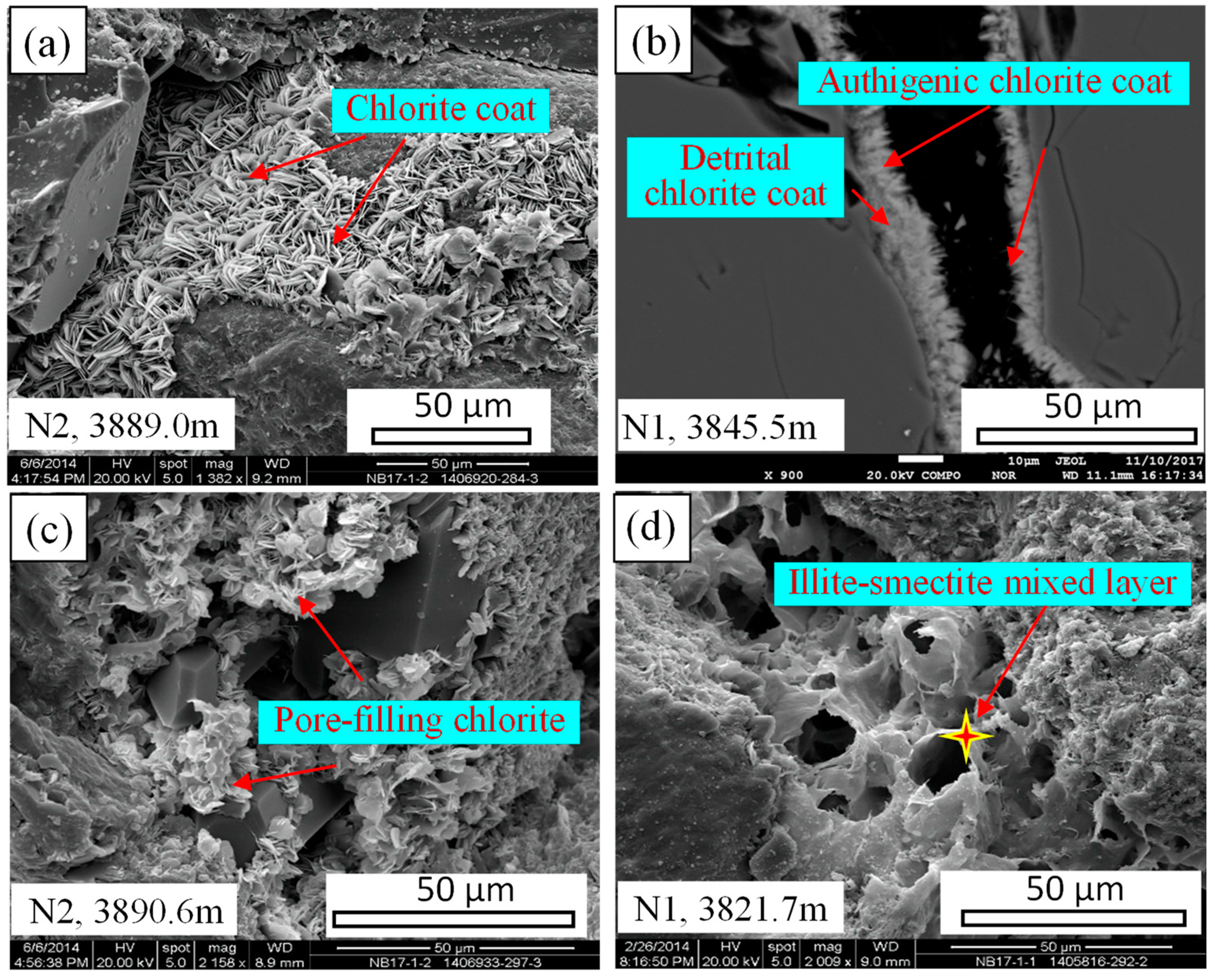
3.2.4. Chlorite and Illite–Smectite Mixed Layers
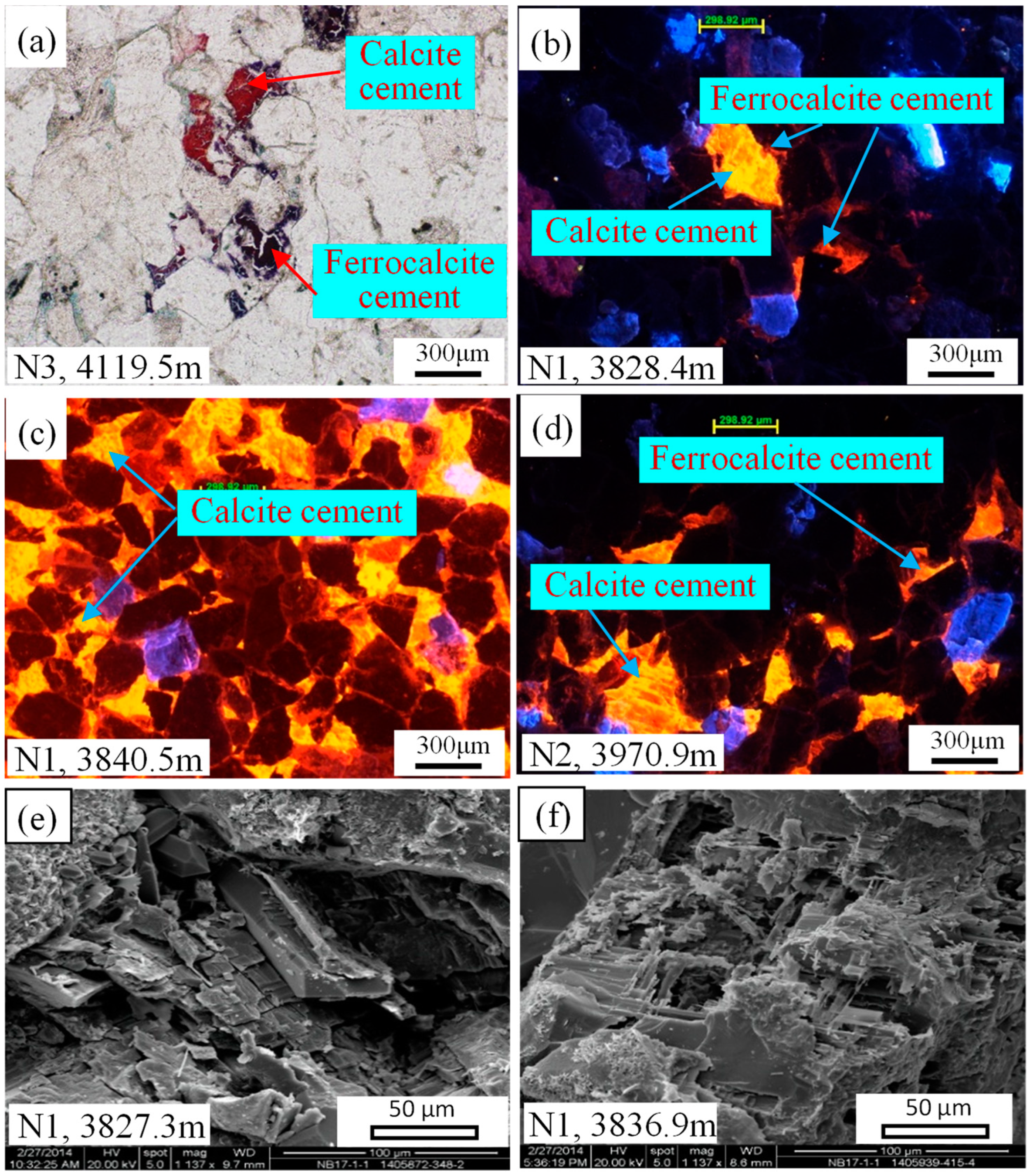
3.2.5. Carbonate Cement
3.2.6. Feldspar Dissolution
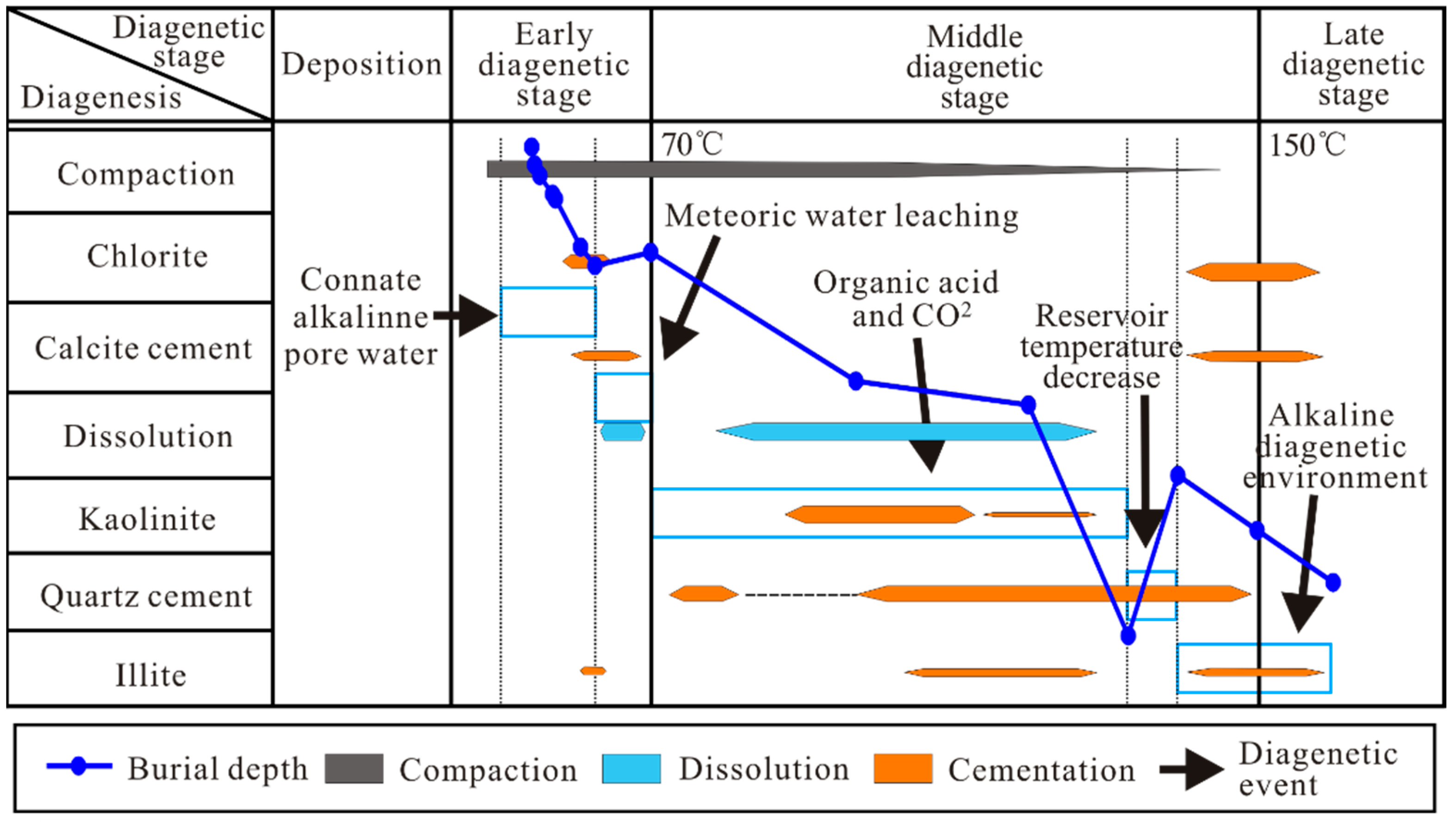
3.2.7. Diagenetic Evolution Sequence
3.3. Pore Throat Structure Characteristics
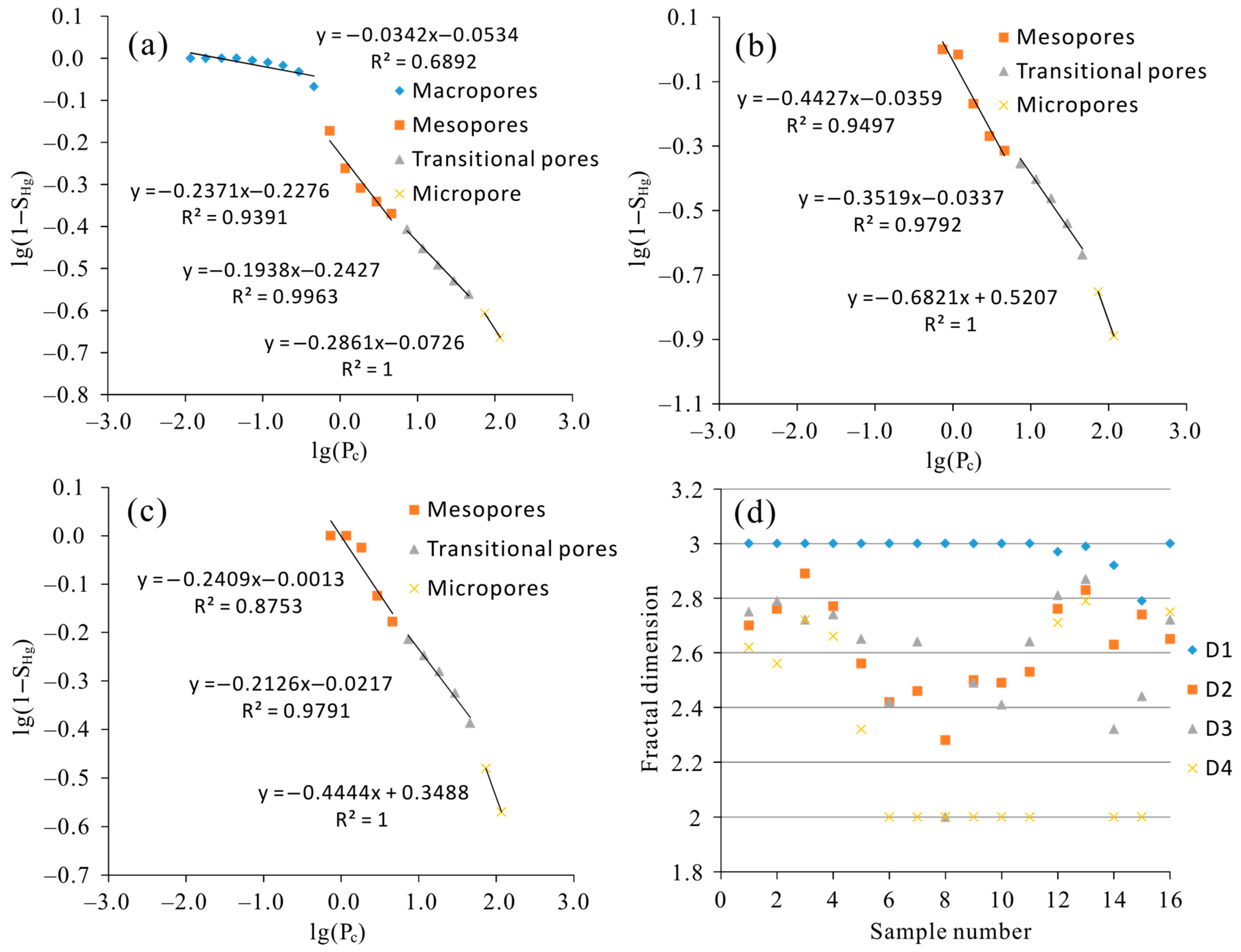
3.4. Fractal Characteristics
4. Discussion
4.1. Relationship between Fractal Dimension, Reservoir Properties and Pore Structure Parameters
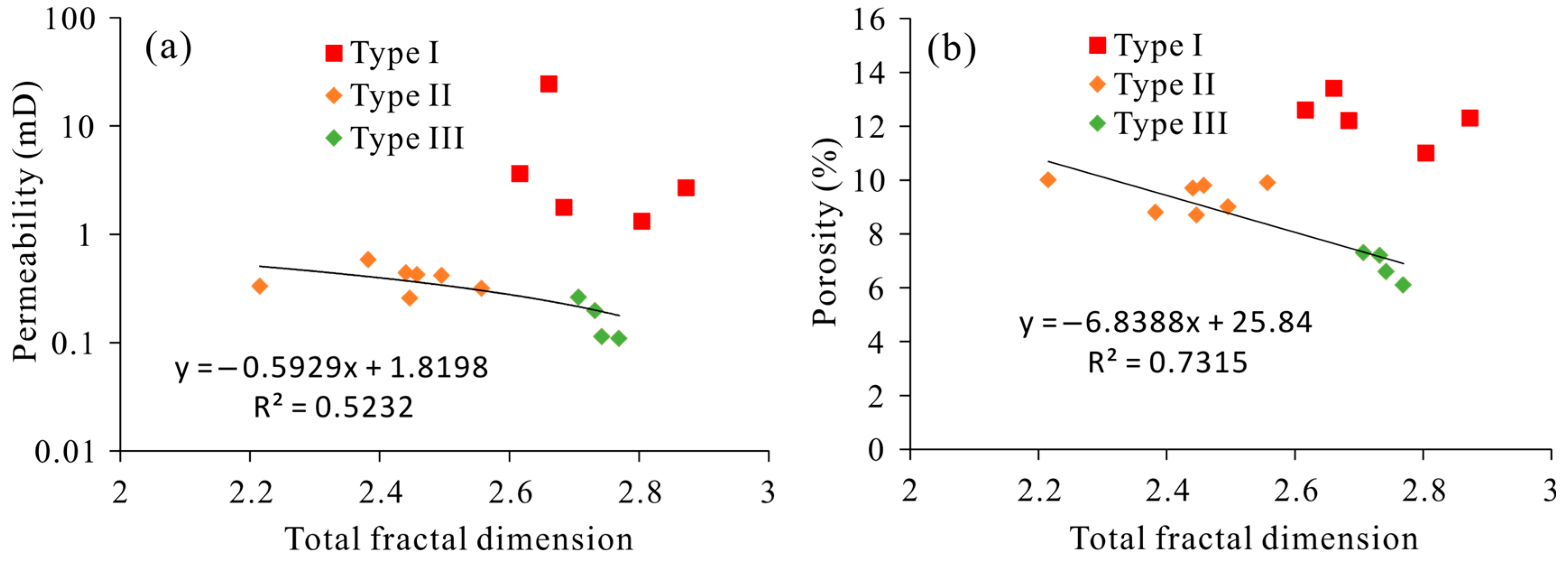
4.1.1. Relationship between Fractal Dimension and Reservoir Properties
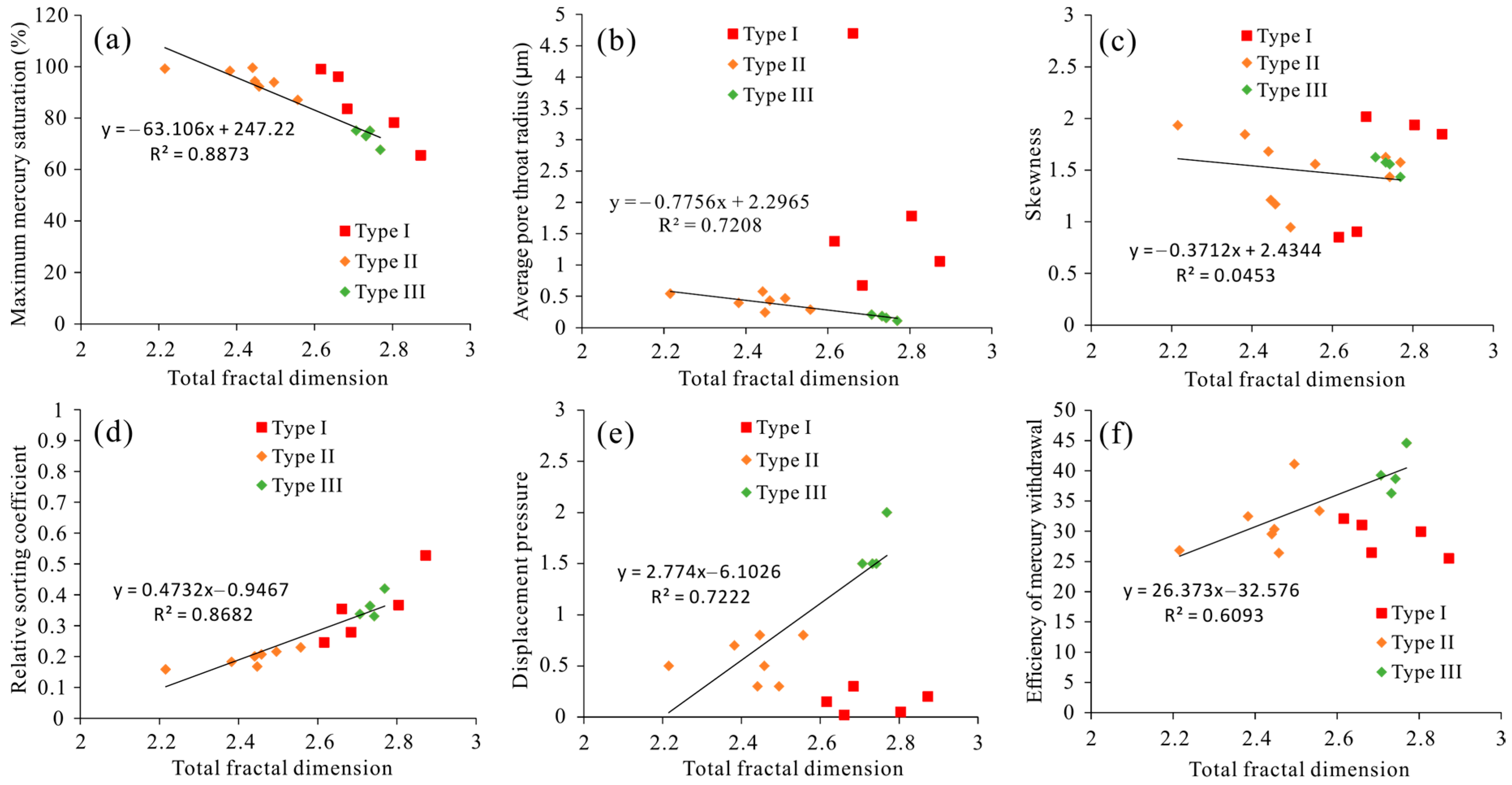
4.1.2. Relationship between Fractal Dimension and Pore Structure Parameters
4.2. Effect of Diagenesis on Fractal Characteristics
4.2.1. Effect of Compaction on Fractal Characteristics
4.2.2. Effect of Cementation on Fractal Characteristics
4.2.3. Effect of Dissolution on Fractal Characteristics
4.3. Implication for Sweet Spot Prediction
5. Conclusions
- (1)
- The H4 and H5 tight sandstone is composed of moderately to well sorted, subangular to subrounded feldspathic lithic quartzose. The average values of quartz, feldspar, and rock fragment grains are 65.04%, 16.29%, and 18.68%, respectively. The H4 and H5 sandstone experienced early diagenesis and middle diagenesis. The early diagenesis stage included compaction, chlorite coat formation, calcite cementation, inner illite coat formation, smectite to illite conversion, feldspar dissolution, kaolinite, and quartz cement precipitation. The middle diagenesis stage contained the dissolution of feldspar, rock fragment and carbonate cement, precipitation of kaolinite and quartz cement, illitization of k-feldspar, and formation of illite, chlorite, and ferrocalcite.
- (2)
- The fractal dimension of tight sandstone sweet spots was investigated. The average fractal dimensions of macropores, mesopores, transitional pores, and micropores of type I sweet spots are 2.93, 2.72, 2.632, and 2.45, respectively, and the corresponding average porosities are 3.24%, 5.6%, 2.6%, and 0.87%, respectively. The average fractal dimensions of mesopores, transitional pores, and micropores are 2.46, 2.46, and 2.05, respectively, and the corresponding average porosities are 6.16%, 2.35%, and 0.85%, respectively. The average fractal dimensions of mesopores, transitional pores, and micropores are 2.78, 2.75, and 2.64, respectively, and the corresponding average porosities are 2.98%, 2.77%, and 1.05%, respectively.
- (3)
- The relationship between fractal dimension, reservoir properties, and pore structure parameters was determined. The negative correlation between total fractal dimension, porosity, and permeability of type I sweet spots was different from that of type II and type III sweet spots. The negative correlation between the total fractal dimensions of type II and type III sweet spots and maximum mercury saturation, average pore throat radius, and skewness were significant, whereas the correlation between total fractal dimensions of type I sweet spots and maximum mercury saturation, average pore throat radius and skewness were not significant. The positive correlation between the total fractal dimension of type II and type III sweet spots and the relative sorting coefficient, displacement pressure, and efficiency of mercury withdrawal were significant, whereas the correlation between the total fractal dimension of type I sweet spots and relative sorting coefficient, displacement pressure and efficiency of mercury withdrawal were not significant.
- (4)
- The effect of diagenesis on fractal dimensions was investigated. Compaction reduced pore space of tight sandstone and increased fractal dimension. Quartz cementation and calcite cementation blocked pores and throats, reduced pore space, and increased fractal dimensions. Chlorite coat can inhibit compaction, protect pore throat structures, and maintain fractal dimensions. Most clay minerals filled primary pores and secondary pores, destroyed sandstone porosity and permeability, and increased fractal dimensions. Dissolution increased the pore space of tight sandstone and decreased the fractal dimension of the pore throat structure.
- (5)
- The pore throat structures of type I sweet spots was mainly composed of macropores, mesopores, transitional pores, and micropores, and fractal dimensions of type I sweet spots was chiefly controlled by chlorite coat formation, dissolution, and a small amount of compaction. This study shows that fractal dimensions of pore throat structures of the sweet spots and the effect of diagenesis on fractal dimensions can improve the understanding of the reservoir quality of tight sandstone.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kong, X.; Xiao, D.; Jiang, S.; Lu, S.; Sun, B.; Wang, J. Application of the combination of high-pressure mercury injection and nuclear magnetic resonance to the classification and evaluation of tight sandstone reservoirs: A case study of the Linxing Block in the Ordos Basin. Nat. Gas Ind. B 2020, 7, 433–442. [Google Scholar] [CrossRef]
- Li, J.J.; Jiang, H.Q.; Wang, C.; Zhao, Y.Y.; Gao, Y.J.; Pei, Y.L.; Wang, C.C.; Dong, H. Pore-scale investigation of microscopic remaining oil variation characteristics in water-wet sandstone using CT scanning. J. Nat. Gas Sci. Eng. 2017, 48, 36–45. [Google Scholar] [CrossRef]
- Zhao, N.; Wang, L.; Zhang, W.; Peng, Y.; Sima, L. Investigation of pore structure characteristics in marine deep-water high-temperature and high-pressure sandstone: A comprehensive characterization combining various experimental techniques. Geoenergy Sci. Eng. 2023, 222, 211433. [Google Scholar] [CrossRef]
- Zhou, Y.; Xu, J.; Lan, Y.; Zi, H.; Cui, Y.; Chen, Q.; You, L.; Fan, X.; Wang, G. New insights into pore fractal dimension from mercury injection capillary pressure in tight sandstone. Geoenergy Sci. Eng. 2023, 228, 212059. [Google Scholar] [CrossRef]
- Ge, X.; Fan, Y.; Deng, S.; Han, Y.; Liu, J. An improvement of the fractal theory and its application in pore structure evaluation and permeability estimation. J. Geophys. Res. Solid Earth 2016, 121, 6333–6345. [Google Scholar] [CrossRef]
- Fan, X.; Wang, G.; Li, Y.; Dai, Q.; Song, L.; Duan, C.; Zhang, C.; Zhang, F. Pore structure evaluation of tight reservoirs in the mixed siliciclasticcarbonate sediments using fractal analysis of NMR experiments and logs. Mar. Pet. Geol. 2019, 109, 484–493. [Google Scholar] [CrossRef]
- Dai, Q.; Wang, G.; Zhao, X.; Han, Z.; Lu, K.; Lai, J.; Wang, S.; Li, D.; Li, Y.; Wu, K. Fractal model for permeability estimation in low-permeable porous media with variable pore sizes and unevenly adsorbed water lay. Mar. Pet. Geol. 2021, 130, 105135. [Google Scholar] [CrossRef]
- Liu, W.; Lu, S.; Wang, M.; Zhang, S.; Liu, Y.; Zhou, N.; Guan, Y.; Wang, H. Overall pore characterization of tight sandstone reservoir space and its significance: A case study of the outer expansion area of Long 26 in Longhupao oilfield, Songliao Basin. J. Northeast Pet. Univ. 2018, 42, 41–51. [Google Scholar]
- Guo, R.; Xie, Q.; Qu, X.; Chu, M.; Li, S.; Ma, D.; Ma, X. Fractal characteristics of pore-throat structure and permeability estimation of tight sandstone reservoirs: A case study of Chang 7 of the Upper Triassic Yanchang Formation in Longdong area, Ordos Basin, China. J. Pet. Sci. Eng. 2019, 184, 106555. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, C.; Ouyang, S.; Luo, B.; Zhao, D.; Sun, W.; Awan, R.S.; Lu, Z.; Li, G.; Zang, Q. Investigation of pore-throat structure and fractal characteristics of tight sandstones using HPMI, CRMI, and NMR methods: A case study of the Lower Shihezi Formation in the Sulige area, Ordos Basin. J. Pet. Sci. Eng. 2022, 210, 110053. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, X. Study on characteristics parameters of coal core structure on fractal theory. J. Henan Univ. Nat. Sci. 2023, 53, 729–737. [Google Scholar]
- Mayo, S.; Josh, M.; Nesterets, Y.; Esteban, L.; Pervukhina, M.; Clennell, M.B.; Maksimenko, A.; Hall, C. Quantitative micro-porosity characterization using synchrotron micro-CT and xenon K-edge subtraction in sandstones, carbonates, shales and coal. Fuel 2015, 154, 167–173. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Freeman, M.; He, L.; Agamalian, M.; Melnichenko, Y.B.; Mastalerz, M.; Bustin, R.M.; Radliński, A.P.; Blach, T.P. Characterization of tight gas reservoir pore structure using USANS/SANS and gas adsorption analysis. Fuel 2012, 95, 371–385. [Google Scholar] [CrossRef]
- Huang, H.; Sun, W.; Ji, W.; Zhang, R.; Du, K.; Zhang, S.; Ren, D.; Wang, Y.; Chen, L.; Zhang, X. Effects of pore-throat structure on gas permeability in the tight sandstone reservoirs of the Upper Triassic Yanchang formation in the Western Ordos Basin, China. J. Pet. Sci. Eng. 2018, 162, 602–616. [Google Scholar] [CrossRef]
- Zhang, F.; Jiang, Z.; Sun, W.; Li, Y.; Zhang, X.; Zhu, L.; Wen, M. A multiscale comprehensive study on pore structure of tight sandstone reservoir realized by nuclear magnetic resonance, high pressure mercury injection and constant-rate mercury injection penetration test. Mar. Pet. Geol. 2019, 109, 208–222. [Google Scholar] [CrossRef]
- Qu, Y.; Sun, W.; Tao, R.; Luo, B.; Chen, L.; Ren, D. Pore–throat structure and fractal characteristics of tight sandstones in Yanchang Formation, Ordos Basin. Mar. Pet. Geol. 2020, 120, 104573. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W.H. Freeman & Co. Ltd.: San Francisco, CA, USA, 1983. [Google Scholar]
- Li, P.; Zheng, M.; Bi, H.; Wu, S.; Wang, X. Pore throat structure and fractal characteristics of tight oil sandstone: A case study in the Ordos Basin, China. J. Pet. Sci. Eng. 2017, 149, 665–674. [Google Scholar] [CrossRef]
- Li, K. Analytical derivation of Brooks-Corey type capillary pressure models using fractal geometry and evaluation of rock heterogeneity. J. Pet. Sci. Eng. 2010, 73, 20–26. [Google Scholar] [CrossRef]
- Giri, A.; Tarafdar, S.; Gouze, P.; Dutta, T. Fractal pore structure of sedimentary rocks: Simulation in 2-D using a relaxed bidisperse ballistic deposition model. J. Appl. Geophys. 2012, 87, 40–45. [Google Scholar] [CrossRef]
- Wang, F.; Cheng, Y.; Lu, S.; Jin, K.; Zhao, W. Influence of coalification on the pore characteristics of middle-high rank coal. Energy Fuels 2014, 28, 5729–5736. [Google Scholar] [CrossRef]
- Hu, Q.; Ewing, R.P.; Dultz, S. Low pore connectivity in natural rock. J. Contam. Hydrol. 2012, 133, 76–83. [Google Scholar] [CrossRef] [PubMed]
- Guo, X.; Huang, Z.; Zhao, L.; Han, W.; Ding, C.; Sun, X.; Yan, R.; Zhang, T.; Yang, X.; Wang, R. Pore structure and multi-fractal analysis of tight sandstone using MIP, NMR and NMRC methods: A case study from the Kuqa depression, China. J. Pet. Sci. Eng. 2019, 178, 544–558. [Google Scholar] [CrossRef]
- Qiao, J.; Zeng, J.; Jiang, S.; Wang, Y. Impacts of sedimentology and diagenesis on pore structure and reservoir quality in tight oil sandstone reservoirs: Implications for macroscopic and microscopic heterogeneities. Mar. Pet. Geol. 2020, 111, 279–300. [Google Scholar] [CrossRef]
- Deng, Y. Analysis on differences of petroleum type and geological conditions between two depression belts in China offshore. Acta Pet. Sin. 2009, 30, 1–8. [Google Scholar] [CrossRef]
- Gao, M.; Lu, Y.; Du, X.; Ma, Y.; Zhang, J.; Deng, K. Multi-factor evaluation for fine grading of tight sandstone reservoirs: A case study from H3 sand group in the upper section of Oligocene Huagang Formation, Xihu Sag, East China Sea Basin. Pet. Geol. Exp. 2021, 43, 1097–1106. [Google Scholar]
- Wang, W.; Lin, C.; Zhang, X.; Dong, C.; Ren, L.; Lin, J. Seismic diagenetic facies prediction of tight sandstone in the offshore sparse well area: An example from the Xihu Depression of the East China Sea Basin. J. Pet. Sci. Eng. 2022, 216, 110825. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, S. Natural gas migration and accumulation patterns in the central-north Xihu Sag, East China Sea Basin. Nat. Gas Geosci. 2021, 32, 1163–1176. [Google Scholar] [CrossRef]
- Zhang, Z.; He, X.; Tang, X.; Zhu, H. Structural trap characteristics and reservoir types in Xihu Sag, East China Sea Basin. Mar. Geol. Front. 2022, 38, 27–35. [Google Scholar] [CrossRef]
- Su, A.; Chen, H.; Chen, X.; He, C.; Liu, H.; Li, Q.; Wang, C. The characterisitcs of low permeability reservoirs, gas origin, generation and charge in the central and western Xihu depression, East China Sea Basin. J. Nat. Gas Sci. Eng. 2018, 53, 94–109. [Google Scholar] [CrossRef]
- Stroker, T.M.; Harris, N.B.; Crawford Elliott, W.; Marion Wampler, J. Diagenesis of a tight gas sand reservoir: Upper Cretaceous Mesaverde Group, Piceance Basin, Colorado. Mar. Pet. Geol. 2013, 40, 48–68. [Google Scholar] [CrossRef]
- Scherer, M. Parameters influencing porosity in sandstones: A model for sandstone porosity prediction. AAPG Bull. 1987, 71, 1508. [Google Scholar] [CrossRef]
- Houseknecht, D.W. Assessing the relative importance of compaction processes and cementation to reduction of porosity in sandstone. AAPG Bull. 1987, 71, 633–642. [Google Scholar] [CrossRef]
- Wang, W.; Lin, C.; Zhang, X.; Dong, C.; Ren, L.; Lin, J. Provenance, clastic composition and their impact on diagenesis: A case study of the Oligocene sandstone in the Xihu sag, East China Sea Basin. Mar. Pet. Geol. 2021, 126, 104890. [Google Scholar] [CrossRef]
- GBT 29171-2012; Rock Capillary Pressure Measurement. Chinese Petroleum Industry Standard: Beijing, China, 2013.
- Liu, S.; Wang, F.; Yu, R.; Gao, J.; Shi, H.; Zhu, Y. Micro pore throat structure and fractal characteristics of tight sandstone reservoir. J. Jilin Univ. Earth Sci. Ed. 2024, 54, 96–107. [Google Scholar]
- Zhu, W.; Zhang, X.; Zhou, D.; Fang, C.; Li, J.; Huang, Z. New cognition on pore structure characteristics of Permian marine shale in the Lower Yangtze Region and its implications for shale gas exploration. Nat. Gas Ind. 2021, 41, 41–55. [Google Scholar] [CrossRef]
- Xoaoth, B. Coal and Gas Outburst; Song, S., Wang, Y., Eds. and Translators; China Industry Press: Beijing, China, 1966. [Google Scholar]
- Loucks, R.G.; Reed, R.M.; Ruppel, S.C.; Hammes, U. Spectrum of pore types and networks in mudrocks and a descriptive classification for matrix-related mudrock pores. AAPG Bull. 2012, 96, 1071–1098. [Google Scholar] [CrossRef]
- Folk, R.L.; Andrews, P.B.; Lewis, D.W. Detrital sedimentary rock classification and nomenclature for use in New Zealand. N. Z. J. Geol. Geophys. 1970, 13, 937–968. [Google Scholar] [CrossRef]
- Spencer, C.W. Review of characteristics of low-permeability gas reservoirs in western United States. AAPG Bull. 1989, 73, 613–629. [Google Scholar] [CrossRef]
- Mahmic, O.; Dypvik, H.; Hammer, E. Diagenetic influence on reservoir quality evolution, examples from Triassic conglomerates/arenites in the Edvard Grieg field, Norwegian North Sea. Mar. Pet. Geol. 2018, 93, 247–271. [Google Scholar] [CrossRef]
- Zhao, D.; Hou, J.; Sarma, H.; Guo, W.; Liu, Y.; Xie, P.; Dou, L.; Chen, R.; Zhang, Z. Pore throat heterogeneity of different lithofacies and diagenetic effects in gravelly braided river deposits: Implications for understanding the formation process of high-quality reservoirs. Geoenergy Sci. Eng. 2023, 221, 111309. [Google Scholar] [CrossRef]
- Wang, W.; Lin, C.; Zhang, X.; Dong, C.; Ren, L.; Lin, J. Effect of burial history on diagenetic and reservoir-forming process of the Oligocene sandstone in Xihu sag, East China Sea Basin. Mar. Pet. Geol. 2019, 112, 104034. [Google Scholar] [CrossRef]
- Wang, W.; Lin, C.; Zhang, X.; Dong, C.; Ren, L.; Lin, J. Discussion of seismic diagenetic facies of deep reservoir in the East China Sea Basin. J. Pet. Sci. Eng. 2022, 208, 109352. [Google Scholar] [CrossRef]
- Wang, W.; Lin, C.; Zhang, X.; Dong, C.; Ren, L.; Lin, J. Diagenesis, diagenetic facies and their relationship with reservoir sweet spot in low-permeability and tight sandstone: Jiaxing area of the Xihu Sag, East China Sea Basin. Minerals 2023, 13, 404. [Google Scholar] [CrossRef]


| Well Name | Depth (m) | Porosity (%) | Permeability (mD) | Sweet Spot | Maximum Mercury Saturation (%) | Relative Sorting Coefficient | Skewness | Average Pore Throat Radius (μm) | Displacement Pressure (MPa) | Efficiency of Mercury Withdrawal (%) |
|---|---|---|---|---|---|---|---|---|---|---|
| N2 | 3960.2 | 7.3 | 0.261 | Type III | 75.1 | 0.34 | 1.62 | 0.21 | 1.5 | 39.25 |
| N2 | 4102.5 | 7.2 | 0.197 | Type III | 73.1 | 0.36 | 1.57 | 0.18 | 1.5 | 36.27 |
| N3 | 4113.7 | 6.1 | 0.109 | Type III | 67.6 | 0.42 | 1.43 | 0.11 | 2 | 44.59 |
| N3 | 4123.8 | 6.6 | 0.113 | Type III | 75.1 | 0.33 | 1.56 | 0.16 | 1.5 | 38.66 |
| N1 | 3823.7 | 9.9 | 0.316 | Type II | 87.1 | 0.23 | 1.85 | 0.29 | 0.8 | 33.36 |
| N1 | 3825.7 | 8.8 | 0.581 | Type II | 98.4 | 0.18 | 1.17 | 0.39 | 0.7 | 32.44 |
| N1 | 3829.3 | 9.8 | 0.424 | Type II | 92.2 | 0.21 | 1.93 | 0.43 | 0.5 | 26.39 |
| N1 | 3831.3 | 10 | 0.331 | Type II | 99.2 | 0.16 | 1.21 | 0.54 | 0.5 | 26.84 |
| N1 | 3833.3 | 8.7 | 0.257 | Type II | 94.4 | 0.17 | 1.68 | 0.24 | 0.8 | 30.34 |
| N1 | 3841.7 | 9.7 | 0.441 | Type II | 99.5 | 0.2 | 0.95 | 0.58 | 0.3 | 29.52 |
| N1 | 3843.3 | 9 | 0.416 | Type II | 93.9 | 0.22 | 1.53 | 0.47 | 0.3 | 41.11 |
| N1 | 3821.7 | 11 | 1.32 | Type I | 78.3 | 0.37 | 1.94 | 1.78 | 0.05 | 29.93 |
| N1 | 3827.7 | 12.3 | 2.67 | Type I | 65.6 | 0.53 | 1.85 | 1.06 | 0.2 | 25.5 |
| N1 | 3846 | 12.6 | 3.63 | Type I | 99 | 0.25 | 0.85 | 1.38 | 0.15 | 32.08 |
| N2 | 3963.1 | 13.4 | 24.3 | Type I | 96.1 | 0.35 | 0.9 | 4.7 | 0.02 | 31.05 |
| N2 | 3973.2 | 12.2 | 1.76 | Type I | 83.6 | 0.28 | 2.02 | 0.67 | 0.3 | 26.45 |
| Well Name | Depth (m) | Sweet Spot | Macropore | Mesopore | Transitional Pore | Micropore | D | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| D1 | Φ1 | D2 | Φ2 | D3 | Φ3 | D4 | Φ4 | ||||
| N2 | 3960.2 | Type III | 2.7 | 3.8 | 2.75 | 2.56 | 2.62 | 0.95 | 2.71 | ||
| N2 | 4102.5 | Type III | 2.76 | 3.31 | 2.79 | 2.52 | 2.56 | 1.37 | 2.73 | ||
| N3 | 4113.7 | Type III | 2.89 | 1.77 | 2.72 | 3.36 | 2.72 | 0.98 | 2.77 | ||
| N3 | 4123.8 | Type III | 2.77 | 3.04 | 2.74 | 2.64 | 2.66 | 0.92 | 2.74 | ||
| N1 | 3823.7 | Type II | 2.56 | 5.84 | 2.65 | 2.87 | 2.32 | 1.19 | 2.56 | ||
| N1 | 3825.7 | Type II | 2.42 | 5.76 | 2.42 | 2.25 | 2 | 0.78 | 2.38 | ||
| N1 | 3829.3 | Type II | 2.46 | 6.76 | 2.64 | 2.16 | 2 | 0.88 | 2.46 | ||
| N1 | 3831.3 | Type II | 2.28 | 7.7 | 2 | 2.1 | 2 | 0.2 | 2.22 | ||
| N1 | 3833.3 | Type II | 2.5 | 5.13 | 2.49 | 2.7 | 2 | 0.87 | 2.45 | ||
| N1 | 3841.7 | Type II | 3 | 0.24 | 2.49 | 6.33 | 2.41 | 2.27 | 2 | 0.85 | 2.44 |
| N1 | 3843.3 | Type II | 3 | 0.18 | 2.53 | 5.58 | 2.64 | 2.07 | 2 | 1.17 | 2.5 |
| N1 | 3821.7 | Type I | 2.97 | 2.01 | 2.76 | 6.03 | 2.81 | 2.15 | 2.71 | 0.81 | 2.8 |
| N1 | 3827.7 | Type I | 2.99 | 2.98 | 2.83 | 5.15 | 2.87 | 2.76 | 2.79 | 1.41 | 2.87 |
| N1 | 3846 | Type I | 2.92 | 3.87 | 2.63 | 5.3 | 2.32 | 2.71 | 2 | 0.72 | 2.62 |
| N2 | 3963.1 | Type I | 2.79 | 6.83 | 2.74 | 3.08 | 2.44 | 2.68 | 2 | 0.8 | 2.66 |
| N2 | 3973.2 | Type I | 3 | 0.49 | 2.65 | 8.42 | 2.72 | 2.68 | 2.75 | 0.61 | 2.68 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Lin, C.; Zhang, X. Fractal Dimension Analysis of Pore Throat Structure in Tight Sandstone Reservoirs of Huagang Formation: Jiaxing Area of East China Sea Basin. Fractal Fract. 2024, 8, 374. https://doi.org/10.3390/fractalfract8070374
Wang W, Lin C, Zhang X. Fractal Dimension Analysis of Pore Throat Structure in Tight Sandstone Reservoirs of Huagang Formation: Jiaxing Area of East China Sea Basin. Fractal and Fractional. 2024; 8(7):374. https://doi.org/10.3390/fractalfract8070374
Chicago/Turabian StyleWang, Wenguang, Chengyan Lin, and Xianguo Zhang. 2024. "Fractal Dimension Analysis of Pore Throat Structure in Tight Sandstone Reservoirs of Huagang Formation: Jiaxing Area of East China Sea Basin" Fractal and Fractional 8, no. 7: 374. https://doi.org/10.3390/fractalfract8070374
APA StyleWang, W., Lin, C., & Zhang, X. (2024). Fractal Dimension Analysis of Pore Throat Structure in Tight Sandstone Reservoirs of Huagang Formation: Jiaxing Area of East China Sea Basin. Fractal and Fractional, 8(7), 374. https://doi.org/10.3390/fractalfract8070374










