Impulsive Control of Variable Fractional-Order Multi-Agent Systems
Abstract
1. Introduction
- -
- We provide two models for a multi-agent system without a leader in which the interaction between agents occurs only at update times. The dynamics in the models are described by a variable-order Caputo fractional derivative with respect to another function.
- -
- We provide a model for a multi-agent system with a leader in which the interaction between any agent and the leader occurs only at update times. The dynamics are described by a Caputo fractional derivative with respect to another function, for which the order is changed at any update time.
- -
- We define and study impulsive Mittag–Leffler stability via impulsive protocol and leader-following consensus via impulsive protocol for the corresponding models.
- -
- All sufficient conditions depend significantly on the impulsive control.
- -
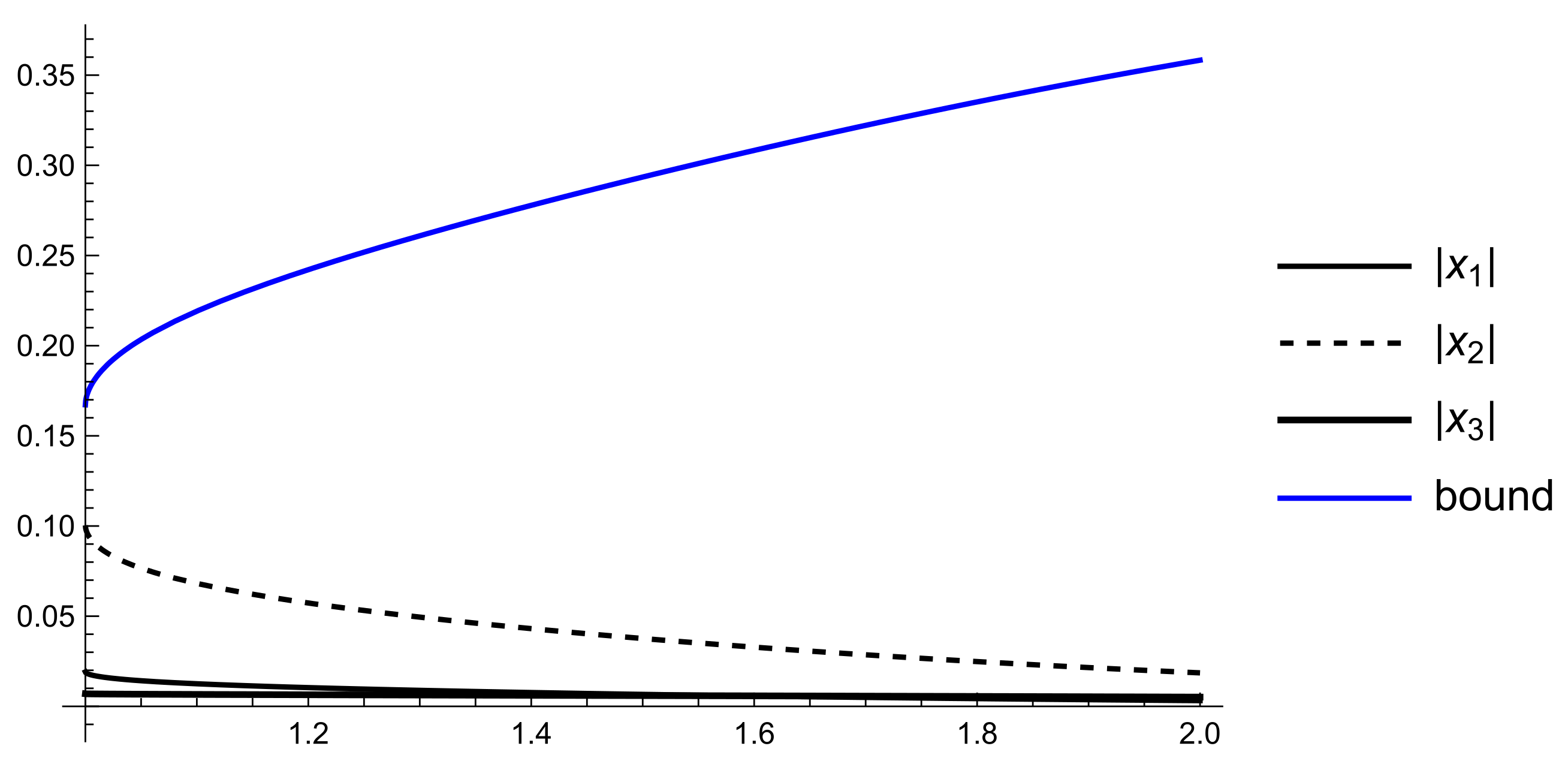
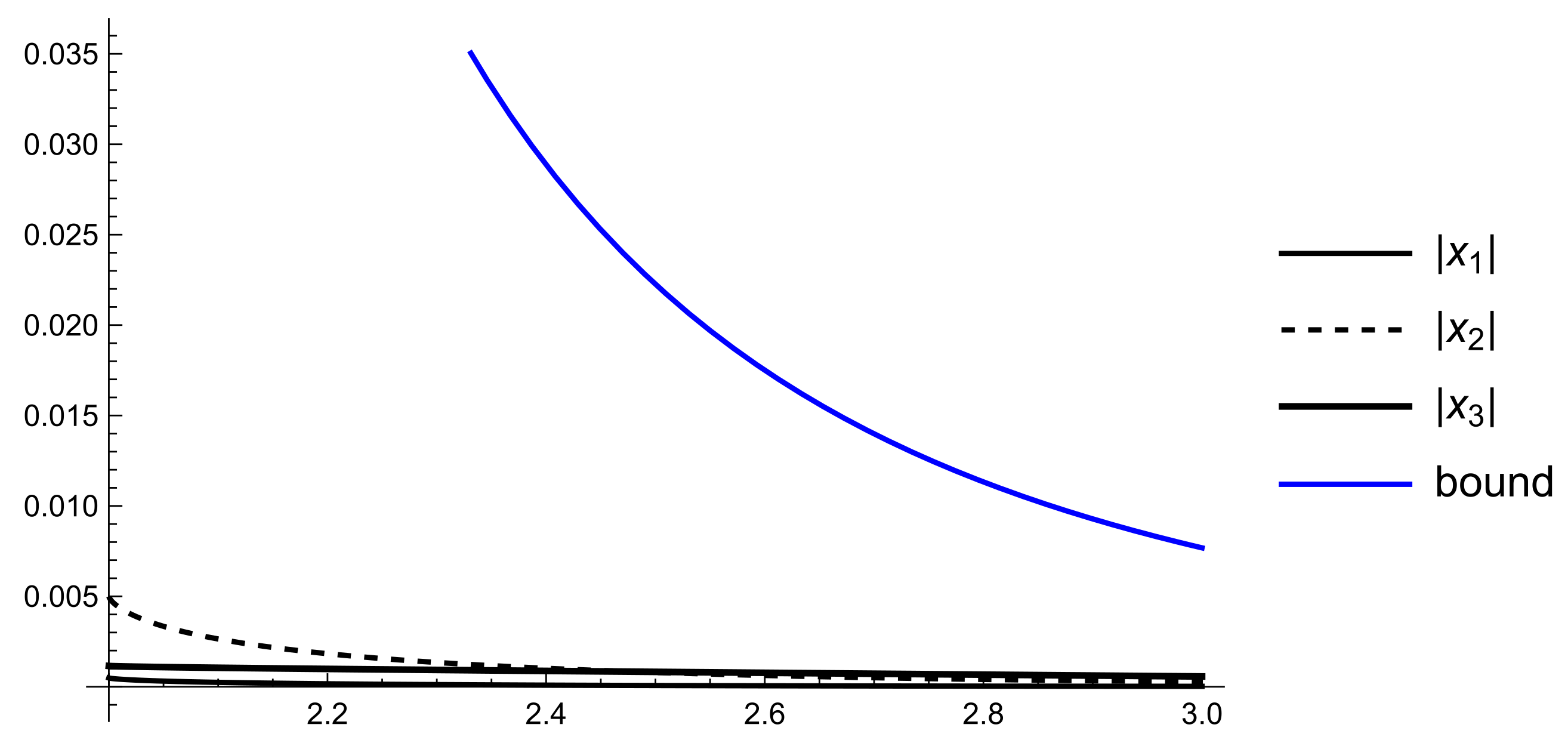
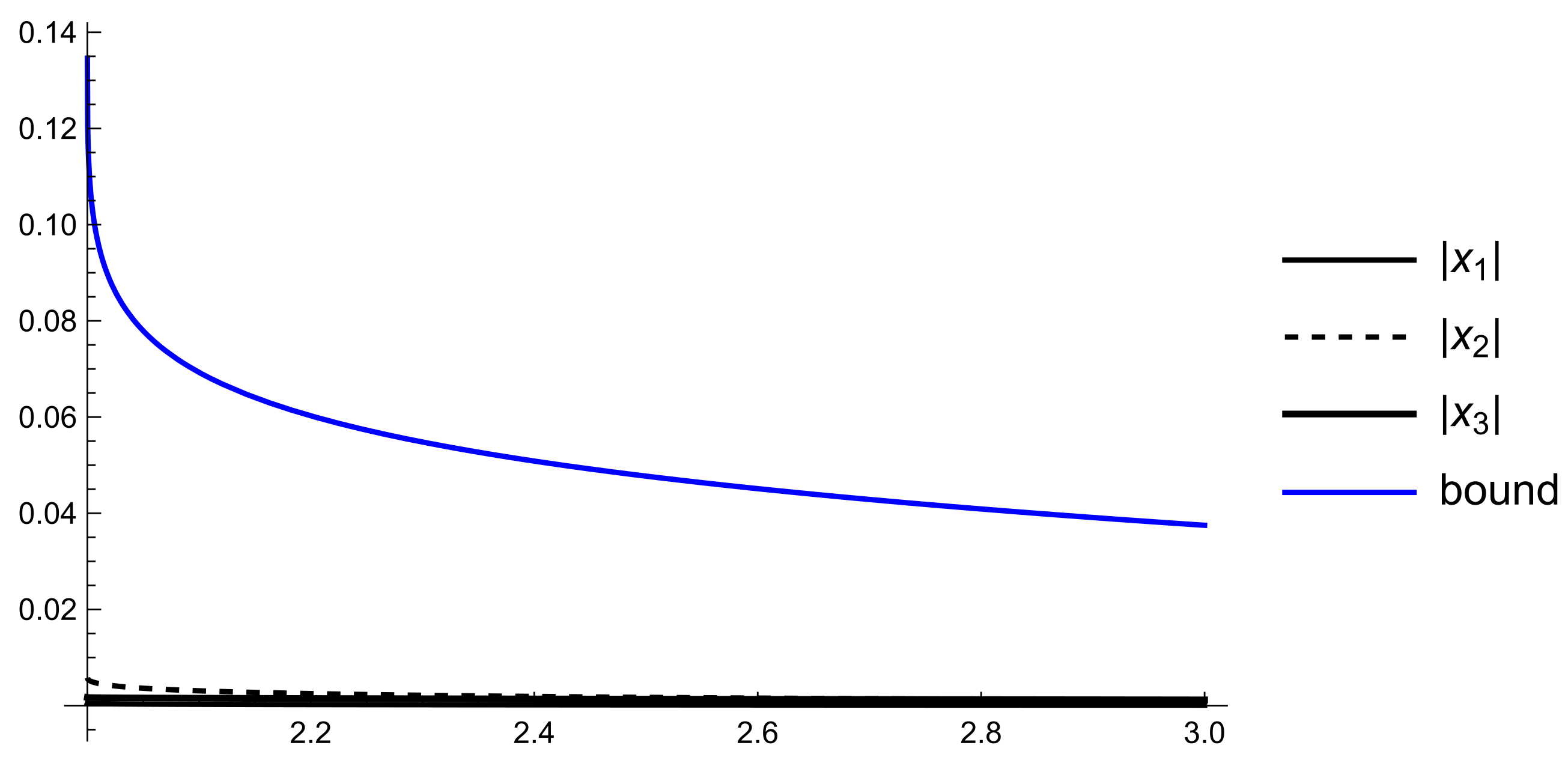
- We provide an example that shows the influence of the impulsive control on a system.
- -
- In Section 2, we give an overview of the Caputo fractional calculus with respect to another function. We give the basic results that will be used in subsequent parts of the paper. Also, we present the basic definitions and results for the variable-order Caputo fractional derivative and discuss its application for modeling multi-agent systems.
- -
- In Section 3, based on the main definitions from the previous section, we set up models for two basic cases: multi-agent systems both without a leader and with a leader. In the case without a leader, in the multi-agent system, we consider two different types of models: one with variable time order of the fractional derivative and one with a piecewise constant order. In the case of the presence of a leader in the system, we consider only a piecewise constant variable order of the corresponding fractional derivative.
- -
- In Section 4, we obtain our main results. In the case of a multi-agent system without a leader we define impulsive Mittag–Leffler stability and obtain sufficient conditions. In the case of the presence of a leader in the system, we study the leader-following consensus. All theoretical results are illustrated with examples.
- -
- To summarize, we finish the paper with a conclusion.
2. Preliminary Notes and Results
2.1. -Fractional Diffintegrals of Constant Order
2.2. -Fractional Diffintegrals of Variable Order
3. Statement of the Problem
3.1. Multi-Agent System without a Leader
3.1.1. Model of Fractional Derivative with Variable Order
3.1.2. Model of Fractional Derivative with Piecewise Constant Order
3.2. Multi-Agent System with a Leader
4. Main Results for the Models of Multi-Agent Systems with Impulsive Control Protocol
4.1. Multi-Agent System without a Leader
4.1.1. Model of Fractional Derivative with Variable Order
4.1.2. Model of Fractional Derivative with Piecewise Constant Order
4.2. Multi-Agent System with a Leader
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Song, C.; Cao, J. Consensus of fractional-order linear systems. In Proceedings of the 2013 9-th Asian Control Conference (ASCC), Istanbul, Turkey, 23–26 June 2013; pp. 1–4. [Google Scholar]
- Wang, F.; Yang, Y. On leaderless consensus of fractional-order nonlinear multi-agent systems via event-triggered control. Nonlinear Anal. Model. Control 2019, 24, 353–367. [Google Scholar] [CrossRef]
- Almeida, R.; Girejko, E.; Hristova, S.; Malinowska, A.B. Leader-following consensus for fractional mul-ti-agent systems. Adv. Differ. Equ. 2019, 2019, 301. [Google Scholar] [CrossRef]
- Ren, G.; Yu, Y.; Zhang, S. Leader-following consensus of fractional nonlinear multiagent systems. Math. Probl. Eng. 2015, 8, 919757. [Google Scholar] [CrossRef]
- Schmeidel, E. The existence of consensus of a leader-following problem with Caputo fractional derivative. Opusc. Math. 2019, 39, 77–89. [Google Scholar] [CrossRef]
- Yu, Z.; Jiang, H.; Hu, C. Leader-following consensus of fractional-order multi-agent systems under fixed topology. Neurocomputing 2015, 149, 613–620. [Google Scholar] [CrossRef]
- Parivallal, A.; Yun, S.; Jung, Y.M. Hybrid-Triggered Output Feedback Containment Control for Multi-Agent Systems with Missing Measurements. IEEE Trans. Signal Inf. Process. Netw. 2024, 10, 108–118. [Google Scholar] [CrossRef]
- Almeida, J.; Silvestre, C.; Pascoal, A.M. Continuous-time consensus with discrete-time communications. Syst. Control Lett. 2012, 61, 788–796. [Google Scholar] [CrossRef]
- Jiang, H.; Yu, J.; Zhou, C. Consensus of multi-agent linear dynamic systems via impulsive control protocols. Int. J. Syst. Sci. 2011, 42, 967–976. [Google Scholar] [CrossRef]
- Wang, F.; Yang, Y. Leader-following exponential consensus of fractional order nonlinear multi-agents system with hybrid time-varying delay: A heterogeneous impulsive method. Phys. A 2017, 482, 158–172. [Google Scholar] [CrossRef]
- Yu, Z.; Jiang, H.; Hu, C.; Yu, J. Necessary and sufficient condi tions for consensus of fractional-order multiagent systems via sampled-data control. IEEE Trans. Cybern. 2017, 47, 1892–1901. [Google Scholar] [CrossRef]
- Almeida, R.; Girejko, E.; Hristova, S.; Malinowska, A.B. On leader-following consensus in multi-agent systems with discrete updates at random times. Entropy 2020, 22, 650. [Google Scholar] [CrossRef] [PubMed]
- Bohner, M.; Hristova, S.; Malinowska, A.B.; Morgado, M.L.; Almeida, R. A generalized proportional Caputo fractional model of multi-agent linear dynamic systems via impulsive control protocol. Commun. Nonlinear Sci. Numer. Simul. 2022, 115, 106756. [Google Scholar] [CrossRef]
- Samko, S.G.; Ross, B. Integration and differentiation to a variable fractional order. Integral Transform. Spec. Funct. 1993, 1, 277–300. [Google Scholar] [CrossRef]
- Tavares, D.; Almeida, R.; Torres, D.F.M. Caputo derivatives of fractional variable order: Numerical approximations. Commun. Nonlinear Sci. Numer. Simul. 2016, 35, 69–87. [Google Scholar] [CrossRef]
- Coimbra, C.F.M. Mechanics with variable-order differential operators. Ann. Phys. 2003, 12, 692–703. [Google Scholar] [CrossRef]
- Sun, H.G.; Chen, W.; Chen, Y.Q. Variable-order fractional differential operators in anomalous diffusion modeling. Phys. A 2009, 388, 4586–4592. [Google Scholar] [CrossRef]
- Sun, H.G.; Chen, W.; Wei, H.; Chen, Y.Q. A comparative study of constant order and variable-order fractional models in characterizing memory property of systems. Eur. Phys. J. Spec. 2011, 193, 185–192. [Google Scholar] [CrossRef]
- Alsaade, F.W.; Al-zahrani, M.S.; Yao, Q.; Jahanshahi, H.A. Model-free finite-time control technique for synchronization of variable-order fractional Hopfield-like neural network. Fractal Fract. 2023, 7, 349. [Google Scholar] [CrossRef]
- Din, A.; Li, Y.; Yusuf, A.; Liu, J.; Aly, A.A. Impact of information intervention on stochastic hepatitis B model and its variable-order fractional network. Eur. Phys. J. Spec. Top. 2022, 231, 1859–1873. [Google Scholar] [CrossRef]
- Sun, H.G.; Chang, A.; Zhang, Y.; Chen, W. A review of variable order fractional differential equations: Mathematical foundations, physical models, numerical methods and applications. Fract. Calc. Appl. Anal. 2019, 22, 27–59. [Google Scholar] [CrossRef]
- Sabir, Z.; Raja, M.A.Z.; Nguyen, T.G.; Fathurrochman, I.; Sadat, R.; Ali, M.R. Applications of neural networks for the novel designed of nonlinear fractional seventh order singular system. Eur. Phys. J. Spéc. Top. 2022, 231, 1831–1845. [Google Scholar] [CrossRef]
- Yousefpour, A.; Jahanshahi, H.; Castillo, O. Application of variable-order fractional calculus in neural net-works: Where do we stand? Eur. Phys. J. Spec. Top. 2022, 231, 1753–1756. [Google Scholar] [CrossRef]
- Almeida, R.; Malinowska, A.; Monteiro, M.T. Fractional differential equations with a Caputo derivative with respect to a kernel function and their applications. Math. Meth. Appl. Sci. 2018, 41, 336–352. [Google Scholar] [CrossRef]
- Kucche, K.D.; Mali, A.D.; Sousa, J.V. Theory of nonlinear Ψ-Hilfer fractional differential equations. arXiv 2018, arXiv:1808.01608. [Google Scholar]
- Donganont, M.; Liu, X. Scaled consensus problems of multi agent systems via impulsive protocols. Appl. Math. Modell. 2023, 116, 532–546. [Google Scholar] [CrossRef]
- Wei, L.; Chen, W.-H.; Luo, S.; Huang, G. Impulsive average-consensus of multi-agent systems with time-delays. J. Frankl. Inst. 2022, 359, 1544–1568. [Google Scholar] [CrossRef]
- Zhang, J.; Peng, S. Exponential Consensus of Multi-Agent Systems under Event-Triggered Impulsive Control with Actuation Delays. Entropy 2023, 25, 877. [Google Scholar] [CrossRef]
- Almeida, R. Functional Differential Equations Involving the Ψ-Caputo Fractional Derivative. Fractal Fract. 2020, 4, 29. [Google Scholar] [CrossRef]
- Almeida, R. A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 460–481. [Google Scholar] [CrossRef]
- Miller, K.S.; Bertram, R. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley & Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Zhang, S.; Wang, J.; Hu, L. On definition of solution of initial value problem for fractional differential equation of variable order. AIMS Math. 2021, 6, 6845–6867. [Google Scholar] [CrossRef]
- Samko, S.G. Fractional integration and differentiation of variable order. Anal. Math. 1995, 21, 213–236. [Google Scholar] [CrossRef]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives (Theory and Applications); Gordon & Breach Sci. Publ.: New York, NY, USA, 1992. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agarwal, R.P.; Hristova, S.; O’Regan, D. Impulsive Control of Variable Fractional-Order Multi-Agent Systems. Fractal Fract. 2024, 8, 259. https://doi.org/10.3390/fractalfract8050259
Agarwal RP, Hristova S, O’Regan D. Impulsive Control of Variable Fractional-Order Multi-Agent Systems. Fractal and Fractional. 2024; 8(5):259. https://doi.org/10.3390/fractalfract8050259
Chicago/Turabian StyleAgarwal, Ravi P., Snezhana Hristova, and Donal O’Regan. 2024. "Impulsive Control of Variable Fractional-Order Multi-Agent Systems" Fractal and Fractional 8, no. 5: 259. https://doi.org/10.3390/fractalfract8050259
APA StyleAgarwal, R. P., Hristova, S., & O’Regan, D. (2024). Impulsive Control of Variable Fractional-Order Multi-Agent Systems. Fractal and Fractional, 8(5), 259. https://doi.org/10.3390/fractalfract8050259