A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis
Abstract
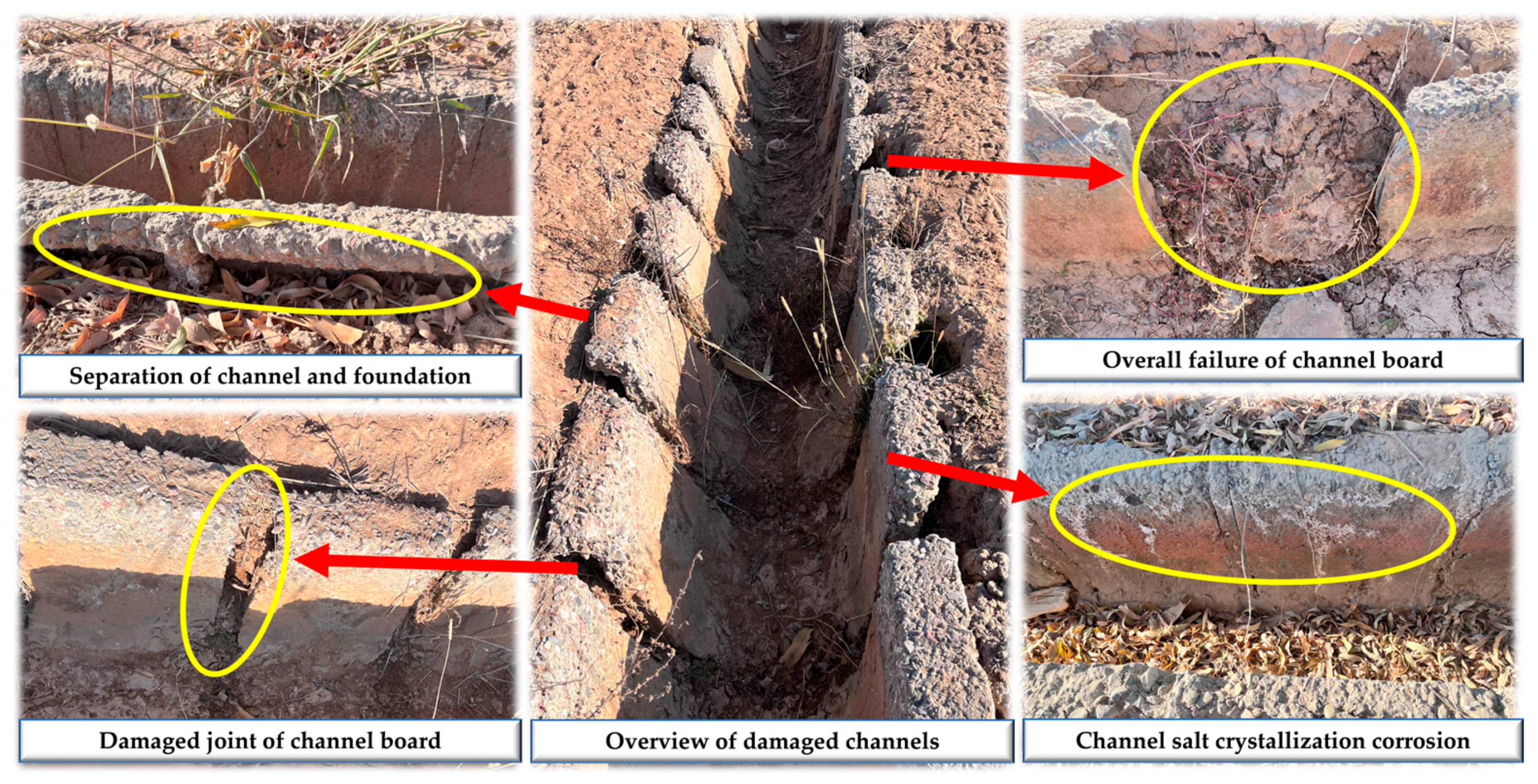
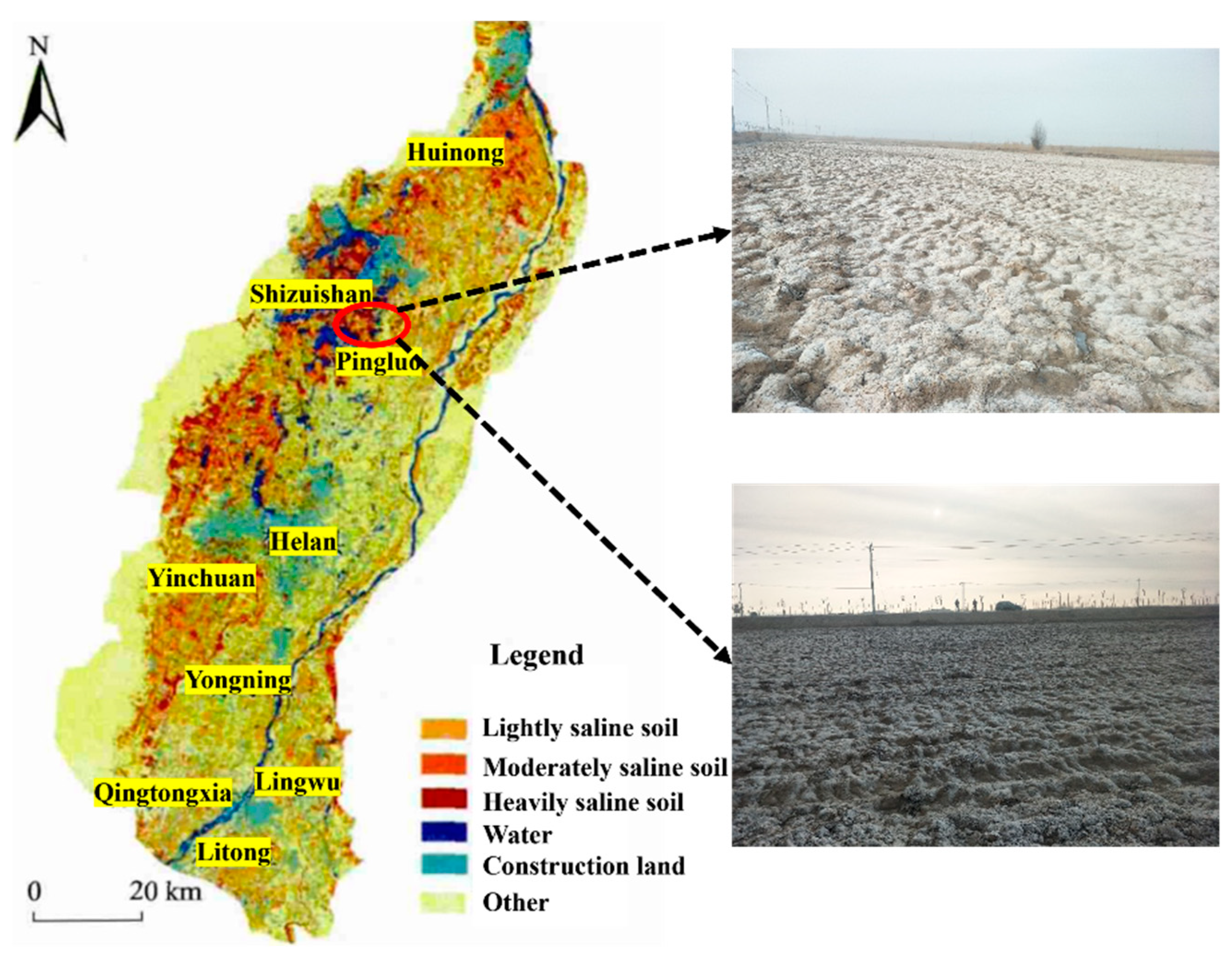
1. Introduction
2. Introduction to the Test
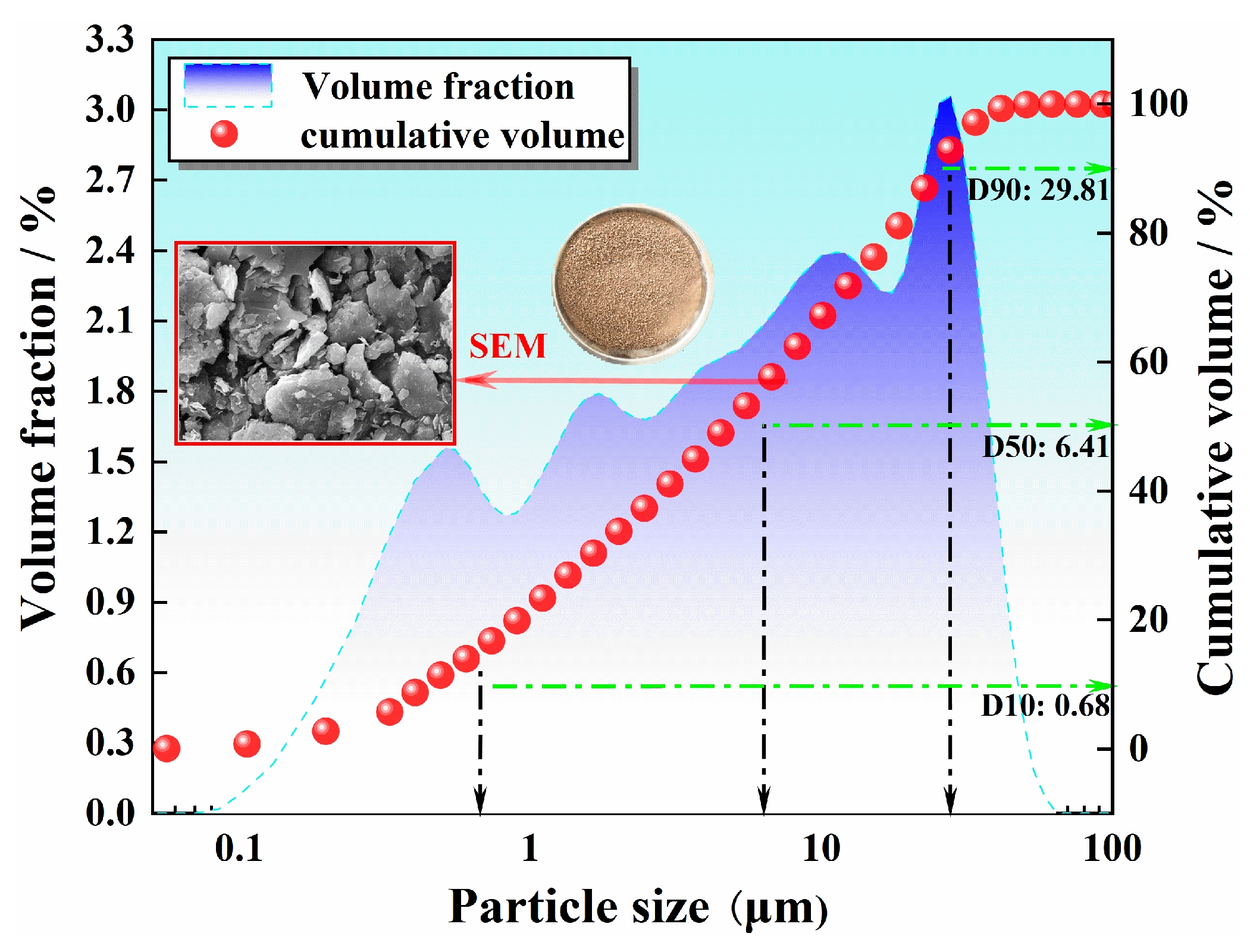
2.1. Physical and Chemical Indicators of Test Materials
2.2. Triaxial Test
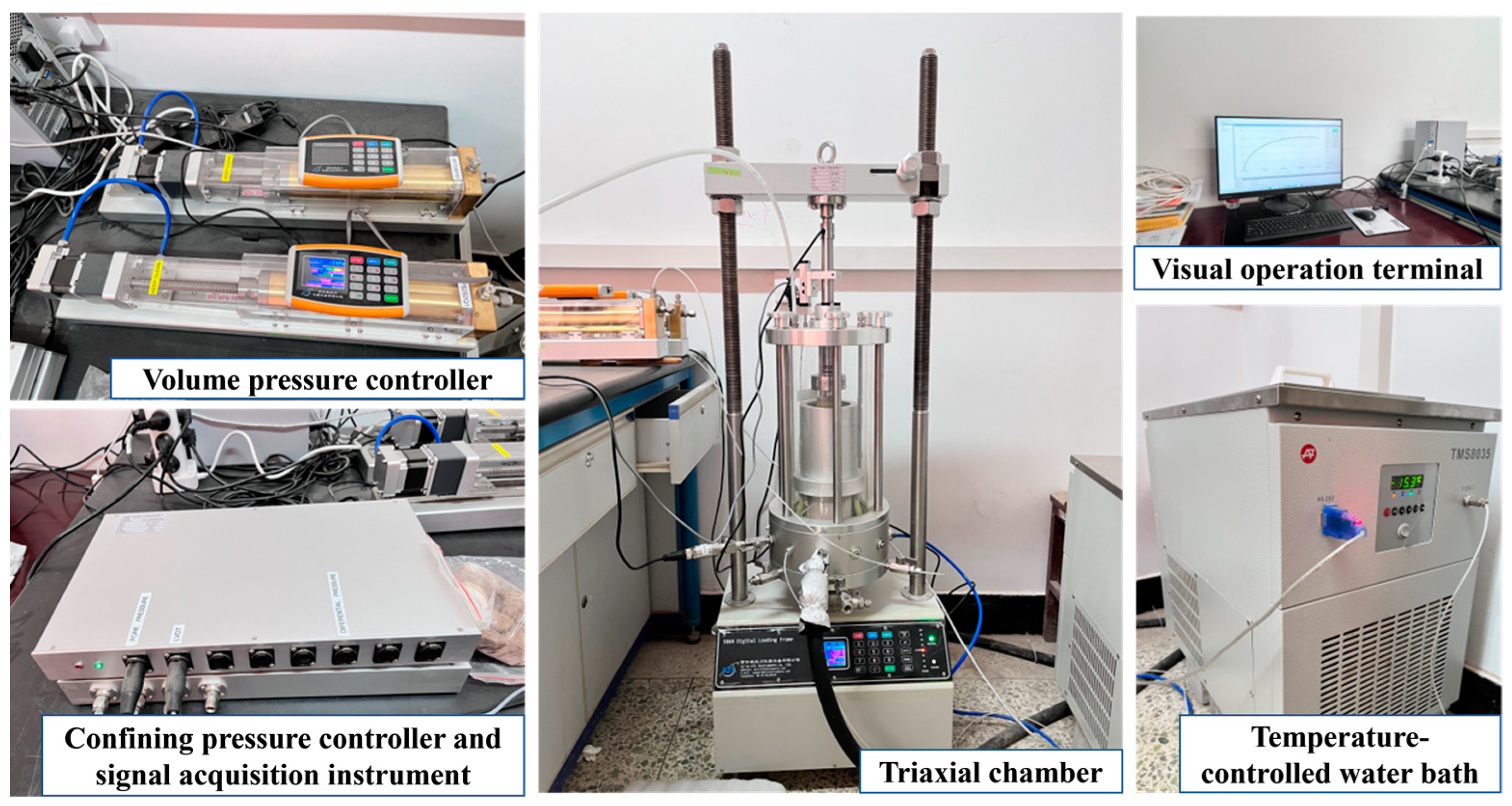
2.2.1. Test Equipment
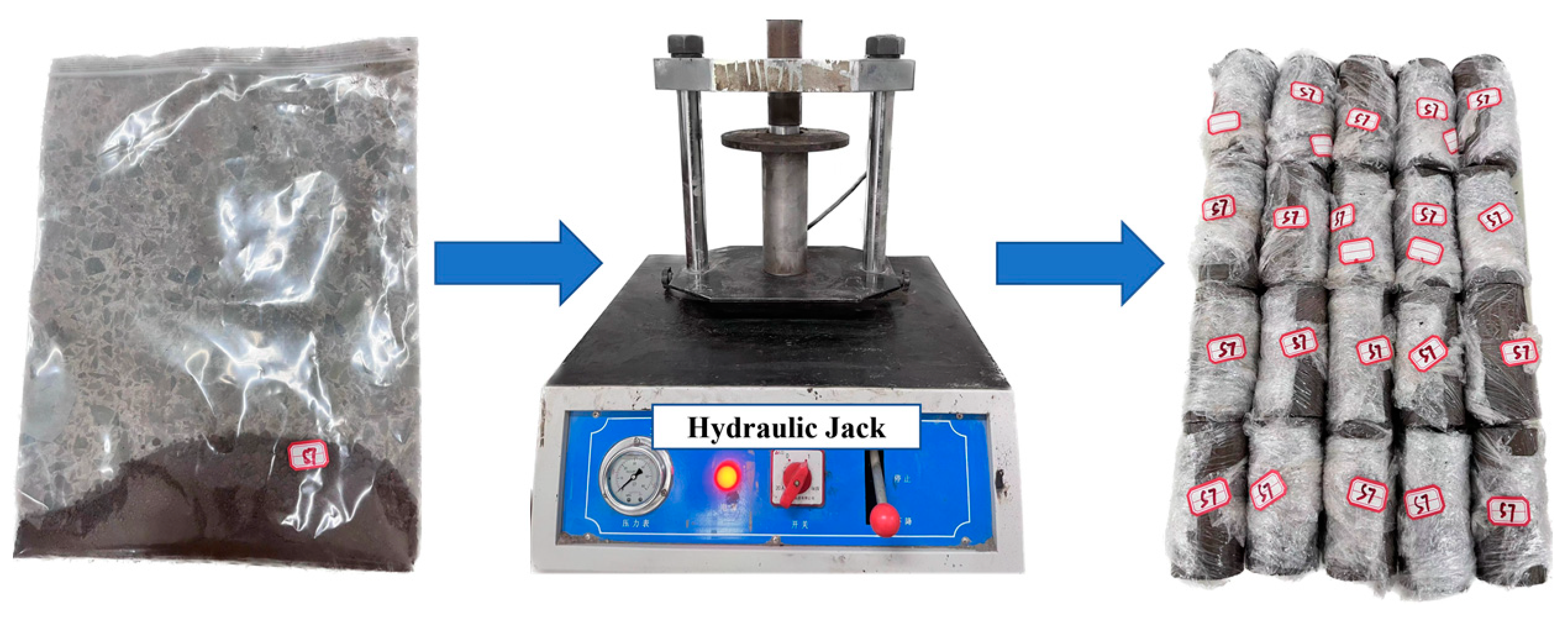
2.2.2. Sample Preparation
2.2.3. Test Program
2.3. MIP Test
2.3.1. Test Equipment
2.3.2. Test Program
3. Binary Medium Model of Frozen Saline Soil
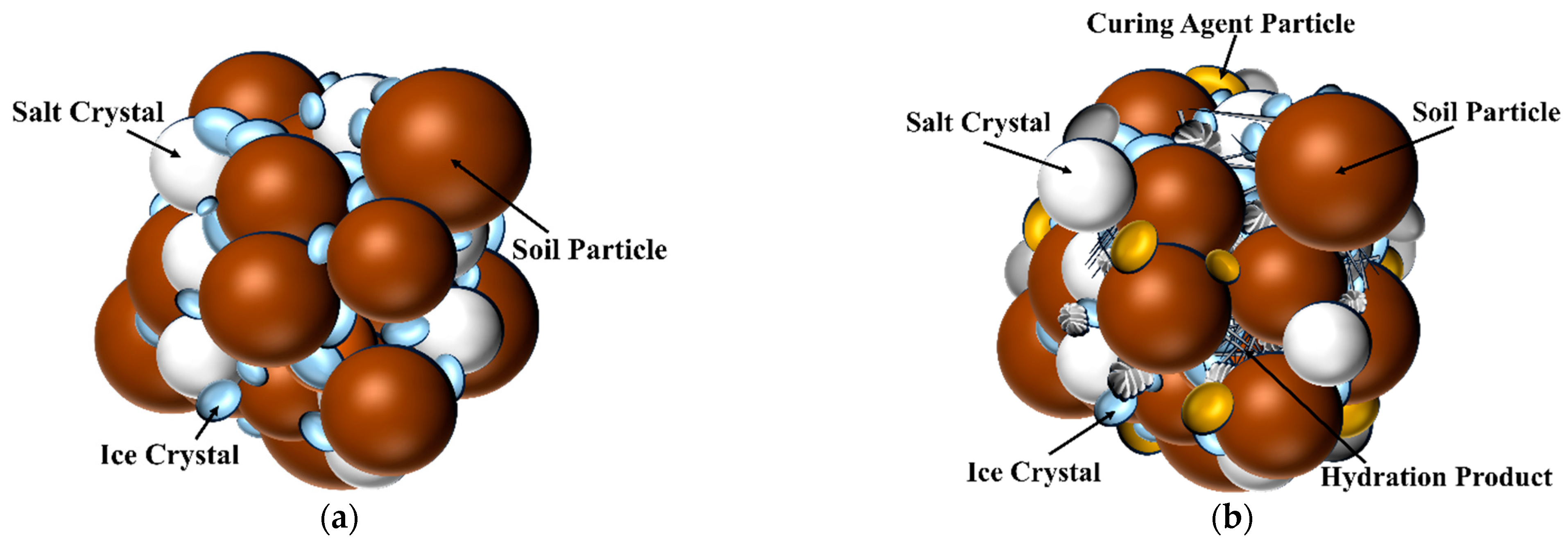
3.1. Structural Model of Frozen Saline Soil
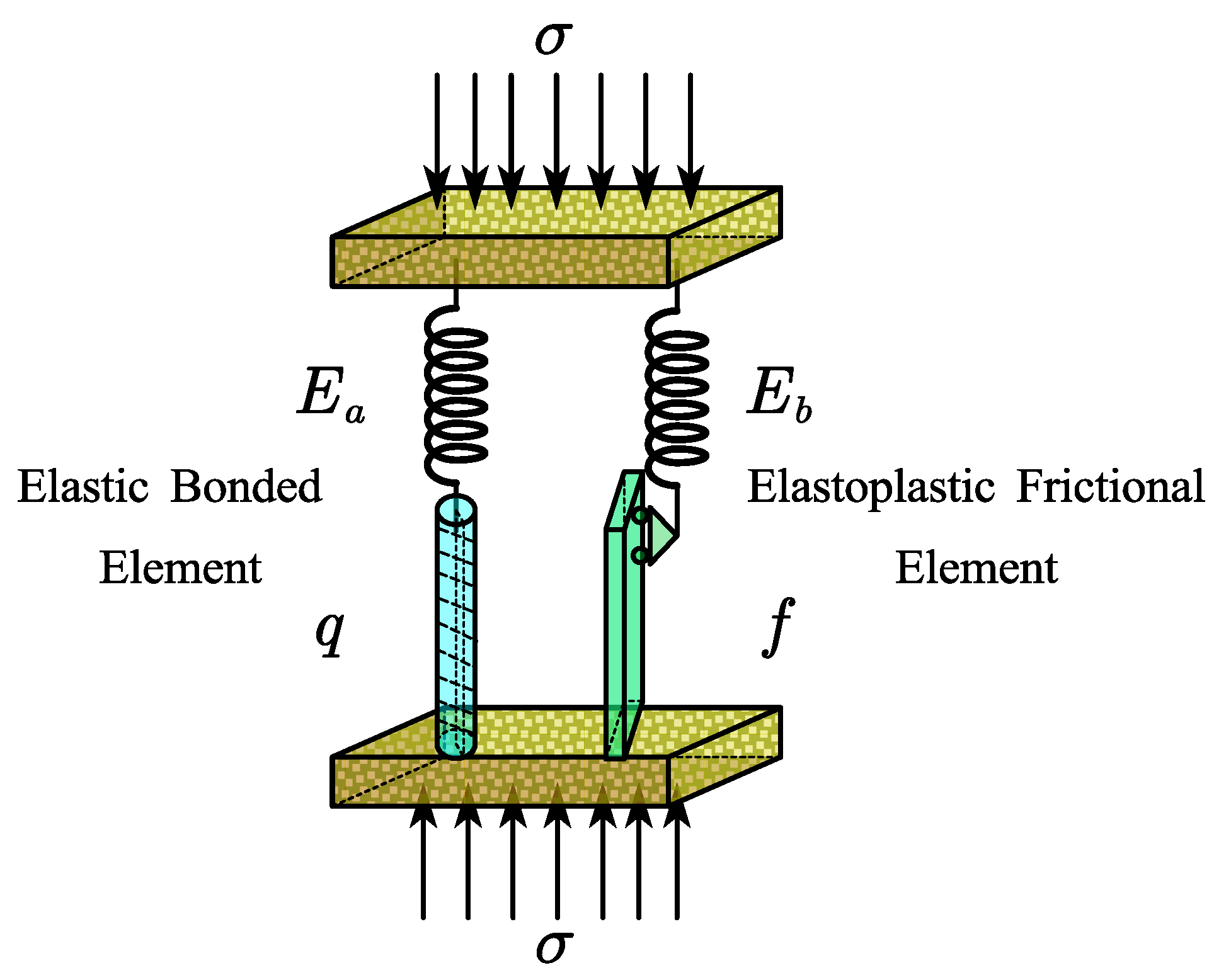
3.2. Binary Medium Modeling of Frozen Saline Soils
3.3. Parameter Determination
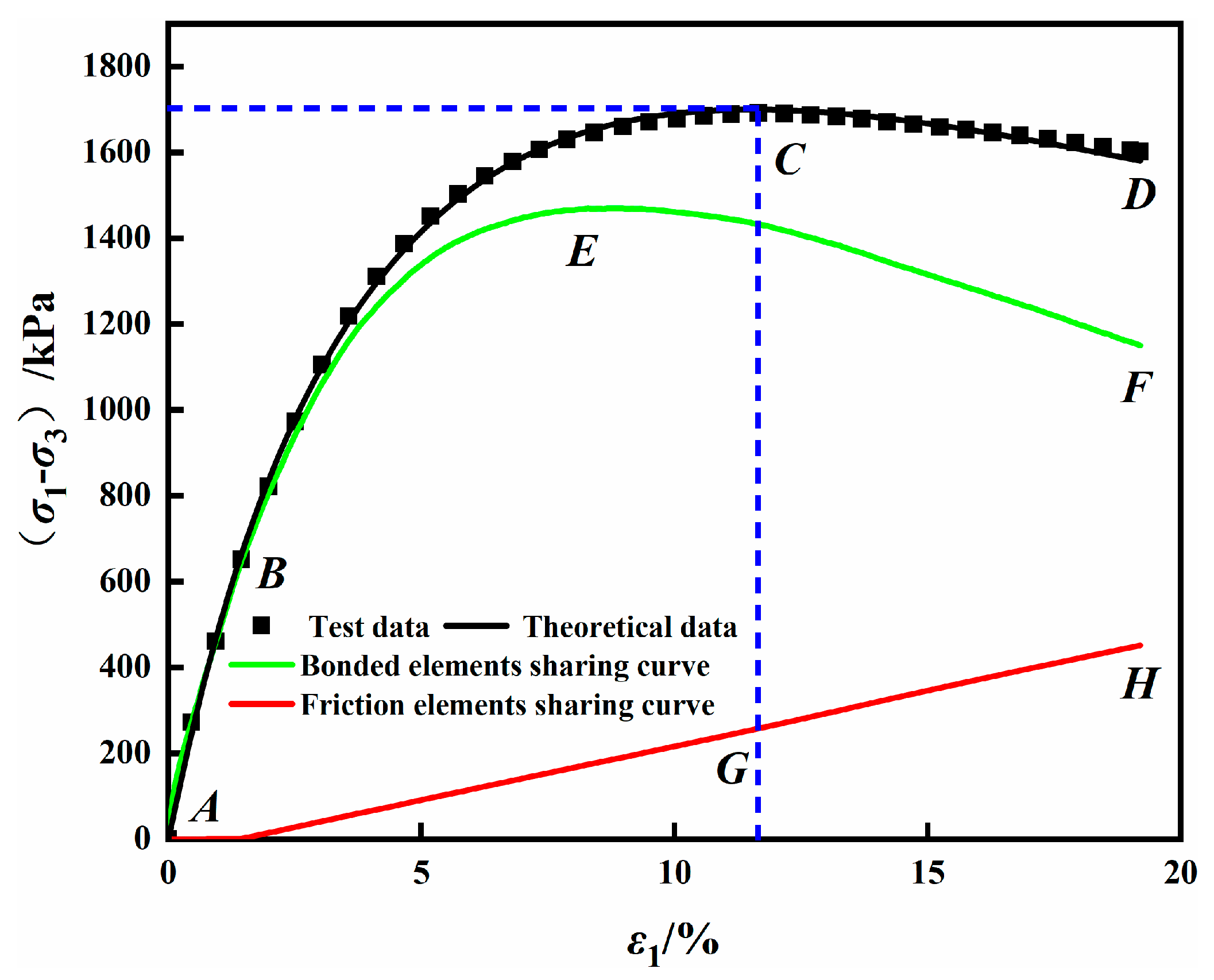
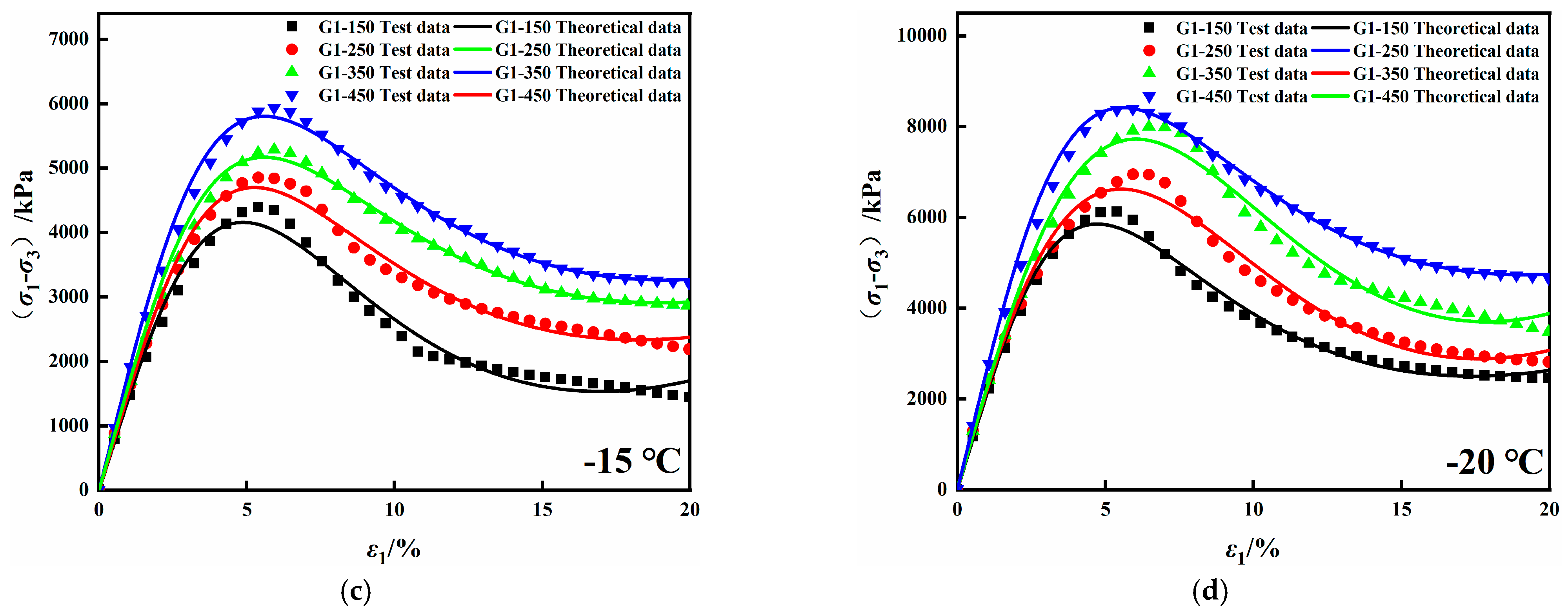
3.4. Model Result Verification
4. Analysis of Triaxial Test Results
4.1. Failure Mode Analysis
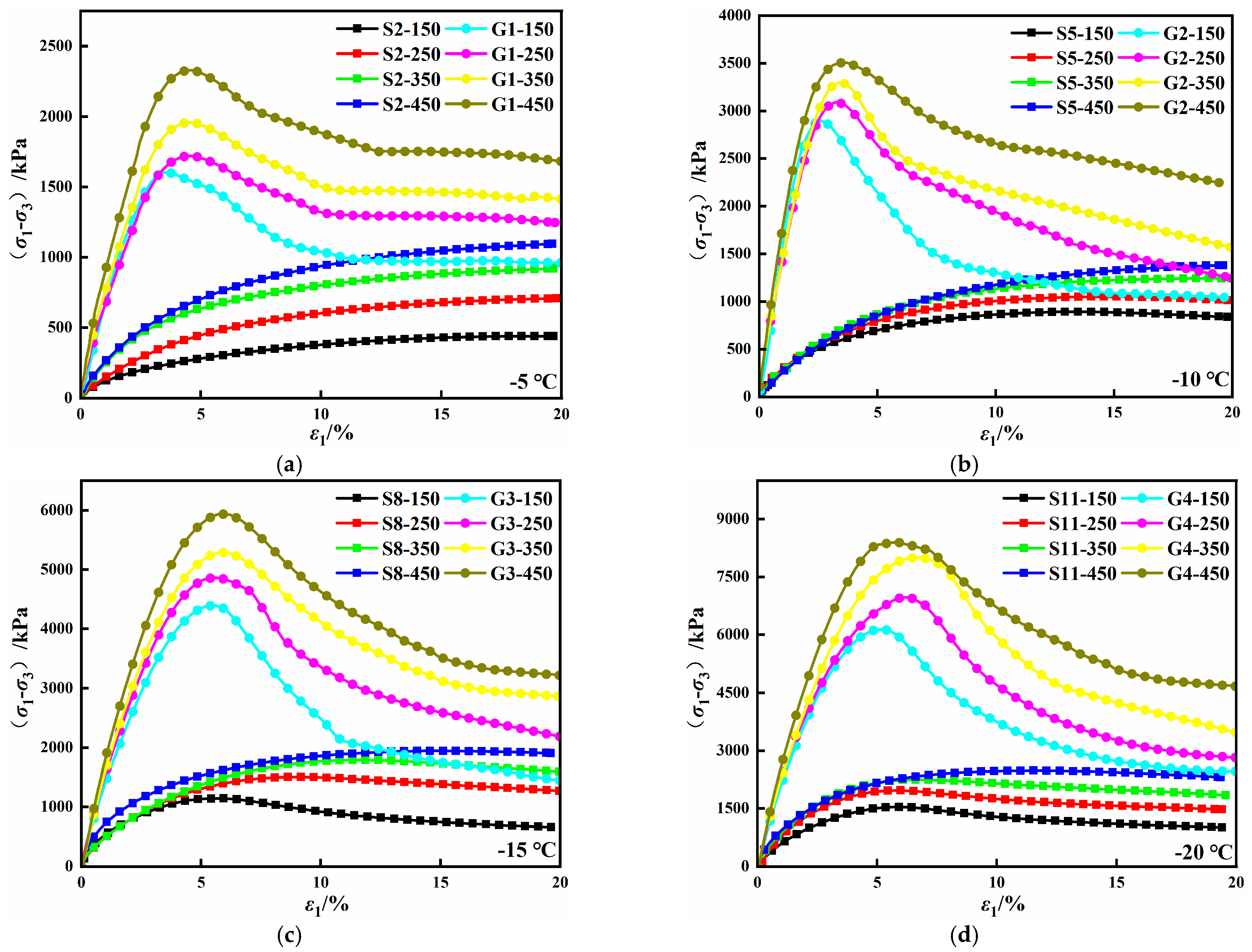
4.2. Stress–Strain Curve Analysis
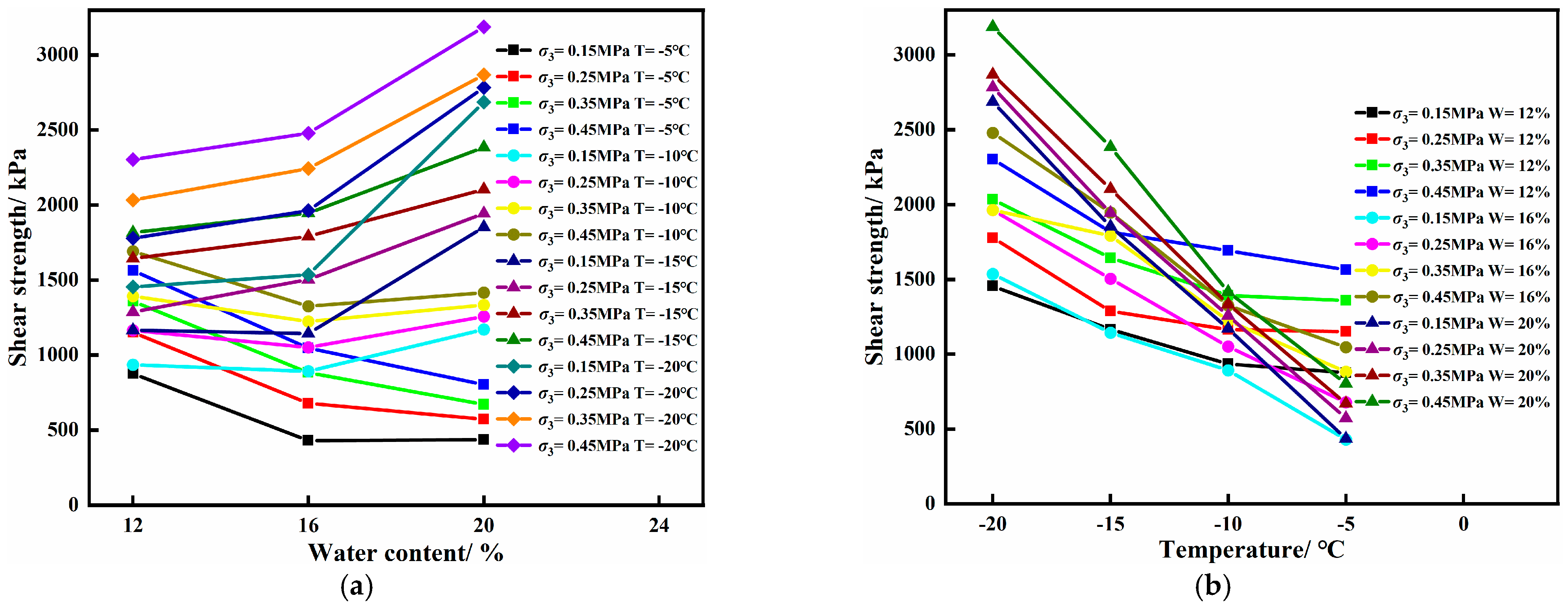
4.3. Shear Strength Index
5. Pore Structure Analysis of Solidified Saline Soil
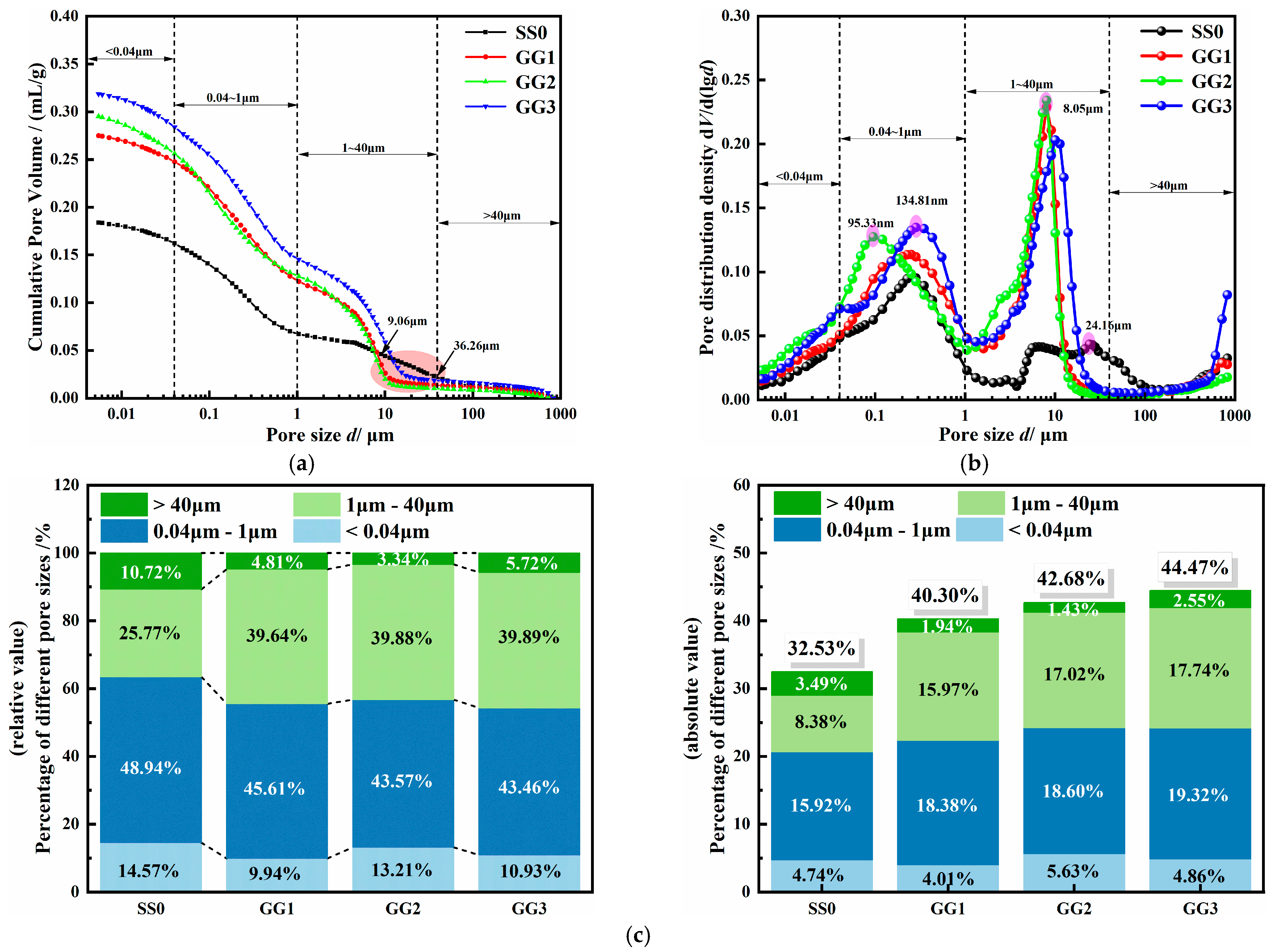
5.1. Distribution of Pore Structure
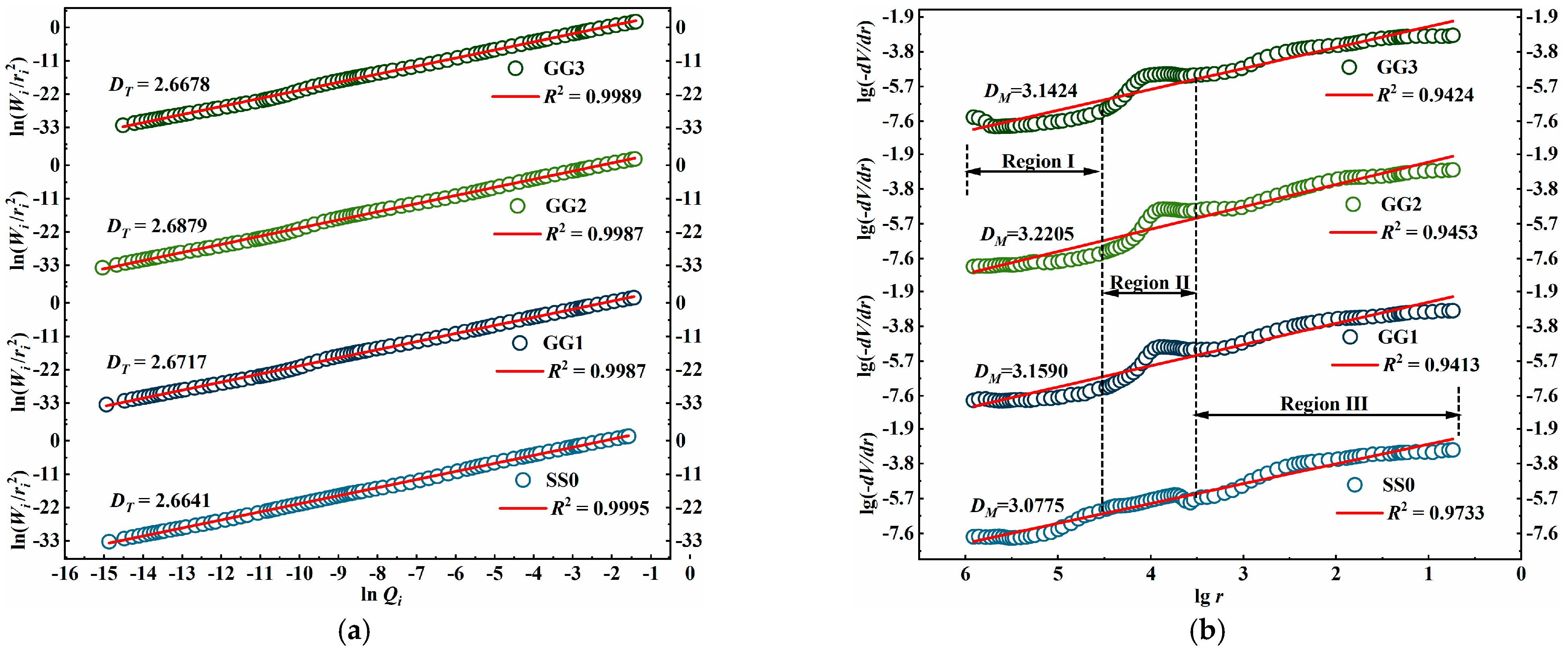
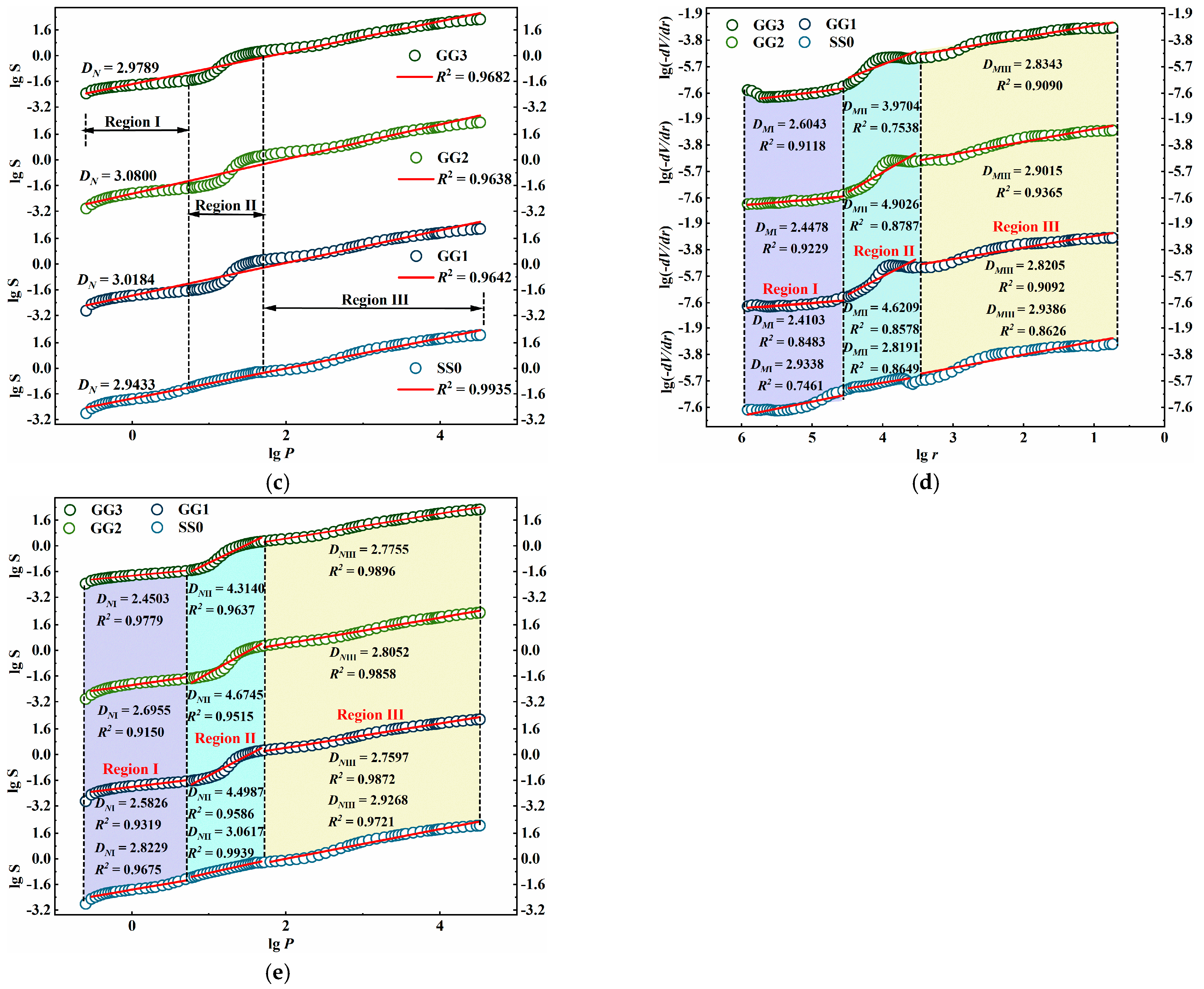
5.2. Analysis of Pore Fractal Characteristics
6. Conclusions
- (1)
- The empirical values derived from the binary medium constitutive model align closely with those obtained from triaxial tests under a variety of conditions. This correlation suggests that the model is effective not only in simulating the stress–strain relationship of saline soil under negative temperature conditions but is also capable of modeling the behavior of solidified saline soil with significant bonding. The model demonstrates robust applicability across both the pre-solidification and post-solidification stages of frozen saline soil.
- (2)
- As the influencing factors vary, the stress–strain curves of both frozen saline soil and frozen solidified saline soil present distinct characteristics. These can be categorized into strain-hardening and strain-softening types, each exhibiting linear elastic, elastoplastic, and corresponding hardening or softening phases. These phases are aptly characterized by the binary medium constitutive relationship theory.
- (3)
- The shear strength and failure patterns of both frozen saline soil and solidified frozen saline soil are influenced by the temperature, water content, and confining pressure. A critical temperature threshold at −9.82 °C marks a transitional point where the shear strength of saline soil shows divergent trends with temperature variations on either side of this threshold. Water content plays a critical role in determining the shear strength of saline soil under different negative temperature conditions, with a direct correlation between the moisture level and the rate of change in shear strength observed at a constant temperature.
- (4)
- The pore structures within saline soil and solidified saline soil are characterized by pronounced fractal dimensions. According to the thermodynamic model, Menger sponge model, and Neimark model, the fractal dimension D is consistently higher in solidified saline soil compared to that in non-solidified saline soil. This complexity in pore distribution signals a marked enhancement in soil solidification efficacy.
- (5)
- Multiple fractal dimensions are evident in both saline and solidified saline soils, with size-dependent variations observed in the context of the Menger sponge and Neimark models. The study therefore segments these soils into subregions. The lower values of DMI, DNI, DMIII, and DNIII in solidified saline soil, compared to those in non-solidified saline soil in Regions I and III, are attributed to the densification effects of hydration products and the presence of unhydrated solidifying agents. Conversely, in Region II, both DMII and DNII of solidified saline soil exhibit a ‘non-physical’ manifestation, a phenomenon largely ascribed to the formation of an extensive network of inkpot-type pores created by the aggregation of soil particles through hydration products.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, W.; Wang, D.T. Studies on frozen soil mechanics in China in past 50 years and their prospect. Chin. J. Geotech. Eng. 2012, 34, 625–640. [Google Scholar]
- Lai, Y.M.; Zhang, M.Y.; Li, S.Y. Theory and Application of Cold Regions; Science Press: Beijing, China, 2009; pp. 13–23. [Google Scholar]
- Li, J.G.; Pu, L.J.; Han, M.F.; Zhu, M.; Zhang, R.S.; Xiang, Y.Z. Soil salinization research in China: Advances and prospects. J. Geogr. Sci. 2014, 24, 943–960. [Google Scholar] [CrossRef]
- Li, H.B. Freeze-Thaw Response Mechanism of Integral Channel Lining and Mode Research of Saline Soil Foundation. Ph.D. Thesis, Ningxia University, Yinchuan, China, 2022. [Google Scholar]
- Tsytovich, N.A. The Mechanics of Frozen Ground; Science Press: Beijing, China, 2009; pp. 15–20. [Google Scholar]
- Chamberlain, E.; Groves, C.; Perham, R. Discussion: The mechanical behaviour of frozen earth materials under high pressure triaxial test conditions. Geotechnique 1973, 23, 136–137. [Google Scholar] [CrossRef]
- Wei, Z.H.; Wang, M.S.; Zhang, D.L. A Research on the Full State Function Constitutive Relation Model. Springer Ser. Geomech. Geoeng. 2012, 7, 654–660. [Google Scholar]
- Zhu, Y.L.; Zhang, J.Y.; Peng, W.W.; Shen, Z.Y.; Miao, L.N. Constitutive Relations of Frozen Soil in Uniaxial Compression. J. Glaciol. Geocryol. 1992, 34, 210–217. [Google Scholar]
- Lai, Y.M.; Li, S.Y.; Qi, J.L.; Gao, Z.H.; Chang, X.X. Strength distributions of warm frozen clay and its stochastic damage constitutive model. Cold Reg. Sci. Technol. 2008, 53, 200–215. [Google Scholar] [CrossRef]
- Lai, Y.M.; Li, J.B.; Li, Q.Z. Study on damage statistical constitutive model and stochastic simulation for warm ice-rich frozen silt. Cold Reg. Sci. Technol. 2012, 71, 102–110. [Google Scholar] [CrossRef]
- Chamberlain, E.J. Shear strength anisotropy in frozen saline and freshwater soils. In Proceedings of the 4th International Symposium on Grounding Freezing, Sapporo, Japan, 5–7 August 1985; pp. 189–194. [Google Scholar]
- Chang, D.; Lai, Y.M.; Zhang, M.Y. A meso-macroscopic constitutive model of frozen saline sandy soil based on homogenization theory. Int. J. Mech. Sci. 2019, 159, 246–259. [Google Scholar] [CrossRef]
- Lai, Y.M.; Jin, L.; Chang, X.X. Yield criterion and elasto-plastic damage constitutive model for frozen sandy soil. Int. J. Plast. 2009, 25, 1177–1205. [Google Scholar] [CrossRef]
- Lai, Y.M.; Liao, M.K.; Hu, K. A constitutive model of frozen saline sandy soil based on energy dissipation theory. Int. J. Plast. 2016, 78, 84–113. [Google Scholar] [CrossRef]
- Wang, P.; Liu, E.L.; Zhang, D.; Liu, X.Y.; Zhang, G.; Song, B.T. An elastoplastic binary medium constitutive model for saturated frozen soils. Cold Reg. Sci. Technol. 2020, 174, 103055. [Google Scholar] [CrossRef]
- Wang, D.; Liu, E.L.; Zhang, D.; Yue, P.; Wang, P.; Kang, J.; Yu, Q.H. An elasto-plastic constitutive model for frozen soil subjected to cyclic loading. Cold Reg. Sci. Technol. 2021, 189, 103341. [Google Scholar] [CrossRef]
- Zhang, D.; Liu, E.L. Binary-medium-based constitutive model of frozen soils subjected to triaxial loading. Results Phys. 2019, 12, 1999–2008. [Google Scholar] [CrossRef]
- Wang, D.; Liu, E.L.; Huang, J. Elastoplastic constitutive model for frozen sands based on framework of homogenization theory. Acta Geotech. 2020, 15, 1831–1845. [Google Scholar]
- Zhang, S.M.; Jiang, G.L.; Ye, X.W.; Cai, J.F.; Yuan, S.Y.; Luo, B. A constitutive model for frozen silty sand based on binary medium model simplified by breakage parameter. Rock Soil Mech. 2022, 43, 1854–1864. [Google Scholar]
- Shen, Z.J. Breakage mechanics and doublemedium model for geological materials. Hydro-Sci. Eng. 2002, 4, 1–6. [Google Scholar]
- Shen, Z.J. Breakage mechanics for geological materials: An ideal brittle-elasticplastic model. Chin. J. Geotech. Eng. 2003, 25, 253–257. [Google Scholar]
- Liu, E.L. Research on Breakage Mechanism of Structural Blocks and Binary Medium Model for Geomaterials. Ph.D. Thesis, Tsinghua University, Beijing, China, 2005. [Google Scholar]
- Liu, E.L.; Tan, Y.L.; Chen, S.S.; Li, G.Y. Investigation on critical state of rockfill materials. J. Hydraul. Eng. 2012, 43, 505–511+519. [Google Scholar]
- Liu, E.L.; Huang, R.Q.; He, S.M. Modeling the deformation properties of rock samples by binary medium model. J. Hydraul. Eng. 2012, 43, 1237–1245. [Google Scholar]
- Yu, D.; Liu, E.L.; Sun, P.; Xiang, B.; Zheng, Q.S. Mechanical properties and binary-medium constitutive model for semi-through jointed mudstone samples. Int. J. Rock Mech. Min. 2020, 132, 104376. [Google Scholar] [CrossRef]
- Chen, Y.B.; Liu, E.L.; Yu, Y.Y.; Luo, H.H.; Chen, P.S. A binary-medium-based constitutive model for porous rocks. Int. J. Rock Mech. Min. 2023, 164, 105345. [Google Scholar] [CrossRef]
- Li, H.R.; Hu, Z.Q.; Feng, F.; Liu, Y. Application of structural loess binary-medium model to localization shear band. Rock Soil Mech. 2012, 33, 2803–2810. [Google Scholar]
- Zhang, Y.; Chen, C.H.; Gao, S.F.; Guo, S.Q.; Li, N.; Hu, Z.Q.; Yang, S.S. A novel binary-medium breakage model for loess in an acidic aqueous environment. Bull. Assoc. Int. Geol. Ing. Environ. 2023, 82, 1–13. [Google Scholar] [CrossRef]
- Lu, Q.Z.; Peng, J.B.; Wang, S.L.; Zhou, B.; Wang, Y. Double-parameter binary-medium model for fissured loess. Chin. J. Geotech. Eng. 2012, 34, 893–898. [Google Scholar]
- Fan, W.; Deng, L.S.; Yuan, W.N. Double parameter binary-medium model of fissured loess. Eng. Geol. 2018, 236, 22–28. [Google Scholar] [CrossRef]
- Yan, F.R.; Fan, W.; He, T.Y. Study on Binary-Medium Model of Fissured Loess. Appl. Mech. Mater. 2013, 256, 240–244. [Google Scholar] [CrossRef]
- Li, H.R.; Feng, F.; Wang, Q. Application of Structural Loess Binary-Medium Mode in Localization Shear Band. Appl. Mech. Mater. 2012, 204, 825–832. [Google Scholar] [CrossRef]
- Wang, D.; Liu, E.L.; Yang, C.S.; Wang, P.; Song, B.T. Micromechanics-based binary-medium constitutive model for frozen soil considering the influence of coarse-grained contents and freeze–thaw cycles. Acta Geotech. 2023, 18, 1–20. [Google Scholar] [CrossRef]
- Liu, Y.N.; Liu, E.L.; Li, X.; Chen, Y.B.; Zhang, H.R.; Li, H.M. Binary-medium constitutive model for freeze-thaw tailings subjected to cyclic triaxial loading. Cold Reg. Sci. Technol. 2023, 213, 103927. [Google Scholar] [CrossRef]
- Pedarla, A.; Aravind, A.; Hoyos, L.; Chittoori, B. Evaluation of swell behavior of expansive clays from internal specific surface and pore size distribution. J. Geotech. Geoenviron. Eng. 2016, 142, 1943–5605. [Google Scholar] [CrossRef]
- Zhang, B.Q.; Li, S.F. Determination of the surface fractal dimension for porous media by mercury porosimetry. Ind. Eng. Chem. Res. 1995, 34, 1383–1386. [Google Scholar] [CrossRef]
- Sun, H.Q.; Mašín, D.; Najser, J.; Neděla, V.; Navrátilová, E. Fractal characteristics of pore structure of compacted bentonite studied by ESEM and MIP methods. Acta Geotech. 2019, 15, 1655–1671. [Google Scholar] [CrossRef]
- Chen, J.Y.; Zhao, H.; Zhao, M.H.; Peng, W.Z.; Hou, J.C. Prediction of permeability for fully weathered granite amended with fly ash by fractal dimensions. J. Sustain. Cem. Based Mater. 2023, 12, 259–270. [Google Scholar] [CrossRef]
- Gao, Y.; Jiang, J.; De Schutter, G.; Ye, G.; Sun, W. Fractal and multifractal analysis on pore structure in cement paste. Constr. Build. Mater. 2014, 69, 253–261. [Google Scholar] [CrossRef]
- Yu, L.H.; Ou, H.; Duan, Q.P. Fractal dimension of perlite doped cement stone pores and its relationship with pore structure and strength. J. Mater. Sci. Eng. 2007, 25, 201–205. [Google Scholar]
- Wei, J.X.; Yu, Q.J.; Zeng, X.X.; Bai, R.Y. Study of fractal dimension of pore structure in concrete. J. South China Univ. Technol. 2007, 35, 121–124. [Google Scholar]
- Zhang, R.Q.; Qiao, Y.X.; Xue, J.N. Spatial Relationship Analysis Between the Soil Salinization and Land Use Intensity in Yinchuan Plain. J. Geo-inf. Sci. 2015, 17, 598–606. [Google Scholar]
- GB/T 50123-2019; Standard for Geotechnical Test Methods. Ministry of Water Resources, People’s Republic of China: Beijing, China, 2019.
- GB 51144-2015; Code for Geotechnical Engineering Investigation of Coal Industrial Mine Construction. China Coal Construction Association: Beijing, China, 2015.
- Li, G.X. Advanced Soil Mechanics; Tsinghua University Press: Beijing, China, 2016; pp. 58–69. [Google Scholar]
- Zhang, D.; Liu, E.L.; Liu, X.Y.; Song, B.T. Investigation on binary medium model taking frozen silt soils under −6 °C for example. Chin. J. Geotech. Eng. 2018, 40, 82–90. [Google Scholar]
- Shen, Z.J.; Liu, E.L.; Chen, T.L. Generalized stress-strain relationship of binary medium model for geological materials. Chin. J. Geotech. Eng. 2005, 05, 489–494. [Google Scholar]
- Lai, Y.M.; Cheng, H.B.; Gao, Z.H.; Zhang, S.J.; Chang, X.X. Stress-strain relationships and nonlinear mohr strength criterion of frozen sand clay. Chin. J. Rock Mech. Eng. 2007, 8, 1612–1617. [Google Scholar]
- Shear, D.L.; Olsen, H.W.; Nelson, K.R. Effects of Desiccation on the Hydraulic Conductivity versus Void Ratio Relationship for Natural Clay; National Academy Press: Washington, DC, USA, 1993; pp. 24–40. [Google Scholar]
- Zhou, Y.F.; Li, W.W.; Peng, Y.X.; Tang, S.W.; Wang, L.; Shi, Y.; Li, Y.; Wang, Y.; Geng, Z.C.; Wu, K. Hydration and fractal analysis on Low-heat Portland cement pastes by thermodynamic-based methods. Fractal Fract. 2023, 7, 606. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, H.; Huang, M.H.; Yin, H.B.; Jiang, K.; Xiao, K.T.; Tang, S.W. Influence of different alkali sulfates on the shrinkage, hydration, pore structure, fractal dimension and microstructure of low-heat Portland cement, medium-heat Portland cement and ordinary Portland cement. Fractal Fract. 2021, 5, 79. [Google Scholar] [CrossRef]
- Liao, Y.S.; Yao, J.X.; Deng, F.; Li, H.; Wang, K.J.; Tang, S.W. Hydration behavior and strength development of supersulfated cement prepared by calcined phosphogypsum and slaked lime. J. Build. Eng. 2023, 80, 108075–108090. [Google Scholar] [CrossRef]
- Peng, Y.X.; Tang, S.W.; Huang, J.S.; Tang, C.; Wang, L.; Liu, Y.F. Fractal analysis on pore structure and modeling of hydration of magnesium phosphate cement paste. Fractal Fract. 2022, 6, 337. [Google Scholar] [CrossRef]
- Hong, G.; Oh, S.; Kim, J.; Chin, W.J.; Kim, Y.J.; Choi, S.; Song, C. Surface-fractal-dimension characteristics of cementitious composites with multi-walled carbon nanotubes dispersed by silica fume. Constr. Build. Mater. 2022, 329, 127182. [Google Scholar] [CrossRef]




| Particle size range/mm | 0.5–2 | 0.25–0.5 | 0.1–0.25 | 0.075–0.1 | <0.075 |
| Percentage/% | 23.67 | 24.48 | 24.76 | 10.56 | 16.53 |
| Ionic Composition/(g·kg−1) | Salinity/% | PH Value | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CO32− | HCO32− | Cl− | SO42− | K+ | Na+ | Ca2+ | Mg2+ | Water-Soluble Salt Ion | ||
| 0.03 | 0 | 4.73 | 24.06 | 0.04 | 0.45 | 2.28 | 0.07 | 31.54 | 5.4 | 8.9 |
| Ingredient | SiO2 | Al2O3 | Fe2O3 | CaO | MgO | K2O | Na2O | TiO2 | SO3 | |
|---|---|---|---|---|---|---|---|---|---|---|
| Solidifying Agent | ||||||||||
| Cement/% | 22.41 | 6.85 | 3.83 | 56.92 | 1.52 | 0.78 | 0.29 | / | / | |
| Fly ash/% | 28.52 | 37.93 | 12.9 | 12.25 | 2.11 | 1.63 | 1.42 | 1.05 | 0.58 | |
| Silica fume/% | 89.42 | 2.33 | 0.77 | 0.65 | 3.29 | 2.90 | / | 0.03 | / | |
| Brick powder/% | 67.83 | 19.56 | 4.65 | 0.66 | 0.96 | 3.43 | 0.34 | 0.62 | / | |
| Serial Number | Temperature/°C | Water Content/% | Dry Density/g·cm−3 |
|---|---|---|---|
| S1 | −5 | 12.20 | 1.71 |
| S2 | 16.20 | 1.71 | |
| S3 | 20.20 | 1.71 | |
| G1 | 20.41 | 1.58 | |
| S4 | −10 | 12.20 | 1.71 |
| S5 | 16.20 | 1.71 | |
| S6 | 20.20 | 1.71 | |
| G2 | 20.41 | 1.58 | |
| S7 | −15 | 12.20 | 1.71 |
| S8 | 16.20 | 1.71 | |
| S9 | 20.20 | 1.71 | |
| G3 | 20.41 | 1.58 | |
| S10 | −20 | 12.20 | 1.71 |
| S11 | 16.20 | 1.71 | |
| S12 | 20.20 | 1.71 | |
| G4 | 20.41 | 1.58 |
| Serial Number | Cement:Fly Ash:Silica Fume:Brick Powder | Dry Density/g·cm−3 | Compaction Factor |
|---|---|---|---|
| SS0 | - | 1.71 | 0.9 |
| GG1 | 2:5:3:9 | 1.58 | 0.9 |
| GG2 | 3:5:5:6 | 1.58 | 0.9 |
| GG3 | 3:10:1:9 | 1.58 | 0.9 |
| Serial Number | Strain Type | E0/kPa | α | m | c | R | a | b | n | l |
|---|---|---|---|---|---|---|---|---|---|---|
| S1-150 | softening | 331.13 | 0.1421 | 12.3965 | 0.9741 | 1 | 0.0030 | - | 2.50 × 10−5 | 0.000103 |
| S1-250 | softening | 371.40 | 0.2283 | 4.0853 | 0.9932 | 1 | 0.0027 | - | 0.000183 | 4.37 × 10−5 |
| S1-350 | softening | 379.58 | 0.5238 | 1.1002 | 0.9989 | 1 | 0.0026 | - | 0.000302 | 1.79 × 10−5 |
| S1-450 | softening | 422.73 | 0.4150 | 1.9569 | 0.9998 | 1 | 0.0024 | - | 0.000322 | 1.06 × 10−5 |
| S2-150 | hardening | 118.93 | 1.4800 | 0.2507 | 0.9861 | 1 | 0.0084 | 0.0023 | - | - |
| S2-250 | hardening | 143.41 | 0.5756 | 0.5723 | 0.9738 | 1 | 0.0070 | 0.0015 | - | - |
| S2-350 | hardening | 240.02 | 0.5969 | 0.7202 | 0.9639 | 1 | 0.0042 | 0.0011 | - | - |
| S2-450 | hardening | 253.65 | 0.8037 | 0.5089 | 0.97226 | 1 | 0.0039 | 0.0010 | - | - |
| S3-150 | hardening | 136.22 | 0.8411 | 0.6005 | 0.9759 | 1 | 0.0073 | 0.0023 | - | - |
| S3-250 | hardening | 195.24 | 0.8041 | 0.6490 | 0.9768 | 1 | 0.0051 | 0.0017 | - | - |
| S3-350 | hardening | 223.30 | 0.7276 | 0.6559 | 0.9790 | 1 | 0.0045 | 0.0015 | - | - |
| S3-450 | hardening | 282.69 | 0.7456 | 0.6503 | 0.9815 | 1 | 0.0035 | 0.0012 | - | - |
| G1-150 | softening | 655.24 | 3.27 × 10−9 | 29.9974 | 0.9658 | 1.3994 | 0.0015 | - | −0.000230 | 0.000118 |
| G1-250 | softening | 650.95 | 4.65 × 10−4 | 3.2478 | 0.9565 | −0.0888 | 0.0015 | - | −8.56 × 10−5 | 7.24 × 10−5 |
| G1-350 | softening | 742.85 | 7.20 × 10−4 | 3.0987 | 0.9565 | −0.0886 | 0.0013 | - | −8.13 × 10−5 | 6.51 × 10−5 |
| G1-450 | softening | 880.70 | 2.21 × 10−2 | 1.8502 | 0.9545 | −0.1409 | 0.0011 | - | −6.96 × 10−5 | 5.48 × 10−5 |
| S4-150 | softening | 451.44 | 0.1616 | 6.7429 | 0.9698 | 1 | 0.0022 | - | 0.000103 | 0.000105 |
| S4-250 | softening | 443.22 | 0.3745 | 1.8808 | 0.9861 | 1 | 0.0023 | - | 0.000273 | 3.79 × 10−5 |
| S4-350 | softening | 447.83 | 0.5181 | 1.1137 | 0.9978 | 1 | 0.0022 | - | 0.000314 | 1.83 × 10−5 |
| S4-450 | softening | 478.44 | 0.4947 | 1.1387 | 0.9994 | 1 | 0.0021 | - | 0.000238 | 1.49 × 10−5 |
| S5-150 | softening | 291.60 | 0.2499 | 3.0489 | 0.9997 | 1 | 0.0034 | - | 0.000599 | 1.98 × 10−5 |
| S5-250 | softening | 294.41 | 0.2418 | 3.5054 | 0.9998 | 1 | 0.0034 | - | 0.000469 | 1.71 × 10−5 |
| S5-350 | hardening | 246.50 | 0.2083 | 0.9621 | 0.9606 | 1 | 0.0041 | 0.0008 | - | - |
| S5-450 | hardening | 258.36 | 0.4710 | 0.6067 | 0.9770 | 1 | 0.0039 | 0.0008 | - | - |
| S6-150 | softening | 375.93 | 0.1949 | 4.1591 | 0.9996 | 1 | 0.0027 | - | 0.000482 | 1.30 × 10−5 |
| S6-250 | hardening | 405.91 | 1.2325 | 0.3182 | 0.9796 | 1 | 0.0025 | 0.0008 | - | - |
| S6-350 | hardening | 407.58 | 1.3187 | 0.3313 | 0.9947 | 1 | 0.0025 | 0.0007 | - | - |
| S6-450 | hardening | 436.18 | 1.1781 | 0.4599 | 0.9767 | 1 | 0.0023 | 0.0007 | - | - |
| G2-150 | softening | 1615.12 | 1.4396 | 2.1666 | 0.9873 | 3.3720 | 0.0006 | - | −0.000140 | 9.54 × 10−5 |
| G2-250 | softening | 1456.87 | 3.86 × 10−11 | 63.0473 | 0.9834 | 0.5974 | 0.0007 | - | −9.41 × 10−5 | 6.35 × 10−5 |
| G2-350 | softening | 1470.11 | 3.31 × 10−9 | 43.0420 | 0.9757 | 0.9361 | 0.0007 | - | −9.22 × 10−5 | 5.75 × 10−5 |
| G2-450 | softening | 1777.93 | 0.9954 | 2.4901 | 0.9693 | 2.7732 | 0.0006 | - | −2.42 × 10−5 | 4.25 × 10−5 |
| S7-150 | softening | 410.25 | 0.0936 | 24.7954 | 0.9735 | 1 | 0.0024 | - | −6.89 × 10−5 | 8.80 × 10−5 |
| S7-250 | softening | 500.71 | 0.4399 | 1.7312 | 0.9895 | 1 | 0.0020 | - | 0.000270 | 3.20 × 10−5 |
| S7-350 | softening | 556.99 | 0.4529 | 1.5723 | 0.9888 | 1 | 0.0018 | - | 0.000160 | 2.80 × 10−5 |
| S7-450 | softening | 672.19 | 0.4783 | 1.1941 | 0.9992 | 1 | 0.0015 | - | 0.000285 | 1.19 × 10−5 |
| S8-150 | softening | 514.56 | 0.1611 | 5.8890 | 0.9993 | 1 | 0.0019 | - | 0.000208 | 5.71 × 10−5 |
| S8-250 | softening | 485.09 | 0.1594 | 5.9600 | 0.9976 | 1 | 0.0021 | - | 0.000200 | 2.62 × 10−5 |
| S8-350 | softening | 483.32 | 0.1301 | 7.5804 | 0.9996 | 1 | 0.0021 | - | 0.000198 | 1.56 × 10−5 |
| S8-450 | softening | 715.09 | 0.2598 | 3.0114 | 0.9997 | 1 | 0.0014 | - | 0.000324 | 6.43 × 10−6 |
| S9-150 | softening | 692.94 | 0.1812 | 4.6181 | 0.9993 | 1 | 0.0014 | - | 0.000282 | 1.15 × 10−5 |
| S9-250 | hardening | 732.15 | 1.0416 | 0.4636 | 0.9968 | 1 | 0.0014 | 0.0005 | - | - |
| S9-350 | hardening | 665.69 | 1.0707 | 0.4085 | 0.9954 | 1 | 0.0015 | 0.0005 | - | - |
| S9-450 | hardening | 773.35 | 1.0419 | 0.4172 | 0.9956 | 1 | 0.0013 | 0.0004 | - | - |
| G3-150 | softening | 1400.35 | 0.2751 | 0.5180 | 0.6810 | −1.9824 | 0.0007 | - | −3.19 × 10−5 | 2.35 × 10−5 |
| G3-250 | softening | 1478.66 | 0.6267 | 0.3236 | 0.7153 | −2.2527 | 0.0007 | - | −3.57 × 10−5 | 2.23 × 10−5 |
| G3-350 | softening | 1604.61 | 6.0135 | 0.0662 | 0.6989 | 1.0372 | 0.0006 | - | −2.54 × 10−5 | 1.85 × 10−5 |
| G3-450 | softening | 1799.37 | 4.3943 | 0.0860 | 0.7197 | 1.3024 | 0.0006 | - | −2.29 × 10−5 | 1.65 × 10−5 |
| S10-150 | softening | 650.78 | 0.0985 | 21.4075 | 0.9928 | 1 | 0.0015 | - | −4.14 × 10−5 | 8.64 × 10−5 |
| S10-250 | softening | 640.02 | 0.1196 | 20.4655 | 0.9778 | 1 | 0.0016 | - | −1.71 × 10−5 | 5.37 × 10−5 |
| S10-350 | softening | 751.96 | 0.1577 | 7.5894 | 0.9884 | 1 | 0.0013 | - | 5.33 × 10−5 | 3.62 × 10−5 |
| S10-450 | softening | 894.44 | 0.4494 | 1.8128 | 0.9872 | 1 | 0.0011 | - | 0.000135 | 2.00 × 10−5 |
| S11-150 | softening | 582.87 | 0.1307 | 10.6561 | 0.9971 | 1 | 0.0017 | - | 5.86 × 10−5 | 5.11 × 10−5 |
| S11-250 | softening | 757.55 | 0.1838 | 4.9452 | 0.9863 | 1 | 0.0013 | - | 6.32 × 10−5 | 3.77 × 10−5 |
| S11-350 | softening | 870.53 | 0.2303 | 4.5985 | 0.9848 | 1 | 0.0011 | - | 0.000101 | 2.59 × 10−5 |
| S11-450 | softening | 910.86 | 0.6635 | 1.1062 | 0.9998 | 1 | 0.0011 | - | 0.000220 | 7.68 × 10−6 |
| S12-150 | softening | 1155.15 | 0.2146 | 3.6436 | 0.9995 | 1 | 0.0009 | - | 0.000242 | 4.90 × 10−6 |
| S12-250 | softening | 1014.03 | 0.2542 | 2.7743 | 0.9997 | 1 | 0.0010 | - | 0.000213 | 5.41 × 10−6 |
| S12-350 | hardening | 1059.35 | 1.1818 | 0.4237 | 0.9954 | 1 | 0.0009 | 0.0003 | - | - |
| S12-450 | hardening | 1072.20 | 1.1690 | 0.4848 | 0.9789 | 1 | 0.0009 | 0.0003 | - | - |
| G4-150 | softening | 2104.34 | 0.3396 | 0.4614 | 0.7668 | −1.2974 | 0.0005 | - | −1.95 × 10−5 | 1.75 × 10−5 |
| G4-250 | softening | 2307.93 | 1.4441 | 0.2117 | 0.4483 | −23.0522 | 0.0004 | - | 4.37 × 10−6 | 1.12 × 10−5 |
| G4-350 | softening | 2279.74 | 0.1648 | 0.6288 | 0.5232 | −3.3686 | 0.0004 | - | −3.54 × 10−6 | 9.40 × 10−6 |
| G4-450 | softening | 2609.08 | 5.9660 | 0.0666 | 0.7109 | −4.8763 | 0.0004 | - | −1.58 × 10−5 | 1.14 × 10−5 |
| Serial Number | (σ1 − σ3)/kPa | Shear Strength Index | ||||
|---|---|---|---|---|---|---|
| 150 | 250 | 350 | 450 | c/kPa | φ/(°) | |
| S1 | 876.38 | 1150.99 | 1359.11 | 1564.32 | 153.02 | 32.18 |
| S2 | 430.06 | 679.20 | 883.14 | 1046.92 | 40.22 | 30.52 |
| S3 | 436.53 | 573.03 | 671.08 | 804.39 | 107.94 | 22.06 |
| S4 | 935.46 | 1164.99 | 1392.83 | 1691.77 | 145.66 | 33.77 |
| S5 | 893.00 | 1051.51 | 1224.36 | 1325.60 | 216.13 | 25.14 |
| S6 | 1170.73 | 1257.38 | 1334.73 | 1416.69 | 404.89 | 16.83 |
| S7 | 1166.12 | 1288.04 | 1644.50 | 1817.30 | 211.03 | 32.72 |
| S8 | 1143.57 | 1504.68 | 1792.00 | 1946.59 | 200.03 | 35.33 |
| S9 | 1853.38 | 1944.51 | 2104.58 | 2384.79 | 484.19 | 28.30 |
| S10 | 1454.57 | 1778.47 | 2033.42 | 2303.77 | 269.19 | 35.73 |
| S11 | 1536.31 | 1963.57 | 2243.68 | 2479.06 | 272.98 | 37.69 |
| S12 | 2686.38 | 2783.23 | 2868.15 | 3188.01 | 752.64 | 27.13 |
| G1 | 1604.84 | 1720.52 | 1864.05 | 2001.54 | 457.25 | 23.59 |
| G2 | 2892.81 | 3090.37 | 3297.42 | 3506.97 | 739.26 | 30.41 |
| G3 | 4397.61 | 4872.26 | 5279.85 | 5929.48 | 734.15 | 45.71 |
| G4 | 6136.53 | 6971.10 | 8006.12 | 8609.51 | 790.17 | 54.08 |
| Serial Number | Model | Region I | Region II | Region III |
|---|---|---|---|---|
| SS0 | Menger sponge | 2.9338 | 2.8191 | 2.9386 |
| GG1 | 2.4103 | 4.6209 | 2.8205 | |
| GG2 | 2.4478 | 4.9026 | 2.9015 | |
| GG3 | 2.6043 | 3.9704 | 2.8343 | |
| SS0 | Neimark | 2.8229 | 3.0617 | 2.9268 |
| GG1 | 2.5826 | 4.4987 | 2.7597 | |
| GG2 | 2.6955 | 4.6745 | 2.8052 | |
| GG3 | 2.4503 | 4.3140 | 2.7755 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, X.; Li, H.; Zhang, G.; Li, S.; Shan, L.; Zhao, J.; Zhang, Z. A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis. Fractal Fract. 2024, 8, 33. https://doi.org/10.3390/fractalfract8010033
Kang X, Li H, Zhang G, Li S, Shan L, Zhao J, Zhang Z. A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis. Fractal and Fractional. 2024; 8(1):33. https://doi.org/10.3390/fractalfract8010033
Chicago/Turabian StyleKang, Xinrui, Hongbo Li, Gang Zhang, Sheng Li, Long Shan, Jing Zhao, and Zhe Zhang. 2024. "A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis" Fractal and Fractional 8, no. 1: 33. https://doi.org/10.3390/fractalfract8010033
APA StyleKang, X., Li, H., Zhang, G., Li, S., Shan, L., Zhao, J., & Zhang, Z. (2024). A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis. Fractal and Fractional, 8(1), 33. https://doi.org/10.3390/fractalfract8010033







