Computational Study for Fiber Bragg Gratings with Dispersive Reflectivity Using Fractional Derivative
Abstract
:1. Introduction
2. Preliminaries
- (a)
- (b)
3. RPST to FBGs for Cubic-Quartic Dispersive Reflectivity with Time-Fractional Derivative
3.1. General Procedure of the RPST
- Step A. Assume the fractional power series solutions of above system regarding the initial point as
- ,for each and ,
- Step C. Substituting , and into (16) and calculating the fractional derivative of , and , at the initial point , together with results mentioned in Step B, the resulting algebraic systems are as follows:
- Step D. The required values of , and , can be derived by solving Systems (17). Finally, the residual power series solutions can be obtained.
3.2. Residual Power Series Solutions of Proposed Model
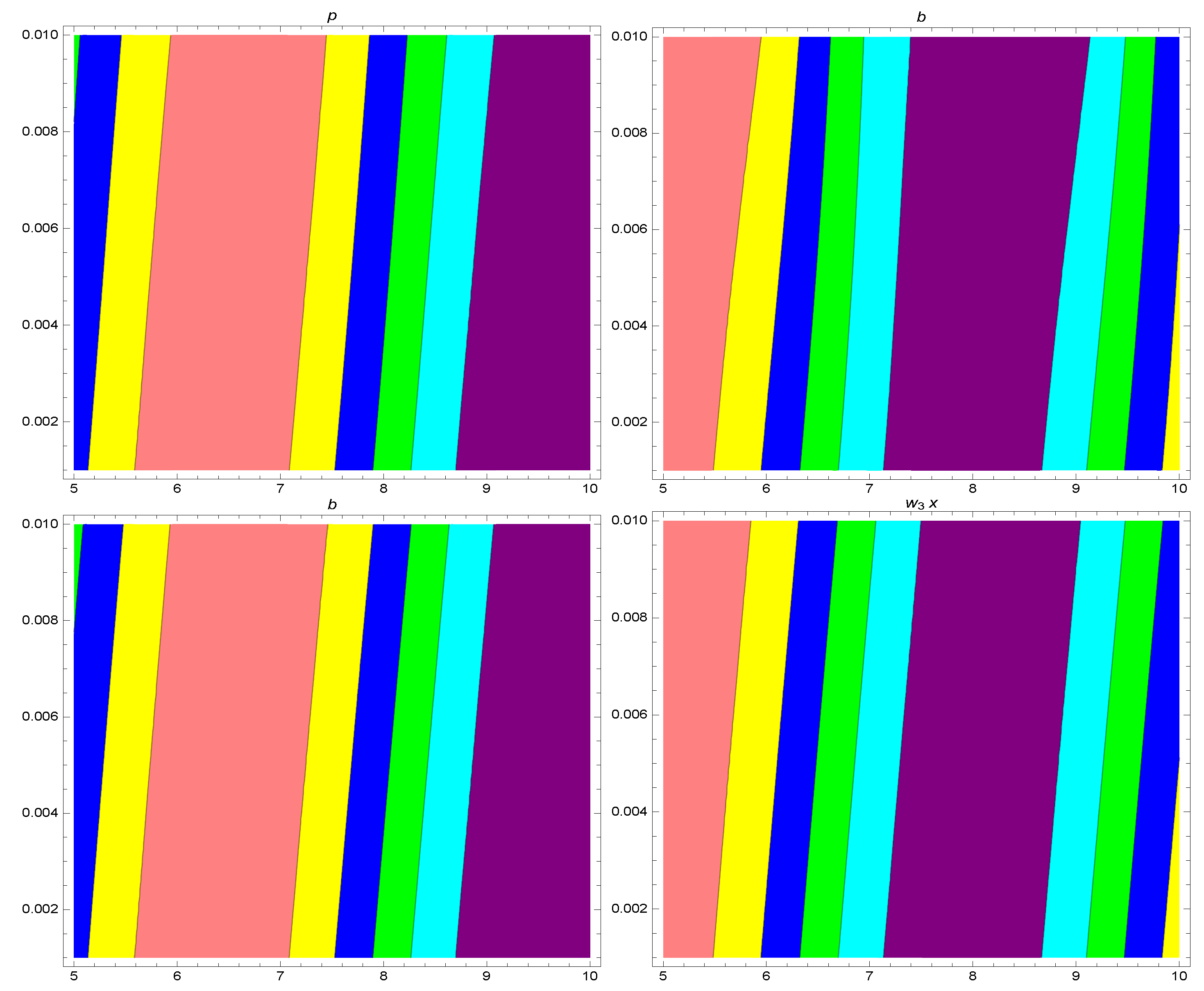
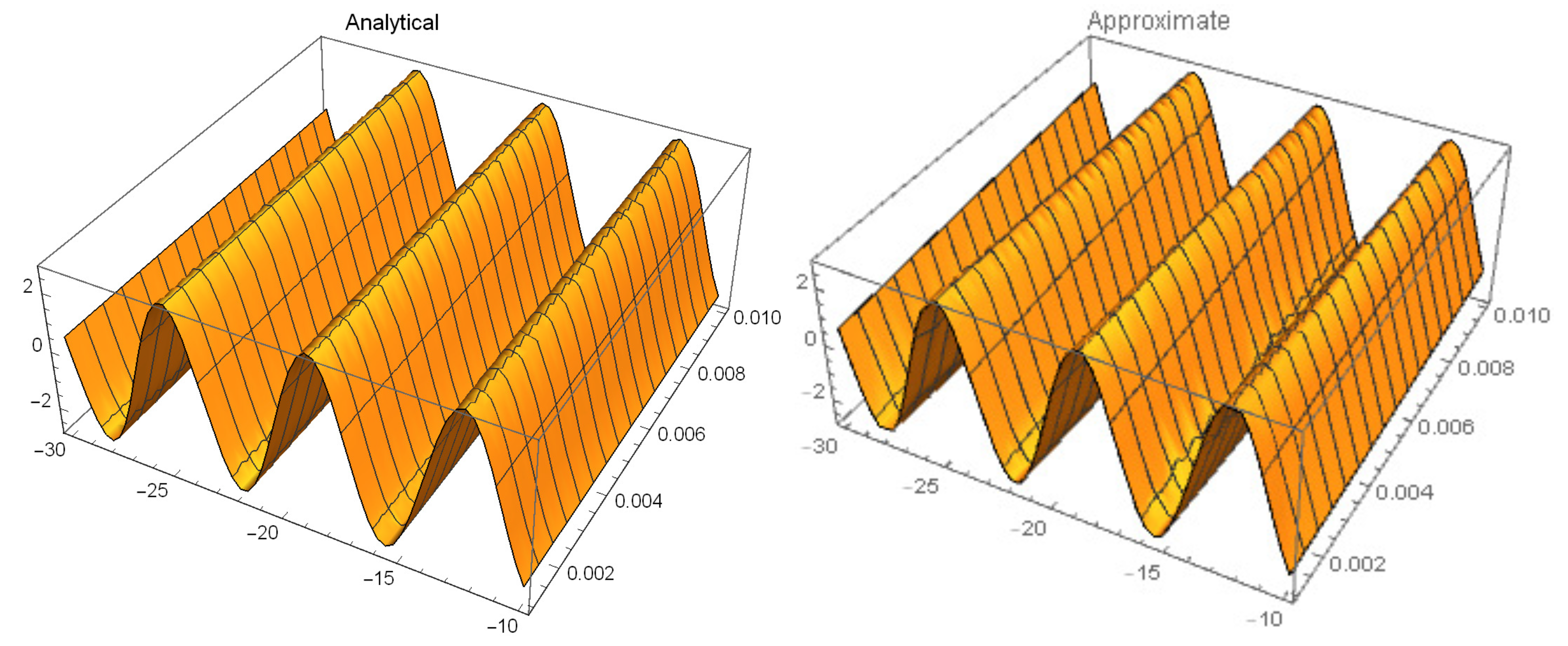
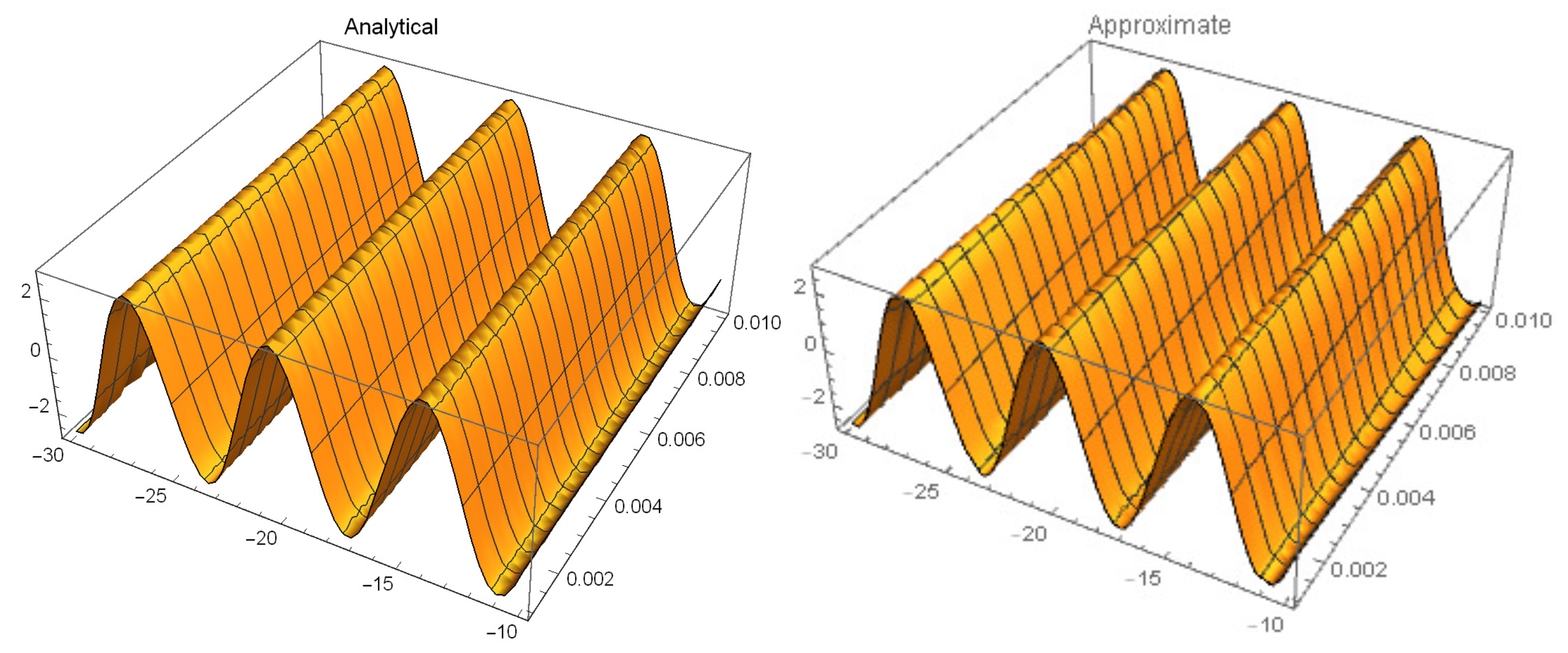
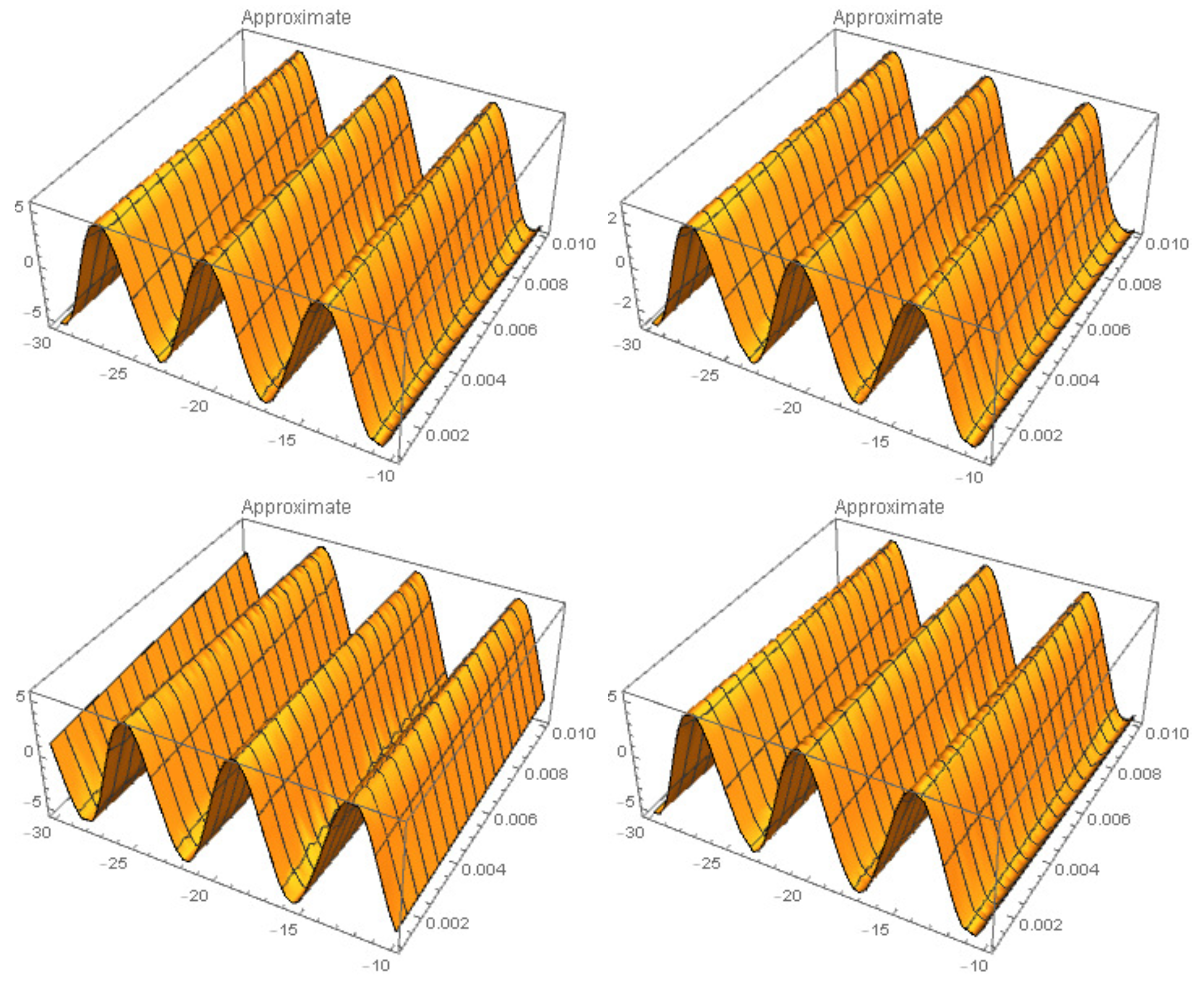
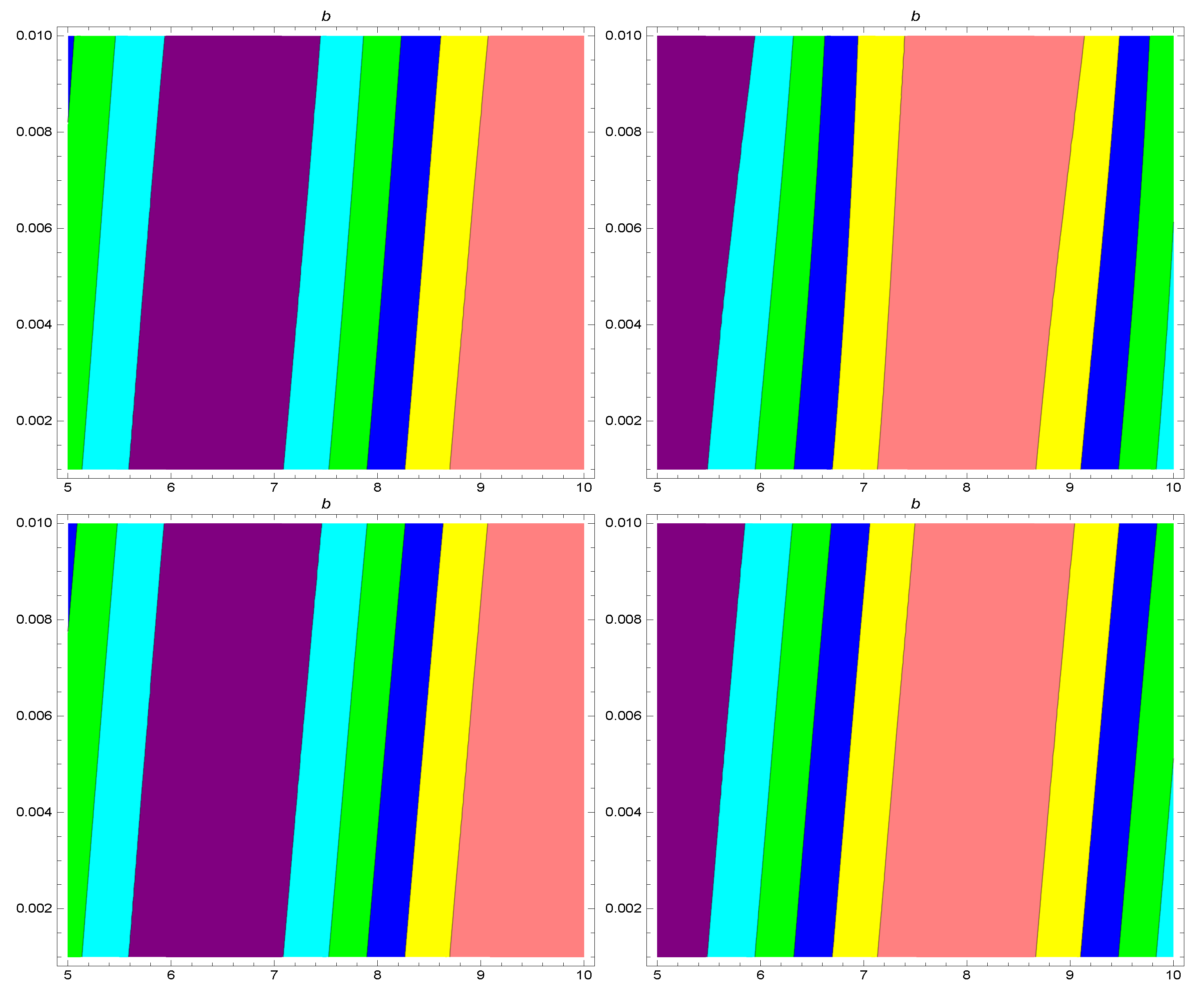
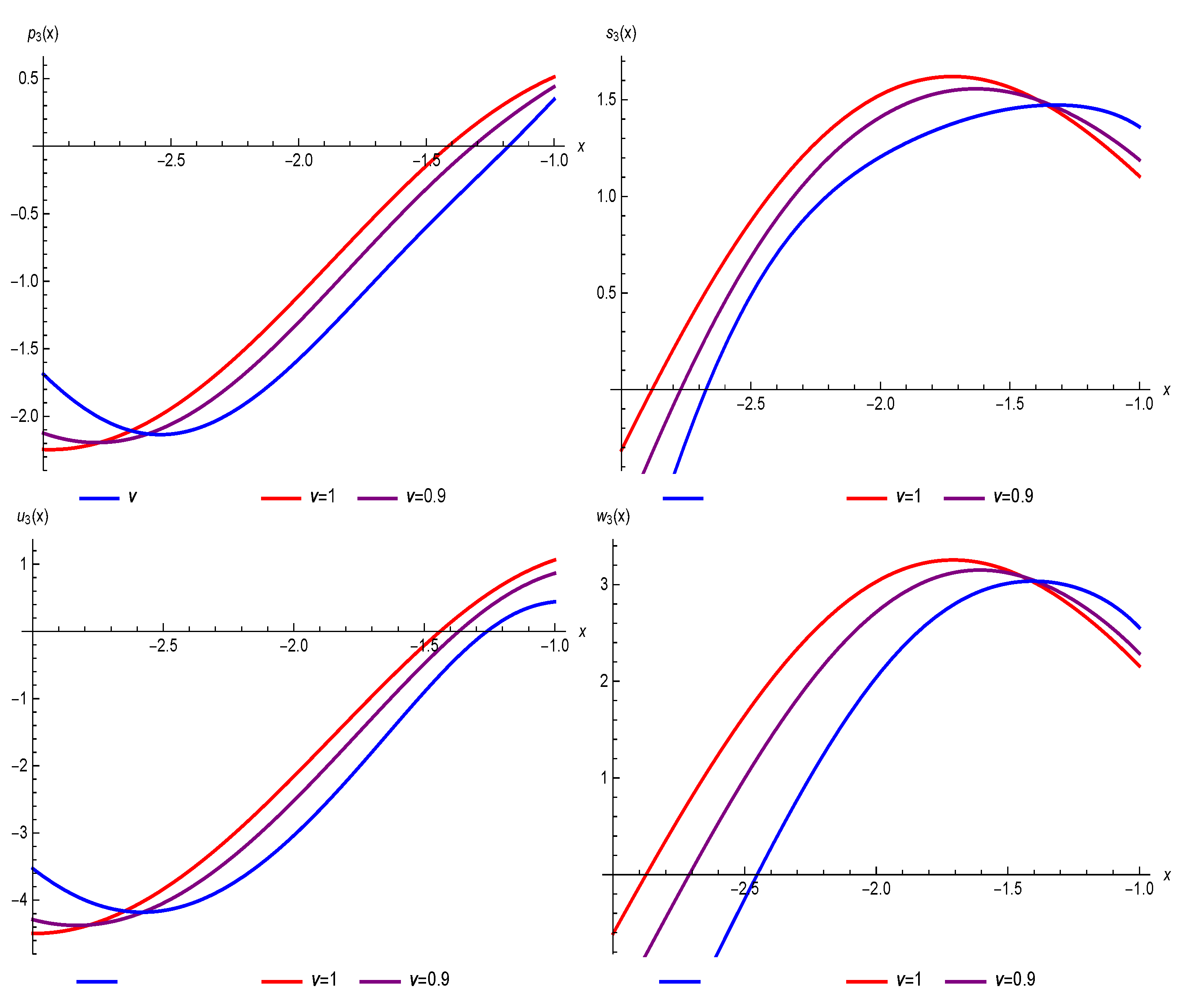
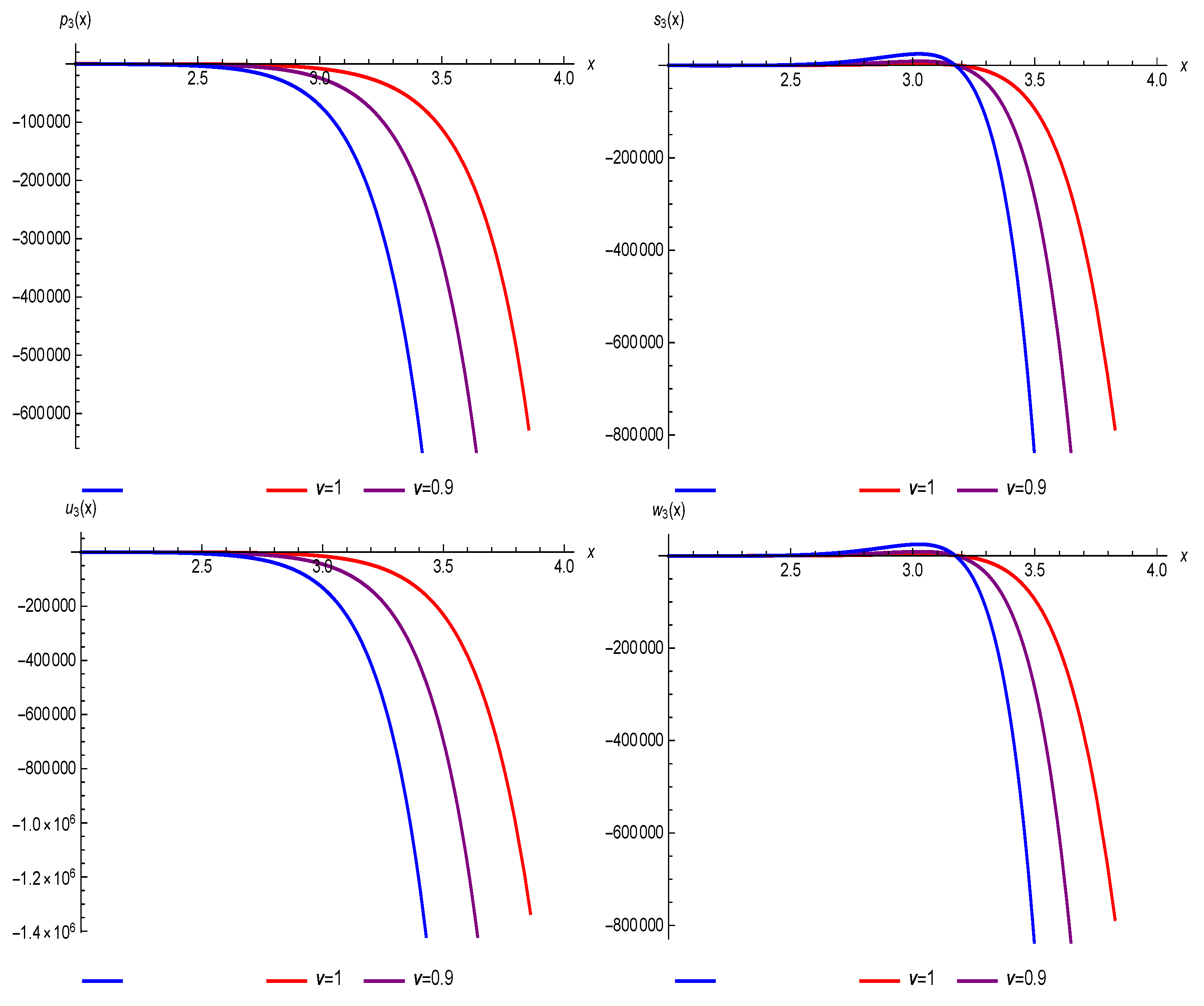
4. Graphical Representation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Biswas, A.; Fessak, M.; Johnson, S.; Beatrice, S.; Milovic, D.; Jovanoski, Z.; Majid, F. Optical soliton perturbation in non-Kerr law media: Traveling wave solution. Opt. Laser Technol. 2012, 44, 263–268. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Periodic and solitary waves in optical fiber Bragg gratings with dispersive reflectivity. Chin. J. Phys. 2020, 66, 401–405. [Google Scholar] [CrossRef]
- Kan, K.V.; Kudryashov, N.A. Solitary waves described by a high-order system in optical fiber Bragg gratings with arbitrary refractive index. Math. Methods Appl. Sci. 2022, 45, 1072–1079. [Google Scholar] [CrossRef]
- Zayed, E.M.; Nofal, T.A.; Gepreel, K.A.; Shohib, R.M.; Alngar, M.E. Cubic-quartic optical soliton solutions in fiber Bragg gratings with Lakshmanan-Porsezian-Daniel model by two integration schemes. Opt. Quantum Electron. 2021, 53, 249. [Google Scholar] [CrossRef]
- Biswas, A.; Konar, S. Introduction to Non-Kerr Law Optical Solitons; Chapman and Hall/CRC: Boca Raton, FL, USA, 2006. [Google Scholar]
- Zhou, Q.; Yao, D.; Xu, Q.; Liu, X. Optical soliton perturbation with time and space dependent dissipation (orgain) and nonlinear dispersion in Kerr and non-Kerr media. Optik 2013, 124, 2368–2372. [Google Scholar] [CrossRef]
- Biswas, A.; Ekici, M.; Sonmezoglu, A.; Belic, M.R. Highly dispersive optical solitons with Kerr law nonlinearity by F-expansion. Optik 2019, 181, 1028–1038. [Google Scholar] [CrossRef]
- Alkhidhr, H.A. Closed-form solutions to the perturbed NLSE with Kerr law nonlinearity in optical fibers. Results Phys. 2021, 22, 103875. [Google Scholar] [CrossRef]
- Gunay, B. Optical soliton solutions to a higher-order nonlinear Schrödinger equation with Kerr law nonlinearity. Results Phys. 2021, 27, 104515. [Google Scholar] [CrossRef]
- Malik, S.; Kumar, S.; Biswas, A.; Yildirim, Y.; Moraru, L.; Moldovanu, S.; Iticescu, C.; Moshokoa, S.P.; Bibicu, D.; Alotaibi, A. Gap Solitons in Fiber Bragg Gratings Having Polynomial Law of Nonlinear Refractive Index and Cubic-Quartic Dispersive Reflectivity by Lie Symmetry. Symmetry 2023, 15, 963. [Google Scholar] [CrossRef]
- Triki, H.; Zhou, Q.; Biswas, A.; Liu, W.; Yildirim, Y.; Alshehri, H.M.; Belic, M.R. Chirped optical solitons having polynomial law of nonlinear refractive index with self-steepening and nonlinear dispersion. Phys. Lett. A 2021, 417, 127698. [Google Scholar] [CrossRef]
- Zayed, E.M.; Shohib, R.M.; Alngar, M.E. Cubic-quartic optical solitons in Bragg gratings fibers for NLSE having parabolic non-local law nonlinearity using two integration schemes. Opt. Quantum Electron. 2021, 53, 452. [Google Scholar] [CrossRef]
- Zayed, E.M.; Alngar, M.E.; El-Horbaty, M.; Biswas, A.; Alshomrani, A.S.; Khan, S.; Triki, H. Optical solitons in fiber Bragg gratings having Kerr law of refractive index with extended Kudryashov method and new extended auxiliary equation approach. Chin. J. Phys. 2020, 66, 187–205. [Google Scholar] [CrossRef]
- Yildirim, Y.; Biswas, A.; Khan, S.; Guggilla, P.; Alzahrani, A.K.; Belic, M.R. Optical solitons in fiber Bragg gratings with dispersive reflectivity by sine-Gordon equation approach. Optik 2021, 237, 166684. [Google Scholar] [CrossRef]
- Sahota, J.K.; Gupta, N.; Dhawan, D. Fiber Bragg grating sensors for monitoring of physical parameters: A comprehensive review. Opt. Eng. 2020, 59, 060901. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, M.; Zhu, C.; Li, H. Multichannel fiber bragg grating based on DC-sampling method. Opt. Commun. 2019, 445, 142–146. [Google Scholar] [CrossRef]
- Wang, M.Y.; Biswas, A.; Yildirim, Y.; Alshehri, H.M.; Moraru, L.; Moldovanu, S. Optical solitons in fiber Bragg gratings with dispersive reflectivity having five nonlinear forms of refractive index. Axioms 2022, 11, 640. [Google Scholar] [CrossRef]
- Yildirim, Y.; Biswas, A.; Guggilla, P.; Khan, S.; Alshehri, H.M.; Belic, M.R. Optical solitons in fibre Bragg gratings with third and fourth order dispersive reflectivities. Ukr. J. Phys. Opt. 2021, 22, 239–254. [Google Scholar] [CrossRef]
- Malik, S.; Kumar, S.; Biswas, A.; Yildirim, Y.; Moraru, L.; Moldovanu, S.; Iticescu, C.; Alshehri, H.M. Cubic-quartic optical solitons in fiber bragg gratings with dispersive reflectivity having parabolic law of nonlinear refractive index by lie symmetry. Symmetry 2022, 14, 2370. [Google Scholar] [CrossRef]
- Arnous, A.H.; Zhou, Q.; Biswas, A.; Guggilla, P.; Khan, S.; Yildirim, Y.; Alshomrani, A.S.; Alshehri, H.M. Optical solitons in fiber Bragg gratings with cubic-quartic dispersive reflectivity by enhanced Kudryashov’s approach. Phys. Lett. A 2022, 422, 127797. [Google Scholar] [CrossRef]
- Zayed, E.M.; Alngar, M.E.; Biswas, A.; Ekici, M.; Alzahrani, A.K.; Belic, M.R. Solitons in fiber Bragg gratings with cubic-quartic dispersive reflectivity having Kerr law of nonlinear refractive index. J. Nonlinear Opt. Phys. Mater. 2020, 29, 2050011. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Sabatier, J.; Agrawal, O.P.; Machado, J.A.T. Advances in Fractional Calculus; Springer: Dordrecht, The Netherlands, 2007; Volume 4. [Google Scholar]
- Tian, Q.; Yang, X.; Zhang, H.; Xu, D. An implicit robust numerical scheme with graded meshes for the modified Burgers model with nonlocal dynamic properties. Comput. Appl. Math. 2023, 42, 246. [Google Scholar] [CrossRef]
- Guo, L.; Zhao, X.L.; Gu, X.M.; Zhao, Y.L.; Zheng, Y.B.; Huang, T.Z. Three-dimensional fractional total variation regularized tensor optimized model for image deblurring. Appl. Math. Comput. 2021, 404, 126224. [Google Scholar] [CrossRef]
- Yang, X.; Wu, L.; Zhang, H. A space-time spectral order sinc-collocation method for the fourth-order nonlocal heat model arising in viscoelasticity. Appl. Math. Comput. 2023, 457, 128192. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, J.; Wang, W.; Zhang, H. A Predictor-Corrector Compact Difference Scheme for a Nonlinear Fractional Differential Equation. Fractal Fract. 2023, 7, 521. [Google Scholar] [CrossRef]
- Wu, G.C. A fractional variational iteration method for solving fractional nonlinear differential equations. Comput. Math. Appl. 2011, 61, 2186–2190. [Google Scholar] [CrossRef]
- Ganjiani, M. Solution of nonlinear fractional differential equations using homotopy analysis method. Appl. Math. Model. 2010, 34, 1634–1641. [Google Scholar] [CrossRef]
- Hashim, D.J.; Jameel, A.F.; Ying, T.Y.; Alomari, A.K.; Anakira, N.R. Optimal homotopy asymptotic method for solving several models of first order fuzzy fractional IVPs. Alex. Eng. J. 2022, 61, 4931–4943. [Google Scholar] [CrossRef]
- Nadeem, M.; He, J.H.; Islam, A. The homotopy perturbation method for fractional differential equations: Part 1 Mohand transform. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 3490–3504. [Google Scholar] [CrossRef]
- Alkresheh, H.A.; Ismail, A.I. Multi-step fractional differential transform method for the solution of fractional order stiff systems. Ain Shams Eng. J. 2021, 12, 4223–4231. [Google Scholar] [CrossRef]
- Zhang, Q.; Qin, Y.; Sun, Z.Z. Linearly compact scheme for 2D Sobolev equation with Burgers’ type nonlinearity. Numer. Algorithms 2022, 91, 1081–1114. [Google Scholar] [CrossRef]
- Jafari, H.; Daftardar-Gejji, V. Solving a system of nonlinear fractional differential equations using Adomian decomposition. J. Comput. Appl. Math. 2006, 196, 644–651. [Google Scholar] [CrossRef]
- Korpinar, T.; Korpinar, Z. Approximate solutions for optical magnetic and electric phase with fractional optical Heisenberg ferromagnetic spin by RPSM. Optik 2021, 247, 167819. [Google Scholar] [CrossRef]
- Tariq, H.; Akram, G. Residual power series method for solving time-space-fractional Benney-Lin equation arising in falling film problems. J. Appl. Math. Comput. 2017, 55, 683–708. [Google Scholar] [CrossRef]
- Burqan, A.; Saadeh, R.; Qazza, A.; Momani, S. ARA-residual power series method for solving partial fractional differential equations. Alex. Eng. J. 2023, 62, 47–62. [Google Scholar] [CrossRef]
- Saadeh, R. A reliable algorithm for solving system of multi-pantograph equations. WSEAS Trans. Math 2022, 21, 792–800. [Google Scholar] [CrossRef]
- Ismail, G.M.; Abdl-Rahim, H.R.; Ahmad, H.; Chu, Y.M. Fractional residual power series method for the analytical and approximate studies of fractional physical phenomena. Open Phys. 2020, 18, 799–805. [Google Scholar] [CrossRef]
- Arqub, O.A. Series solution of fuzzy differential equations under strongly generalized differentiability. J. Adv. Res. Appl. Math 2013, 5, 31–52. [Google Scholar] [CrossRef]
- Wang, L.; Chen, X. Approximate analytical solutions of time fractional Whitham-Broer-Kaup equations by a residual power series method. Entropy 2015, 17, 6519–6533. [Google Scholar] [CrossRef]
- Dubey, V.P.; Kumar, R.; Kumar, D. A reliable treatment of residual power series method for time-fractional Black-Scholes European option pricing equations. Phys. A Stat. Mech. Its Appl. 2019, 533, 122040. [Google Scholar] [CrossRef]
- Senol, M.; Ata, A. Approximate solution of time-fractional KdV equations by residual power series method. BAUN Fen Bil. Enst. Dergisi 2018, 20, 430–439. [Google Scholar] [CrossRef]
- Tariq, H.; Sadaf, M.; Akram, G.; Rezazadeh, H.; Baili, J.; Lv, Y.P.; Ahmad, H. Computational study for the conformable nonlinear Schrödinger equation with cubic-quintic-septic nonlinearities. Results Phys. 2021, 30, 104839. [Google Scholar] [CrossRef]
- Korpinar, Z.; Inc, M. Numerical simulations for fractional variation of (1 + 1)-dimensional Biswas-Milovic equation. Optik 2018, 166, 77–85. [Google Scholar] [CrossRef]
- Tariq, H.; Ahmed, H.; Rezazadeh, H.; Javeed, S.; Alimgeer, K.S.; Nonlaopon, K.; Khedher, K.M. New travelling wave analytic and residual power series solutions of conformable Caudrey-Dodd-Gibbon-Sawada-Kotera equationequation. Results Phys. 2021, 29, 104591. [Google Scholar] [CrossRef]
- Tariq, H.; Akram, G. New traveling wave exact and approximate solutions for the nonlinear Cahn-Allen equation: Evolution of a nonconserved quantity. Nonlinear Dyn. 2017, 88, 581–594. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A.; Baleanu, D. Two analytical methods for time-fractional nonlinear coupled Boussinesq-Burger’s equations arise in propagation of shallow water waves. Nonlinear Dyn. 2016, 85, 699–715. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tariq, H.; Akram, G.; Sadaf, M.; Iftikhar, M.; Guran, L. Computational Study for Fiber Bragg Gratings with Dispersive Reflectivity Using Fractional Derivative. Fractal Fract. 2023, 7, 625. https://doi.org/10.3390/fractalfract7080625
Tariq H, Akram G, Sadaf M, Iftikhar M, Guran L. Computational Study for Fiber Bragg Gratings with Dispersive Reflectivity Using Fractional Derivative. Fractal and Fractional. 2023; 7(8):625. https://doi.org/10.3390/fractalfract7080625
Chicago/Turabian StyleTariq, Hira, Ghazala Akram, Maasoomah Sadaf, Maria Iftikhar, and Liliana Guran. 2023. "Computational Study for Fiber Bragg Gratings with Dispersive Reflectivity Using Fractional Derivative" Fractal and Fractional 7, no. 8: 625. https://doi.org/10.3390/fractalfract7080625
APA StyleTariq, H., Akram, G., Sadaf, M., Iftikhar, M., & Guran, L. (2023). Computational Study for Fiber Bragg Gratings with Dispersive Reflectivity Using Fractional Derivative. Fractal and Fractional, 7(8), 625. https://doi.org/10.3390/fractalfract7080625






