Fractional-Order Impulsive Delayed Reaction-Diffusion Gene Regulatory Networks: Almost Periodic Solutions
Abstract
1. Introduction
2. Problem Introduction and Preliminary Notes
2.1. Fractional-Order Impulsive Delayed Reaction-Diffusion GRN Model
2.2. Almost Periodicity Definitions
2.3. Fundamentals of Fractional Lyapunov Method
3. Almost Periodicity Results
3.1. Boundedness
3.2. Almost Periodicity
3.3. Perfect Mittag–Leffler Stability
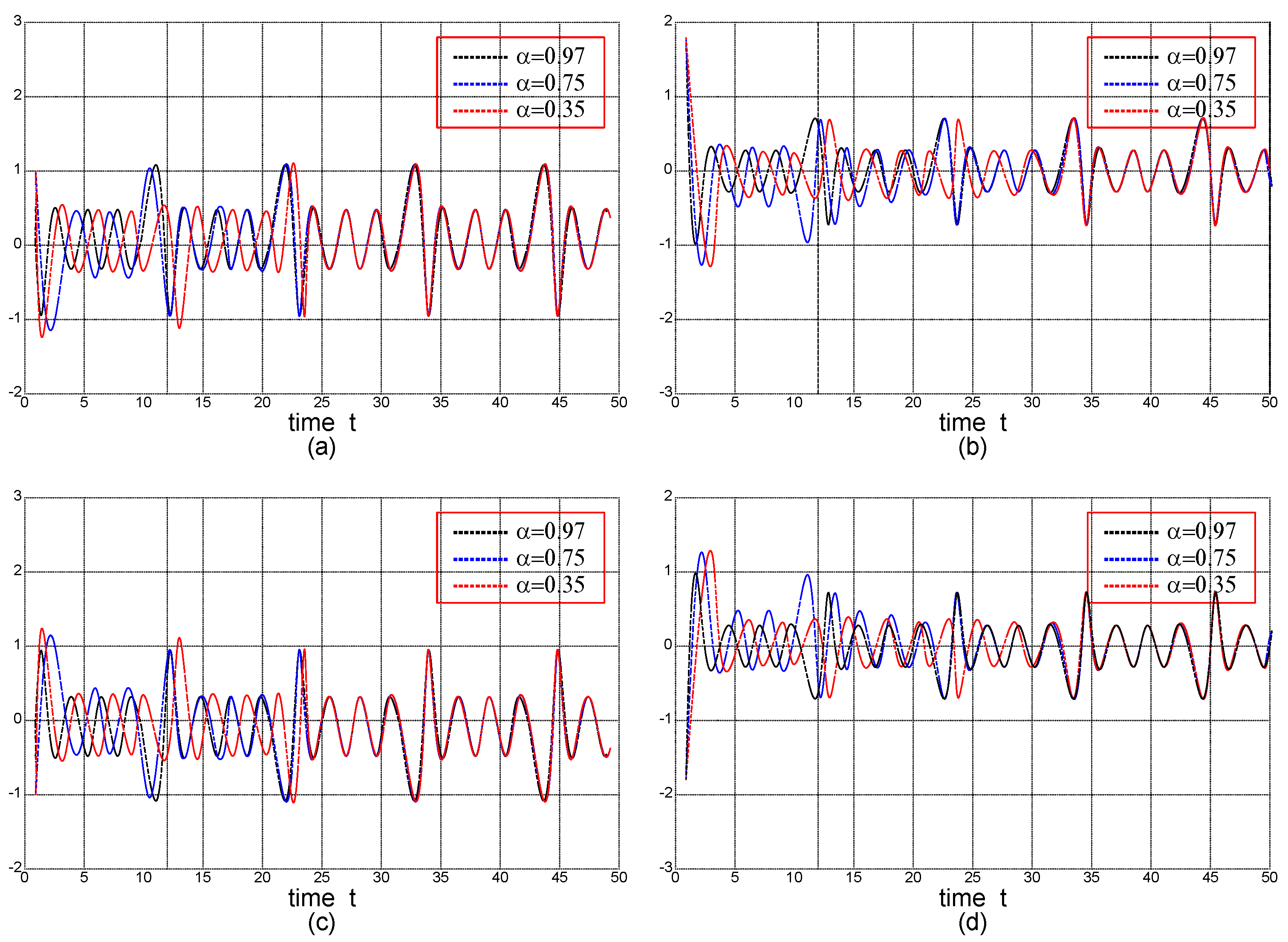
4. Illustrative Examples
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chaouiya, C.; Remy, E.; Thieffry, D. Petri net modelling of biological regulatory networks. J. Discret. Algorithms 2008, 6, 165–177. [Google Scholar] [CrossRef][Green Version]
- Friedman, N.; Linial, M.; Nachman, I.; Pe’er, D. Using Bayesian networks to analyze expression data. J. Comput. Biol. 2000, 7, 601–620. [Google Scholar] [CrossRef] [PubMed]
- De Jong, H. Modeling and simulation of genetic regulatory systems: A literature review. J. Comput. Biol. 2002, 9, 67–103. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Karlebach, G.; Shamir, R. Modelling and analysis of gene regulatory networks. Nat. Rev. Mol. Cell Biol. 2008, 9, 770–780. [Google Scholar] [CrossRef]
- Porreca, R.; Drulhe, S.; de Jong, H.; Ferrari-Trecate, G. Structural identification of piecewise-linear models of genetic regulatory networks. J. Comput. Biol. 2008, 15, 1365–1380. [Google Scholar] [CrossRef][Green Version]
- Wu, L.; Liu, K.; Lü, J.; Gu, H. Finite-time adaptive stability of gene regulatory networks stability. Neurocomputing 2019, 338, 222–232. [Google Scholar] [CrossRef]
- Li, X.; Rakkiyappan, R.; Pradeep, C. Robust μ-stability analysis of Markovian switching uncertain stochastic genetic regulatory networks with unbounded time-varying delays. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 3894–3905. [Google Scholar] [CrossRef]
- Ren, F.; Cao, J. Asymptotic and robust stability of genetic regulatory networks with time-varying delays. Neurocomputing 2008, 71, 834–842. [Google Scholar] [CrossRef]
- Wang, W.; Zhong, S.; Liu, F. New delay-dependent stability criteria for uncertain genetic regulatory networks with time-varying delays. Neurocomputing 2012, 93, 19–26. [Google Scholar] [CrossRef]
- Yu, T.; Zhang, X.; Zhang, G.; Niu, B. Hopf bifurcation analysis for genetic regulatory networks with two delays. Neurocomputing 2015, 164, 190–200. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Wu, L. Analysis and Design of Delayed Genetic Regulatory Networks, 1st ed.; Springer: Cham, Switzerland, 2019; ISBN 978-3-030-17097-4/978-3-030-17098-1. [Google Scholar]
- Zhang, L.N.; Zhang, X.Y.; Xue, Y.; Zhang, X. New method to global exponential stability analysis for switched genetic regulatory networks with mixed delays. IEEE Trans. Nanobioscience 2020, 19, 308–314. [Google Scholar] [CrossRef] [PubMed]
- Han, Y.; Zhang, X.; Wang, Y. Asymptotic stability criteria for genetic regulatory networks with time-varying delays and reaction-diffusion terms. Circuits Syst. Signal Process 2015, 34, 3161–3190. [Google Scholar] [CrossRef]
- Fan, X.; Xue, Y.; Zhang, X.; Ma, J. Finite-time state observer for delayed reaction-diffusion genetic regulatory networks. Neurocomputing 2017, 227, 18–28. [Google Scholar] [CrossRef]
- Fan, X.; Zhang, X.; Wu, L.; Shi, M. Finite-time stability analysis of reaction-diffusion genetic regulatory networks with time-varying delays. IEEE/ACM Trans. Comput. Biol. Bioinform. 2017, 14, 868–879. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Dong, Y.; Zhong, S.; Shi, K.; Liu, F. Secondary delay-partition approach to finite-time stability analysis of delayed genetic regulatory networks with reaction-diffusion terms. Neurocomputing 2019, 359, 368–383. [Google Scholar] [CrossRef]
- Zhang, X.; Han, Y.Y.; Wu, L.G.; Wang, Y.T. State estimation for delayed genetic regulatory networks with reaction-diffusion terms. IEEE Trans. Neural Netw. Learn. Syst. 2018, 29, 299–309. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, H.H.; Yan, F.; Zhou, J. Oscillatory behaviors in genetic regulatory networks mediated by microRNA with time delays and reaction–diffusion terms. IEEE Trans. Nanobiosci. 2017, 16, 166–176. [Google Scholar] [CrossRef]
- Zou, J.P.; Xu, S.Y.; Shen, H. Finite-time robust stability of uncertain stochastic delayed reaction-diffusion genetic regulatory networks. Neurocomputing 2011, 74, 2790–2796. [Google Scholar] [CrossRef]
- Qiu, J.; Sun, K.; Yang, C.; Chen, X.; Chen, X.; Zhang, A. Finite-time stability of genetic regulatory networks with impulsive effects. Neurocomputing 2017, 219, 9–14. [Google Scholar] [CrossRef][Green Version]
- Sakthivel, R.; Raja, R.; Anthoni, S.M. Asymptotic stability of delayed stochastic genetic regulatory networks with impulses. Phys. Scr. 2010, 82, 055009. [Google Scholar] [CrossRef]
- Senthilraj, S.; Raja, R.; Zhu, Q.; Samidurai, R.; Zhou, H. Delay-dependent asymptotic stability criteria for genetic regulatory networks with impulsive perturbations. Neurocomputing 2016, 214, 981–990. [Google Scholar] [CrossRef]
- Stamov, G.; Stamov, T.; Stamova, I. On the almost periodicity in discontinuous impulsive gene regulatory networks. Math. Methods Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Benchohra, M.; Henderson, J.; Ntouyas, J. Impulsive Differential Equations and Inclusions, 1st ed.; Hindawi Publishing Corporation: New York, NY, USA, 2006; ISBN 977594550X/978-9775945501. [Google Scholar]
- Li, X.; Song, S. Impulsive Systems with Delays: Stability and Control, 1st ed.; Science Press & Springer: Singapore, 2022; ISBN 978-981-16-4686-7. [Google Scholar]
- Stamova, I.M.; Stamov, G.T. Applied Impulsive Mathematical Models, 1st ed.; Springer: Cham, Switzerland, 2016; ISBN 978-3-319-28060-8/978-3-319-28061-5. [Google Scholar]
- Li, F.; Sun, J. Asymptotic stability of a genetic network under impulsive control. Phys. Lett. A 2010, 374, 3177–3184. [Google Scholar] [CrossRef]
- Yosef, N.; Regev, A. Impulse control: Temporal dynamics in gene transcription. Cell 2011, 144, 886–896. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Yang, T. Impulsive Control Theory, 1st ed.; Springer: Berlin, Germany, 2001; ISBN 978-3-540-47710-5. [Google Scholar]
- Yang, X.; Peng, D.; Lv, X.; Li, X. Recent progress in impulsive control systems. Math. Comput. Simul. 2019, 155, 244–268. [Google Scholar] [CrossRef]
- Abbasi, Z.; Zamani, I.; Mehra, A.H.A.; Shafieirad, M.; Ibeas, A. Optimal control design of impulsive SQEIAR epidemic models with application to COVID-19. Chaos Solitons Fract. 2020, 139, 110054. [Google Scholar] [CrossRef] [PubMed]
- Chatterjee, A.N.; Al Basir, F.; Takeuchi, Y. Effect of DAA therapy in hepatitis C treatment–an impulsive control approach. Math. Biosci. Eng. 2021, 18, 1450–1464. [Google Scholar] [CrossRef]
- Liu, H.; Yu, J.; Zhu, G. Global behaviour of an age-infection structured HIV model with impulsive drug-treatment strategy. J. Theor. Biol. 2008, 253, 749–754. [Google Scholar] [CrossRef]
- Lou, J.; Lou, Y.; Wu, J. Threshold virus dynamics with impulsive antiretroviral drug effects. J. Math. Biol. 2012, 65, 623–652. [Google Scholar] [CrossRef][Green Version]
- Cao, B.; Zhang, Q.; Ye, M. Exponential stability of impulsive stochastic genetic regulatory networks with time-varying delays and reaction-diffusion. Adv. Differ. Equ. 2016, 2016, 307. [Google Scholar] [CrossRef][Green Version]
- Huang, C.; Cao, J.; Xiao, M. Hybrid control on bifurcation for a delayed fractional gene regulatory network. Chaos Solitons Fract. 2016, 87, 19–29. [Google Scholar] [CrossRef]
- Tao, B.; Xiao, M.; Sun, Q.; Cao, J. Hopf bifurcation analysis of a delayed fractional-order genetic regulatory network model. Neurocomputing 2018, 275, 677–686. [Google Scholar] [CrossRef]
- Wu, Z.; Wang, Z.; Zhou, T. Global stability analysis of fractional-order gene regulatory networks with time delay. Int. J. Biomath. 2019, 12, 1950067. [Google Scholar] [CrossRef]
- Wu, Z.; Wang, Z.; Zhou, T.; Tan, S. Global synchronization and anti-synchronization of fractional-order complex-valued gene regulatory networks with time-varying delays. IEEE Access 2020, 8, 150555–150572. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, J.; Ai, Z. A novel stability criterion of the time-lag fractional-order gene regulatory network system for stability analysis. Commun. Nonlinear Sci. Numer. Simul. 2019, 66, 96–108. [Google Scholar] [CrossRef]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Methods, 1st ed.; World Scientific: Singapore, 2012; ISBN 978-981-4355-20-9. [Google Scholar]
- Kilbas, A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, 1st ed.; Elsevier: New York, NY, USA, 2006; ISBN 9780444518323. [Google Scholar]
- Petráš, I. Fractional-Order Nonlinear Systems, 1st ed.; Springer: Heidelberg, Germany; Dordrecht, The Netherlands; London UK; New York, NY, USA, 2011; ISBN 978-3-642-18101-6. [Google Scholar]
- Podlubny, I. Fractional Differential Equations, 1st ed.; Academic Press: San Diego, CA, USA, 1999; ISBN 558840-2. [Google Scholar]
- Stamova, I.M.; Stamov, G.T. Functional and Impulsive Differential Equations of Fractional Order: Qualitative Analysis and Applications, 1st ed.; CRC Press/Taylor and Francis Group: Boca Raton, FL, USA, 2017; ISBN 9781498764834. [Google Scholar]
- Odibat, Z.; Baleanu, D. Nonlinear dynamics and chaos in fractional differential equations with a new generalized Caputo fractional derivative. Chin. J. Phys. 2022, 77, 1003–1014. [Google Scholar] [CrossRef]
- Sun, H.G.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y.Q. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Zhou, Y.; Ionescu, C.; Tenreiro Machado, J.A. Fractional dynamics and its applications. Nonlinear Dyn. 2015, 80, 1661–1664. [Google Scholar] [CrossRef][Green Version]
- Baleanu, D.; Wu, G.C. Some further results of the Laplace transform for variable–order fractional difference equations. Fract. Calc. Appl. Anal. 2019, 22, 1641–1654. [Google Scholar] [CrossRef]
- Wu, G.C.; Deng, Z.G.; Baleanu, D.; Zeng, D.Q. New variable-order fractional chaotic systems for fast image encryption. Chaos 2019, 29, 083103. [Google Scholar] [CrossRef]
- Wu, G.C.; Zeng, D.Q.; Baleanu, D. Fractional impulsive differential equations: Exact solutions, integral equations and short memory case. Fract. Calc. Appl. Anal. 2019, 22, 180–192. [Google Scholar] [CrossRef]
- Arjunan, M.M.; Abdeljawad, T.; Anbalagan, P. Impulsive effects on fractional order time delayed gene regulatory networks: Asymptotic stability analysis. Chaos Solitons Fract. 2022, 154, 111634. [Google Scholar] [CrossRef]
- Narayanan, G.; Syed Ali, M.; Alsulami, H.; Ahmad, B.; Trujillo, J.J. A hybrid impulsive and sampled-data control for fractional-order delayed reaction-diffusion system of mRNA and protein in regulatory mechanisms. Commun. Nonlinear Sci. Numer. Simul. 2022, 111, 106374. [Google Scholar] [CrossRef]
- Stamov, T.; Stamova, I. Design of impulsive controllers and impulsive control strategy for the Mittag–Leffler stability behavior of fractional gene regulatory networks. Neurocomputing 2021, 424, 54–62. [Google Scholar] [CrossRef]
- Stamova, I.; Stamov, G. Lyapunov approach for almost periodicity in impulsive gene regulatory networks of fractional order with time-varying delays. Fractal Fract. 2021, 5, 268. [Google Scholar] [CrossRef]
- Yue, D.; Guan, Z.H.; Chi, M.; Hu, B.; Liu, Z.W.; Chen, J. Stability and Hopf bifurcation of fractional genetic regulatory networks with diffusion. IFAC PapersOnLine 2017, 50, 10443–10448. [Google Scholar] [CrossRef]
- Alshammari, S.; Al-Sawalha, M.M.; Humaidi, J.R. Fractional view study of the brusselator reaction–diffusion model occurring in chemical reactions. Fractal Fract. 2023, 7, 108. [Google Scholar] [CrossRef]
- Lu, J.G. Global exponential stability and periodicity of reaction-diffusion delayed recurrent neural networks with Dirichlet boundary conditions. Chaos Solitons Fract. 2008, 35, 116–125. [Google Scholar] [CrossRef]
- Stamova, I.; Stamov, G. Mittag–Leffler synchronization of fractional neural networks with time-varying delays and reaction-diffusion terms using impulsive and linear controllers. Neural Netw. 2017, 96, 22–32. [Google Scholar] [CrossRef]
- Yang, S.; Jiang, H.; Hu, C.; Yu, J. Synchronization for fractional-order reaction-diffusion competitive neural networks with leakage and discrete delays. Neurocomputing 2021, 436, 47–57. [Google Scholar] [CrossRef]
- Benchohra, M.; Henderson, J.; Ntouyas, S.K.; Ouahab, A. Impulsive functional differential equations with variable times. Comput. Math. Appl. 2004, 47, 1659–1665. [Google Scholar] [CrossRef][Green Version]
- Song, Q.; Yang, X.; Li, C.; Huang, T.; Chen, X. Stability analysis of nonlinear fractional-order systems with variable-time impulses. J. Franklin Inst. 2017, 354, 2959–2978. [Google Scholar] [CrossRef]
- Yang, X.; Li, C.; Song, Q.; Huang, T.; Chen, X. Mittag–Leffler stability analysis on variable-time impulsive fractional-order neural networks. Neurocomputing 2016, 207, 276–286. [Google Scholar] [CrossRef]
- Yilmaz, E. Almost periodic solutions of impulsive neural networks at non-prescribed moments of time. Neurocomputing 2014, 141, 148–152. [Google Scholar] [CrossRef]
- Duan, L.; Di, F.; Wang, Z. Existence and global exponential stability of almost periodic solutions of genetic regulatory networks with time-varying delays. J. Exp. Theor. Artif. Intell. 2020, 32, 453–463. [Google Scholar] [CrossRef]
- Du, W.S.; Kostić, M.; Pinto, M. Almost periodic functions and their applications: A survey of results and perspectives. J. Math. 2021, 2021, 1–21. [Google Scholar] [CrossRef]
- Levitan, M.; Zhikov, V.V. Almost Periodic Functions and Differential Equations, 1st ed.; Cambridge University Press: London, UK, 1982; ISBN 9780521244077. [Google Scholar]
- Fink, A.M. Almost Periodic Differential Equations, 1st ed.; Springer: Berlin, Germany, 1974; ISBN 978-3-540-38307-9. [Google Scholar]
- Samoilenko, A.M.; Perestyuk, N.A. Impulsive Differential Equations, 1st ed.; World Scientific: River Edge, NJ, USA, 1995; ISBN 978-981-02-2416-5. [Google Scholar]
- Stamov, G.T. Almost Periodic Solutions of Impulsive Differential Equations, 1st ed.; Springer: Heidelberg, Germany, 2012; ISBN 978-3-642-27545-6. [Google Scholar]
- Cao, J.; Stamov, G.; Stamova, I.; Simeonov, S. Almost periodicity in reaction-diffusion impulsive fractional neural networks. IEEE Trans. Cybern. 2021, 51, 151–161. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Ruan, C.; Li, B. Existence and finite-time stability of Besicovitch almost periodic solutions of fractional-order quaternion-valued neural networks with time-varying delays. Neural Process. Lett. 2022, 54, 2127–2141. [Google Scholar] [CrossRef]
- Rakkiyappan, R.; Velmurugan, G.; Soundharajan, P.; Joo, Y.H. Almost periodic dynamics of memristive inertial neural networks with mixed delays. Inf. Sci. 2020, 536, 332–350. [Google Scholar]
- Stamov, G.; Stamova, I. Impulsive fractional-order neural networks with time-varying delays: Almost periodic solutions. Neural Comput. Appl. 2017, 28, 3307–3316. [Google Scholar] [CrossRef]
- Wan, P.; Sun, D.; Zhao, M.; Zhao, H. Monostability and multistability for almost-periodic solutions of fractional-order neural networks with unsaturating piecewise linear activation functions. IEEE Trans. Neural. Netw. Learn. Syst. 2020, 12, 5138–5152. [Google Scholar] [CrossRef] [PubMed]
- Xue, Y.; Xie, X.; Lin, Q. Almost periodic solutions of a commensalism system with Michaelis–Menten type harvesting on time scales. Open Math. 2019, 17, 1503–1514. [Google Scholar] [CrossRef]
- Kaslik, E.; Sivasundaram, S. Non-existence of periodic solutions in fractional-order dynamical systems and a remarkable difference between integer and fractional-order derivatives of periodic functions. Nonlinear Anal. Real World Appl. 2012, 13, 1489–1497. [Google Scholar] [CrossRef][Green Version]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stamov, T.; Stamov, G.; Stamova, I. Fractional-Order Impulsive Delayed Reaction-Diffusion Gene Regulatory Networks: Almost Periodic Solutions. Fractal Fract. 2023, 7, 384. https://doi.org/10.3390/fractalfract7050384
Stamov T, Stamov G, Stamova I. Fractional-Order Impulsive Delayed Reaction-Diffusion Gene Regulatory Networks: Almost Periodic Solutions. Fractal and Fractional. 2023; 7(5):384. https://doi.org/10.3390/fractalfract7050384
Chicago/Turabian StyleStamov, Trayan, Gani Stamov, and Ivanka Stamova. 2023. "Fractional-Order Impulsive Delayed Reaction-Diffusion Gene Regulatory Networks: Almost Periodic Solutions" Fractal and Fractional 7, no. 5: 384. https://doi.org/10.3390/fractalfract7050384
APA StyleStamov, T., Stamov, G., & Stamova, I. (2023). Fractional-Order Impulsive Delayed Reaction-Diffusion Gene Regulatory Networks: Almost Periodic Solutions. Fractal and Fractional, 7(5), 384. https://doi.org/10.3390/fractalfract7050384






