Approximate Analytical Methods for a Fractional-Order Nonlinear System of Jaulent–Miodek Equation with Energy-Dependent Schrödinger Potential
Abstract
1. Introduction
2. Basic Preliminaries
3. The Methodology of Adomian Decomposition Transform Method
4. The Producer of Yang Variational Iteration Method
5. Implementation of Techniques
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Said, L.A.; Radwan, A.G.; Madian, A.H.; Soliman, A.M. Three fractional-order-capacitors-based oscillators with controllable phase and frequency. J. Circuits Syst. Comput. 2017, 26, 1750160. [Google Scholar] [CrossRef]
- Said, L.A.; Madian, A.H.; Radwan, A.G.; Soliman, A.M. Fractional order oscillator with independent control of phase and frequency. In 2014 2nd International Conference on Electronic Design (ICED). In Proceedings of the 2014 2nd International Conference on Electronic Design (ICED), Penang, Malaysia, 19–21 August 2014; pp. 224–229. [Google Scholar]
- Machado, J.T.; Kiryakova, V.; Mainardi, F. Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef]
- Sabatier, J.A.T.M.J.; Agrawal, O.P.; Machado, J.T. Advances in Fractional Calculus; Springer: Dordrecht, The Netherlands, 2007; p. 9. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Method; World Scientific: Singapore, 2012; Volume 3. [Google Scholar]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 54, 3413–3442. [Google Scholar] [CrossRef]
- Al-Sawalha, M.M.; Alshehry, A.S.; Nonlaopon, K.; Shah, R.; Ababneh, O.Y. Fractional view analysis of delay differential equations via numerical method. AIMS Math. 2022, 7, 20510–20523. [Google Scholar] [CrossRef]
- Mukhtar, S.; Shah, R.; Noor, S. The Numerical Investigation of a Fractional-Order Multi-Dimensional Model of Navier-Stokes Equation via Novel Techniques. Symmetry 2022, 14, 1102. [Google Scholar] [CrossRef]
- Al-Sawalha, M.M.; Alshehry, A.S.; Nonlaopon, K.; Shah, R.; Ababneh, O.Y. Approximate analytical solution of time-fractional vibration equation via reliable numerical algorithm. AIMS Math. 2022, 7, 19739–19757. [Google Scholar] [CrossRef]
- Al-Sawalha, M.M.; Khan, A.; Ababneh, O.Y.; Botmart, T. Fractional view analysis of Kersten-Krasil’shchik coupled KdV-mKdV systems with non-singular kernel derivatives. AIMS Math. 2022, 7, 18334–18359. [Google Scholar] [CrossRef]
- Kai, Y.; Chen, S.; Zhang, K.; Yin, Z. Exact solutions and dynamic properties of a nonlinear fourth-order time-fractional partial differential equation. Waves Random Complex Media 2022, 1–12. [Google Scholar] [CrossRef]
- Jaulent, M.; Miodek, I. Nonlinear evolution equations associated with energy-dependent Schrodinger potentials. Lett. Math. Phys. 1976, 1, 243–250. [Google Scholar] [CrossRef]
- Jaulent, M. Inverse scattering problems in absorbing media. J. Math. Phys. 1976, 17, 1351–1360. [Google Scholar] [CrossRef]
- Hong, T.; Wang, Y.Z.; Huo, Y.S. Bogoliubov quasiparticles carried by dark solitonic excitations in non-uniform Bose-Einstein condensates. Chin. Phys. Lett. 1998, 15, 550–552. [Google Scholar] [CrossRef]
- Ma, W.X.; Li, C.X.; He, J. A second Wronskian formulation of the Boussinesq equation. Nonlinear Anal. Theory Methods Appl. 2009, 70, 4245–4258. [Google Scholar] [CrossRef]
- Jie-Fang, Z. Multiple soliton solutions of the dispersive long-wave equations. Chin. Phys. Lett. 1999, 16, 4. [Google Scholar]
- Das, G.C.; Sarma, J.; Uberoi, C. Explosion of a soliton in a multicomponent plasma. Phys. Plasmas 1997, 4, 2095–2100. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D. Nonlinear Fractional Jaulent-Miodek and Whitham-Broer-Kaup Equations within Sumudu Transform. Abstr. Appl. Anal. 2013, 2013, 160681. [Google Scholar] [CrossRef]
- Gupta, A.K.; Ray, S.S. An investigation with Hermite Wavelets for accurate solution of fractional Jaulent-Miodek equation associated with energy-dependent Schrodinger potential. Appl. Math. Comput. 2015, 270, 458–471. [Google Scholar] [CrossRef]
- Majlesi, A.; Ghehsareha, H.R.; Zaghian, A. On the fractional Jaulent-Miodek equation associated with energy-dependent Schrodinger potential: Lie symmetry reductions, explicit exact solutions and conservation laws. Eur. Phys. J. Plus 2015, 132, 1–13. [Google Scholar] [CrossRef]
- Yildirim, A.; Kelleci, A. Numerical simulation of the Jaulent-Miode equation by He’s homotopy perturbation method. World Appl. Sci. J. 2009, 7, 84–89. [Google Scholar]
- He, J.H.; Zhang, L.N. Generalized solitary solution and compacton-like solution of the Jaulent- Miodek equations using the Exp-function method. Phys. Lett. A 2008, 372, 1044–1047. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Domairry, G.; Dinarvand, S. The homotopy analysis method for explicit analytical solutions of Jaulent-Miodek equations. Numer. Meth. Partial Differ. Equ. 2009, 25, 430–439. [Google Scholar] [CrossRef]
- Keskin, Y.; Oturanc, G. Reduced differential transform method for partial differential equations. Int. J. Nonlienar Sci. Numer. Simul. 2009, 10, 741–749. [Google Scholar] [CrossRef]
- Saravanan, A.; Magesh, N. An efficient computational technique for solving the Fokker-Planck equation with space and time fractional derivatives. J. King Saud Univ.-Sci. 2016, 28, 160–166. [Google Scholar] [CrossRef]


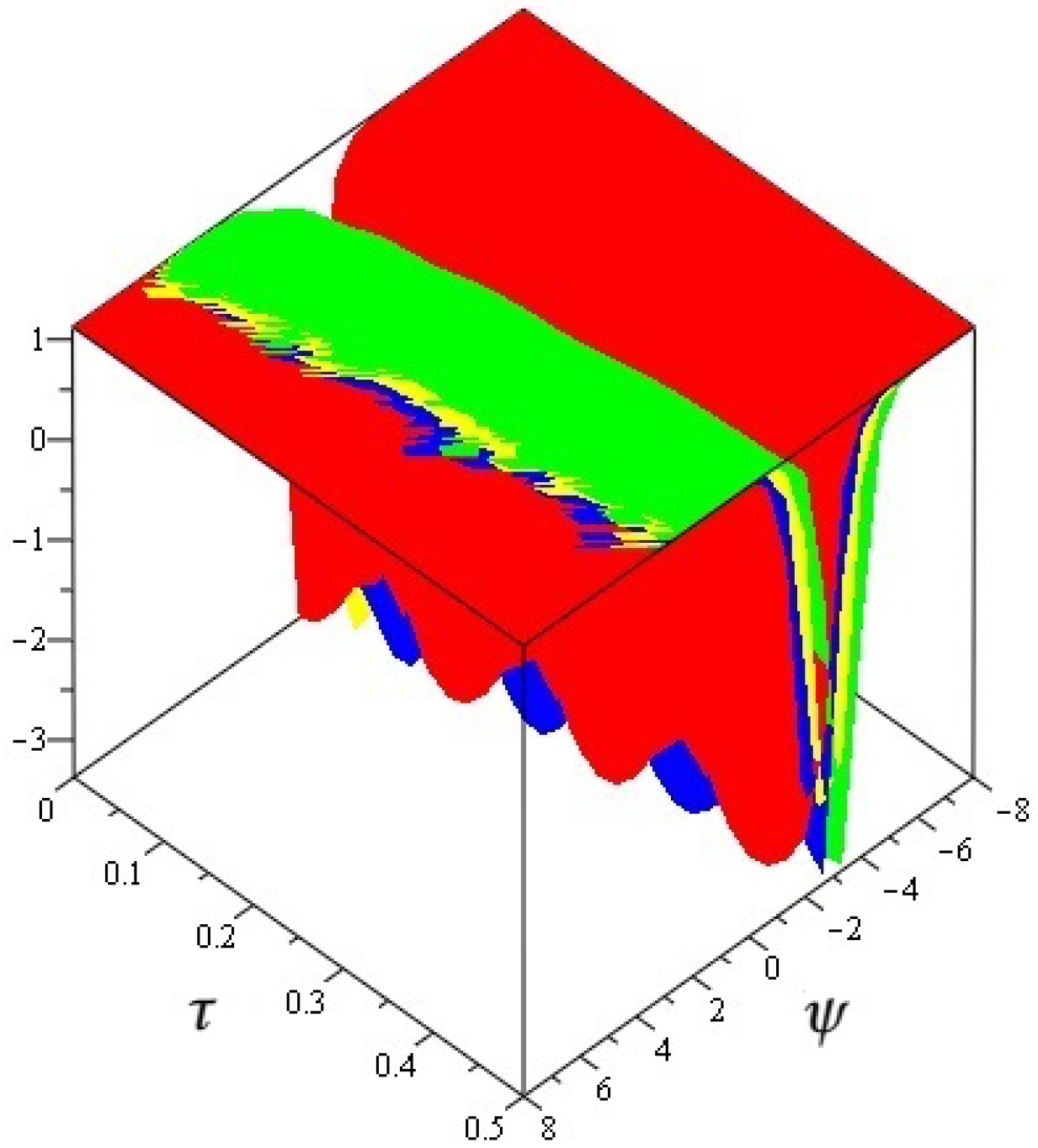
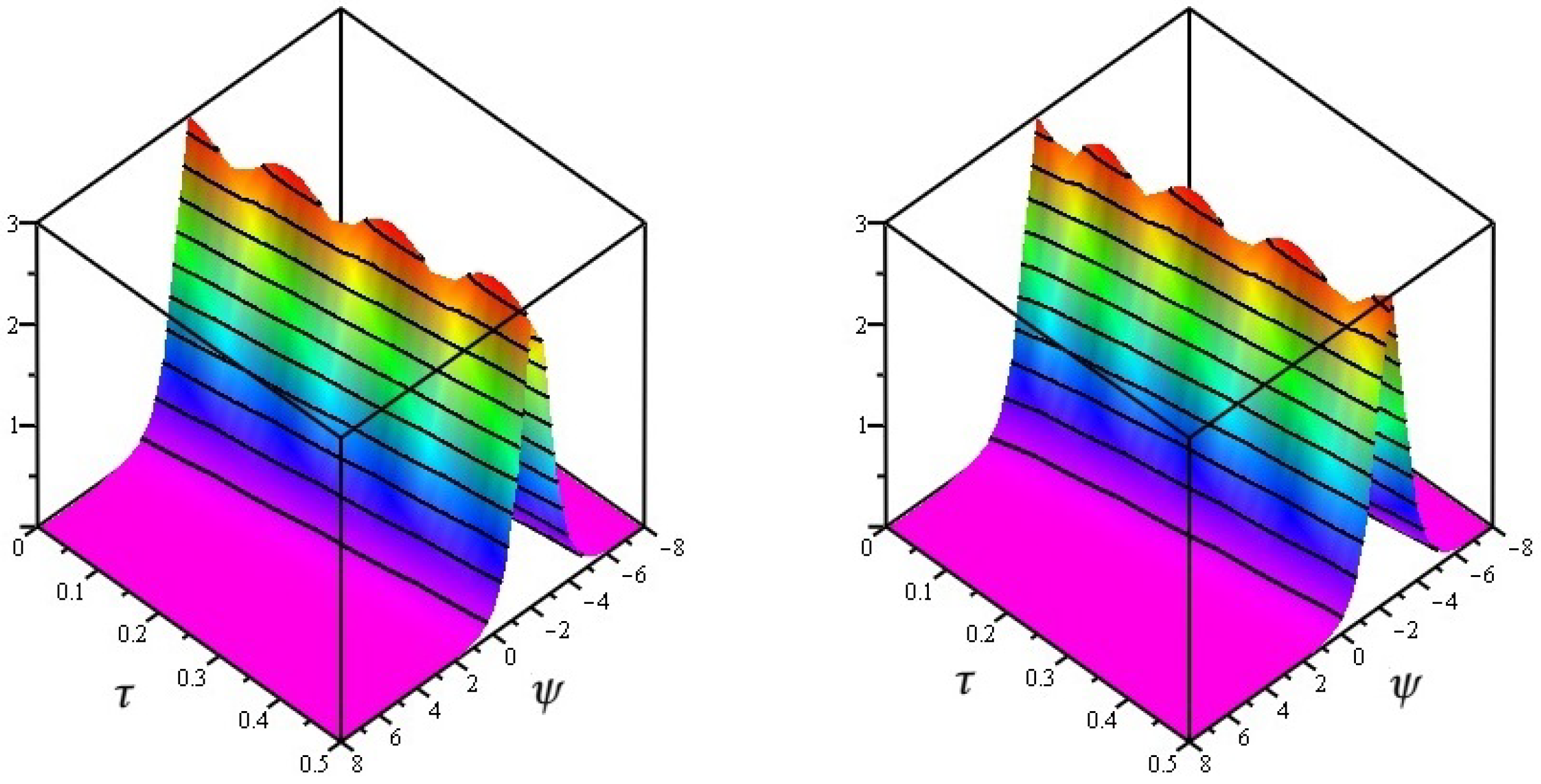
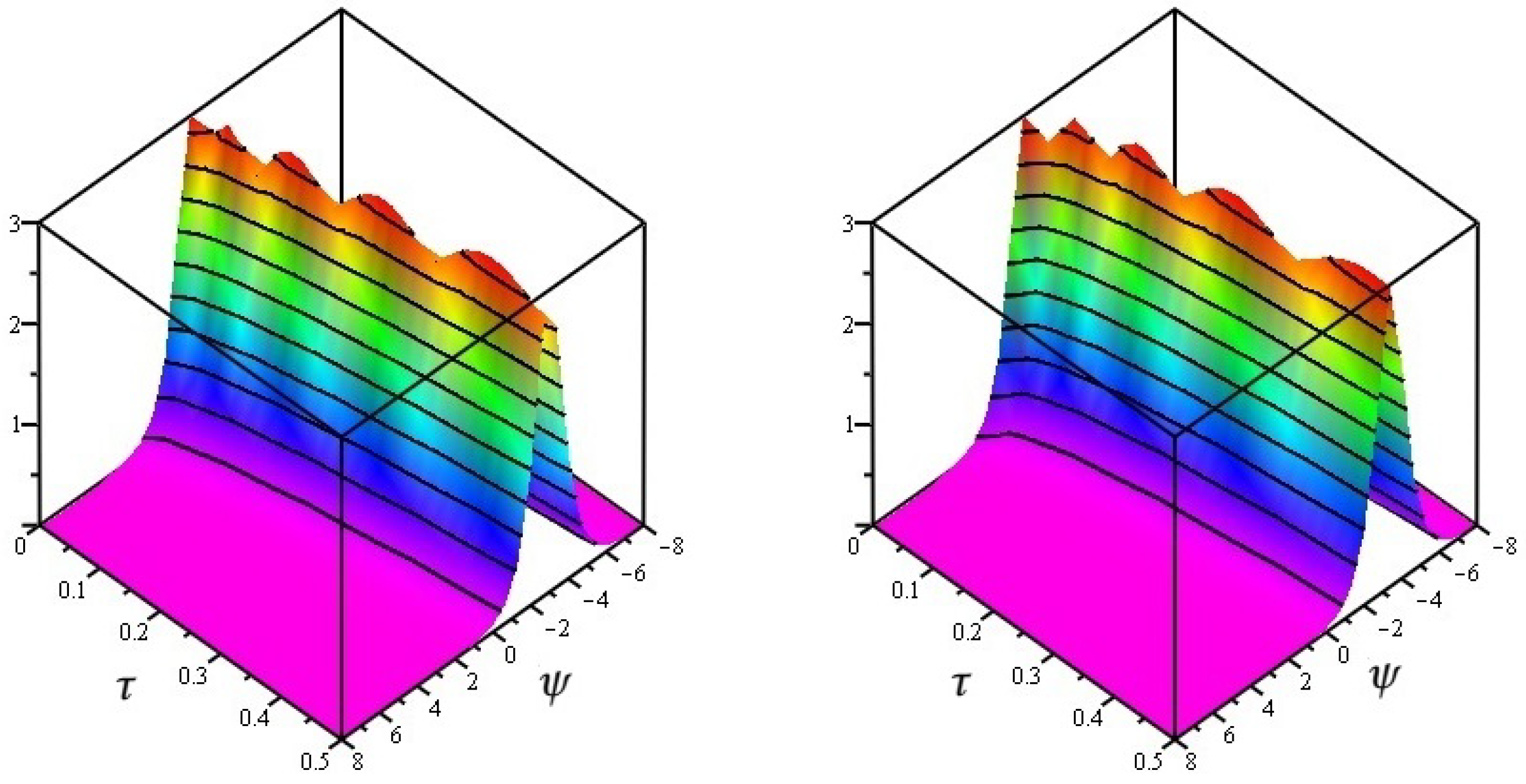
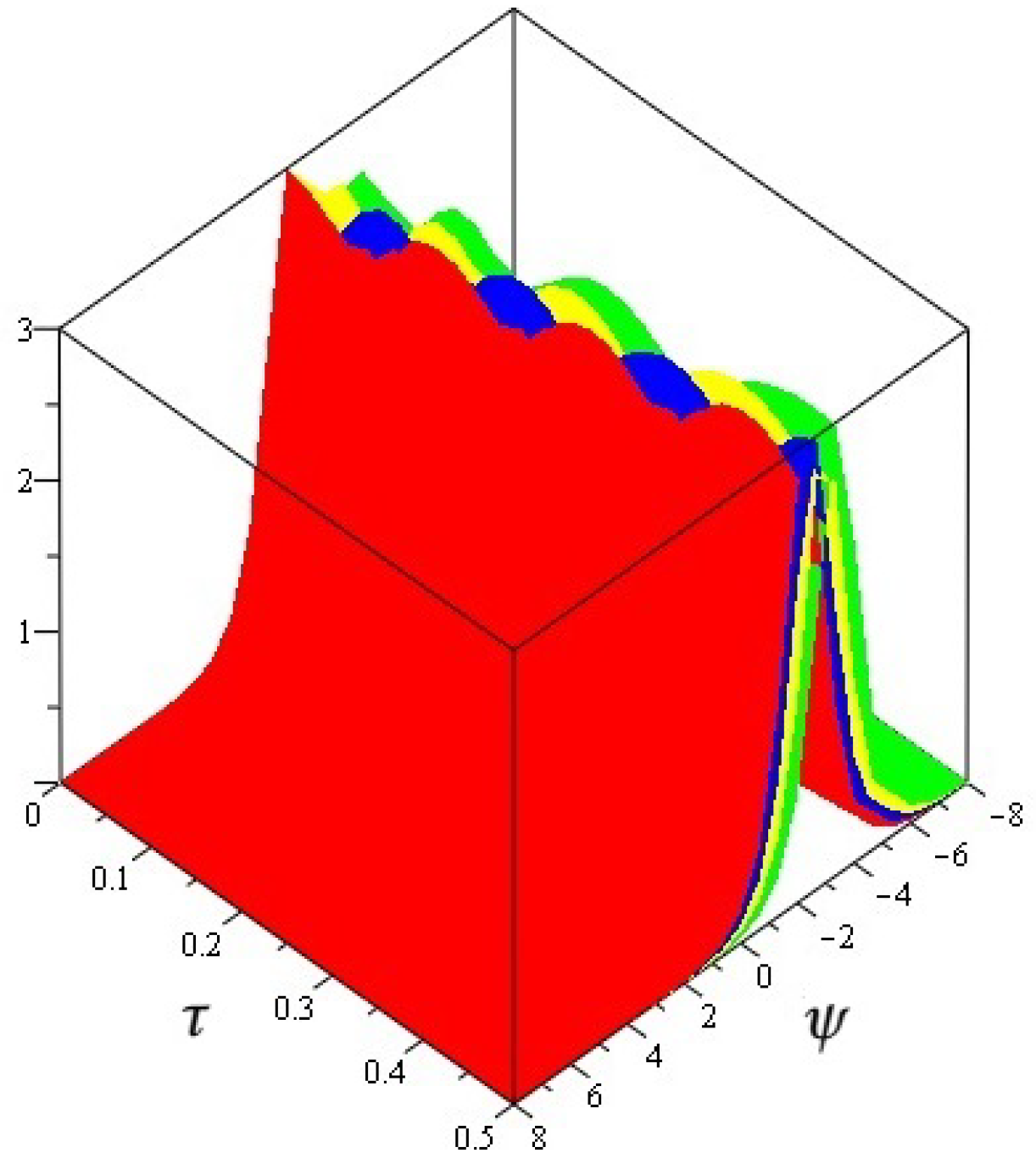
| x | |||||
|---|---|---|---|---|---|
| 0.2 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 | |||||
| 0.4 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 | |||||
| 0.6 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 | |||||
| 0.8 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 |
| x | |||||
|---|---|---|---|---|---|
| 0.2 | |||||
| 1 | |||||
| 0.4 | |||||
| 1 | |||||
| 0.6 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 | |||||
| 0.8 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 | |||||
| 1 | 0.2 | ||||
| 0.4 | |||||
| 0.6 | |||||
| 0.8 | |||||
| 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alshammari, S.; Al-Sawalha, M.M.; Shah, R. Approximate Analytical Methods for a Fractional-Order Nonlinear System of Jaulent–Miodek Equation with Energy-Dependent Schrödinger Potential. Fractal Fract. 2023, 7, 140. https://doi.org/10.3390/fractalfract7020140
Alshammari S, Al-Sawalha MM, Shah R. Approximate Analytical Methods for a Fractional-Order Nonlinear System of Jaulent–Miodek Equation with Energy-Dependent Schrödinger Potential. Fractal and Fractional. 2023; 7(2):140. https://doi.org/10.3390/fractalfract7020140
Chicago/Turabian StyleAlshammari, Saleh, M. Mossa Al-Sawalha, and Rasool Shah. 2023. "Approximate Analytical Methods for a Fractional-Order Nonlinear System of Jaulent–Miodek Equation with Energy-Dependent Schrödinger Potential" Fractal and Fractional 7, no. 2: 140. https://doi.org/10.3390/fractalfract7020140
APA StyleAlshammari, S., Al-Sawalha, M. M., & Shah, R. (2023). Approximate Analytical Methods for a Fractional-Order Nonlinear System of Jaulent–Miodek Equation with Energy-Dependent Schrödinger Potential. Fractal and Fractional, 7(2), 140. https://doi.org/10.3390/fractalfract7020140








