Numerical Solutions of the Multi-Space Fractional-Order Coupled Korteweg–De Vries Equation with Several Different Kernels
Abstract
:1. Introduction
2. The First-Kind Chebyshev Polynomials and Function Approximations
3. Implementation the Proposed Method and Numerical Simulation
3.1. Fractional Derivative Involving the Power-Law Kernel
3.2. Fractional Derivative Involving the Exponential-Decay Kernel
3.3. Fractional Derivative Involving the Mittag–Leffler Kernel
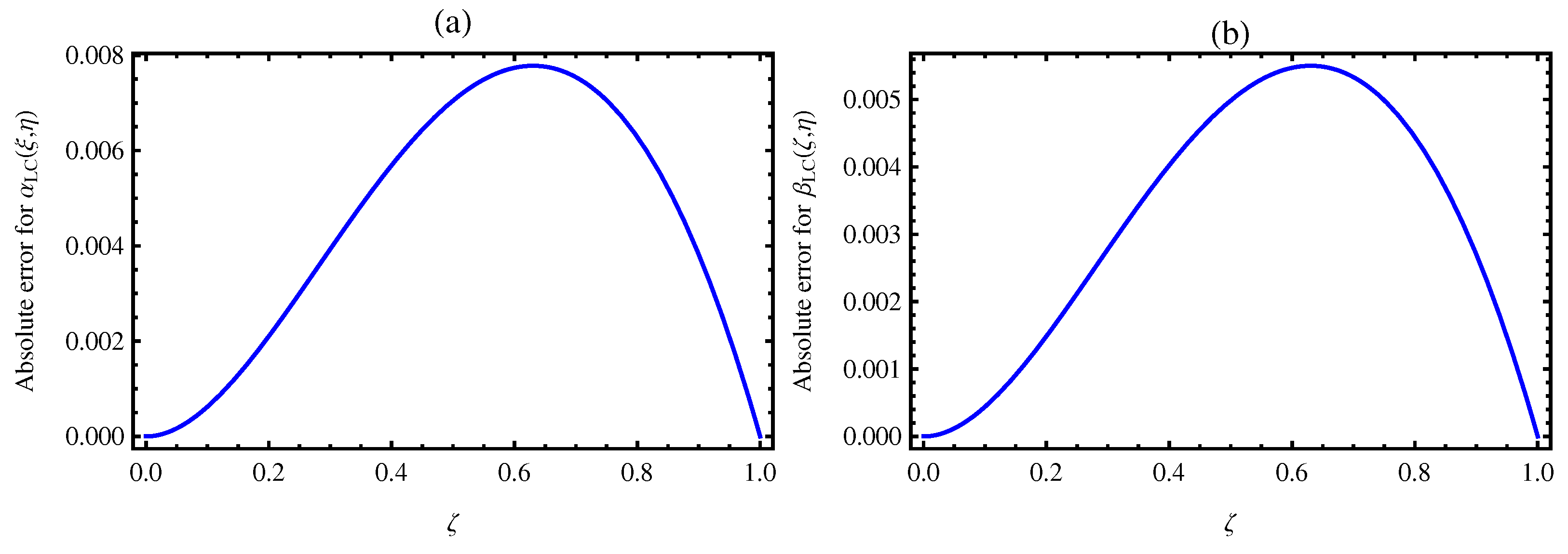
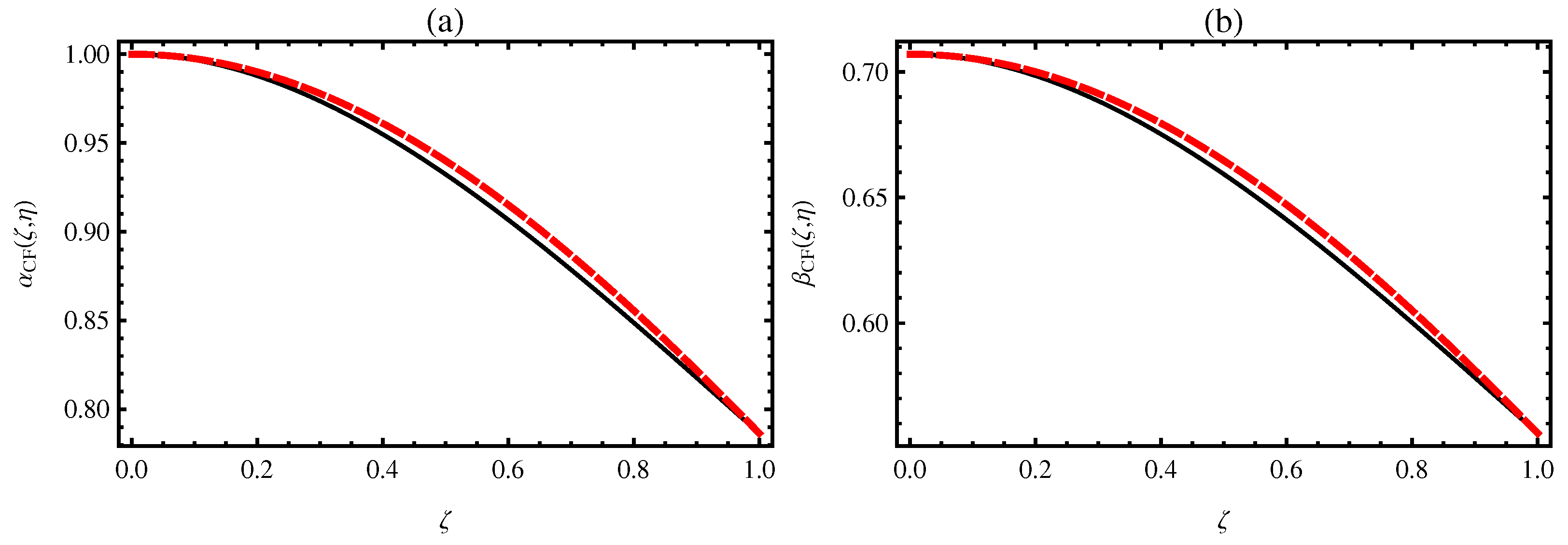
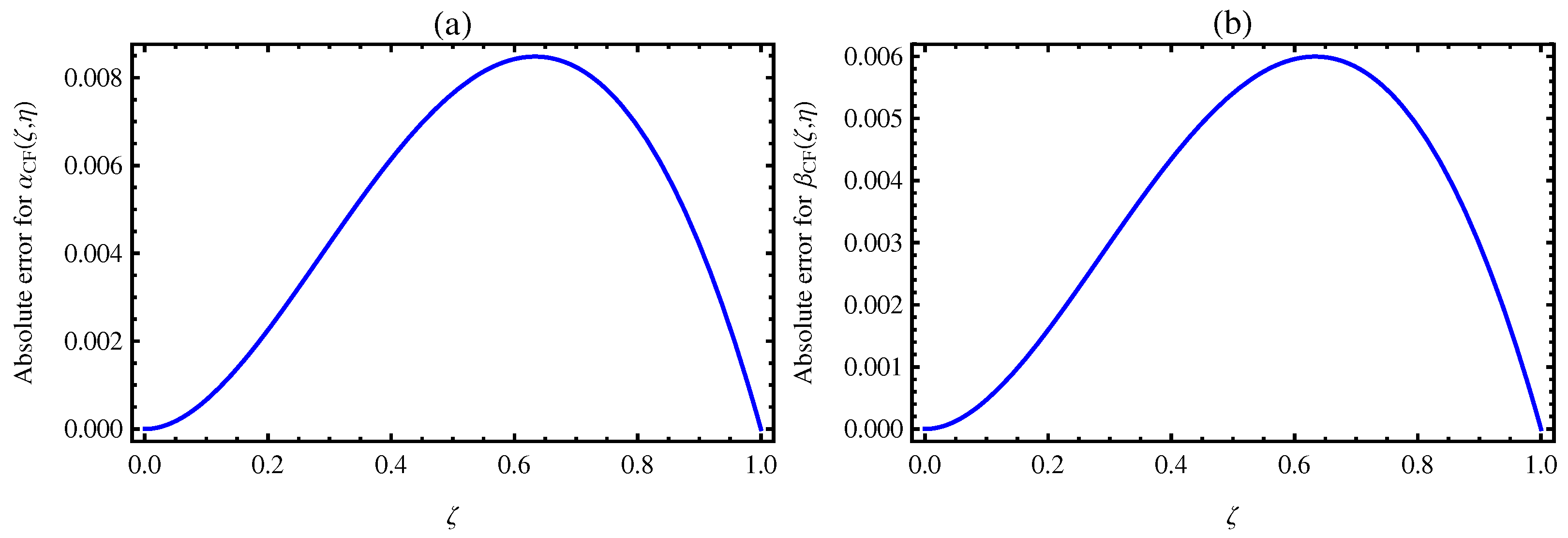
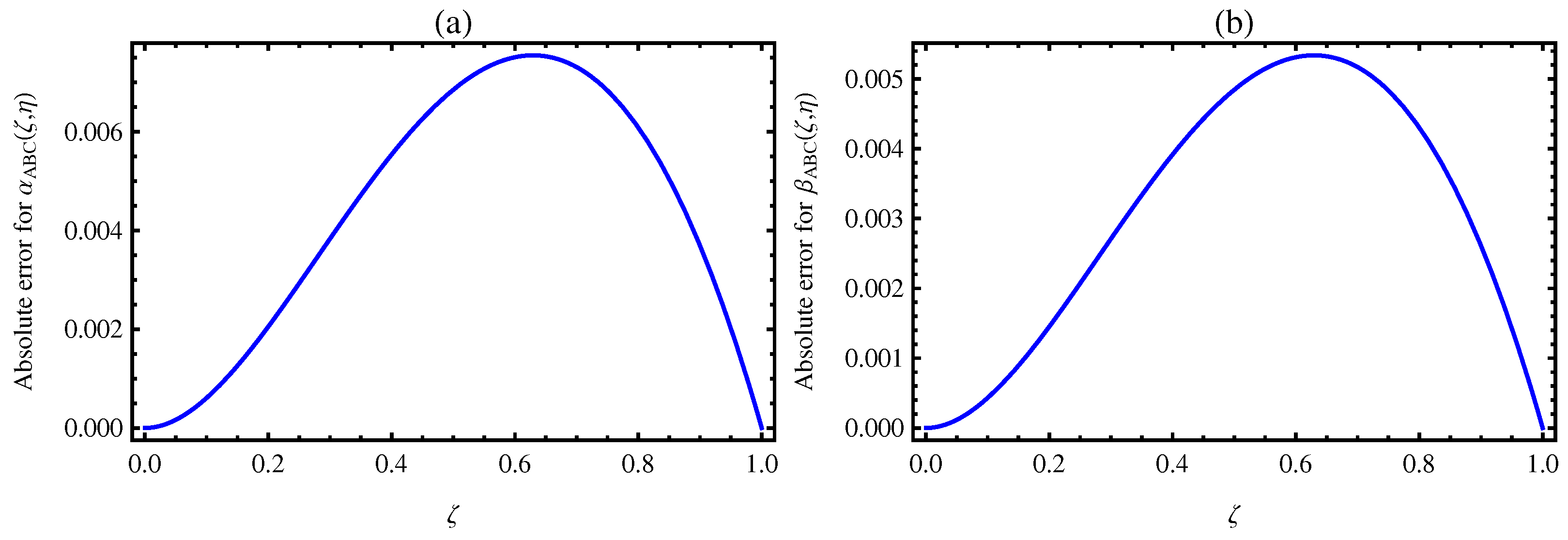
4. Numerical Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theoryand Applications of Fractional Differential Equations; North-Holland Mathematical Studies; Elsevier (North-Holland) Science Publishers: Amsterdam, The Netherlands; London, UK; New York, NY, USA, 2006; Volume 204. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Mathematics in Science and Engineering; Academic Press: New York, NY, USA; London, UK; Sydney, Australia; Tokyo, Japan; Toronto, ON, Canada, 1999; Volume 198. [Google Scholar]
- Hilfer, R. (Ed.) Applications of Fractional Calculus in Physics; World Scientific Publishing Company: Singapore; Hoboken, NJ, USA; London, UK; Hong Kong, China, 2000. [Google Scholar]
- Shishkina, E.; Sitnik, S. Transmutations, Singular and Fractional Differential Equations with Applications to Mathematical Physics; Mathematics in Science and Engineering; Academic Press (Elsevier Science Publishers): New York, NY, USA; London, UK; Toronto, ON, Canada, 2020. [Google Scholar]
- Anastassiou, G.A. Generalized Fractional Calculus: New Advancements and Applications; Studies in Systems, Decision and Control; Springer: Cham, Switzerland, 2021; Volume 305. [Google Scholar]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific Publishing Company: Singapore; Hoboken, NJ, USA; London, UK; Hong Kong, China, 2010. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach: Yverdon, Switzerland, 1993. [Google Scholar]
- Liouville, J. Mémoire sur quelques quéstions de géometrie et de mécanique, et sur un nouveau genre de calcul pour résoudre ces quéstions. J. École Polytech. 1832, 13, 1–69. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley and Sons: New York, NY, USA; Chischester, UK; Brisbane, Australia; Toronto, ON, Canada,, 1993. [Google Scholar]
- Sabir, Z.; Said, S.B.; Al-Mdallal, Q. A fractional order numerical study for the influenza disease mathematical model. Alex. Eng. J. 2023, 65, 615–626. [Google Scholar] [CrossRef]
- Alqhtani, M.; Owolabi, K.M.; Saad, K.M. Spatiotemporal (target) patterns in sub-diffusive predator-prey system with the Caputo operator. Chaos Solitons Fractals 2022, 160, 112267. [Google Scholar] [CrossRef]
- Abdon, M.A.; Hasan, F.L. Advantages of the differential equations for solving problems in mathematical physics with symbolic computation. Math. Model. Eng. Probl. 2022, 9, 268–276. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Saad, K.M.; Hamanah, W.M. Certain new models of the multi-space fractal-fractional Kuramoto-Sivashinsky and Korteweg-de Vries equations. Mathematics 2022, 10, 1089. [Google Scholar] [CrossRef]
- Waheed, W.; Deng, G.; Liu, B. Discrete Laplacian operator and its Applications in signal processing. IEEE ACCESS 2020, 8, 89692–89707. [Google Scholar] [CrossRef]
- Lin, Z.; Wang, H. Modeling and application of fractional-order economic growth model with time delay. Fractal Fract. 2021, 5, 74. [Google Scholar] [CrossRef]
- Pakhira, R.; Ghosh, U.; Garg, H. An inventory model for partial backlogging items with memory effect. Soft Comput. 2023, 27, 9533–9550. [Google Scholar] [CrossRef]
- Korteweg, D.J.; de Vries, G. On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Philos. Mag. 1985, 39, 422–443. [Google Scholar] [CrossRef]
- Wu, Y.; Geng, X.; Hu, X.; Zhu, S. A generalized Hirota-Satsuma coupled Korteweg-de Vries equation and Miura transformations. Phys. Lett. A 1999, 255, 259–264. [Google Scholar] [CrossRef]
- Hirota, R.; Satsuma, J. Soliton solutions of a coupled Korteweg-de Vries equation. Phys. Lett. A 1981, 85, 407–418. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Mandal, H.; Bira, B. Lie symmetry and exact solution of the time-fractional Hirota-Satsuma Korteweg-de Vries system. Russ. J. Math. Phys. 2021, 28, 284–292. [Google Scholar] [CrossRef]
- Akinyemi, L.; Iyiola, O.S. A reliable technique to study nonlinear time-fractional coupled Korteweg-de Vries equations. Adv. Differ. Equ. 2020, 169, 2020. [Google Scholar] [CrossRef]
- Albuohimad, B.; Adibi, H.; Kazem, S. A numerical solution of time-fractional coupled Korteweg-de Vries equation by using spectral collection method. Ain Shams Eng. J. 2018, 9, 1897–1905. [Google Scholar] [CrossRef]
- Dabiri, A.; Butcher, E.A. Numerical solution of multi-order fractional differential equations with multiple delays via spectral collocation methods. Appl. Math. Model. 2018, 56, 424–448. [Google Scholar] [CrossRef]
- Dabiri, A.; Butcher, E.A. Efficient modified Chebyshev differentiation matrices for fractional differential equations. Commun. Nonlinear Sci. Numer. Simul. 2017, 50, 284–310. [Google Scholar] [CrossRef]
- Dabiri, A.; Butcher, E.A. Stable fractional Chebyshev differentiation matrix for the numerical solution of multi-order fractional differential equations. Nonlinear Dyn. 2017, 90, 185–201. [Google Scholar] [CrossRef]
- Yang, X.-J.; Hristov, J.; Srivastava, H.M.; Ahmad, B. Modelling fractal waves on shallow water surfaces via local fractional Korteweg-de Vries equation. Abstr. Appl. Anal. 2014, 2014, 278672. [Google Scholar] [CrossRef]
- Baleanu, D.; Shiri, B.; Srivastava, H.M.; Al Qurashi, M. A Chebyshev spectral method based on operational matrix for fractional differential equations involving non-singular Mittag-Leffler kernel. Adv. Differ. Equ. 2018, 2018, 353. [Google Scholar] [CrossRef]
- Hadhoud, A.R.; Srivastava, H.M.; Rageh, A.A.M. Non-polynomial B-spline and shifted Jacobi spectral collocation techniques to solve time-fractional nonlinear coupled Burgers’ equations numerically. Adv. Differ. Equ. 2021, 2021, 439. [Google Scholar] [CrossRef]
- Khader, M.M.; Saad, K.M. A numerical approach for solving the fractional Fisher equation using Chebyshev spectral collocation method. Chaos Solitons Fractals 2018, 110, 169–177. [Google Scholar] [CrossRef]
- Khader, M.M.; Saad, K.M.; Hammouch, Z.; Baleanu, D. A spectral collocation method for solving fractional KdV and KdV-Burgers equations with non-singular kernel derivatives. Appl. Numer. Math. 2021, 161, 137–146. [Google Scholar] [CrossRef]
- Khader, M.M.; Saad, K.M.; Gómez-Aguilar, J.F.; Baleanu, D. Numerical solutions of the fractional Fisher’s type equations with Atangana-Baleanu fractional derivative by using spectral collocation methods. Chaos 2019, 29, 023116. [Google Scholar]
- Szegö, G. Orthogonal Polynomials, 4th ed.; American Mathematical Society Colloquium Publications; American Mathematical Society: Providence, RI, USA, 1975; Volume 23. [Google Scholar]
- Mason, J.C.; Handscomb, D.C. Chebyshev Polynomials; Chapman and Hall (CRC Press): Baton Roca, FL, USA, 2003. [Google Scholar]
- Srivastava, H.M. An introductory overview of fractional-calculus operators based upon the Fox-Wright and related higher transcendental functions. J. Adv. Eng. Comput. 2021, 5, 135–166. [Google Scholar] [CrossRef]
- Sweilam N, H.; Khader, M.M. A Chebyshev pseudo-spectral method for solving fractional integro-differential equations. ANZIAM J. 2010, 51, 464–475. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 1, 1–13. [Google Scholar]
- Loh, J.R.; Isah, A.; Phang, C.; Toh, Y.T. On the new properties of Caputo-Fabrizio operator and its application in deriving shifted Legendre operational matrix. Appl. Numer. Math. 2018, 132, 138–153. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D. New fractional derivative with non-local and non-singular kernel. Therm. Sci. 2016, 20, 757–763. [Google Scholar] [CrossRef]
- Abdeljawad, T.; Baleanu, D. Integration by parts and its applications of a new nonlocal fractional derivative with Mittag-Leffler nonsingular kernel. J. Nonlinear Sci. Appl. 2017, 9, 1098–1107. [Google Scholar] [CrossRef]
- Srivastava, H.M. A survey of some recent developments on higher transcendental functions of analytic number theory and applied mathematics. Symmetry 2021, 13, 2294. [Google Scholar] [CrossRef]

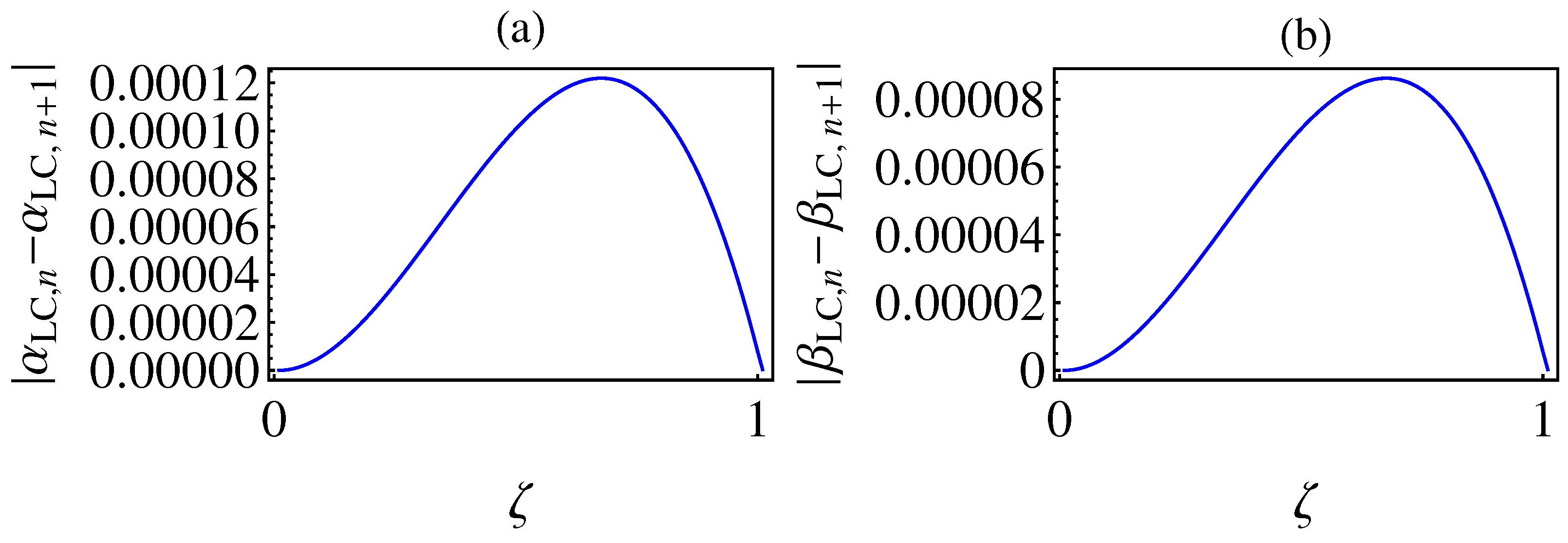


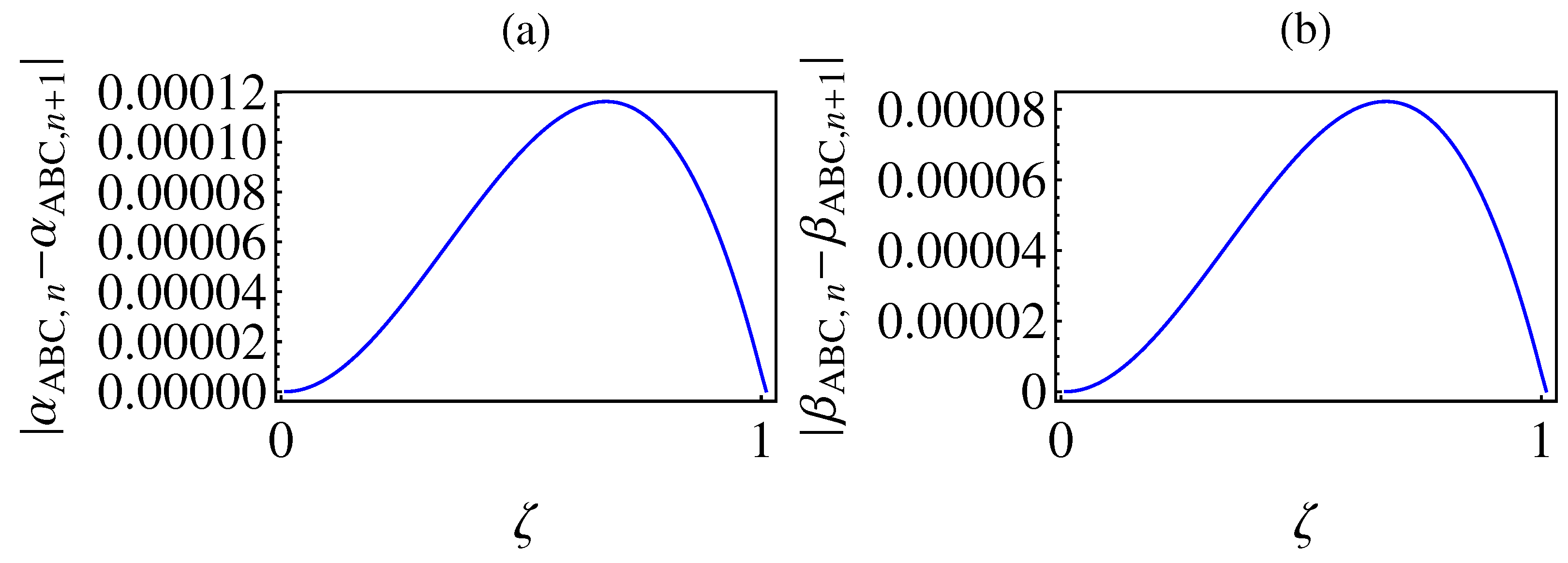
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saad, K.M.; Srivastava, H.M. Numerical Solutions of the Multi-Space Fractional-Order Coupled Korteweg–De Vries Equation with Several Different Kernels. Fractal Fract. 2023, 7, 716. https://doi.org/10.3390/fractalfract7100716
Saad KM, Srivastava HM. Numerical Solutions of the Multi-Space Fractional-Order Coupled Korteweg–De Vries Equation with Several Different Kernels. Fractal and Fractional. 2023; 7(10):716. https://doi.org/10.3390/fractalfract7100716
Chicago/Turabian StyleSaad, Khaled Mohammed, and Hari Mohan Srivastava. 2023. "Numerical Solutions of the Multi-Space Fractional-Order Coupled Korteweg–De Vries Equation with Several Different Kernels" Fractal and Fractional 7, no. 10: 716. https://doi.org/10.3390/fractalfract7100716
APA StyleSaad, K. M., & Srivastava, H. M. (2023). Numerical Solutions of the Multi-Space Fractional-Order Coupled Korteweg–De Vries Equation with Several Different Kernels. Fractal and Fractional, 7(10), 716. https://doi.org/10.3390/fractalfract7100716








