A Review on Fractional-Order Modelling and Control of Robotic Manipulators
Abstract
1. Introduction
- Different conventional and fractional-order modelling strategies for lower and higher DOF robotic manipulators are included in the review.
- A review of developed fractional-order controllers for various robotic manipulators evolved from PID, sliding mode, fuzzy, backstepping, active disturbance rejection control, and impedance control is presented.
- Fractional-order derivative definitions and approximation techniques are also presented.
- Trends for existing research and future developments in this area have been broadly presented and depicted in a graphical layout.
2. Preliminaries of Fractional Calculus
3. Survey with Trend Analysis
4. Modelling of Robotic Manipulators
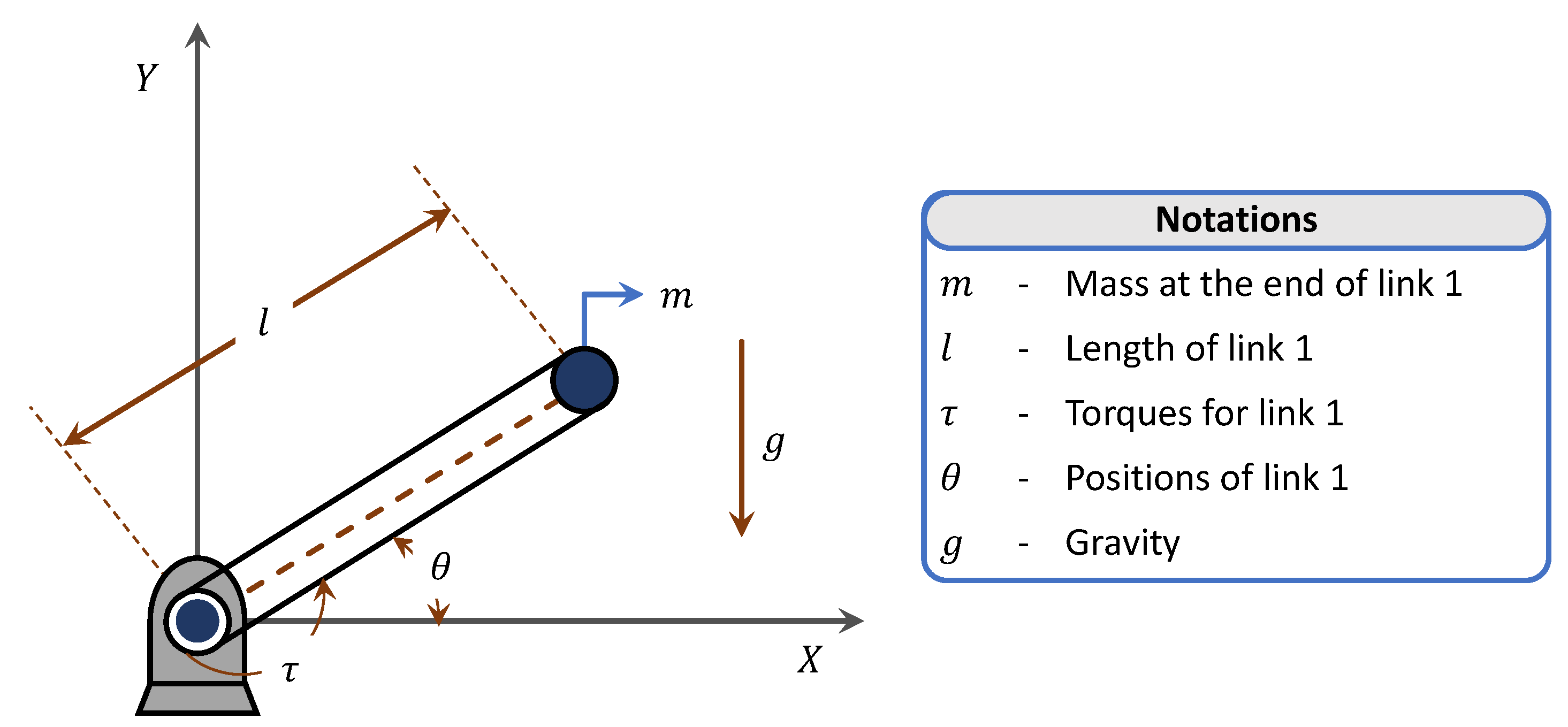
4.1. Single-Link Rigid and Flexible Robotic Manipulators
4.2. Two-Link Planar Rigid Robotic Manipulator
4.3. Three-Link Planar Rigid Robotic Manipulator
4.4. Generalized Model of Serial Link Planar Rigid Robotic Manipulator
- and are the mass matrices related to rigid and flexible degrees of freedom, respectively,
- row matrix that defines the coupling between manipulators’ rigid and flexible motions,
- row matrix that defines the coupling between manipulators’ flexible and rigid motions,
- and are the manipulators’ rigid and flexible degrees of freedom representing the motions of joints and elastic motions of flexible links, respectively,
- and are the centrifugal and Coriolis matrix related to rigid and flexible motion, respectively,
- and are the gravity matrix related to rigid and flexible motion, respectively,
- is the torque vector.
4.5. Other Robotic Manipulators
5. Fractional-Order Control of Robotic Manipulators
5.1. Fractional-Order PID Controllers
5.2. Fractional-Order Fuzzy PID Controllers
5.3. Fractional-Order Sliding Mode Controllers
5.4. Fractional-Order Adaptive Backstepping Controller
6. Conclusions
6.1. Findings
6.2. Future Perspectives
- There is broad scope for exploring the fractional-order modelling concept for various industrial robots, including Delta robot, KUKA youBot, Staubli RX-60, Robotino-XT, etc.
- The performance of fractional-order PID controllers can be further improved using the fractional-order form of predictive PI controllers for achieving robust servo and regulatory responses. Additionally, the performance of fractional-order PID controllers needs to be improved in the presence of uncertainties and faults.
- Even though fractional-order fuzzy PID controllers have achieved better servo and regulatory responses for proper industrial applications, the proof for analytical stability is a considerable research gap.
- The fractional-order nonsingular terminal sliding mode controller has achieved better response and surpassed the issues of singularity, uncertainties, and chattering effects. However, the controller configuration is very complex, and more parameters must be tuned. Thus, research on developing simple, evolved versions of controllers is inevitable.
- The adaptive backstepping controller provided an improved tracking performance in the presence of uncertainties and faults, thanks to the controllers’ adaptation law. However, the controller parameters are chosen using the trial and error method. Thus, there is scope to develop a tuning approach for controller parameters.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DOF | Degrees of freedom |
| FOMCON | Fractional-order modeling and control |
| IACCO | Integral of absolute change in controller output |
| IAE | Integral absolute error |
| ISCCO | Integral square of change in controller output |
| ISE | Integral square error |
| ISV | Integral of the square value |
| ITACO | Integral of time absolute change in controller output |
| ITAE | Integral time absolute error |
| ITSE | Integral time square error |
| LQR | Linear-quadratic regulator |
| MAD | Mean absolute deviation |
| MAE | Mean absolute error |
| MSE | Mean square error |
| MMFAE | Mean minimum fuel and absolute error |
| RMSE | Root mean squared error |
| STD | Standard deviation |
References
- Wu, X.; Huang, Y. Adaptive fractional-order non-singular terminal sliding mode control based on fuzzy wavelet neural networks for omnidirectional mobile robot manipulator. ISA Trans. 2022, 121, 258–267. [Google Scholar] [CrossRef]
- Xie, Y.; Zhang, X.; Meng, W.; Zheng, S.; Jiang, L.; Meng, J.; Wang, S. Coupled fractional-order sliding mode control and obstacle avoidance of a four-wheeled steerable mobile robot. ISA Trans. 2021, 108, 282–294. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, F.; Jiang, S.; Chen, B. Time delay control of cable-driven manipulators with adaptive fractional-order nonsingular terminal sliding mode. Adv. Eng. Softw. 2018, 121, 13–25. [Google Scholar] [CrossRef]
- Sharma, R.; Rana, K.; Kumar, V. Performance analysis of fractional order fuzzy PID controllers applied to a robotic manipulator. Expert Syst. Appl. 2014, 41, 4274–4289. [Google Scholar] [CrossRef]
- Sharma, R.; Gaur, P.; Mittal, A. Performance analysis of two-degree of freedom fractional order PID controllers for robotic manipulator with payload. ISA Trans. 2015, 58, 279–291. [Google Scholar] [CrossRef] [PubMed]
- Shah, P.; Agashe, S. Review of fractional PID controller. Mechatronics 2016, 38, 29–41. [Google Scholar] [CrossRef]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassan, S.M.; Harindran, V.R. Fractional-Order Systems and PID Controllers; Springer: London, UK, 2020. [Google Scholar]
- Bouzoualegh, S.; Guechi, E.H.; Kelaiaia, R. Model predictive control of a differential-drive mobile robot. Acta Univ. Sapientiae, Electr. Mech. Eng. 2018, 10, 20–41. [Google Scholar] [CrossRef]
- Machado, J.T.; Azenha, A. Fractional-order hybrid control of robot manipulators. In Proceedings of the SMC’98 Conference Proceedings. 1998 IEEE International Conference on Systems, Man, and Cybernetics (Cat. No. 98CH36218), San Diego, CA, USA, 11–14 October 1998; Volume 1, pp. 788–793. [Google Scholar]
- Duarte, F.; Machado, J. Chaotic phenomena and fractional-order dynamics in the trajectory control of redundant manipulators. Nonlinear Dyn. 2002, 29, 315–342. [Google Scholar] [CrossRef]
- Monje, C.; Ramos, F.; Feliu, V.; Vinagre, B. Tip position control of a lightweight flexible manipulator using a fractional order controller. IET Control. Theory Appl. 2007, 1, 1451–1460. [Google Scholar] [CrossRef]
- Efe, M.Ö. Fractional fuzzy adaptive sliding-mode control of a 2-DOF direct-drive robot arm. IEEE Trans. Syst. Man. Cybern. Part (Cybern.) 2008, 38, 1561–1570. [Google Scholar] [CrossRef]
- Ferreira, N.F.; Machado, J.T.; Tar, J.K. Two cooperating manipulators with fractional controllers. Int. J. Adv. Robot. Syst. 2009, 6, 31. [Google Scholar] [CrossRef]
- Delavari, H.; Ghaderi, R.; Ranjbar, N.; HosseinNia, S.H.; Momani, S. Adaptive fractional PID controller for robot manipulator. arXiv 2012, arXiv:1206.2027. [Google Scholar]
- Delavari, H.; Ghaderi, R.; Ranjbar, A.; Momani, S. Fuzzy fractional order sliding mode controller for nonlinear systems. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 963–978. [Google Scholar] [CrossRef]
- Fayazi, A.; Rafsanjani, H.N. Fractional order fuzzy sliding mode controller for robotic flexible joint manipulators. In Proceedings of the 2011 9th IEEE International Conference on Control and Automation (ICCA), Santiago, Chile, 19–21 December 2011; pp. 1244–1249. [Google Scholar]
- Bingul, Z.; Karahan, O. Tuning of fractional PID controllers using PSO algorithm for robot trajectory control. In Proceedings of the 2011 IEEE International Conference on Mechatronics, Istanbul, Turkey, 13–15 April 2011; pp. 955–960. [Google Scholar]
- Machado, J.T. The effect of fractional order in variable structure control. Comput. Math. Appl. 2012, 64, 3340–3350. [Google Scholar] [CrossRef]
- Bingül, Z.; KARAHAN, O. Fractional PID controllers tuned by evolutionary algorithms for robot trajectory control. Turk. J. Electr. Eng. Comput. Sci. 2012, 20, 1123–1136. [Google Scholar] [CrossRef]
- Copot, C.; Burlacu, A.; Ionescu, C.M.; Lazar, C.; De Keyser, R. A fractional order control strategy for visual servoing systems. Mechatronics 2013, 23, 848–855. [Google Scholar] [CrossRef]
- Dumlu, A.; Erenturk, K. Trajectory tracking control for a 3-dof parallel manipulator using fractional-order PIλDμ control. IEEE Trans. Ind. Electron. 2013, 61, 3417–3426. [Google Scholar] [CrossRef]
- Delavari, H.; Lanusse, P.; Sabatier, J. Fractional order controller design for a flexible link manipulator robot. Asian J. Control. 2013, 15, 783–795. [Google Scholar] [CrossRef]
- Moreno, A.R.; Sandoval, V.J. Fractional order PD and PID position control of an angular manipulator of 3DOF. In Proceedings of the 2013 Latin American Robotics Symposium and Competition, Arequipa, Peru, 21–27 October 2013; pp. 89–94. [Google Scholar]
- Copot, C.; Ionescu, C.M.; Lazar, C.; De Keyser, R. Fractional order PDμ control of a visual servoing manipulator system. In Proceedings of the 2013 European Control Conference (ECC), Zurich, Switzerland, 17–19 July 2013; pp. 4015–4020. [Google Scholar]
- Mujumdar, A.; Tamhane, B.; Kurode, S. Fractional order modeling and control of a flexible manipulator using sliding modes. In Proceedings of the 2014 American Control Conference, Portland, OR, USA, 4–6 June 2014; pp. 2011–2016. [Google Scholar]
- Wang, Y.; Luo, G.; Gu, L.; Li, X. Fractional-order nonsingular terminal sliding mode control of hydraulic manipulators using time delay estimation. J. Vib. Control 2016, 22, 3998–4011. [Google Scholar] [CrossRef]
- Mujumdar, A.; Tamhane, B.; Kurode, S. Observer-based sliding mode control for a class of noncommensurate fractional-order systems. IEEE/ASME Trans. Mechatronics 2015, 20, 2504–2512. [Google Scholar] [CrossRef]
- Nojavanzadeh, D.; Badamchizadeh, M. Adaptive fractional-order non-singular fast terminal sliding mode control for robot manipulators. IET Control. Theory Appl. 2016, 10, 1565–1572. [Google Scholar] [CrossRef]
- Fani, D.; Shahraki, E. Two-link robot manipulator using fractional order PID controllers optimized by evolutionary algorithms. Biosci. Biotechnol. Res. Asia 2016, 13, 589–598. [Google Scholar] [CrossRef]
- Mohammed, R.H.; Bendary, F.; Elserafi, K. Trajectory tracking control for robot manipulator using fractional order-fuzzy-PID controller. Int. J. Comput. Appl. 2016, 134, 22–29. [Google Scholar]
- Sharma, R.; Gaur, P.; Mittal, A. Design of two-layered fractional order fuzzy logic controllers applied to robotic manipulator with variable payload. Appl. Soft Comput. 2016, 47, 565–576. [Google Scholar] [CrossRef]
- Nikdel, N.; Badamchizadeh, M.; Azimirad, V.; Nazari, M.A. Fractional-order adaptive backstepping control of robotic manipulators in the presence of model uncertainties and external disturbances. IEEE Trans. Ind. Electron. 2016, 63, 6249–6256. [Google Scholar] [CrossRef]
- Łegowski, A.; Niezabitowski, M. Manipulator path control with variable order fractional calculus. In Proceedings of the 2016 21st International Conference on Methods and Models in Automation and Robotics (MMAR), Miedzyzdroje, Poland, 29 August–1 September 2016; pp. 1127–1132. [Google Scholar]
- Rahmani, M.; Ghanbari, A.; Ettefagh, M.M. Hybrid neural network fraction integral terminal sliding mode control of an Inchworm robot manipulator. Mech. Syst. Signal Process. 2016, 80, 117–136. [Google Scholar] [CrossRef]
- Ghasemi, I.; Ranjbar Noei, A.; Sadati, J. Sliding mode based fractional-order iterative learning control for a nonlinear robot manipulator with bounded disturbance. Trans. Inst. Meas. Control. 2018, 40, 49–60. [Google Scholar] [CrossRef]
- Wang, Y.; Gu, L.; Xu, Y.; Cao, X. Practical tracking control of robot manipulators with continuous fractional-order nonsingular terminal sliding mode. IEEE Trans. Ind. Electron. 2016, 63, 6194–6204. [Google Scholar] [CrossRef]
- Rahmani, M.; Ghanbari, A.; Ettefagh, M.M. Robust adaptive control of a bio-inspired robot manipulator using bat algorithm. Expert Syst. Appl. 2016, 56, 164–176. [Google Scholar] [CrossRef]
- Aghababa, M.P. Optimal design of fractional-order PID controller for five bar linkage robot using a new particle swarm optimization algorithm. Soft Comput. 2016, 20, 4055–4067. [Google Scholar] [CrossRef]
- Kumar, A.; Kumar, V. A novel interval type-2 fractional order fuzzy PID controller: Design, performance evaluation, and its optimal time domain tuning. ISA Trans. 2017, 68, 251–275. [Google Scholar] [CrossRef]
- Feliu-Talegon, D.; Feliu-Batlle, V. A fractional-order controller for single-link flexible robots robust to sensor disturbances. IFAC-PapersOnLine 2017, 50, 6043–6048. [Google Scholar] [CrossRef]
- Machado, J.T.; Lopes, A.M. A fractional perspective on the trajectory control of redundant and hyper-redundant robot manipulators. Appl. Math. Model. 2017, 46, 716–726. [Google Scholar] [CrossRef]
- Guo, Y.; Ma, B.L. Global sliding mode with fractional operators and application to control robot manipulators. Int. J. Control. 2019, 92, 1497–1510. [Google Scholar] [CrossRef]
- Kumar, A.; Kumar, V. Hybridized ABC-GA optimized fractional order fuzzy pre-compensated FOPID control design for 2-DOF robot manipulator. AEU-Int. J. Electron. Commun. 2017, 79, 219–233. [Google Scholar] [CrossRef]
- Kumar, V.; Rana, K. Nonlinear adaptive fractional order fuzzy PID control of a 2-link planar rigid manipulator with payload. J. Frankl. Inst. 2017, 354, 993–1022. [Google Scholar] [CrossRef]
- De la Fuente, M.S.L. Trajectory Tracking Error Using Fractional Order PID Control Law for Two-Link Robot Manipulator via Fractional Adaptive Neural Networks. In Robotics—Legal, Ethical and Socioeconomic Impacts; IntechOpen: London, UK, 2017; p. 35. [Google Scholar] [CrossRef]
- Kumar, V.; Rana, K. Comparative study on fractional order PID and PID controllers on noise suppression for manipulator trajectory control. In Fractional Order Control and Synchronization of Chaotic Systems; Springer: New York, NY, USA, 2017; pp. 3–28. [Google Scholar]
- Al-Saggaf, U.M.; Mehedi, I.M.; Mansouri, R.; Bettayeb, M. Rotary flexible joint control by fractional order controllers. Int. J. Control. Autom. Syst. 2017, 15, 2561–2569. [Google Scholar] [CrossRef]
- Kumar, J.; Kumar, V.; Rana, K. A fractional order fuzzy PD+I controller for three-link electrically driven rigid robotic manipulator system. J. Intell. Fuzzy Syst. 2018, 35, 5287–5299. [Google Scholar] [CrossRef]
- Bensafia, Y.; Ladaci, S.; Khettab, K.; Chemori, A. Fractional order model reference adaptive control for SCARA robot trajectory tracking. Int. J. Ind. Syst. Eng. 2018, 30, 138–156. [Google Scholar] [CrossRef]
- Ahmed, S.; Wang, H.; Tian, Y. Fault tolerant control using fractional-order terminal sliding mode control for robotic manipulators. Stud. Inform. Control. 2018, 27, 55–64. [Google Scholar] [CrossRef]
- Azar, A.T.; Kumar, J.; Kumar, V.; Rana, K. Control of a two link planar electrically-driven rigid robotic manipulator using fractional order SOFC. In Proceedings of the International Conference on Advanced Intelligent Systems and Informatics, Cairo, Egypt, 9–11 September 2017; Springer: Cham, Switzerland, 2017; pp. 57–68. [Google Scholar]
- Fareh, R.; Bettayeb, M.; Rahman, M. Control of serial link manipulator using a fractional order controller. Int. Rev. Autom. Control. 2018, 11, 1–6. [Google Scholar] [CrossRef]
- Kumar, A.; Kumar, V.; Gaidhane, P.J. Optimal design of fuzzy fractional order PIλDμ controller for redundant robot. Procedia Comput. Sci. 2018, 125, 442–448. [Google Scholar] [CrossRef]
- Ataşlar-Ayyıldız, B.; Karahan, O. Tuning of fractional order PID controller using cs algorithm for trajectory tracking control. In Proceedings of the 2018 6th International Conference on Control Engineering & Information Technology (CEIT), Istanbul, Turkey, 25–27 October 2018; pp. 1–6. [Google Scholar]
- Yin, C.; Xue, J.; Cheng, Y.; Zhang, B.; Zhou, J. Fractional order nonsingular fast terminal sliding mode control technique for 6-DOF robotic manipulator. In Proceedings of the 2018 37th Chinese Control Conference (CCC), Wuhan, China, 25–27 July 2018; pp. 10186–10190. [Google Scholar]
- Kathuria, T.; Kumar, V.; Rana, K.; Azar, A.T. Control of a three-link manipulator using fractional-order pid controller. In Fractional Order Systems; Elsevier: Berkeley, CA, USA, 2018; pp. 477–510. [Google Scholar]
- Kumar, J.; Kumar, V.; Rana, K. Design of robust fractional order fuzzy sliding mode PID controller for two link robotic manipulator system. J. Intell. Fuzzy Syst. 2018, 35, 5301–5315. [Google Scholar] [CrossRef]
- Kumar, J.; Azar, A.T.; Kumar, V.; Rana, K.P.S. Design of fractional order fuzzy sliding mode controller for nonlinear complex systems. In Mathematical Techniques of Fractional Order Systems; Elsevier: Amsterdam, The Netherlands, 2018; pp. 249–282. [Google Scholar]
- Angel, L.; Viola, J. Fractional order PID for tracking control of a parallel robotic manipulator type delta. ISA Trans. 2018, 79, 172–188. [Google Scholar] [CrossRef]
- Coronel-Escamilla, A.; Torres, F.; Gómez-Aguilar, J.; Escobar-Jiménez, R.; Guerrero-Ramírez, G. On the trajectory tracking control for an SCARA robot manipulator in a fractional model driven by induction motors with PSO tuning. Multibody Syst. Dyn. 2018, 43, 257–277. [Google Scholar] [CrossRef]
- Yin, C.; Zhou, J.; Xue, J.; Zhang, B.; Huang, X.; Cheng, Y. Design of the fractional-order adaptive nonsingular terminal sliding mode controller for 6-DOF robotic manipulator. In Proceedings of the 2018 Chinese Control And Decision Conference (CCDC), Shenyang, China, 9–11 June 2018; pp. 5954–5959. [Google Scholar]
- Muñoz-Vázquez, A.J.; Gaxiola, F.; Martínez-Reyes, F.; Manzo-Martínez, A. A fuzzy fractional-order control of robotic manipulators with PID error manifolds. Appl. Soft Comput. 2019, 83, 105646. [Google Scholar] [CrossRef]
- Sharma, R.; Bhasin, S.; Gaur, P.; Joshi, D. A switching-based collaborative fractional order fuzzy logic controllers for robotic manipulators. Appl. Math. Model. 2019, 73, 228–246. [Google Scholar] [CrossRef]
- Mohan, V.; Chhabra, H.; Rani, A.; Singh, V. An expert 2DOF fractional order fuzzy PID controller for nonlinear systems. Neural Comput. Appl. 2019, 31, 4253–4270. [Google Scholar] [CrossRef]
- Kumar, V.; Rana, K.; Kler, D. Efficient control of a 3-link planar rigid manipulator using self-regulated fractional-order fuzzy PID controller. Appl. Soft Comput. 2019, 82, 105531. [Google Scholar] [CrossRef]
- Fareh, R. Sliding mode fractional order control for a single flexible link manipulator. Int. J. Mech. Eng. Robot. Res. 2019, 8, 228–232. [Google Scholar] [CrossRef]
- Ardeshiri, R.R.; Kashani, H.N.; Reza-Ahrabi, A. Design and simulation of self-tuning fractional order fuzzy PID controller for robotic manipulator. Int. J. Autom. Control 2019, 13, 595–618. [Google Scholar] [CrossRef]
- Hamzeh Nejad, F.; Fayazi, A.; Ghayoumi Zadeh, H.; Fatehi Marj, H.; HosseinNia, S.H. Precise tip-positioning control of a single-link flexible arm using a fractional-order sliding mode controller. J. Vib. Control. 2020, 26, 1683–1696. [Google Scholar] [CrossRef]
- Wang, Y.; Li, B.; Yan, F.; Chen, B. Practical adaptive fractional-order nonsingular terminal sliding mode control for a cable-driven manipulator. Int. J. Robust Nonlinear Control 2019, 29, 1396–1417. [Google Scholar] [CrossRef]
- Singh, A.P.; Deb, D.; Agarwal, H. On selection of improved fractional model and control of different systems with experimental validation. Commun. Nonlinear Sci. Numer. Simul. 2019, 79, 104902. [Google Scholar] [CrossRef]
- Ahmed, T.M.; Gaber, A.N.A.; Hamdy, R.; Abdel-Khalik, A.S. Position Control of Arm Manipulator Within Fractional Order PID Utilizing Particle Swarm Optimization Algorithm. In Proceedings of the 2019 IEEE Conference on Power Electronics and Renewable Energy (CPERE), Aswan City, Egypt, 23–25 October 2019; pp. 135–139. [Google Scholar]
- Ahmed, S.; Lochan, K.; Roy, B.K. Fractional-Order Adaptive Sliding Mode Control for a Two-Link Flexible Manipulator. In Innovations in Infrastructure; Springer: Singapore, 2019; pp. 33–53. [Google Scholar]
- Rahmani, M.; Rahman, M.H. Adaptive neural network fast fractional sliding mode control of a 7-DOF exoskeleton robot. Int. J. Control. Autom. Syst. 2020, 18, 124–133. [Google Scholar] [CrossRef]
- Ahmed, S.; Wang, H.; Tian, Y. Adaptive fractional high-order terminal sliding mode control for nonlinear robotic manipulator under alternating loads. Asian J. Control 2021, 23, 1900–1910. [Google Scholar] [CrossRef]
- Lavín-Delgado, J.; Solís-Pérez, J.; Gómez-Aguilar, J.; Escobar-Jiménez, R. Trajectory tracking control based on non-singular fractional derivatives for the PUMA 560 robot arm. Multibody Syst. Dyn. 2020, 50, 259–303. [Google Scholar] [CrossRef]
- Al-Mayyahi, A.; Aldair, A.A.; Chatwin, C. Control of a 3-RRR planar parallel robot using fractional order PID controller. Int. J. Autom. Comput. 2020, 17, 822–836. [Google Scholar] [CrossRef]
- Tenreiro Machado, J.A.; Lopes, A.M. Fractional-order kinematic analysis of biomechanical inspired manipulators. J. Vib. Control. 2020, 26, 102–111. [Google Scholar] [CrossRef]
- Zhou, M.; Feng, Y.; Xue, C.; Han, F. Deep convolutional neural network based fractional-order terminal sliding-mode control for robotic manipulators. Neurocomputing 2020, 416, 143–151. [Google Scholar] [CrossRef]
- Chhabra, H.; Mohan, V.; Rani, A.; Singh, V. Robust nonlinear fractional order fuzzy PD plus fuzzy I controller applied to robotic manipulator. Neural Comput. Appl. 2020, 32, 2055–2079. [Google Scholar] [CrossRef]
- Yousfi, N.; Almalki, H.; Derbel, N. Robust control of industrial MIMO systems based on fractional order approaches. In Proceedings of the 2020 Industrial & Systems Engineering Conference (ISEC), New Orleans, LA, USA, 30 May–2 June 2020; pp. 1–6. [Google Scholar]
- Zhang, Y.; Yang, X.; Wei, P.; Liu, P.X. Fractional-order adaptive non-singular fast terminal sliding mode control with time delay estimation for robotic manipulators. IET Control. Theory Appl. 2020, 14, 2556–2565. [Google Scholar] [CrossRef]
- Shi, X.; Huang, J.; Gao, F. Fractional-order active disturbance rejection controller for motion control of a novel 6-dof parallel robot. Math. Probl. Eng. 2020, 2020, 3657848. [Google Scholar] [CrossRef]
- Singh, A.P.; Deb, D.; Agrawal, H.; Balas, V.E. Fractional Modeling and Controller Design of Robotic Manipulators: With Hardware Validation; Springer Nature: Cham, Switzerland, 2020; Volume 194. [Google Scholar]
- Al-Sereihy, M.H.; Mehedi, I.M.; Al-Saggaf, U.M.; Bettayeb, M. State-feedback-based fractional-order control approximation for a rotary flexible joint system. Mechatron. Syst. Control. 2020, 48. [Google Scholar] [CrossRef]
- Anjum, Z.; Guo, Y. Finite time fractional-order adaptive backstepping fault tolerant control of robotic manipulator. Int. J. Control Autom. Syst. 2021, 19, 301–310. [Google Scholar] [CrossRef]
- Su, L.; Guo, X.; Ji, Y.; Tian, Y. Tracking control of cable-driven manipulator with adaptive fractional-order nonsingular fast terminal sliding mode control. J. Vib. Control 2021, 27, 2482–2493. [Google Scholar] [CrossRef]
- Singh, A.P.; Deb, D.; Agrawal, H.; Bingi, K.; Ozana, S. Modeling and control of robotic manipulators: A fractional calculus point of view. Arab. J. Sci. Eng. 2021, 46, 9541–9552. [Google Scholar] [CrossRef]
- Gupta, S.; Singh, A.P.; Deb, D.; Ozana, S. Kalman Filter and Variants for Estimation in 2DOF Serial Flexible Link and Joint Using Fractional Order PID Controller. Appl. Sci. 2021, 11, 6693. [Google Scholar] [CrossRef]
- Bingul, Z.; Karahan, O. Real-time trajectory tracking control of Stewart platform using fractional order fuzzy PID controller optimized by particle swarm algorithm. Ind. Robot. Int. J. Robot. Res. Appl. 2021, 49, 708–725. [Google Scholar] [CrossRef]
- Anjum, Z.; Guo, Y.; Yao, W. Fault tolerant control for robotic manipulator using fractional-order backstepping fast terminal sliding mode control. Trans. Inst. Meas. Control 2021, 43, 3244–3254. [Google Scholar] [CrossRef]
- Ding, Y.; Liu, X.; Chen, P.; Luo, X.; Luo, Y. Fractional-Order Impedance Control for Robot Manipulator. Fractal Fract. 2022, 6, 684. [Google Scholar] [CrossRef]
- Abdulameer, H.I.; Mohamed, M.J. Fractional Order Fuzzy PID Controller Design for 2-Link Rigid Robot Manipulator. Int. J. Intell. Eng. Syst. 2021, 15, 103–117. [Google Scholar]
- Violia, J.; Angel, L. Control Performance Assessment of Fractional-Order PID Controllers Applied to Tracking Trajectory Control of Robotic Systems. WSEAS Trans. Syst. Control. 2022, 17, 62–73. [Google Scholar]
- Mishra, M.K.; Samantaray, A.K.; Chakraborty, G. Fractional-order Bouc-wen hysteresis model for pneumatically actuated continuum manipulator. Mech. Mach. Theory 2022, 173, 104841. [Google Scholar] [CrossRef]
- Gaidhane, P.J.; Adam, S. The Enhanced Robotic Trajectory Tracking by Optimized Fractional-Order Fuzzy Controller Using GWO-ABC Algorithm. In Soft Computing: Theories and Applications; Springer: Singapore, 2022; pp. 611–620. [Google Scholar]
- Azar, A.T.; Serrano, F.E.; Kamal, N.A.; Kumar, S.; Ibraheem, I.K.; Humaidi, A.J.; Gorripotu, T.S.; Pilla, R. Fractional-Order Euler–Lagrange Dynamic Formulation and Control of Asynchronous Switched Robotic Systems. In Proceedings of the Third International Conference on Sustainable Computing; Springer: Singapore, 2022; pp. 479–490. [Google Scholar]
- Bruzzone, L.; Polloni, A. Fractional Order KDHD Impedance Control of the Stewart Platform. Machines 2022, 10, 604. [Google Scholar] [CrossRef]
- Bruzzone, L.; Fanghella, P.; Basso, D. Application of the Half-Order Derivative to Impedance Control of the 3-PUU Parallel Robot. Actuators 2022, 11, 45. [Google Scholar] [CrossRef]
- Feliu-Talegon, D.; Feliu-Batlle, V. Improving the position control of a two degrees of freedom robotic sensing antenna using fractional-order controllers. Int. J. Control. 2017, 90, 1256–1281. [Google Scholar] [CrossRef]
- Feliu-Talegon, D.; Feliu-Batlle, V.; Tejado, I.; Vinagre, B.M.; HosseinNia, S.H. Stable force control and contact transition of a single link flexible robot using a fractional-order controller. ISA Trans. 2019, 89, 139–157. [Google Scholar] [CrossRef]
- Ventura, A.; Tejado, I.; Valério, D.; Martins, J. Fractional Control of a 7-DOF Robot to Behave Like a Human Arm. Prog. Fract. Differ. Appl. 2019, 5, 99–110. [Google Scholar] [CrossRef]
- Feliu-Talegon, D.; Feliu-Batlle, V. Control of very lightweight 2-DOF single-link flexible robots robust to strain gauge sensor disturbances: A fractional-order approach. IEEE Trans. Control Syst. Technol. 2021, 30, 14–29. [Google Scholar] [CrossRef]
- Craig, J.J. Introduction to Robotics: Mechanics and Control; Pearson Educacion: London, UK, 2005. [Google Scholar]
- Mishra, N.; Singh, S. Dynamic modelling and control of flexible link manipulators: Methods and scope-Part-1. Indian J. Sci. Technol. 2021, 14, 3210–3226. [Google Scholar] [CrossRef]
- Sahu, V.S.D.M.; Samal, P.; Panigrahi, C.K. Modelling, and control techniques of robotic manipulators: A review. Mater. Today Proc. 2021, 56, 2758–2766. [Google Scholar] [CrossRef]
- Lee, C.Y.; Lee, J.J. Adaptive control of robot manipulators using multiple neural networks. In Proceedings of the 2003 IEEE International Conference on Robotics and Automation (Cat. No. 03CH37422), Taipei, Taiwan, 14–19 September 2003; Volume 1, pp. 1074–1079. [Google Scholar]
- Lin, F. Robust Control Design: An Optimal Control Approach; John Wiley & Sons: New York, NY, USA, 2007. [Google Scholar]
- Devan, P.A.M.; Hussin, F.A.; Ibrahim, R.; Bingi, K.; Abdulrab, H. Fractional-order predictive PI controller for process plants with deadtime. In Proceedings of the 2020 IEEE 8th R10 Humanitarian Technology Conference (R10-HTC), Kuching, Sarawak, Malaysia, 1–3 December 2020; pp. 1–6. [Google Scholar]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassan, S.M.; Harindran, V.R. Real-time control of pressure plant using 2DOF fractional-order PID controller. Arab. J. Sci. Eng. 2019, 44, 2091–2102. [Google Scholar] [CrossRef]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassan, S.M. Fractional order set-point weighted PID controller for pH neutralization process using accelerated PSO algorithm. Arab. J. Sci. Eng. 2018, 43, 2687–2701. [Google Scholar] [CrossRef]
- Abdelhedi, F.; Bouteraa, Y.; Chemori, A.; Derbel, N. Nonlinear PID and feedforward control of robotic manipulators. In Proceedings of the 2014 15th International Conference on Sciences and Techniques of Automatic Control and Computer Engineering (STA), Hammamet, Tunisia, 21–23 December 2014; pp. 349–354. [Google Scholar]

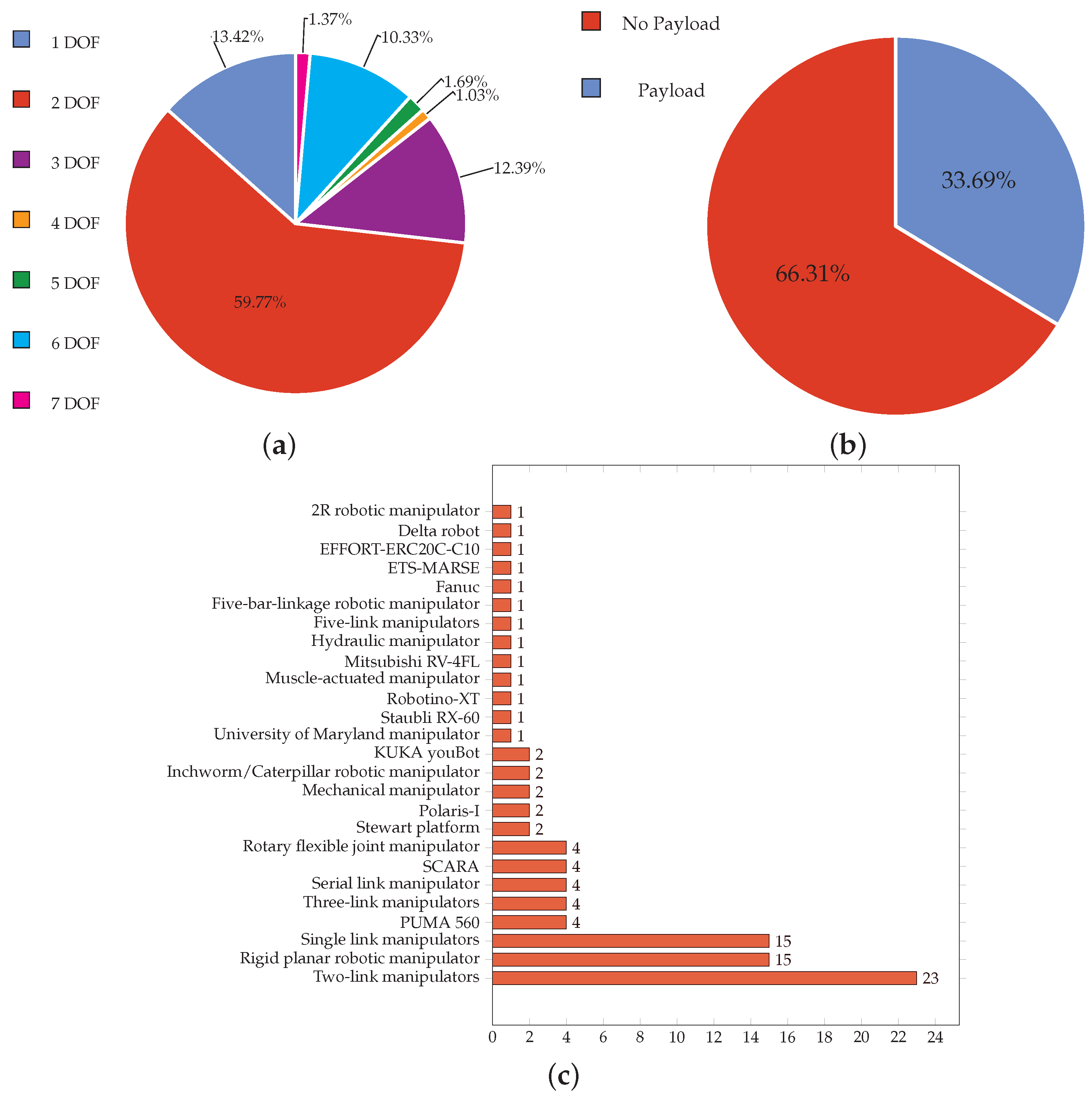

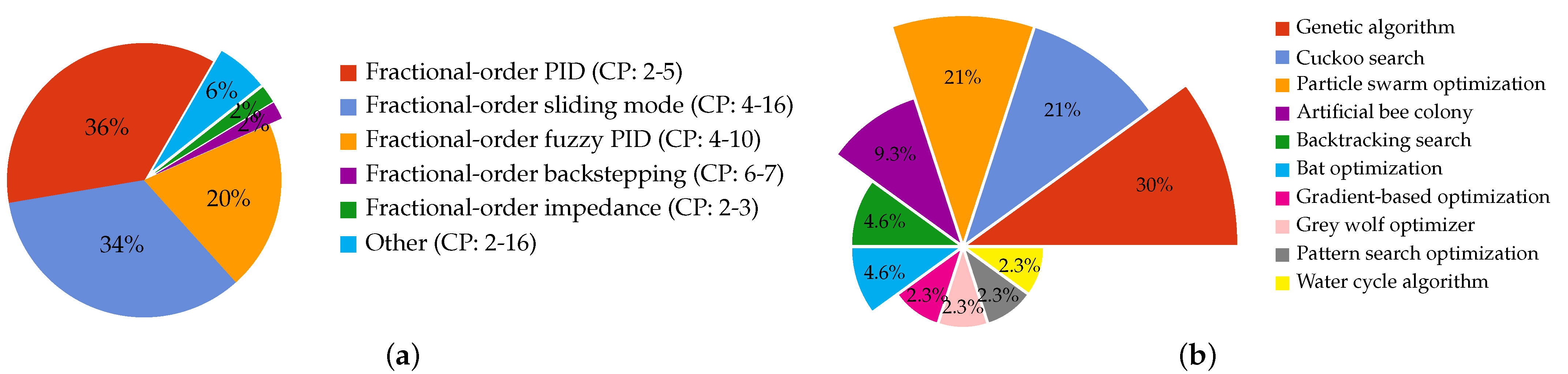
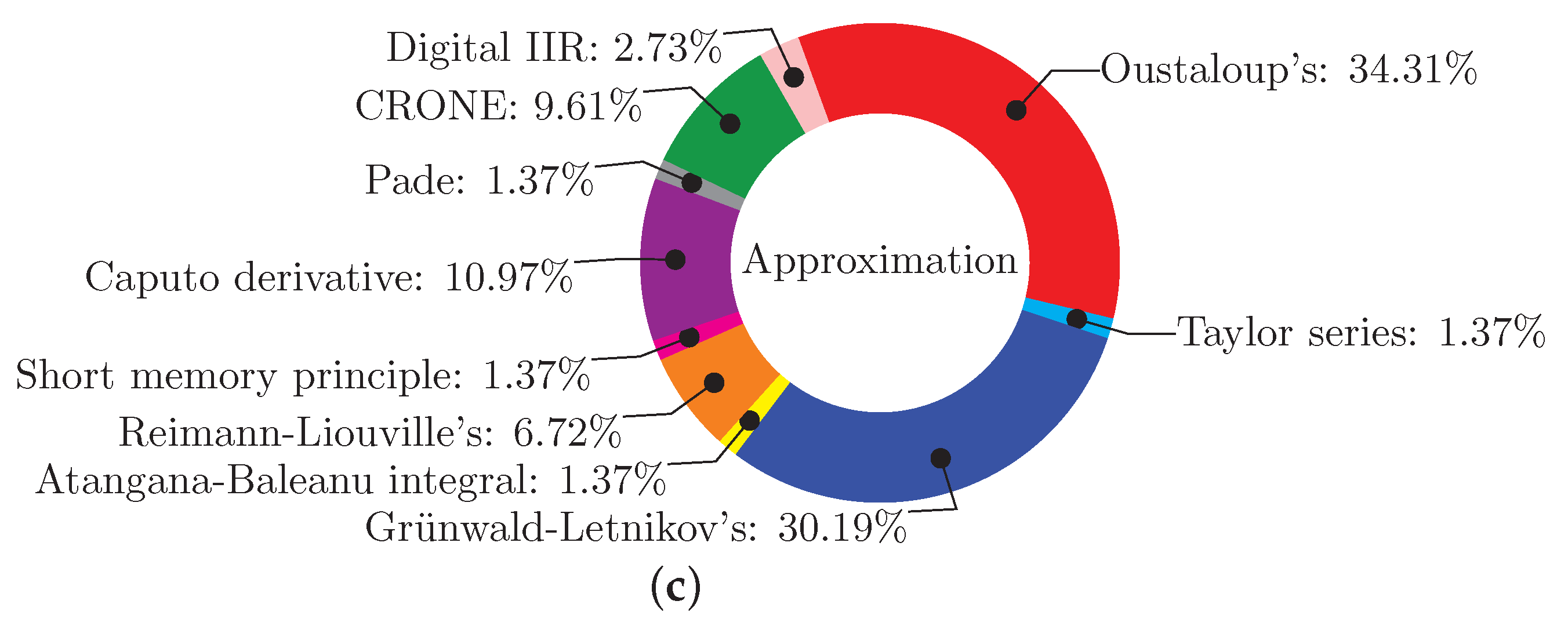
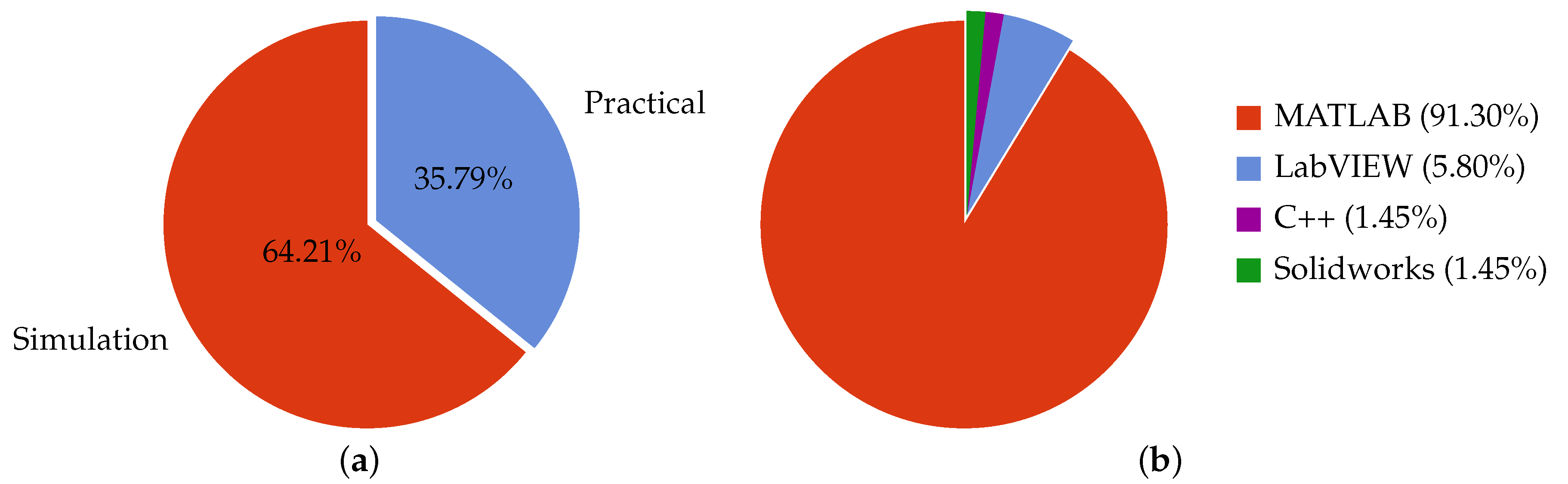



| Ref. | Manipulator Details | Modelling Details | Controller Details | Tool | S/P | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Type | DOF | Payload | FOM | Method | Order | FOC | Controller | CP | Tuning Technique | Comparison Controllers | OF | Approximation | |||
| [9] | 2R robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order D controller | 2 | Trial and error | PI and PD controllers | Transient response characteristics | Padé approximation | — | S |
| [10] | Redundant manipulator | — | ✗ | ✗ | Closed-Loop Pseudoinverse | 2 | ✓ | Pseudoinverse Algorithm | 5 | — | — | Tracking error | Grünwald–Letnikov’s method | — | S |
| [11] | Single-link flexible manipulator | 1 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PD controller | 3 | Trial and error | PD controller | Stability | Digital IIR filter approximation | M | P |
| [12] | Robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional fuzzy adaptive sliding mode controller | 5 | Trial and error | — | Tracking error | CRONE approximations | M | S |
| [13] | Rotational joints robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PD-PI controller | 5 | Trial and error | PD-PI controller | Transient response characteristics | — | — | S |
| [14] | Two-link robotic manipulator | 2 | ✗ | ✗ | Lagrangian formulation | 2 | ✓ | Adaptive fractional-order PID controller | 5 | Genetic Algorithm | PID controller | ISE | CRONE approximations | — | S |
| [15] | Polar robotic manipulator | 2 | ✓ | ✗ | State space model | 4 | ✓ | Fuzzy Fractional-order PD surface sliding mode controller | 8 | Genetic Algorithm | Classical PD surface sliding mode controller | RMSE | Caputo derivative | — | S |
| [16] | Two-link flexible joint manipulator | 2 | ✗ | ✗ | Lagrangian formulation | 8 | ✓ | Fractional order fuzzy sliding mode controller | 6 | Genetic Algorithm | Sliding mode controller, PD surface sliding mode controller, Sliding surfaces through fractional PD controller | IAE, ITAE, ISV | Caputo derivative | — | S |
| [17] | Two-link planar rigid robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Particle Swarm Optimization | Fuzzy and PID controllers | RMSE, MAE, MMFAE | Riemann–Liouville method | — | S |
| [18] | Mechanical manipulator | 2 | ✗ | ✗ | Mathematical modelling | 3 | ✓ | Fractional variable structure control and sliding mode control | 6 | Trial and error | Integer variable structure control and sliding mode control | Switching activity | Taylor series expansion | — | P |
| [19] | Two-link planar rigid robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Genetic Algorithm, Particle Swarm Optimization | — | RMSE, MAE, MMFAE | — | M | S |
| [20] | Manipulator robot (Fanuc) | 6 | ✓ | ✗ | Robust disturbance observer | 1 | ✓ | Fractional-order PI controller | 3 | Decentralized tuning | PI controller | Gain Margins | Refined Oustaloup Filter | M | P |
| [21] | University of Maryland (UMD) manipulator | 3 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Pattern search optimization | PID controller | MSE | — | — | S |
| [22] | Flexible link manipulator | 2 | ✓ | ✗ | Euler-Bernoulli method | 2 | ✓ | Fractional-order sliding mode controller | 6 | Particle Swarm Optimization | Sliding mode controller | ISE | Riemann–Liouville method | — | S |
| [23] | Angular manipulator | 3 | ✗ | ✗ | Lagrange model | 2 | ✓ | Fractional-order PID controller | 5 | Trial and error | — | — | Riemann–Liouville method | M, L | P |
| [24] | Robotic manipulator | 6 | ✓ | ✗ | Mathematical modelling | 6 | ✓ | Fractional-order PD controller | 3 | Bode tuning | PD controller | Linear and angular velocities | Grünwald–Letnikov method | M | S |
| [25] | Single-link flexible manipulator | 1 | ✗ | ✓ | Non-commensurate fractional-order model | 0.71, 0.92 | ✓ | Fractional order sliding mode controller | 4 | QR decomposition method | Sliding mode controller | Tracking error | Caputo derivative | M | P |
| [4] | Two-link planar rigid robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy PID controller | 6 | Cuckoo Search Algorithm | Fuzzy PID, fractional-order PID and PID controllers | IAE, IACCO | Oustaloup’s approximation | M | S |
| [26] | Hydraulic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order nonsingular terminal sliding mode controller | 16 | Trial and error | Integer-order nonsingular terminal sliding mode controller | RMSE | Refined Oustaloup filter | M | P |
| [27] | Single-link flexible manipulator | 1 | ✗ | ✓ | Non-commensurate fractional-order model | 0.71, 0.92 | ✓ | Observer-based fractional-order sliding mode controller | 8 | Stability criterion | Sliding mode controller | Tracking error | Caputo derivative | — | P |
| [5] | Two-link planar rigid robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Two-degree of freedom fractional-order PID controller | 8 | Cuckoo Search Algorithm | Two-degree of freedom PID controller | Weighted sum of ITAE and IACCO | Oustaloup’s approximation | M | S |
| [28] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Adaptive fractional-order nonsingular fast terminal sliding mode controller | 13 | Trial and error | Nonsingular terminal, Second-order sliding mode controllers | Error, Reaching time, Chattering effect | Riemann–Liouville method | — | S |
| [29] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Particle swarm optimization, Genetic algorithm and Estimation of distribution algorithm | — | RMSE | Riemann–Liouville method | M | S |
| [30] | Robotic manipulator (PUMA 560) | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy PID controller | 5 | Genetic Algorithm | PID, fractional-order PID and fuzzy PID controllers | ISE | — | M | S |
| [31] | Two-link planar rigid robotic manipulator (SCARA) | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Two-layered fractional-order fuzzy logic controller | 10 | Cuckoo Search Algorithm | Two-layered, single-layred fuzzy logic, PID controllers | IAE | Oustaloup’s approximation | M | S |
| [32] | Rotary manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order adaptive backstepping controller | 7 | Trial and error | Adaptive backstepping controllers | Tracking performance | Caputo derivative | M | P |
| [33] | Robotic manipulator | 4 | ✗ | ✓ | Pseudoinverse algorithm | 0.5, 0.6, 0.8, 0.9, 0.99 | ✗ | — | — | — | — | Tracking accuracy | Grünwald–Letnikov method | M | S |
| [34] | Inchworm/ Caterpillar robotic manipulator | 1 | ✗ | ✗ | Euler–Lagrange method | 2 | ✓ | Neural network-based fraction integral terminal sliding mode controller | 5 | Trial and error | Sliding mode controller, Integral terminal sliding mode controller, Fraction integral terminal sliding mode controller | Tracking error | — | M | S |
| [35] | Single-link direct joint driven robotic manipulator | 1 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Sliding mode based fractional-order PD type iterative learning control | 5 | Trial and error | Sliding mode based fractional-order D type iterative learning control, Higher-order iterative learning control | Tracking error | CRONE approximations | M | S |
| [36] | Robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Time delay estimation-based fractional-order nonsingular terminal sliding mode controller | 9 | Trial and error | Time delay estimation-based, continuous nonsingular terminal, Time delay estimation-based integer-order nonsingular terminal sliding mode controllers | Tracking error | Riemann–Liouville method | M | P |
| [37] | Inchworm/ Caterpillar robotic manipulator | 1 | ✗ | ✗ | Euler–Lagrange formalism | 2 | ✓ | Adaptive fractional-order PID sliding mode controller | 5 | Bat optimization algorithm | PID, fractional-order PID, sliding mode controller | Weighted sum of IAE and ISV | Oustaloup’s recursive approximation | M | S |
| [38] | Five-bar-linkage robotic manipulator | - | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Modified Particle Swarm Optimization | Fractional-order PID controller tuned using standard, constriction factor approach, random inertia weight-based particle swarm optimization algorithms | IAE, ISE, ITSE | Oustaloup’s approximation | M | P |
| [39] | Two-link robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Interval type-2 fractional-order fuzzy PID controller | 6 | Artificial Bee Colony-Genetic Algorithm | Interval type-2 fuzzy PID, Type-1 fractional-order fuzzy PID, Type-1 fuzzy PID, PID | ITAE | Oustaloup’s approximation | M | S |
| [40] | Single-link flexible manipulator | 1 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order phase-lead compensator | 4 | Nyquist criterion | PID controller | Gain Margin | Grünwald–Letnikov method | — | P |
| [41] | Three and five links redundant manipulators | 3, 5 | ✗ | ✓ | Moore-Penrose pseudoinverse | — | ✗ | — | — | — | — | — | Grünwald–Letnikov method | M | S |
| [42] | Robotic manipulator | 2 | ✓ | ✗ | State space model | 4 | ✓ | Fractional-order global sliding mode controller | 10 | Trial and error | Sliding mode controller | Tracking error | Riemann–Liouville method | — | S |
| [43] | Robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy pre-compensated fractional-order PID controller | 9 | Hybrid artificial bee colony-genetic algorithm | Fuzzy pre-compensated PID, fuzzy PID and PID controllers | ITAE | Oustaloup’s recursive approximation | M | S |
| [44] | Two-link planar rigid robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Non-linear adaptive fractional-order fuzzy PID controller | 7 | Backtracking search algorithm | Non-linear adaptive fuzzy PID controller | ITAE, ITACO | Grünwald–Letnikov method | L | S |
| [45] | Two-link robotic manipulator | 2 | ✗ | ✓ | Fractional adaptive neural network | — | ✓ | Fractional-order PID controller | 5 | Trial and error | — | Tracking error | Caputo derivative | — | S |
| [46] | Two-link rigid planar manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID controller | 5 | Genetic Algorithm | PID controller | Weighted sum of IAE and ISCCO | Short memory principle | L | P |
| [47] | Rotary flexible joint manipulator | 1 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order integral controller | 2 | Gain margins | Integral controller | Tracking accuracy | Oustaloup’s approximation | M | P |
| [49] | Robotic manipulator (SCARA) | 2 | ✗ | ✗ | Linear model | 2 | ✓ | Fractional-order model reference adaptive controller | 3 | Trial and error | Model reference adaptive controller | Delay time | Oustaloup’s approximation | — | S |
| [50] | Robotic manipulator (PUMA 560) | 3 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order nonsingular fast terminal sliding mode control based fault tolerant control | 7 | Trial and error | Adaptive fractional-order nonsingular fast terminal sliding mode controller, Nonsingular fast terminal sliding mode control based active fault tolerant control | Convergence speed | Riemann–Liouville method | — | S |
| [51] | Two-link planar electrically-driven rigid robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order self organizing fuzzy controller | 6 | Cuckoo Search Algorithm | Fractional-order fuzzy PID | IAE | Grünwald–Letnikov method | M | S |
| [52] | Serial link manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID and auxiliary controllers | 5 | Trial and error | Torque approach controller | Tracking error | CRONE approximations | M | S |
| [53] | Redundant manipulator (SCARA) | 5 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fuzzy fractional-order PID controller | 6 | Artificial Bee Colony Algorithm | PID and fuzzy PID controllers | ITAE | — | M | S |
| [54] | Three-link robotic manipulator (Staubli RX-60) | 6 | ✗ | ✗ | Mathematical modelling | 3 | ✓ | Fractional-order PID controller | 5 | Cuckoo Search Algorithm | PID controller | IAE, ITAE, ISE and IACCO | — | M | S |
| [55] | Robotic manipulator | 6 | ✗ | ✗ | Kinematic modelling | 2 | ✓ | Fractional order nonsingular fast terminal sliding mode control | 13 | Trial and error | — | Tracking error | Riemann–Liouville method | — | S |
| [56] | Three-link planar rigid robotic manipulator | 3 | ✗ | ✗ | Euler–Lagrange formalism | 3 | ✓ | Fractional-order PID controller | 5 | Evaporation Rate-Based Water Cycle Algorithm | PID controller | Weighted sum of IAE and IACCO | Grünwald–Letnikov method | M | S |
| [57] | Two-link planar rigid robotic manipulator | 2 | ✗ | ✗ | Euler–Lagrange formalism | 2 | ✓ | Fractional-order fuzzy sliding mode PD/PID controller | 8 | Cuckoo Search Algorithm | Integer-order fuzzy sliding mode PD/PID controller | Weighted sum of IAE and chatter | Grünwald–Letnikov method | M | S |
| [58] | Two-link planar rigid robotic manipulator | 2 | ✗ | ✗ | Lagrangian-Euler formulation | 2 | ✓ | Fractional-order fuzzy sliding mode controller with proportional derivative surface | 6 | Genetic Algorithm | Integer-order fuzzy SMC with proportional derivative surface | Weighted sum of IAE and chatter | Grünwald–Letnikov method | M | S |
| [59] | Parallel robotic manipulators (Delta Robot) | 3 | ✓ | ✗ | Inverse kinematic model | 3 | ✓ | Fractional-order PID controller | 5 | FMINCON (Gradient descent algorithm) | PID controller | RMSE | — | M | P |
| [60] | Robotic manipulator (SCARA) | 2 | ✗ | ✓ | Euler–Lagrange and Hamilton formalisms | 1.14 | ✓ | Fractional-order PI/PD controller | 3 | Particle Swarm Optimization | PI/PD controller | ITAE | Grünwald–Letnikov method | M | S |
| [61] | Serial robotic manipulator | 6 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order adaptive nonsingular terminal siding mode controller | 8 | Trial and error | — | Tracking error | Riemann–Liouville method | M | S |
| [3] | Cable-driven manipulator (Polaris-I) | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Time delay control scheme-based adaptive fractional-order nonsingular terminal sliding mode controller | 15 | Trial and error | Time delay estimation-based adaptive, continuous fractional-order nonsingular terminal sliding mode controller | RMSE | Riemann–Liouville method | M | P |
| [62] | Robotic manipulator | 2 | ✗ | ✗ | Euler–Lagrange formalism | 2 | ✓ | Fuzzy fractional-order PID controller | 3 | Heuristic Tuning | Sliding mode control, Super twisting sliding mode control, Fuzzy PID | ITAE, ISE | Grünwald–Letnikov method | C++ | P |
| [63] | Rigid planar robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Collaborative fractional order PID and fractional order fuzzy logic controller | 9 | Cuckoo Search Algorithm | PID, Fractional-order PID, Fractional-order fuzzy PID | ITAE | Oustaloup’s recursive approximation | M | S |
| [64] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Two-degree-of-freedom fractional-order fuzzy PI-D | 16 | Multi-objective non-dominated sorting genetic algorithm-II | Two-degree-of-freedom fractional-order PI-D | IAE | Grünwald–Letnikov method | M | S |
| [65] | Three-link planar rigid robotic manipulator | 3 | ✓ | ✗ | Euler–Lagrange formalism | 3 | ✓ | Self-regulated fractional-order fuzzy PID controller | 6 | Backtracking Search Algorithm | Self-regulated integer-order fuzzy PID controller | IAE, IACCO | Grünwald–Letnikov method | L | S |
| [66] | Single-link flexible manipulator | 1 | ✓ | ✗ | Lagrangian formulation | 2 | ✓ | Sliding fractional order controller | 6 | Trial and error | PD controller | Tracking error | — | — | S |
| [67] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy PID controller | 6 | Particle Swarm Optimization | Fractional-order PID controller | IAE, IACCO | Oustaloup’s approximation | M | S |
| [68] | Single-link flexible manipulator | 1 | ✓ | ✗ | State space model | 4 | ✓ | Fractional-order sliding mode controller | 10 | Trial and error | PID, Sliding mode controller | RMSE, MAE | CRONE approximations | M | S |
| [69] | Cable-driven manipulator (Polaris-I) | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order nonsingular terminal sliding mode controller | 12 | Closed-loop control tuning | Time delay estimation-based and continuous fractional-order nonsingular terminal sliding mode controller | RMSE | Refined Oustaloup filter | M | P |
| [70] | Serial Flexible Link Robotic Manipulator, Serial Flexible Joint Robotic Manipulator | 2 | ✗ | ✓ | Fractional transfer function model | 0.3, 0.9 | ✓ | Fractional-order PID controller | 5 | Trial and error | PID controller | Transient response characteristics | Oustaloup’s approximation | M | P |
| [71] | Robotic manipulator | 2 | ✗ | ✗ | Kinematic modelling | 2 | ✓ | Fractional-order PID controller | 5 | Particle Swarm Optimization | PID controller | Error | — | — | S |
| [72] | Two-link flexible robotic manipulator | 3 | ✗ | ✓ | Euler–Lagrange formulation | 0.98 | ✓ | Fractional-order adaptive sliding mode controller | 13 | Trial and error | Adaptive sliding mode controller | Tracking error | — | M | S |
| [73] | Exoskeleton Robot (ETS-MARSE) | 7 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Adaptive neural network fast fractional integral terminal sliding mode control | 6 | Trial and error | Fast fractional integral terminal sliding mode controller | Tracking error | Grünwald–Letnikov method | M | P |
| [74] | Robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | 2 | ✓ | Adaptive fractional high-order terminal sliding mode controller | 10 | Trial and error | H∞-Adaptive control, intelligent PD, intelligent PID, Adaptive third-order sliding mode controller | Convergence speed and precision | Oustaloup method | M | S |
| [75] | Robotic manipulator (PUMA 560) | 6 | ✓ | ✓ | Euler–Lagrange formalism | 12 | ✓ | Fractional-order PI, PD controllers | 9 | Cuckoo Search Algorithm | PI, PD controllers | RMSE | Caputo–Fabrizio derivative, Atangana–Baleanu integral | — | P |
| [76] | 3-RRR planar parallel robots | 3 | ✗ | ✗ | Inverse kinematics using Cayley–Menger determinants and bilateration | 2 | ✓ | Fractional-order PID controller | 5 | Bat optimization algorithm | PID controller | Weighted function | — | M | P |
| [77] | Muscle-actuated manipulator | 2 | ✗ | ✓ | Fractional order describing functions | 2 | ✗ | — | — | — | — | — | Grünwald–Letnikov method | — | P |
| [79] | Robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy PD and I controller | 8 | Multi-objective non-dominated sorting genetic algorithm-II, dragonfly algorithm, multi-verse optimization, ant lion optimizer algorithms | PID, fuzzy PID controllers | IAE | Grünwald–Letnikov method | M | P |
| [80] | Robotic manipulator (SCARA) | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order PID and Fractional-order pre-filter | 5, 4 | Genetic Algorithm, Trial and error | — | Gain Margins | CRONE approximations | M | S |
| [81] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Time delay estimation-based adaptive fractional-order nonsingular terminal sliding mode controller | 12 | Trial and error | Nonsingular fast terminal sliding mode controller, Second order nonsingular fast terminal sliding mode controller | Tracking error | Riemann–Liouville method | M | S |
| [82] | Parallel robotic manipulator | 6 | ✓ | ✗ | Kinematic modelling | 3 | ✓ | Fractional-order active disturbance rejection controller | 16 | Trial and error | Active disturbance rejection controller | Tracking accuracy | — | M | P |
| [83] | Single-link robotic manipulator | 1 | ✗ | ✓ | Euler–Lagrange formulation | 0.5 | ✗ | Feedback controller | 8 | Pole placement method | PID, LQR controllers | Tracking accuracy | Oustaloup’s approximation | M | P |
| [83] | Serial-link flexible robotic manipulator, Serial flexible joint robotic manipulator | 2 | ✗ | ✓ | Fractional value selection algorithm | 0.3, 0.9 | ✓ | Fractional-order PID controller | 5 | Trial and error | PID controller | Tracking accuracy | Oustaloup’s approximation | M | P |
| [84] | Rotary flexible joint manipulator | 1 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | State-feedback-based fractional-order integral controller | 2 | Trial and error | Pure state-feedback control scheme and the modified state-feedback-based fractional-order integral controllers | Tracking error | CRONE, Oustaloup’s approximations | M | S |
| [86] | Two-link robotic manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Time delay estimation-based adaptive fractional-order nonsingular terminal sliding mode controller | 10 | Trial and error | — | Tracking performance and speed | Oustaloup’s recursive approximation | — | S |
| [83] | Single Rigid Link Robotic Manipulator, Serial Link Robotic Manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Adaptive fractional-order controller | 5 | Trial and error | Integer-order and adaptive controllers | Transient response characteristics | Oustaloup’s approximation | M | P |
| [2] | Cooperative manipulator (Mitsubishi RV-4FL) | 6 | ✓ | ✗ | Kinematic modelling | 3 | ✓ | Coupled fractional-order sliding mode control | 5 | Fuzzy tuning | PI, Sliding mode controllers, fractional-order sliding mode controller | IAE, ISE, STD | Oustaloup’s approximation | M | P |
| [87] | Single flexible link robotic manipulator, Serial flexible joint robotic manipulator | 1,2 | ✗ | ✓ | Euler–Lagrange formulation | 0.5 | No | Feedback controller | 8 | Pole placement method | PID, LQR controllers | Tracking accuracy | Oustaloup’s approximation | M | P |
| [88] | Single flexible link robotic manipulator, Serial flexible joint robotic manipulator | 1,2 | ✗ | ✗ | Euler–Lagrange formulation | 2 | ✓ | Fractional-order PID controller | 5 | Trial and error | PID controller | Transient response characteristics | Oustaloup’s approximation | M | S |
| [89] | Stewart Platform | 6 | ✗ | ✗ | Lagrange-Euler approach | 3 | ✓ | Fractional order fuzzy PID controller | 8 | Particle Swarm Optimization | PID, fractional-order PID and fuzzy PID controllers | MAE, RMSE | Oustaloup’s approximation | M | P |
| [90] | Robotic manipulator (PUMA 560) | 3 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order backstepping fast terminal sliding mode controller | 15 | Trial and error | PID, Computed torque controller, Nonsingular fast terminal sliding mode controller | Position tracking error | Oustaloup’s approximation | M | S |
| [1] | Three-link omnidirectional mobile robot manipulator (KUKA youBot) | 5 | ✗ | ✗ | Lagrangian dynamics equation | 3 | ✓ | Adaptive fractional-order nonsingular terminal sliding mode controller | 9 | Trial and error | Fractional-order terminal sliding mode controller, Nonsingular terminal sliding mode controller | Tracking speed and accuracy | Riemann–Liouville method | M | P |
| [92] | Two-link Rigid Robotic Manipulator | 2 | ✗ | ✗ | Mathematical modelling | 2 | ✓ | Fractional-order fuzzy PID controller | 6 | Most valuable player algorithm | Integer-order fuzzy PID, One block fractional/Integer order fuzzy PID, Two block Fractional/Integer order fuzzy PID controllers | ITSE | Grünwald–Letnikov method | M | S |
| [93] | Robotic manipulator | 2 | ✓ | ✗ | Euler–Lagrange method | 2 | Yes | Fractional-order PID controller | 5 | Gradient-based optimization | PID controller | ISE | — | M | S |
| [94] | Single-segment soft continuum manipulator (Robotino-XT) | — | ✓ | ✓ | Fractional-order Bouc–Wen hysteresis model | 16 | — | — | — | — | — | Absolute pose error | Grünwald–Letnikov method | — | P |
| [95] | Two-link robotic manipulator | 2 | ✓ | ✗ | Mathematical modelling | — | ✓ | Fractional-order fuzzy PID controller | 8 | Hybrid grey wolf optimizer and artificial bee colony algorithm | PID | Tracking error | — | M | P |
| [96] | Robotic manipulator | — | ✗ | ✓ | Fractional-order Euler–Lagrange formulation | — | — | — | — | — | — | — | — | — | P |
| [97] | Stewart Platform | 6 | ✓ | ✗ | Kinematic modelling | 2 | ✓ | Fractional-order KDHD impedance control | 2 | Transient response-based tuning | KD controller | Error | Grünwald–Letnikov method | M | S |
| [98] | 3-PUU parallel robotic manipulator | 3 | ✗ | ✗ | Kinematic modelling | 2 | ✓ | PDD1/2 controller | 2 | Transient response-based tuning | PD controller | Error | Grünwald–Letnikov method | M | S |
| [99] | Flexible link manipulator | 2 | ✗ | ✗ | Euler–Lagrange formulation | 2 | ✓ | Fractional-order phase-lag compensator | 3 | Optimization process | 2DOF PID controller | Tracking error | Grünwald–Letnikov method | M | P |
| [100] | Single-link flexible manipulator | 2 | ✓ | ✗ | Euler–Bernoull formulation | 2 | ✓ | Fractional-order PD | 2 | Bode Specifications | PD controller | Bode Margins | Grünwald–Letnikov method | M | P |
| [101] | KUKA LWR IV | 7 | ✓ | ✓ | Inverse Kinematics Model | 3.04 | ✓ | Impedance control | 4 | Genetic Algorithm | — | MSE, MAD | — | — | P |
| [102] | Single-link flexible manipulator | 2 | ✓ | ✗ | Pseudo-clamped approach | 2 | ✓ | Fractional-order PID | 2 | Bode Specifications | PID controller | Tracking error | Frequency response-based technique | M | P |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bingi, K.; Rajanarayan Prusty, B.; Pal Singh, A. A Review on Fractional-Order Modelling and Control of Robotic Manipulators. Fractal Fract. 2023, 7, 77. https://doi.org/10.3390/fractalfract7010077
Bingi K, Rajanarayan Prusty B, Pal Singh A. A Review on Fractional-Order Modelling and Control of Robotic Manipulators. Fractal and Fractional. 2023; 7(1):77. https://doi.org/10.3390/fractalfract7010077
Chicago/Turabian StyleBingi, Kishore, B Rajanarayan Prusty, and Abhaya Pal Singh. 2023. "A Review on Fractional-Order Modelling and Control of Robotic Manipulators" Fractal and Fractional 7, no. 1: 77. https://doi.org/10.3390/fractalfract7010077
APA StyleBingi, K., Rajanarayan Prusty, B., & Pal Singh, A. (2023). A Review on Fractional-Order Modelling and Control of Robotic Manipulators. Fractal and Fractional, 7(1), 77. https://doi.org/10.3390/fractalfract7010077








