On the Variable Order Fractional Calculus Characterization for the Hidden Variable Fractal Interpolation Function
Abstract
1. Introduction
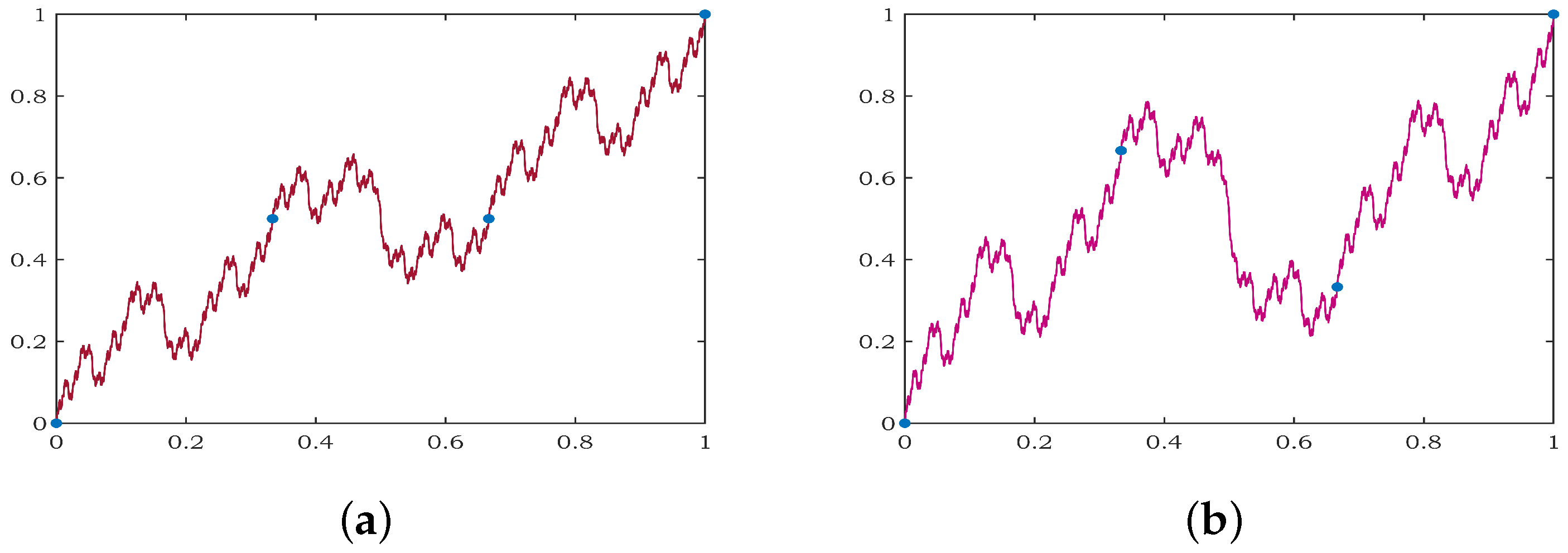
2. Brief of the Hidden Variable Fractal Interpolation Function
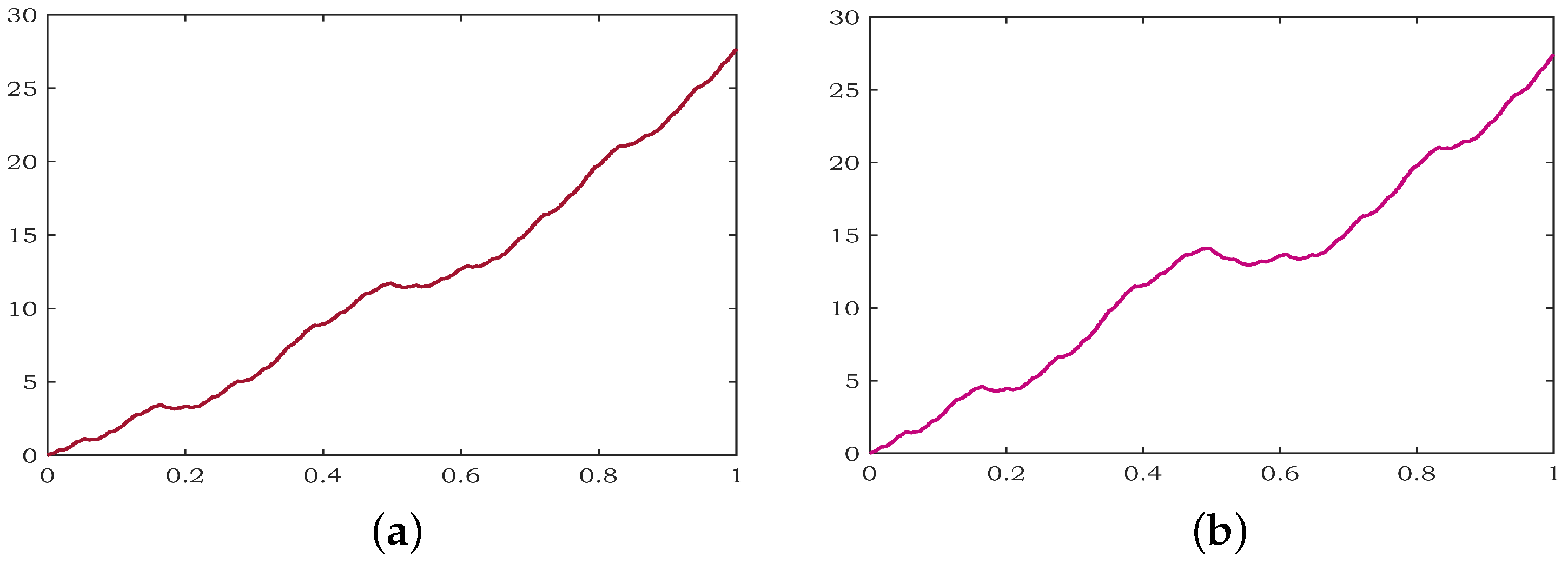
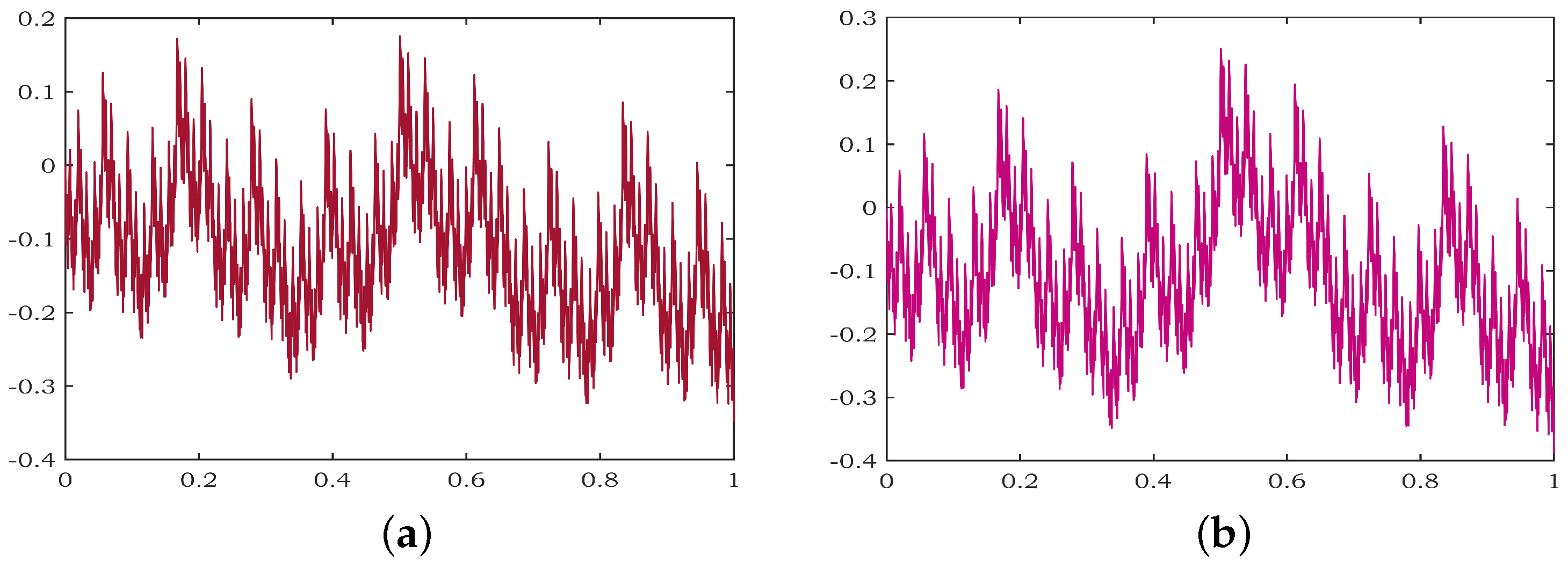
3. Main Results
4. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Barnsley, M.F. Fractal functions and interpolation. Constr. Approx. 1986, 2, 303–329. [Google Scholar] [CrossRef]
- Hutchinson, J.E. Fractals and self similarity. Indiana Univ. Math. J. 1981, 30, 713–747. [Google Scholar] [CrossRef]
- Easwaramoorthy, D.; Uthayakumar, R. Analysis on fractals in fuzzy metric spaces. Fractals 2011, 19, 379–386. [Google Scholar] [CrossRef]
- Banerjee, S.; Easwaramoorthy, D.; Gowrisankar, A. Fractal Functions, Dimensions and Signal Analysis; Springer: Cham, Switzerland, 2021. [Google Scholar]
- Banerjee, S.; Hassan, M.K.; Mukherjee, S.; Gowrisankar, A. Fractal Patterns in Nonlinear Dynamics and Applications; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Ruan, H.J.; Su, W.Y.; Yao, K. Box dimension and fractional integral of linear fractal-interpolation functions. J. Approx. Theory 2009, 161, 187–197. [Google Scholar] [CrossRef]
- Balasubramani, N. Affine recurrent fractal-interpolation functions. Eur. Phys. J. Spec. Top. 2021, 230, 3765–3779. [Google Scholar] [CrossRef]
- Barnsley, M.F.; Elton, J.; Hardin, D.; Massopust, P. Hidden variable fractal interpolation functions. Siam J. Math. Anal. 1989, 20, 1218–1242. [Google Scholar] [CrossRef]
- Chand, A.K.B.; Kapoor, G.P. Hidden variable bivariate fractal interpolation surfaces. Fractals 2003, 11, 277–288. [Google Scholar] [CrossRef]
- Bouboulis, P.; Dalla, L. Hidden variable vector valued fractal-interpolation functions. Fractals-London 2003, 13, 227. [Google Scholar] [CrossRef]
- Bouboulis, P.; Dalla, L.; Drakopoulos, V. Construction of recurrent bivariate fractal interpolation surfaces and computation of their box-counting dimension. J. Approx. Theory 2006, 141, 99–117. [Google Scholar] [CrossRef][Green Version]
- Chand, A.K.B.; Kapoor, G.P. Cubic spline coalescence fractal interpolation through moments. Fractals 2007, 15, 41–53. [Google Scholar] [CrossRef]
- Kapoor, G.P.; Prasad, S.A. Smoothness of hidden variable bivariate coalescence fractal interpolation surfaces. Int. J. Bifurcat. Chaos 2009, 19, 2321–2333. [Google Scholar] [CrossRef]
- Prasad, S.A. Fractional calculus of coalescence hidden variable fractal interpolation functions. Fractals 2009, 25, 1750019. [Google Scholar] [CrossRef]
- Priyanka, T.M.C.; Gowrisankar, A. Analysis on Weyl–Marchaud Fractional Derivative for Types of fractal-interpolation function with Fractal Dimension. Fractals 2021, 29, 2150215. [Google Scholar] [CrossRef]
- Ri, M.G.; Yun, C.H.; Kim, M.H. Construction of cubic spline hidden variable recurrent fractal-interpolation function and its fractional calculus. Chaos Solitons Fractals 2021, 150, 111177. [Google Scholar] [CrossRef]
- Ri, M.G.; Yun, C.H. Smoothness and fractional integral of hidden variable recurrent fractal-interpolation function with function vertical scaling factors. Fractals 2021, 29, 2150136. [Google Scholar] [CrossRef]
- Gowrisankar, A.; Uthayakumar, R. Fractional calculus on fractal interpolation for a sequence of data with countable iterated function system. Mediterr. J. Math. 2016, 13, 3887–3906. [Google Scholar] [CrossRef]
- Gowrisankar, A.; Khalili Golmankhaneh, A.; Serpa, C. Fractal Calculus on fractal-interpolation functions. Fractal Fract. 2021, 5, 157. [Google Scholar] [CrossRef]
- Yao, K.; Su, W.Y.; Zhou, S.P. The fractional derivatives of a fractal function. Acta Math. Sin. 2006, 22, 719–722. [Google Scholar] [CrossRef]
- Ri, M.G.; Yun, C.H. Riemann–Liouville fractional integral of hidden variable fractal interpolation function. Chaos Solitons Fractals 2020, 140, 110126. [Google Scholar] [CrossRef]
- Liang, Y.S.; Su, W.Y. Fractal dimensions of fractional integral of continuous functions. Acta Math. Sin.-Engl. Ser. 2016, 32, 1494–1508. [Google Scholar] [CrossRef]
- Zhang, Q. Some remarks on one-dimensional functions and their Riemann–Liouville fractional calculus. Acta Math. Sin.-Engl. Ser. 2014, 30, 517–524. [Google Scholar] [CrossRef]
- Gowrisankar, A.; Prasad, M. Riemann–Liouville calculus on quadratic fractal-interpolation function with variable scaling factors. J. Anal. 2019, 27, 347–363. [Google Scholar] [CrossRef]
- Peng, W.L.; Yao, K.; Zhang, X.; Yao, J. Box dimension of Weyl–Marchaud fractional derivative of linear fractal-interpolation functions. Fractals 2019, 27, 1950058. [Google Scholar] [CrossRef]
- Ferrari, F. Weyl and Marchaud derivatives: A forgotten history. Mathematics 2018, 6, 6. [Google Scholar] [CrossRef]
- Priyanka, T.M.C.; Gowrisankar, A. Riemann–Liouville fractional integral of non-affine fractal-interpolation function and its fractional operator. Eur. Phys. J. Spec. Top. 2021, 230, 3789–3805. [Google Scholar] [CrossRef]
- Ri, M.G.; Yun, C.H. Riemann–Liouville fractional derivatives of hidden variable recurrent fractal-interpolation functions with function scaling factors and box dimension. Chaos Solitons Fractals 2022, 156, 111793. [Google Scholar] [CrossRef]
- Samko, S.G.; Ross, B. Integration and differentiation to a variable fractional order. Integr. Transform. Spec. Funct. 1993, 1, 277–300. [Google Scholar] [CrossRef]
- Samko, S.G. Fractional integration and differentiation of variable order. Anal. Math. 1995, 21, 213–236. [Google Scholar] [CrossRef]
- Zhao, X.; Sun, Z.Z.; Karniadakis, G.E. Second-order approximations for variable order fractional derivatives: Algorithms and applications. J. Comput. Phys. 2015, 293, 184–200. [Google Scholar] [CrossRef]
- Garrappa, R.; Giusti, A.; Mainardi, F. variable order fractional calculus: A change of perspective. Commun. Nonlinear Sci. Numer. Simul. 2021, 102, 105904. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raja, V.; Gowrisankar, A. On the Variable Order Fractional Calculus Characterization for the Hidden Variable Fractal Interpolation Function. Fractal Fract. 2023, 7, 34. https://doi.org/10.3390/fractalfract7010034
Raja V, Gowrisankar A. On the Variable Order Fractional Calculus Characterization for the Hidden Variable Fractal Interpolation Function. Fractal and Fractional. 2023; 7(1):34. https://doi.org/10.3390/fractalfract7010034
Chicago/Turabian StyleRaja, Valarmathi, and Arulprakash Gowrisankar. 2023. "On the Variable Order Fractional Calculus Characterization for the Hidden Variable Fractal Interpolation Function" Fractal and Fractional 7, no. 1: 34. https://doi.org/10.3390/fractalfract7010034
APA StyleRaja, V., & Gowrisankar, A. (2023). On the Variable Order Fractional Calculus Characterization for the Hidden Variable Fractal Interpolation Function. Fractal and Fractional, 7(1), 34. https://doi.org/10.3390/fractalfract7010034






