Application of the Explicit Euler Method for Numerical Analysis of a Nonlinear Fractional Oscillation Equation
Abstract
:1. Introduction
2. Preliminaries
3. Problem Statement
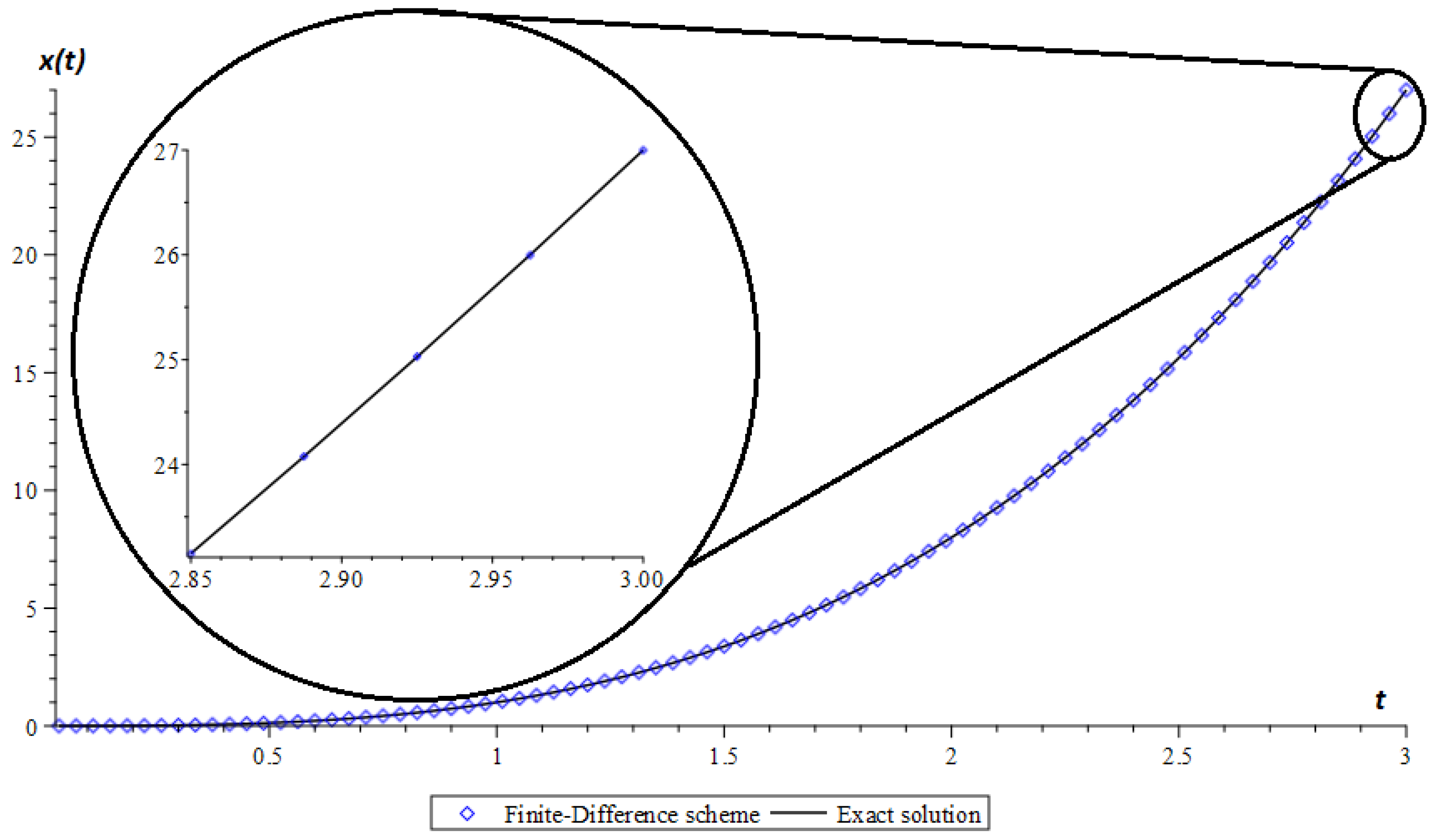
4. Numerical Solution
5. Convergence and Stability Issues
6. The Adams–Bashford–Moulton Method
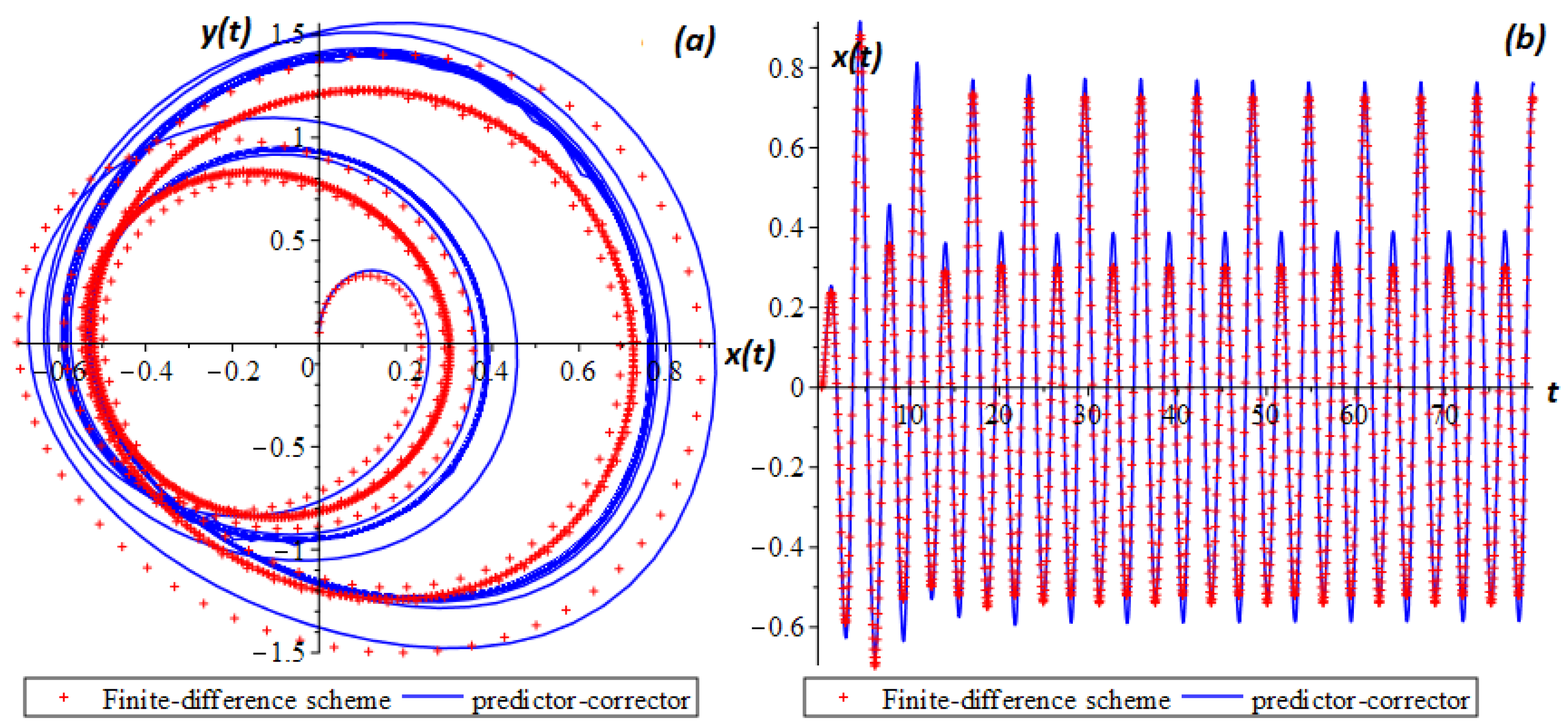
7. Simulation Results and Some Applications
7.1. Test Examples
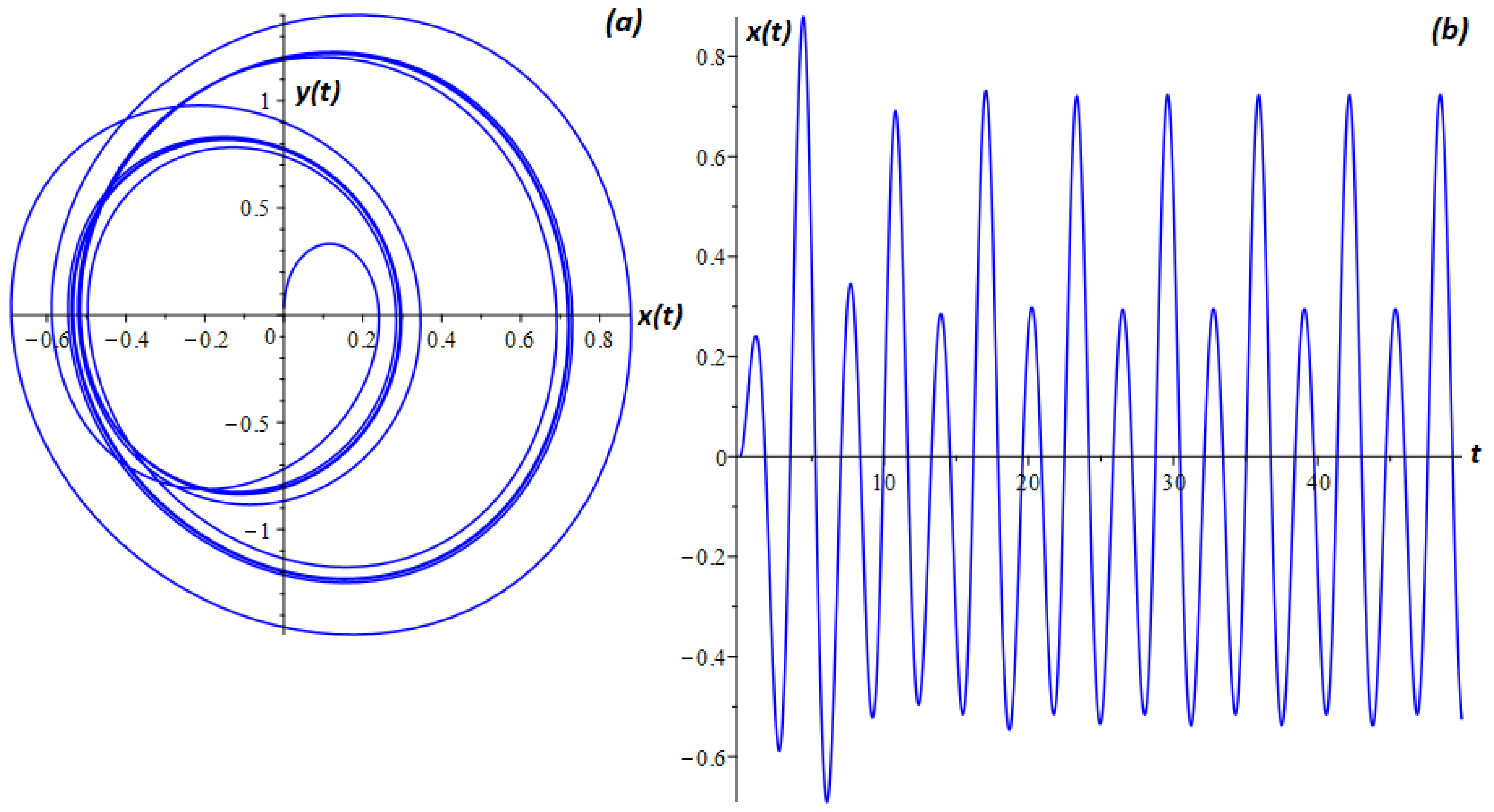
7.2. Fractional Duffing Oscillator
7.3. Amplitude–Frequency Characteristic of a Fractional Duffing oscillator
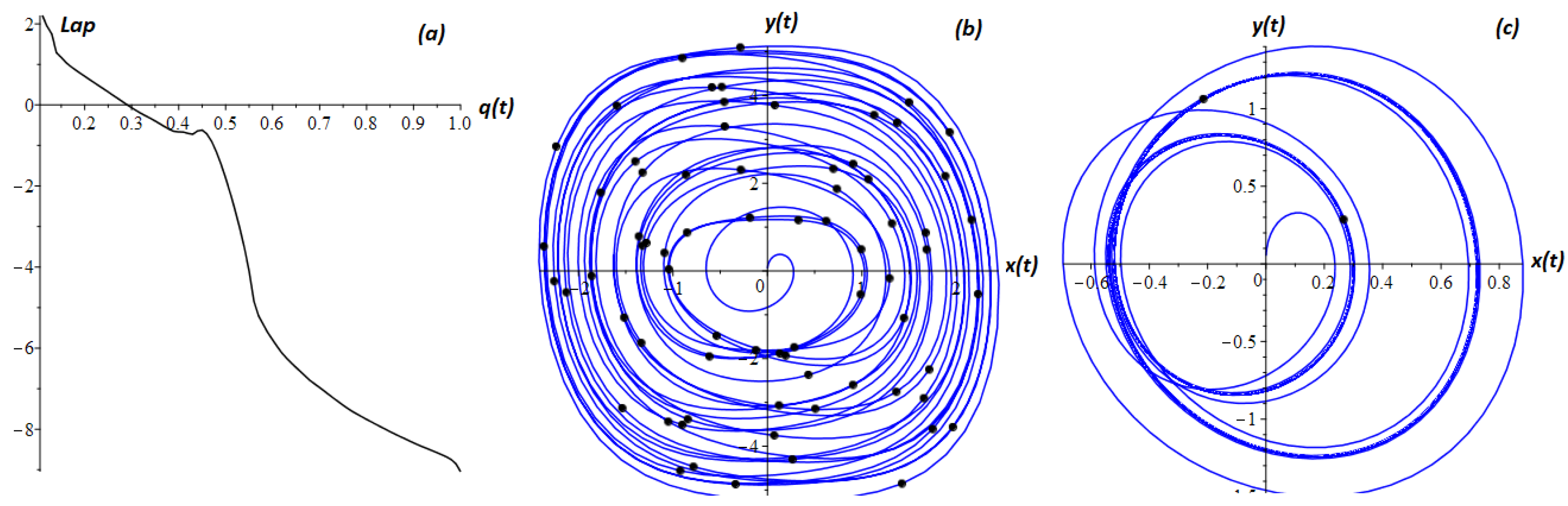
8. The Study of Chaotic and Regular Modes
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Petras, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer: New York, NY, USA, 2010; p. 180. [Google Scholar]
- Shitikova, M.V. Fractional Operator Viscoelastic Models in Dynamic Problems of Mechanics of Solids: A Review. Mech. Solids 2021, 57, 1–33. [Google Scholar] [CrossRef]
- Rekhviashvili, S.S.; Pskhu, A.V. New method for describing damped vibrations of a beam with a built-in end. Tech. Phys. 2019, 64, 1237–1241. [Google Scholar] [CrossRef]
- Parovik, R.I. Quality factor of forced oscillations of a linear fractional oscillator. Tech. Phys. 2020, 65, 1059–1063. [Google Scholar] [CrossRef]
- Parovik, R.I. Amplitude-frequency and phase-frequency performances of forced oscillations of a nonlinear fractional oscillator. Tech. Phys. Lett. 2019, 45, 660–663. [Google Scholar] [CrossRef]
- Rekhviashvili, S.S.; Pskhu, A.V. Fractional oscillator with exponential power function of memory. Lett. ZhTF 2022, 48, 33–35. (In Russian) [Google Scholar]
- Syta, A.; Litak, G.; Lenci, S.; Scheffler, M. Chaotic vibrations of the Duffing system with fractional damping. Chaos Interdiscip. J. Nonlinear Sci. 2014, 24, 10–16. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Y.; Liu, D.; Jia, W.; Huang, H. Responses of Duffing oscillator with fractional damping and random phase. Nonlinear Dyn. 2013, 74, 745–753. [Google Scholar] [CrossRef]
- Shen, Y.; Li, H.; Yang, S.; Peng, M.; Han, Y. Primary and subharmonic simultaneous resonance of fractional-order Duffing oscillator. Nonlinear Dyn. 2020, 102, 1485–1497. [Google Scholar] [CrossRef]
- Xing, W. Threshold for chaos of a duffing oscillator with fractional-order derivative. Shock Vib. 2019, 2019, 1–16. [Google Scholar] [CrossRef]
- Gao, X. Chaos in the fractional order periodically forced complex Duffing’s oscillators. Chaos Solitons Fractals 2005, 24, 97–104. [Google Scholar] [CrossRef]
- El-Dib, Y.O. Stability approach of a fractional-delayed Duffing oscillator. Discontinuity Nonlinearity Complex 2020, 9, 367–376. [Google Scholar] [CrossRef]
- Eze, S.C. Analysis of fractional Duffing oscillator. Rev. Mex. Física 2020, 66, 187–191. [Google Scholar] [CrossRef]
- Gouari, Y.; Dahmani, Z.; Jebril, I. Application of fractional calculus on a new differential problem of duffing type. Adv. Math. Sci. J. 2020, 9, 10989–11002. [Google Scholar] [CrossRef]
- Barba-Franco, J.J.; Gallegos, A.; Jaimes-Reátegui, R.; Pisarchik, A.N. Dynamics of a ring of three fractional-order Duffing oscillators. Chaos Solitons Fractals 2022, 155, 111–747. [Google Scholar] [CrossRef]
- Ejikeme, C.L.; Oyesanya, M.O.; Agbebaku, D.F.; Okofu, M.B. Solution to nonlinear Duffing oscillator with fractional derivatives using homotopy analysis method (HAM). Glob. J. Pure Appl. Math. 2018, 14, 1363–1388. [Google Scholar]
- Syam, M.I. The Modified Fractional Power Series Method for Solving Fractional Undamped Duffing Equation with Cubic Nonlinearity. Nonlinear Dyn. Syst. Theory 2020, 20, 568–577. [Google Scholar]
- Alvaro, H.; Salas, S. Analytical Approximant to a Quadratically Damped Duffing Oscillator. Sci. World J. 2022, 2022, 10–21. [Google Scholar]
- Wawrzynski, W. The origin point of the unstable solution area of a forced softening Duffing oscillator. Sci. Rep. 2022, 12, 4518. [Google Scholar] [CrossRef]
- Chen, T.; Cao, X.; Niu, D. Model modification and feature study of Duffing oscillator. J. Low Freq. Noise 2022, 41, 230–243. [Google Scholar] [CrossRef]
- Kim, V.A. Duffing oscillator with an external harmonic impact and derived variables fractional Remann-Liouville, is characterized by viscous friction. Bulletin KRASEC. Phys. Math. Sci. 2016, 13, 46–49. [Google Scholar]
- Kim, V.A.; Parovik, R.I. Mathematical model of fractional Duffing oscillator with variable memory. Mathematics 2020, 8, 2063. [Google Scholar] [CrossRef]
- Nakhushev, A.M. Fractional Calculus and Its Applications; Fizmatlit: Moscow, Russia, 2003; p. 272. (In Russian) [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier Science Limited: Amsterdam, The Netherlands, 2006; Volume 204, p. 523. [Google Scholar]
- Zhuang, P.; Liu, F.; Anh, V.; Turner, I. Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 2009, 47, 1760–1781. [Google Scholar] [CrossRef] [Green Version]
- Sun, H.; Chang, A.; Zhang, Y.; Chen, W. Variable-order fractional differential equations: Mathematical foundations, physical models, numerical methods and applications. Fract. Calc. Appl. Anal. 2019, 22, 27–59. [Google Scholar] [CrossRef] [Green Version]
- Nigmatullin, R.R. Fractional integral and its physical interpretation. Theor. Math. Phys. 1992, 90, 242–251. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection–dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef] [Green Version]
- Parovik, R.I. Mathematical modeling of linear fractional oscillators. Mathematics 2020, 8, 1879. [Google Scholar] [CrossRef]
- Yang, C.; Liu, F. A computationally effective predictor-corrector method for simulating fractional-order dynamical control system. ANZIAM J. 2006, 47, 168–184. [Google Scholar] [CrossRef] [Green Version]
- Niu, J.; Shen, Y.; Yang, S.; Li, S. Analysis of Duffing oscillator with time-delayed fractional-order PID controller. Int. J. Non-Linear Mech. 2017, 92, 65–75. [Google Scholar] [CrossRef]
- Wang, Y.; An, J.Y. Amplitude–frequency relationship to a fractional Duffing oscillator arising in microphysics and tsunami motion. J. Low Freq. Noise Vib. Act. Control 2019, 38, 1008–1012. [Google Scholar] [CrossRef]
- Li, Y.; Duan, J.-S. The periodic response of a fractional oscillator with a spring-pot and an inerter-pot. J. Mech. 2020, 37, 108–117. [Google Scholar] [CrossRef]
- Yang, J.H.; Sanjuán, M.A.; Wang, C.J.; Zhu, H. Vibrational Resonance in a Duffing System with a Generalized Delayed Feedback. J. Appl. Nonlinear Dyn. 2013, 2, 397–408. [Google Scholar] [CrossRef]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Phys. Nonlinear Phenom. 1985, 16, 285–317. [Google Scholar] [CrossRef] [Green Version]
- Parovik, R.I. Dynamic hysteresis of a fractional Duffing oscillator. Mat. Instituti Byulleteni 2019, 6, 47–51. (In Russian) [Google Scholar]




| N | h | (25) | (26) |
|---|---|---|---|
| 10 | 0.1 | 0.010416664 | - |
| 20 | 0.05 | 0.005137254 | 1.019824008 |
| 40 | 0.025 | 0.002556096 | 1.007055385 |
| 80 | 0.0125 | 0.001275018 | 1.003424407 |
| 160 | 0.00625 | 0.000636774 | 1.001664279 |
| 320 | 0.003125 | 0.000318209 | 1.00080679 |
| 640 | 0.0015625 | 0.000159059 | 1.000412635 |
| N | h | (18) | (19) |
|---|---|---|---|
| 10 | 0.1 | 216.8838 | - |
| 20 | 0.05 | 11004.94 | −5.665085173 |
| 40 | 0.025 | 22.6488 | 8.92450095 |
| 80 | 0.0125 | 0.012252099 | 10.85218997 |
| 160 | 0.00625 | 0.006278409 | 0.96455801 |
| 320 | 0.003125 | 0.0031782 | 0.9821891 |
| 640 | 0.0015625 | 0.0015990701 | 0.990976729 |
| N | h = T/N | (Finite-Difference Scheme (10)) | (Finite-Difference Scheme (10)) | (Predictor–Corrector (19)) | (Predictor–Corrector (19)) |
|---|---|---|---|---|---|
| 10 | 0.1 | 0.010416664 | - | 0.022014375 | - |
| 20 | 0.05 | 0.005137254 | 1.019824008 | 0.006517327 | 0.758163925 |
| 40 | 0.025 | 0.002556096 | 1.007055385 | 0.002125805 | 0.817941863 |
| 80 | 0.0125 | 0.001275018 | 1.003424407 | 0.004337276 | 1.131071553 |
| 160 | 0.00625 | 0.000636774 | 1.001664279 | 0.005145645 | 1.03243208 |
| 320 | 0.003125 | 0.000318209 | 1.00080679 | 0.005452087 | 1.011099493 |
| 640 | 0.0015625 | 0.000159059 | 1.000412635 | 0.005571391 | 1.004170658 |
| N | h | (28) | (16) |
|---|---|---|---|
| 10 | 0.1 | 0.010416664 | - |
| 20 | 0.05 | 0.005137254 | 1.019824008 |
| 40 | 0.025 | 0.002556096 | 1.007055385 |
| 80 | 0.0125 | 0.001275018 | 1.003424407 |
| 160 | 0.00625 | 0.000636774 | 1.001664279 |
| 320 | 0.003125 | 0.000318209 | 1.00080679 |
| 640 | 0.0015625 | 0.000159059 | 1.000412635 |
| N | h | (20) | (19) |
|---|---|---|---|
| 10 | 0.1 | 0.010416664 | - |
| 20 | 0.05 | 0.055137254 | −2.404134099 |
| 40 | 0.025 | 0.006556096 | 3.072118534 |
| 80 | 0.0125 | 0.003275018 | 1.001334144 |
| 160 | 0.00625 | 0.001536774 | 1.09159782 |
| 320 | 0.003125 | 0.00078209 | 0.974498474 |
| 640 | 0.0015625 | 0.000039059 | 1.001679624 |
| N | (Finite-Difference Scheme (10)) | (Finite-Difference Scheme (10)) | (Predictor–Corrector (19)) | (Predictor–Corrector (19)) | |
|---|---|---|---|---|---|
| 10 | 0.1 | 0.010416664 | - | 0.012159916 | - |
| 20 | 0.05 | 0.005137254 | 1.019824008 | 0.005933937 | 1.035071832 |
| 40 | 0.025 | 0.002556096 | 1.007055385 | 0.002947911 | 1.009296592 |
| 80 | 0.0125 | 0.001275018 | 1.003424407 | 0.001468966 | 1.004891965 |
| 160 | 0.00625 | 0.000636774 | 1.001664279 | 0.000733796 | 1.001351042 |
| 320 | 0.003125 | 0.000318209 | 1.00080679 | 0.000366708 | 1.000745531 |
| 640 | 0.0015625 | 0.000159059 | 1.000412635 | 0.00018197 | 1.000434281 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, V.A.; Parovik, R.I. Application of the Explicit Euler Method for Numerical Analysis of a Nonlinear Fractional Oscillation Equation. Fractal Fract. 2022, 6, 274. https://doi.org/10.3390/fractalfract6050274
Kim VA, Parovik RI. Application of the Explicit Euler Method for Numerical Analysis of a Nonlinear Fractional Oscillation Equation. Fractal and Fractional. 2022; 6(5):274. https://doi.org/10.3390/fractalfract6050274
Chicago/Turabian StyleKim, Valentine Aleksandrovich, and Roman Ivanovich Parovik. 2022. "Application of the Explicit Euler Method for Numerical Analysis of a Nonlinear Fractional Oscillation Equation" Fractal and Fractional 6, no. 5: 274. https://doi.org/10.3390/fractalfract6050274
APA StyleKim, V. A., & Parovik, R. I. (2022). Application of the Explicit Euler Method for Numerical Analysis of a Nonlinear Fractional Oscillation Equation. Fractal and Fractional, 6(5), 274. https://doi.org/10.3390/fractalfract6050274