The Influence of Noise on the Solutions of Fractional Stochastic Bogoyavlenskii Equation

Abstract

We look at the stochastic fractional-space Bogoyavlenskii equation in the Stratonovich sense, which is driven by multiplicative noise. Our aim is to acquire analytical fractional stochastic solutions to this stochastic fractional-space Bogoyavlenskii equation via two different methods such as the $exp(-\mathsf{\Phi }(\eta \left)\right)$-expansion method and sine–cosine method. Since this equation is used to explain the hydrodynamic model of shallow-water waves, the wave of leading fluid flow, and plasma physics, scientists will be able to characterize a wide variety of fascinating physical phenomena with these solutions. Furthermore, we evaluate the influence of noise on the behavior of the acquired solutions using 2D and 3D graphical representations.

1. Introduction

Fractional partial differential equations (FPDEs) have received much interest due to their application in several fields of science including biochemistry and chemistry [1,2], hydrology [3], biology [4,5], physics [6,7], finance [8], etc.

As a result, many researchers have recently focused their efforts on discovering new and improved general closed form exact wave solutions of FPDEs such as ($G^{\prime }/G)$-expansion [9,10], Fan sub-equation [11], improved extended Fan subequation [12], tanh-sech [13,14], sine–cosine [15], perturbation [16,17], Jacobi elliptic function [18,19], F-expansion [20], $exp(-\phi (\eta \left)\right)$-expansion [21,22,23] methods, and the references therein.

On the other hand, stochastic partial differential equations (SPDEs) have been extensively studied as mathematical models for spatial–temporal physical, biological, and chemical systems subject to random perturbations during the last few years. The importance of including stochastic effects in complicated system modeling has been highlighted. For instance, there is a significant focus on using SPDEs to mathematically model complex phenomena in finance, materials sciences, electrical and mechanical engineering, information systems, condensed matter physics, biology, and climate systems [24,25,26,27].

It seems that studying FPDE models with stochastic influences is more important. To the best of knowledge, little research has been conducted in order to obtain exact solutions to fractional SPDEs, for instance [28,29,30,31]. As a result, the purpose of this paper is to find the exact solution to the following space-fractional stochastic Bogoyavlenskii equation (SFSBE) [32] in the Stratonovich sense:

$$\begin{array}{c}\hfill 4d\psi +[{\mathcal{D}}_{xxy}^{3\alpha }\psi -4{\psi }^2{\mathcal{D}}_y^{\alpha }\psi -4w{\mathcal{D}}_x^{\alpha }\psi ]dt+\rho \psi \circ d\beta =0,\\ \hfill \psi {\mathcal{D}}_y^{\alpha }\psi ={\mathcal{D}}_x^{\alpha }w,\mathrm{for}0<\alpha \le 1,\end{array}$$

(1)

where $\psi (x,y,t)$ and $w(x,y,t)$ are real functions, ${\mathcal{D}}^{\alpha }$ is the conformable derivative (CD) [33], $\rho$ is the noise strength, and $\beta \left(t\right)$ is the standard Brownian motion.

Many authors have reviewed the deterministic Bogoyavlenskii Equation (1) with integer-order derivatives (i.e., $\rho =0$ and $\alpha =1$) to achieve analytical solutions using different techniques for example exp $(-\mathsf{\Phi }(\zeta \left)\right)$-expansion [34], Khater [35], multiple ($G^{\prime }/G)$-expansion [36], singular manifold [37], modified simple equation [38,39], modified extended tanh-function [40], generalized Riccati equation mapping [41], and sine–cosine [42]. Furthermore, the deterministic fractional Bogoyavlenskii Equation (1) has been solved by utilizing multiple techniques including $tan\left(\mathsf{\Phi }\right(\zeta )/2)$-expansion [43], exp $(-\mathsf{\Phi }(\zeta \left)\right)$-expansion and rational $tan\left(\mathsf{\Phi }\right(\zeta \left)\right)$-expansion [44], first integral [45], ($G^{\prime }/G)$-expansion [46], improved fractional sub-equation [47], Bäcklund transformation [48], Jacobi elliptic equation [49], ($G^{\prime }/G$, $1/G)$-expansion and ($1/G^{\prime }$)-expansion [50], and numerical multistep approach [51]. On the other hand, the stochastic Bogoyavlenskii equation has not yet been investigated.

Our contribution of this work is to consider the stochastic fractional-space Bogoyavlenskii equation in the Stratonovich sense, which is driven by multiplicative noise. This equation has never been discussed before via a combination of fractional space and multiplicative noise. Specifically, the exact solutions for the stochastic Bogoyavlenskii equation have never been obtained before. In addition, after we get the solutions by two various methods including the exp $(-\mathsf{\Phi }(\zeta \left)\right)$-expansion and sine–cosine, we address the impact of noise on these solutions. As we know, the stochastic solutions are more accurate than deterministic solutions. Therefore, the acquired solutions are very useful for scientists to describe a wide variety of complicated physical phenomena because Equation (1) is used to explain the wave of leading fluid flow, plasma physics, and the hydrodynamic model of shallow-water waves. We also show how the stochastic term affects the behavior of SFSBE analytical solutions by using graphical representations for various noise intensity values. Moreover, some previously obtained solutions, for instance the one stated in [34,42], were expanded.

The following is a summary of this paper: In Section 2, we give a definition and features of the CD and Brownian motion. In Section 3, we utilize a convenient wave transformation to attain the wave equation of the SFSBE (1). In Section 4, the analytical space-fractional stochastic solution of the SFSBE (1) is obtained. While in Section 5, we investigate how the Brownian motion influences the SFSBE (1) solution’s behavior. Finally, we present the paper’s conclusions.

2. Preliminaries

Here, we state some definitions and features of the CD [33] and Brownian motion. First, we define the CD as follows:

Definition 1.

Define the CD of $\varphi :(0,\infty )\to \mathbb{R}$ of order $\alpha \in (0,1]$ as

$${\mathcal{D}}_x^{\alpha }\varphi \left(x\right)=\underset{\kappa \to 0}{lim}\frac{\varphi (x+\kappa x^{1-\alpha })-\varphi \left(x\right)}{\kappa }.$$

Theorem 1.

Suppose $\varphi ,g:(0,\infty )\to \mathbb{R}$ are differentiable, and α is a differentiable function too, then, the next rule satisfies:

$${\mathcal{D}}_x^{\alpha }(\varphi \circ g)\left(x\right)=x^{1-\alpha }{g}^{\prime }\left(x\right){\varphi }^{\prime }\left(g\left(x\right)\right).$$

The following include some features of the CD:

• ${\mathcal{D}}_x^{\alpha }[c_1\varphi \left(x\right)+c_{2}g\left(x\right)]=c_1{\mathcal{D}}_x^{\alpha }\varphi \left(x\right)+c_2{\mathcal{D}}_x^{\alpha }g\left(x\right),c_1,c_2\in \mathbb{R}$
• ${\mathcal{D}}_x^{\alpha }\left[C\right]=0,C$ is a constant,
• ${\mathcal{D}}_x^{\alpha }\left[x^{\gamma }\right]=\gamma x^{\gamma -\alpha },\gamma \in \mathbb{R},$
• ${\mathcal{D}}_x^{\alpha }g\left(x\right)=x^{1-\alpha }\frac{dg}{dx}.$

In the next, we define the Brownian motion:

Definition 2

(cf. [52]). For $t\ge 0$, $\beta \left(t\right)$ is called Brownian motion if it satisfies: (1) $\beta \left(t\right)$ is continuous, (2) $\beta \left(0\right)=0$, (3) $\beta \left(t\right)$ has independent increments, (4) $\beta \left(t\right)-\beta \left(s\right)$ has normal distribution with variance $t-s$ and mean $0.$

It is worth noting that there are two types of stochastic integrals that are widely used: Stratonovich and Itô [53]. Modeling considerations usually decide the type is appropriate, but once one is selected, a similar equation of the other type can be established with the same solutions. Hence, the following is a possible switch between Stratonovich (written as ${\int }_0^t\Lambda \circ d\beta$) and Itô (written as ${\int }_0^t\Lambda d\beta$):

$${\int }_0^t\Lambda (s,Y_s)d\beta \left(s\right)={\int }_0^t\Lambda (s,Y_s)\circ d\beta \left(s\right)-\frac{1}{2}{\int }_0^t\Lambda (s,Y_s)\frac{\partial \Lambda (s,Y_s)}{\partial x}ds,$$

(2)

where $\{Y_t,$$t\ge 0\}$ is a stochastic process and $\Lambda$ is considered to be sufficiently regular.

3. The Wave Equation

Here, we implement the following wave transformation

$$\psi (x,y,t)=\chi \left(\eta \right)e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},w(x,y,t)=\mathsf{\Theta }\left(\eta \right),\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt,$$

(3)

to get wave equation for SFSBE (1). $\chi$ and $\mathsf{\Theta }$ defined in (3) are real deterministic functions, $\ell ,$$m,$k are constants. We see that

$$\begin{array}{ccc}\hfill {\mathcal{D}}_x^{\alpha }\psi & =& \ell {\chi }^{\prime }{e}^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},{\mathcal{D}}_y^{\alpha }\psi =m{\chi }^{\prime }{e}^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},\hfill \\ \hfill {\mathcal{D}}_{xxy}^{3\alpha }\psi & =& m{\ell }^2{\chi }^{‴}{e}^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},{\mathcal{D}}_x^{\alpha }w=\ell {\mathsf{\Theta }}^{\prime },\hfill \end{array}$$

(4)

and

$$\begin{array}{ccc}\hfill d\psi & =& [(k{\chi }^{\prime }+\frac{1}{2}{\rho }^2\chi -{\rho }^2\chi )dt-\rho \chi d\beta ]e^{[-\rho \beta (t)-{\rho }^{2}t]}\hfill \\ & =& [(k{\chi }^{\prime }-\frac{1}{2}{\rho }^2\chi )dt-\rho \chi d\beta ]e^{[-\rho \beta (t)-{\rho }^{2}t]},\hfill \\ & =& [k{\chi }^{\prime }dt-\rho \chi \circ d\beta ]e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},\hfill \end{array}$$

(5)

using Equation (2) in differential form and multiplying it by $-1$, we get

$$d\psi =[k{\chi }^{\prime }dt-\rho \chi \circ d\beta ]e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}.$$

Embedding Equation (3) into (1) and utilizing (4) and (6), we obtain

$$\begin{array}{c}\hfill 4k{\chi }^{\prime }+m{\ell }^2{\chi }^{‴}-4m{\chi }^2{\chi }^{\prime }{e}^{(2\rho \beta \left(t\right)-2{\rho }^{2}t)}-4\ell {\chi }^{\prime }\mathsf{\Theta }=0,\\ m{\chi }^{\prime }\chi e^{[2\rho \beta \left(t\right)-2{\rho }^{2}t]}=\ell {\mathsf{\Theta }}^{\prime }.\end{array}$$

(6)

Considering expectation on both sides, where $\mathsf{\Theta }$ and $\chi$ are deterministic functions, we get

$$\begin{array}{ccc}\hfill 4k{\chi }^{\prime }+m{\ell }^2{\chi }^{‴}-4m{\chi }^2{\chi }^{\prime }{e}^{-2{\rho }^{2}t}\mathbb{E}[e^{2\rho \beta \left(t\right)}]-4\ell {\chi }^{\prime }\mathsf{\Theta }& =& 0,\hfill \\ \hfill m{\chi }^{\prime }\chi e^{-2{\rho }^{2}t}\mathbb{E}[e^{2\rho \beta \left(t\right)}]& =& \ell {\mathsf{\Theta }}^{\prime }.\hfill \end{array}$$

(7)

In fact, for every standard normal process $Z,$$\rho \beta \left(t\right)$ is distributed similarly to $\rho \sqrt{t}Z$. Therefore $\mathbb{E}(e^{2\rho \beta \left(t\right)})=e^{2{\rho }^{2}t}$. Now, Equation (7) takes the form

$$\begin{array}{ccc}\hfill 4k{\chi }^{\prime }+m{\ell }^2{\chi }^{‴}-4m{\chi }^2{\chi }^{\prime }-4\ell {\chi }^{\prime }\mathsf{\Theta }& =& 0,\hfill \\ \hfill m{\chi }^{\prime }\chi & =& \ell {\mathsf{\Theta }}^{\prime }.\hfill \end{array}$$

(8)

We have by integrating the second equation in (8) once and setting the integration constant to zero

$$\frac{1}{2}m{\chi }^2=\ell \mathsf{\Theta }.$$

(9)

By plugging Equation (9) into first equation in (8), we get

$${\chi }^{‴}-\frac{6}{{\ell }^2}{\chi }^2{\chi }^{\prime }+\frac{4k}{m{\ell }^2}{\chi }^{\prime }=0.$$

(10)

Integrating (10) once and putting the constant of integration equal zero, we obtain

$${\chi }^{″}-\frac{2}{{\ell }^2}{\chi }^3+\frac{4k}{m{\ell }^2}\chi =0.$$

(11)

4. Analytical Solutions of SFSBE

To get different analytical solutions of the SFSBE, we can apply many methods such as Lie symmetry methods, Painlevé expansion, sine–cosine, generalized Riccati equation, tanh–coth, $exp(-\mathsf{\Phi })$-expansion, auxiliary equation, variational iteration, Backlund transformation, first integral, etc. However, in this section, we use two distinct methods such as $exp(-\mathsf{\Phi })$-expansion and sine–cosine methods.

4.1. The $exp(-\mathsf{\Phi }(\eta \left)\right)$-Expansion Method

Let us employ here the $exp(-\mathsf{\Phi }(\eta \left)\right)$-expansion method [21,22,23,34] to find the traveling wave solutions of (11) and then the exact solutions shown in Equation (1). First, we assume the solutions of (11) are

$$\chi \left(\eta \right)=\sum _{i=0}^N{\hslash }_i{[exp(-\mathsf{\Phi }\left(\eta \right))]}^i,\mathrm{such}\mathrm{that}{\hslash }_N\ne 0,$$

(12)

where ${\hslash }_0,{\hslash }_1\cdots ,{\hslash }_N$ are constants to be calculated later. $\mathsf{\Phi }=\mathsf{\Phi }\left(\eta \right)$ fulfills the next ODE:

$${\mathsf{\Phi }}^{\prime }=exp(-\mathsf{\Phi })+aexp\left(\mathsf{\Phi }\right)+b,$$

(13)

where $b,a$ are arbitrary constants. By balancing ${\chi }^3$ and ${\chi }^{″}$ in (11), yields $N=1$.

Hence, the solution of Equation (11) becomes

$$\chi \left(\eta \right)={\hslash }_0+{\hslash }_1[exp(-\mathsf{\Phi }\left(\eta \right))].$$

(14)

By plugging Equation (14) into Equation (11), and utilizing Equation (13), we obtain a polynomial of $exp(-\mathsf{\Phi }).$ After that, we set the coefficients of $exp(-\mathsf{\Phi })$ to zero, which gives

$$2{\hslash }_1-\frac{2}{{\ell }^2}{\hslash }_1^3=0,$$

$$3b{\hslash }_1-\frac{6}{{\ell }^2}{\hslash }_0{\hslash }_1^2=0,$$

$$2a{\hslash }_1+b^2{\hslash }_1-\frac{6}{{\ell }^2}{\hslash }_0^2{\hslash }_1+\frac{4k}{m{\ell }^2}{\hslash }_1=0,$$

$$ab{\hslash }_1-\frac{2}{{\ell }^2}{\hslash }_0^3+\frac{4k}{m{\ell }^2}{\hslash }_0=0.$$

(15)

Solving the above equations, we have

$${\hslash }_0=\frac{\ell b}{2},{\hslash }_1=\ell ,k=\frac{m{\ell }^2}{8}(4a-b^2),$$

(16)

where $b,a$ are arbitrary constants.

Putting the values of ${\hslash }_0,{\hslash }_1$ into Equation (14), we get

$$\chi \left(\eta \right)=\pm \frac{\ell b}{2}\pm \ell exp(-\mathsf{\Phi }\left(\eta \right)).$$

(17)

There are five cases for solutions of Equation (13) depending on the value of b and a:

Case I: When $a\ne 0,b^2-4a>0$, then the solution of Equation (13) is

$$\mathsf{\Phi }\left(\eta \right)=ln\left(\frac{\sqrt{(b^2-4a)}tanh\left(\frac{\sqrt{(b^2-4a)}}{2}(\eta +\mathcal{C})\right)+b}{-2a}\right).$$

(18)

where $\mathcal{C}$ is an arbitrary constant. Plugging Equation (18) into (17), we attain

$${\chi }_1\left(\eta \right)=\pm \frac{\ell b}{2}\mp \frac{2\ell a}{\sqrt{(b^2-4a)}tanh(\frac{\sqrt{(b^2-4a)}}{2}(\eta +\mathcal{C}))+b}.$$

(19)

Therefore, the solutions of SFSBE (1), by substituting Equation (19) into Equation (3) and using Equation (9), are

$${\psi }_1(t,x,y)=\pm e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}\left(\frac{\ell b}{2}-\frac{2\ell a}{\sqrt{(b^2-4a)}tanh(\frac{\sqrt{(b^2-4a)}}{2}(\eta +\mathcal{C}))+b}\right),$$

(20)

$$w_1(t,x,y)=\pm \frac{m}{2}{\left(\frac{b}{2}+\frac{2a}{\sqrt{(b^2-4a)}tanh\left(\frac{\sqrt{(b^2-4a)}}{2}(\eta +\mathcal{C})\right)+b}\right)}^2,$$

(21)

where $\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }-\frac{m{\ell }^2}{8}(b^2-4a)t$.

Case II: When $a\ne 0,b^2-4a<0$, then the solution of Equation (13) is

$$\mathsf{\Phi }\left(\eta \right)=ln\left(\frac{\sqrt{(4a-b^2)}tan\left(\frac{\sqrt{(4a-b^2)}}{2}(\eta +\mathcal{C})\right)-b}{2a}\right).$$

(22)

Substituting Equation (22) into (17), we have

$${\chi }_2\left(\eta \right)=\pm \frac{\ell b}{2}\pm \frac{2\ell a}{\sqrt{(4a-b^2)}tan(\frac{\sqrt{(4a-b^2)}}{2}(\eta +\mathcal{C}))-b}.$$

(23)

Thus, the solutions of SFSBE (1), by substituting Equation (23) into Equation (3) and using Equation (9), are

$${\psi }_2(t,x,y)=\pm e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}\left(\frac{\ell b}{2}+\frac{2\ell a}{\sqrt{(4a-b^2)}tan(\frac{\sqrt{(4a-b^2)}}{2}(\eta +\mathcal{C}))-b}\right),$$

(24)

$$w_2(t,x,y)=\pm \frac{m}{2}{\left(\frac{b}{2}+\frac{2a}{\sqrt{(4a-b^2)}tan(\frac{\sqrt{(4a-b^2)}}{2}(\eta +\mathcal{C}))-b}\right)}^2,$$

(25)

where $\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+\frac{m{\ell }^2}{8}(4a-b^2)t$.

Case III: When $a=0$ and $b\ne 0$, then the solutions of Equation (13) is

$$\mathsf{\Phi }\left(\eta \right)=-ln\left(\frac{b}{exp\left(b\right(\eta +\mathcal{C}\left)\right)-1}\right).$$

(26)

Substituting Equation (18) into (17), we obtain

$${\chi }_3\left(\eta \right)=\pm \frac{\ell b}{2}\pm \frac{\ell b}{exp\left(b\right(\eta +\mathcal{C}\left)\right)-1}.$$

(27)

Therefore, the solutions of SFSBE (1), by substituting Equation (27) into Equations (3) and using Equation (9), are

$${\psi }_3(t,x,y)=\pm e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}\left(\frac{\ell b}{2}+\frac{\ell b}{exp\left(b\right(\eta +\mathcal{C}\left)\right)-1}\right),$$

(28)

$$w_3(t,x,y)=\pm \frac{m}{2}{\left(\frac{b}{2}+\frac{b}{exp\left(b\right(\eta +\mathcal{C}\left)\right)-1}\right)}^2,$$

(29)

where $\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }-\frac{m{\ell }^2{b}^2}{8}t$.

Case IV: When $a\ne 0,b\ne 0$ and $b^2-4a=0$, then the solutions of Equation (13) is

$$\mathsf{\Phi }\left(\eta \right)=ln\left(-\frac{2b(\eta +\mathcal{C})+4}{b^2(\eta +\mathcal{C}))}\right).$$

(30)

Substituting Equation (30) into (17), we obtain

$${\chi }_4\left(\eta \right)=\pm \frac{\ell b}{2}\mp \frac{b^2\ell (\eta +\mathcal{C})}{2b(\eta +\mathcal{C}))+4}.$$

(31)

Thus, the solutions of SFSBE (1), by substituting Equation (31) into Equation (3) and using Equation (9), are

$${\psi }_4(t,x,y)=\pm e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}\left(\frac{\ell b}{2}-\frac{b^2\ell (\eta +\mathcal{C})}{2b(\eta +\mathcal{C}))+4}\right),$$

(32)

$$w_4(t,x,y)=\pm \frac{m}{2}{\left(\frac{b}{2}+\frac{b^2(\eta +\mathcal{C})}{2b(\eta +\mathcal{C})+4}\right)}^2,$$

(33)

where $\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }$.

Case V: When $a=0,b=0$ and $b^2-4a=0$, then the solution of Equation (13) is

$$\mathsf{\Phi }\left(\varsigma \right)=ln(\varsigma +E).$$

(34)

Substituting Equation (34) into (17), we obtain

$${\chi }_5\left(\eta \right)=\frac{\pm \ell }{\eta +\mathcal{C}}.$$

(35)

Therefore, the solutions of SFSBE (1), by substituting Equation (35) into Equation (3) and using Equation (9), are

$${\psi }_5(t,x,y)=\pm \ell e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]}\left(\frac{1}{\eta +\mathcal{C}}\right),$$

(36)

$$w_5(t,x,y)=\pm \frac{m}{\ell }{\left(\frac{1}{\eta +\mathcal{C}}\right)}^2,$$

(37)

where $\eta =\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }.$

Remark 1.

If we set $\rho =0$ and $\alpha =1$ in Equations (20), (21), (24), (25), (28), (29), (32), (33), (36) and (37), then we get the same results reported in [34].

4.2. Sine–Cosine Method

We implement here the sine–cosine method. We assume the solution of Equation (11) depend on [15] are

$$\chi \left(\eta \right)=A{\mathsf{\Psi }}^n,$$

(38)

where

$$\mathsf{\Psi }\left(\eta \right)=cos\left(B\eta \right)\mathrm{or}\mathsf{\Psi }\left(\eta \right)=sin\left(B\eta \right),$$

(39)

where A and B are undefined constants. Putting Equation (38) into Equation (11), we have

$$A{B}^2[-n^2{\mathsf{\Psi }}^n+n(n-1){\mathsf{\Psi }}^{n-2}]-\frac{2}{{\ell }^2}{A}^3{\mathsf{\Psi }}^{3n}+\frac{4k}{m{\ell }^2}A{\mathsf{\Psi }}^n=0,$$

rewriting the equation above

$$(\frac{4k}{m{\ell }^2}A-A{B}^2{n}^2){\mathsf{\Psi }}^n+n(n-1)A{B}^2{\mathsf{\Psi }}^{n-2}-\frac{2}{{\ell }^2}{A}^3{\mathsf{\Psi }}^{3n}=0.$$

(40)

Comparing the $\mathsf{\Psi }$ term in Equation (40), we get

$$3n=n-2,$$

hence

$$n=-1.$$

Now, Equation (14) becomes

$$(\frac{4k}{m{\ell }^2}A-A{B}^2){\mathsf{\Psi }}^{-1}+(\frac{-2}{{\ell }^2}{A}^3+2A{B}^2){\mathsf{\Psi }}^{-3}=0.$$

Inserting each coefficient of ${\mathsf{\Psi }}^{-3}$ and ${\mathsf{\Psi }}^{-1}$ equal zero, we obtain

$$\frac{-2}{{\ell }^2}{A}^3+2A{B}^2=0,$$

(41)

and

$$\frac{4k}{m{\ell }^2}A-A{B}^2=0.$$

(42)

By solving these equation we get

$$A=\pm 2\sqrt{\frac{k}{m}}\mathrm{and}B=\pm \frac{2}{\ell }\sqrt{\frac{k}{m}}.$$

(43)

There are two cases:

First case: If $\frac{k}{m}>0,$ hence the solutions of Equation (11) take the form:

$$\chi \left(\eta \right)=\pm 2\sqrt{\frac{k}{m}}sec(\frac{2}{\ell }\sqrt{\frac{k}{m}}\eta )\mathrm{or}\chi \left(\eta \right)=\pm 2\sqrt{\frac{k}{m}}csc(\frac{2}{\ell }\sqrt{\frac{k}{m}}\eta ).$$

Therefore, the solutions of SFSBE (1) are

$${\psi }_{2,1}(x,y,t)=\pm 2\sqrt{\frac{k}{m}}sec(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt))e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},$$

(44)

$$w_{2,1}(x,y,t)=\frac{2k}{\ell }{sec}^2(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt)),$$

(45)

or

$${\psi }_{2,2}(x,y,t)=\pm 2\sqrt{\frac{k}{m}}csc(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt))e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},$$

(46)

$$w_{2,2}(x,y,t)=\frac{2k}{\ell }{csc}^2(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt)).$$

(47)

Second case: If $\frac{k}{m}<0,$ then the solutions of Equation (11) are

$$\chi \left(\eta \right)=\pm 2\sqrt{\frac{k}{m}}\sec \mathrm{h}(\frac{2}{\ell }\sqrt{\frac{k}{m}}\eta )\mathrm{or}\chi \left(\eta \right)=\pm 2\sqrt{\frac{k}{m}}\csc \mathrm{h}(\frac{2}{\ell }\sqrt{\frac{k}{m}}\eta ).$$

Therefore, the solutions of SFSBE (1) take the form

$${\psi }_{2,3}(x,y,t)=\pm 2\sqrt{\frac{k}{m}}\sec \mathrm{h}(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt))e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},$$

(48)

$$w_{2,3}(x,y,t)=\frac{2k}{\ell }{\sec \mathrm{h}}^2(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt)),$$

(49)

or

$${\psi }_{2,4}(x,y,t)=\pm 2\sqrt{\frac{k}{m}}\csc \mathrm{h}(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt))e^{[-\rho \beta \left(t\right)-{\rho }^{2}t]},$$

(50)

$$w_{2,4}(x,y,t)=\frac{2k}{\ell }{\csc \mathrm{h}}^2(\frac{2}{\ell }\sqrt{\frac{k}{m}}(\frac{\ell }{\alpha }{x}^{\alpha }+\frac{m}{\alpha }{y}^{\alpha }+kt)).$$

(51)

Remark 2.

If we set $\rho =0$ and $\alpha =1$ in Equations (44)–(51), then we acquire the same solutions stated in [42].

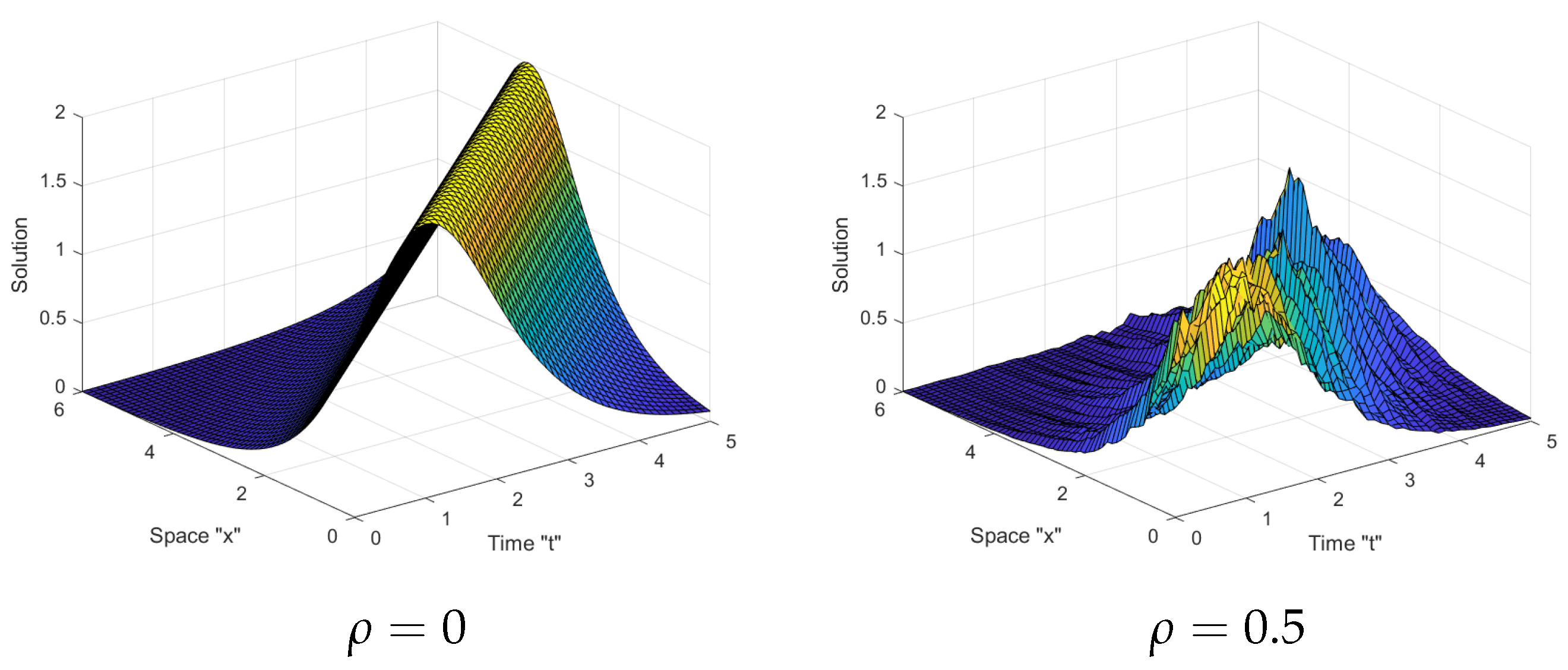
5. Impact of Multiplicative Brownian Motion

We address in this section the influence of the multiplicative Brownian motion on the solutions of the SFSBE (1). We employ MATLAB tools [54] to display some graphical representations for distinct values of the noise strength and explore the influence of multiplicative Brownian motion on these solutions. We fixed the parameters $\ell =1,k=-2,m=1.$ In the following, we plot the solution (48) for $x\in [0,5]$, $y=1$ and $t\in [0,5]$:

When we look at Figure 1 and Figure 2 below, we can see that:

• The surface shrank as the order of the fractional operator $\alpha$ decreases,
• At $\rho =0,$ the surface is not completely flat and has some fluctuation,
• After minor transit patterns, the surface becomes considerably flatter when noise is included and its strength is increased $\rho =0.5,1,2$.

Thus, we can deduce from Figure 1, Figure 2 and Figure 3 that the multiplicative Brownian motion influences the solutions of SFSBE and it stabilizes the solutions around zero.

6. Conclusions

In this study, we considered the stochastic fractional-space Bogoyavlenskii equation with multiplicative Brownian motion in the Stratonovich sense. We attained the exact fractional stochastic solutions of the SFSBE via two distinct methods: for instance, the $exp(-\mathsf{\Phi }(\eta \left)\right)$-expansion method and sine–cosine method. We extended some previously acquired results, including the results sated in [34,42]. These forms of solutions can be applied to a wide range of complex physical phenomena because Equation (1) is used to explain the wave of leading fluid-flow, plasma physics, and the hydrodynamic model of shallow-water waves. Finally, we demonstrated how Brownian motion affects solution behavior and indicated that Brownian motion stabilizes the solutions of SFSBE around zero. We can consider multi-dimensional multiplicative noise and additive noise in future work.