Optimal Frequency Control of Multi-Area Hybrid Power System Using New Cascaded TID-PIλDμN Controller Incorporating Electric Vehicles
Abstract
:1. Introduction
1.1. Literature Review
1.2. Paper Contribution
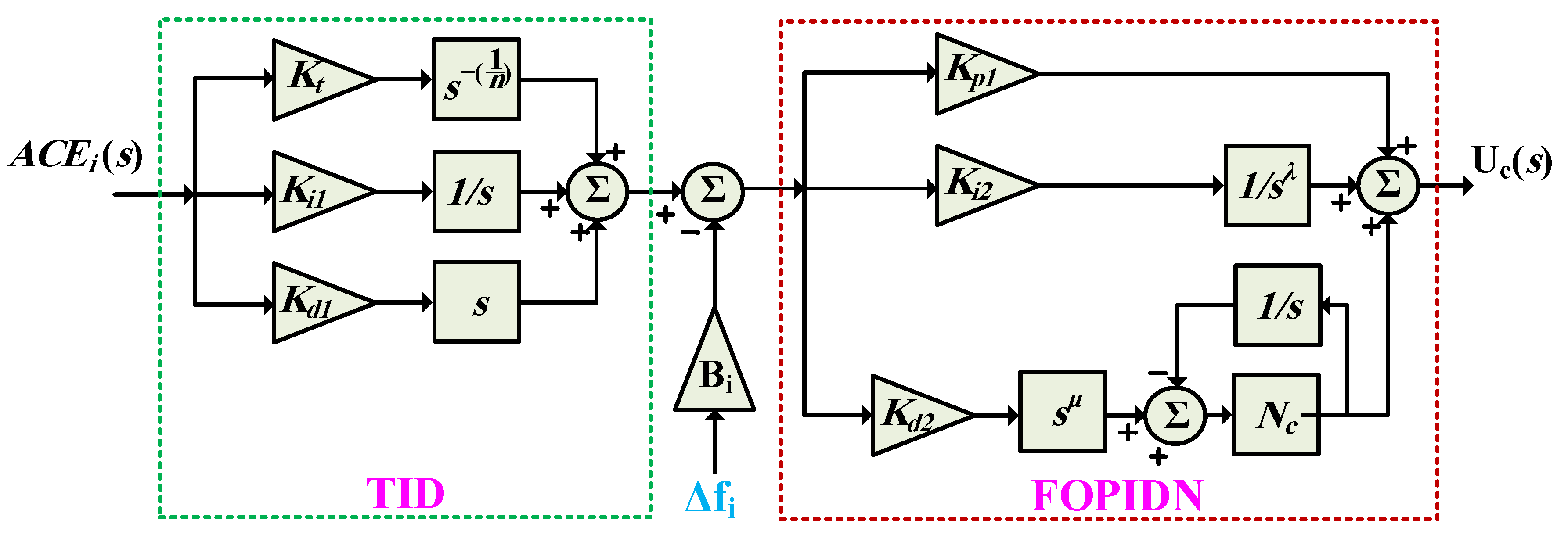
- An improved cascaded fractional order based load frequency control method is proposed for the two-area interconnected power system. The proposed controller uses the cascade structure of the tilt-integral-derivative (TID) with the fractional order proportional-integral-derivative with filter (FOPID or PIDN) controller (Cascaded TID-FOPIDN or TID-PIDN controller). Based on the authors’ knowledge, this is the first time that a cascaded TID-FOPIDN control structure has been proposed for LFC in interconnected power systems.
- Compared to existing LFC systems and existing cascaded LFC structures, the proposed controller is advantageous at mitigating the frequency and tie-line power fluctuations in comparison to the studied LFC from the literature. Comparisons are provided to verify the superiority of the proposed controller over the existing featured FO LFC methods. Moreover, various performance metrics are compared with the featured cascaded control structure in the literature for the various considered scenarios.
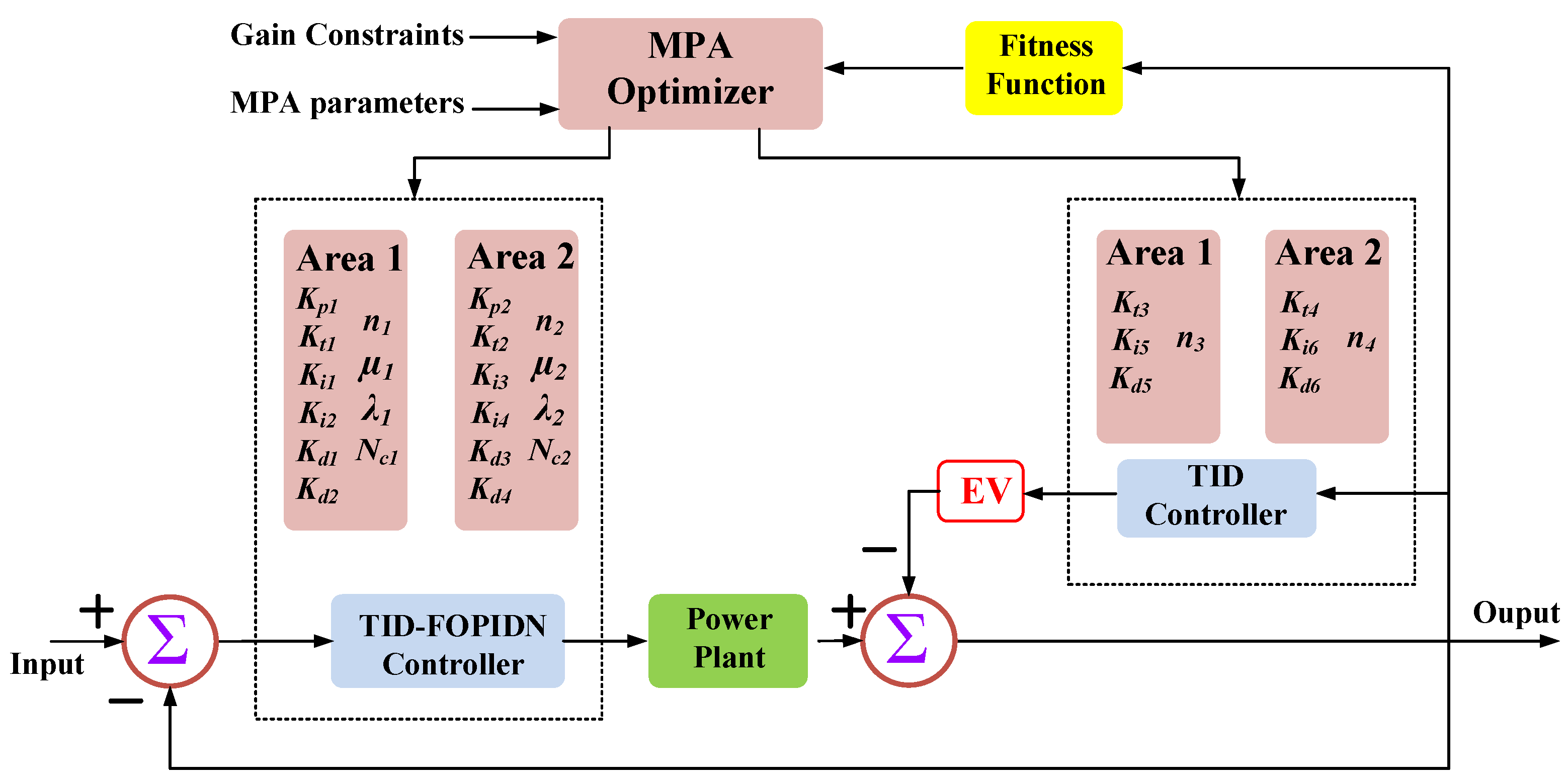
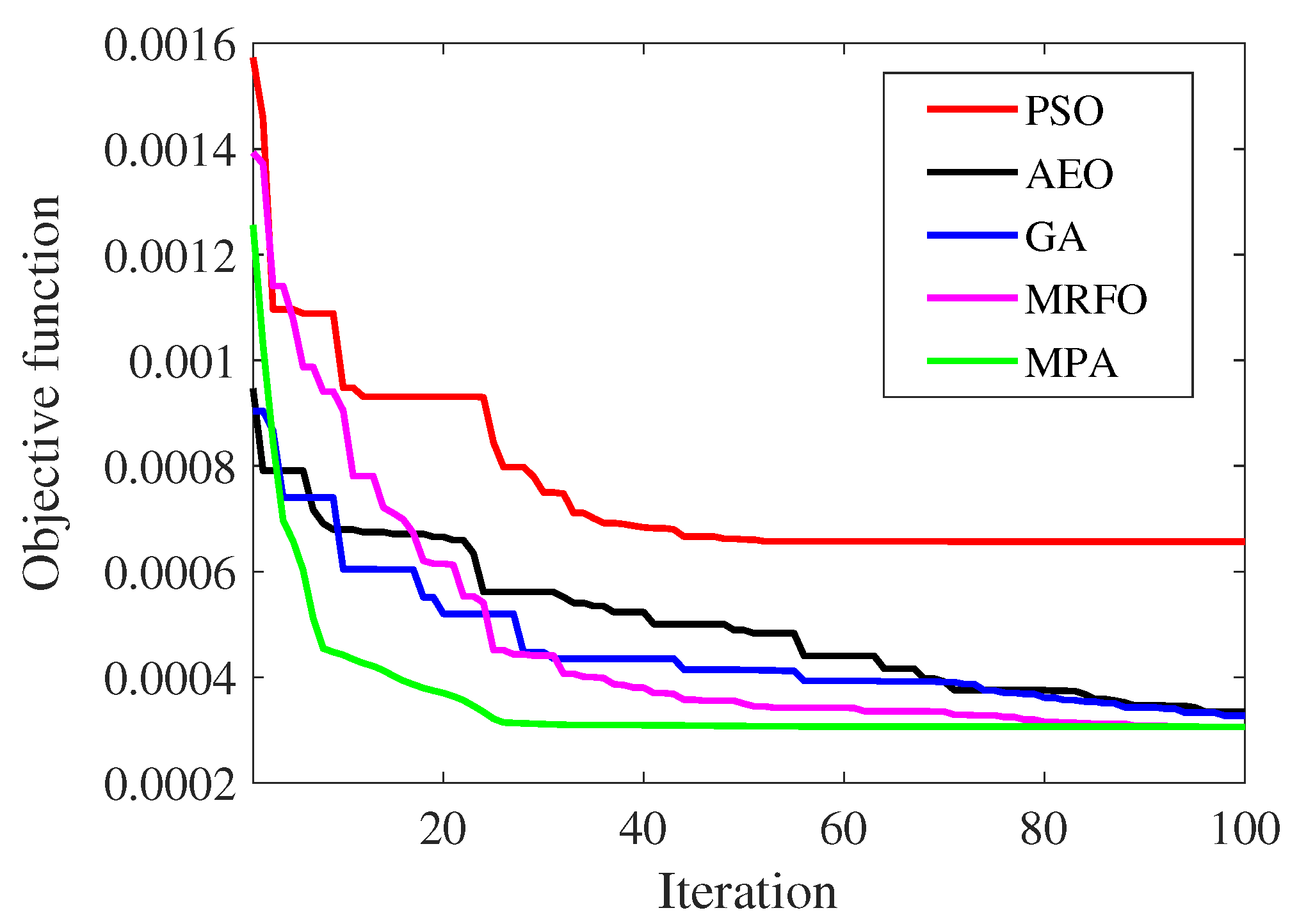
- The performance of the proposed LFC method is enhanced using the marine predators optimization algorithm (MPA) to optimally determine the parameters of the proposed controller. Additionally, the MPA performance is verified through comparisons with the other existing algorithms.
- A cooperative control of the connected electric vehicles (EVs) based on TID fractional order control is also proposed in this paper. The proposed controller is capable of effectively participateing in regulating the frequency of the interconnected power systems.
- The proposed LFC and EV control system are also integrated with the stochastic conditions and characteristics of renewable energy sources to demonstrate the robustness and superiority of the proposed work.
2. Models of Various Elements in the Multi-Source Power System
2.1. The Case Study
2.2. The PV Plant Model
2.3. The Wind Turbine Model
2.4. The Model of EV Systems
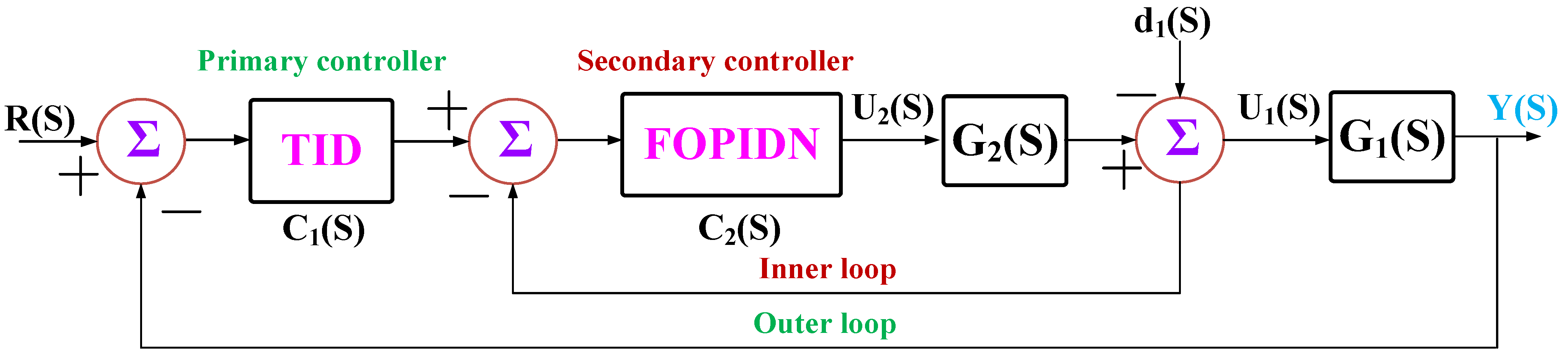
3. The Proposed Optimized Controller
3.1. Overview of Existing Controllers
3.2. The Proposed TID-FOPIDN Controller
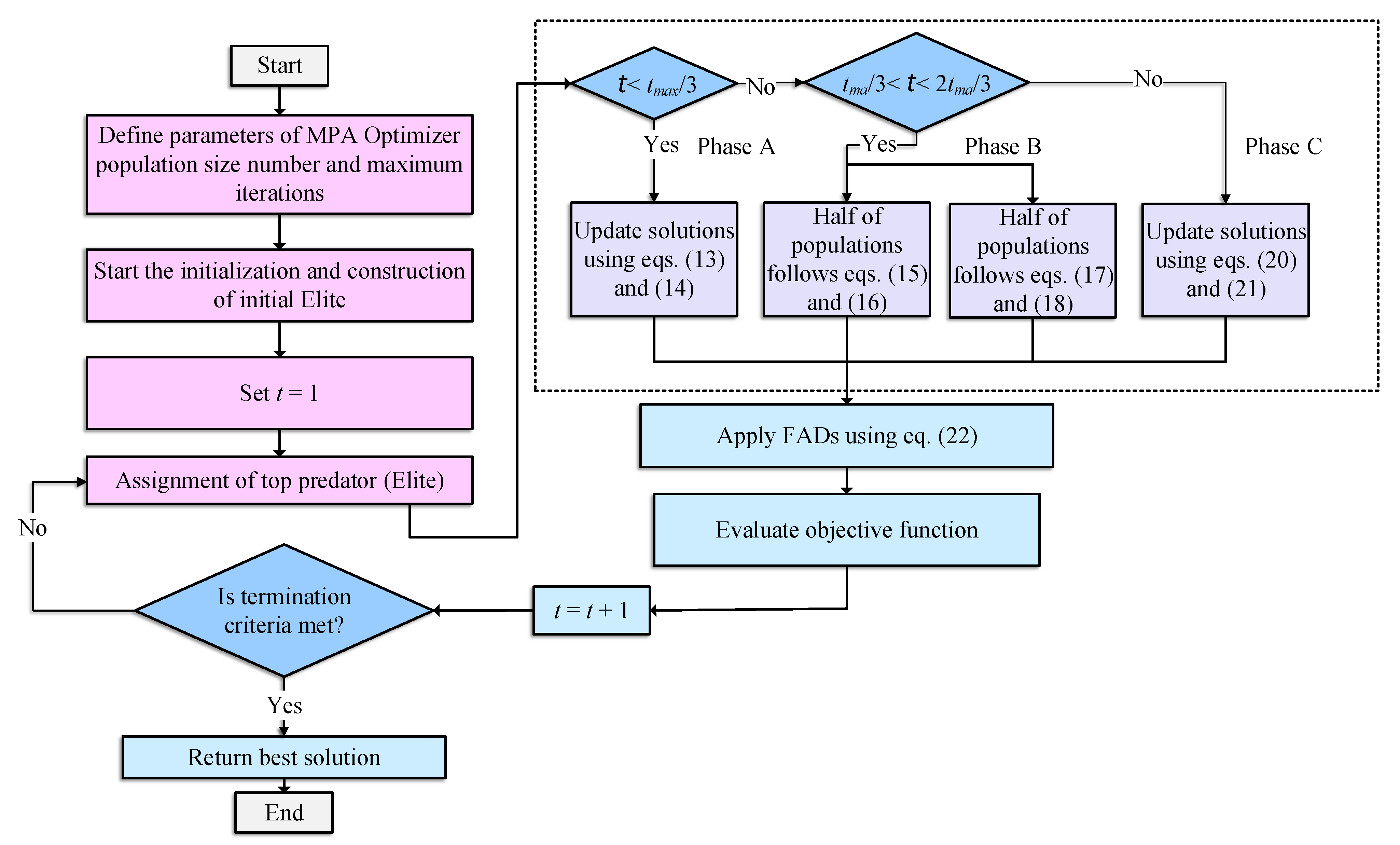
3.3. The MPA Optimization
3.4. The Proposed Optimization Process
- The integral squared errors (ISE),
- The integral time squared errors (ITSE),
- The integral absolute errors (IAE), and
- The integral time absolute errors (ITAE),
4. Simulation Results and Performance Verification
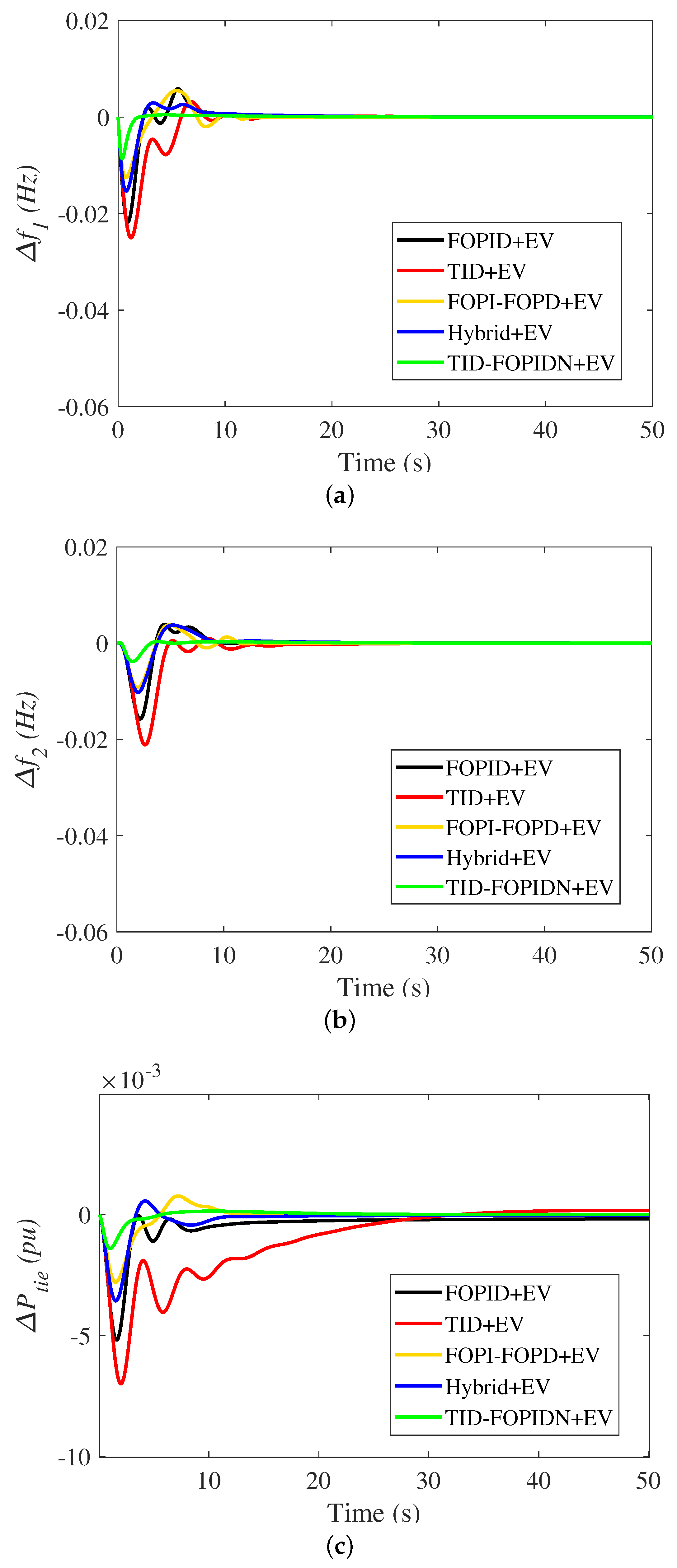
- Scenario 1: The impact of step load perturbation (SLP) with and without EVs;
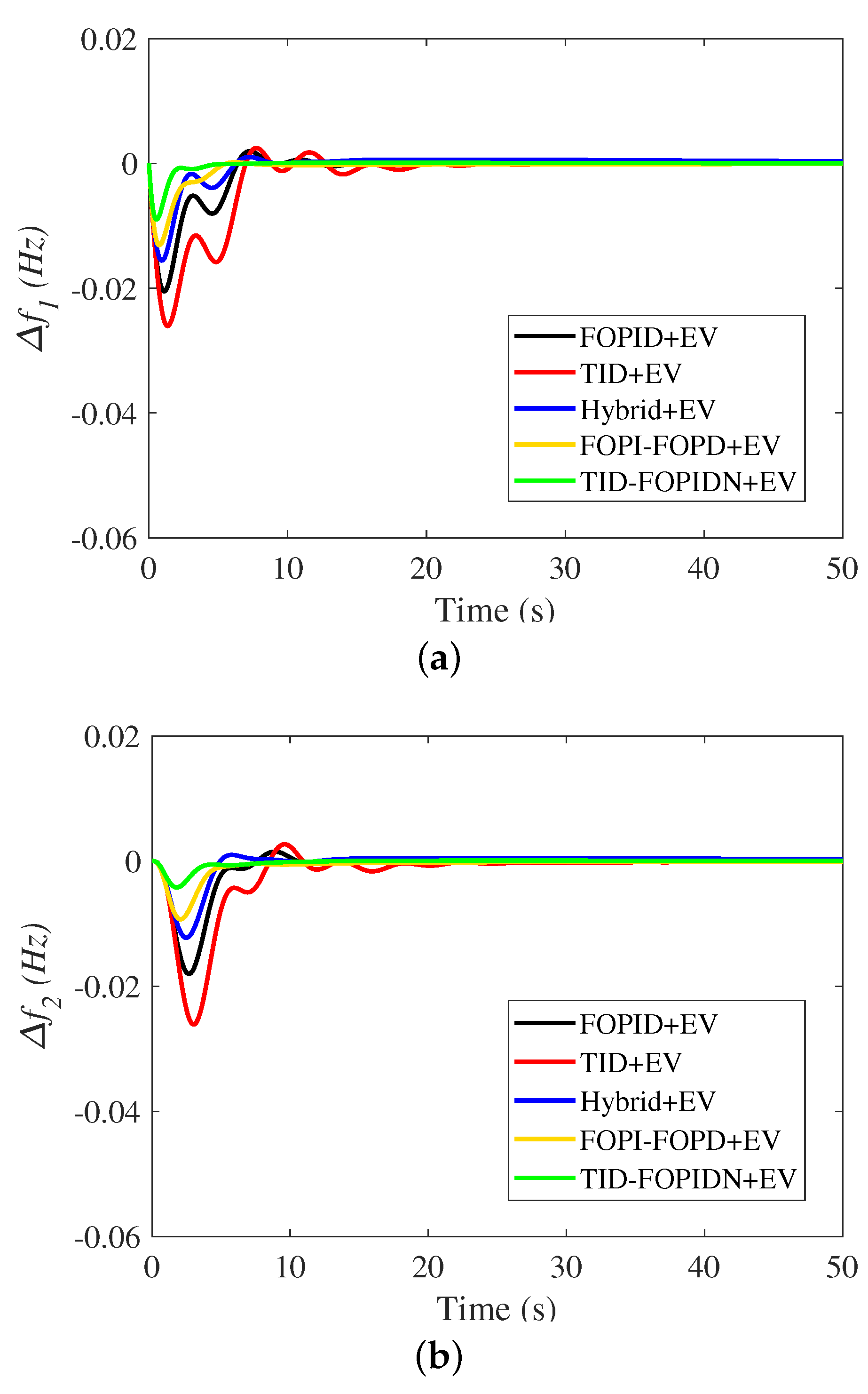
- Scenario 2: The impact of SLP under the generation outage effects;
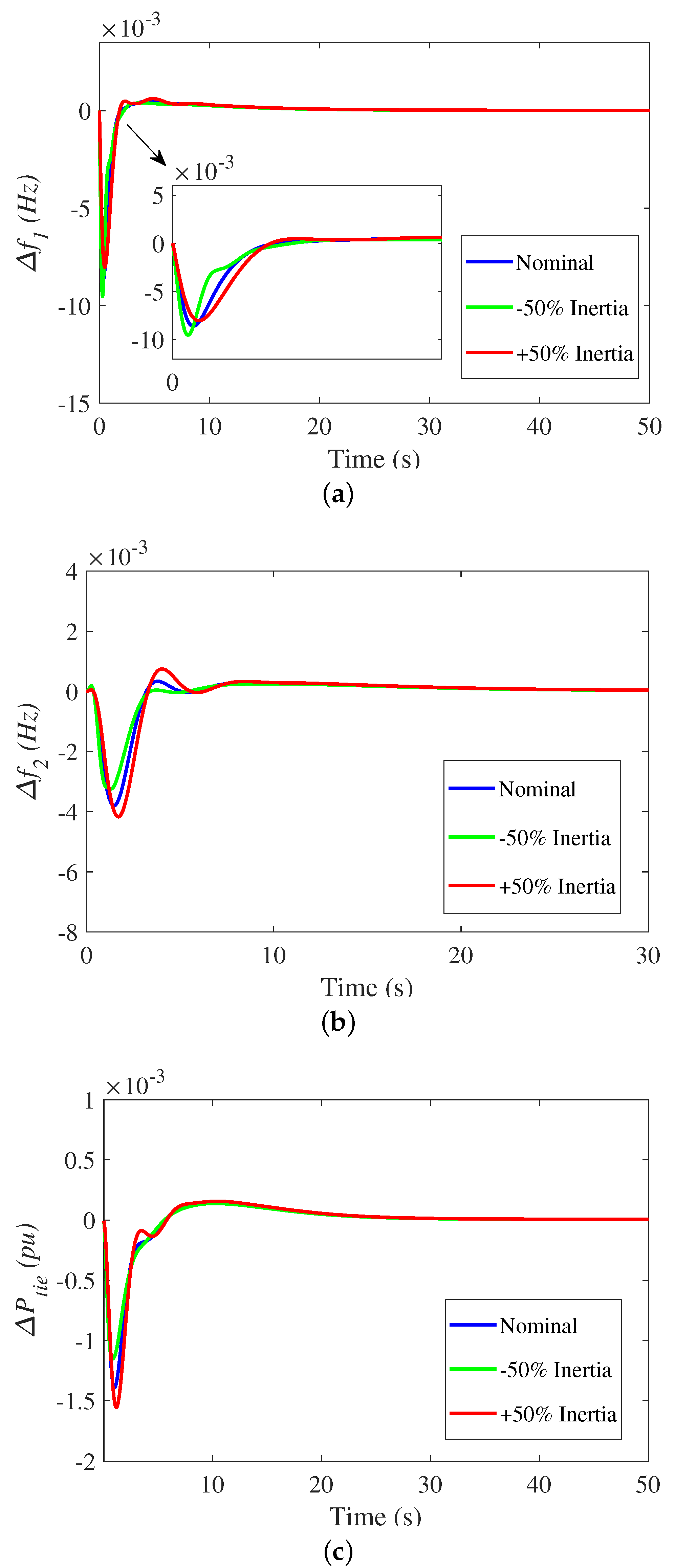
- Scenario 3: The impact of uncertainties in the power system inertia;
- Scenario 4: The impact of random load pattern;
- Scenario 5: The impact of RES fluctuations and load variations.
4.1. Scenario 1: Impact of SLP
4.2. Scenario 2: The Impact of Generation Outage
4.3. Scenario 3: The Impact of Uncertainties in the Power System Inertia
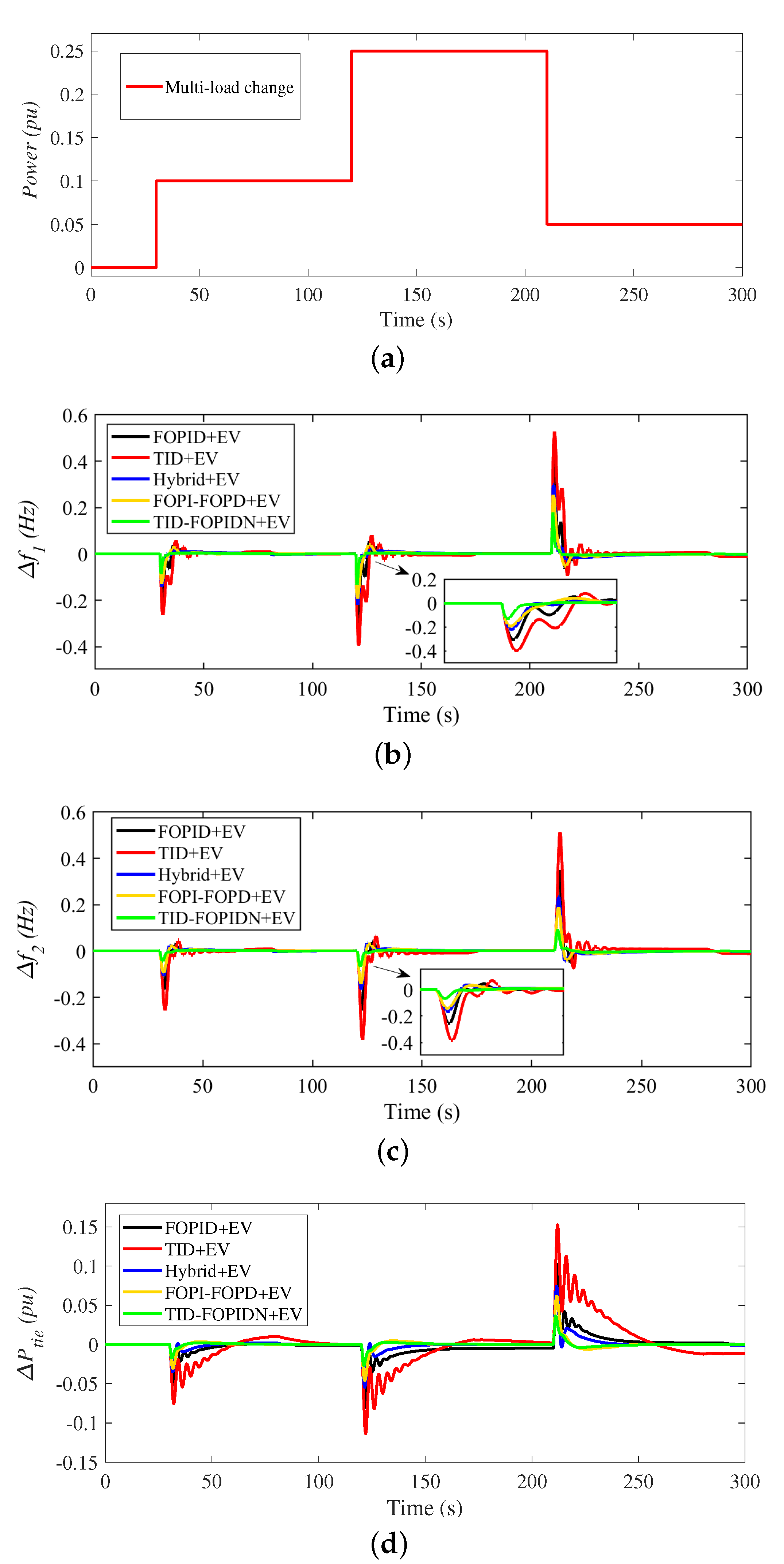
4.4. Scenario 4: The Impact of Multi-Step Load
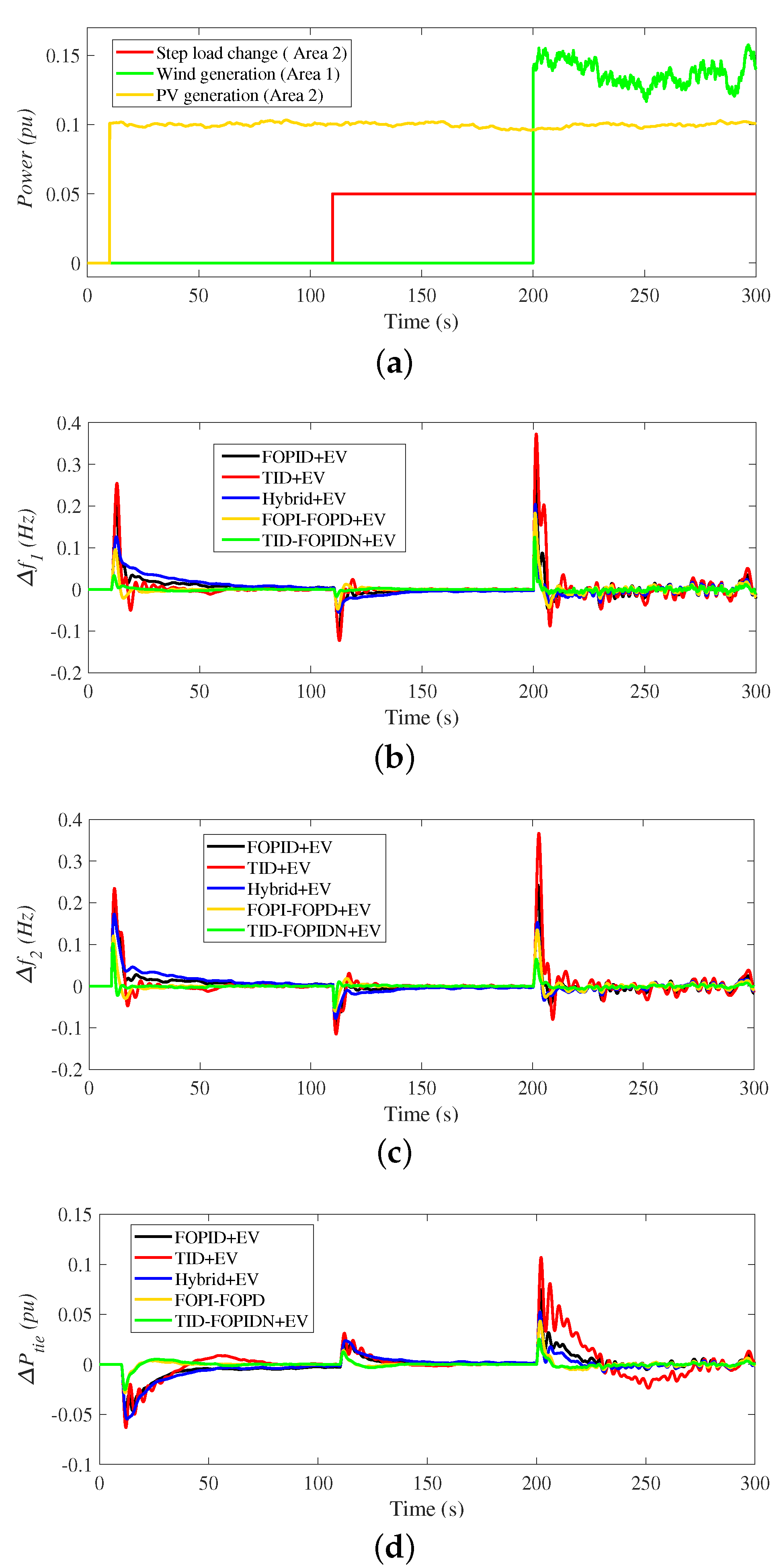
4.5. Scenario 5: The Impact of RES Fluctuations
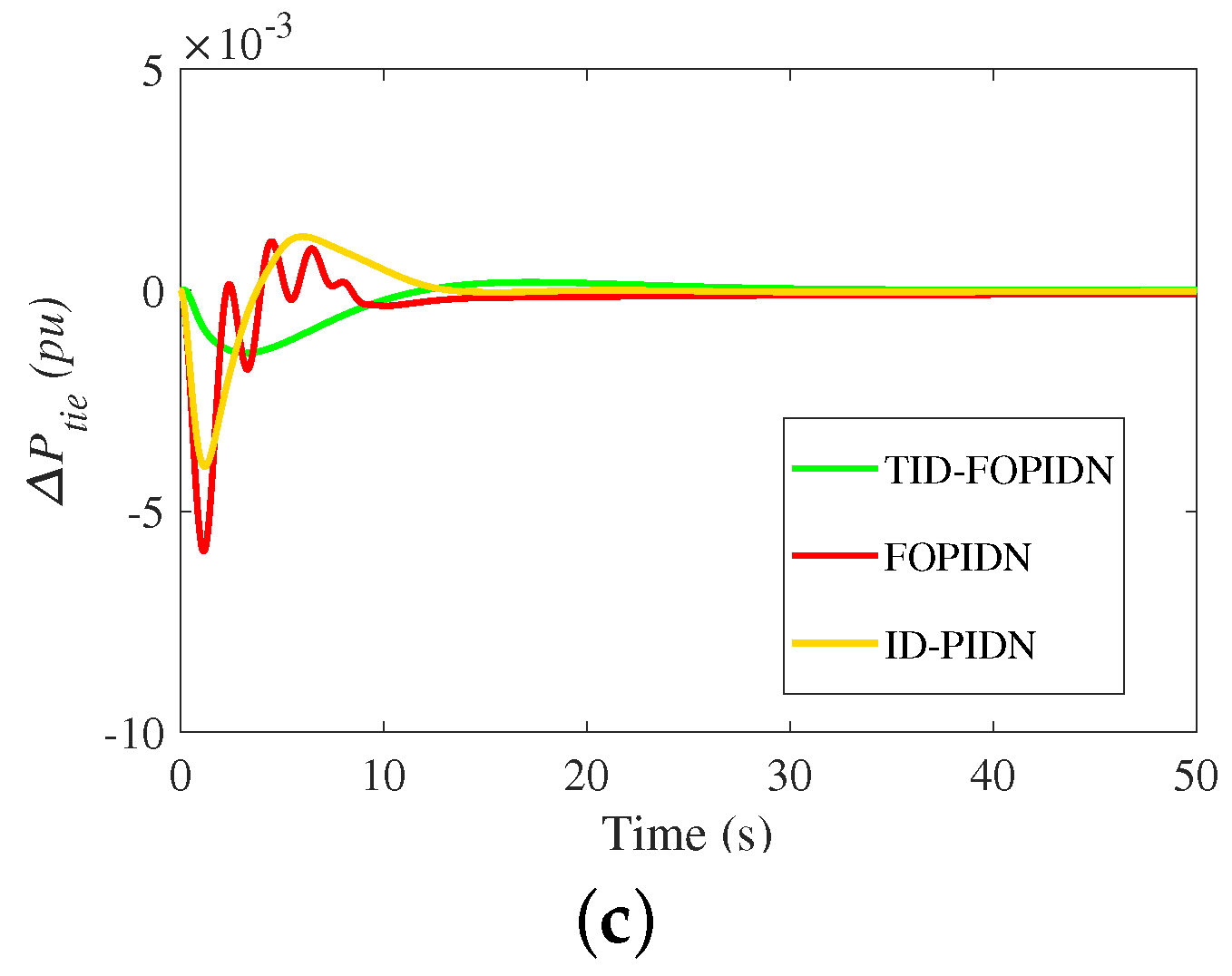
4.6. Additional Comparisons
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Said, S.M.; Aly, M.; Hartmann, B.; Alharbi, A.G.; Ahmed, E.M. SMES-Based Fuzzy Logic Approach for Enhancing the Reliability of Microgrids Equipped With PV Generators. IEEE Access 2019, 7, 92059–92069. [Google Scholar] [CrossRef]
- REN21. Renewables 2021: Global Status Report. 2019. Available online: https://www.ren21.net/reports/global-status-report/ (accessed on 1 June 2022).
- Siti, M.; Mbungu, N.; Tungadio, D.; Banza, B.; Ngoma, L. Application of load frequency control method to a multi-microgrid with energy storage system. J. Energy Storage 2022, 52, 104629. [Google Scholar] [CrossRef]
- Irudayaraj, A.X.R.; Wahab, N.I.A.; Premkumar, M.; Radzi, M.A.M.; Sulaiman, N.B.; Veerasamy, V.; Farade, R.A.; Islam, M.Z. Renewable sources-based automatic load frequency control of interconnected systems using chaotic atom search optimization. Appl. Soft Comput. 2022, 119, 108574. [Google Scholar] [CrossRef]
- Hu, Z.; Liu, S.; Wu, L. Credibility-based distributed frequency estimation for plug-in electric vehicles participating in load frequency control. Int. J. Electr. Power Energy Syst. 2021, 130, 106997. [Google Scholar] [CrossRef]
- Said, S.M.; Aly, M.; Hartmann, B.; Mohamed, E.A. Coordinated fuzzy logic-based virtual inertia controller and frequency relay scheme for reliable operation of low-inertia power system. IET Renew. Power Gener. 2021, 15, 1286–1300. [Google Scholar] [CrossRef]
- da Silva, G.S.; de Oliveira, E.J.; de Oliveira, L.W.; de Paula, A.N.; Ferreira, J.S.; Honório, L.M. Load frequency control and tie-line damping via virtual synchronous generator. Int. J. Electr. Power Energy Syst. 2021, 132, 107108. [Google Scholar] [CrossRef]
- Ranjan, M.; Shankar, R. A literature survey on load frequency control considering renewable energy integration in power system: Recent trends and future prospects. J. Energy Storage 2022, 45, 103717. [Google Scholar] [CrossRef]
- Pandey, S.K.; Mohanty, S.R.; Kishor, N. A literature survey on load–frequency control for conventional and distribution generation power systems. Renew. Sustain. Energy Rev. 2013, 25, 318–334. [Google Scholar] [CrossRef]
- Shayeghi, H.; Shayanfar, H.; Jalili, A. Load frequency control strategies: A state-of-the-art survey for the researcher. Energy Convers. Manag. 2009, 50, 344–353. [Google Scholar] [CrossRef]
- Latif, A.; Hussain, S.S.; Das, D.C.; Ustun, T.S. State-of-the-art of controllers and soft computing techniques for regulated load frequency management of single/multi-area traditional and renewable energy based power systems. Appl. Energy 2020, 266, 114858. [Google Scholar] [CrossRef]
- Elkasem, A.H.A.; Kamel, S.; Hassan, M.H.; Khamies, M.; Ahmed, E.M. An Eagle Strategy Arithmetic Optimization Algorithm for Frequency Stability Enhancement Considering High Renewable Power Penetration and Time-Varying Load. Mathematics 2022, 10, 854. [Google Scholar] [CrossRef]
- Arya, Y. ICA assisted FTIλDN controller for AGC performance enrichment of interconnected reheat thermal power systems. J. Ambient. Intell. Humaniz. Comput. 2021. [Google Scholar] [CrossRef]
- Bu, X.; Yu, W.; Cui, L.; Hou, Z.; Chen, Z. Event-triggered Data-driven Load Frequency Control for Multi-Area Power Systems. IEEE Trans. Ind. Inf. 2021, 8, 5982–5991. [Google Scholar] [CrossRef]
- Adibi, M.; der Woude, J.V. Secondary Frequency Control of Microgrids: An Online Reinforcement Learning Approach. IEEE Trans. Autom. Control. 2022, 67, 4824–4831. [Google Scholar] [CrossRef]
- Latif, A.; Hussain, S.S.; Das, D.C.; Ustun, T.S.; Iqbal, A. A review on fractional order (FO) controllers’ optimization for load frequency stabilization in power networks. Energy Rep. 2021, 7, 4009–4021. [Google Scholar] [CrossRef]
- Delassi, A.; Arif, S.; Mokrani, L. Load frequency control problem in interconnected power systems using robust fractional PI λ D controller. Ain Shams Eng. J. 2018, 9, 77–88. [Google Scholar] [CrossRef]
- Fathy, A.; Alharbi, A.G. Recent Approach Based Movable Damped Wave Algorithm for Designing Fractional-Order PID Load Frequency Control Installed in Multi-Interconnected Plants With Renewable Energy. IEEE Access 2021, 9, 71072–71089. [Google Scholar] [CrossRef]
- Sahin, E. Design of an Optimized Fractional High Order Differential Feedback Controller for Load Frequency Control of a Multi-Area Multi-Source Power System With Nonlinearity. IEEE Access 2020, 8, 12327–12342. [Google Scholar] [CrossRef]
- Ayas, M.S.; Sahin, E. FOPID controller with fractional filter for an automatic voltage regulator. Comput. Electr. Eng. 2021, 90, 106895. [Google Scholar] [CrossRef]
- Oshnoei, S.; Oshnoei, A.; Mosallanejad, A.; Haghjoo, F. Contribution of GCSC to regulate the frequency in multi-area power systems considering time delays: A new control outline based on fractional order controllers. Int. J. Electr. Power Energy Syst. 2020, 123, 106197. [Google Scholar] [CrossRef]
- Sahu, R.K.; Panda, S.; Biswal, A.; Sekhar, G.C. Design and analysis of tilt integral derivative controller with filter for load frequency control of multi-area interconnected power systems. ISA Trans. 2016, 61, 251–264. [Google Scholar] [CrossRef]
- Oshnoei, A.; Khezri, R.; Muyeen, S.M.; Oshnoei, S.; Blaabjerg, F. Automatic Generation Control Incorporating Electric Vehicles. Electr. Power Components Syst. 2019, 47, 720–732. [Google Scholar] [CrossRef]
- Priyadarshani, S.; Subhashini, K.R.; Satapathy, J.K. Pathfinder algorithm optimized fractional order tilt-integral-derivative (FOTID) controller for automatic generation control of multi-source power system. Microsyst. Technol. 2021, 27, 23–35. [Google Scholar] [CrossRef]
- Malik, S.; Suhag, S. A Novel SSA Tuned PI-TDF Control Scheme for Mitigation of Frequency Excursions in Hybrid Power System. Smart Sci. 2020, 8, 202–218. [Google Scholar] [CrossRef]
- Elmelegi, A.; Mohamed, E.A.; Aly, M.; Ahmed, E.M.; Mohamed, A.A.A.; Elbaksawi, O. Optimized Tilt Fractional Order Cooperative Controllers for Preserving Frequency Stability in Renewable Energy-Based Power Systems. IEEE Access 2021, 9, 8261–8277. [Google Scholar] [CrossRef]
- Mohamed, E.A.; Ahmed, E.M.; Elmelegi, A.; Aly, M.; Elbaksawi, O.; Mohamed, A.A.A. An Optimized Hybrid Fractional Order Controller for Frequency Regulation in Multi-Area Power Systems. IEEE Access 2020, 8, 213899–213915. [Google Scholar] [CrossRef]
- Ahmed, E.M.; Elmelegi, A.; Shawky, A.; Aly, M.; Alhosaini, W.; Mohamed, E.A. Frequency Regulation of Electric Vehicle-Penetrated Power System Using MPA-Tuned New Combined Fractional Order Controllers. IEEE Access 2021, 9, 107548–107565. [Google Scholar] [CrossRef]
- Ahmed, E.M.; Mohamed, E.A.; Elmelegi, A.; Aly, M.; Elbaksawi, O. Optimum Modified Fractional Order Controller for Future Electric Vehicles and Renewable Energy-Based Interconnected Power Systems. IEEE Access 2021, 9, 29993–30010. [Google Scholar] [CrossRef]
- Arya, Y. A new optimized fuzzy FOPI-FOPD controller for automatic generation control of electric power systems. J. Frankl. Inst. 2019, 356, 5611–5629. [Google Scholar] [CrossRef]
- Gheisarnejad, M.; Khooban, M.H. Design an optimal fuzzy fractional proportional integral derivative controller with derivative filter for load frequency control in power systems. Trans. Inst. Meas. Control. 2019, 41, 2563–2581. [Google Scholar] [CrossRef]
- Arya, Y.; Dahiya, P.; Çelik, E.; Sharma, G.; Gözde, H.; Nasiruddin, I. AGC performance amelioration in multi-area interconnected thermal and thermal-hydro-gas power systems using a novel controller. Eng. Sci. Technol. Int. J. 2021, 24, 384–396. [Google Scholar] [CrossRef]
- Arya, Y. Impact of ultra-capacitor on automatic generation control of electric energy systems using an optimal FFOID controller. Int. J. Energy Res. 2019, 43, 8765–8778. [Google Scholar] [CrossRef]
- Barakat, M.; Donkol, A.; Salama, G.M.; Hamed, H.F.A. Optimal Design of Fuzzy Plus Fraction-Order-Proportional-Integral-Derivative Controller for Automatic Generation Control of a Photovoltaic–Reheat Thermal Interconnected Power System. Process. Integr. Optim. Sustain. 2022. [Google Scholar] [CrossRef]
- Arya, Y. Improvement in automatic generation control of two-area electric power systems via a new fuzzy aided optimal PIDN-FOI controller. ISA Trans. 2018, 80, 475–490. [Google Scholar] [CrossRef]
- Morsali, J.; Zare, K.; Hagh, M.T. Performance comparison of TCSC with TCPS and SSSC controllers in AGC of realistic interconnected multi-source power system. Ain Shams Eng. J. 2016, 7, 143–158. [Google Scholar] [CrossRef]
- Zare, K.; Hagh, M.T.; Morsali, J. Effective oscillation damping of an interconnected multi-source power system with automatic generation control and TCSC. Int. J. Electr. Power Energy Syst. 2015, 65, 220–230. [Google Scholar] [CrossRef]
- Morsali, J.; Zare, K.; Hagh, M.T. Comparative performance evaluation of fractional order controllers in LFC of two-area diverse-unit power system with considering GDB and GRC effects. J. Electr. Syst. Inf. Technol. 2018, 5, 708–722. [Google Scholar] [CrossRef]
- Khokhar, B.; Dahiya, S.; Parmar, K.P.S. A Robust Cascade Controller for Load Frequency Control of a Standalone Microgrid Incorporating Electric Vehicles. Electr. Power Components Syst. 2020, 48, 711–726. [Google Scholar] [CrossRef]
- Ray, P.K.; Mohanty, S.R.; Kishor, N. Proportional–integral controller based small-signal analysis of hybrid distributed generation systems. Energy Convers. Manag. 2011, 52, 1943–1954. [Google Scholar] [CrossRef]
- Das, D.C.; Roy, A.; Sinha, N. GA based frequency controller for solar thermal–diesel–wind hybrid energy generation/energy storage system. Int. J. Electr. Power Energy Syst. 2012, 43, 262–279. [Google Scholar] [CrossRef]
- Singh, K.; Amir, M.; Ahmad, F.; Khan, M.A. An Integral Tilt Derivative Control Strategy for Frequency Control in Multimicrogrid System. IEEE Syst. J. 2021, 15, 1477–1488. [Google Scholar] [CrossRef]
- Daraz, A.; Malik, S.A.; Azar, A.T.; Aslam, S.; Alkhalifah, T.; Alturise, F. Optimized Fractional Order Integral-Tilt Derivative Controller for Frequency Regulation of Interconnected Diverse Renewable Energy Resources. IEEE Access 2022, 10, 43514–43527. [Google Scholar] [CrossRef]
- Ali, M.; Kotb, H.; Aboras, K.M.; Abbasy, N.H. Design of Cascaded PI-Fractional Order PID Controller for Improving the Frequency Response of Hybrid Microgrid System Using Gorilla Troops Optimizer. IEEE Access 2021, 9, 150715–150732. [Google Scholar] [CrossRef]
- Aly, M.; Ahmed, E.M.; Rezk, H.; Mohamed, E.A. Marine Predators Algorithm Optimized Reduced Sensor Fuzzy-Logic Based Maximum Power Point Tracking of Fuel Cell-Battery Standalone Applications. IEEE Access 2021, 9, 27987–28000. [Google Scholar] [CrossRef]
- Faramarzi, A.; Heidarinejad, M.; Mirjalili, S.; Gandomi, A.H. Marine Predators Algorithm: A nature-inspired metaheuristic. Expert Syst. Appl. 2020, 152, 113377. [Google Scholar] [CrossRef]
- Çelik, E. Design of new fractional order PI–fractional order PD cascade controller through dragonfly search algorithm for advanced load frequency control of power systems. Soft Comput. 2020, 25, 1193–1217. [Google Scholar] [CrossRef]








| Acronyms | Study Factors | |
|---|---|---|
| Area 1 | Area 2 | |
| LFC model | ||
| ,, (Hz/MW) | 2.4 | 2.4 |
| , (MW/Hz) | 0.4312 | 0.4312 |
| Power system | ||
| , (MW) | 2000 | 2000 |
| (MW) | 1740 | 1740 |
| , | 68.9655 | 68.9655 |
| , | 11.49 | 11.49 |
| 0.0433 | ||
| −1 | ||
| Reheating thermal plant | ||
| (MW) | 946 | 946 |
| 0.08 | 0.08 | |
| 0.3 | 0.3 | |
| 0.3 | 0.3 | |
| 10 | 10 | |
| 0.54367 | 0.54367 | |
| Hydro power plant | ||
| (MW) | 567 | 567 |
| 0.2 | 0.2 | |
| 28.749 | 28.749 | |
| 5 | 5 | |
| 1 | 1 | |
| 0.32586 | 0.32586 | |
| Gas unit | ||
| (MW) | 227 | 227 |
| 0.049 | 0.049 | |
| 1 | 1 | |
| 0.6 | 0.6 | |
| 1.1 | 1.1 | |
| 0.01 | 0.01 | |
| 0.239 | 0.239 | |
| 0.2 | 0.2 | |
| 0.130459 | 0.130459 | |
| Acronyms | Study Factors | |
|---|---|---|
| Area 1 | Area 2 | |
| PV power plant | ||
| (s) | - | 1.3 |
| (s) | - | 1 |
| Wind power plant | ||
| (s) | 1.5 | - |
| (s) | 1 | - |
| EV model | ||
| Penetration Level | 5–10% | 5–10% |
| (V) | 364.8 | 364.8 |
| (Ah) | 66.2 | 66.2 |
| (ohms) | 0.074 | 0.074 |
| (ohms) | 0.047 | 0.047 |
| (farad) | 703.6 | 703.6 |
| 0.02612 | 0.02612 | |
| Maximum % | 95 | 95 |
| (kWh) | 24.15 | 24.15 |
| Controller | Area | Control | Coefficients | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| n | ||||||||||||
| Proposed | Area 1 | LFC | 1.9674 | 0.6976 | 1.57 | 0.33289 | 0.92242 | 0.80982 | 3.8677 | 245.0444 | 0.45757 | 0.5531 |
| Area 2 | LFC | 1.9358 | 0.3844 | 0.7888 | 1.3744 | 0.71797 | 1.3931 | 4.6014 | 300 | 0.46516 | 0.55918 | |
| Hybrid [24] | Area 1 | LFC | 2.0000 | 1.9943 | 1.3884 | - | - | - | 3 | - | 0.955 | 1.3646 |
| Area 2 | LFC | 0.0012 | 0.3572 | 1.9997 | - | - | - | 2.9537 | - | 0.0008 | 1.2693 | |
| TID [38] | Area 1 | LFC | 0.1884 | 0.1238 | 0.4095 | - | - | - | 3 | - | - | - |
| Area 2 | LFC | 0.2239 | 0.1131 | 0.4990 | - | - | - | 3 | - | - | - | |
| FOPID [38] | Area 1 | LFC | - | - | - | 0.8615 | 1.8463 | 1.9990 | - | - | 0.6494 | 0.9990 |
| Area 2 | LFC | - | - | - | 0.0510 | 0.3561 | 1.6478 | - | - | 0.4003 | 0.9826 | |
| FOPI-FOPD [47] | Area 1 | LFC | - | - | - | 2/1.8732 | 2 | 1.5629 | - | - | 0.8606 | 0.0997 |
| Area 2 | LFC | - | - | - | 2/1.9725 | 2 | 1.998 | - | - | 0.5130 | 0.0310 | |
| Controller | Area | Control | Coefficients | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| n | ||||||||||||
| Proposed | Area 1 | LFC | 1.037 | 1.7698 | 1.5695 | 1.3481 | 1.0339 | 0.57491 | 4.0673 | 296.7003 | 0.60512 | 0.93462 |
| EV | 1.745 | 1.5096 | 0.40002 | - | - | - | 2.3908 | - | - | - | ||
| Area 2 | LFC | 1.5215 | 0.246 | 0.69809 | 0.75802 | 0.98775 | 0.10823 | 4.3335 | 295.7854 | 0.62899 | 0.73299 | |
| EV | 1.193 | 1.2462 | 0.60999 | - | - | - | 3.116 | - | - | - | ||
| Hybrid | Area 1 | LFC | 1.2906 | 1.063 | 1.6957 | - | - | - | 3.6475 | - | 0.77626 | 0.90608 |
| EV | 1.9722 | 1.9295 | 1.2382 | - | - | - | 4.4185 | - | - | - | ||
| Area 2 | LFC | 1.0859 | 0.68601 | 1.2588 | - | - | - | 4.8724 | - | 0.60716 | 0.10543 | |
| EV | 1.7877 | 0.5144 | 0.12329 | - | - | - | 3.5308 | - | - | - | ||
| TID | Area 1 | LFC | 1.6069 | 0.97697 | 1.6355 | - | - | - | 2.9463 | - | - | - |
| EV | 1.9995 | 1.9586 | 0.44039 | - | - | - | 4.0746 | - | - | - | ||
| Area 2 | LFC | 0.55574 | 1.5664 | 1.7384 | - | - | - | 3.2124 | - | - | - | |
| EV | 1.481 | 0.67578 | 1.5763 | - | - | - | 2.6727 | - | - | - | ||
| FOPID | Area 1 | LFC | - | - | - | 1.057 | 1.7666 | 1.5353 | - | - | 0.74518 | 0.94931 |
| EV | 1.9581 | 1.8882 | 1.5734 | - | - | - | 3.486 | - | - | - | ||
| Area 2 | LFC | - | - | - | 0.44181 | 1.0682 | 0.32657 | - | - | 0.42262 | 0.45609 | |
| EV | 1.8372 | 0.97982 | 1.529 | - | - | - | 3.5885 | - | - | - | ||
| FOPI-FOPD | Area 1 | LFC | - | - | - | 2/1.9991 | 2 | 1.6731 | - | - | 0.7421 | 0.2451 |
| EV | 0.17532 | 0.1421 | 0.5197 | - | - | - | 2.753 | - | - | - | ||
| Area 2 | LFC | - | - | - | 2/1.342 | 2 | 1.989 | - | - | 0.7845 | 0.04710 | |
| EV | 0.3517 | 0.1982 | 0.6781 | - | - | - | 3.691 | - | - | - | ||
| Scenario | Controller | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| PO | PU | ST (s) | PO | PU | ST (s) | PO | PU | ST (s) | ||
| No.1 1% SLP without EV | TID | 0.0096 | 0.0404 | 29 | 0.0116 | 0.0498 | 25 | 0.0002 | 0.0079 | 33 |
| FOPID | 0.0111 | 0.0355 | 21 | 0.0103 | 0.0334 | 20 | 0.0011 | 0.0059 | 15 | |
| Hybrid | 0.0085 | 0.0277 | 15 | 0.0114 | 0.0253 | 14 | 0.0024 | 0.0048 | 14 | |
| FOPI-FOPD | 0.0066 | 0.0162 | 13 | 0.0038 | 0.0109 | 11 | 0.0008 | 0.0026 | 18 | |
| Proposed | 0.0004 | 0.0136 | 8 | 0.0002 | 0.0057 | 9 | 0.0001 | 0.0014 | 12 | |
| No.1 1% SLP with EV | TID | 0.0032 | 0.0249 | 16 | 0.0009 | 0.0211 | 22 | 0.0002 | 0.0069 | 30 |
| FOPID | 0.0058 | 0.0217 | 15 | 0.0039 | 0.0157 | 10 | 0.0001 | 0.0052 | 35 | |
| Hybrid | 0.0029 | 0.0153 | 13 | 0.0037 | 0.0103 | 9 | 0.0002 | 0.0431 | 17 | |
| FOPI-FOPD | 0.0052 | 0.0125 | 14 | 0.0036 | 0.0092 | 13 | 0.0005 | 0.0036 | 19 | |
| Proposed | ― | 0.0084 | 5 | 0.0003 | 0.0037 | 6 | 0.0001 | 0.0013 | 11 | |
| No.2 1% SLP Outage | TID | 0.0025 | 0.0261 | 27 | 0.0027 | 0.0261 | 26 | ― | 0.0077 | >50 |
| FOPID | 0.0019 | 0.0205 | 19 | 0.0015 | 0.0181 | 18 | 0.0024 | 0.0056 | >50 | |
| Hybrid | 0.001 | 0.0156 | 16 | 0.0009 | 0.0123 | 14 | 0.0014 | 0.0041 | >50 | |
| FOPI-FOPD | 0.0002 | 0.0131 | 12 | ― | 0.0093 | 12 | 0.001 | 0.0031 | >50 | |
| Proposed | 0.0001 | 0.0088 | 9 | 0.0002 | 0.0042 | 10 | ― | 0.0018 | 12 | |
| No.4 at 30 s | TID | 0.0591 | 0.2651 | 55 | 0.0464 | 0.2554 | 52 | 0.0103 | 0.0754 | 76 |
| FOPID | 0.0385 | 0.2031 | 51 | 0.0286 | 0.1666 | 49 | 0.0013 | 0.0516 | 48 | |
| Hybrid | 0.0155 | 0.1421 | 47 | 0.0238 | 0.1078 | 43 | 0.0011 | 0.0351 | 41 | |
| FOPI-FOPD | 0.0281 | 0.1271 | 23 | 0.0214 | 0.0945 | 21 | 0.0034 | 0.0304 | 28 | |
| Proposed | 0.0002 | 0.0851 | 13 | 0.0034 | 0.0444 | 13 | 0.0016 | 0.0171 | 17 | |
| No.5 at 10 s | TID | 0.2542 | 0.0501 | 57 | 0.2345 | 0.0473 | 56 | 0.0089 | 0.0632 | 67 |
| FOPID | 0.2014 | ― | >120 | 0.1977 | ― | >120 | ― | 0.0502 | >120 | |
| Hybrid | 0.1264 | ― | >120 | 0.1728 | ― | >120 | ― | 0.0541 | >120 | |
| FOPI-FOPD | 0.0955 | 0.0211 | 28 | 0.1205 | 0.0295 | 24 | 0.0036 | 0.0285 | 25 | |
| Proposed | 0.0331 | ― | 23 | 0.1021 | 0.0212 | 21 | 0.0021 | 0.0251 | 25 | |
| Scenario | Controller Technique | Performance Indices | |||
|---|---|---|---|---|---|
| ISE | ITSE | IAE | ITAE | ||
| No. 1 (1% SLP without EV) | TID | 0.0082 | 0.0209 | 0.3672 | 1.9818 |
| FOPID | 0.0027 | 0.0056 | 0.1918 | 1.17 | |
| Hybrid | 0.0022 | 0.0046 | 0.1622 | 0.536 | |
| FOPI-FOPD | 0.000588 | 0.0012 | 0.0899 | 0.3404 | |
| Proposed | 0.000212 | 0.000441 | 0.0501 | 0.1856 | |
| No. 1 (1% SLP with EV) | TID | 0.0019 | 0.0047 | 0.1854 | 1.0967 |
| FOPID | 0.000954 | 0.0018 | 0.1123 | 0.6465 | |
| Hybrid | 0.000485 | 0.000958 | 0.0886 | 0.5212 | |
| FOPI-FOPD | 0.000426 | 0.001 | 0.0797 | 0.3293 | |
| Proposed | 0.0000138 | 0.00001804 | 0.0143 | 0.0995 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassan, A.; Aly, M.; Elmelegi, A.; Nasrat, L.; Watanabe, M.; Mohamed, E.A. Optimal Frequency Control of Multi-Area Hybrid Power System Using New Cascaded TID-PIλDμN Controller Incorporating Electric Vehicles. Fractal Fract. 2022, 6, 548. https://doi.org/10.3390/fractalfract6100548
Hassan A, Aly M, Elmelegi A, Nasrat L, Watanabe M, Mohamed EA. Optimal Frequency Control of Multi-Area Hybrid Power System Using New Cascaded TID-PIλDμN Controller Incorporating Electric Vehicles. Fractal and Fractional. 2022; 6(10):548. https://doi.org/10.3390/fractalfract6100548
Chicago/Turabian StyleHassan, Amira, Mokhtar Aly, Ahmed Elmelegi, Loai Nasrat, Masayuki Watanabe, and Emad A. Mohamed. 2022. "Optimal Frequency Control of Multi-Area Hybrid Power System Using New Cascaded TID-PIλDμN Controller Incorporating Electric Vehicles" Fractal and Fractional 6, no. 10: 548. https://doi.org/10.3390/fractalfract6100548
APA StyleHassan, A., Aly, M., Elmelegi, A., Nasrat, L., Watanabe, M., & Mohamed, E. A. (2022). Optimal Frequency Control of Multi-Area Hybrid Power System Using New Cascaded TID-PIλDμN Controller Incorporating Electric Vehicles. Fractal and Fractional, 6(10), 548. https://doi.org/10.3390/fractalfract6100548








