Lyapunov Approach for Almost Periodicity in Impulsive Gene Regulatory Networks of Fractional Order with Time-Varying Delays
Abstract
1. Introduction
- (1)
- An impulsive control strategy is considered for a class of fractional-order GRNs with time-varying delays;
- (2)
- The almost periodicity notion is introduced to the model under consideration which initiates the development of the almost periodicity theory for impulsive fractional GRNs;
- (3)
- New existence and uniqueness results for the almost periodic states are established;
- (4)
- New criteria for global Mittag–Leffler stability of an almost periodic state of the impulsive model under consideration are also proved;
- (5)
- We apply an extended Lyapunov function approach which allows representing the obtained results in terms of the model’s parameters, and leads to a better exploration of the impulsive effect.
2. The Fractional-Order Impulsive Delayed GRN Model. Preliminaries
- P1.
- P2.
- .
- P3.
- Ref. [51]
- , , ;
- The parameters are the same as in (1);
- The functions of regulation , are the same as in (1) and satisfyfor some constants and all , ;
- The translation rates and basal rates are extended to functions and , respectively, , , where is the set of all the j which are repressors of the gene i;
- The functions are represented as:
- The time-varying delays and are different for different mRNA and protein molecules, respectively, and satisfy and ( = const), , ( = const), ;
- are the impulsive moments (impulsive control instants) and , where is the set of all sequences of the typewith a distance denoted as ;
- and denote the i-th mRNA concentration and i-th protein concentration at time , respectively, and and denote the level of the i-th mRNA concentration and i-th protein concentration, respectively, at , i.e., after an impulsive short-term effect on them at ;
- The impulsive functions and denote the amounts of the abrupt variation in and , respectively, at the impulsive instants , i.e., and , , .
- A1.
- A2.
- The sequences of functions and , , are almost periodic in the sense of Bohr;
- A3.
- The initial functions and are almost periodic;
- A4.
- The set of sequences , , is UAP.
- (i)
- ;
- (ii)
- The inequalityis valid whenever
3. Fractional Order Almost Periodicity Theorems
- (i)
- There exists a real numberwhere ;
- (ii)
- The functions , , , where , and are positive constants and the constants , , are such that
- (iii)
- A solution of the model (2) exists such thatfor .
- (a)
- ;
- (b)
- , where is the hull of the almost periodic solution .
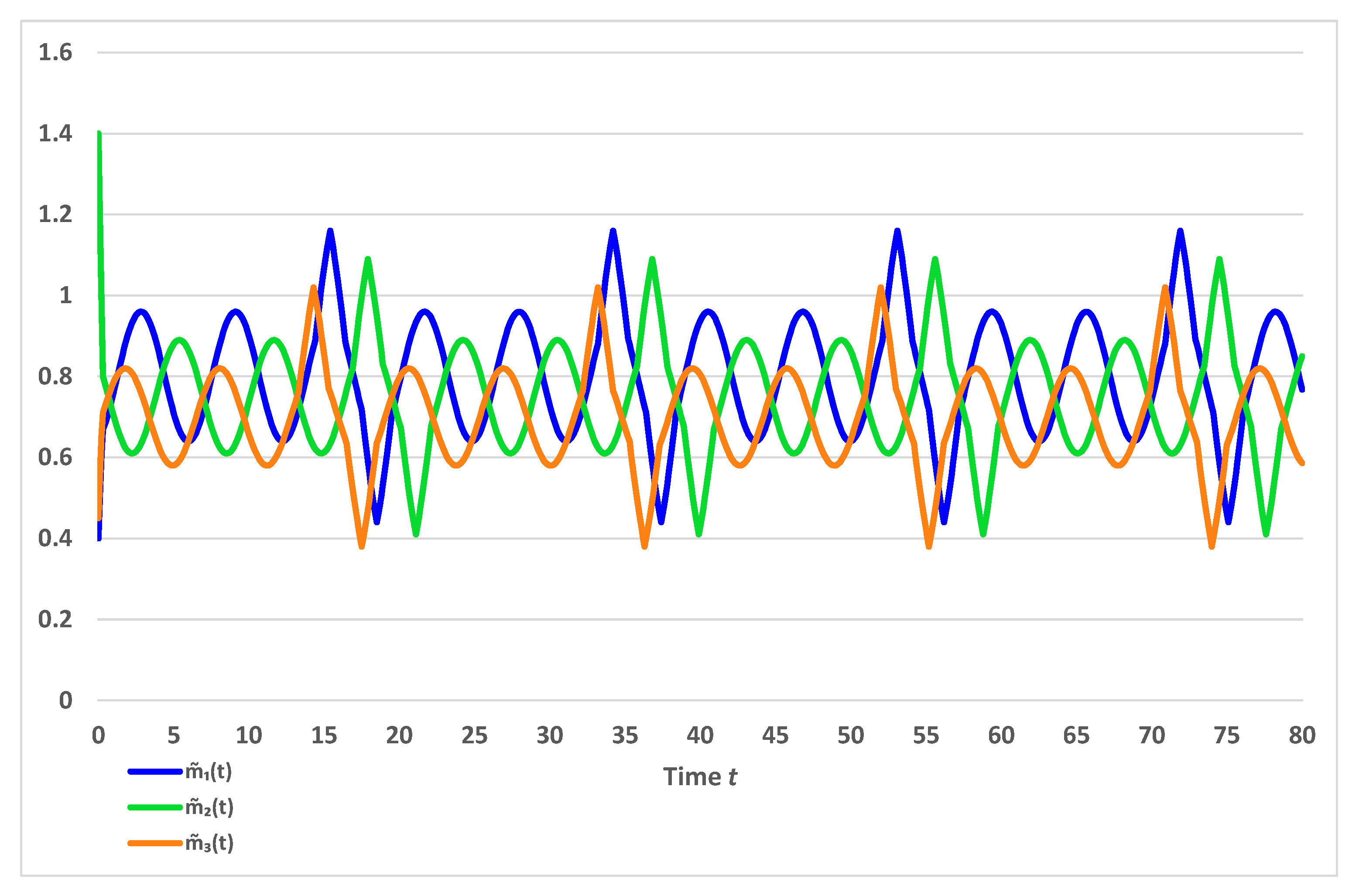
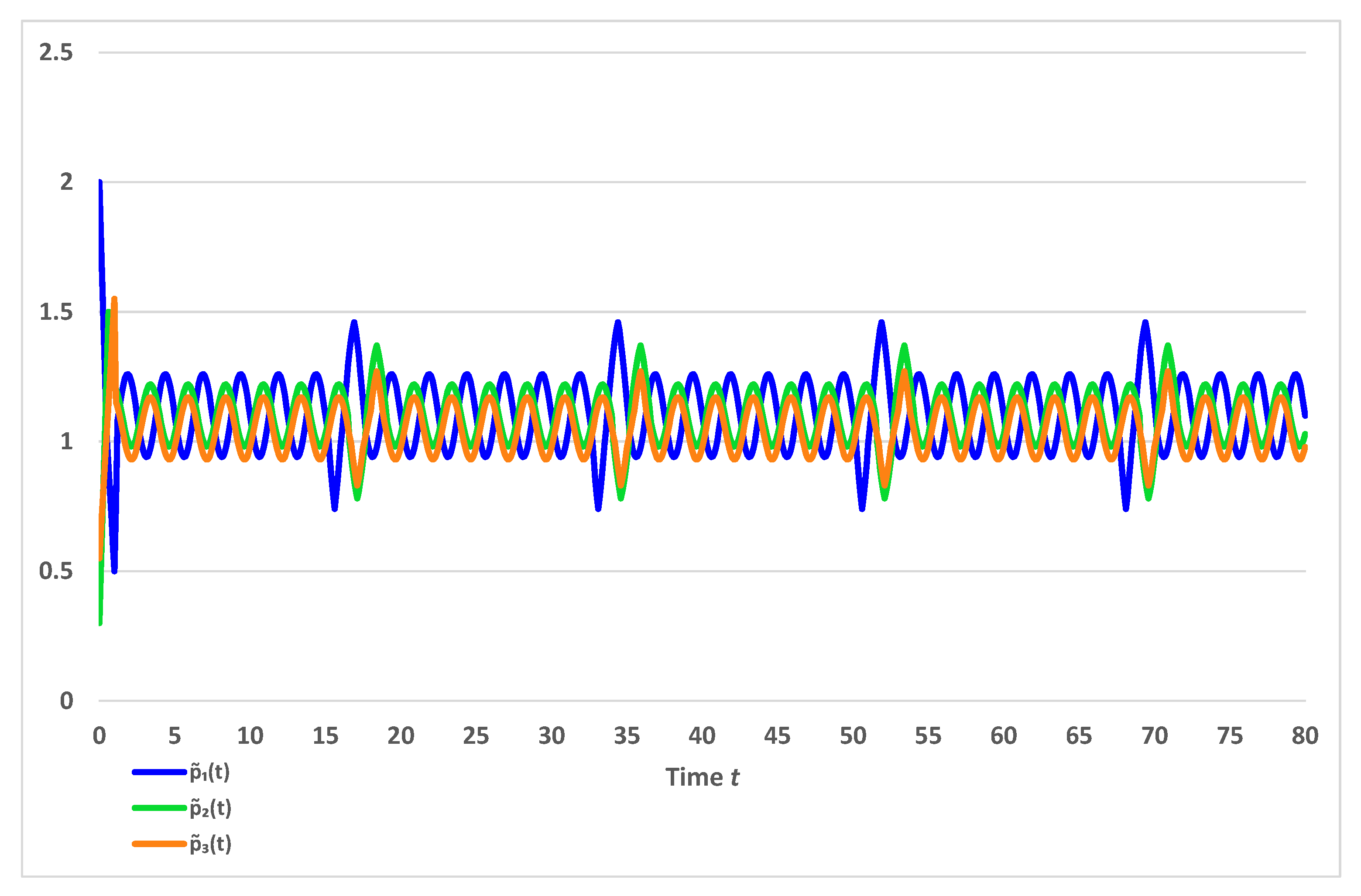
4. A Numerical Example
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- De Jong, H. Modeling and simulation of genetic regulatory systems: A literature review. J. Comput. Biol. 2002, 9, 67–103. [Google Scholar] [CrossRef]
- Karlebach, G.; Shamir, R. Modelling and analysis of gene regulatory networks. Nat. Rev. Mol. Cell Biol. 2008, 9, 770–780. [Google Scholar] [CrossRef]
- Porreca, R.; Drulhe, S.; de Jong, H.; Ferrari-Trecate, G. Structural identification of piecewise-linear models of genetic regulatory networks. J. Comput. Biol. 2008, 15, 1365–1380. [Google Scholar] [CrossRef]
- Wu, L.; Liu, K.; Lü, J.; Gu, H. Finite-time adaptive stability of gene regulatory networks stability. Neurocomputing 2019, 338, 222–232. [Google Scholar] [CrossRef]
- Ren, F.; Cao, J. Asymptotic and robust stability of genetic regulatory networks with time-varying delays. Neurocomputing 2008, 71, 834–842. [Google Scholar] [CrossRef]
- Wang, W.; Zhong, S.; Liu, F. New delay-dependent stability criteria for uncertain genetic regulatory networks with time-varying delays. Neurocomputing 2012, 93, 19–26. [Google Scholar] [CrossRef]
- Zhang, L.N.; Zhang, X.Y.; Xue, Y.; Zhang, X. New method to global exponential stability analysis for switched genetic regulatory networks with mixed delays. IEEE Trans. Nanobiosci. 2020, 19, 308–314. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Wang, Y.; Wu, L. Analysis and Design of Delayed Genetic Regulatory Networks, 1st ed.; Springer: Cham, Switzerland, 2019; ISBN 978-3-030-17097-4. [Google Scholar]
- Qiu, J.; Sun, K.; Yang, C.; Chen, X.; Chen, X.; Zhang, A. Finite-time stability of genetic regulatory networks with impulsive effects. Neurocomputing 2017, 219, 9–14. [Google Scholar] [CrossRef]
- Sakthivel, R.; Raja, R.; Anthoni, S.M. Asymptotic stability of delayed stochastic genetic regulatory networks with impulses. Phys. Scr. 2010, 82, 055009. [Google Scholar] [CrossRef]
- Senthilraj, S.; Raja, R.; Zhu, Q.; Samidurai, R.; Zhou, H. Delay-dependent asymptotic stability criteria for genetic regulatory networks with impulsive perturbations. Neurocomputing 2016, 214, 981–990. [Google Scholar] [CrossRef]
- Benchohra, M.; Henderson, J.; Ntouyas, J. Impulsive Differential Equations and Inclusions, 1st ed.; Hindawi Publishing Corporation: New York, NY, USA, 2006; ISBN 978-9775945501. [Google Scholar]
- Li, X.; Song, S. Impulsive Systems with Delays: Stability and Control, 1st ed.; Science Press & Springer: Singapore, 2022; ISBN 978-981-16-4686-7. [Google Scholar]
- Stamova, I.M.; Stamov, G.T. Applied Impulsive Mathematical Models, 1st ed.; Springer: Cham, Switzerland, 2016; ISBN 978-3-319-28061-5. [Google Scholar]
- Li, X.; Li, P. Stability of time-delay systems with impulsive control involving stabilizing delays. Autom. J. IFAC 2021, 124, 109336. [Google Scholar] [CrossRef]
- Li, X.; Wu, J. Sufficient stability conditions of nonlinear differential systems under impulsive control with state-dependent delay. IEEE Trans. Autom. Control 2018, 63, 306–311. [Google Scholar] [CrossRef]
- Stamova, I.M. Impulsive control for stability of n-species Lotka-Volterra cooperation models with finite delays. Appl. Math. Lett. 2010, 23, 1003–1007. [Google Scholar] [CrossRef][Green Version]
- Stamova, I.; Stamov, G. Mittag–Leffler synchronization of fractional neural networks with time-varying delays and reaction-diffusion terms using impulsive and linear controllers. Neural Netw. 2017, 96, 22–32. [Google Scholar] [CrossRef]
- Yang, X.; Peng, D.; Lv, X.; Li, X. Recent progress in impulsive control systems. Math. Comput. Simul. 2019, 155, 244–268. [Google Scholar] [CrossRef]
- Yosef, N.; Regev, A. Impulse control: Temporal dynamics in gene transcription. Cell 2011, 144, 886–896. [Google Scholar] [CrossRef] [PubMed]
- Hilfer, R. Applications of Fractional Calculus in Physics, 1st ed.; World Scientific: Singapore, 2000; ISBN 9810234570. [Google Scholar]
- Podlubny, I. Fractional Differential Equations, 1st ed.; Academic Press: San Diego, CA, USA, 1999; ISBN 558840-2. [Google Scholar]
- Stamova, I.M.; Stamov, G.T. Functional and Impulsive Differential Equations of Fractional Order: Qualitative Analysis and Applications, 1st ed.; CRC Press, Taylor and Francis Group: Boca Raton, FL, USA, 2017; ISBN 9781498764834. [Google Scholar]
- Li, H.-L.; Hu, C.; Cao, J.; Jiang, H.; Alsaedi, A. Quasi-projective and complete synchronization of fractional-order complex-valued neural networks with time delays. Neural Netw. 2019, 118, 102–109. [Google Scholar] [CrossRef]
- Li, H.-L.; Jiang, H.; Cao, J.; Jiang, H. Global synchronization of fractional-order quaternion-valued neural networks with leakage and discrete delays. Neurocomputing 2020, 385, 211–2019. [Google Scholar] [CrossRef]
- Stamova, I. Global Mittag-Leffler stability and synchronization of impulsive fractional-order neural networks with time-varying delays. Nonlinear Dynam. 2014, 77, 1251–1260. [Google Scholar] [CrossRef]
- Udhayakumar, K.; Rakkiyappan, R.; Cao, J.; Tan, X. Mittag-Leffler stability analysis of multiple equilibrium points in impulsive fractional-order quaternion-valued neural networks. Front. Inform. Technol. Electron. Eng. 2020, 21, 234–246. [Google Scholar] [CrossRef]
- Anbalagan, P.; Hincal, E.; Ramachandran, R.; Baleanu, D.; Cao, J.; Niezabitowski, M. A Razumikhin approach to stability and synchronization criteria for fractional order time delayed gene regulatory networks. AIMS Math. 2021, 6, 4526–4555. [Google Scholar] [CrossRef]
- Ren, F.; Cao, F.; Cao, J. Mittag–Leffler stability and generalized Mittag–Leffler stability of fractional-order gene regulatory networks. Neurocomputing 2015, 160, 185–190. [Google Scholar] [CrossRef]
- Qiao, Y.; Yan, H.; Duan, L.; Miao, J. Finite-time synchronization of fractional-order gene regulatory networks with time delay. Neural Netw. 2020, 126, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Wang, Z.; Zhou, T. Global stability analysis of fractional-order gene regulatory networks with time delay. Int. J. Biomath. 2019, 12, 1950067. [Google Scholar] [CrossRef]
- Wu, Z.; Wang, Z.; Zhou, T. Global uniform asymptotical stability for fractional-order gene regulatory networks with time-varying delays and structured uncertainties. Adv. Differ. Equ. 2021, 2021, 93. [Google Scholar] [CrossRef]
- Liu, F.; Zhang, Z.; Wang, X.; Sun, F. Stability and synchronization control of fractional-order gene regulatory network system with delay. J. Adv. Comput. Intell. Intell. Inform. 2017, 21, 148–152. [Google Scholar] [CrossRef]
- Stamov, T.; Stamova, I. Design of impulsive controllers and impulsive control strategy for the Mittag–Leffler stability behavior of fractional gene regulatory networks. Neurocomputing 2021, 424, 54–62. [Google Scholar] [CrossRef]
- Du, W.S.; Kostić, M.; Pinto, M. Almost periodic functions and their applications: A survey of results and perspectives. J. Math. 2021, 2021, 5536018. [Google Scholar] [CrossRef]
- Fink, A.M. Almost Periodic Differential Equations, 1st ed.; Springer: Berlin, Germany, 1974; ISBN 978-3-540-38307-9. [Google Scholar]
- Levitan, M.; Zhikov, V.V. Almost Periodic Functions and Differential Equations, 1st ed.; Cambridge University Press: London, UK, 1982; ISBN 9780521244077. [Google Scholar]
- Samoilenko, A.M.; Perestyuk, N.A. Impulsive Differential Equations, 1st ed.; World Scientific: River Edge, NJ, USA, 1995; ISBN 978-981-02-2416-5. [Google Scholar]
- Stamov, G.T. Almost Periodic Solutions of Impulsive Differential Equations, 1st ed.; Springer: Heidelberg, Germany, 2012; ISBN 978-3-642-27545-6. [Google Scholar]
- Luo, D.; Wang, Q. Dynamic analysis on an almost periodic predator-prey system with impulsive effects and time delay. Discret. Contin. Dyn. Syst. Ser. B 2021, 26, 3427–3453. [Google Scholar] [CrossRef]
- Rakkiyappan, R.; Velmurugan, G.; Soundharajan, P.; Joo, Y.H. Almost periodic dynamics of memristive inertial neural networks with mixed delays. Inf. Sci. 2020, 536, 332–350. [Google Scholar]
- Wang, L.; Zhang, H.; Liu, S. On the existence of almost periodic solutions of impulsive non-autonomous Lotka-Volterra predator-prey system with harvesting terms. AIMS Math. 2021, 7, 925–938. [Google Scholar] [CrossRef]
- Stamov, G.; Stamova, I. Impulsive fractional-order neural networks with time-varying delays: Almost periodic solutions. Neural Comput. Appl. 2017, 28, 3307–3316. [Google Scholar] [CrossRef]
- Stamov, G.; Stamova, I.; Martynyuk, A.; Stamov, T. Almost periodic dynamics in a new class of impulsive reaction-diffusion neural networks with fractional-like derivatives. Chaos Solitons Fractals 2021, 143, 110647. [Google Scholar] [CrossRef]
- Wan, P.; Sun, D.; Zhao, M.; Zhao, H. Monostability and multistability for almost-periodic solutions of fractional-order neural networks with unsaturating piecewise linear activation functions. IEEE Trans. Neural. Netw. Learn. Syst. 2020, 12, 5138–5152. [Google Scholar] [CrossRef] [PubMed]
- Kaslik, E.; Sivasundaram, S. Non-existence of periodic solutions in fractional-order dynamical systems and a remarkable difference between integer and fractional-order derivatives of periodic functions. Nonlinear Anal. Real World Appl. 2012, 13, 1489–1497. [Google Scholar] [CrossRef]
- Duan, L.; Di, F.; Wang, Z. Existence and global exponential stability of almost periodic solutions of genetic regulatory networks with time-varying delays. J. Exp. Theor. Artif. Intell. 2020, 32, 453–463. [Google Scholar] [CrossRef]
- Stamov, G.; Stamov, T.; Stamova, I. On the almost periodicity in discontinuous impulsive gene regulatory networks. Math. Methods Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Aguila-Camacho, N.; Duarte-Mermoud, M.A.; Gallegos, J.A. Lyapunov functions for fractional order systems. Comm. Nonlinear Sci. Numer. Simul. 2014, 19, 2951–2957. [Google Scholar] [CrossRef]
- Li, X.; Peng, D.; Cao, J. Lyapunov stability for impulsive systems via event-triggered impulsive control. IEEE Trans. Autom. Control 2020, 65, 4908–4913. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag-Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef]
- Yoshizawa, T. Stability Theory and the Existence of Periodic Solutions and Almost Periodic Solutions, 1st ed.; Springer: New York, NY, USA, 1975; ISBN 978-1-4612-6376-0. [Google Scholar]
- Choi, S.K.; Kang, B.; Koo, N. Stability for Caputo fractional differential systems. Abstr. Appl. Anal. 2014, 2014, 631419. [Google Scholar] [CrossRef]
- Stamova, I.; Sotirov, S.; Stamov, G.; Sotirova, E. Impulsive fractional Cohen–Grossberg neural networks: Almost periodicity analysis. Fractal Fract. 2021, 5, 78. [Google Scholar] [CrossRef]
- Wu, H.; Zhang, X.; Xue, S.; Wang, L.; Wang, Y. LMI conditions to global Mittag-Leffler stability of fractional-order neural networks with impulses. Neurocomputing 2016, 193, 148–154. [Google Scholar] [CrossRef]
- Li, X.; Rakkiyappan, R.; Pradeep, C. Robust μ-stability analysis of Markovian switching uncertain stochastic genetic regulatory networks with unbounded time-varying delays. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 3894–3905. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stamova, I.; Stamov, G. Lyapunov Approach for Almost Periodicity in Impulsive Gene Regulatory Networks of Fractional Order with Time-Varying Delays. Fractal Fract. 2021, 5, 268. https://doi.org/10.3390/fractalfract5040268
Stamova I, Stamov G. Lyapunov Approach for Almost Periodicity in Impulsive Gene Regulatory Networks of Fractional Order with Time-Varying Delays. Fractal and Fractional. 2021; 5(4):268. https://doi.org/10.3390/fractalfract5040268
Chicago/Turabian StyleStamova, Ivanka, and Gani Stamov. 2021. "Lyapunov Approach for Almost Periodicity in Impulsive Gene Regulatory Networks of Fractional Order with Time-Varying Delays" Fractal and Fractional 5, no. 4: 268. https://doi.org/10.3390/fractalfract5040268
APA StyleStamova, I., & Stamov, G. (2021). Lyapunov Approach for Almost Periodicity in Impulsive Gene Regulatory Networks of Fractional Order with Time-Varying Delays. Fractal and Fractional, 5(4), 268. https://doi.org/10.3390/fractalfract5040268






