Rational Approximations of Arbitrary Order: A Survey
Abstract
:1. Introduction
2. Basic Definitions
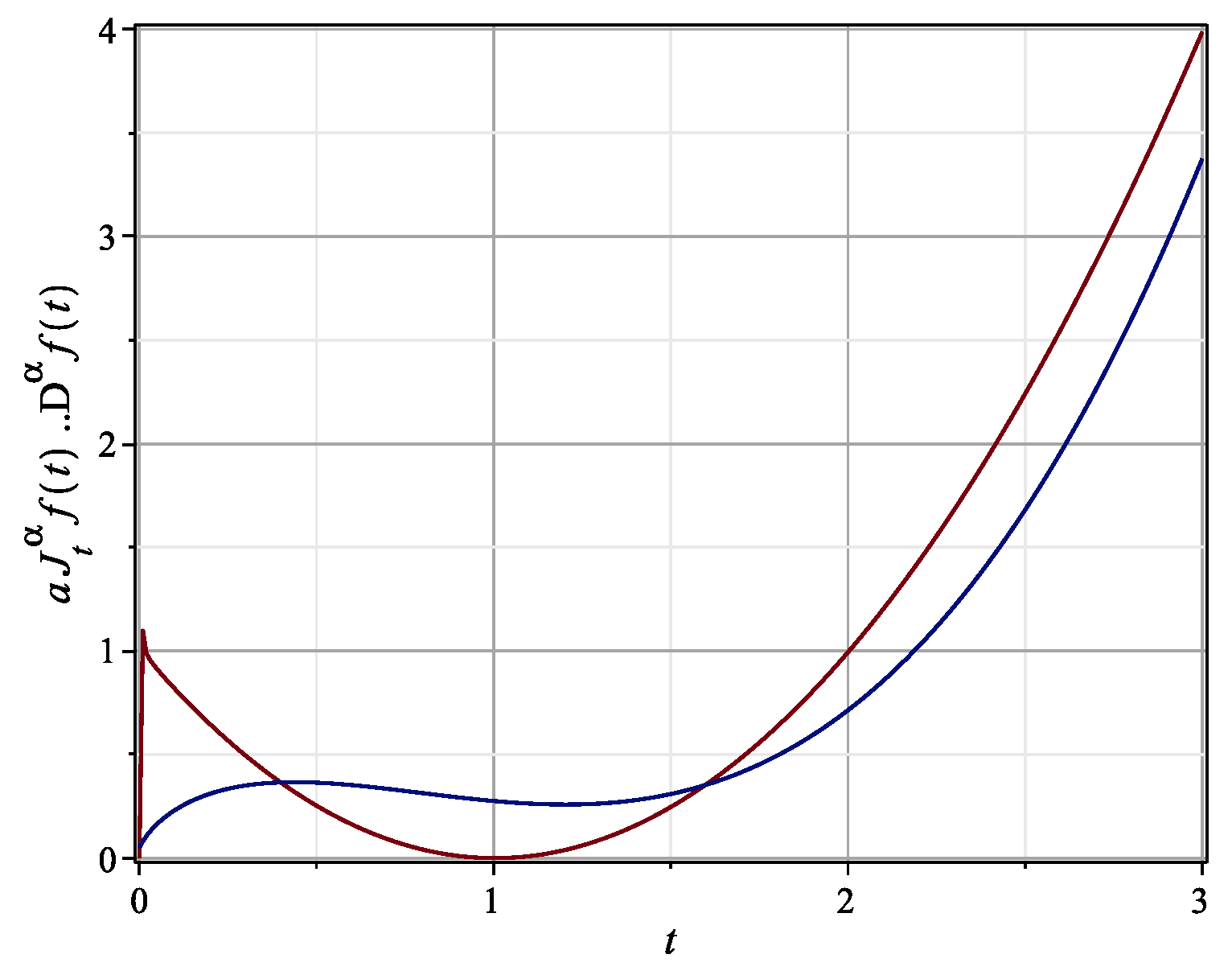
2.1. Arbitrary-Order Integral and Derivative of Riemann–Liouville
2.2. Arbitrary-Order Integral and Derivative of Grünwald–Letnikov
2.3. Arbitrary-Order Derivative of Caputo
3. Rational Approximations in the Frequency Domain
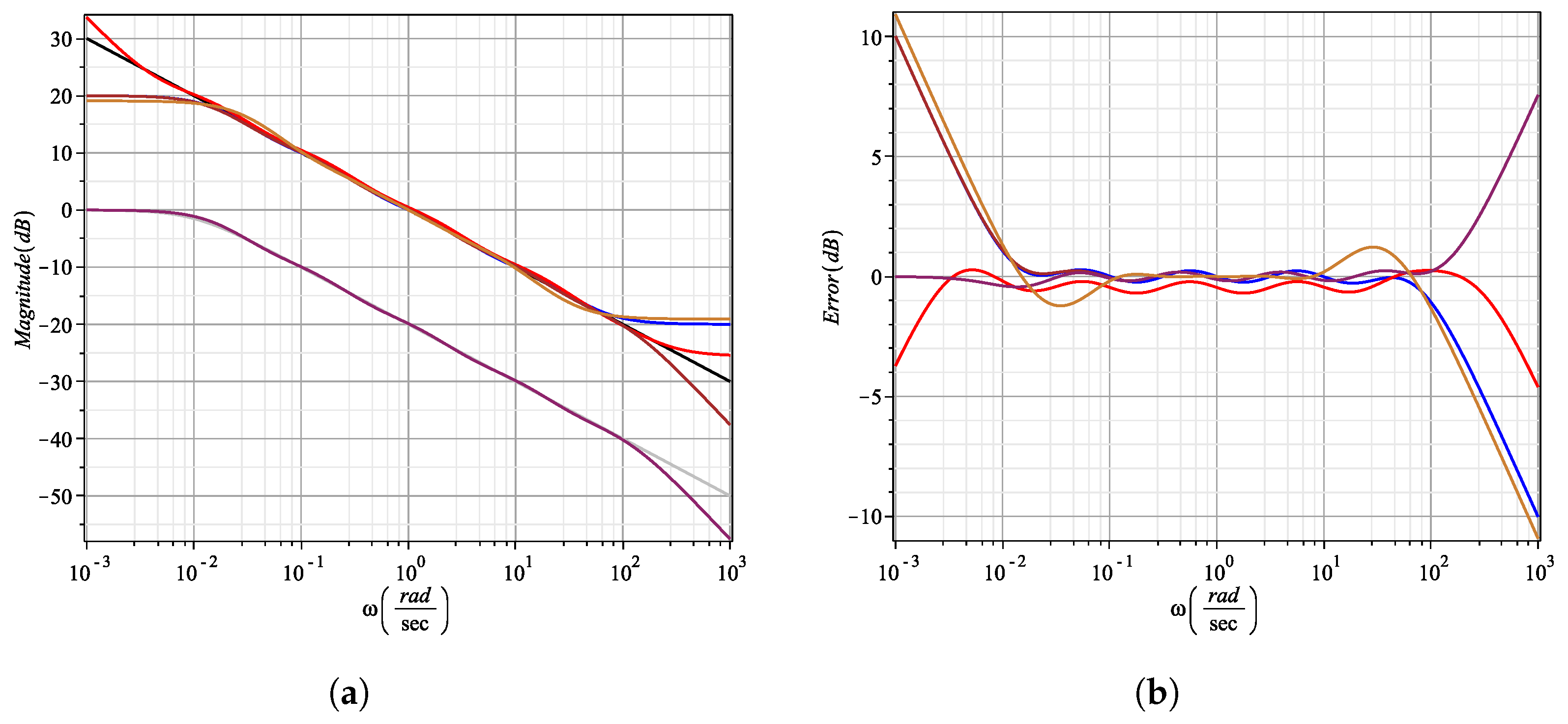
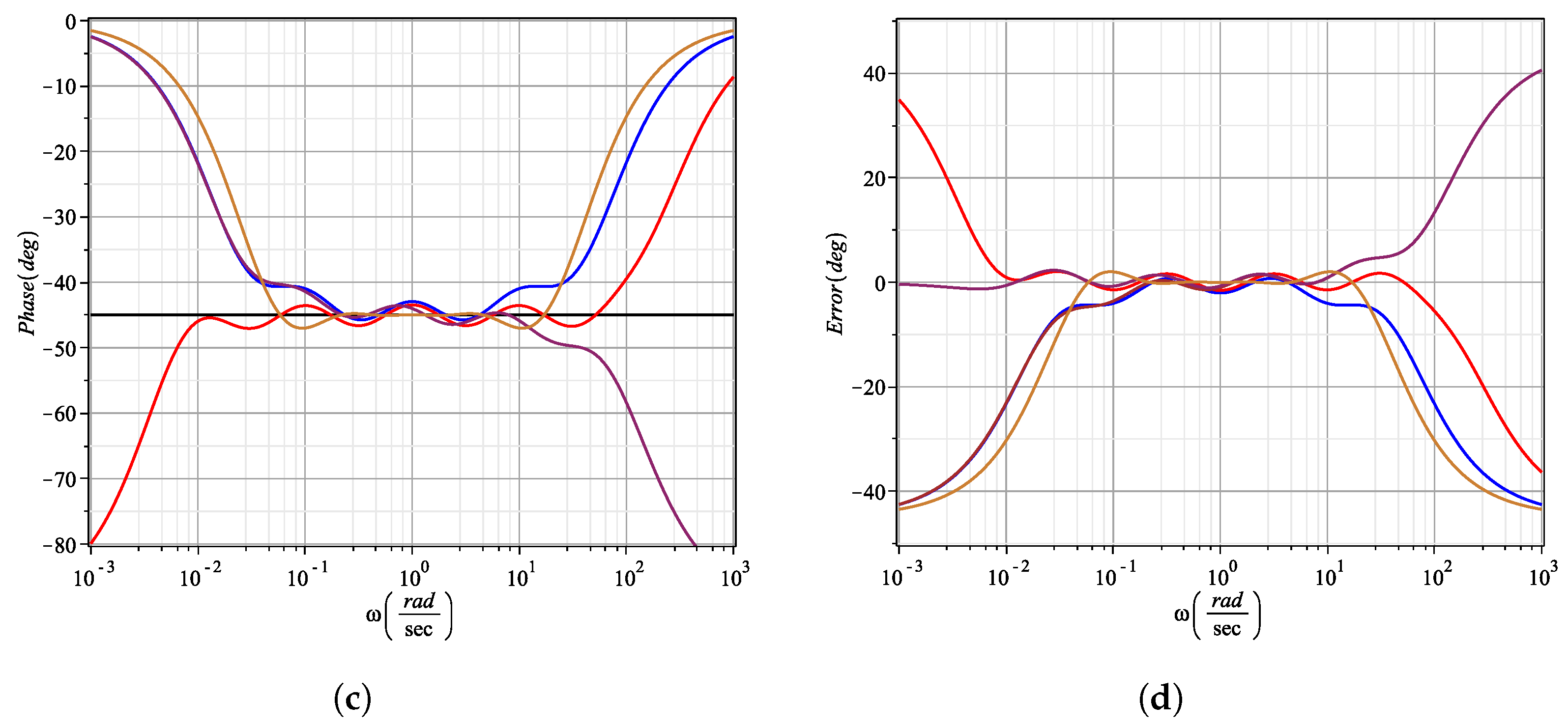
3.1. Oustaloup’s Approximation
3.2. Refined Oustaloup’s Approximation
3.3. Charef’s Approximation Version 1
3.4. Charef’s Approximation Version 2
3.5. Carlson’s Approximation
3.6. Matsuda’s Approximation
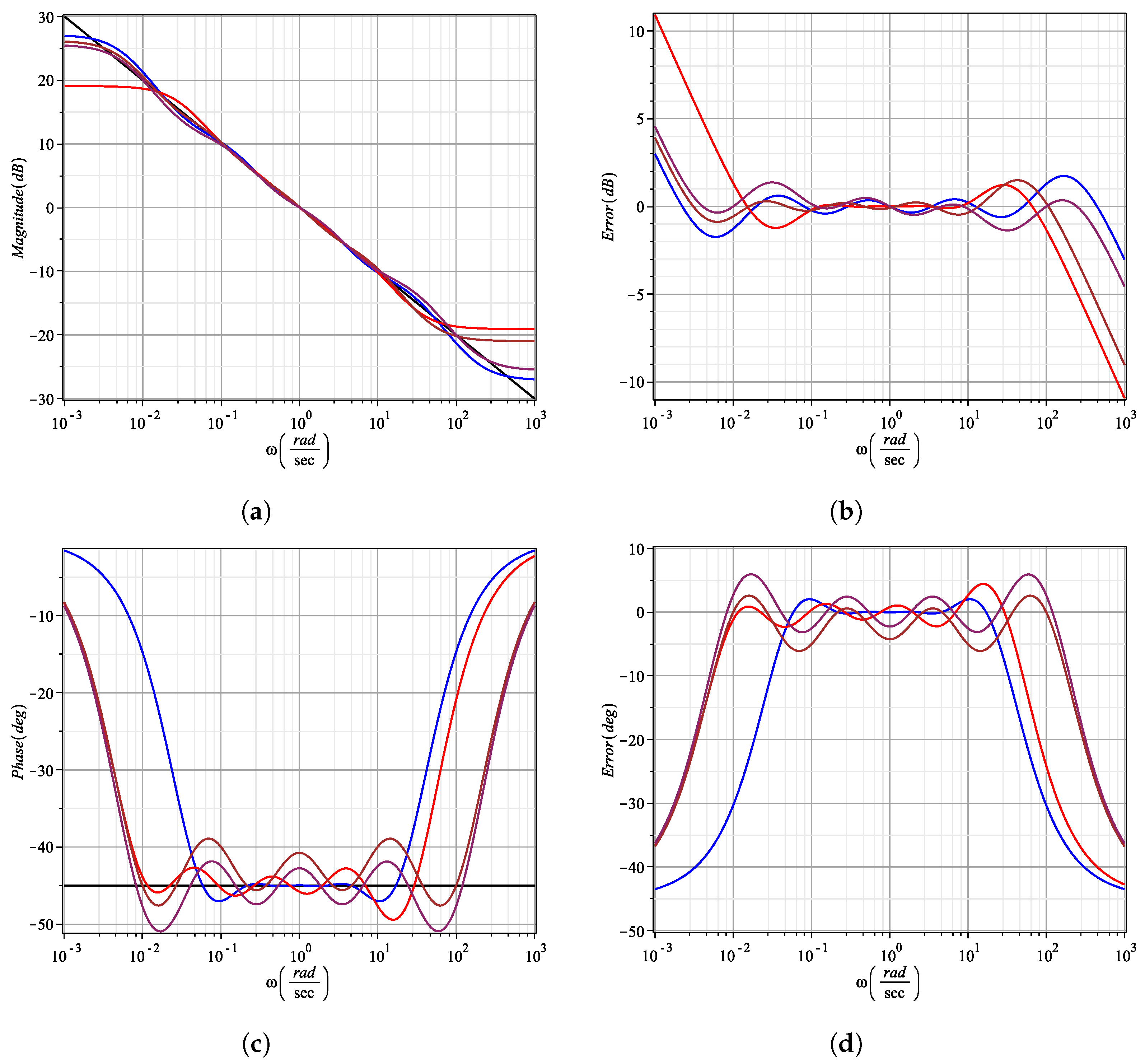
3.7. Continued Fraction Expansion Approximation
3.8. Curve-Fitting Approximation
3.9. Modified Stability Boundary Locus (MSBL) Fitting Approximation
4. Model Order Reduction
4.1. Pade’s Approximation
4.2. Stochastic Balancing Method
5. Results and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Oldham, K.B.; Spanier, J. The Fractional Calculus: Theory and Applications of Differentiation and Integration to Arbitrary Order, 1st ed.; Dover Publications: Mineola, NY, USA, 2006. [Google Scholar]
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–223. [Google Scholar] [CrossRef]
- Petráš, I. Fractional-Order Nonlinear Systems, Modeling, Analysis and Simulation, 1st ed.; Springer: London, UK; New York, NY, USA, 2011. [Google Scholar]
- Oustaloup, A.; Levron, F.; Mathieu, B.; Nanot, F.M. Frequency band complex noninteger differentiator: Characterization and synthesis. Trans. Circuits Syst. I 2000, 47, 25–39. [Google Scholar] [CrossRef]
- Xue, D.; Zhao, C.; Chen, Y. A Modified Approximation Method of Fractional Order System. In Proceedings of the International Conference on Mechatronics and Automation, Luoyang, China, 25–28 June 2006; pp. 1043–1048. [Google Scholar]
- Charef, A.; Sun, H.H.; Tsao, Y.Y.; Onaral, B. Fractal system as represented by singularity function. Trans. Autom. Control 1992, 37, 1465–1470. [Google Scholar] [CrossRef]
- Carlson, G.E.; Halijak, C.A. Approximation of a fractional capacitors (1/s) by a regular Newton process. Trans. Circuits Theory CT-11 1964, 2, 210–213. [Google Scholar] [CrossRef]
- Shrivastava, N.; Varshney, P. Rational Approximation of Fractional Order Systems Using Carlson Method. In Proceedings of the International Conference on Soft Computing Techniques and Implementation, Faridabad, India, 8–10 October 2015; pp. 76–80. [Google Scholar]
- Matsuda, K.; Fujii, H. H∞ optimized wave-absorbing control: Analytical and experimental results. J. Guid. Control Dyn. 1993, 16, 1146–1153. [Google Scholar] [CrossRef]
- Sánchez-López, C. An experimental synthesis methodology of fractional-order chaotic attractors. Nonlinear Dyn. 2020, 100, 3907–3923. [Google Scholar] [CrossRef]
- Sánchez-López, C.; Carbajal-Gómez, V.H.; Carrasco-Aguilar, M.A.; Carro-Pérez, I. Fractional-Order Memristor Emulator Circuits. Complexity 2018, 2018, 2806976. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional-order systems and PIλDμ-controllers. IEEE Trans. Autom. Control 1999, 44, 208–214. [Google Scholar] [CrossRef]
- Muñiz-Montero, C.; García-Jiménez, L.V.; Sánchez-Gaspariano, L.A.; Sánchez-López, C.; González-Díaz, V.R.; Tlelo-Cuautle, E. New alternatives for analog implementation of fractional-order integrators, differentiators and PID controllers based on integer-order integrators. Nonlinear Dyn. 2017, 90, 241–256. [Google Scholar] [CrossRef]
- Raynaud, H.F.; Zergaïnoh, A. State-space representation for fractional order controllers. Automatica 2000, 36, 1017–1021. [Google Scholar] [CrossRef]
- Monje, C.A.; Vinagre, B.M.; Calderon, A.J.; Feliu, V.; Chen, Y.Q. Auto-tuning of fractional lead-lag compensators. In Proceedings of the 16th IFAC World Congress, Prague, Czech Republic, 4–8 July 2005; pp. 319–324. [Google Scholar]
- Kapoulea, S.; Psychalinos, C.; Elwakil, A.S. Double exponent fractional-order filters: Approximation methods and realization. Circuits Syst. Signal Process. 2021, 40, 993–1004. [Google Scholar] [CrossRef]
- Kapoulea, S.; Psychalinos, C.; Elwakil, A.S. Power law filters: A new class of fractional-order filters without a fractional-order Laplacian operator. AEU-Int. J. Electron. Commun. 2021, 129, 153537. [Google Scholar] [CrossRef]
- Krishna, B.T. Studies on fractional order differentiators and integrators: A survey. Signal Process. 2011, 91, 386–426. [Google Scholar] [CrossRef]
- Nur-Deniz, F.; Baykant-Alagoz, B.; Tan, N.; Koseoglu, M. Revisiting four approximation methods for fractional order transfer function implementations: Stability preservation, time and frequency response matching analyses. Annu. Rev. Control 2020, 49, 239–257. [Google Scholar] [CrossRef]
- Atherton, D.P.; Tan, N.; Yüce, A. Methods for computing the time response of fractional-order systems. IET Control Theory Appl. 2015, 9, 817–830. [Google Scholar] [CrossRef]
- Cafagna, D.; Grassi, G. Fractional-order Chua’s circuit: Time-domain analysis, bifurcation, chaotic behaviour and test for chaos. Int. J. Bifur. Chaos. 2008, 18, 261–267. [Google Scholar] [CrossRef]
- Diethelm, K.; Ford, N.J.; Freed, A.D. A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn. 2002, 29, 3–22. [Google Scholar] [CrossRef]
- Deng, W. Numerical algorithm for the time fractional Fokker-Planck equation. J. Comput. Phy. 2007, 227, 1510–1522. [Google Scholar] [CrossRef]
- Li, C.; Xiong, J.; Li, W.; Tong, Y.; Zeng, Y. Robust synchronization for a class of fractional-order dynamical system via linear state variable. Indian J. Phys. 2013, 87, 673–678. [Google Scholar] [CrossRef]
- Parsa-Moghaddam, B.; Yaghoobi, S.; Tenreiro-Machado, J.A. An extended predictor-corrector algorithm for variable-order fractional delay differential equations. J. Compu. Nonlinear Dyn. 2016, 11. [Google Scholar] [CrossRef]
- Dabiri, A.; Butcher, E.A. Stable fractional Chebyshev differentiation matrix for the numerical solution of multi-order fractional differential equations. Nonlinear Dyn. 2017, 90, 185–201. [Google Scholar] [CrossRef]
- Dabiri, A.; Butcher, E.A. Numerical solution of multi-order fractional differential equations with multiple delays via spectral collocation methods. Appl. Math. Model. 2018, 56, 424–448. [Google Scholar] [CrossRef]
- Khovanskii, A.N. The Application of Continued Fractions and Their Generalizations to Problems in Approximation Theory; Noordhoff, Ltd.: Groningen, The Netherlands, 1963; pp. 100–110. [Google Scholar]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassam, S.M.; Harindran, V.R. Frequency response based curve fitting approximation of fractional-order PID controllers. Int. J. Appl. Math. Comput. Sci. 2019, 29, 311–326. [Google Scholar] [CrossRef] [Green Version]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassam, S.M.; Harindran, V.R. Fractional-Order Systems and PID Controllers; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Sanathanan, C.; Koerner, J. Transfer function synthesis as a ratio of two complex polynomials. IEEE Trans. Autom. Control 1963, 8, 56–58. [Google Scholar] [CrossRef]
- Koseoglu, M.; Nur-Deniz, F.; Baykant-Alagoz, B.; Alisoy, H. An effective analog circuit design of approximate fractional-order derivative models of M-SBL fitting method. Int. J. Eng. Sci. Technol. 2021, 1, 1–15. [Google Scholar] [CrossRef]
- Nur-Deniz, F.; Baykant-Alagoz, B.; Tan, N.; Atherton, D.P. An integer order approximation method based on stability boundary locus for fractional order derivative/integrator operators. ISA Trans. 2016, 62, 154–163. [Google Scholar] [CrossRef]
- Gustavsen, B.; Semlyen, A. Rational approximation of frequency domain responses by vector fitting. IEEE Trans. Power Deliv. 1999, 14, 1052–1061. [Google Scholar] [CrossRef] [Green Version]
- Djouambi, A.; Charef, A.; Besancon, A.V. Optimal approximation, simulation and analog realization of the fundamental fractional order transfer function. Int. J. Appl. Math. Comput. Sci. 2007, 17, 455–462. [Google Scholar] [CrossRef] [Green Version]
- Maione, G. Continued fractions approximation of the impulse response of fractional order dynamic systems. IET Control Theory Appl. 2008, 2, 564–572. [Google Scholar] [CrossRef]
- Maione, G. Closed-Form Rational Approximations of Fractional, Analog and Digital Differentiators/Integrators. J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 322–329. [Google Scholar] [CrossRef]
- El-Khazali, R. Discretization of fractional-order Laplacian operators. In Proceedings of the 19th IFAC Congress, Cape Town, South Africa, 24–29 August 2014; pp. 2016–2021. [Google Scholar]
- AbdelAty, A.M.; Elwakil, A.S.; Radwan, A.G.; Psychalinos, C.; Maundy, B.J. Approximation of the fractional-order Laplacian sα as a weighted sum of first-order high-pass filters. IEEE Trans. Circuit. Sys. II Express Briefs 2018, 65, 1114–1118. [Google Scholar]
- Baker George, A. Essentials of Padé Approximants, 1st ed.; Academic Press: New York, NY, USA, 1975. [Google Scholar]
- Green, M. A Relative Error Bound for Balanced Stochastic Truncation. Trans. Autom. Control 1998, 62, 961–965. [Google Scholar] [CrossRef]
- Varga, A. On stochastic balancing related model reduction. In Proceedings of the 39th IEEE Conference on Decision and Control, Sydney, NSW, Australia, 12–15 December 2000; pp. 2385–2390. [Google Scholar]
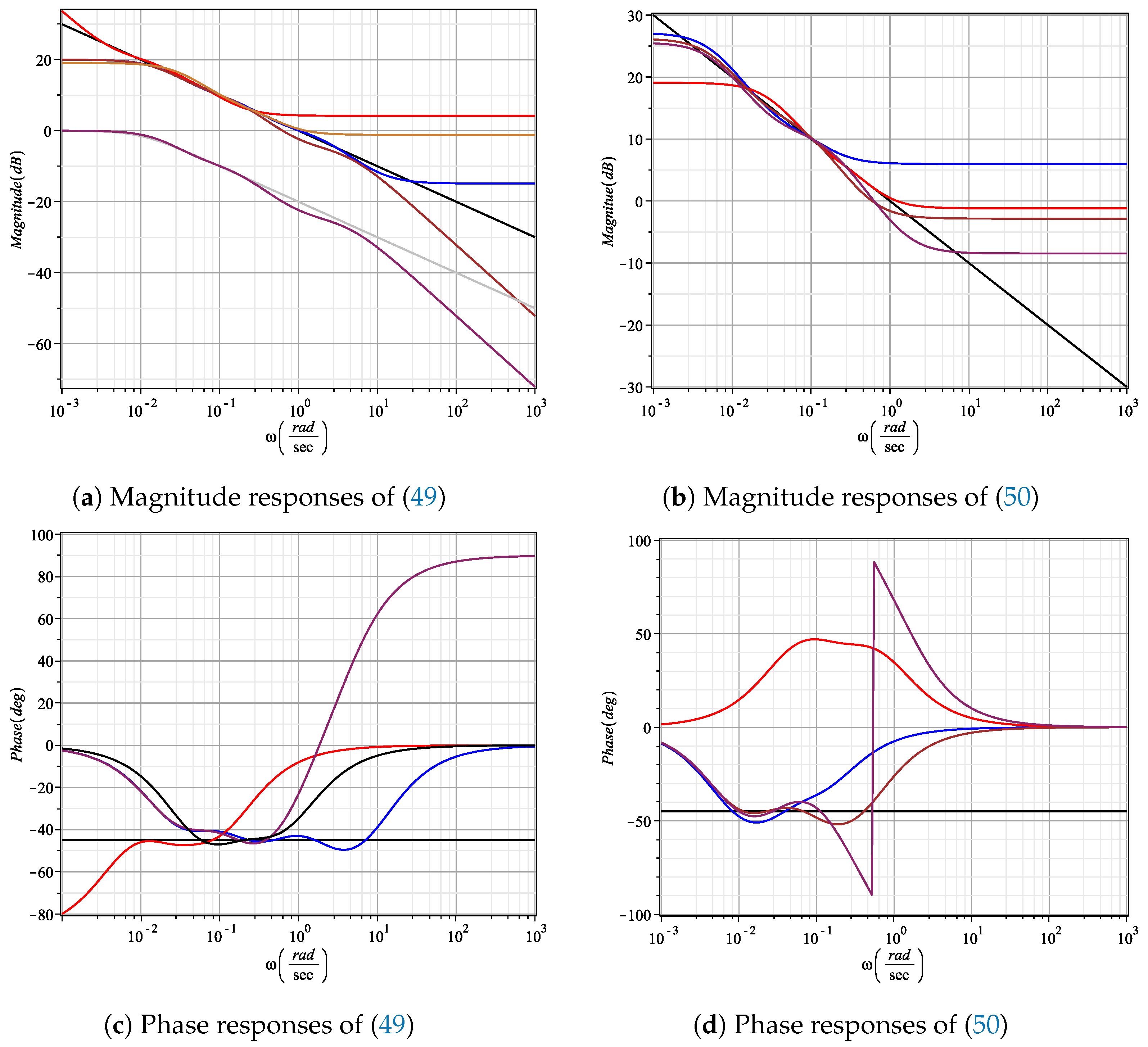
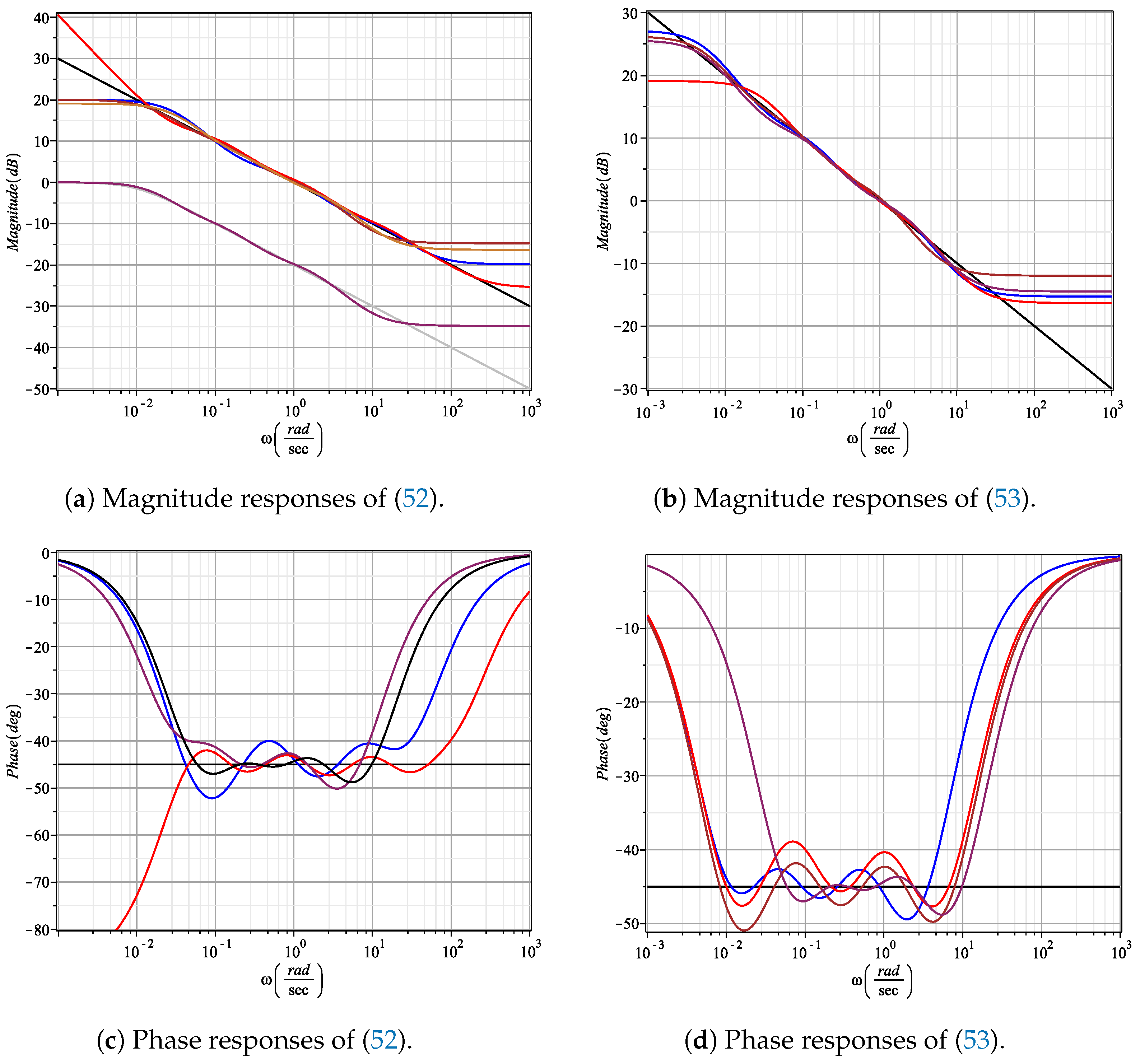
| restart: |
| assume(alpha <= 1); additionally(0 < alpha); |
| AOI_RL := proc(alpha,f) |
| 1/GAMMA(alpha)*int(f*(t-tau)(alpha-1),tau = 0..t,‘AllSolutions’) assuming t > 0; |
| end proc: |
| restart: |
| assume(p-1 < alpha);additionally(alpha <= p); |
| AOD_RL := proc(alpha,f,p) |
| diff(1/GAMMA(p-alpha)*int((t-tau)(p-alpha-1)*f,tau = 0..t),t$p) assuming t >= 0; |
| end proc: |
| restart: |
| f := subs(t = t − p*h,f): f := unapply(f,t,p,h): |
| AOI_GL := proc(alpha,h,tf,a) |
| local t,p,Sa,S1 := []: |
| for t from 0 by h to tf do |
| Sa := 0: |
| for p from 0 by 1 to (t-a)/h do |
| Sa := Sa + halpha*GAMMA(alpha + p)/(p!*GAMMA(alpha))*f(t,p,h): |
| od: |
| S1 := [op(S1),[t,Sa]]: |
| od:return(S1): |
| end proc: |
| restart: |
| f := subs(t = t − p*h,f): f := unapply(f,t,p,h): |
| AOD_GL := proc(alpha,h,tf,a) |
| local t,p,Sa,S1 := []: |
| for t from 0 by h to tf do |
| Sa := 0: |
| for p from 0 by 1 to (t-a)/h do |
| Sa := Sa + (−1)p/halpha*GAMMA(alpha + 1)/(p!*GAMMA(1 + alpha-p))*f(t,p,h): |
| od: |
| S1 := [op(S1),[t,Sa]]: |
| od:return(S1): |
| end proc: |
| restart: |
| assume(p − 1 < alpha);additionally(alpha <= p); |
| AOD_C := proc(alpha,f,p) |
| 1/GAMMA(p-alpha)*int(diff(f,tau$p)/(t-tau)(1 + alpha-p),tau = 0..t) assuming t >= 0; |
| end proc: |
| restart:Digits := 6: |
| Oustaloup := proc(alpha,wl,wh,N) |
| local p,z,Ha; |
| z := wl*(wh/wl)((2*k − 1 − alpha)/(2*N)): |
| p := wl*(wh/wl)((2*k − 1 + alpha)/(2*N)): |
| Ha := whalpha*factor(product((s + z(k))/(s + p(k)),k = 1..N)): |
| return(Ha): |
| end proc: |
| restart:Digits := 6: |
| Ref_Oustaloup := proc(alpha,wl,wh,N) |
| local p,z,Ha,b := 10,d := 9; |
| z := wl*(wh/wl)((2*k − 1 − alpha)/(2*N)): |
| p := wl*(wh/wl)((2*k − 1 + alpha)/(2*N)): |
| Ha := factor((d*wh/b)alpha*(d*s2 + b*wh*s)/(d*(1 − alpha)*s2 + b*wh*s + d*alpha)) |
| *factor(product((s + z(k))/(s + p(k)),k = 1..N)): |
| return(Ha): |
| end proc: |
| restart:Digits := 6: |
| Charef_V1 := proc(alpha,wl,wh,epsilon) |
| local p0,p,z,a,b,k,Ha,N; |
| p0 := wl*10(epsilon/(20*alpha)): |
| a := 10(epsilon/(10*(1-alpha))): |
| b := 10(epsilon/(10*alpha)): |
| N := ceil(log10(wh/p0)/log10(a*b)): |
| p := (a*b)k*p0: z := (a*b)k*a*p0: |
| Ha := factor(product(1 + s/z,k = 0..N-1)/product(1 + s/p,k = 0..N))*eval(1/salpha,s = wl): |
| return(Ha): |
| end proc: |
| restart:Digits := 6: |
| Charef_V2 := proc(alpha,wl,wh,epsilon) |
| local p0,p,z,a,b,k,Ha,N; |
| p0 := wl*10(epsilon/(20*alpha)): |
| a := 10(epsilon/(10*(1-alpha))): |
| b := 10(epsilon/(10*alpha)): |
| N := ceil(log10(wh/p0)/log10(a*b)): |
| p := (a*b)k*p0: z := (a*b)k*a*p0: |
| Ha := factor(product(1 + s/z,k = 0..N − 1)/product(1 + s/p,k = 0..N)): |
| return(Ha): |
| end proc: |
| restart:Digits := 6: |
| Carlson := proc(alpha,G,N) |
| local k,Ha := 1: |
| for k from 1 to N do |
| Ha := Ha*((1-alpha)*Ha(1/alpha) + (1+alpha)*G)/((1 + alpha)*Ha(1/alpha) + (1-alpha)*G): |
| od: |
| return(simplify(expand(numer(Ha))/expand(denom(Ha)))): |
| end proc: |
| restart:Digits := 6: |
| logspace := proc(a,b,n) evalf(10 <seq(evalf(a)..evalf(b),evalf((b − a)/(n − 1),7))>) end proc: |
| Matsuda := proc(alpha,wl,wh,N,k) |
| local w,wa,d,c,r,Ha,Dp := []: |
| w := convert(logspace(log10(wl),log10(wh),N + 1),list): |
| wa := [seq(abs(w[r]alpha),r = 1..nops(w))]: |
| d := matrix(nops(w),nops(w),0): |
| for c from 1 to N + 1 do |
| d[1,c] := wa[c]: |
| for r from 2 to c do |
| d[r,c] := (w[c] − w[r − 1])/(d[r − 1,c] − d[r − 1,r − 1]): |
| od: |
| Dp := [op(Dp),d[c,c]]: |
| od: |
| Ha := op(nops(Dp),Dp): |
| for r from nops(Dp) − 1 by −1 to 1 do |
| Ha := Dp[r] + (s-w[r])/Ha: |
| od:return(simplify(k*Ha)): |
| end proc: |
| restart:Digits := 6:with(DynamicSystems):with(numtheory): |
| CFE := proc(alpha,N) |
| local x,Ha:unassign(‘x’,‘s’): |
| Ha := cfrac(cfrac((x + 1)alpha,x,N)):x := s − 1: |
| return(expand(numer(eval(Ha)))/expand(denom(eval(Ha)))): |
| end proc: |
| CF := proc(alpha,wl,wh,N) |
| Matlab[setvar](“alpha”,alpha):Matlab[setvar](“wl”,wl): |
| Matlab[setvar](“wh”,wh):Matlab[setvar](“N”,N): |
| Matlab[evalM](“CF(alpha,wl,wh,N)”); |
| end proc: |
| Matlab script of (39)–(41) |
| function Ha = CF(alpha,wl,wh,N) |
| w = logspace(log10(wl),log10(wh)); |
| A = (j*w).alpha; |
| Ha = fitfrd(frd(A,w),N); |
| [num,den] = ss2tf(Ha.A,Ha.B,Ha.C,Ha.D); |
| Ha = minreal(tf(num,den)) |
| end |
| restart:Digits := 6:with(LinearAlgebra): |
| logspace := proc(a,b,n) evalf(10 <seq(evalf(a)..evalf(b),evalf((b-a)/(n-1),7))>) end proc: |
| MSBL := proc(alpha,wl,wh,N) |
| local A := Matrix(N),B := Vector[row](N),C,k,r,w,Ha:unassign(‘s’): |
| if N = 1 then w[1] := wh: |
| else w := convert(logspace(log10(wl),log10(wh),N),list): fi: |
| for k from 1 to N do |
| for r from 1 to N do |
| A[r,k] := (I*w[k])r-(I*w[k])(N-r + 2)*cos(Pi/2*alpha)/w[k]alpha |
| –(I*w[k])(N-r + 1)*sin(Pi/2*alpha)/w[k](alpha-1): |
| A[r,k] := Re(A[r,k]) + Im(A[r,k]): |
| B[k] := –(I*w[k])(N + 1) + I*w[k]*cos(Pi/2*alpha)/w[k]alpha |
| +sin(Pi/2*alpha)/w[k](alpha-1): |
| B[k] := Re(B[k]) + Im(B[k]): |
| od: |
| od:unassign(’k’): |
| C := convert(((1/A)%T.B%T)%T,list): |
| Ha := sort(sum(1/N + C[k + 1]*s(N-k),k = 0..N-1),s,descending)/ |
| sort(sum(sN/N + C[N-k]*s(N-k-1),k = 0..N-1),s,descending): |
| return(Ha): |
| end proc: |
| restart:Digits := 6:with(SignalProcessing): |
| Pade := proc(Ha,m,n) |
| local A,Hp:unassign(‘s’): |
| A := series(Ha,s = 0.200): |
| Hp := convert(A,ratpoly,m,n): |
| end proc: |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Colín-Cervantes, J.D.; Sánchez-López, C.; Ochoa-Montiel, R.; Torres-Muñoz, D.; Hernández-Mejía, C.M.; Sánchez-Gaspariano, L.A.; González-Hernández, H.G. Rational Approximations of Arbitrary Order: A Survey. Fractal Fract. 2021, 5, 267. https://doi.org/10.3390/fractalfract5040267
Colín-Cervantes JD, Sánchez-López C, Ochoa-Montiel R, Torres-Muñoz D, Hernández-Mejía CM, Sánchez-Gaspariano LA, González-Hernández HG. Rational Approximations of Arbitrary Order: A Survey. Fractal and Fractional. 2021; 5(4):267. https://doi.org/10.3390/fractalfract5040267
Chicago/Turabian StyleColín-Cervantes, José Daniel, Carlos Sánchez-López, Rocío Ochoa-Montiel, Delia Torres-Muñoz, Carlos Manuel Hernández-Mejía, Luis Abraham Sánchez-Gaspariano, and Hugo Gustavo González-Hernández. 2021. "Rational Approximations of Arbitrary Order: A Survey" Fractal and Fractional 5, no. 4: 267. https://doi.org/10.3390/fractalfract5040267
APA StyleColín-Cervantes, J. D., Sánchez-López, C., Ochoa-Montiel, R., Torres-Muñoz, D., Hernández-Mejía, C. M., Sánchez-Gaspariano, L. A., & González-Hernández, H. G. (2021). Rational Approximations of Arbitrary Order: A Survey. Fractal and Fractional, 5(4), 267. https://doi.org/10.3390/fractalfract5040267