Investigation and Application of Fractal Theory in Cement-Based Materials: A Review
Abstract
1. Introduction
2. Fractal Dimensions
2.1. Fractal Dimension of Pore Structure
2.1.1. Fractal Dimension of Pore Surface (Ds)
2.1.2. Fractal Dimension of Pore Volume (Dv)
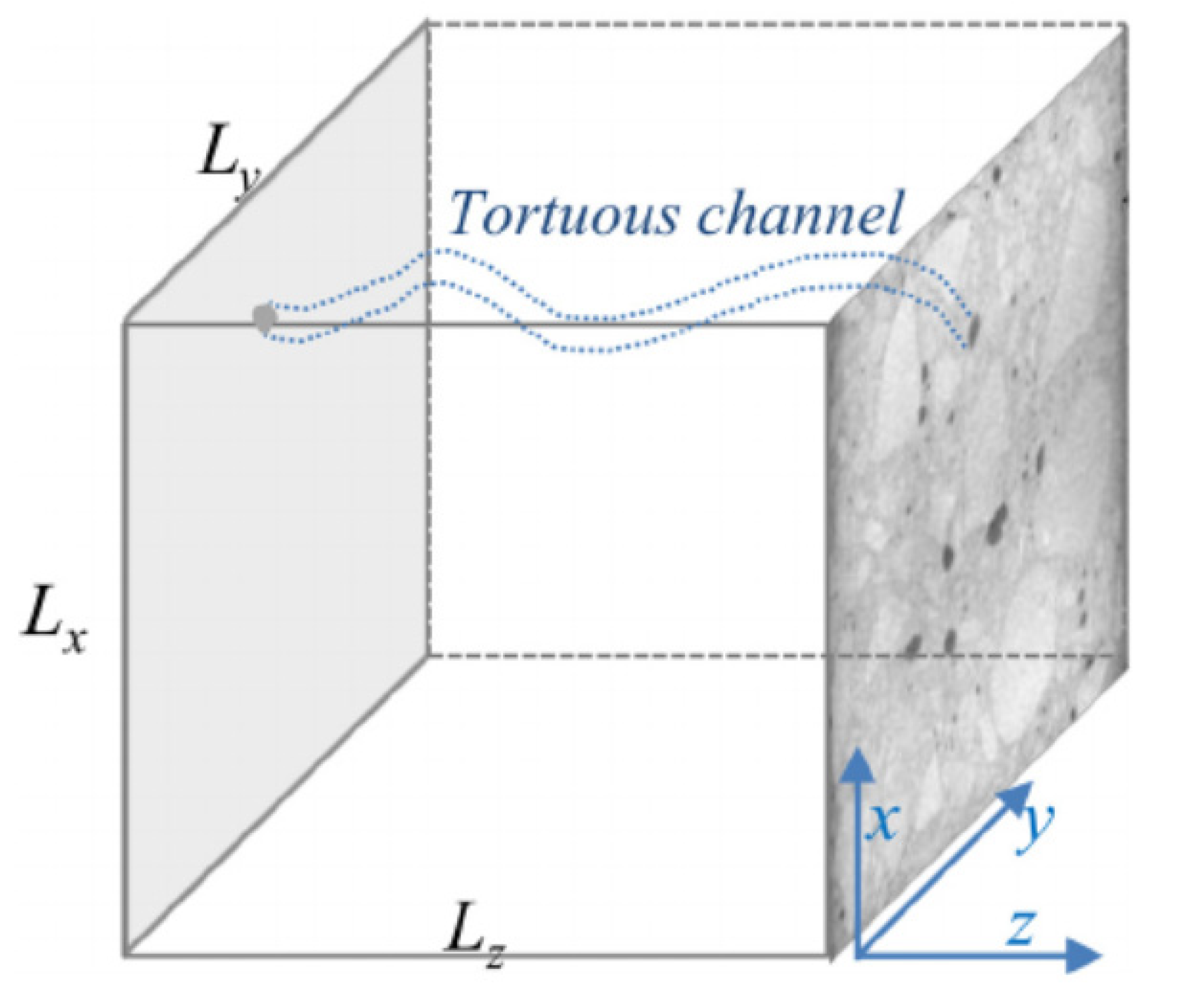
2.1.3. Fractal Dimension of Pore Tortuosity (Dt)
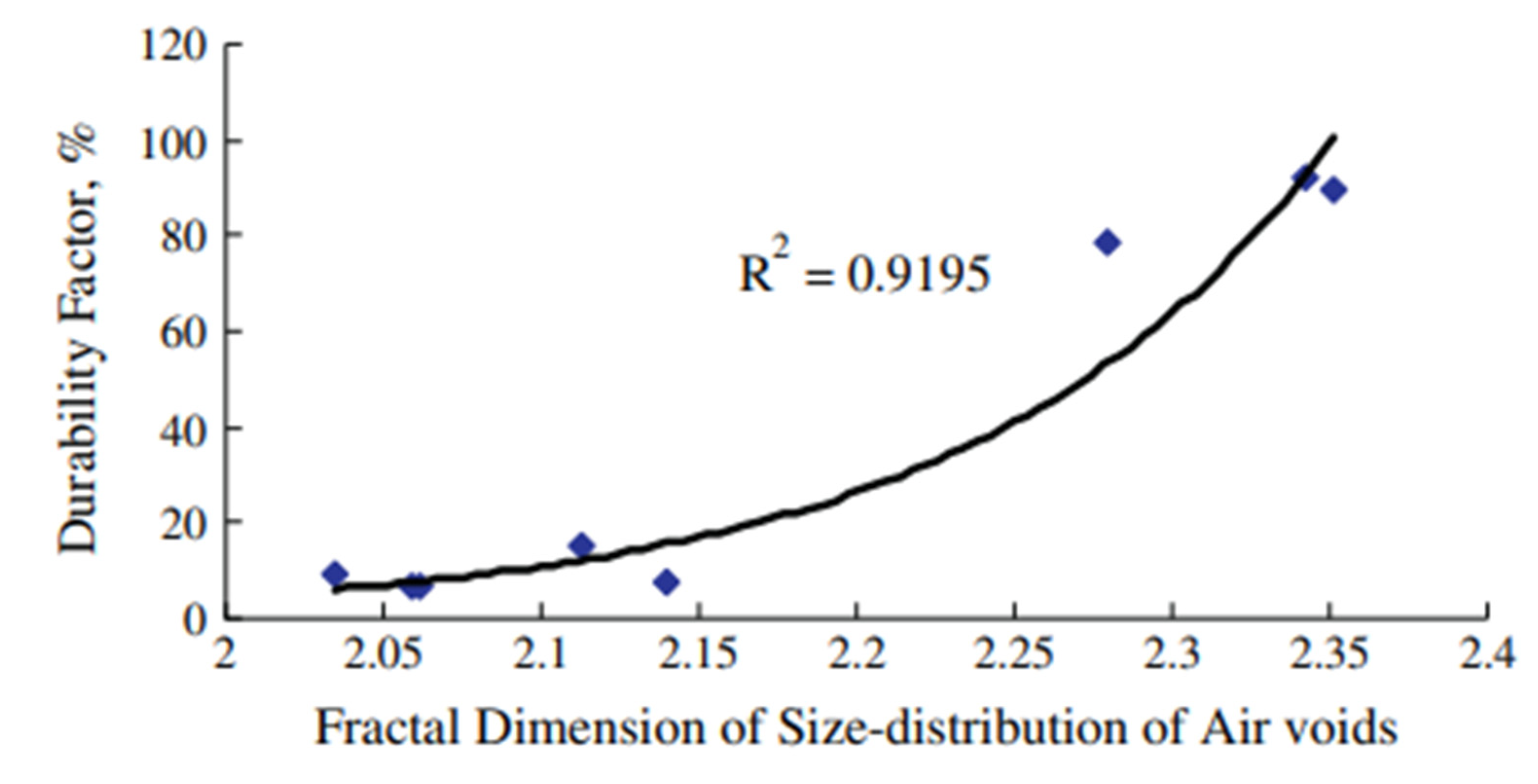
2.2. Fractal Dimension of Air Void (Da)
2.3. Fractal Dimension of Fracture Surface (Dfs)
2.4. Fractal Dimension of Crack (Dc)
2.5. Fractal Dimension of Particle Distribution (Dp)
3. Investigation of the Test Methods and Fractal Models of Cement-Based Materials
3.1. Fractal Dimension of Pore Structure
3.1.1. Fractal Dimension of Pore Surface (Ds)
Ds by MIP, Zhang’s Model, and Neimark’s Model
Ds by NAD and FHH Isotherm Equation
Ds by NAD and Neimark’s Thermodynamic Method
3.1.2. Fractal Dimension of Pore Volume (Dv)
Dv by the MIP, the Space-Filling Model, and the Menger Sponge Model
Dv by LT-DSC and the Menger Sponge Model
Dv by 1H Low-Field NMR
Dv by SANS or SAXS and a Simple Logarithmic Equation
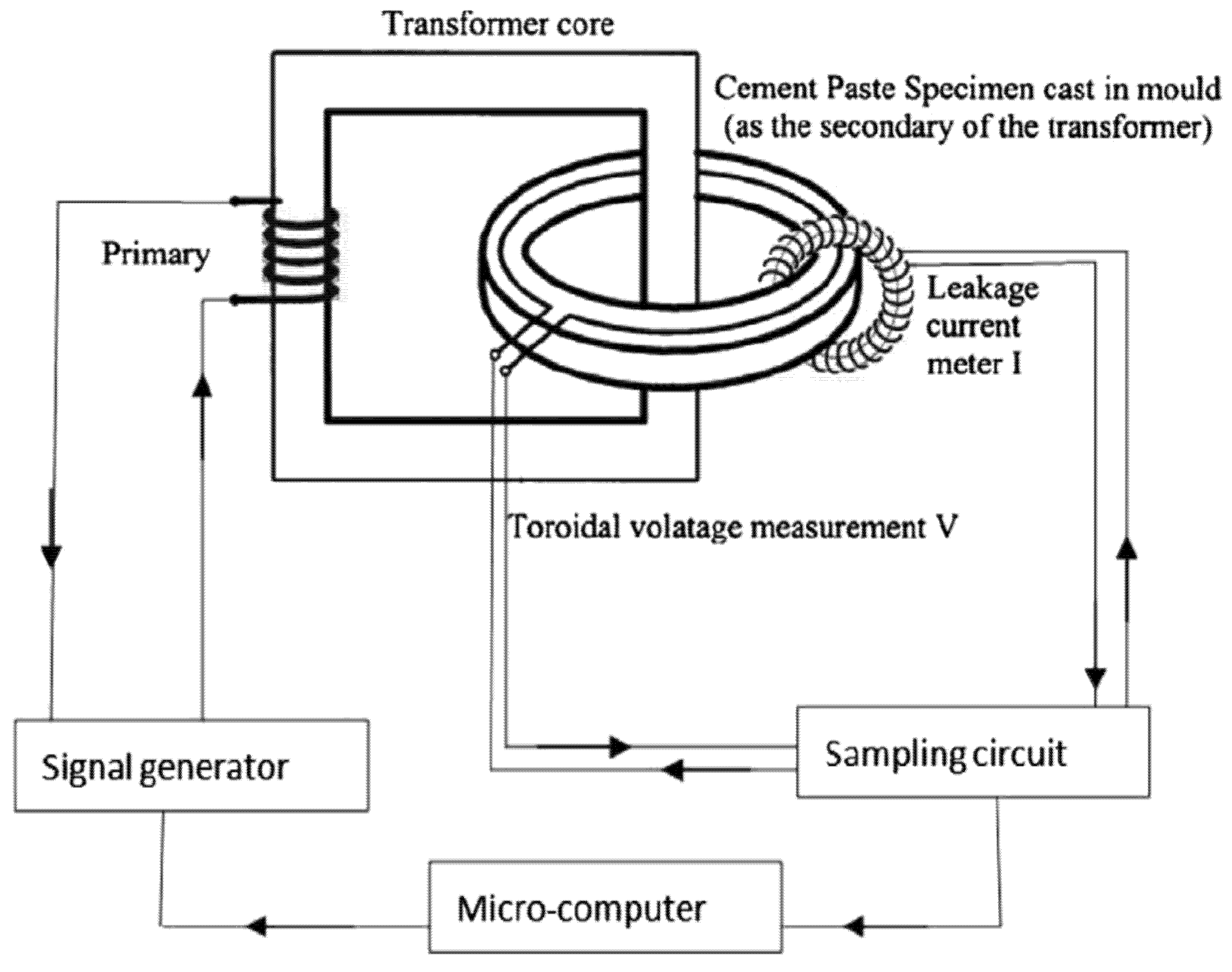
3.1.3. Fractal Dimension of Pore Tortuosity (Dt)
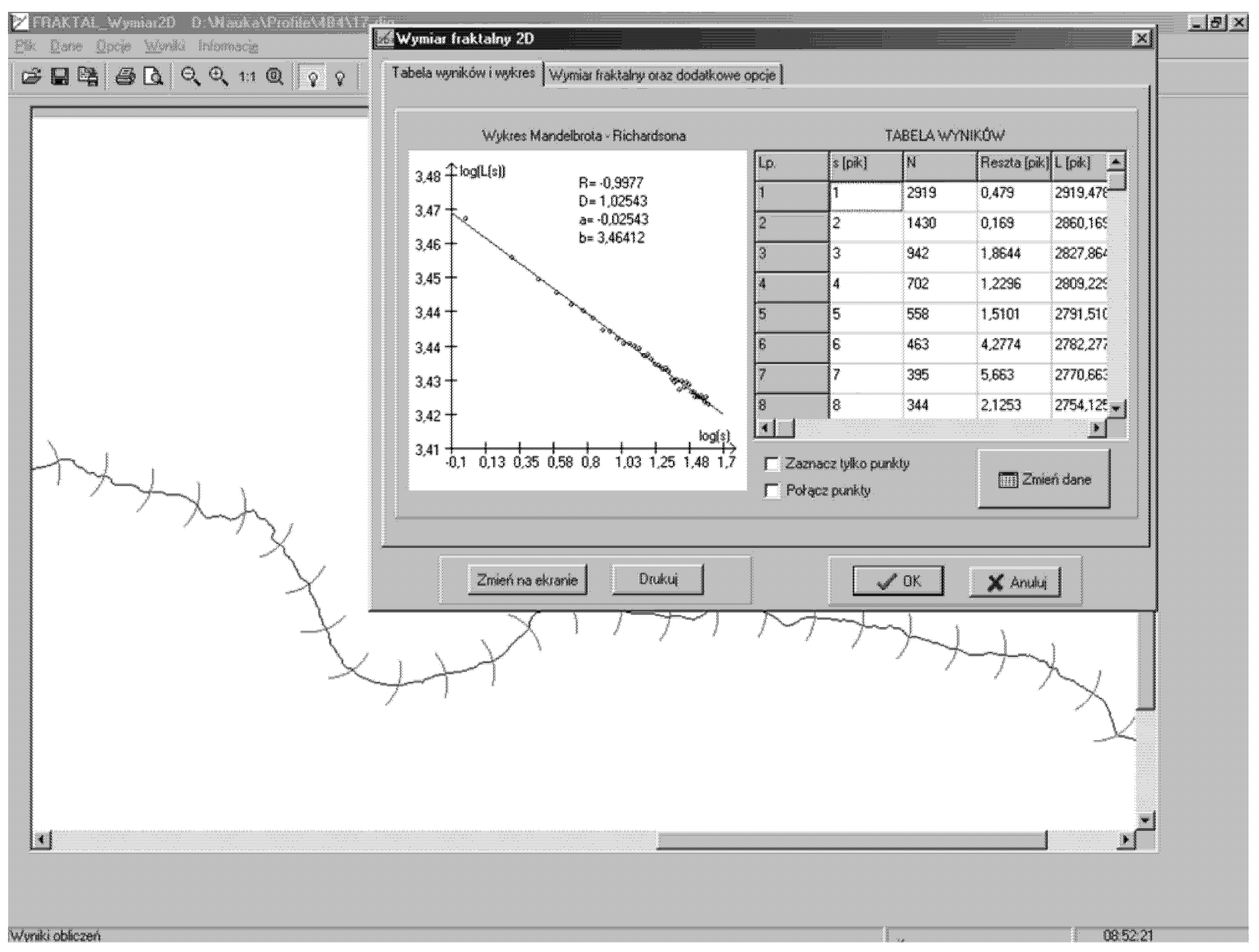
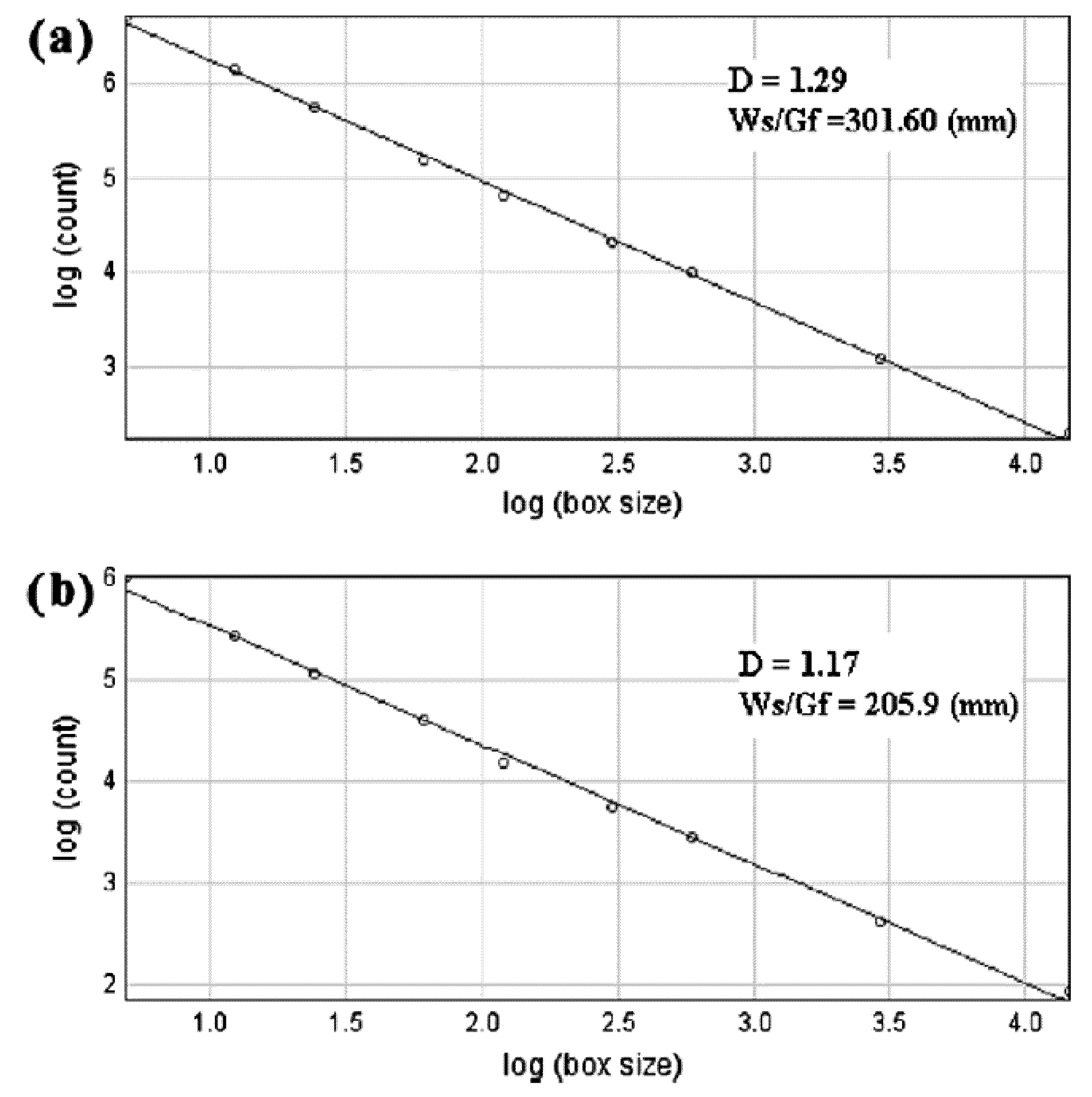
Dt by Image Analysis and the Box-Counting Method
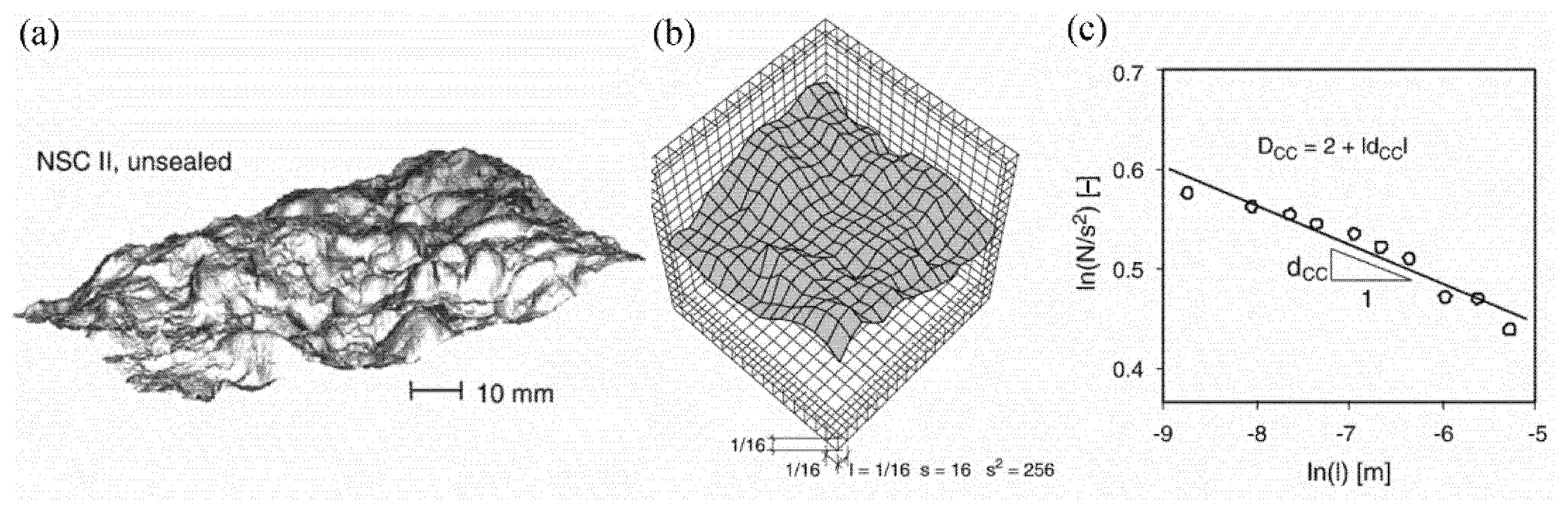
Dt by the Backscattered Electron (BSE) Imaging Model
Dt by NCIM Method and Two-Phase Fractal Model
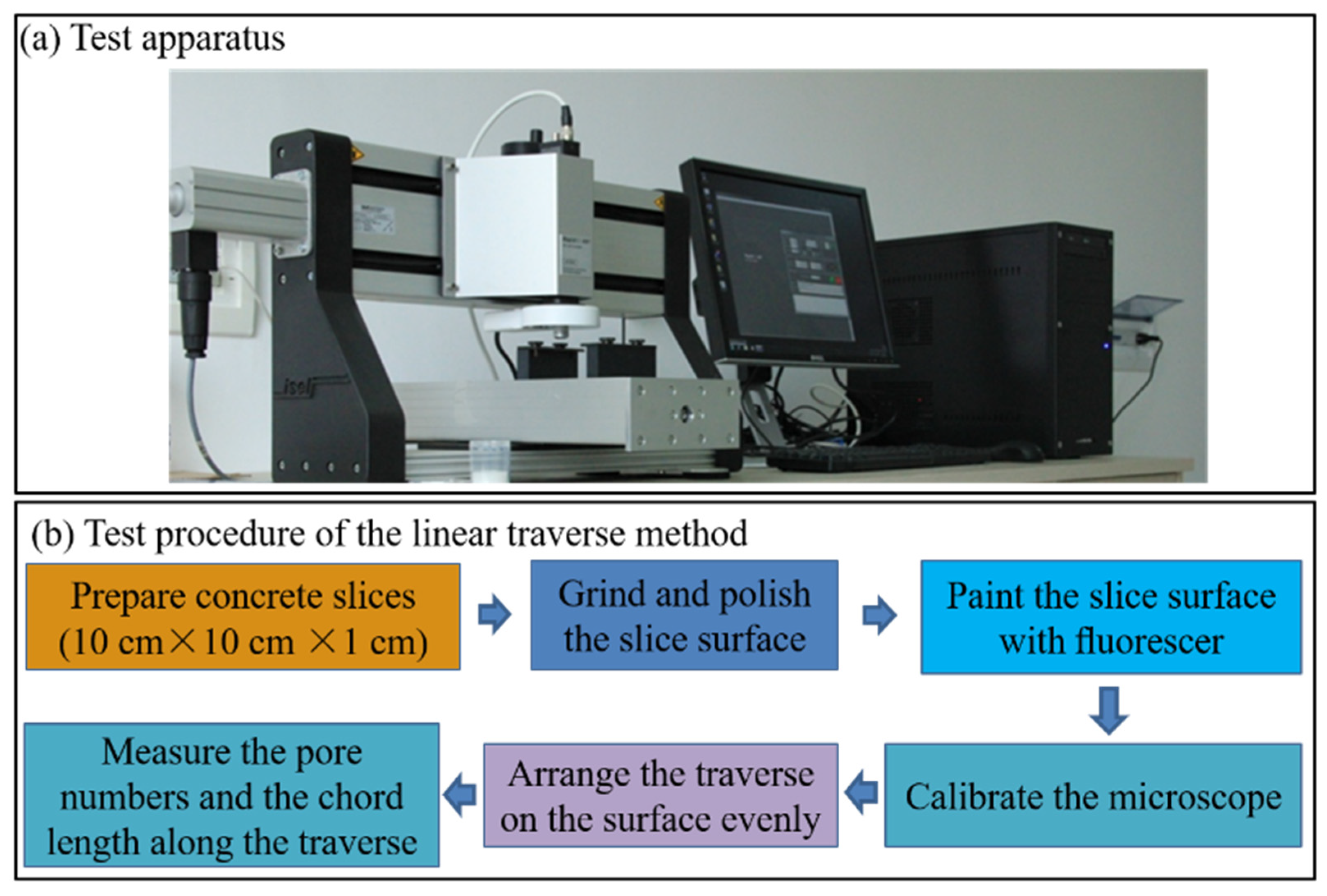
3.2. Fractal Dimension of Air Void (Da) by Air-Void Analyzer and Box-Counting Method
3.3. Fractal Dimension of Fracture Surface (Dfs)
3.3.1. Dfs by Stereoscopic SEM and Friel’s Method
3.3.2. Dfs by 3D Laser Scanning Technology and the Projective Covering Method
3.3.3. Dfs by Image Analyzer Method and 2D Fast Fourier Transform Function
3.3.4. Dfs by Laser Profilometer and the Chord Method
3.3.5. Dfs by Confocal Microscope/SEM and the Box-Counting Method
3.4. Fractal Dimension of Crack (Dc) by Various Testing Techniques and Box-Counting Method
3.5. Fractal Dimension of Particle Distribution (Dp)
3.5.1. Dp by Laser Granulometry and Yu’s Method
3.5.2. Dp by Image Analysis System
4. Application of Fractal Theory on Performance of Cement-Based Materials
4.1. Mechanical Properties
4.2. Volume Stability
4.3. Durability
4.3.1. Transport Properties
4.3.2. Frost Resistance
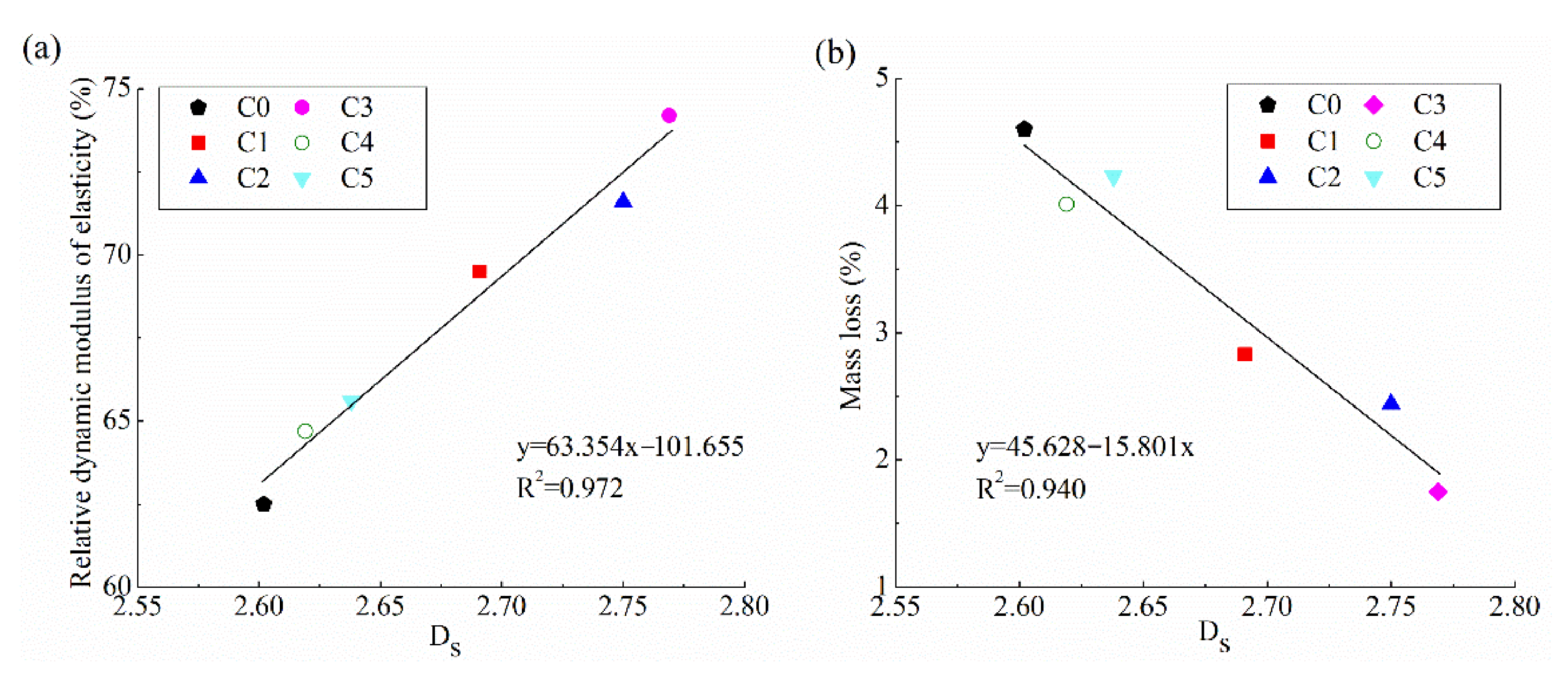
4.3.3. Corrosion Resistance
4.4. Fracture Mechanism and Fracture Mechanics
4.5. Evaluation of the Crack Irregularity, Concrete Flexural Performance, and Fracture Energy
4.6. Evaluation of Pozzolanic Reactivity and Dispersion State of Powders
5. Conclusions and Outlook
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wang, L.; Li, G.; Li, X.; Guo, F.; Tang, S.; Lu, X.; Haniff, A. Influence of reactivity and dosage of MgO expansive agent on shrinkage and crack resistance of face slab concrete. Cem. Concr. Compos. 2021, 125, 104333. [Google Scholar] [CrossRef]
- Zhang, P.; Wang, K.; Wang, J.; Guo, J.; Ling, Y. Macroscopic and microscopic analyses on mechanical performance of metakaolin/fly ash based geopolymer mortar. J. Clean. Prod. 2021, 294, 126193. [Google Scholar] [CrossRef]
- Gao, Y.; Wu, K.; Yuan, Q. Limited fractal behavior in cement paste upon mercury intrusion porosimetry test: Analysis and models. Constr. Build. Mater. 2021, 276, 122231. [Google Scholar] [CrossRef]
- Zeng, Q.; Jike, N.; Liu, J.; Wang, Z.; Wang, J. Fractal analysis of stress-dependent diffusivity of porous cementitious materials. Fractals 2020, 28, 2050117. [Google Scholar] [CrossRef]
- Wang, X.; Yu, R.; Shui, Z.; Song, Q.; Zhang, Z. Mix design and characteristics evaluation of an eco-friendly Ultra-High Performance Concrete incorporating recycled coral based materials. J. Clean. Prod. 2017, 165, 70–80. [Google Scholar] [CrossRef]
- Lv, X.; Shen, W.; Wang, L.; Dong, Y.; Zhang, J.; Xie, Z. A comparative study on the practical utilization of iron tailings as a complete replacement of normal aggregates in dam concrete with different gradation. J. Clean. Prod. 2019, 211, 704–715. [Google Scholar] [CrossRef]
- Zhang, P.; Wang, K.; Wang, J.; Guo, J.; Hu, S.; Ling, Y. Mechanical properties and prediction of fracture parameters of geopolymer/alkali-activated mortar modified with PVA fiber and nano-SiO2. Ceram. Int. 2020, 46, 20027–20037. [Google Scholar] [CrossRef]
- Wang, L.; Tang, S. Editorial: An introduction to fractals in construction materials. Fractals 2021, 29, 2102001. [Google Scholar] [CrossRef]
- Lü, Q.; Qiu, Q.; Zheng, J.; Wang, J.; Zeng, Q. Fractal dimension of concrete incorporating silica fume and its correlations to pore structure, strength and permeability. Constr. Build. Mater. 2019, 228, 116986. [Google Scholar] [CrossRef]
- Wang, L.; Chen, E.; Ruan, S.; Tang, S. Editorial: New Technologies for Investigating Microstructures and Enhancing Performance of Cementitious Materials. Front. Mater. 2021, 8, 669862. [Google Scholar] [CrossRef]
- Zhang, L.; Zhou, J. Fractal characteristics of pore structure of hardened cement paste prepared by pressurized compact molding. Constr. Build. Mater. 2020, 259, 119856. [Google Scholar] [CrossRef]
- Zeng, Q.; Luo, M.; Pang, X.; Li, L.; Li, K. Surface fractal dimension: An indicator to characterize the microstructure of cement-based porous materials. Appl. Surf. Sci. 2013, 282, 302–307. [Google Scholar] [CrossRef]
- Zeng, Q.; Li, K.; Fen-Chong, T.; Dangla, P. Surface fractal analysis of pore structure of high-volume fly-ash cement pastes. Appl. Surf. Sci. 2010, 257, 762–768. [Google Scholar] [CrossRef]
- Yu, P.; Duan, Y.; Chen, E.; Tang, S.; Wang, X.R. Microstructure-based fractal models for heat and mass transport properties of cement paste. Int. J. Heat Mass Transf. 2018, 126, 432–447. [Google Scholar] [CrossRef]
- Jin, S.; Zhang, J.; Huang, B. Fractal analysis of effect of air void on freeze–thaw resistance of concrete. Constr. Build. Mater. 2013, 47, 126–130. [Google Scholar] [CrossRef]
- Tang, S.; Wang, Y.; Geng, Z.; Xu, X.; Yu, W.; Chen, J. Structure, Fractality, Mechanics and Durability of Calcium Silicate Hydrates. Fractal Fract. 2021, 5, 47. [Google Scholar] [CrossRef]
- Zhang, P.; Gao, Z.; Wang, J.; Wang, K. Numerical modeling of rebar-matrix bond behaviors of nano-SiO2 and PVA fiber reinforced geopolymer composites. Ceram. Int. 2021, 47, 11727–11737. [Google Scholar] [CrossRef]
- Xie, Z.; Wang, L.; Zhu, Z.; Fu, Z.; Lv, X. Simulation of the temperature field for massive concrete structures using an interval finite element method. Eng. Comput. 2020, 37, 45. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, S.; Shi, Y.; Tang, S.; Chen, E. Effect of silica fume and PVA fiber on the abrasion resistance and volume stability of concrete. Compos. Part B: Eng. 2017, 130, 28–37. [Google Scholar] [CrossRef]
- Erdem, S.; Dawson, A.; Thom, N.H. Influence of the micro- and nanoscale local mechanical properties of the interfacial transition zone on impact behavior of concrete made with different aggregates. Cem. Concr. Res. 2012, 42, 447–458. [Google Scholar] [CrossRef]
- Peled, A.; Castro, J.; Weiss, W. Atomic force and lateral force microscopy (AFM and LFM) examinations of cement and cement hydration products. Cem. Concr. Compos. 2013, 36, 48–55. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, P.; Guo, J.; Wang, K. Bonding behavior of concrete matrix and alkali-activated mortar incorporating nano-SiO2 and polyvinyl alcohol fiber: Theoretical analysis and prediction model. Ceram. Int. 2021, 47, 31638–31649. [Google Scholar] [CrossRef]
- Wang, L.; Jin, M.; Zhou, S.; Tang, S.; Lu, X. Investigation of microstructure of C-S-H and micro-mechanics of cement pastes under NH4NO3 dissolution by 29Si MAS NMR and microhardness. Measurement 2021, 185, 110019. [Google Scholar] [CrossRef]
- Mandelbrot, B.B.; Wheeler, J.A. The Fractal Geometry of Nature. Am. J. Phys. 1983, 51, 286–287. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal GEOMETRY of Nature; W.H. Freeman and Company: San Francisco, CA, USA, 1983. [Google Scholar]
- Shang, X.; Yang, J.; Wang, S.; Zhang, M. Fractal analysis of 2D and 3D mesocracks in recycled aggregate concrete using X-ray computed tomography images. J. Clean. Prod. 2021, 304, 127083. [Google Scholar] [CrossRef]
- Yin, Y.; Ren, Q.; Shen, L. Study on the effect of aggregate distribution on mechanical properties and damage cracks of concrete based on multifractal theory. Constr. Build. Mater. 2020, 262, 120086. [Google Scholar] [CrossRef]
- Winslow, D.N. The fractal nature of the surface of cement paste. Cem. Concr. Res. 1985, 15, 817–824. [Google Scholar] [CrossRef]
- Gao, Y.; Gu, Y.; Mu, S.; Jiang, J.; Liu, J. The multifractal property of heterogeneous microstructure in cement paste. Fractals 2021, 29, 2140006. [Google Scholar] [CrossRef]
- Tang, S.; He, Z.; Cai, X.; Cai, R.; Zhou, W.; Li, Z.; Shao, H.; Wu, T.; Chen, E. Volume and surface fractal dimensions of pore structure by NAD and LT-DSC in calcium sulfoaluminate cement pastes. Constr. Build. Mater. 2017, 143, 395–418. [Google Scholar] [CrossRef]
- Jin, S.; Zhang, J.; Han, S. Fractal analysis of relation between strength and pore structure of hardened mortar. Constr. Build. Mater. 2017, 135, 1–7. [Google Scholar] [CrossRef]
- Tang, S.; Huang, J.; Duan, L.; Yu, P.; Chen, E. A review on fractal footprint of cement-based materials. Powder Technol. 2020, 370, 237–250. [Google Scholar] [CrossRef]
- Zarnaghi, V.N.; Fouroghi-Asl, A.; Nourani, V.; Ma, H. On the pore structures of lightweight self-compacting concrete containing silica fume. Constr. Build. Mater. 2018, 193, 557–564. [Google Scholar] [CrossRef]
- Wang, L.; Jin, M.M.; Guo, F.X.; Wang, Y.; Tang, S.W. Pore Structural and Fractal Analysis of the Influence of FLY ASH and Silica Fume on the Mechanical Property and Abrasion Resistance of Concrete. Fractals 2021, 29, 2140003. [Google Scholar] [CrossRef]
- Liu, P.; Chen, Y.; Sha, F.; Yu, Z.; Shao, G. Study on micro structure and composition distribution of concrete surface zone based on fractal theory and XCT technology. Constr. Build. Mater. 2020, 263, 120209. [Google Scholar] [CrossRef]
- Rolph, S. Fractal geometry: Mathematical foundations and applications. Math. Gaz. 1990, 74, 288–317. [Google Scholar]
- Wang, L.; Jin, M.; Wu, Y.; Zhou, Y.; Tang, S. Hydration, shrinkage, pore structure and fractal dimension of silica fume modified low heat Portland cement-based materials. Constr. Build. Mater. 2021, 272, 121952. [Google Scholar] [CrossRef]
- Huang, J.; Li, W.; Huang, D.; Wang, L.; Chen, E.; Wu, C.; Wang, B.; Deng, H.; Tang, S.; Shi, Y.; et al. Fractal Analysis on Pore Structure and Hydration of Magnesium Oxysulfate Cements by First Principle, Thermodynamic and Microstructure-Based Methods. Fractal Fract. 2021, 5, 164. [Google Scholar] [CrossRef]
- Zhang, B.; Li, S. Determination of the Surface Fractal Dimension for Porous Media by Mercury Porosimetry. Ind. Eng. Chem. Res. 1995, 34, 1383–1386. [Google Scholar] [CrossRef]
- Zhang, B.; Liu, W.; Liu, X. Scale-dependent nature of the surface fractal dimension for bi- and multi-disperse porous solids by mercury porosimetry. Appl. Surf. Sci. 2006, 253, 1349–1355. [Google Scholar] [CrossRef]
- Neimark, A. A new approach to the determination of the surface fractal dimension of porous solids. Phys. A: Stat. Mech. its Appl. 1992, 191, 258–262. [Google Scholar] [CrossRef]
- Pfeifer, P.; Avnir, D. Chemistry in noninteger dimensions between two and three. I. Fractal theory of heterogeneous surfaces. J. Chem. Phys. 1983, 79, 3558–3565. [Google Scholar] [CrossRef]
- Jaroniec, M. Evaluation of the fractal dimension from a single adsorption isotherm. Langmuir 1995, 11, 2316–2317. [Google Scholar] [CrossRef]
- Neimark, A.V.; Unger, K.K. Method of Discrimination of Surface Fractality. J. Colloid Interface Sci. 1993, 158, 412–419. [Google Scholar] [CrossRef]
- Avnir, D.; Jaroniec, M. An isotherm equation for adsorption on fractal surfaces of heterogeneous porous materials. Langmuir 1989, 5, 1431–1433. [Google Scholar] [CrossRef]
- Pfeifer, P.; Cole, M.W.; Krim, J. Pfeifer, Cole, and Krim reply. Phys. Rev. Lett. 1990, 65, 663. [Google Scholar] [CrossRef] [PubMed]
- Ji, X.; Chan, S.; Feng, N. Fractal model for simulating the space-filling process of cement hydrates and fractal dimensions of pore structure of cement-based materials. Cem. Concr. Res. 1997, 27, 1691–1699. [Google Scholar] [CrossRef]
- Li, L.; Li, Z.; Cao, M.; Tang, Y.; Zhang, Z. Nanoindentation and porosity fractal dimension of calcium carbonate whisker reinforced cement paste after elevated temperatures (up to 900 °C). Fractals 2021, 29, 2140001. [Google Scholar] [CrossRef]
- Wang, L.; He, T.; Zhou, Y.; Tang, S.; Tan, J.; Liu, Z.; Su, J. The influence of fiber type and length on the cracking resistance, durability and pore structure of face slab concrete. Constr. Build. Mater. 2021, 282, 122706. [Google Scholar] [CrossRef]
- Yu, B.; Cheng, P. A fractal permeability model for bi-dispersed porous media. Int. J. Heat Mass Transf. 2002, 45, 2983–2993. [Google Scholar] [CrossRef]
- Liang, M.; Yang, S.; Miao, T.; Yu, B. Analysis of electroosmotic characters in fractal porous media. Chem. Eng. Sci. 2015, 127, 202–209. [Google Scholar] [CrossRef]
- Tang, S.; Cai, X.; Zhou, W.; Shao, H.; He, Z.; Li, Z.; Ji, W.; Chen, E. In-situ and continuous monitoring of pore evolution of calcium sulfoaluminate cement at early age by electrical impedance measurement. Constr. Build. Mater. 2016, 117, 8–19. [Google Scholar] [CrossRef]
- Wheatcraft, S.W.; Tyler, S.W. An explanation of scale-dependent dispersivity in heterogeneous aquifers using concepts of fractal geometry. Water Resour. Res. 1988, 24, 566–578. [Google Scholar] [CrossRef]
- Yu, B. Analysis of Flow in Fractal Porous Media. Appl. Mech. Rev. 2008, 61, 050801. [Google Scholar] [CrossRef]
- Tang, S.; Chen, E.; Shao, H.; Li, Z. A fractal approach to determine thermal conductivity in cement pastes. Constr. Build. Mater. 2015, 74, 73–82. [Google Scholar] [CrossRef]
- Tang, S.; Li, Z.; Zhu, H.; Shao, H.; Chen, E. Permeability interpretation for young cement paste based on impedance measurement. Constr. Build. Mater. 2014, 59, 120–128. [Google Scholar] [CrossRef]
- Tang, S.W.; Li, Z.J.; Chen, E.; Shao, H.Y. Impedance measurement to characterize the pore structure in Portland cement paste. Constr. Build. Mater. 2014, 51, 106–112. [Google Scholar] [CrossRef]
- Issa, M.A.; Issa, M.A.; Islam, M.S.; Chudnovsky, A. Fractal dimension—A measure of fracture roughness and toughness of concrete. Eng. Fract. Mech. 2003, 70, 125–137. [Google Scholar] [CrossRef]
- Lange, D.; Jennings, H.M.; Shah, S.P. Relationship between Fracture Surface Roughness and Fracture Behavior of Cement Paste and Mortar. J. Am. Ceram. Soc. 1993, 76, 589–597. [Google Scholar] [CrossRef]
- Wang, Y.; Diamond, S. A fractal study of the fracture surfaces of cement pastes and mortars using a stereoscopic SEM method. Cem. Concr. Res. 2001, 31, 1385–1392. [Google Scholar] [CrossRef]
- Yan, A.; Wu, K.-R.; Zhang, N.; Yao, W. Influence of concrete composition on the characterization of fracture surface. Cem. Concr. Compos. 2003, 25, 153–157. [Google Scholar] [CrossRef]
- Konkol, J.; Prokopski, G. Fracture toughness and fracture surfaces morphology of metakaolinite-modified concrete. Constr. Build. Mater. 2016, 123, 638–648. [Google Scholar] [CrossRef]
- Ficker, T. Fractal strength of cement gels and universal dimension of fracture surfaces. Theor. Appl. Fract. Mech. 2008, 50, 167–171. [Google Scholar] [CrossRef]
- Erdem, S. X-ray computed tomography and fractal analysis for the evaluation of segregation resistance, strength response and accelerated corrosion behaviour of self-compacting lightweight concrete. Constr. Build. Mater. 2014, 61, 10–17. [Google Scholar] [CrossRef]
- Zheng, D.; Song, W.; Fu, J.; Xue, G.; Li, J.; Cao, S. Research on mechanical characteristics, fractal dimension and internal structure of fiber reinforced concrete under uniaxial compression. Constr. Build. Mater. 2020, 258, 120351. [Google Scholar] [CrossRef]
- Ringot, E.; Bascoul, A. About the analysis of microcracking in concrete. Cem. Concr. Compos. 2001, 23, 261–266. [Google Scholar] [CrossRef]
- Szeląg, M. Fractal characterization of thermal cracking patterns and fracture zone in low-alkali cement matrix modified with microsilica. Cem. Concr. Compos. 2020, 114, 103732. [Google Scholar] [CrossRef]
- Zhu, D.; Tang, A.; Wan, C.; Zeng, Y.; Wang, Z. Investigation on the flexural toughness evaluation method and surface cracks fractal characteristics of polypropylene fiber reinforced cement-based composites. J. Build. Eng. 2021, 43, 103045. [Google Scholar] [CrossRef]
- Zhuang, N.; Dong, H.; Zhou, Y.; Chen, D. Cracking behavior of reinforced concrete piles externally bonded with carbon fiber reinforced polymer in a marine environment. Constr. Build. Mater. 2018, 190, 1154–1162. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhou, Y.; Zhou, Y.; Pan, T.; Zhang, Q.; Liu, D. Cracking behavior of reinforced concrete beams strengthened with CFRP anchorage system under cyclic and monotonic loading. Eng. Struct. 2020, 207, 110222. [Google Scholar] [CrossRef]
- Peng, J.; Wu, Z.; Zhao, G. Fractal analysis of fracture in concrete. Theor. Appl. Fract. Mech. 1997, 27, 135–140. [Google Scholar] [CrossRef]
- Li, L.; Sun, H.-X.; Zhang, Y.; Yu, B. Surface Cracking and Fractal Characteristics of Bending Fractured Polypropylene Fiber-Reinforced Geopolymer Mortar. Fractal Fract. 2021, 5, 142. [Google Scholar] [CrossRef]
- Yu, K.; Zheng, Z.S. Fractal study on granularity distribution of powder. Mat. Sci. Eng. 1995, 13, 30–34. [Google Scholar] [CrossRef]
- Wang, L.J.; Huang, F.Y.; Ma, X.C. Experimental research on the saturation point of superplasticizers in cement based on frac-tal dimension. J. Wuhan Univ. Technol. 2008, 30, 36–39. [Google Scholar] [CrossRef]
- Zhang, Y.; Kong, X.; Gao, L.; Bai, Y. Characterization of the mesostructural organization of cement particles in fresh cement paste. Constr. Build. Mater. 2016, 124, 1038–1050. [Google Scholar] [CrossRef]
- Mindess, S.; Young, J.F.; Darwin, D. Concrete, 2nd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2002. [Google Scholar]
- Duan, P.; Shui, Z.; Chen, W.; Shen, C. Effects of metakaolin, silica fume and slag on pore structure, interfacial transition zone and compressive strength of concrete. Constr. Build. Mater. 2013, 44, 1–6. [Google Scholar] [CrossRef]
- An, M.; Wang, Y.; Yu, Z. Damage mechanisms of ultra-high-performance concrete under freeze–thaw cycling in salt solution considering the effect of rehydration. Constr. Build. Mater. 2019, 198, 546–552. [Google Scholar] [CrossRef]
- Jin, S.; Zheng, G.; Yu, J. A micro freeze-thaw damage model of concrete with fractal dimension. Constr. Build. Mater. 2020, 257, 119434. [Google Scholar] [CrossRef]
- Aili, A.; Maruyama, I. Review of Several Experimental Methods for Characterization of Micro- and Nano-Scale Pores in Cement-Based Material. Int. J. Concr. Struct. Mater. 2020, 14, 14. [Google Scholar] [CrossRef]
- Honorio, T.; Bary, B.; Benboudjema, F. Thermal properties of cement-based materials: Multiscale estimations at early-age. Cem. Concr. Compos. 2018, 87, 205–219. [Google Scholar] [CrossRef]
- Wang, L.; Guo, F.X.; Yang, H.M.; Wang, Y.; Tang, S.W. Comparison of FLY ASH, PVA Fiber, MgO and Shrinkage-reducing Admixture on the Frost Resistance of Face Slab Concrete via Pore Structural and Fractal Analysis. Fractals 2021, 29, 2140002. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, G.; Pang, C.; Yan, X.; Qin, H. Influence of pore structures on the frost resistance of concrete. Mag. Concr. Res. 2017, 69, 271–279. [Google Scholar] [CrossRef]
- Hu, W. Modeling the Influence of Composition and Pore Structure on Mechanical Properties of Autoclaved Cellular Concrete. Ph.D. Dissertation, University of Pittsburgh, Pittsburgh, PA, USA, 1997. [Google Scholar]
- Mechtcherine, V. Fracture mechanical behavior of concrete and the condition of its fracture surface. Cem. Concr. Res. 2009, 39, 620–628. [Google Scholar] [CrossRef]
- Erdem, S.; Blankson, M.A. Fractal–fracture analysis and characterization of impact-fractured surfaces in different types of concrete using digital image analysis and 3D nanomap laser profilometery. Constr. Build. Mater. 2013, 40, 70–76. [Google Scholar] [CrossRef]
- Matsuoka, S. Fractal Character of Fracture Surfaces on Metals. Zairyo 1991, 40, 498–505. [Google Scholar] [CrossRef][Green Version]
- Kim, J.; Choi, Y.C.; Choi, S. Fractal Characteristics of Pore Structures in GGBFS-based Cement Pastes. Appl. Surf. Sci. 2018, 428, 304–314. [Google Scholar] [CrossRef]
- Guo, W.; Qin, H.G.; Chen, H.S.; Sun, W. Fractal theory and its applications in the study of concrete materials. J. Chin. Silic. Soc. 2010, 38, 1362–1368. [Google Scholar] [CrossRef]
- Moskovits, M. The fractal nature of particle size distributions of grounds clinker. Cem. Concr. Res. 1990, 20, 499–505. [Google Scholar] [CrossRef]
- Guo, W.; Qin, H.; Ji, X.; Shui, Z. Fractal study on properties of general Portland cement. China Concr. Cem. Prod. 2018, 4, 22–25. (In Chinese) [Google Scholar]
- Zhu, M.; Hu, S.; He, Z. Research on the physiochemical properties of Portland cement and its fractal theory. J. Wuhan Univ. Technol. 2008, 30, 28–31. [Google Scholar] [CrossRef]
- Tang, M.; Ba, H. Study on fractal characteristics and activity of superfine fly ash. Mater. Sci. Technol. 2002, 10, 89–92. (In Chinese) [Google Scholar] [CrossRef]
- Tang, M.; Yang, F.; Chen, Z. Chaos and fractal characteristics and durablity of cement- based materials. Concrete 2010, 6, 1–6. (In Chinese) [Google Scholar] [CrossRef]
- Zeng, Q.; Li, K.; Fen-Chong, T.; Dangla, P. Pore structure of cement pastes through NAD and MIP analysis. Adv. Cem. Res. 2016, 28, 23–32. [Google Scholar] [CrossRef]
- Zeng, Q.; Li, K.; Fen-Chong, T.; Dangla, P. Pore structure characterization of cement pastes blended with high-volume fly-ash. Cem. Concr. Res. 2012, 42, 194–204. [Google Scholar] [CrossRef]
- Washburn, E.W. Note on a Method of Determining the Distribution of Pore Sizes in a Porous Material. Proc. Natl. Acad. Sci. USA 1921, 7, 115–116. [Google Scholar] [CrossRef] [PubMed]
- Liu, P.; Cui, S.; Li, Z.; Xu, X.; Guo, C. Influence of surrounding rock temperature on mechanical property and pore structure of concrete for shotcrete use in a hot-dry environment of high-temperature geothermal tunnel. Constr. Build. Mater. 2019, 207, 329–337. [Google Scholar] [CrossRef]
- Aligizaki, K.K. Pore Structure of Cement-Based Materials: Testing, Interpretation and Requirements; CRC Press: Boca Raton, FL, USA, 2005; pp. 1349–1355. [Google Scholar]
- Tang, M.; Li, J.Q. Research on Fractal Characteristics of Cement-Based Materials by Nitrogen Adsorption Method. Adv. Mater. Res. 2011, 415–417, 1545–1552. [Google Scholar] [CrossRef]
- Ridi, F.; Fratini, E.; Baglioni, P. Fractal Structure Evolution during Cement Hydration by Differential Scanning Calorimetry: Effect of Organic Additives. J. Phys. Chem. C 2013, 117, 25478–25487. [Google Scholar] [CrossRef]
- Halperin, W.P.; Jehng, J.-Y.; Song, Y.-Q. Application of spin-spin relaxation to measurement of surface area and pore size distributions in a hydrating cement paste. Magn. Reson. Imaging 1994, 12, 169–173. [Google Scholar] [CrossRef]
- She, A.; Ma, K.; Liao, G.; Yao, W.; Zuo, J. Investigation of hydration and setting process in nanosilica-cement blended pastes: In situ characterization using low field nuclear magnetic resonance. Constr. Build. Mater. 2021, 304, 124631. [Google Scholar] [CrossRef]
- Ji, Y.; Sun, Z.; Yang, X.; Li, C.; Tang, X. Assessment and mechanism study of bleeding process in cement paste by 1H low-field NMR. Constr. Build. Mater. 2015, 100, 255–261. [Google Scholar] [CrossRef]
- Wang, L.; He, Z.; Cai, X. Characterization of pozzolanic reaction and its effect on the C-S-H Gel in fly Ash-cement paste. J. Wuhan Univ. Technol. Sci. Ed. 2011, 26, 319–324. [Google Scholar] [CrossRef]
- Wang, L.; Yang, H.; Zhou, S.; Chen, E.; Tang, S. Hydration, mechanical property and C-S-H structure of early-strength low-heat cement-based materials. Mater. Lett. 2018, 217, 151–154. [Google Scholar] [CrossRef]
- He, Z.; Liang, W.; Wang, L.; Wang, J. Synthesis of C3S by sol-gel technique and its features. J. Wuhan Univ. Technol. Sci. Ed. 2010, 25, 138–141. [Google Scholar] [CrossRef]
- Pop, A.; Badea, C.; Ardelean, I. The Effects of Different Superplasticizers and Water-to-Cement Ratios on the Hydration of Gray Cement Using T2-NMR. Appl. Magn. Reson. 2013, 44, 1223–1234. [Google Scholar] [CrossRef]
- Cai, J.; Yu, B.; Zou, M.; Luo, L. Fractal characterization of spontaneous co-current imbibition in porous media. Energy Fuels 2010, 24, 1860–1867. [Google Scholar] [CrossRef]
- ASTM International. ASTM C457/457M-16. In Standard Test Method for Microscopical Determination of Parameters of the Air-Void System in Hardened Concrete; ASTM International: West Conshohocken, PA, USA, 2016. [Google Scholar]
- China Electricity Council. DL/T 5150-2017. In Test Code for Hydraulic Concrete; China Electric Power Press: Beijing, China, 2017. [Google Scholar]
- Friel, J.; Pande, C. A direct determination of fractal dimension of fracture surfaces using scanning electron microscopy and stereoscopy. J. Mater. Res. 1993, 8, 100–104. [Google Scholar] [CrossRef]
- Goff, T. Comment on “Fractal mapping of digitized images: Application to the topography of Arizona and comparison with synthetic images” by J. Huang and DL Turcotte. J. Geophys. Res.-Sol. Ea. 1990, 95, 5159. [Google Scholar] [CrossRef]
- Cao, T.; Wang, W.; Tighe, S.; Wang, S. Crack image detection based on fractional differential and fractal dimension. IET Comput. Vis. 2019, 13, 79–85. [Google Scholar] [CrossRef]
- Wang, L.; Yang, H.; Zhou, S.; Chen, E.; Tang, S. Mechanical properties, long-term hydration heat, shinkage behavior and crack resistance of dam concrete designed with low heat Portland (LHP) cement and fly ash. Constr. Build. Mater. 2018, 187, 1073–1091. [Google Scholar] [CrossRef]
- Yang, X.; Li, L.; Dai, H.; Jia, M. Effect of fractal dimension in concrete meso-structure on its axial mechanical behavior: A numerical case study. Fractals 2021, 29, 2140011. [Google Scholar] [CrossRef]
- Wang, L.; Yang, H.; Dong, Y.; Chen, E.; Tang, S. Environmental evaluation, hydration, pore structure, volume deformation and abrasion resistance of low heat Portland (LHP) cement-based materials. J. Clean. Prod. 2018, 203, 540–558. [Google Scholar] [CrossRef]
- Shi, Y.; Dong, Y.; Wang, L.; Chen, X.; Li, X. Different Chemical Composition of Aggregate Impact on Hydraulic Concrete Interfacial Transition Zone. Asian J. Chem. 2014, 26, 1267–1270. [Google Scholar] [CrossRef]
- Wang, L.; Guo, F.; Lin, Y.; Yang, H.; Tang, S. Comparison between the effects of phosphorous slag and fly ash on the C-S-H structure, long-term hydration heat and volume deformation of cement-based materials. Constr. Build. Mater. 2020, 250, 118807. [Google Scholar] [CrossRef]
- Wang, L.; Dong, Y.; Zhou, S.; Chen, E.; Tang, S. Energy saving benefit, mechanical performance, volume stabilities, hydration properties and products of low heat cement-based materials. Energy Build. 2018, 170, 157–169. [Google Scholar] [CrossRef]
- Li, Y.; Bao, J.; Guo, Y. The relationship between autogenous shrinkage and pore structure of cement paste with mineral admixtures. Constr. Build. Mater. 2010, 24, 1855–1860. [Google Scholar] [CrossRef]
- Ma, Y.; Ye, G. The shrinkage of alkali activated fly ash. Cem. Concr. Res. 2015, 68, 75–82. [Google Scholar] [CrossRef]
- Wang, L.; Luo, R.Y.; Zhang, W.; Jin, M.M.; Tang, S.W. Effects of Fineness and Content of Phosphorus Slag on Cement Hydration, Permeability, Pore Structure and Fractal Dimension of Concrete. Fractals 2021, 29, 2140004. [Google Scholar] [CrossRef]
- Zheng, J.-J.; Wong, H.; Buenfeld, N.R. Assessing the influence of ITZ on the steady-state chloride diffusivity of concrete using a numerical model. Cem. Concr. Res. 2009, 39, 805–813. [Google Scholar] [CrossRef]
- Lv, X.; Dong, Y.; Wang, R.; Lu, C.; Wang, X. Resistance improvement of cement mortar containing silica fume to external sulfate attacks at normal temperature. Constr. Build. Mater. 2020, 258, 119630. [Google Scholar] [CrossRef]
- Dong, Y. Effects of mineral admixture on the carbonic acid leaching resistance of cement-based materials. Ceram.-Silik. 2017, 61, 276–284. [Google Scholar] [CrossRef][Green Version]
- Awoyera, P.; Akinmusuru, J.; Dawson, A.; Ndambuki, J.; Thom, N. Microstructural characteristics, porosity and strength development in ceramic-laterized concrete. Cem. Concr. Compos. 2018, 86, 224–237. [Google Scholar] [CrossRef]
- Scrivener, K.L.; Crumbie, A.K.; Laugesen, P. The Interfacial Transition Zone (ITZ) Between Cement Paste and Aggregate in Concrete. Interface Sci. 2004, 12, 411–421. [Google Scholar] [CrossRef]
- Xu, P.; Yu, B. Developing a new form of permeability and Kozeny–Carman constant for homogeneous porous media by means of fractal geometry. Adv. Water Resour. 2008, 31, 74–81. [Google Scholar] [CrossRef]
- Katz, A.J.; Thompson, A.H. Quantitative prediction of permeability in porous rock. Phys. Rev. B 1986, 34, 8179–8181. [Google Scholar] [CrossRef] [PubMed]
- Şahmaran, M.; Özbay, E.; Yücel, H.E.; Lachemi, M.; Li, V. Frost resistance and microstructure of Engineered Cementitious Composites: Influence of fly ash and micro polyvinyl alcohol fiber. Cem. Concr. Compos. 2012, 34, 156–165. [Google Scholar] [CrossRef]
- ASTM International. ASTM C666/666M-15. In Standard Test Method for Resistance of Concrete to Rapid freezing and Thawing; ASTM International: West Conshohocken, PA, USA, 2015. [Google Scholar]
- Powers, T.C.; Brownyard, T.L. Studies of the physical properties of hardened Portland cement paste—Part 8. The freezing of water in hardened Portland cement paste. J. Am. Concr. Inst. 1947, 18, 933–969. [Google Scholar]
- Xiao, J.; Qu, W.; Jiang, H.; Li, L.; Huang, J.; Chen, L. Fractal characterization and mechanical behavior of pile-soil interface Subjected to sulfuric acid. Fractals 2021, 29, 2140010. [Google Scholar] [CrossRef]
- Xiao, J.; Long, X.; Qu, W.; Li, L.; Jiang, H.; Zhong, Z. Influence of sulfuric acid corrosion on concrete stress-strain relationship under uniaxial compression. Measurement 2021, 185, 110318. [Google Scholar] [CrossRef]
- Xiao, J.; Long, X.; Li, L.; Jiang, H.; Zhang, Y.; Qu, W. Study on the Influence of Three Factors on Mass Loss and Surface Fractal Dimension of Concrete in Sulfuric Acid Environments. Fractal Fract. 2021, 5, 146. [Google Scholar] [CrossRef]
- Xiao, J.; Qu, W.; Jiang, H.; Dong, W. Three-Dimensional Fractal Characterization of Concrete Surface Subjected to Sulfuric Acid Attacks. J. Nondestruct. Eval. 2020, 39, 57. [Google Scholar] [CrossRef]
- Issa, M.; Hammad, A. Assessment and evaluation of fractal dimension of concrete fracture surface digitized images. Cem. Concr. Res. 1994, 24, 325–334. [Google Scholar] [CrossRef]
- Cai, W.; Cen, G.; Wang, H. Fracture Surface Fractal Characteristics of Alkali-Slag Concrete under Freeze-Thaw Cycles. Adv. Mater. Sci. Eng. 2017, 2017, 168913. [Google Scholar] [CrossRef]
- Ebrahimkhanlou, A.; Athanasiou, A.; Hrynyk, T.; Bayrak, O.; Salamone, S. Fractal and Multifractal Analysis of Crack Patterns in Prestressed Concrete Girders. J. Bridg. Eng. 2019, 24, 04019059. [Google Scholar] [CrossRef]

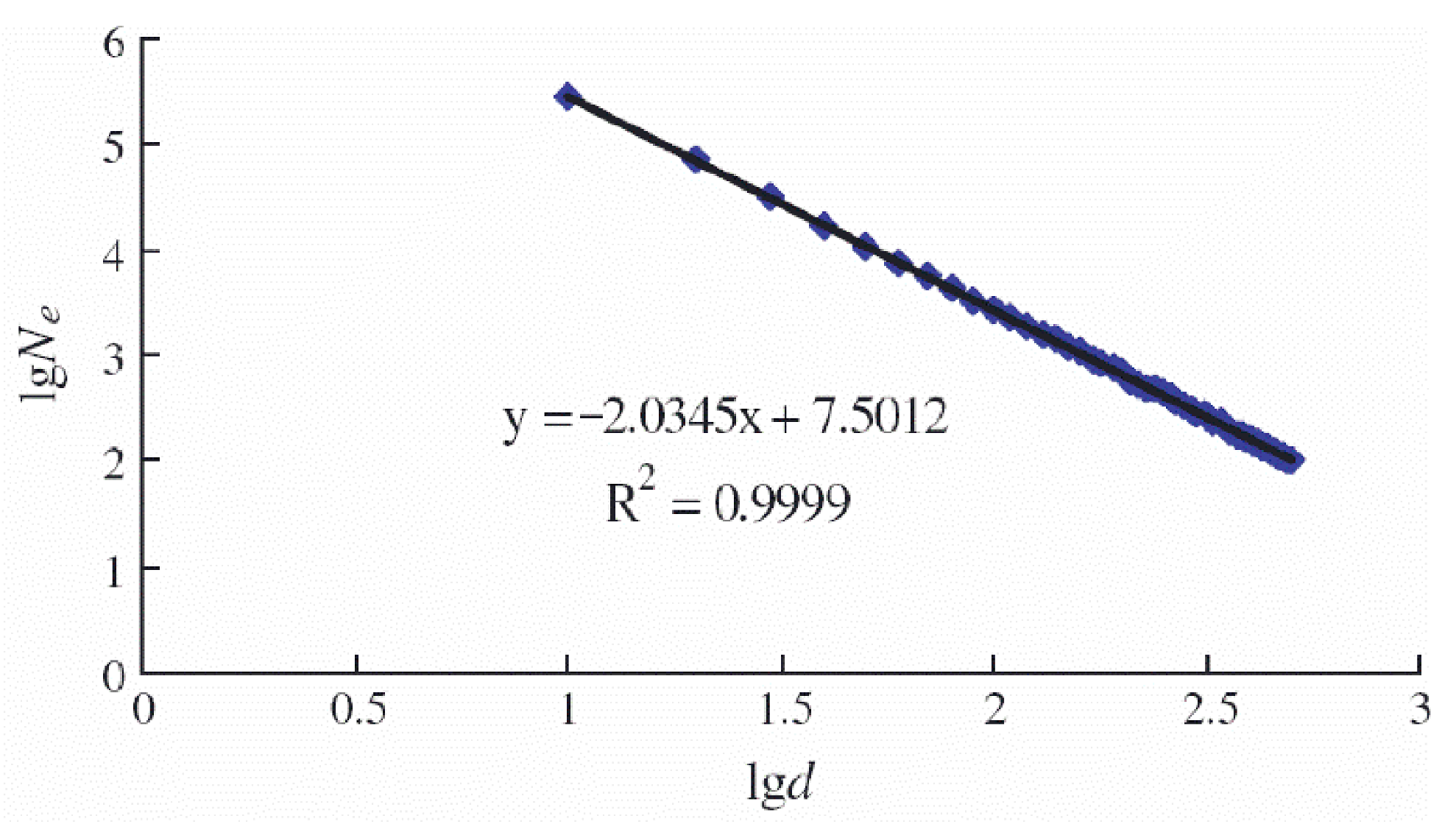

| Fractal Dimension Type | Physical Meaning | Test Methods | Fractal Models | Refs. |
|---|---|---|---|---|
| Fractal dimension of pore surface (Ds) | Pore surface roughness and pore size distribution | Mercury intrusion porosimeter (MIP), nitrogen adsorption/desorption (NAD) method, etc. | Zhang’s model and Neimark’s model, FHH equation, Neimark’s thermodynamic method | [39,40,41,42,43,44,45,46] |
| Fractal dimension of pore volume (Dv) | Pore volume distribution in porous materials | MIP, low-temperature differential scanning calorimetry (LT-DSC), nuclear magnetic resonance (NMR), small-angle scattering of X-rays (SAXS) and neutrons (SANS), etc. | Space-filling model and Menger sponge model | [30,42,47,48,49,50,51,52] |
| Fractal dimension of pore tortuosity (Dt) | Tortuousness of capillary pores | Optical microscope, SEM, backscattered electron (BSE), noncontact impedance measurement (NCIM), etc. | Box-counting method, imaging model, two-phase fractal model | [4,50,53,54,55,56,57] |
| Fractal dimension of air void (Da) | Distribution of air voids | Air-void analyzer | Box-counting method | [15] |
| Fractal dimension of fracture surface (Dfs) | Roughness of the fracture surface | Stereoscopic SEM, SEM, 3D laser scanning technology, image analyzer method, laser profilometer, etc., confocal microscope | Friel’s method, the projective covering method, 2D fast Fourier transform function, the chord method, box-counting method. | [58,59,60,61,62,63] |
| Fractal dimension of crack (Dc) | Distribution of concrete cracks | X-ray computed tomography (X-ray CT), visual observation, tracing drawing technology of plastic sheet, photoelastic coating set-up, optical scanner (or digital camera) combined with machine learning algorithms, etc. | Box-counting method | [26,64,65,66,67,68,69,70,71,72] |
| Fractal dimension of particle distribution (Dp) | Particle size distribution of powders, or particle spatial distribution of particles in fresh cement paste | Laser granulometry, optical microscope, image analysis system, etc. | Yu’s method | [73,74,75] |
| Cement-Based Materials | Fractal Model | Fractal Dimension Type | Mechanical Property | Correlations | Correlation Coefficient R2 | Refs. |
|---|---|---|---|---|---|---|
| Cement mortar | Zhang’s model | Fractal dimension of pore surface (Ds) | Compressive strength | Y * = C·Ds21.573 | 0.7214 | [31] |
| OPC Concrete | Zhang’s model | Ds | Compressive strength | y = 445.366 Ds − 1268.01 | 0.878 | [34] |
| Low-heat cement concrete | Zhang’s model | Ds | Compressive strength | y = 200.66 Ds − 536.1 | 0.924 | [37] |
| GGBFS-based cement pastes | Zhang’s model | Ds | Compressive strength | y = −58.082 Ds + 254.29 (only valid for pores exceeding 100 nm) | 0.493 | [88] |
| Self-compacting concrete containing silica fume | Menger sponge model | Fractal dimension of pore volume (Dv) | Compressive strength | y = 11.037 Dv − 9.0578 | 0.8036 | [33] |
| Cement Pastes at high temperatures | Menger sponge model | Dv | Compressive strength | y = 3.20 − 0.01 Dv | 0.660 | [48] |
| Flexural strength | y = 3.32 − 0.37 Dv | 0.730 | ||||
| Splitting-tensile strength | y = 3.36 − 0.19 Dv | 0.660 | ||||
| Concrete | Box-counting method | Fractal dimension of fracture surface (Dfs) | Compressive strength | y = 176.20 − 52.05 Dfs | 0.940 | [9] |
| Cement paste | Box-counting method | Dfs | Compressive strength | A positive exponential relation | 0.999 | [63] |
| Cement-Based Materials | Fractal Model | Fractal Dimension Type | Transport Properties | Correlations | Correlation Coefficient R2 | Refs. |
|---|---|---|---|---|---|---|
| Porous medium | Box-counting method | Fractal dimension of pore tortuosity (Dt) | Permeability | A negative relation | - | [50] |
| Cement paste | Two-phase fractal model | Dt | K | A negative relation | - | [56] |
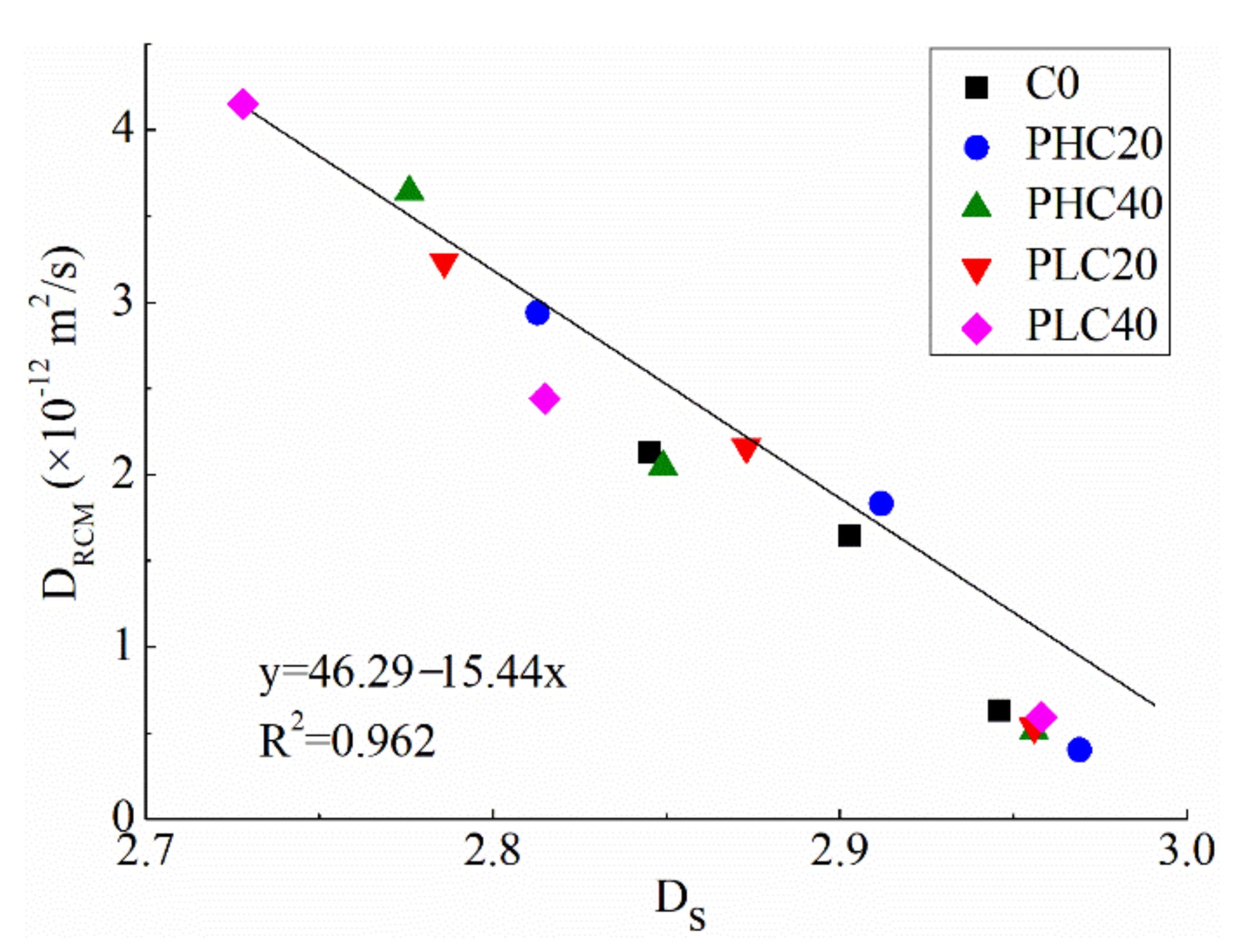
| Concrete containing phosphorus slag | Zhang’s model | The fractal dimension of pore surface (Ds) | DRCM | y * = −15.44 Ds + 46.29 | 0.962 | [123] |
| Self-compacting concrete containing silica fume | Menger sponge model | The fractal dimension of pore volume (Dv) | Water penetration depth | y = 2.924 Dv − 4.164 | 0.706 | [33] |
| Concrete containing silica fume | Box-counting method | Fractal dimension of fracture surface (Dfs) | K | A positive exponential function | - | [9] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Zeng, X.; Yang, H.; Lv, X.; Guo, F.; Shi, Y.; Hanif, A. Investigation and Application of Fractal Theory in Cement-Based Materials: A Review. Fractal Fract. 2021, 5, 247. https://doi.org/10.3390/fractalfract5040247
Wang L, Zeng X, Yang H, Lv X, Guo F, Shi Y, Hanif A. Investigation and Application of Fractal Theory in Cement-Based Materials: A Review. Fractal and Fractional. 2021; 5(4):247. https://doi.org/10.3390/fractalfract5040247
Chicago/Turabian StyleWang, Lei, Xiaoman Zeng, Huamei Yang, Xingdong Lv, Fanxing Guo, Yan Shi, and Asad Hanif. 2021. "Investigation and Application of Fractal Theory in Cement-Based Materials: A Review" Fractal and Fractional 5, no. 4: 247. https://doi.org/10.3390/fractalfract5040247
APA StyleWang, L., Zeng, X., Yang, H., Lv, X., Guo, F., Shi, Y., & Hanif, A. (2021). Investigation and Application of Fractal Theory in Cement-Based Materials: A Review. Fractal and Fractional, 5(4), 247. https://doi.org/10.3390/fractalfract5040247








