Effect of Grain Size on Microscopic Pore Structure and Fractal Characteristics of Carbonate-Based Sand and Silicate-Based Sand
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Test Equipment and Specimen Preparation
2.3. Calculation Method for Determining Pore Size Distribution Using NMR
2.4. Calculation Method of Fractal Dimension Using Fractal Theory
3. Results and Discussion
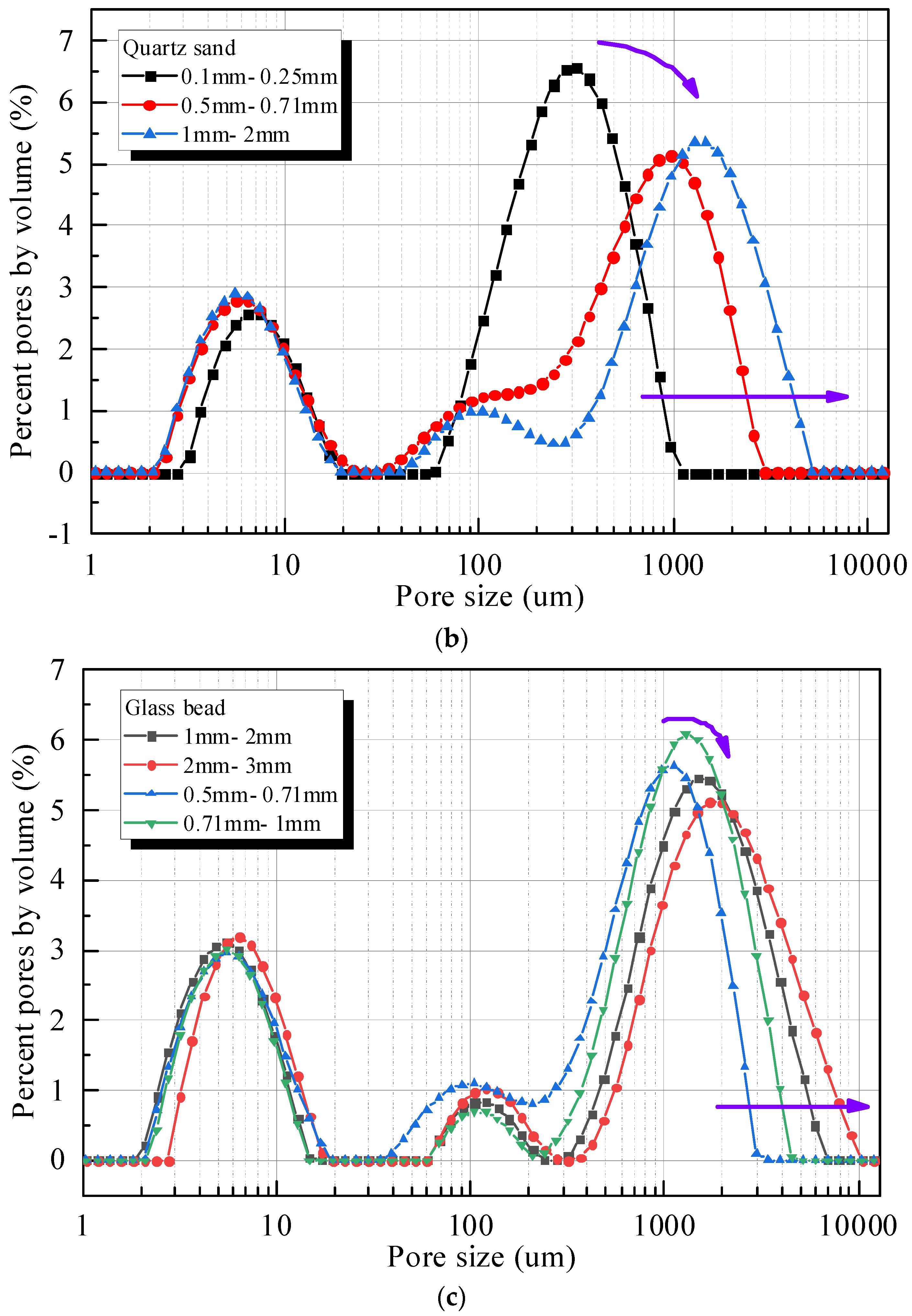
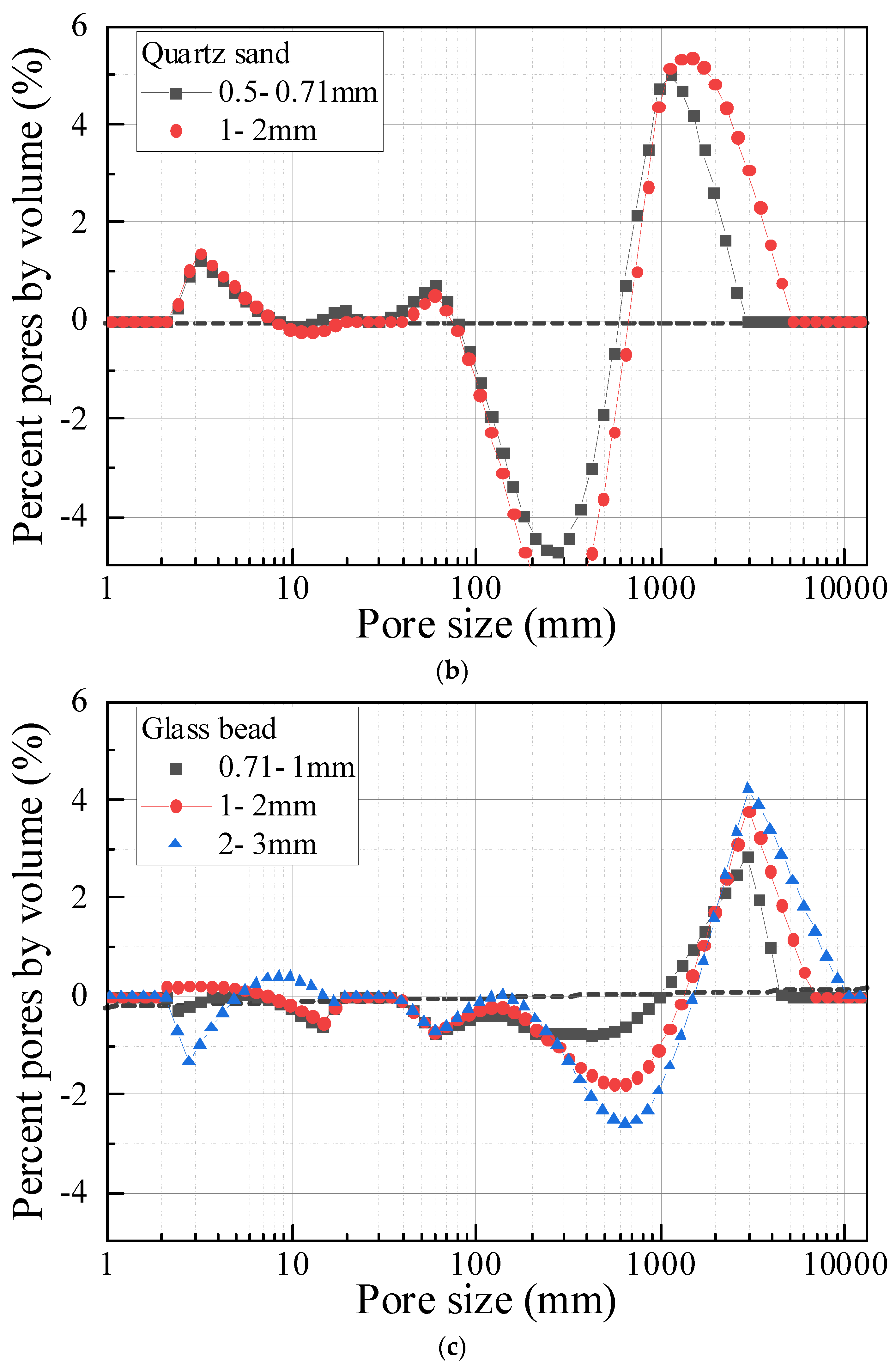
3.1. Effect of Grain Size on the Pore Size Distribution
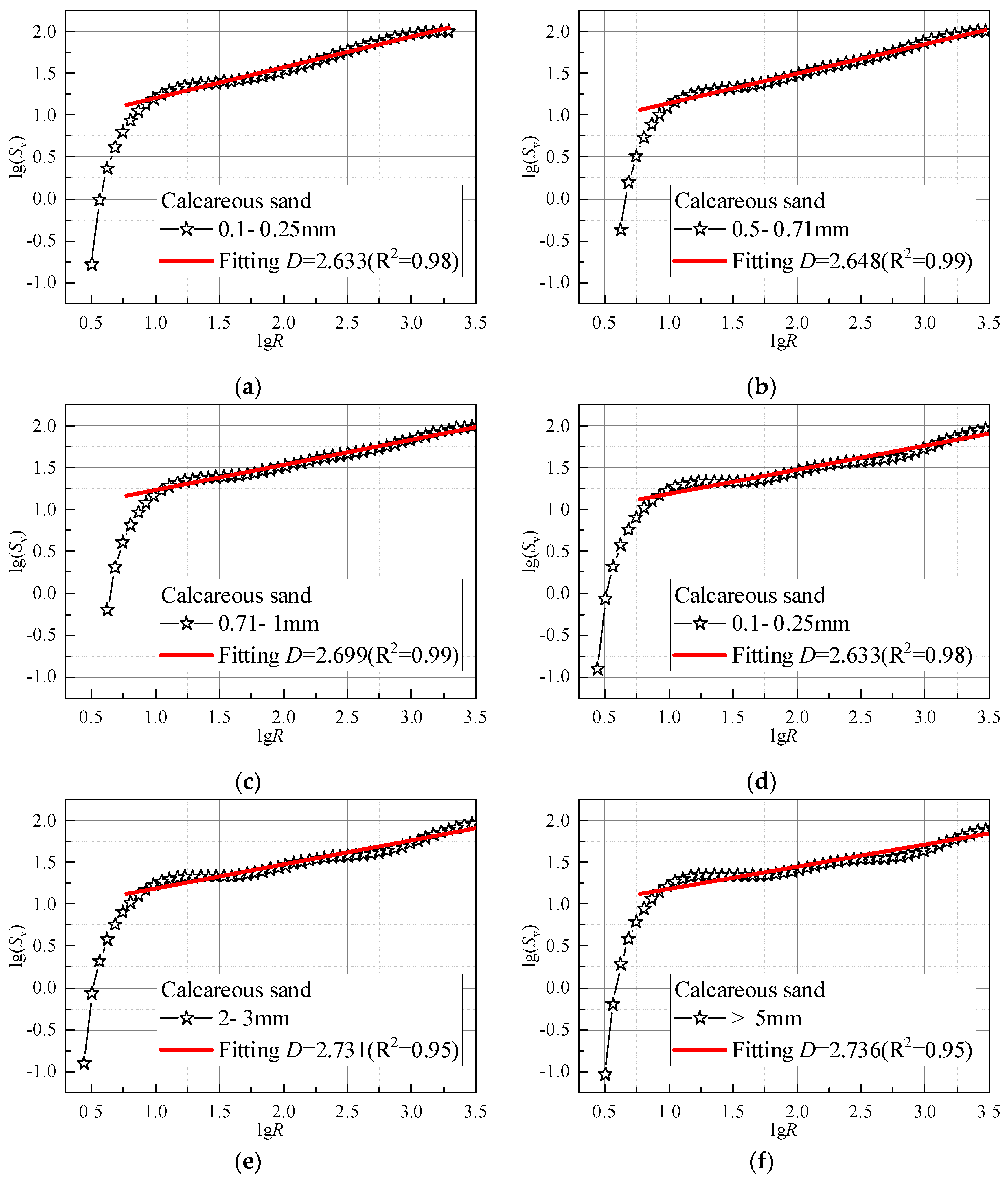
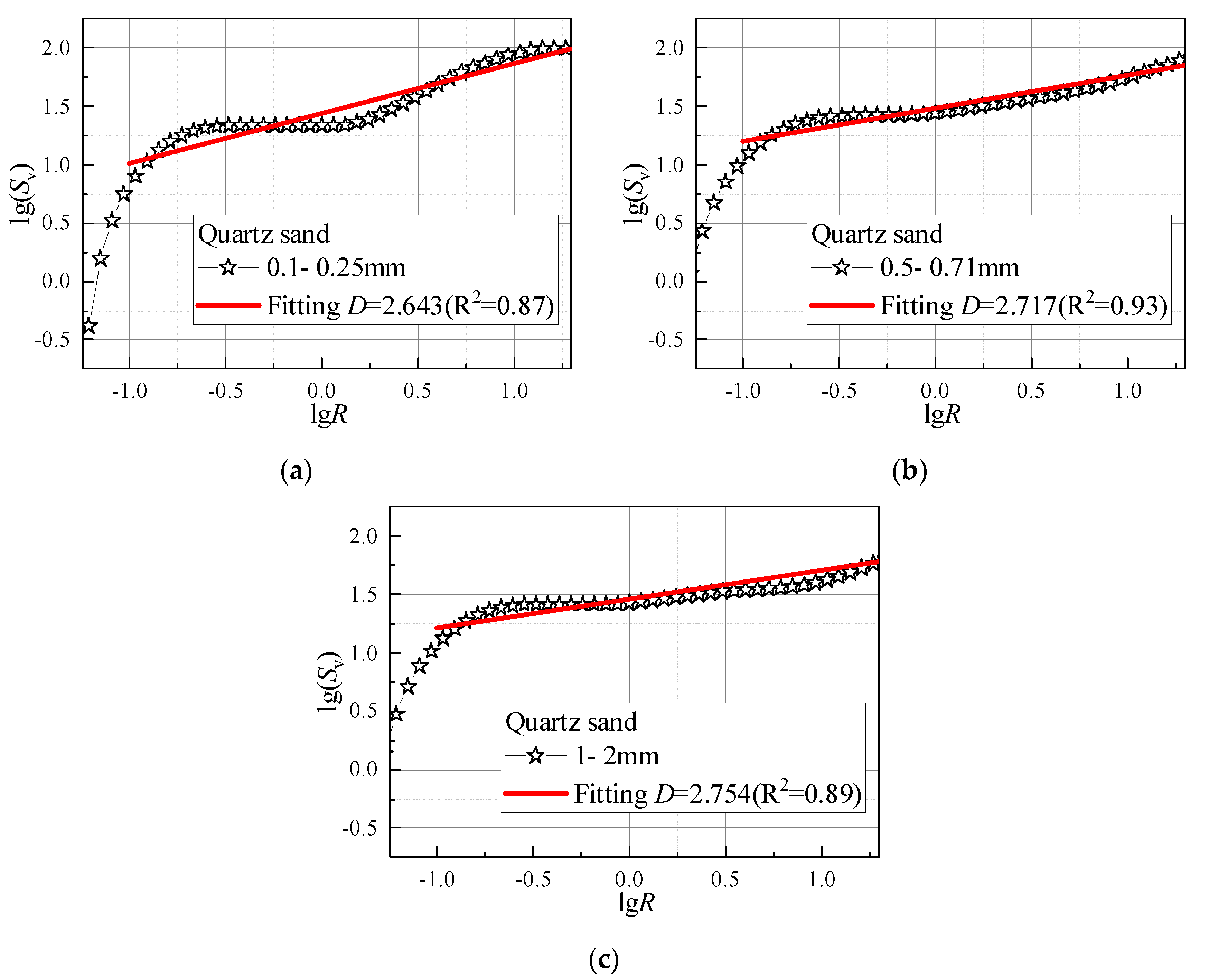
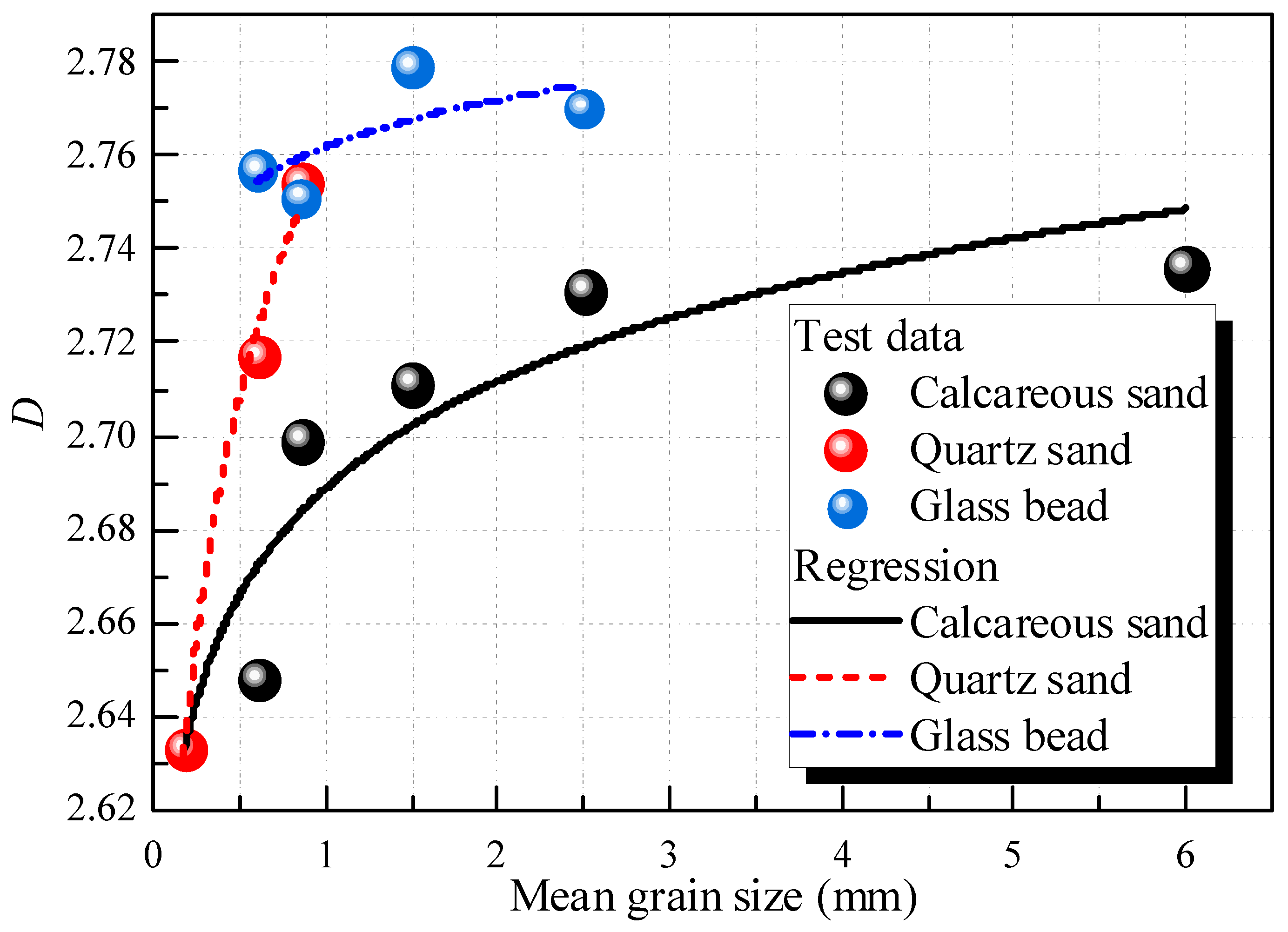
3.2. Effect of Grain Size on the Microsrcture’s Fractal Dimension
4. Conclusions
- (1)
- Grain size had a significant effect on the pore size distribution of carbonate-based sand and silicate-based sand. As the grain size increased, the pore size distribution curve of sands gradually evolved from a binary structure with two peaks to a ternary structure with three peaks. The increase in the grain size could cause a remarkable increase in the maximum pore size. Moreover, the increase in grain size increased the proportion of large pores with a size larger than 1000 μm, and correspondingly reduced the proportion of medium pores with a size between 100 μm–1000 μm. However, grain size had a minor effect on small pores with a size less than 100 um.
- (2)
- Carbonate-based sand differed significantly from silicate-based sand with respect to the pore size distribution, owing to the significant divergence in particle shapes. In the pore size range between 100 μm and 1000 μm, carbonate-based sand had a continuous pore size distribution, whereas the pore size distribution of silicate-based sand was discontinuous. This indicated that the more irregular the particle shape, the better the continuity between the large and medium pores.
- (3)
- The pore size distribution of calcareous sand, quartz sand and glass beads all exhibited good fractal characteristics. The fractal dimension (D) of the pore size distribution of the three materials was determined by fractal theory. Compared with the quartz sand and glass beads, the calcareous sand with the most complex particle shape had the most significant fractal characteristics. Moreover, grain size had a considerable effect on the fractal dimension of the carbonate-based sand and the silicate-based sand. The increase of grain size could lead to a significant increase of D for calcareous sand, quartz sand and glass bead, indicating that the increase of grain size would increase the heterogeneity of pore structure distribution in sands. A refined correlation was proposed to determine D for carbonate-based sand and silicate-based sand with different grain sizes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ding, Z.; He, S.-H.; Sun, Y.; Xia, T.-D.; Zhang, Q.-F. Comparative study on cyclic behavior of marine calcareous sand and terrigenous siliceous sand for transportation infrastructure applications. Constr. Build. Mater. 2021, 283, 122740. [Google Scholar] [CrossRef]
- He, S.H.; Ding, Z.; Xia, T.D.; Zhou, W.H.; Gan, X.L.; Chen, Y.Z.; Xia, F. Long-term behaviour and degradation of calcareous sand under cyclic loading. Eng. Geol. 2020, 276, 105756. [Google Scholar] [CrossRef]
- Peng, Y.; Ding, X.; Xiao, Y.; Deng, X.; Deng, W. Detailed amount of particle breakage in non-uniformly graded sands under one-dimensional compression. Can. Geotech. J. 2019, 57, 1239–1246. [Google Scholar] [CrossRef]
- Rui, S.; Wang, L.; Guo, Z.; Cheng, X.; Wu, B. Monotonic behavior of interface shear between carbonate sands and steel. Acta Geotech. 2021, 16, 167–187. [Google Scholar] [CrossRef]
- Shahnazari, H.; Rezvani, R. Effective parameters for the particle breakage of calcareous sands: An experimental study. Eng. Geol. 2013, 159, 98–105. [Google Scholar] [CrossRef]
- Sharma, S.S.; Ismail, M.A. Monotonic and Cyclic Behavior of Two Calcareous Soils of Different Origins. J. Geotech. Geoenviron. 2006, 132, 1581–1591. [Google Scholar] [CrossRef]
- Wang, G.; Wang, Z.; Ye, Q.; Wei, X. Particle Breakage and Deformation Behavior of Carbonate Sand under Drained and Undrained Triaxial Compression. Int. J. Geomech. 2020, 20, 04020012. [Google Scholar] [CrossRef]
- Wang, G.; Wang, Z.; Ye, Q.; Zha, J. Particle breakage evolution of coral sand using triaxial compression tests. J. Rock Mech. Geotech. Eng. 2021, 13, 321–334. [Google Scholar] [CrossRef]
- Wang, H.; Sun, P.; Liu, E.; Li, R. Dynamic properties of Tianshui saturated remolded loess: A laboratory study. Eng. Geol. 2020, 272, 105570. [Google Scholar] [CrossRef]
- Wang, J.; Li, P.; Ma, Y.; Vanapalli, S. Evolution of pore-size distribution of intact loess and remolded loess due to consolidation. J. Soils Sediments 2019, 19, 1226–1238. [Google Scholar] [CrossRef]
- Wang, J.-D.; Li, P.; Ma, Y.; Vanapalli, S.; Wang, X. Change in pore-size distribution of collapsible loess due to loading and inundating. Acta Geotech. 2020, 15, 1081–1094. [Google Scholar] [CrossRef]
- Wang, X.Z.; Weng, Y.L.; Wei, H.Z.; Meng, Q.S.; Hu, M.J. Particle obstruction and crushing of dredged calcareous soil in the Nansha Islands, South China Sea. Eng. Geol. 2019, 261, 105274. [Google Scholar] [CrossRef]
- Wu, Y.; Li, N.; Wang, X.Z.; Cui, J.; Chen, Y.L.; Wu, Y.H.; Yamamoto, H. Experimental investigation on mechanical behavior and particle crushing of calcareous sand retrieved from South China Sea. Eng. Geol. 2021, 280, 105932. [Google Scholar] [CrossRef]
- Cao, Z.; Chen, J.; Ye, X.; Gu, C.; Guo, Z.; Cai, Y. Experimental study on particle breakage of carbonate gravels under cyclic loadings through large-scale triaxial tests. Transp. Geotech. 2021, 30, 100632. [Google Scholar] [CrossRef]
- Donohue, S.; O’sullivan, C.; Long, M. Particle breakage during cyclic triaxial loading of a carbonate sand. Géotechnique 2009, 59, 477–482. [Google Scholar] [CrossRef]
- Gao, Q.-F.; Jrad, M.; Hattab, M.; Fleureau, J.-M.; Ighil Ameur, L. Pore Morphology, Porosity, and Pore Size Distribution in Kaolinitic Remolded Clays under Triaxial Loading. Int. J. Geomech. 2020, 20, 04020057. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, H.; Huang, M.; Yin, H.; Jiang, K.; Xiao, K.; Tang, S. Influence of Different Alkali Sulfates on the Shrinkage, Hydration, Pore Structure, Fractal Dimension and Microstructure of Low-Heat Portland Cement, Medium-Heat Portland Cement and Ordinary Portland Cement. Fractal Fract. 2021, 5, 79. [Google Scholar] [CrossRef]
- Fujikura, Y. Estimation of Permeability for Sand and Gravel Based on Pore-Size Distribution Model. J. Mater. Civ. Eng. 2019, 31, 04019289. [Google Scholar] [CrossRef]
- Gao, Q.-F.; Zhao, D.; Zeng, L.; Dong, H. A Pore Size Distribution-based Microscopic Model for Evaluating the Permeability of Clay. KSCE J. Civ. Eng. 2019, 23, 5002–5011. [Google Scholar] [CrossRef]
- Romero, E.; Gens, A.; Lloret, A. Water permeability, water retention and microstructure of unsaturated compacted Boom clay. Eng. Geol. 1999, 54, 117–127. [Google Scholar] [CrossRef]
- Tang, S.; Wang, Y.; Geng, Z.; Xu, X.; Yu, W.; Hubao, A.; Chen, J. Structure, Fractality, Mechanics and Durability of Calcium Silicate Hydrates. Fractal Fract. 2021, 5, 47. [Google Scholar] [CrossRef]
- Ullah, A.S.; D’Addona, D.M.; Seto, Y.; Yonehara, S.; Kubo, A. Utilizing Fractals for Modeling and 3D Printing of Porous Structures. Fractal Fract. 2021, 5, 40. [Google Scholar] [CrossRef]
- Feng, S.; Xu, Z.; Chai, J.; Li, Y. Using pore size distribution and porosity to estimate particle size distribution by nuclear magnetic resonance. Soils Found. 2020, 60, 1011–1019. [Google Scholar] [CrossRef]
- Griffiths, F.; Joshi, R. Discussion: Change in pore size distribution due to consolidation of clays. Geotechnique 1990, 40, 303–309. [Google Scholar] [CrossRef]
- Kong, B.; Xia, F.; Yu, B.; Xia, T.; Ding, Z. Pore Size Changes in Marine Soft Soil under Various Freezing Conditions. J. Mar. Sci. Eng. 2020, 8, 170. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Zhang, L. Characterization of dual-structure pore-size distribution of soil. Can. Geotech. J. 2009, 46, 129–141. [Google Scholar] [CrossRef] [Green Version]
- Mukunoki, T.; Miyata, Y.; Mikami, K.; Shiota, E. X-ray CT analysis of pore structure in sand. Solid Earth 2016, 7, 929–942. [Google Scholar] [CrossRef] [Green Version]
- Cai, Y.Q.; Hao, B.B.; Gu, C.; Wang, J.; Pan, L.Y. Effect of anisotropic consolidation stress paths on the undrained shear behavior of reconstituted Wenzhou clay. Eng. Geol. 2018, 242, 23–33. [Google Scholar] [CrossRef]
- Tang, l.; Song, Y.; Jiang, Z.; Jiang, S.; Li, Q. Pore Structure and Fractal Characteristics of Distinct Thermally Mature Shales. Energy Fuels 2019, 33, 5116–5128. [Google Scholar] [CrossRef]
- Yao, Y.; Liu, D.; Tang, D.; Tang, S.; Huang, W. Fractal characterization of adsorption-pores of coals from North China: An investigation on CH4 adsorption capacity of coals. Int. J. Coal Geol. 2008, 73, 27–42. [Google Scholar] [CrossRef]
- Sun, Y.; Gao, Y.; Zhu, Q. Fractional Order Plasticity Modelling of State-Dependent Behaviour of Granular Soils without Using Plastic Potential. Int. J. Plast. 2018, 102, 53–69. [Google Scholar] [CrossRef]
- Sun, Y.; Sumelka, W. Multiaxial stress-fractional plasticity model for anisotropically overconsolidated clay. Int. J. Mech. Sci. 2021, 205, 106598. [Google Scholar] [CrossRef]
- Shao, X.; Pang, X.; Li, H.; Zhang, X. Fractal Analysis of Pore Network in Tight Gas Sandstones Using NMR Method: A Case Study from the Ordos Basin, China. Energy Fuels 2017, 31, 10358–10368. [Google Scholar] [CrossRef]
- Sun, W.; Zuo, Y.; Zhonghu, W.; Liu, H.; Xi, S.; Shui, Y.; Wang, J.; Liu, R.; Lin, J. Fractal analysis of pores and the pore structure of the Lower Cambrian Niutitang shale in northern Guizhou province: Investigations using NMR, SEM and image analyses. Mar. Pet. Geol. 2018, 99, 416–428. [Google Scholar] [CrossRef]
- Li, A.; Ding, W.; Jiu, K.; Wang, Z.; Wang, R.; He, J. Investigation of the pore structures and fractal characteristics of marine shale reservoirs using NMR experiments and image analyses: A case study of the Lower Cambrian Niutitang Formation in northern Guizhou Province, South China. Mar. Pet. Geol. 2017, 89, 530–540. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, N.; Li, D.; Liu, H.; Cai, Y.; Wu, Y. Effects of Topographic Amplification Induced by a U-Shaped Canyon on Seismic Waves. Bull. Seismol. Soc. Am. 2012, 102, 1748–1763. [Google Scholar] [CrossRef]
- Gao, Y.; Wu, Y.; Li, D.; Liu, H.; Zhang, N. An improved approximation for the spectral representation method in the simulation of spatially varying ground motions. Probabilistic Eng. Mech. 2012, 29, 7–15. [Google Scholar] [CrossRef]
- Zhang, N.; Gao, Y.; Cai, Y.; Li, D.; Wu, Y. Scattering of SH waves induced by a non-symmetrical V-shaped canyon. Geophys. J. Int. 2012, 191, 243–256. [Google Scholar] [CrossRef] [Green Version]
- Jafarian, Y.; Javdanian, H. Small-strain dynamic properties of siliceous-carbonate sand under stress anisotropy. Soil Dyn. Earthq. Eng. 2020, 131, 106045. [Google Scholar] [CrossRef]
- Tian, H.; Wei, C.; Wei, H.; Yan, R.; Chen, P. An NMR-Based Analysis of Soil–Water Characteristics. Appl. Magn. Reson. 2013, 45, 49–61. [Google Scholar] [CrossRef]
- Zhou, S.; Liu, D.; Cai, Y.; Yao, Y. Fractal characterization of pore–fracture in low-rank coals using a low-field NMR relaxation method. Fuel 2016, 181, 218–226. [Google Scholar] [CrossRef]
- Turcotte, D.L. Fractals and fragmentation. J. Geophys. Res. Atmos. 1986, 91, 1921–1926. [Google Scholar] [CrossRef]
- Tyler, S.; Wheatcraft, S. Fractal Scaling of Soil Particle-Size Distributions: Analysis and Limitations. Soil Sci. Soc. Am. J. 1992, 56, 362–369. [Google Scholar] [CrossRef]
- Liang, Z.K.; Li, Z.; Jiang, Z.X.; Gao, F.L.; Zhang, Y.H.; Xiao, L.; Yang, Y.D.; Hou, Y.F.; Wang, L.W. Characteristics of pore structure and fractal dimension in continental shale based on NMR experiments and SEM image analyses—A case study of Shahezi formation shale in Changling fault depression of Songliao Basin, China. J. Earth Sci. Environ. 2020, 42, 313–328. [Google Scholar]
- Huang, Y.; Zhang, P.; Zhang, J.; Tang, X.; Liu, C.; Yang, J. Fractal Characteristics of Pores in the Longtan Shales of Guizhou, Southwest China. Geofluids 2020, 2020, 8834758. [Google Scholar] [CrossRef]
- Dou, W.; Liu, L.; Jia, L.; Xu, Z.; Wang, M.; Du, C. Pore structure, fractal characteristics and permeability prediction of tight sandstones: A case study from Yanchang Formation, Ordos Basin, China. Mar. Pet. Geol. 2021, 123, 104737. [Google Scholar] [CrossRef]
- Kong, L.; Ostadhassan, M.; Liu, B.; Li, C.; Liu, K. Multifractal Characteristics of MIP-Based Pore Size Distribution of 3D-Printed Powder-Based Rocks: A Study of Post-Processing Effect. Transp. Porous Media 2019, 129, 599–618. [Google Scholar] [CrossRef]


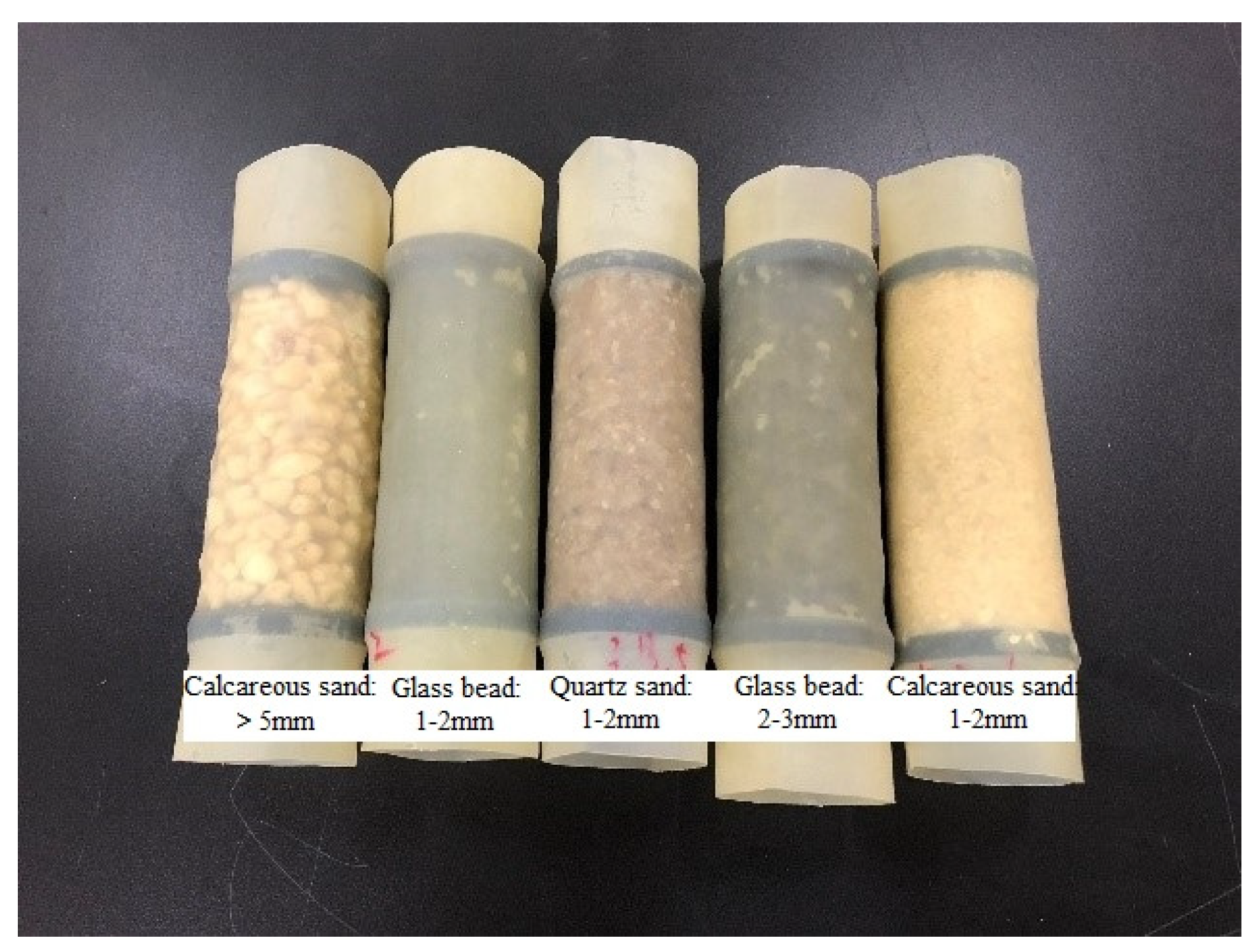



| No. | Material | Grain Size (mm) | Void Ratio |
|---|---|---|---|
| C-D-1 | Calcareous sand | 0.1–0.25 | 1.143 |
| C-D-2 | 0.5–0.71 | 1.142 | |
| C-D-3 | 0.71–1 | 1.146 | |
| C-D-4 | 1–2 | 1.145 | |
| C-D-5 | 2–3 | 1.144 | |
| C-D-6 | >5 | 1.143 | |
| S-D-1 | Quartz sand | 0.1–0.25 | 0.507 |
| S-D-2 | 0.5–0.71 | 0.508 | |
| S-D-3 | 1–2 | 0.509 | |
| G-D-1 | Glass sand | 0.5–0.71 | 0.631 |
| G-D-2 | 0.71–1.0 | 0.630 | |
| G-D-3 | 1–2 | 0.632 | |
| G-D-4 | 2–3 | 0.633 |
| Parameter | Calcareous Sand | Quartz Sand | Glass Bead |
|---|---|---|---|
| α | 2.689 | 2.761 | 2.762 |
| β | 0.0122 | 0.0276 | 0.00524 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, S.-H.; Ding, Z.; Hu, H.-B.; Gao, M. Effect of Grain Size on Microscopic Pore Structure and Fractal Characteristics of Carbonate-Based Sand and Silicate-Based Sand. Fractal Fract. 2021, 5, 152. https://doi.org/10.3390/fractalfract5040152
He S-H, Ding Z, Hu H-B, Gao M. Effect of Grain Size on Microscopic Pore Structure and Fractal Characteristics of Carbonate-Based Sand and Silicate-Based Sand. Fractal and Fractional. 2021; 5(4):152. https://doi.org/10.3390/fractalfract5040152
Chicago/Turabian StyleHe, Shao-Heng, Zhi Ding, Hai-Bo Hu, and Min Gao. 2021. "Effect of Grain Size on Microscopic Pore Structure and Fractal Characteristics of Carbonate-Based Sand and Silicate-Based Sand" Fractal and Fractional 5, no. 4: 152. https://doi.org/10.3390/fractalfract5040152
APA StyleHe, S.-H., Ding, Z., Hu, H.-B., & Gao, M. (2021). Effect of Grain Size on Microscopic Pore Structure and Fractal Characteristics of Carbonate-Based Sand and Silicate-Based Sand. Fractal and Fractional, 5(4), 152. https://doi.org/10.3390/fractalfract5040152






