Novel Computations of the Time-Fractional Fisher’s Model via Generalized Fractional Integral Operators by Means of the Elzaki Transform
Abstract
:1. Introduction
2. Preliminaries
3. Application of Caputo-Liouville and ABC Fractional Derivatives to the Non-Linear Fisher’s Model
3.1. Description of IETM
3.2. Stability Analysis
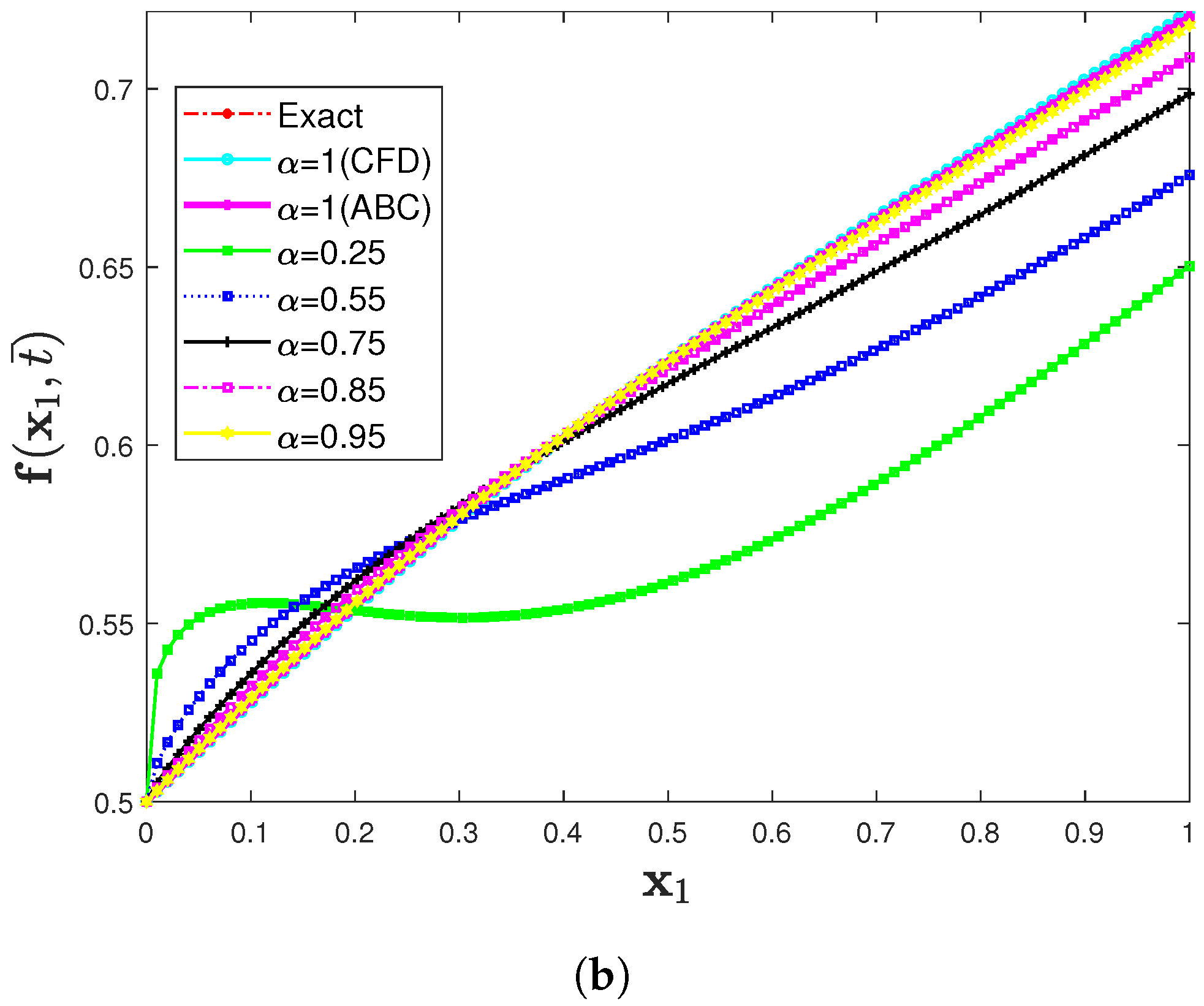
4. Evaluation of the Fractional Fisher Model via IETM
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 73, 1–13. [Google Scholar]
- Atangana, A.; Baleanu, D. New fractional derivatives with non-local and non-singular kernel. Theory and Application to Heat Transfer Model. arXiv 2016, arXiv:1602.03408. [Google Scholar]
- Scherer, R.; Kalla, S.L.; Tang, Y.; Huanget, J. The Grünwald-Letnikov method for fractional differential equations. Comput. Math. Appl. 2011, 62, 902–917. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Qian, D.; Chen, Y.Q. On Riemann-Liouville and Caputo Derivatives. Discret. Dyn. Nat. Soc. 2011, 2011, 562494. [Google Scholar] [CrossRef] [Green Version]
- Morales-Delgado, V.F.; Gómez-Aguilar, J.F.; Yepez-Martínez, H.; Baleanu, D.; Escobar-Jimenez, R.F.; Olivares-Peregrino, V.H. Laplace homotopy analysis method for solving linear partial differential equations using a fractional derivative with and without kernel singular. Adv. Differ. Equ. 2016, 2016, 164. [Google Scholar] [CrossRef] [Green Version]
- Sheikh, N.A.; Ali, F.; Saqib, M.; Khan, I.; Jan, S.A.A.; Alshomrani, A.S.; Alghamdi, M.S. Comparison and analysis of the Atangana-Baleanu and Caputo-Fabrizio fractional derivatives for generalized Casson fluid model with heat generation and chemical reaction. Results Phys. 2017, 7, 789. [Google Scholar] [CrossRef]
- Atangana, A.; Alkahtani, B.S.T. New model of groundwater flowing within a confine aquifer: Application of Caputo-Fabrizio derivative. Arab. J. Geo-Sci. 2016, 9, 8. [Google Scholar] [CrossRef]
- Kumar, D.; Singh, J.; Baleanu, D. Analysis of regularized long-wave equation associated with a new fractional operator with Mittag-Leffler type kernel. Physica A 2018, 492, 155–167. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Baleanu, D. On the analysis of chemical kinetics system pertaining to a fractional derivative with Mittag-Leffler type kernel. Chaos 2017, 27, 103113. [Google Scholar] [CrossRef]
- Baleanu, D.; Jajarmi, A.; Hajipour, M. A new formulation of the fractional optimal control problems involving Mittag-Leffler nonsingular kernel. J. Optim. Theory Appl. 2017, 175, 718–737. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Gorguis, A. An analytic study of Fisher’s equation by using Adomian decomposition method. Appl. Math. Comput. 2004, 154, 609–620. [Google Scholar] [CrossRef]
- Rashid, S.; Khalid, A.; Sultana, S.; Hammouch, Z.; Shah, R.; Alsharif, A.M. A novel analytical view of time-fractional Korteweg-De Vries equations via a new integral transform. Symmetry 2021, 13, 1254. [Google Scholar] [CrossRef]
- Rashid, S.; Kubra, K.T.; Rauf, A.; Chu, Y.-M.; Hamed, Y.S. New numerical approach for time-fractional partial differential equations arising in physical system involving natural decomposition method. Phys. Scr. 2021, 96, 105204. [Google Scholar] [CrossRef]
- Abedle-Rady, A.S.; Rida, S.Z.; Arafa, A.A.M.; Adedl-Rahim, H.R. Approximate analytical solutions of the fractional nonlinear dispersive equations using homotopy perturbation Sumudu transform method. Int. J. Innov. Sci. Eng. Technol. 2014, 1, 257–267. [Google Scholar]
- Rashid, S.; Kubra, K.T.; Lehre, S.U. Fractional spatial diffusion of a biological population model via a new integral transform in the settings of power and Mittag-Leffler nonsingular kernel. Phys. Scr. A 2021, 96, 114003. [Google Scholar] [CrossRef]
- Jafari, H.; Nazari, M.; Baleanu, D.; Khalique, C.M. A new approach for solving a system of fractional partial differential equations. Comput. Math. Appl. 2013, 66, 838–843. [Google Scholar] [CrossRef]
- Veeresha, P.; Prakasha, D.G.; Baskonus, H.M. Novel simulations to the time-fractional Fisher’s equation. Math. Sci. 2019, 13, 33–42. [Google Scholar] [CrossRef]
- Gupta, A.K.; Ray, S.S. On the solutions of fractional Burgers-Fisher and generalized Fisher’s equations using two reliable methods. Int. J. Math. Math. Sci. 2014, 2014, 682910. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Zhang, J. Lie symmetry analysis and exact solutions of generalized fractional Zakharov-Kuznetsov equations. Symmetry 2019, 11, 601. [Google Scholar] [CrossRef] [Green Version]
- Khader, M.M.; Saad, K.M. A numerical approach for solving the fractional Fisher equation using Chebyshev spectral collocation method. Chaos Solitons Fract. 2018, 110, 169–177. [Google Scholar] [CrossRef]
- Rossa, J.; Villaverdeb, A.F.; Bangab, J.R.; Vazquezc, S.; Moranc, F. A generalized Fisher equation and its utility in chemical kinetics. Proc. Natl. Acad. Sci. USA 2010, 107, 12777–12781. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ammerman, A.J.; Cavalli-Sforza, L.L. The Neolithic Transition and the Genetics of Population in Europe; Princeton University Press: Princeton, NJ, USA, 1984. [Google Scholar]
- Merdan, M. Solutions of time-fractional reaction-diffusion equation with modified Riemann-Liouville derivative. Int. J. Phys. Sci. 2012, 7, 2317–2326. [Google Scholar] [CrossRef]
- Kerke, V.M. Results from variants of the Fisher equation in the study of epidemics and bacteria. Physica A 2004, 342, 242–248. [Google Scholar]
- Dag, I.; Sahin, A.; Korkmaz, A. Numerical investigation of the solution of Fisher’s equation via the B-spline galerkin method. Numer. Meth. Partial Differ. Equ. 2010, 26, 1483–1503. [Google Scholar] [CrossRef]
- Bastani, M.; Salkuyeh, D.K. A highly accurate method to solve Fisher’s equation. Pramana 2012, 78, 335–346. [Google Scholar] [CrossRef]
- Gazdag, J.; Canosa, J. Numerical solution of Fisher’s equation. J. Appl. Probab. 1974, 11, 445–457. [Google Scholar] [CrossRef]
- Zhao, T.; Li, C.; Zang, Z.; Wu, Y. Chebyshev-Legendre pseudo-spectral method for the generalised Burgers-Fisher equation. Appl. Math. Model. 2012, 36, 1046–1056. [Google Scholar] [CrossRef]
- Elzaki, T.M. The new integral transform Elzaki transform. Glob. J. Pure Appl. Math. 2011, 7, 57–64. [Google Scholar]
- Daftardar-Gejji, V.; Jafari, H. An iterative method for solving nonlinear functional equations. J. Math. Anal. Appl. 2006, 316, 753–763. [Google Scholar] [CrossRef] [Green Version]
- Belgacem, F.B.M.; Karaballi, A.A.; Kalla, S.L. Analytical investigations of the sumudu transform and applications to integral production equations. Math. Probab. Eng. 2003, 2003, 103–118. [Google Scholar] [CrossRef] [Green Version]
- Aboodh, K.S. The new integral transform “Aboodh Transform”. Glob. J. Pure Appl. Math. 2013, 9, 35–43. [Google Scholar]
- Elzaki, T.M. Application of new transform Elzaki transform to partial differential equations. Glob. J. Pure Appl. Math. 2011, 7, 65–70. [Google Scholar]
- Mahgoub, M.M.A. The new integral transform “Mohand Transform”. Adv. Theor. Appl. Math. 2017, 12, 113–120. [Google Scholar]
- Alderremy, A.A.; Elzaki, T.M.; Chamekh, M. New transform iterative method for solving some Klein-Gordon equations. Results Phys. 2018, 10, 655–659. [Google Scholar] [CrossRef]
- Sedeeg, A.H. A coupling Elzaki transform and homotopy perturbation method for solving nonlinear fractional heat-like equations. Am. J. Math. Comput. Model 2016, 1, 15–20. [Google Scholar]
- Yavuz, M.; Abdeljawad, T. Nonlinear regularized long-wave models with a new integral transformation applied to the fractional derivative with power and Mittag-Leffler kernel. Adv. Differ. Equ. 2020, 2020, 367. [Google Scholar] [CrossRef]
- Odibat, Z.M.; Momani, S. Application of variational iteration method to nonlinear differential equation of fractional order. Int. J. Nonlinear Sci. Numer. Simul. 2006, 7, 27–34. [Google Scholar] [CrossRef]
- Agrseven, D.; Öziş, T. An analytical study for Fisher type equations by using homotopy perturbation method. Comput. Math. Appl. 2010, 60, 602–609. [Google Scholar]
- Jone, D.S.; Sleeman, B.D. Differential Equations and Mathematical Biology; Chapman & Hall/CRC: New York, NY, USA, 2003. [Google Scholar]
- Kawahara, T.; Tanaka, M. Interactions of traveling fronts: An exact solution of a nonlinear diffusion equation. Phys. Lett. A 1983, 97, 311–314. [Google Scholar] [CrossRef]
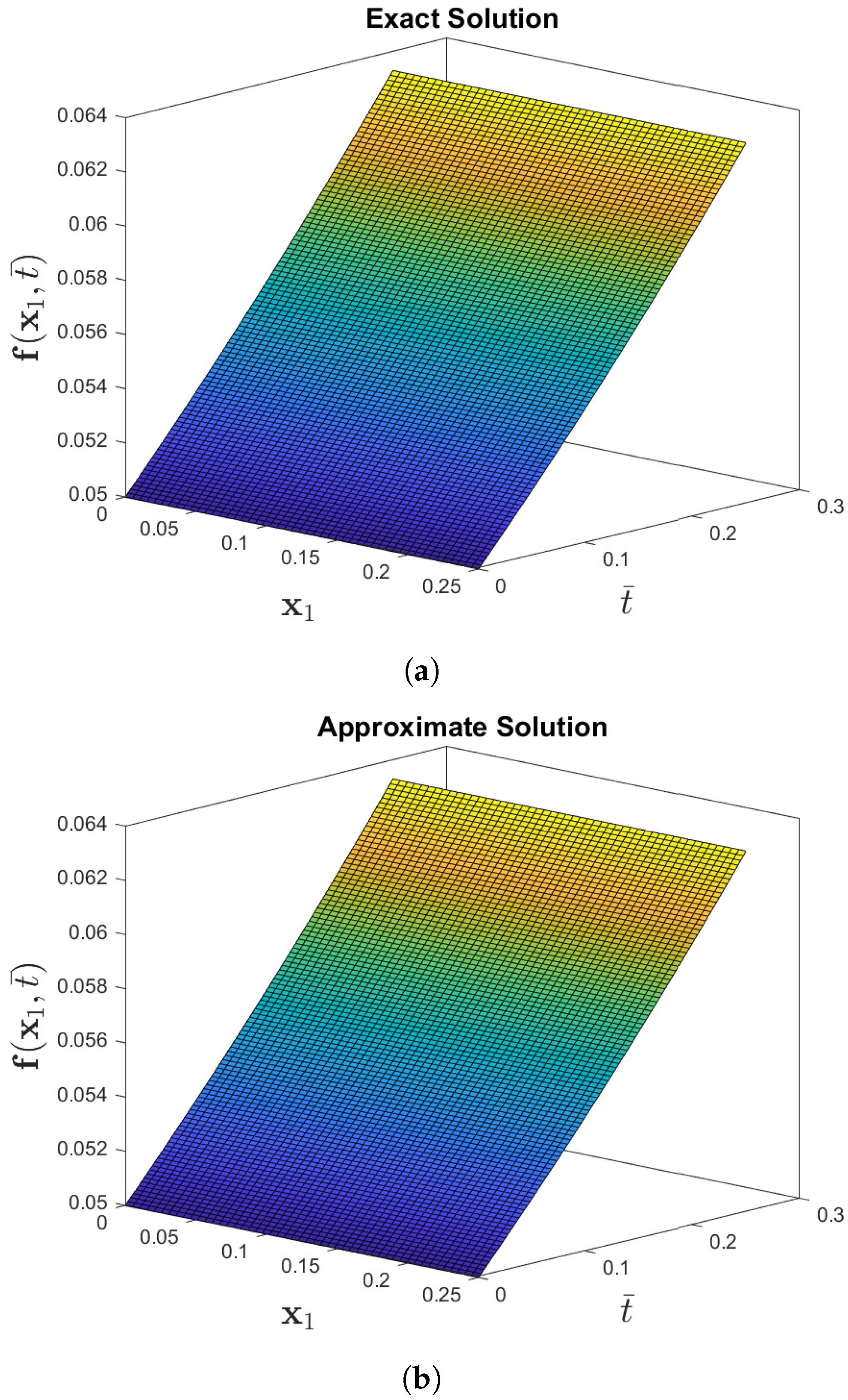
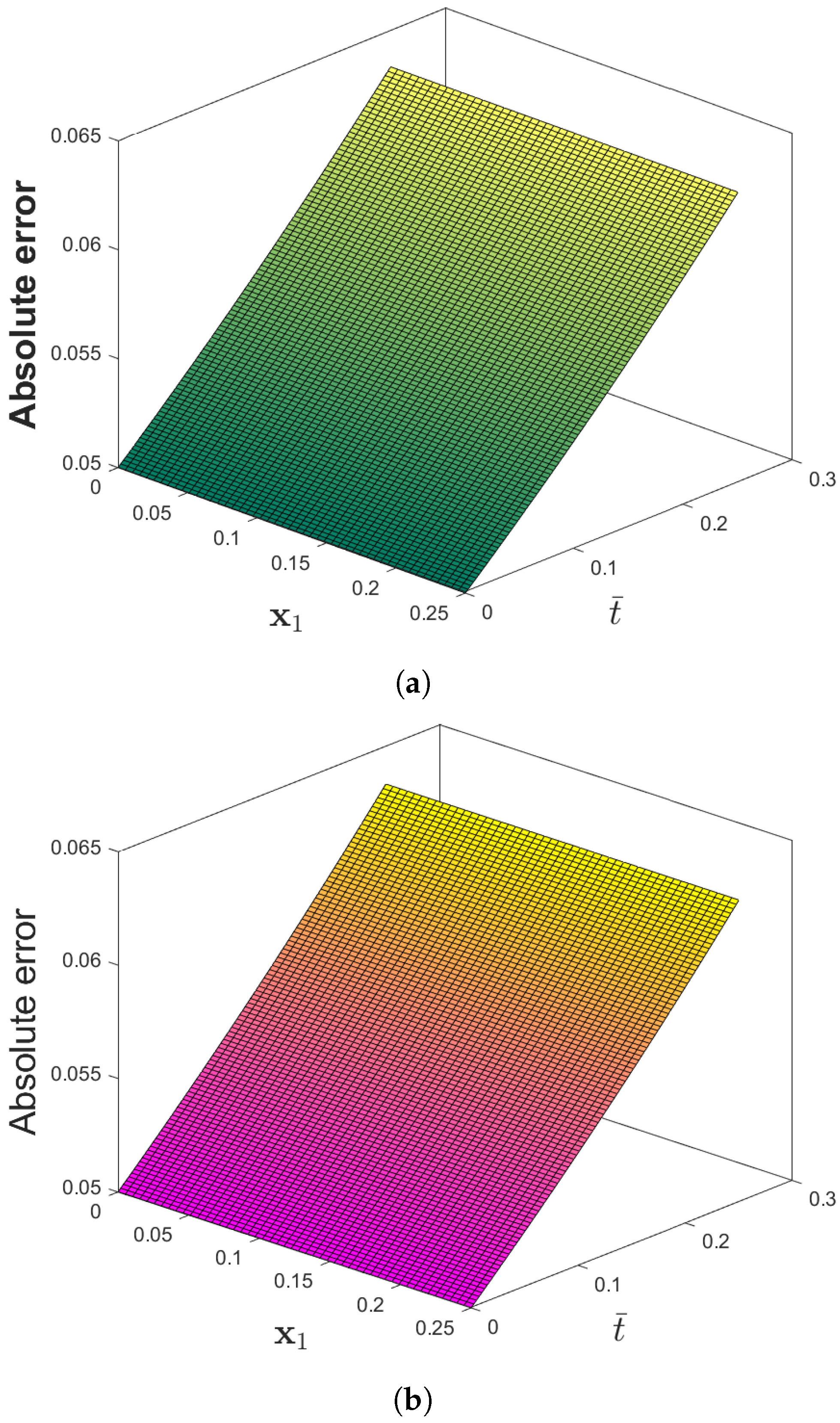

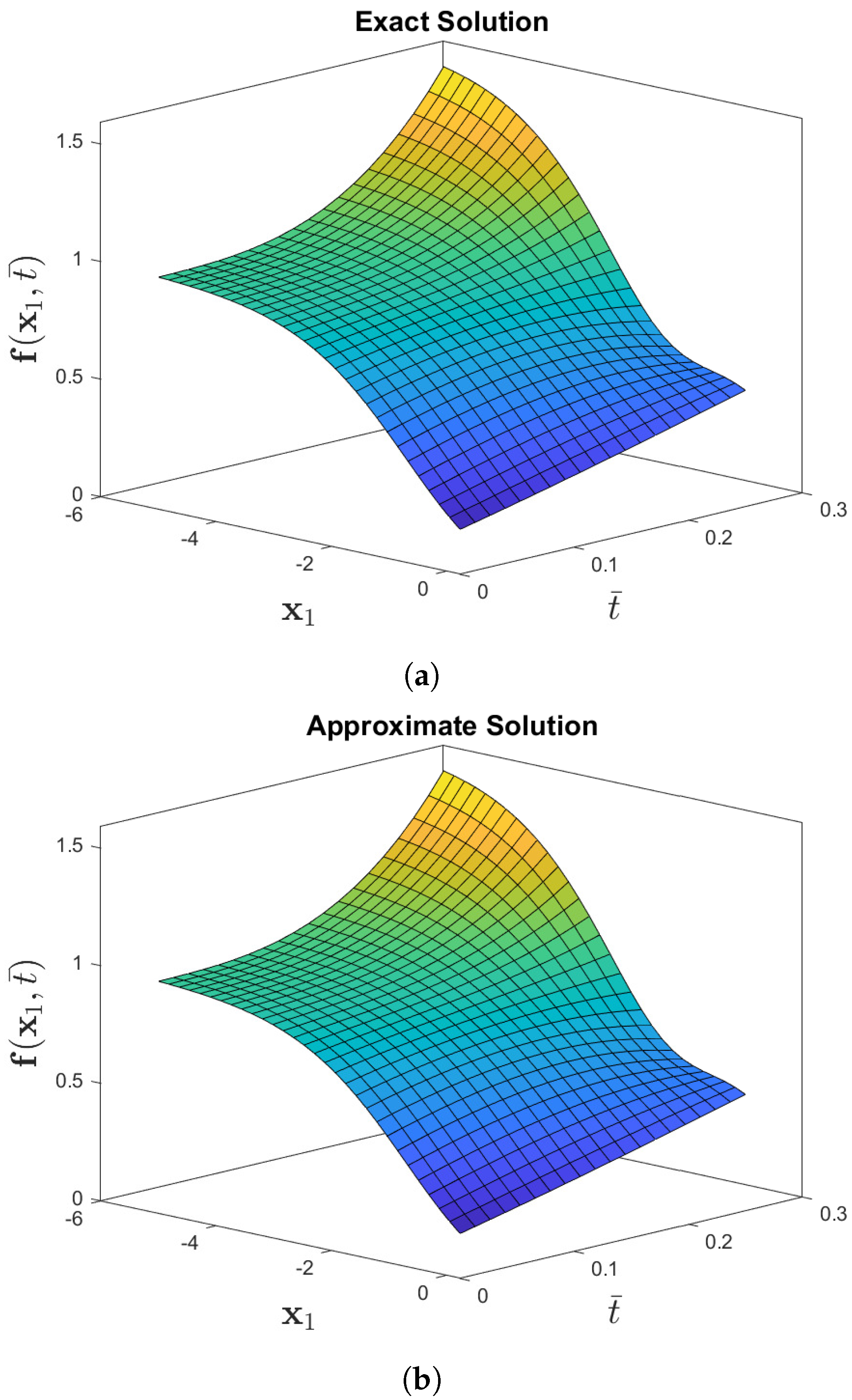



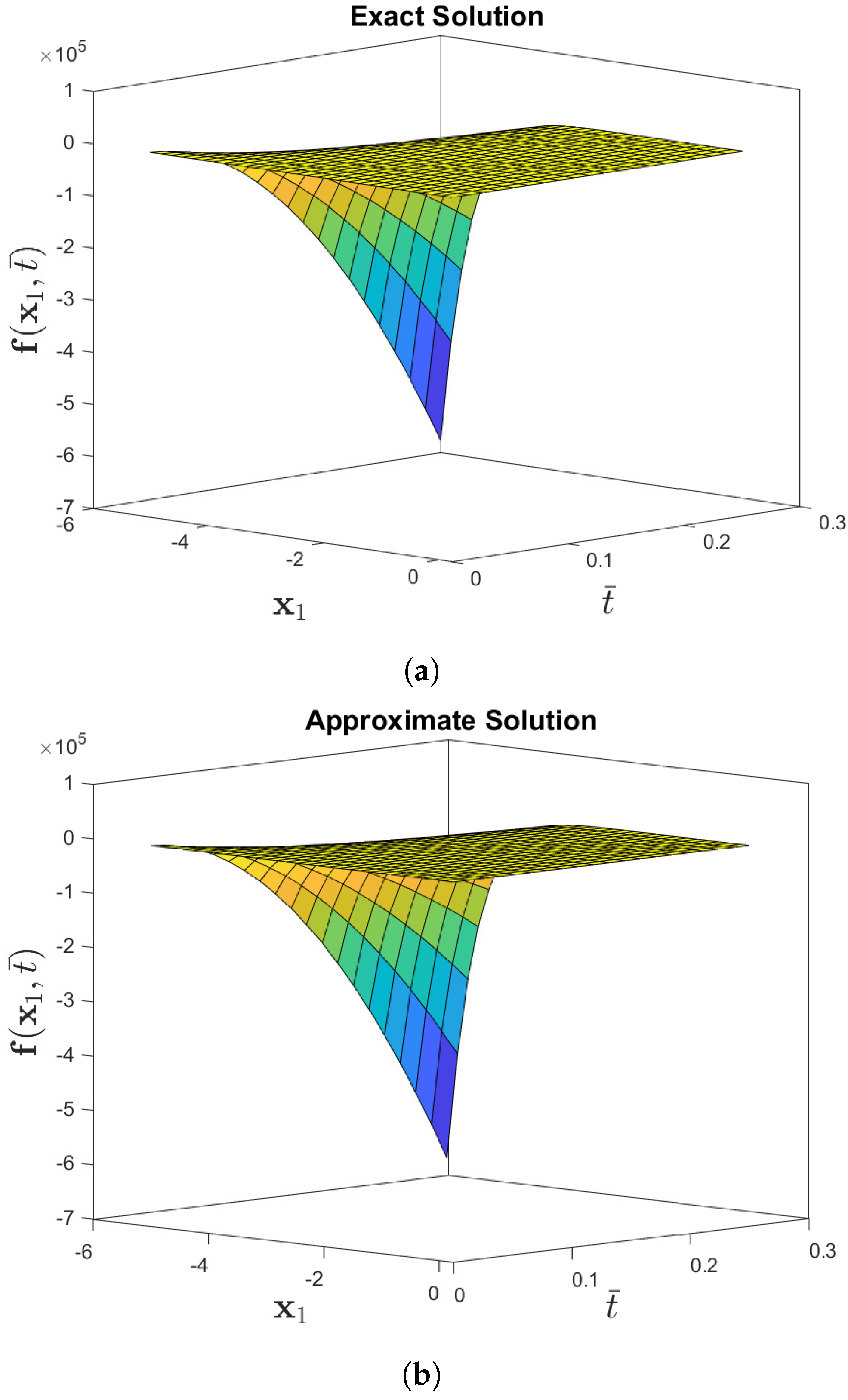
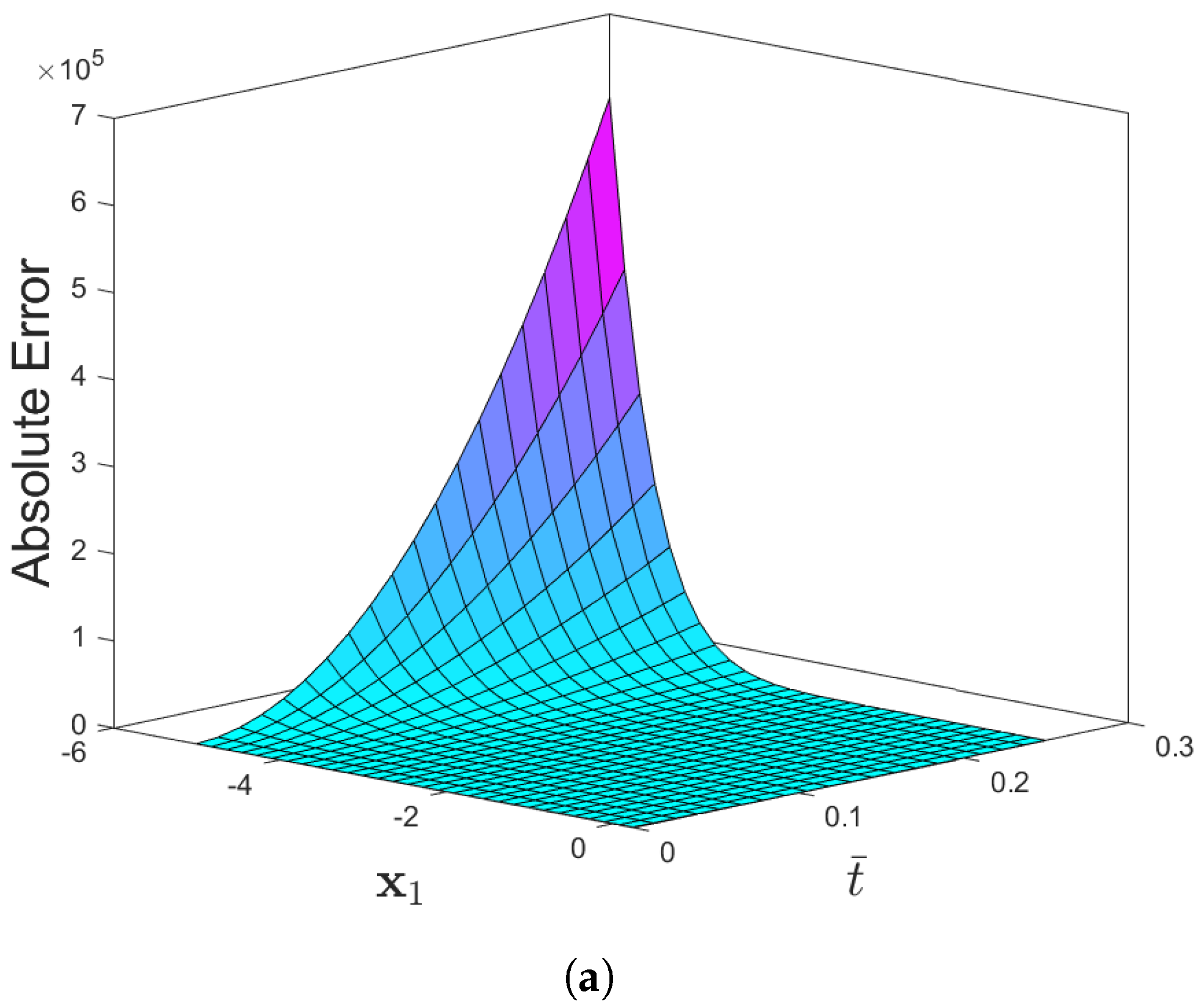

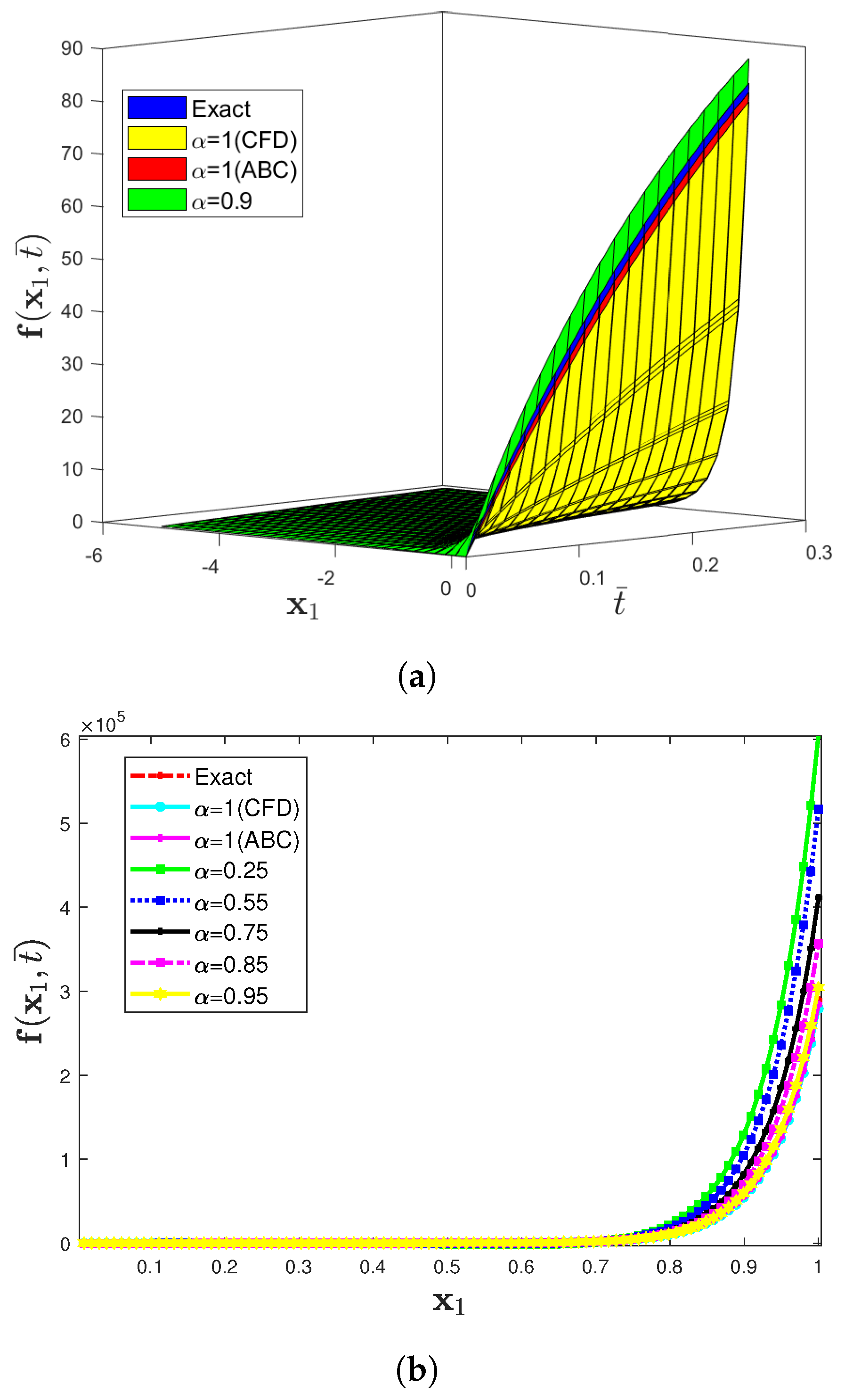

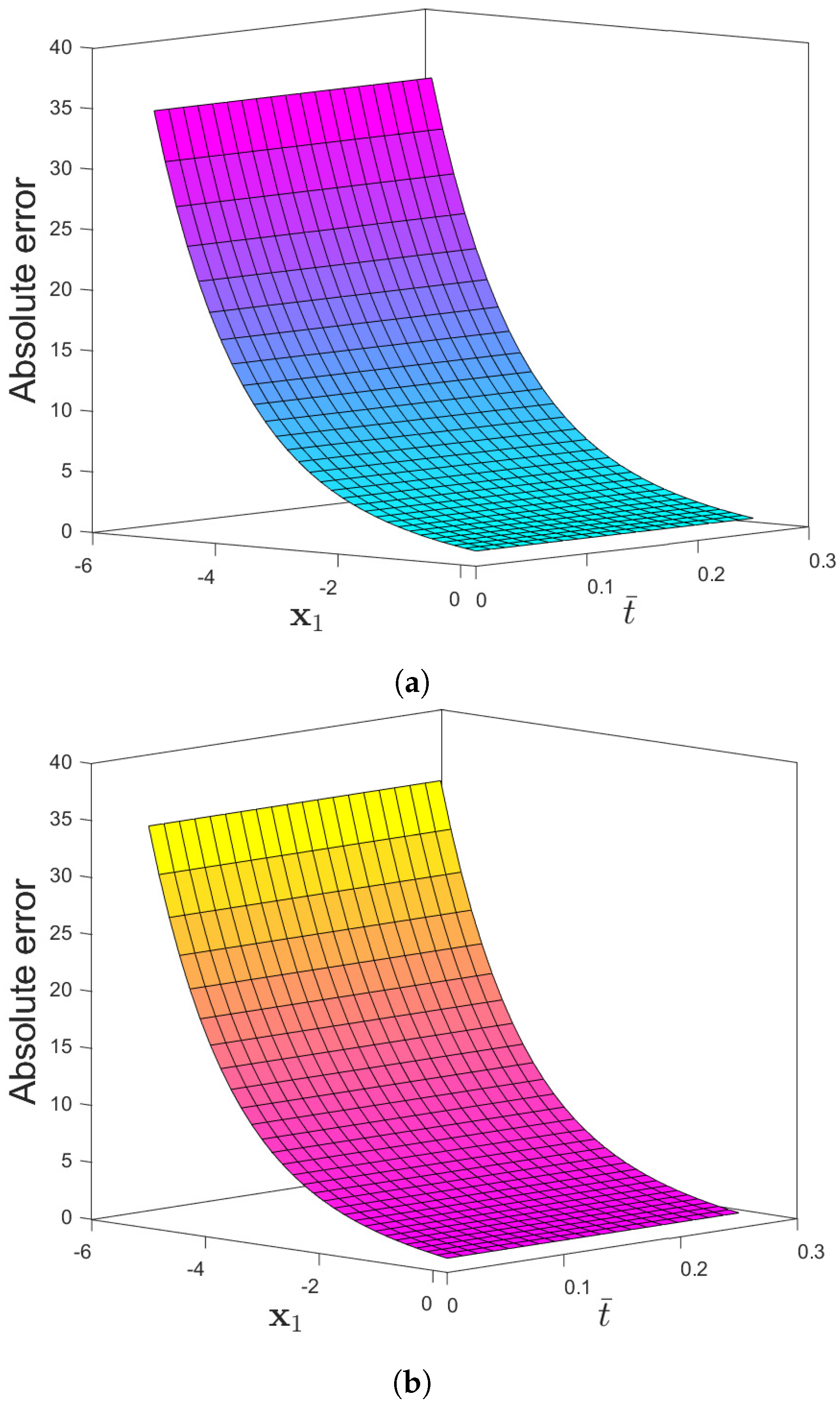
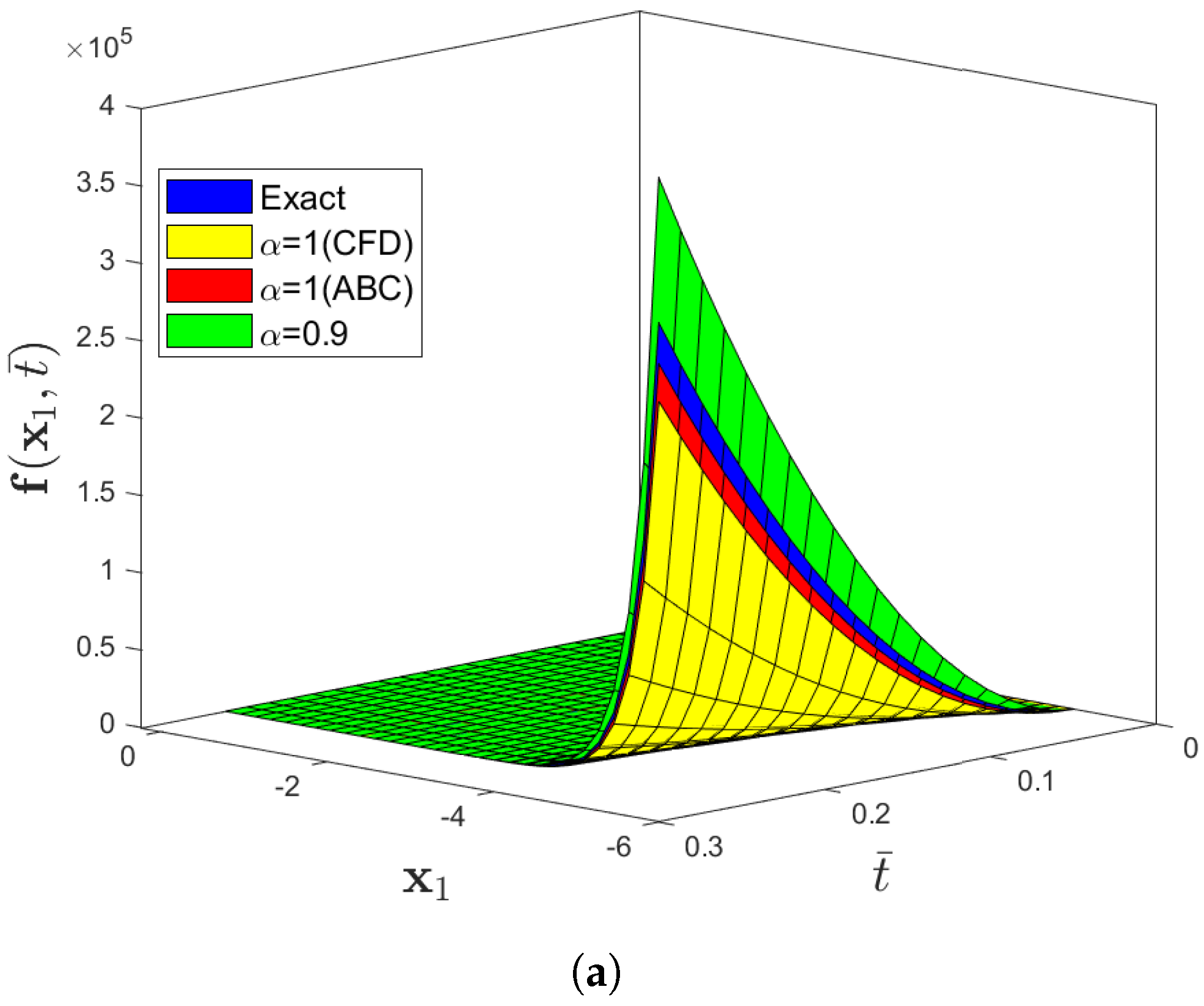
| sol. | sol. | HPM sol. [39] | ||||
|---|---|---|---|---|---|---|
| 0.1 | 1.009 | 1.009 | 2.329 | 0 | 1.319 | 8.999 × |
| 0.2 | 2.016 | 2.016 | 4.091 | 0 | 2.017 | 7.987 |
| 0.3 | 3.021 | 3.021 | 5.429 | 0 | 2.408 | 9.210 |
| 0.4 | 4.024 | 4.024 | 6.464 | 1.00 | 2.440 | 7.540 |
| 0.5 | 5.025 | 5.025 | 7.292 | 3.00 | 2.267 | 8.908 |
| 0.6 | 6.024 | 6.024 | 7.984 | 1.00 | 1.960 | 9.765 |
| 0.7 | 7.021 | 7.021 | 8.589 | 0 | 1.568 | 9.344 |
| 0.8 | 8.016 | 8.016 | 9.131 | 3.00 | 1.115 | 9.123 |
| 0.9 | 9.009 | 9.009 | 9.609 | 3.00 | 6.000 | 9.777 |
| 1.0 | 1.000 | 1.000 | 1.000 | 0 | 0 | 1.000 |
| sol. | sol. | HPM sol. [39] | ||||
|---|---|---|---|---|---|---|
| 0.1 | 2.377 | 2.375 | 2.432 | 1.394 | −5.7000 | 8.387 |
| 0.2 | 2.140 | 2.138 | 2.159 | 1.119 | −0.019 | 9.567 |
| 0.3 | 1.917 | 1.915 | 2.000 | 9.68 | −0.083 | 4.534 |
| 0.4 | 1.709 | 1.708 | 1.888 | 7.32 | −0.179 | 8.887 |
| 0.5 | 1.516 | −0.18 | 1.575 | 4.85 | −0.059 | 7.337 |
| 0.6 | 1.339 | 1.338 | 1.958 | 2.35 | −0.619 | 9.337 |
| 0.7 | 1.176 | 1.175 | 1.234 | 4.85 | −0.58 | 7.337 |
| 0.8 | 1.029 | 1.029 | 1.416 | 2.51 | −0.055 | 9.998 |
| 0.9 | 8.966 | 8.971 | 9.516 | 4.76 | 3.426 | 9.001 |
| 1.0 | 7.778 | 7.784 | 9.001 | 6.75 | −1.223 | 7.337 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rashid, S.; Hammouch, Z.; Aydi, H.; Ahmad, A.G.; Alsharif, A.M. Novel Computations of the Time-Fractional Fisher’s Model via Generalized Fractional Integral Operators by Means of the Elzaki Transform. Fractal Fract. 2021, 5, 94. https://doi.org/10.3390/fractalfract5030094
Rashid S, Hammouch Z, Aydi H, Ahmad AG, Alsharif AM. Novel Computations of the Time-Fractional Fisher’s Model via Generalized Fractional Integral Operators by Means of the Elzaki Transform. Fractal and Fractional. 2021; 5(3):94. https://doi.org/10.3390/fractalfract5030094
Chicago/Turabian StyleRashid, Saima, Zakia Hammouch, Hassen Aydi, Abdulaziz Garba Ahmad, and Abdullah M. Alsharif. 2021. "Novel Computations of the Time-Fractional Fisher’s Model via Generalized Fractional Integral Operators by Means of the Elzaki Transform" Fractal and Fractional 5, no. 3: 94. https://doi.org/10.3390/fractalfract5030094
APA StyleRashid, S., Hammouch, Z., Aydi, H., Ahmad, A. G., & Alsharif, A. M. (2021). Novel Computations of the Time-Fractional Fisher’s Model via Generalized Fractional Integral Operators by Means of the Elzaki Transform. Fractal and Fractional, 5(3), 94. https://doi.org/10.3390/fractalfract5030094






