Performance of Rim-Seals in Upstream and Downstream Cavities over a Range of Flow Coefficients †
Abstract
1. Introduction
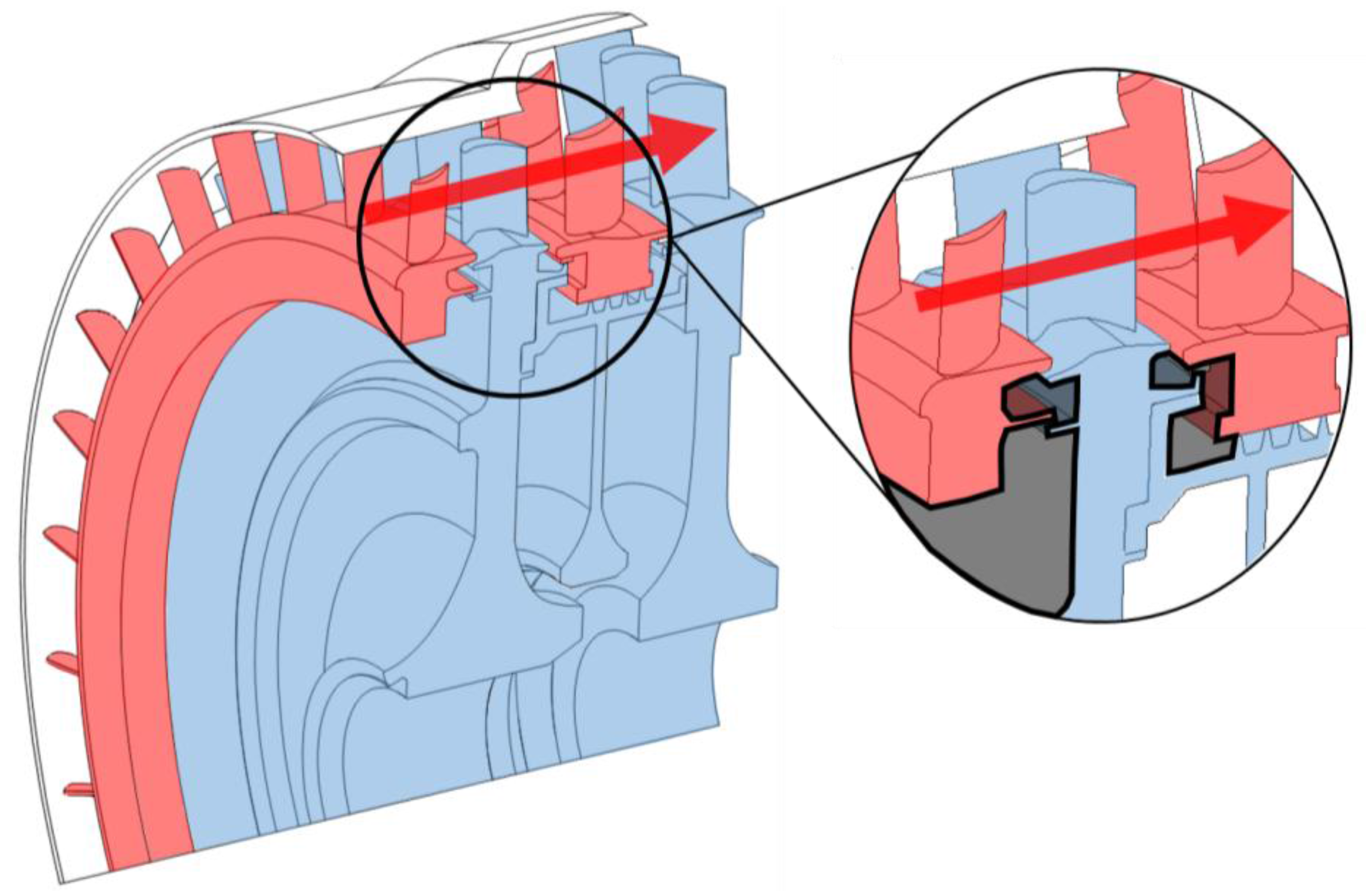
2. Rotor-Stator Systems
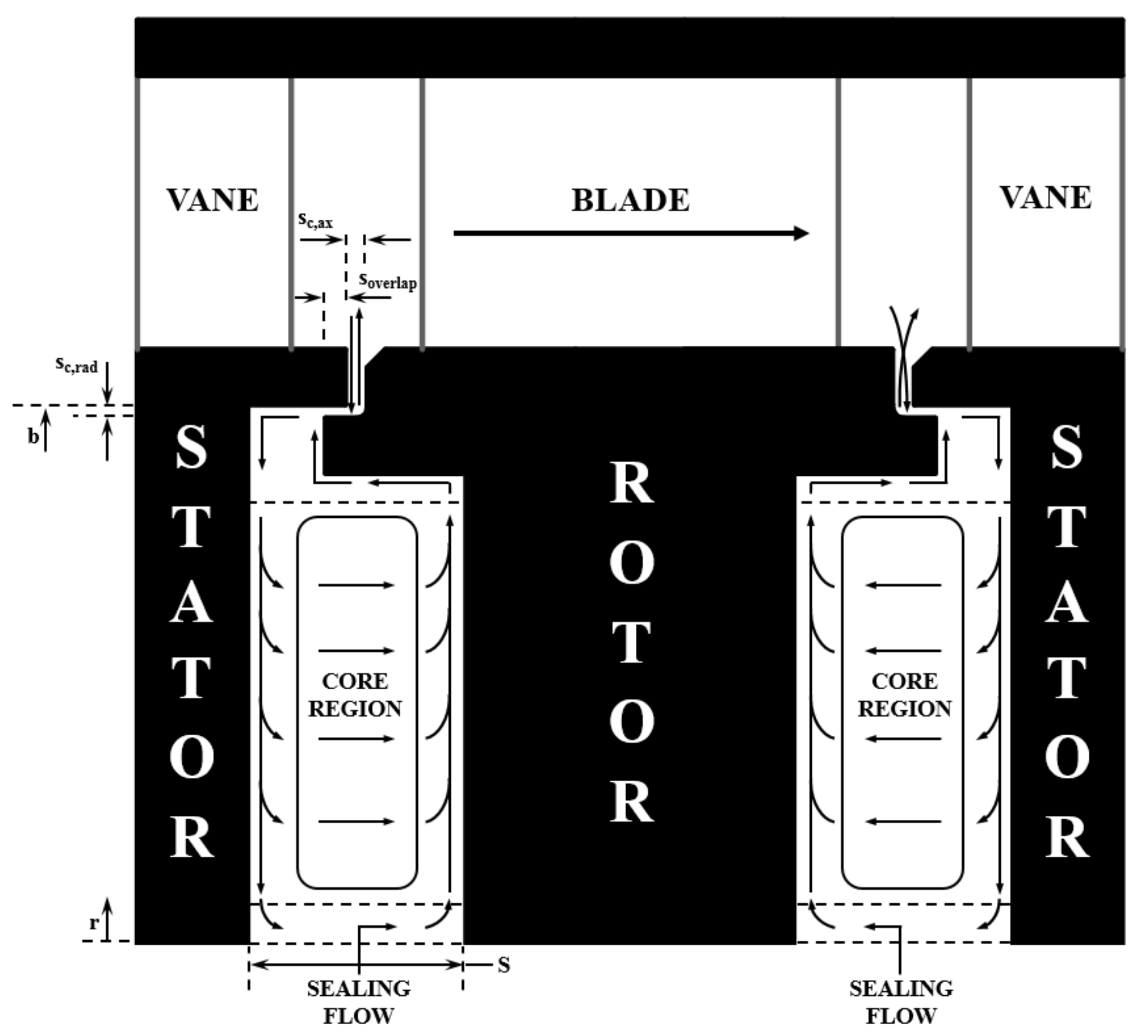
2.1. Fluid Structure in the Wheel-Space
2.2. Governing Non-Dimensional Parameters for Ingress
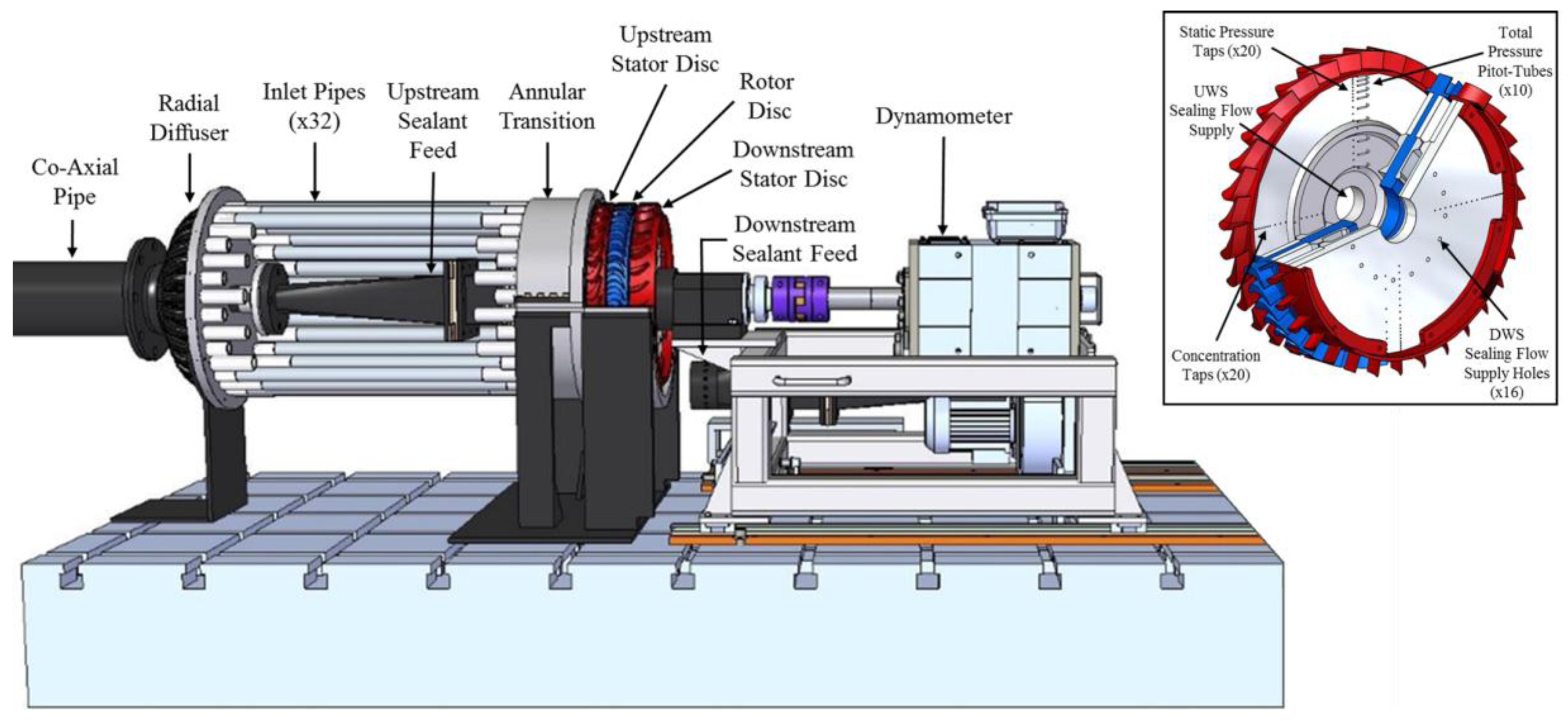
3. The 1.5-Stage Gas Turbine Test Facility
3.1. Facility Overview
3.2. Operating Conditions
3.3. Experimental Measurements and Instrumentation
3.4. Geometry of Single Radial-Clearance Seals
4. Experimental Results and Discussion
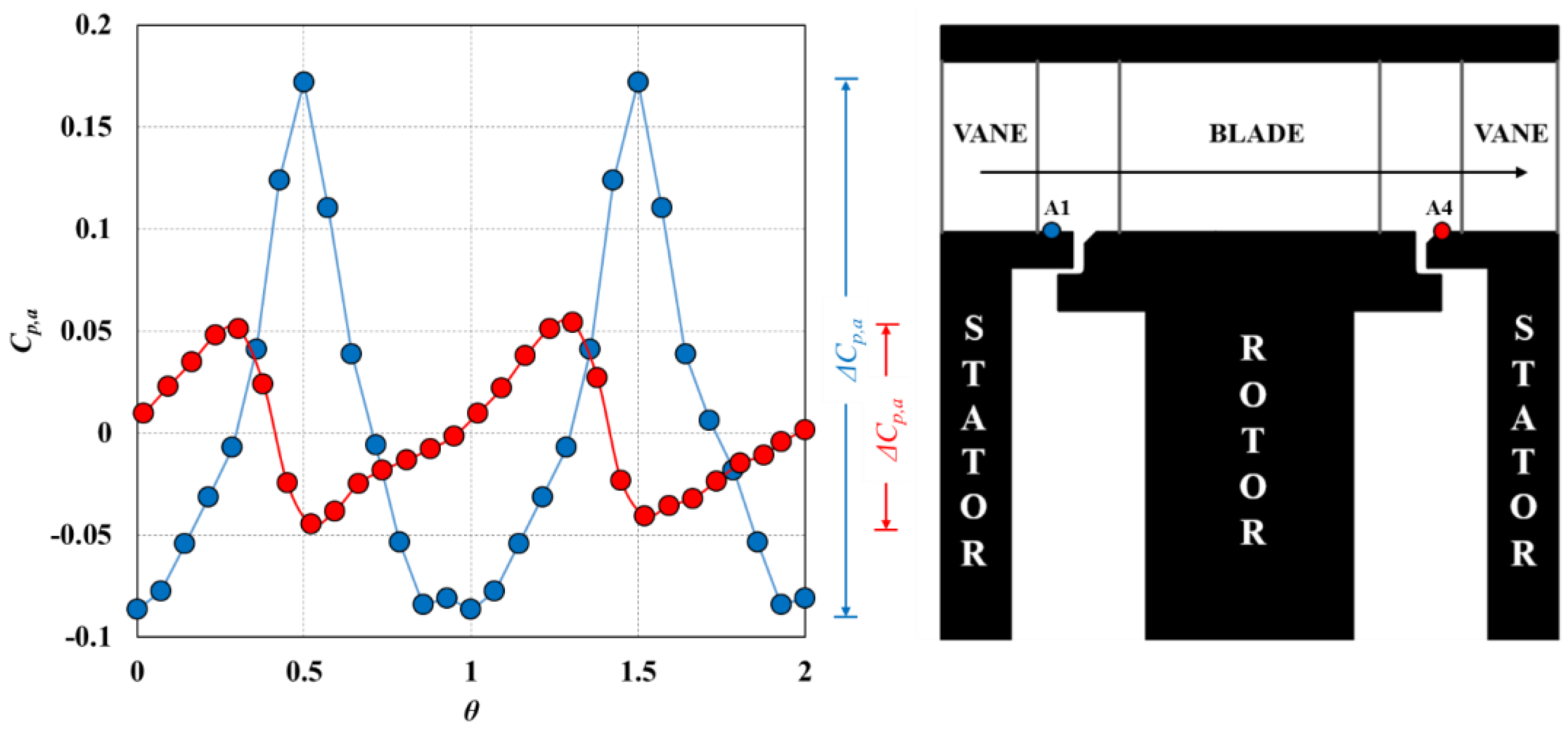
4.1. Annulus Pressure Measurements
4.2. Wheel-Space Concentration Measurements
4.2.1. Radial Distribution of Effectiveness
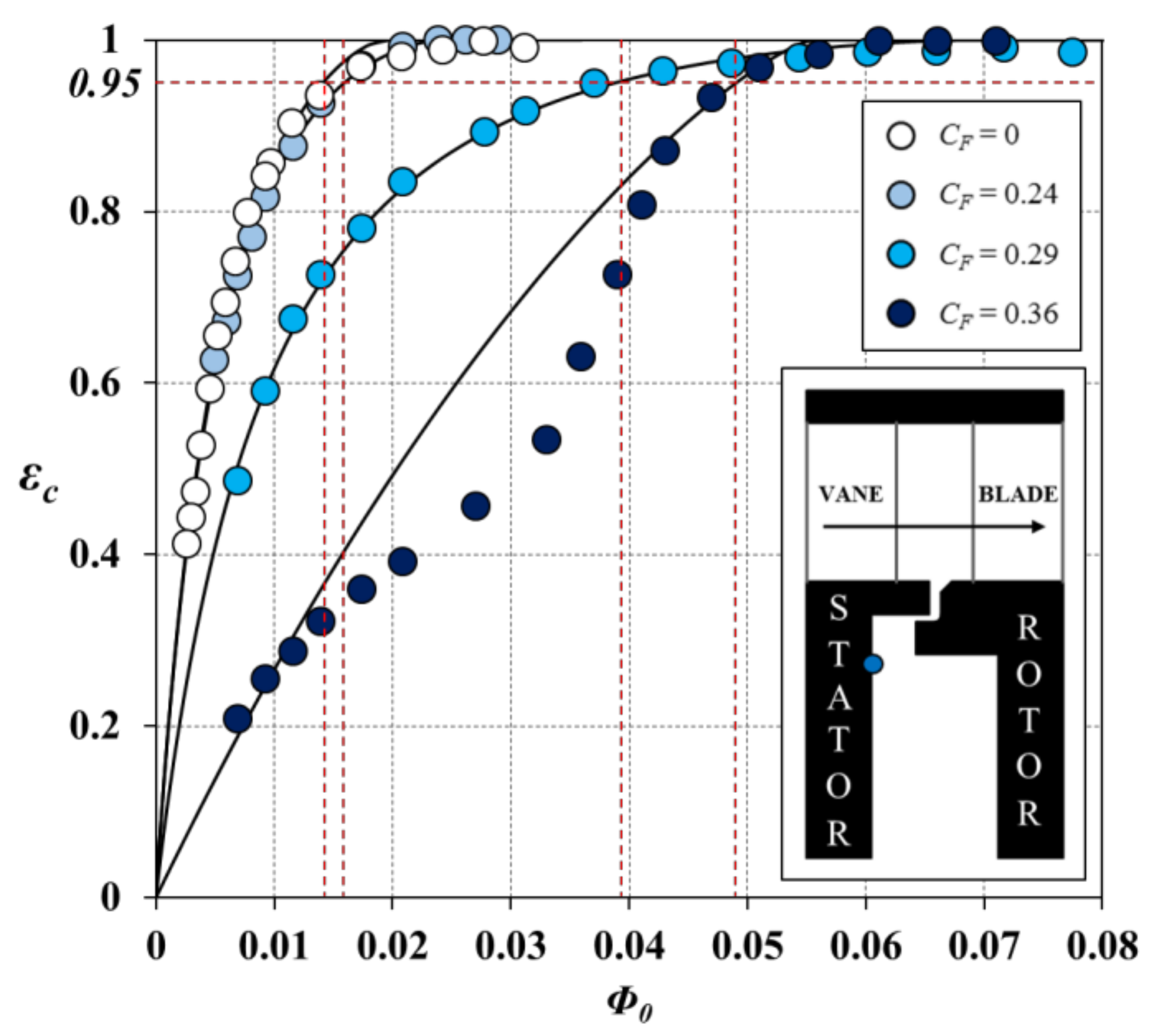
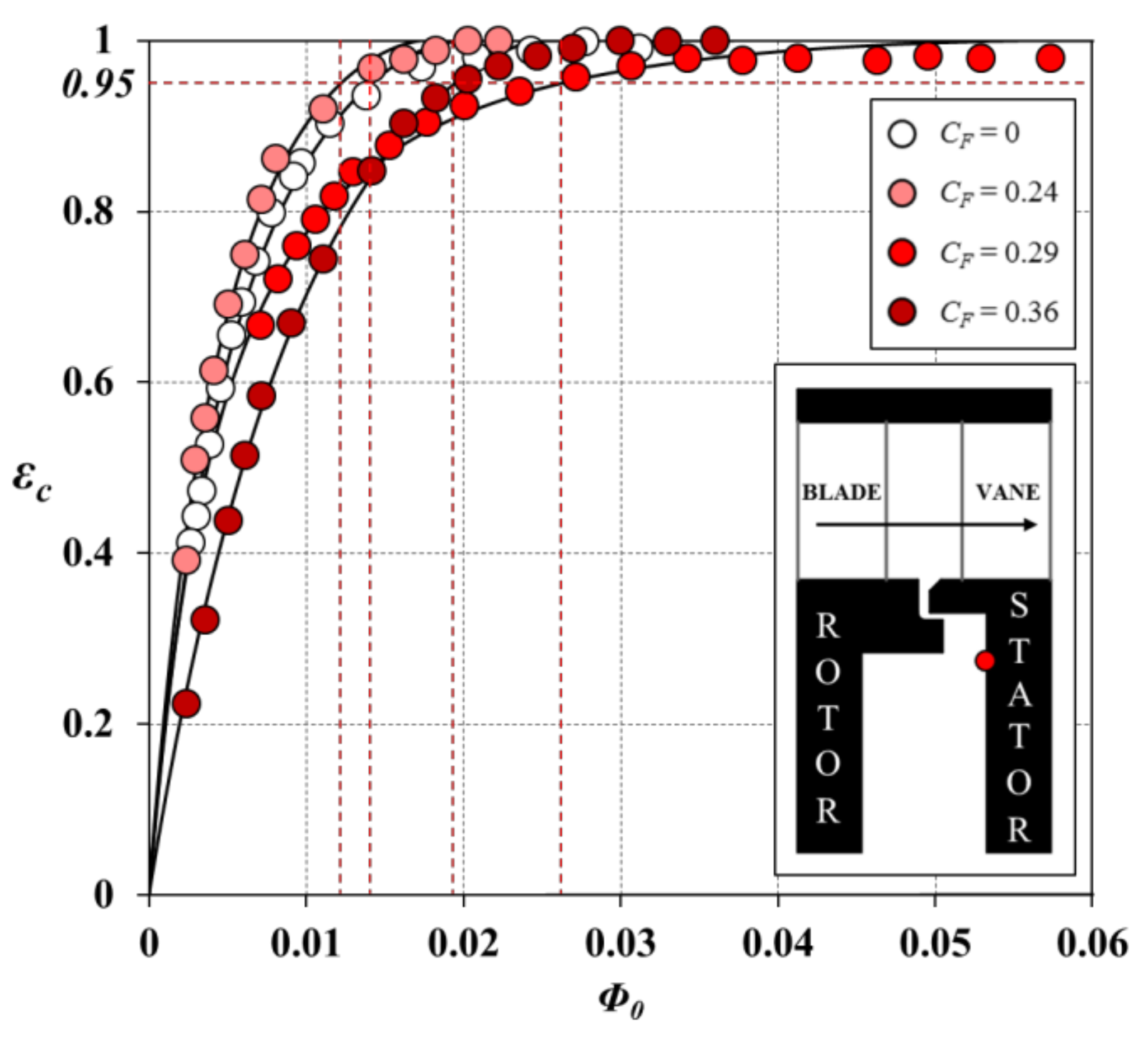
4.2.2. Variation of Concentration Effectiveness with Sealing Flow
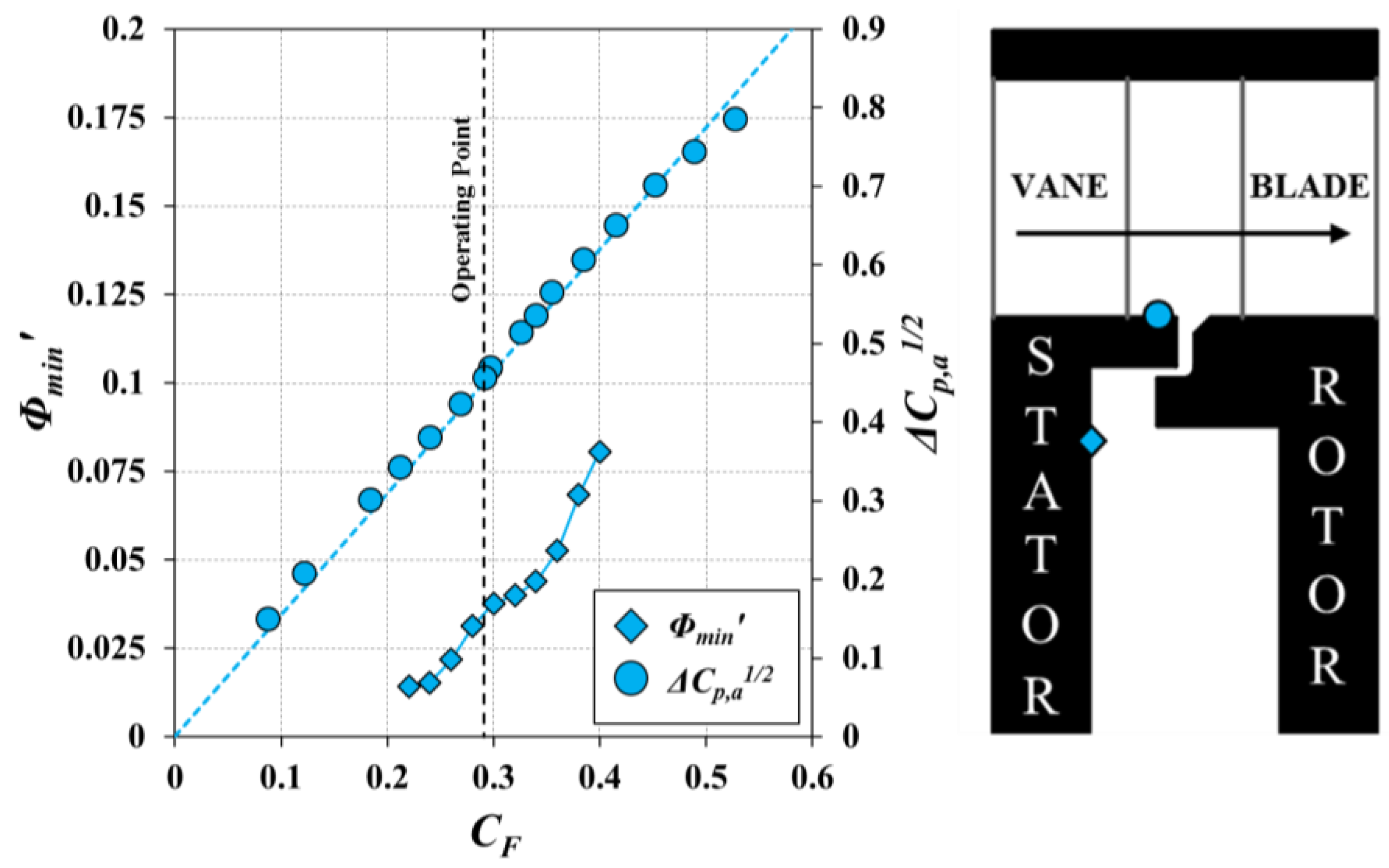
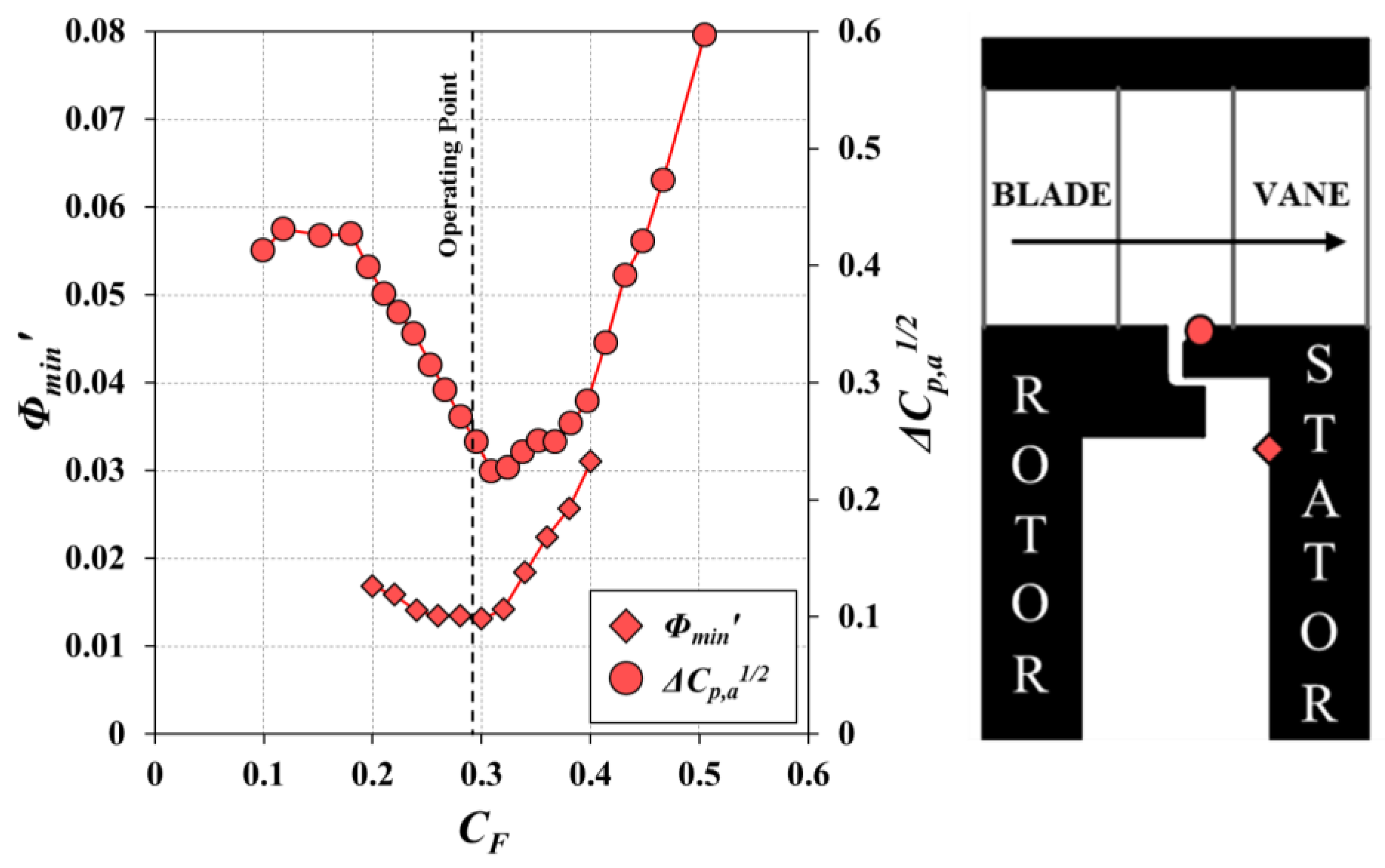
4.3. Measurements of Ingress at Off-Design Conditions
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| b | radius of seal (m) |
| c | concentration of tracer gas |
| CF | flow coefficient [=W/Ωb] |
| Cp,a | pressure coefficient in annulus [=pa−a/½ρΩ2b2] |
| Cw,0 | non-dimensional sealing flow rate [=ṁ/μb] |
| G | gap ratio [=S/b] |
| Gc | seal-clearance ratio [=sc,ax/b] |
| ṁ | mass flow rate (kg/s) |
| ka | empirical constant |
| M | Mach number |
| p | static pressure (Pa) |
| r | radius (m) |
| Rew | axial Reynolds number in annulus based on radius [=ρWb/μ] |
| Reφ | rotational Reynolds number [=ρΩb2/μ] |
| sc | seal clearance (m) |
| S | axial clearance between rotor and stator (m) |
| U | bulk mean radial seal velocity [=ṁ0/2πρbsc] |
| W | axial velocity in annulus (m/s) |
| z | axial coordinate in wheel-space (m) |
| εc | concentration effectiveness |
| Φ0 | non-dimensional sealing parameter [=U/Ωb] |
| Φmin | minimum value of Φ0 to seal wheel-space |
| Φmin' | value of Φ0 when εc = 0.95 |
| λT | turbulent flow parameter [=Cw,0 Reϕ−0.8] |
| μ | dynamic viscosity (kg/ms) |
| ρ | density (kg/m3) |
| Ω | angular speed of rotating disc (rad/s) |
| Subscripts | |
| a | annulus |
| ax | axial |
| min | minimum |
| rad | radial |
| 0 | sealing flow |
| s | stator surface |
References
- Owen, J.M. Prediction of Ingestion through Turbine Rim Seals—Part II: Externally Induced and Combined Ingress. ASME J. Turbomach. 2011, 133, 031006. [Google Scholar] [CrossRef]
- Owen, J.M. Prediction of Ingestion through Turbine Rim Seals—Part I: Rotationally Induced Ingress. ASME J. Turbomach. 2011, 133, 031005. [Google Scholar] [CrossRef]
- Patinios, M.; Scobie, J.A.; Sangan, C.M.; Owen, J.M.; Lock, G.D. Measurements and Modeling of Ingress in a New 1.5-Stage Turbine Research Facility. ASME J. Eng. Gas Turbines Power 2016, 139, 012603. [Google Scholar] [CrossRef]
- Childs, P.R.N. Rotating Flow; Butterworth-Heinemann: Oxford, UK, 2011. [Google Scholar]
- Scobie, J.A.; Sangan, C.M.; Owen, J.M.; Lock, G.D. Review of Ingress in Gas Turbines. ASME J. Eng. Gas Turbines Power 2016, 138, 120801. [Google Scholar] [CrossRef]
- Scobie, J.A.; Hualca, F.P.; Sangan, C.M.; Lock, G.D. Egress Interaction through Turbine Rim Seals. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. Paper No. GT2017-64632. [Google Scholar] [CrossRef]
- Scobie, J.A. An Experimental Study of Gas Turbine Rim Seals. Ph.D. Thesis, University of Bath, Bath, UK, May 2014. [Google Scholar]
- Owen, J.M.; Rogers, R.H. Flow and Heat Transfer in Rotating-Disc Systems, Vol. 1: Rotor-Stator Systems; Research Studies Press LTD: Taunton, Somerset, UK, 1989. [Google Scholar]
- Daily, J.W.; Nece, R.E. Chamber Dimension Effects on Induced Flow and Frictional Resistance of Enclosed Rotating Discs. J. Basic Eng. 1960, 82, 217–232. [Google Scholar] [CrossRef]
- Scobie, J.A.; Hualca, F.P.; Patinios, M.; Sangan, C.M.; Owen, M.J.; Lock, G.D. Re-Ingestion of Upstream Egress in a 1.5-Stage Gas Turbine Rig. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. Paper No. GT2017-64620. [Google Scholar] [CrossRef]
- Sangan, C.M.; Pountney, O.J.; Zhou, K.; Wilson, M.; Owen, J.M.; Lock, G.D. Experimental Measurements of Ingestion through Turbine Rim Seals—Part 1: Externally-Induced Ingress. ASME J. Turbomach. 2013, 135, 021012. [Google Scholar] [CrossRef]
- Zhou, K.; Wood, S.N.; Owen, J.M. Statistical and Theoretical Models of Ingestion through Turbine Rim Seals. ASME J. Turbomach. 2012, 135, 021014. [Google Scholar] [CrossRef]
- Scobie, J.A.; Sangan, C.M.; Owen, J.M.; Wilson, M.; Lock, G.D. Experimental Measurements of Hot Gas Ingestion Through Turbine Rim Seals at Off-Design Conditions. IMechE J. Power Energy 2014, 228, 491–507. [Google Scholar] [CrossRef]

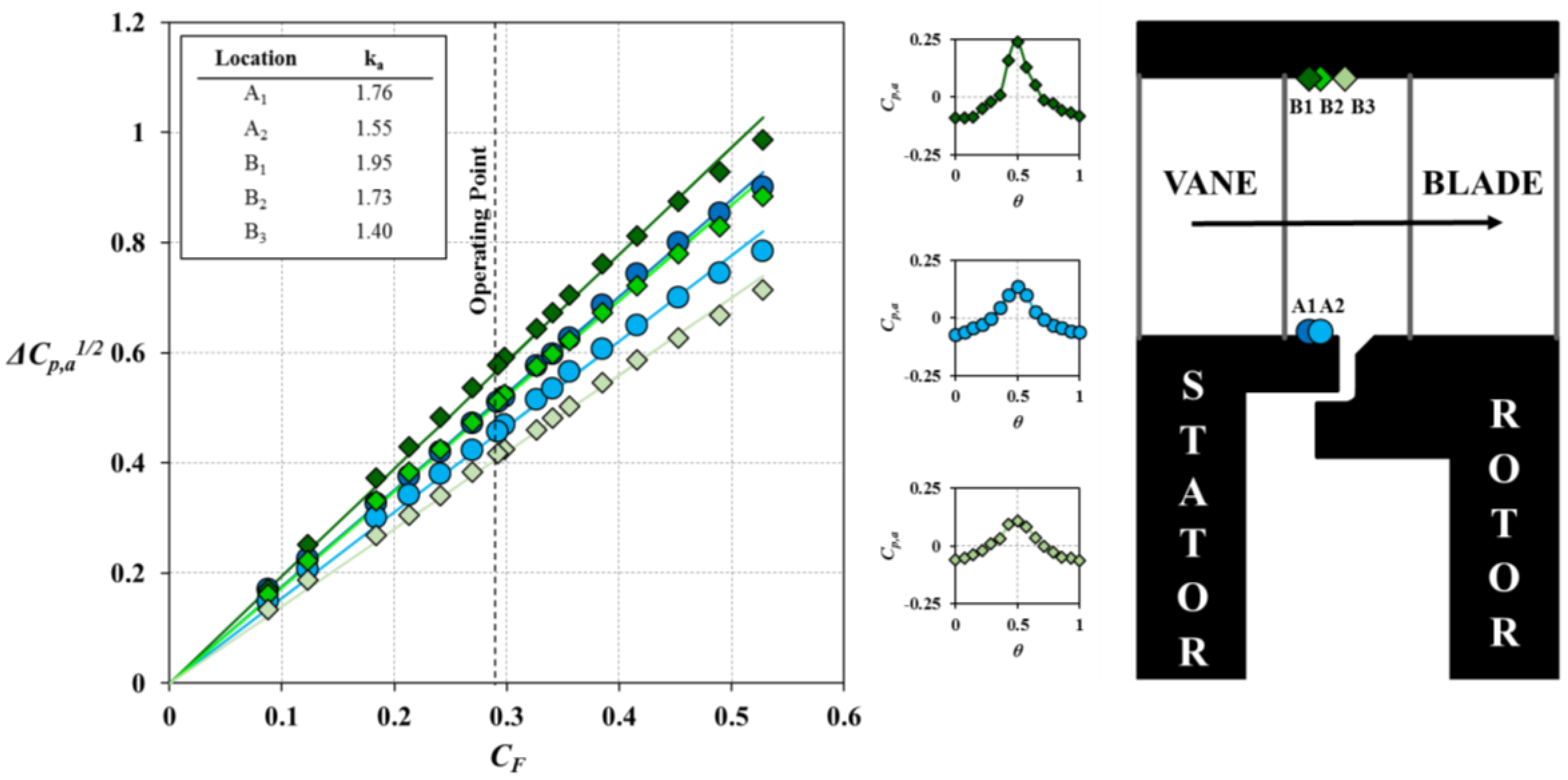
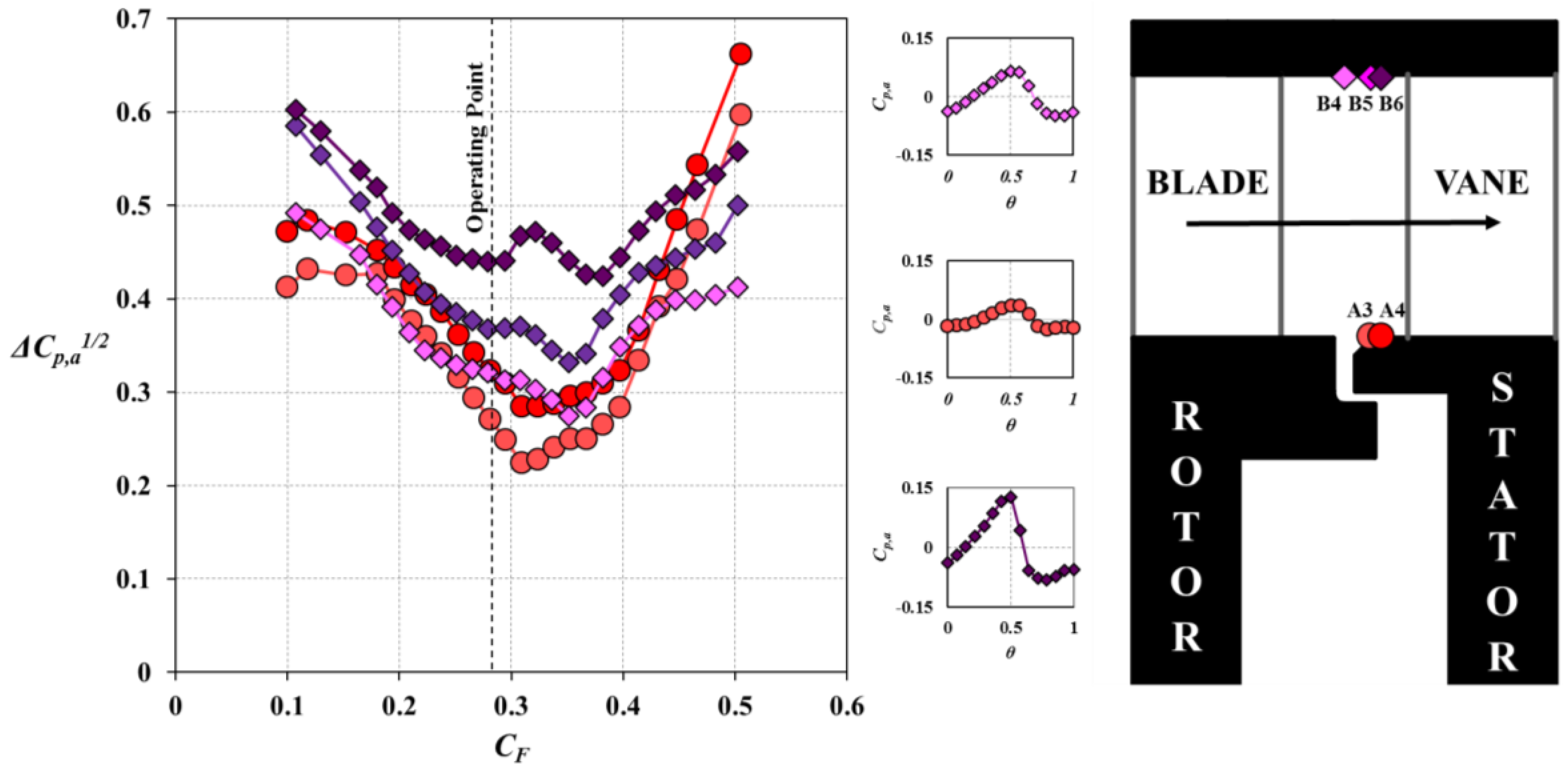
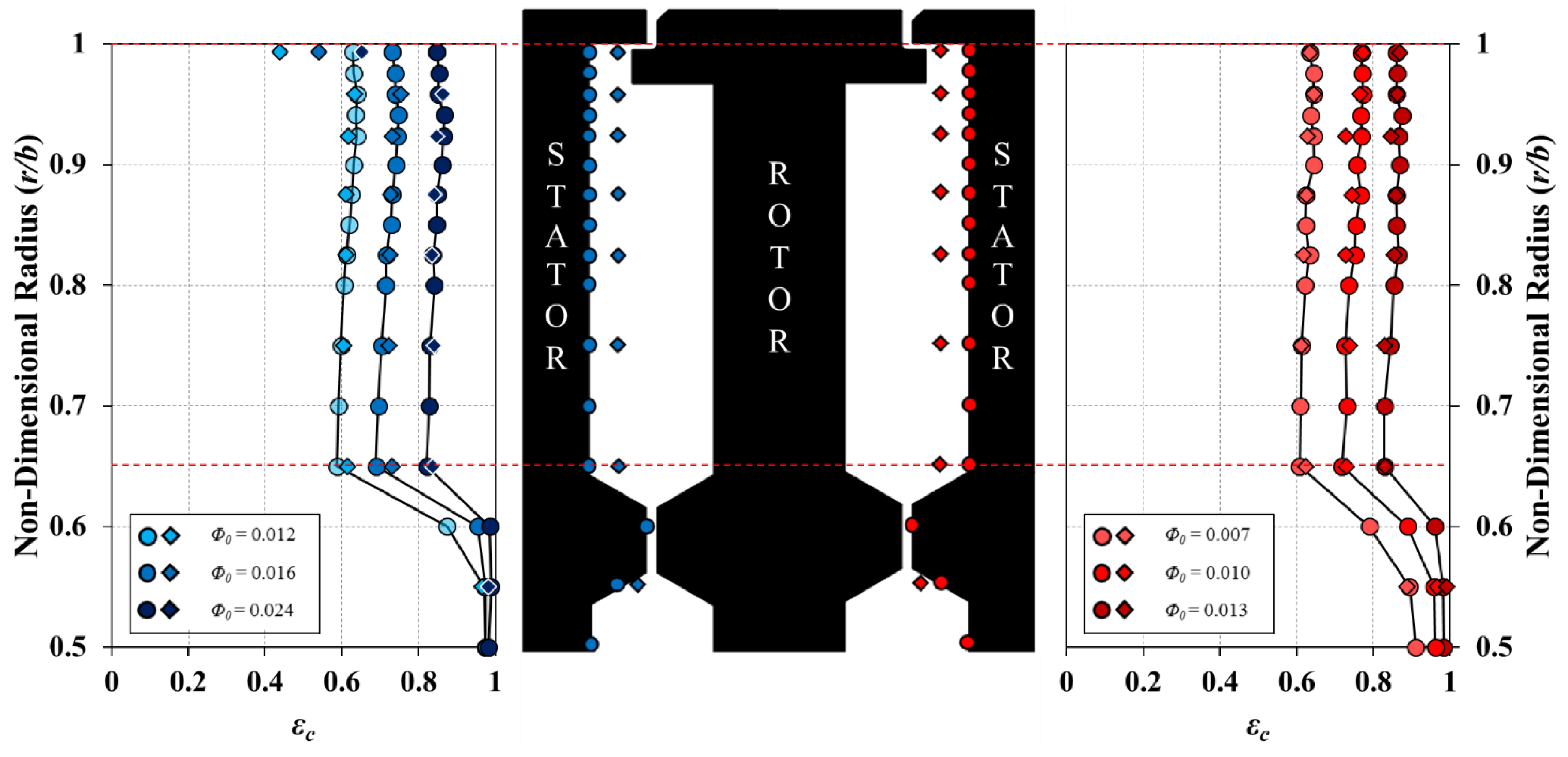
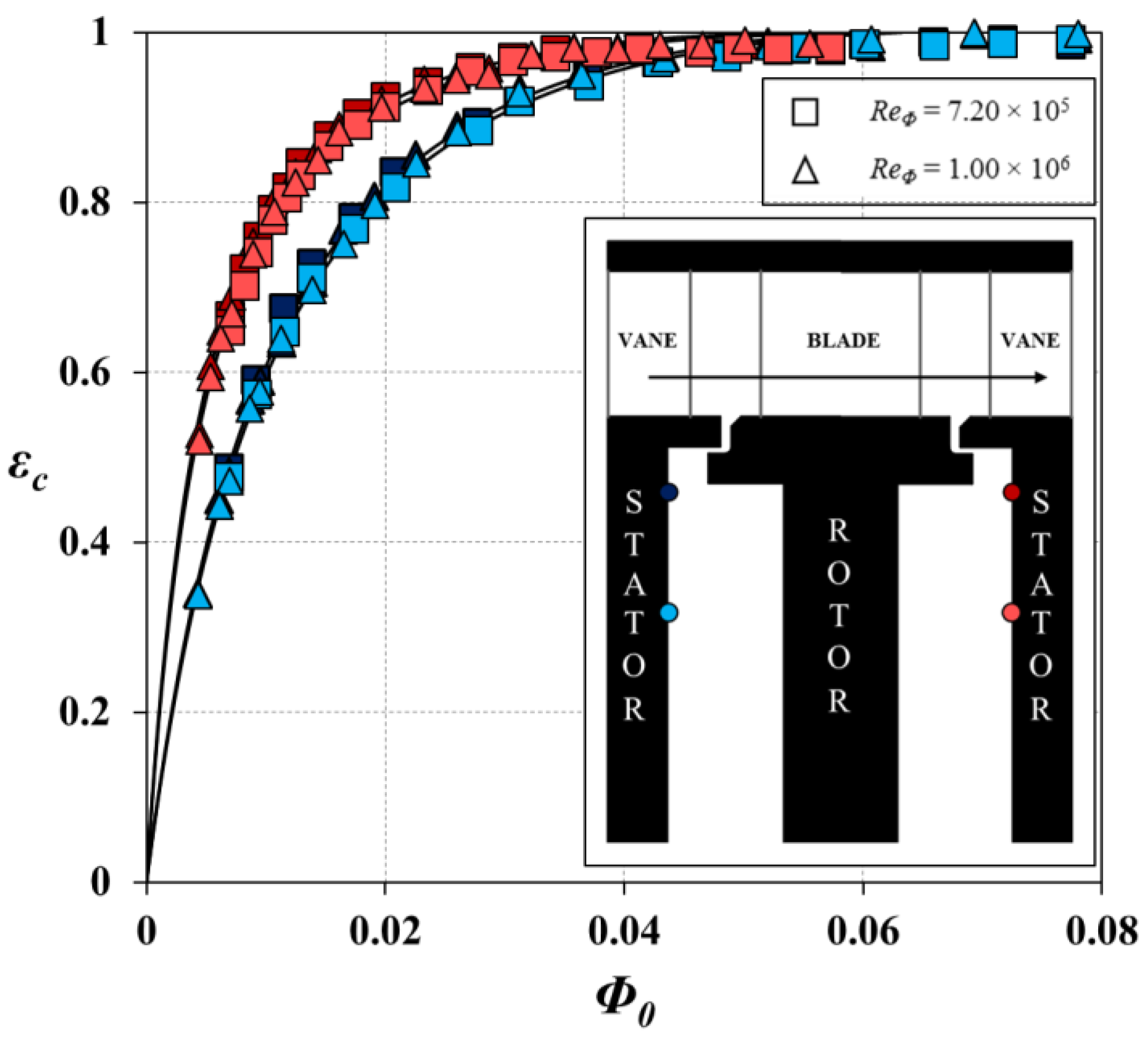
| Parameters | Disc Speed (rpm) | |
|---|---|---|
| 3000 | 4000 | |
| Rotational Reynolds Number (Reφ) | 7.2 × 105 | 1.0 × 106 |
| Axial Reynolds Number (Rew) | 2.1 × 105 | 2.9 × 105 |
| Flow Coefficient (CF) | 0.29 | |
| Vane Exit Mach Number (M) | 0.23 | 0.32 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Patinios, M.; Scobie, J.A.; Sangan, C.M.; Lock, G.D. Performance of Rim-Seals in Upstream and Downstream Cavities over a Range of Flow Coefficients. Int. J. Turbomach. Propuls. Power 2017, 2, 21. https://doi.org/10.3390/ijtpp2040021
Patinios M, Scobie JA, Sangan CM, Lock GD. Performance of Rim-Seals in Upstream and Downstream Cavities over a Range of Flow Coefficients. International Journal of Turbomachinery, Propulsion and Power. 2017; 2(4):21. https://doi.org/10.3390/ijtpp2040021
Chicago/Turabian StylePatinios, Marios, James A. Scobie, Carl M. Sangan, and Gary D. Lock. 2017. "Performance of Rim-Seals in Upstream and Downstream Cavities over a Range of Flow Coefficients" International Journal of Turbomachinery, Propulsion and Power 2, no. 4: 21. https://doi.org/10.3390/ijtpp2040021
APA StylePatinios, M., Scobie, J. A., Sangan, C. M., & Lock, G. D. (2017). Performance of Rim-Seals in Upstream and Downstream Cavities over a Range of Flow Coefficients. International Journal of Turbomachinery, Propulsion and Power, 2(4), 21. https://doi.org/10.3390/ijtpp2040021







