Review of Current Software for Analyzing Total X-ray Scattering Data from Liquids
Abstract
1. Introduction
2. Fundamentals of Extracting Structure Factors and Pair Distribution Functions from X-ray Total Scattering Data
3. Methods and Discussion of PDF Extraction Software Process and Available Corrections
3.1. Original Analysis
3.2. Fast PDF Extraction Software
3.2.1. PDFgetX2
3.2.2. GSAS-II
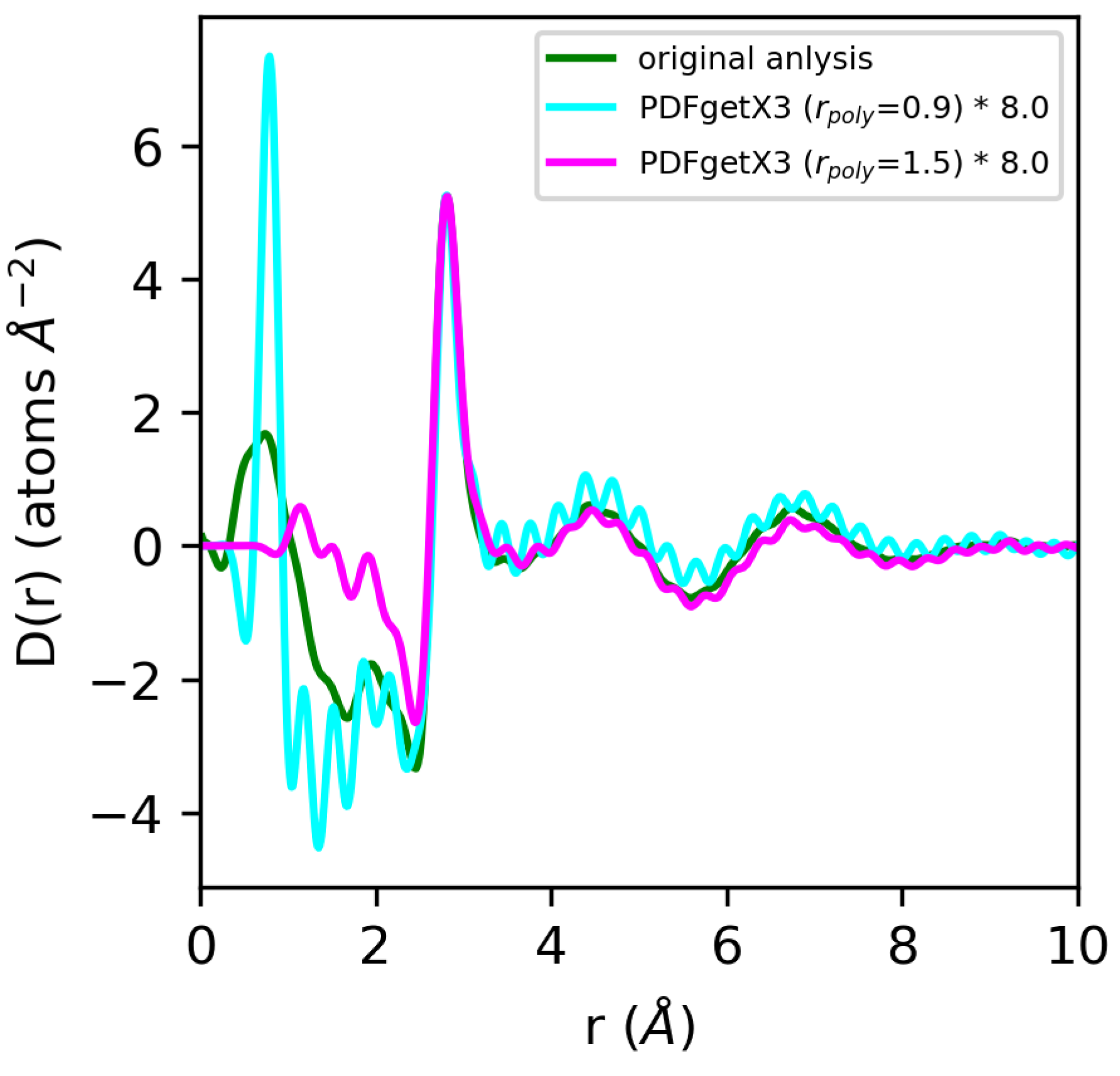
3.2.3. PDFgetX3
3.2.4. LiquidDiffract
3.2.5. GudrunX
3.2.6. BL04B2anaGUI
| Software | Original Analysis | PDFgetX2 [50] | GSAS-II [49] | PDFgetX3 [51] | Liquid Diffract [52] | GudrunX [53] | BL04B2anaGUI [54] |
|---|---|---|---|---|---|---|---|
| Q range (water, Sulfur) | 0.5–20 Å−1 0.5–28 Å−1 | 0.5–20 Å−1 0.5–26 Å−1 | 0.5–20 Å−1 0.5–26 Å−1 | 0.5–20 Å−1 0.5–26 Å−1 | 0.5–20 Å−1 0.5–26 Å−1 | 0.5–20 Å−1 0.5–20 Å−1 | 0.4–18 Å−1 0.45–22 Å−1 |
| Density (water, Sulfur) | 0.1003, 0.0334 (atoms/Å3) [not used in software] | 0.1003, 0.0334 (atoms/Å3) [not used in software] | 29.969 Å3, 29.272 Å3 (Å3/formula unit) | N/A | 0.1003, 0.0334 (atoms/Å3) | 0.1003, 0.0334 (atoms/Å3) | 0.1003, 0.0334 (atoms/Å3) |
| Background/ container scale factor | 1 (manually set) | 1 (manually set) | 1 (manually set, is refinable) | 1 (manually set) | 1 (manually set, is refinable) | 1 (manually set) | 1 (manually set) refinable |
| Compton scattering | Breit–Dirac (order 2) 1/E quadratic (refined) | 1/E quadratic (refined) | Ruland width (refined) background ratio (refined) | Empirical polynomial fit | yes (tabulated values from Hubell) | Breit–Dirac (order 2) | yes (tabulated values from Corner and Mann) |
| Constant offset/flat correction | yes (“fluorescence” refined) | yes (“add background” manually set) | yes (refined) | Empirical polynomial fit | no | yes (“fluorescence” manually adjusted) | yes |
| Self-absorption | yes | no | no | no | no | yes | yes |
| Multiple scattering | yes | no | no | no | no | yes | no |
| Oblique incidence | yes | yes | yes | no | no | [manual pre-correction] | no |
| Optimization Range | 10–20 Å−1 20–26 Å−1 F(Q)→0 | 10–20 Å−1 20–26 Å−1 S(Q)→1 | 10–20 Å−1 20–26 Å−1 (scaling range) | Does not use form factors | Automatic | Automatic | Automatic/manual S(Q)→1 |
| Modification function | Lorch | Lorch | Lorch | Polynomial smoothing (rpoly) | Lorch | Lorchtop hat convolution | Lorch, modified Lorch, modified Welch [65] |
| Output Functions | S(Q), F(Q) **, D(r) | S(Q), F(Q) **, D(r) | S(Q), F(Q) **, D(r), g(r) * | S(Q), F(Q) **, D(r) | S(Q), D(r) *, g(r), T(r) | S(Q) − 1, g(r) − 1 | S(Q), D(r), g(r), T(r), |
3.3. Comparison of Structure Factors and Pair Distribution Functions Obtained using Fast PDF Extraction Software Prior to Analyses
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Benmore, C.J. X-ray and neutron diffraction from glasses and liquids. In Reference Module in Chemistry, Molecular Sciences and Chemical Engineering; Elsevier: Amsterdam, The Netherlands, 2021. [Google Scholar]
- Skinner, L.B.; Benmore, C.J.; Parise, J.B. Area detector corrections for high quality synchrotron X-ray structure factor measurements. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2012, 662, 61–70. [Google Scholar] [CrossRef]
- Chupas, P.J.; Chapman, K.W.; Lee, P.L. Applications of an amorphous silicon-based area detector for high-resolution, high-sensitivity and fast time-resolved pair distribution function measurements. J. Appl. Crystallogr. 2007, 40, 463–470. [Google Scholar] [CrossRef]
- Hubbell, J.H.; Veigele, W.J.; Briggs, E.A.; Brown, R.T.; Cromer, D.T.; Howerton, R.J. Atomic form factors, incoherent scattering functions, and photon scattering cross sections. J. Phys. Chem. Ref. Data 1975, 4, 471–538. [Google Scholar] [CrossRef]
- Waasmaier, D.; Kirfel, A. New analytical scattering-factor functions for free atoms and ions. Acta Crystallogr. Sect. A Found. Crystallogr. 1995, 51, 416–431. [Google Scholar] [CrossRef]
- Paalman, H.H.; Pings, C.J. Numerical Evaluation of X-ray Absorption Factors for Cylindrical Samples and Annular Sample Cells. J. Appl. Phys. 1962, 33, 2635–2639. [Google Scholar] [CrossRef]
- Neuefeind, J.; Poulsen, H.F. Diffraction on disordered materials using “neutron-like” photons. Phys. Scr. 1995, 1995, 112–116. [Google Scholar] [CrossRef]
- Soper, A.K. Multiple scattering from an infinite plane slab. Nucl. Instrum. Methods Phys. Res. 1983, 212, 337–347. [Google Scholar] [CrossRef]
- Soper, A.K.; Egelstaff, P.A. Multiple scattering and attenuation of neutrons in concentric cylinders: I. Isotropic first scattering. Nucl. Instrum. Methods 1980, 178, 415–425. [Google Scholar] [CrossRef]
- Zaleski, J.; Wu, G.; Coppens, P. On the correction of reflection intensities recorded on imaging plates for incomplete absorption in the phosphor layer. J. Appl. Crystallogr. 1998, 31, 302–304. [Google Scholar] [CrossRef]
- Kohara, S.; Pusztai, L. Reverse Monte Carlo Simulations of Noncrystalline Solids. In Atomistic Simulations of Glasses: Fundamentals and Applications; Du, J., Cormack, A., Eds.; Wiley-American Ceramic Society: Westerville, OH, USA, 2022; pp. 60–88. [Google Scholar]
- Laaziri, K.; Kycia, S.; Roorda, S.; Chicoine, M.; Robertson, J.L.; Wang, J.; Moss, S.C. High-energy x-ray diffraction study of pure amorphous silicon. Phys. Rev. B 1999, 19, 13520–13533. [Google Scholar] [CrossRef]
- Klein, O.; Nishina, Y. Über die Streuung von Strahlung durch freie Elektronen nach der neuen relativistischen Quantendynamik von Dirac. Z. Für Phys. 1929, 52, 853–868. [Google Scholar] [CrossRef]
- Narten, A.H.; Levy, H.A. Liquid Water: Molecular Correlation Functions from X-ray Diffraction. J. Chem. Phys. 1971, 55, 2263–2269. [Google Scholar] [CrossRef]
- Narten, A.H. X-ray diffraction pattern and models of liquid benzene. J. Chem. Phys. 1977, 67, 2102–2108. [Google Scholar] [CrossRef]
- Narten, A.H.; Habenschuss, A. Hydrogen bonding in liquid methanol and ethanol determined by x-ray diffraction. J. Chem. Phys. 1984, 80, 3387–3391. [Google Scholar] [CrossRef]
- Soper, A.K. Joint structure refinement of x-ray and neutron diffraction data on disordered materials: Application to liquid water. J. Phys. Condens. Matter 2007, 19, 335206. [Google Scholar] [CrossRef]
- Skinner, L.B.; Huang, C.; Schlesinger, D.; Pettersson, L.G.M.; Nilsson, A.; Benmore, C.J. Benchmark oxygen-oxygen pair-distribution function of ambient water from x-ray diffraction measurements with a wide Q-range. J. Chem. Phys. 2013, 138, 074506. [Google Scholar] [CrossRef]
- Keen, D.A. A comparison of various commonly used correlation functions for describing total scattering. J. Appl. Crystallogr. 2001, 34, 172–177. [Google Scholar] [CrossRef]
- Hannon, A.C.; Howells, W.S.; Soper, A.K. ATLAS: A Suite of Programs for the Analysis of Time-of-flight Neutron Diffraction Data from Liquid and Amorphous Samples. Inst. Phys. Conf. Ser. 1990, 107, 193–211. [Google Scholar]
- Egelstaff, P.A. An Introduction to the Liquid State; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- Benmore, C.J.; Alderman, O.L.G.; Robinson, D.S.; Jennings, G.; Tamalonis, A.; Ilavsky, J.; Clark, E.; Soignard, E.; Yarger, J.; Weber, J. Extended range X-ray pair distribution functions. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 955, 163318. [Google Scholar] [CrossRef]
- Winter, R.; Szornel, C.; Pilgrim, W.-C.; Howells, W.S.; Egelstaff, P.A.; Bodensteiner, T. The structural properties of liquid sulphur. J. Phys. Condens. Matter 1990, 2, 8427–8437. [Google Scholar] [CrossRef]
- Clark, G.N.I.; Hura, G.L.; Teixeira, J.; Soper, A.K.; Head-Gordon, T. Small-angle scattering and the structure of ambient liquid water. Proc. Natl. Acad. Sci. USA 2010, 107, 14003–14007. [Google Scholar] [CrossRef] [PubMed]
- Fine, R.A.; Millero, F.J. Compressibility of water as a function of temperature and pressure. J. Chem. Phys. 1973, 59, 5529–5536. [Google Scholar] [CrossRef]
- Fehér, F.; Hellwig, E. Beiträge zur Chemie des Schwefels, 45. Zur Kenntnis des flüssigen Schwefels. (Dichte, Ausdehnungskoeffizient und Kompressibilität). Z. Für Anorg. Und Allg. Chem. 1958, 294, 63–70. [Google Scholar] [CrossRef]
- Wright, A. Longer range order in single component network glasses? Phys. Chem. Glasses Eur. J. Glass Sci. Technol. Part B 2008, 49, 103–117. [Google Scholar]
- Soper, A.K.; Barney, E. On the use of modification functions when Fourier transforming total scattering data. J. Appl. Crystallogr. 2012, 45, 1314–1317. [Google Scholar] [CrossRef]
- Lorch, E. Neutron diffraction by germania, silica and radiation-damaged silica glasses. J. Phys. C Solid State Phys. 1969, 2, 229–237. [Google Scholar] [CrossRef]
- Krogh-Moe, J. A method for converting experimental X-ray intensities to an absolute scale. Acta Crystallogr. 1956, 9, 951–953. [Google Scholar] [CrossRef]
- Norman, N. The Fourier transform method for normalizing intensities. Acta Crystallogr. 1957, 10, 370–373. [Google Scholar] [CrossRef]
- Wright, A.C. Neutron scattering from vitreous silica. V. The structure of vitreous silica: What have we learned from 60 years of diffraction studies? J. Non-Cryst. Solids 1994, 179, 84–115. [Google Scholar] [CrossRef]
- Simmons, C.J.; El-Bayoumi, O.H. Experimental Techniques of Glass Science; Amer Ceramic Society: Columbus, OH, USA, 1993. [Google Scholar]
- Fischer, H.E.; Barnes, A.C.; Salmon, P.S. Neutron and x-ray diffraction studies of liquids and glasses. Rep. Prog. Phys. 2005, 69, 233–299. [Google Scholar] [CrossRef]
- Faber, T.E.; Ziman, J.M. A theory of the electrical properties of liquid metals. Philos. Mag. 1965, 11, 153–173. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Cryst. A 1976, 32, 751–766. [Google Scholar] [CrossRef]
- Shi, C. xINTERPDF: A graphical user interface for analyzing intermolecular pair distribution functions of organic compounds from X-ray total scattering data. J. Appl. Crystallogr. 2018, 51, 1498–1499. [Google Scholar] [CrossRef]
- McGreevy, R.L. Reverse Monte Carlo modelling. J. Phys. Condens. Matter 2001, 13, R877–R913. [Google Scholar] [CrossRef]
- McGreevy, R.L.; Pusztai, L. Reverse Monte Carlo Simulation: A New Technique for the Determination of Disordered Structures. Mol. Simul. 1988, 1, 359–367. [Google Scholar] [CrossRef]
- Soper, A.K. Empirical potential Monte Carlo simulation of fluid structure. Chem. Phys. 1996, 202, 295–306. [Google Scholar] [CrossRef]
- Soper, A.K. Tests of the empirical potential structure refinement method and a new method of application to neutron diffraction data on water. Mol. Phys. 2001, 99, 1503–1516. [Google Scholar] [CrossRef]
- Coelho, A. TOPAS and TOPAS-Academic: An optimization program integrating computer algebra and crystallographic objects written in C++. J. Appl. Crystallogr. 2018, 51, 210–218. [Google Scholar] [CrossRef]
- Coelho, A.A.; Chater, P.A.; Kern, A. Fast synthesis and refinement of the atomic pair distribution function. J. Appl. Crystallogr. 2015, 48, 869–875. [Google Scholar] [CrossRef]
- Farrow, C.L.; Juhas, P.; Liu, J.W.; Bryndin, D.; Božin, E.S.; Bloch, J.; Proffen, T.; Billinge, S.J.L. PDFfit2 and PDFgui: Computer programs for studying nanostructure in crystals. J. Phys. Condens. Matter 2007, 19, 335219. [Google Scholar] [CrossRef]
- Skinner, L.B.; Benmore, C.J.; Weber, J.K.R.; Du, J.; Neuefeind, J.; Tumber, S.K.; Parise, J.B. Low Cation Coordination in Oxide Melts. Phys. Rev. Lett. 2014, 112, 157801. [Google Scholar] [CrossRef] [PubMed]
- Soper, A.K. Network structure and concentration fluctuations in a series of elemental, binary, and tertiary liquids and glasses. J. Phys. Condens. Matter 2010, 22, 404210. [Google Scholar] [CrossRef] [PubMed]
- Hammersley, A.P.; Svensson, S.O.; Hanfland, M.; Fitch, A.N.; Hausermann, D. Two-dimensional detector software: From real detector to idealised image or two-theta scan. High Press. Res. 1996, 14, 235–248. [Google Scholar] [CrossRef]
- Qiu, X.; Thompson, J.W.; Billinge, S.J.L. PDFgetX2: A GUI-driven program to obtain the pair distribution function from X-ray powder diffraction data. J. Appl. Crystallogr. 2004, 37, 678. [Google Scholar] [CrossRef]
- Toby, B.H.; Von Dreele, R.B. GSAS-II: The genesis of a modern open-source all purpose crystallography software package. J. Appl. Crystallogr. 2013, 46, 544–549. [Google Scholar] [CrossRef]
- Juhás, P.; Davis, T.; Farrow, C.L.; Billinge, S.J.L. PDFgetX3: A rapid and highly automatable program for processing powder diffraction data into total scattering pair distribution functions. J. Appl. Crystallogr. 2013, 46, 560–566. [Google Scholar] [CrossRef]
- Heinen, B.J.; Drewitt, J.W.E. LiquidDiffract: Software for liquid total scattering analysis. Phys. Chem. Miner. 2022, 49, 9. [Google Scholar] [CrossRef]
- Soper, A.K.; Barney, E.R. Extracting the pair distribution function from white-beam X-ray total scattering data. J. Appl. Crystallogr. 2011, 44, 714–726. [Google Scholar] [CrossRef]
- Kohara, S.; Itou, M.; Suzuya, K.; Inamura, Y.; Sakurai, Y.; Ohishi, Y.; Takata, M. Structural studies of disordered materials using high-energy x-ray diffraction from ambient to extreme conditions. J. Phys. Condens. Matter 2007, 19, 506101. [Google Scholar] [CrossRef]
- Egami, T.; Billinge, S.J.L. Underneath the Bragg Peaks: Structural Analysis of Complex Materials; Elsevier: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Ruland, W. The separation of coherent and incoherent Compton X-ray scattering. Br. J. Appl. Phys. 1964, 15, 1301–1307. [Google Scholar] [CrossRef]
- Yang, X.; Juhas, P.; Farrow, C.L.; Billinge, S.J. xPDFsuite: An end-to-end software solution for high throughput pair distribution function transformation, visualization and analysis. arXiv 2014, arXiv:1402.3163. [Google Scholar]
- Eggert, J.H.; Weck, G.; Loubeyre, P.; Mezouar, M. Quantitative structure factor and density measurements of high-pressure fluids in diamond anvil cells by x-ray diffraction: Argon and water. Phys. Rev. B 2002, 65, 174105. [Google Scholar] [CrossRef]
- Soper, A.K. Inelasticity corrections for time-of-flight and fixed wavelength neutron diffraction experiments. Mol. Phys. 2009, 107, 1667–1684. [Google Scholar] [CrossRef]
- Igor Pro, Version 8; WaveMetrics, Inc.: Lake Oswego, OR, USA. Available online: http://www.wavemetrics.com (accessed on 1 May 2023).
- Hart, R.T.; Benmore, C.J.; Neuefeind, J.; Kohara, S.; Tomberli, B.; Egelstaff, P.A. Temperature Dependence of Isotopic Quantum Effects in Water. Phys. Rev. Lett. 2005, 94, 047801. [Google Scholar] [CrossRef]
- Ohara, K.; Onodera, Y.; Murakami, M.; Kohara, S. Structure of disordered materials under ambient to extreme conditions revealed by synchrotron x-ray diffraction techniques at SPring-8—Recent instrumentation and synergic collaboration with modelling and topological analyses. J. Phys. Condens. Matter 2021, 33, 383001. [Google Scholar] [CrossRef]
- Sasaki, S. KEK Report 83-22, 88-14; National Laboratory for High Energy Physics: Tsukuba, Japan, 1984; Volume 1989. [Google Scholar]
- Cromer, D.T.; Mann, J.B. Compton Scattering Factors for Spherically Symmetric Free Atoms. J. Chem. Phys. 1967, 47, 1892–1893. [Google Scholar] [CrossRef]
- Waseda, Y. The Structure of Non-Crystalline Materials: Liguids and Amorphous Solids; Mcgraw-Hill Publishing: New York, NY, USA, 1980. [Google Scholar]
- Press, W. Numerical Recipes in Fortran 77: The Art of Scientific Computing; Cambridge University Press: New York, NY, USA, 1992. [Google Scholar]
- Soper, A.K. GudrunN and GudrunX: Programs for Correcting Raw Neutron and X-ray Diffraction Data to Differential Scattering Cross Section; Science & Technology Facilities Council: Swindon, UK, 2010. [Google Scholar]
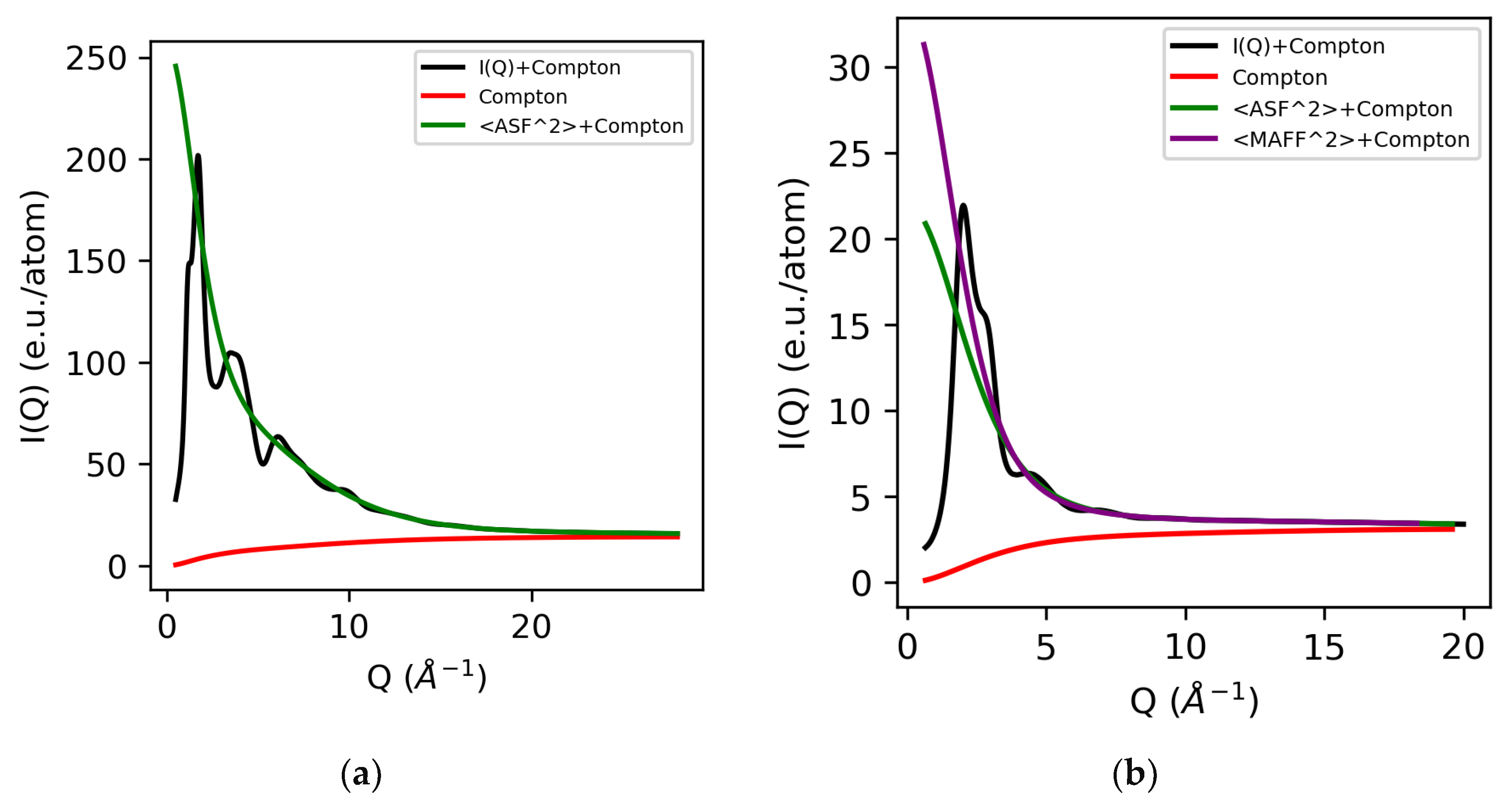
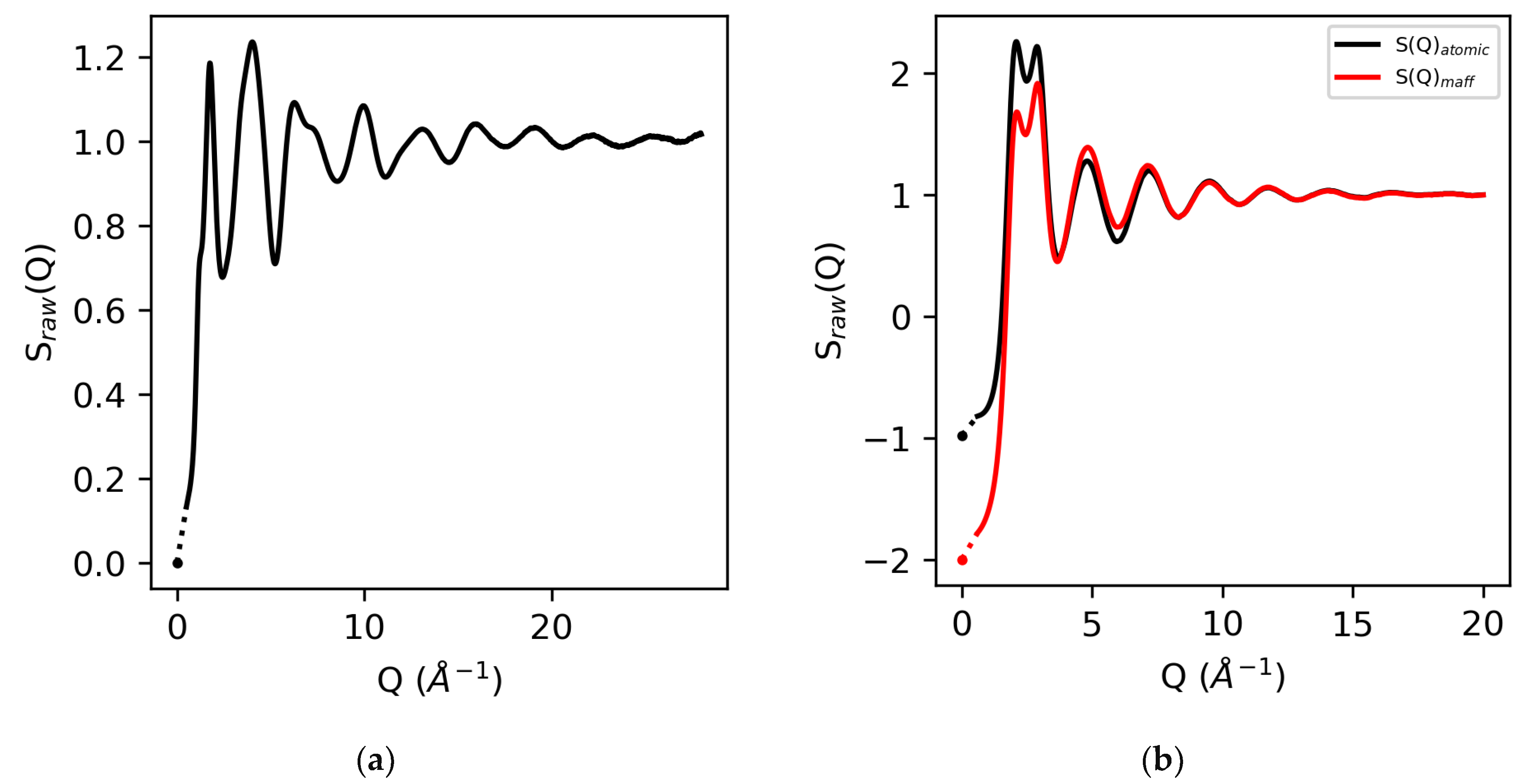
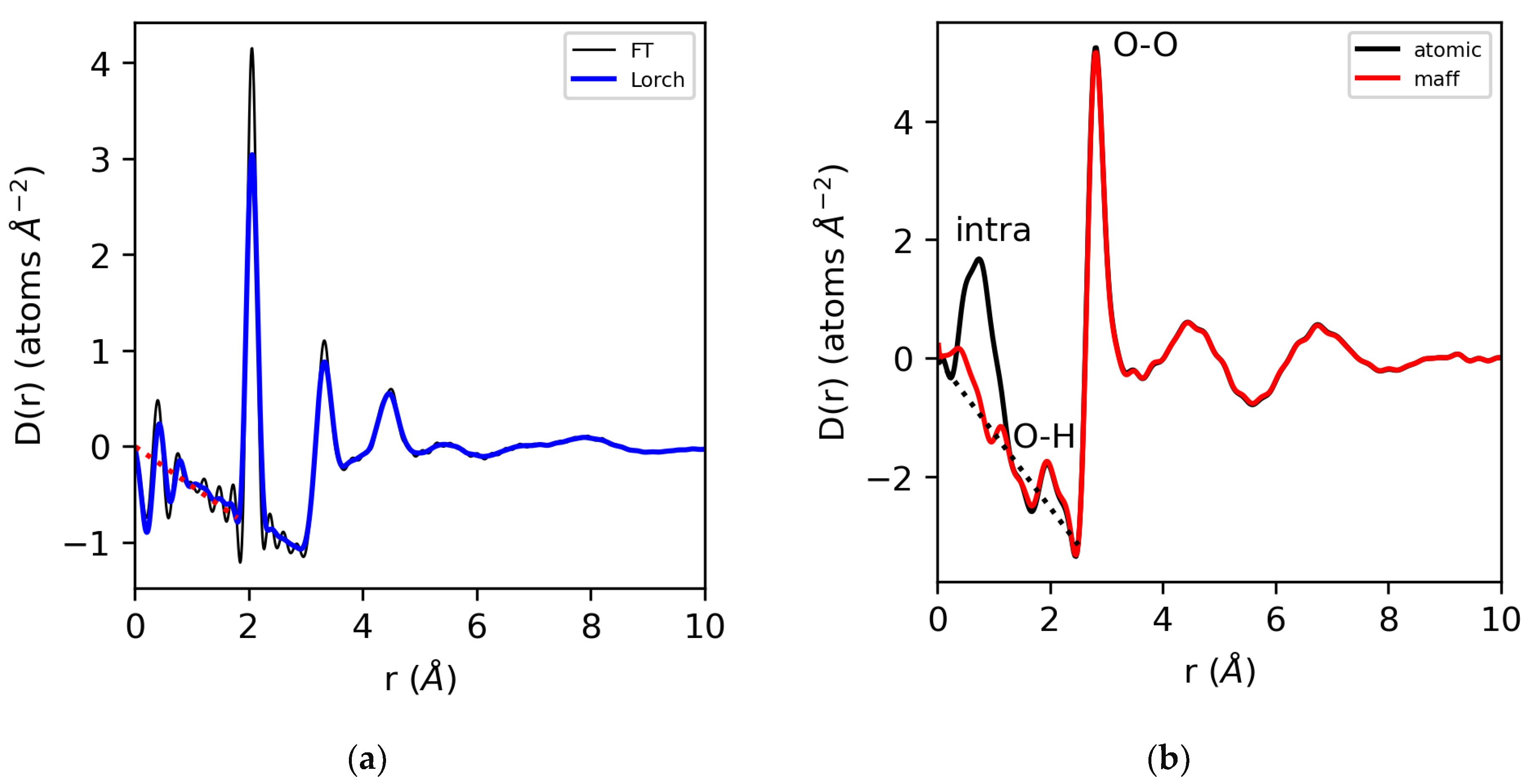
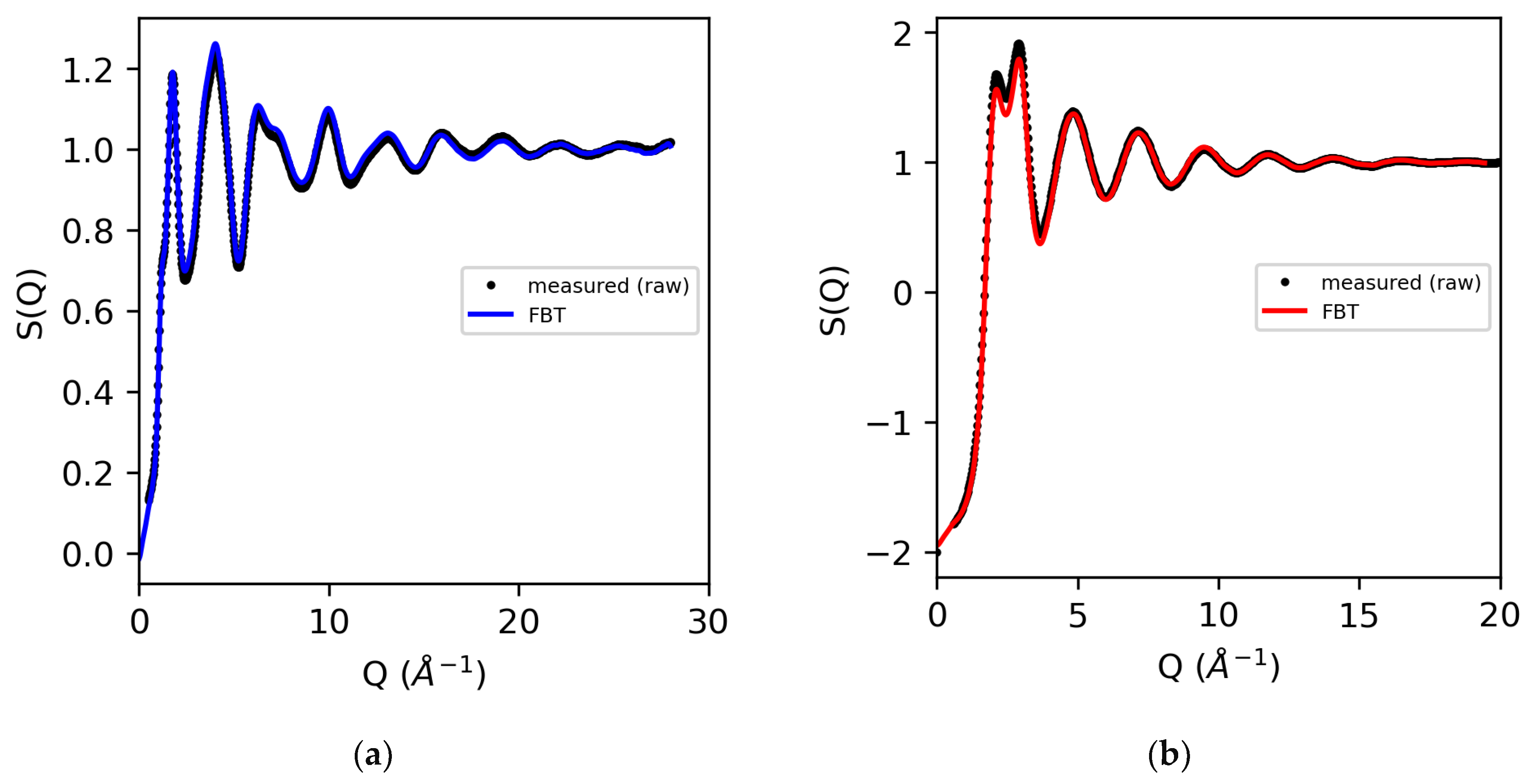
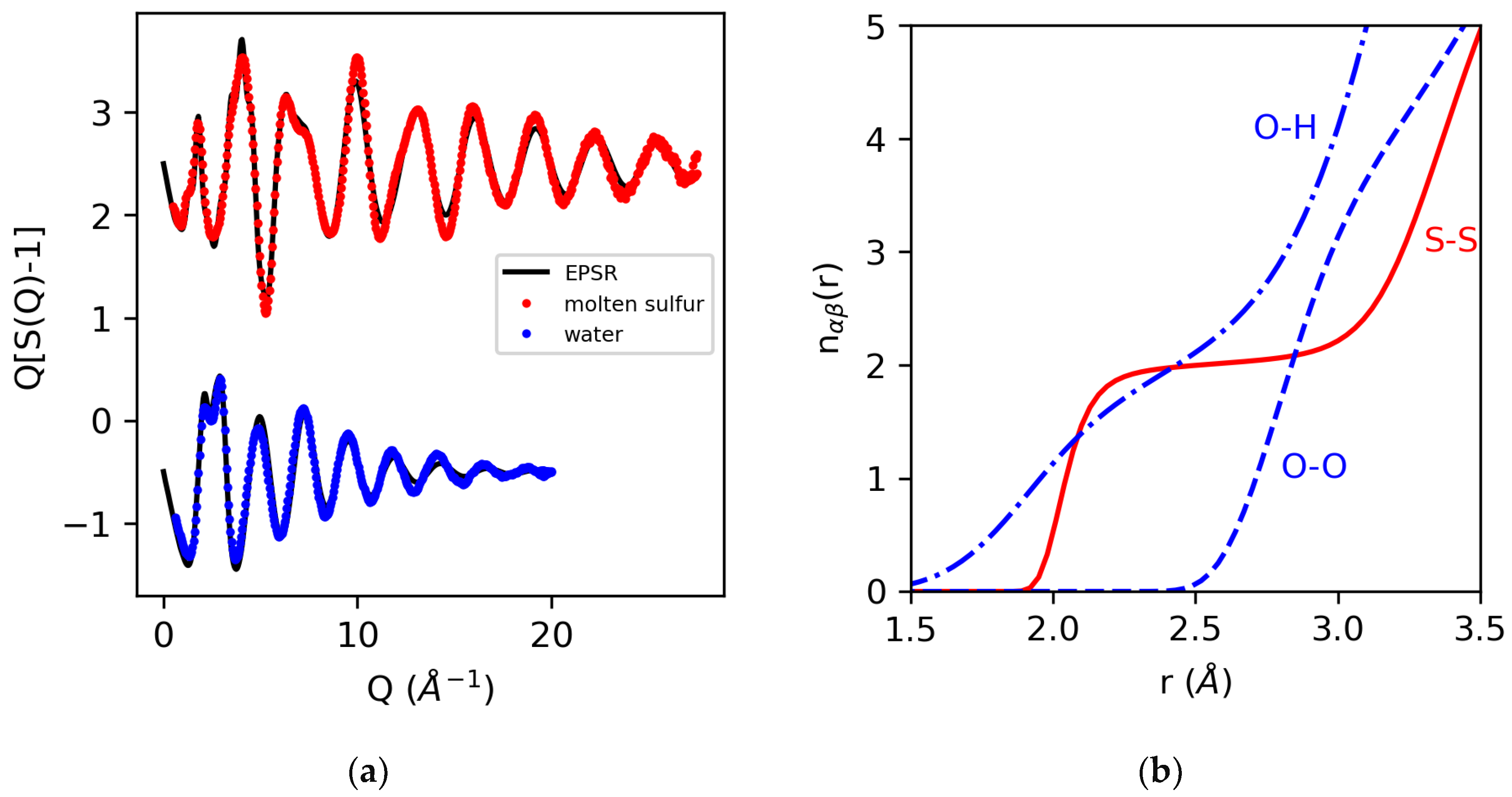
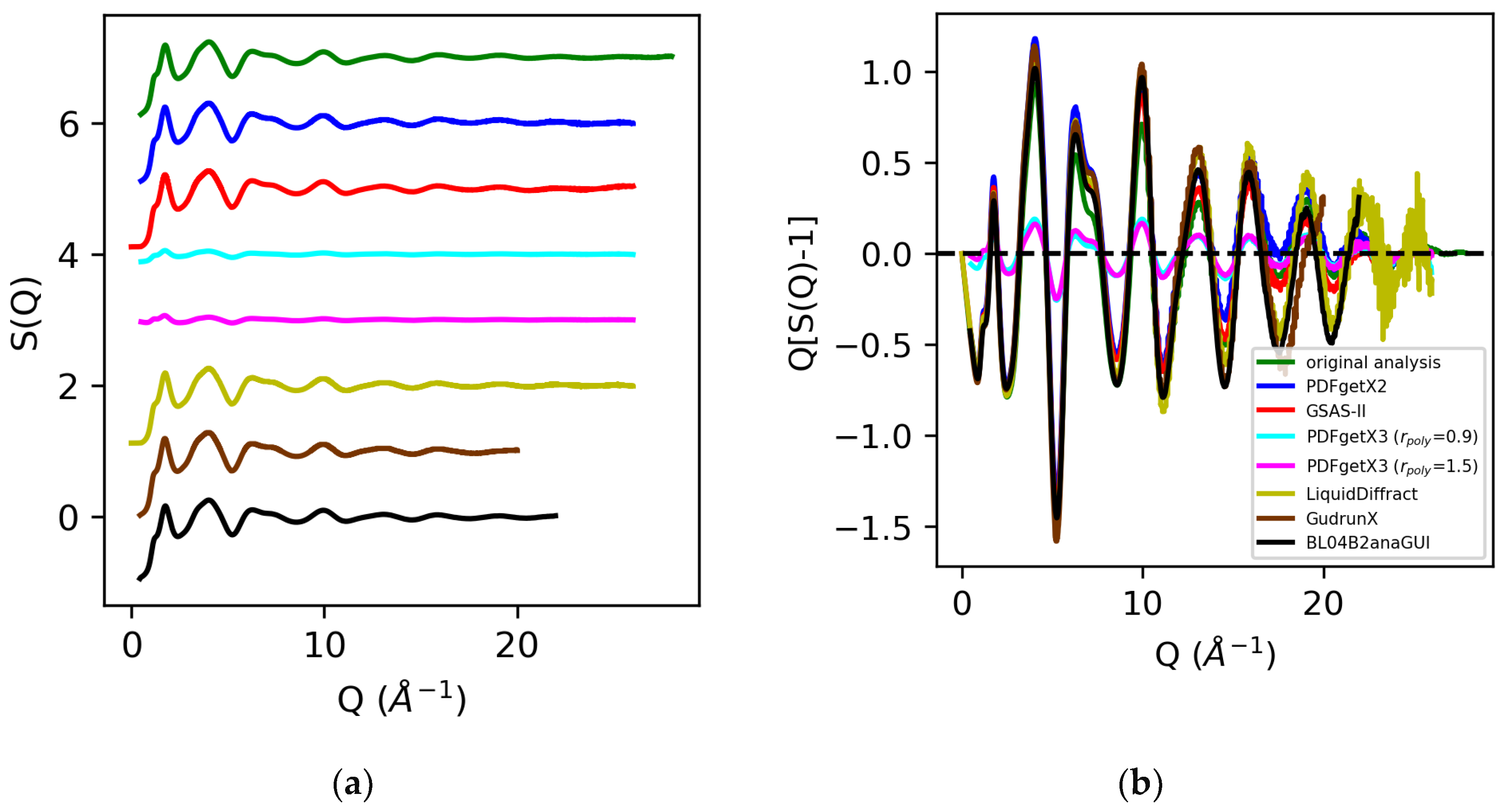
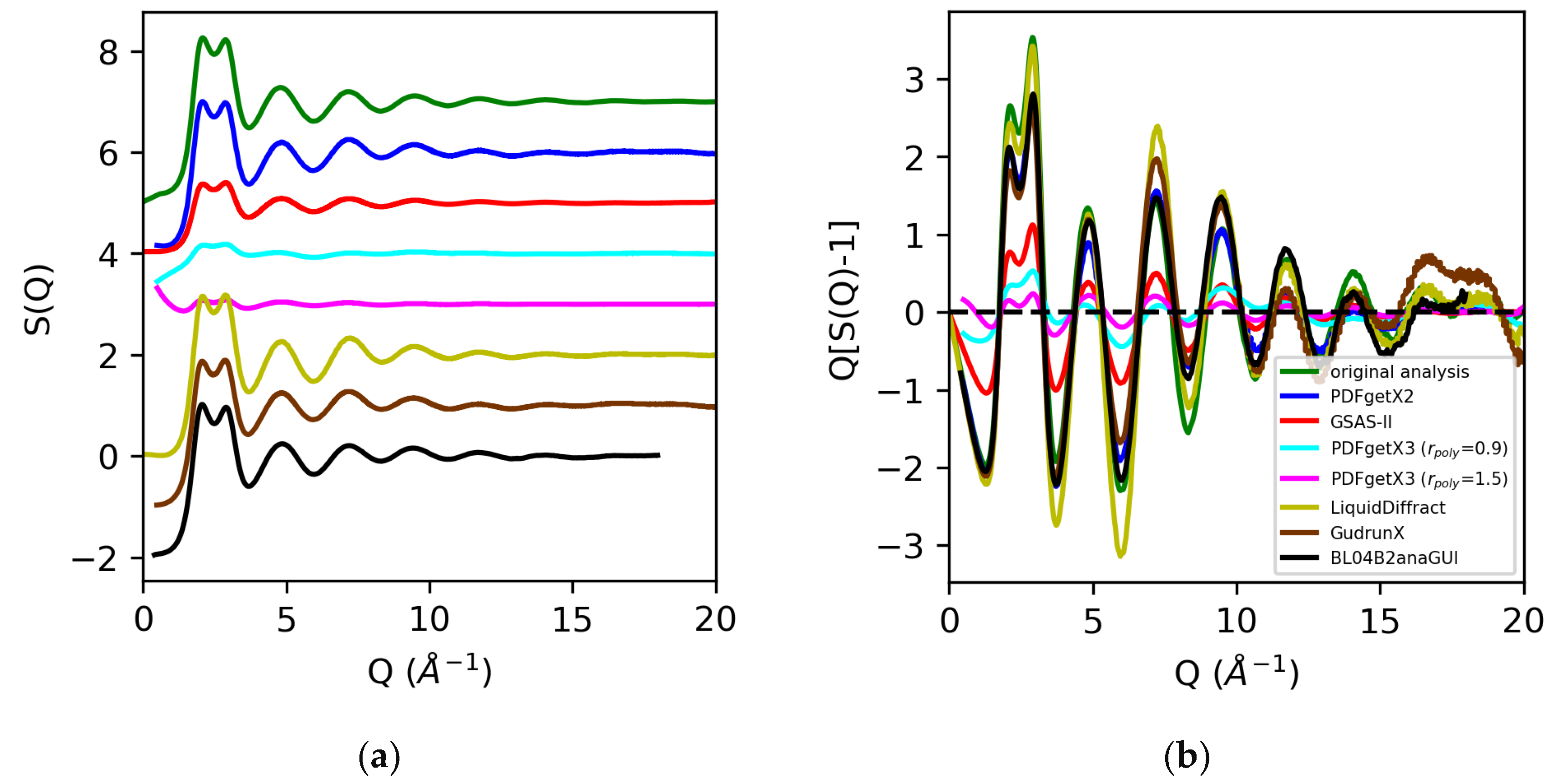
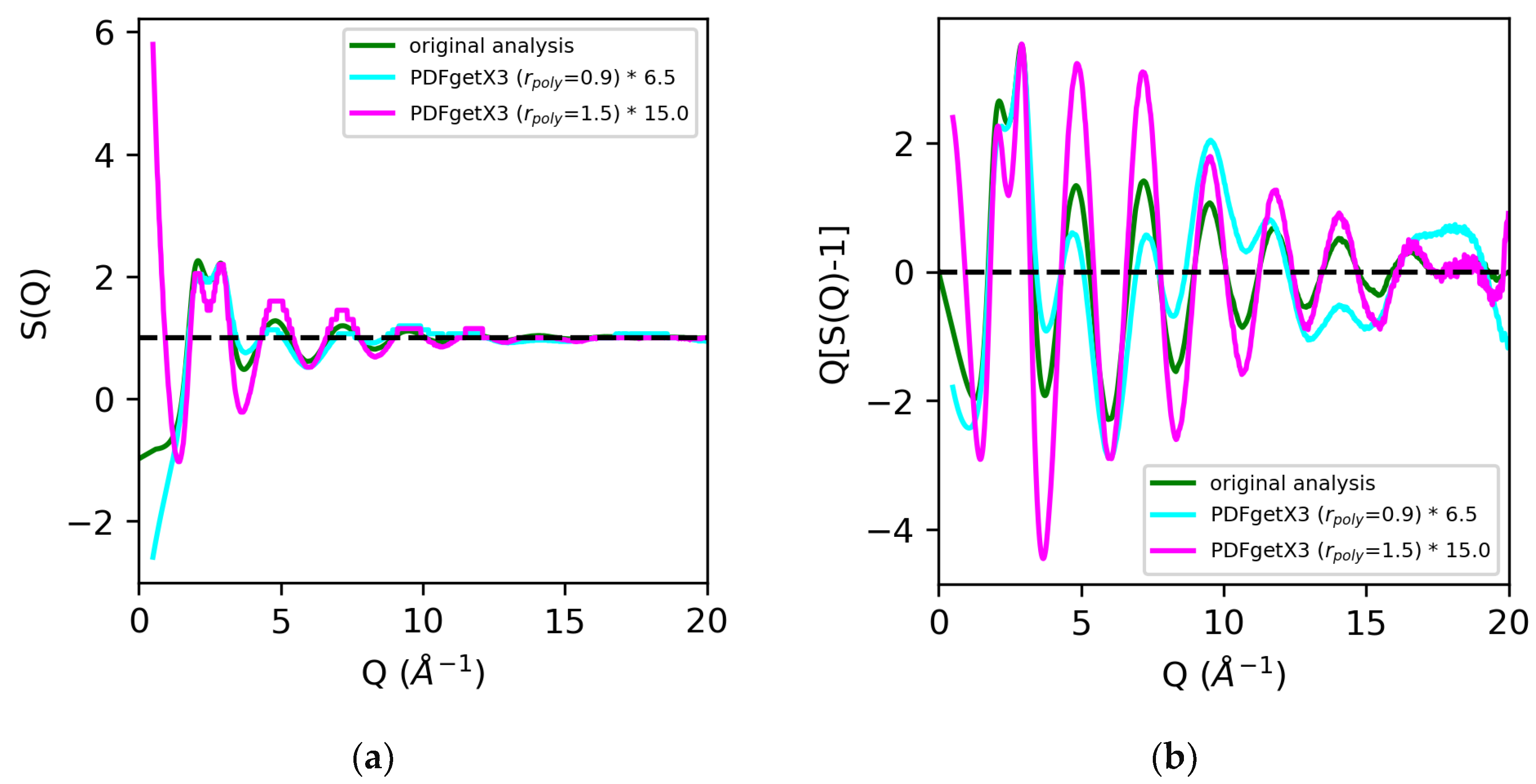


| Program | rSS(1) (Å) | nSS(1) (1.80–2.30 Å) | rSS(2) (Å) | nSS(2) (3.00–3.60 Å) | rSS(3) (Å) | nSS(3) (4.20–4.74 Å) |
|---|---|---|---|---|---|---|
| Original analysis | 2.05 | 1.88 | 3.32 | 3.13 | 4.46 | 5.54 |
| PDFgetX2 | 2.06 | 2.01 | 3.33 | 3.24 | 4.46 | 5.75 |
| GSAS-II | 2.05 | 1.9 | 3.34 | 3.06 | 4.47 | 5.52 |
| LiquidDiffract | 2.07 | 1.9 | 3.3 | 3.13 | 4.45 | 5.98 |
| GudrunX | 2.04 | 1.92 | 3.32 | 3.06 | 4.44 | 5.55 |
| BL04B2anaGUI | 2.06 | 1.91 | 3.33 | 3.20 | 4.46 | 5.64 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gallington, L.C.; Wilke, S.K.; Kohara, S.; Benmore, C.J. Review of Current Software for Analyzing Total X-ray Scattering Data from Liquids. Quantum Beam Sci. 2023, 7, 20. https://doi.org/10.3390/qubs7020020
Gallington LC, Wilke SK, Kohara S, Benmore CJ. Review of Current Software for Analyzing Total X-ray Scattering Data from Liquids. Quantum Beam Science. 2023; 7(2):20. https://doi.org/10.3390/qubs7020020
Chicago/Turabian StyleGallington, Leighanne C., Stephen K. Wilke, Shinji Kohara, and Chris J. Benmore. 2023. "Review of Current Software for Analyzing Total X-ray Scattering Data from Liquids" Quantum Beam Science 7, no. 2: 20. https://doi.org/10.3390/qubs7020020
APA StyleGallington, L. C., Wilke, S. K., Kohara, S., & Benmore, C. J. (2023). Review of Current Software for Analyzing Total X-ray Scattering Data from Liquids. Quantum Beam Science, 7(2), 20. https://doi.org/10.3390/qubs7020020







