A Methodology for Reconstructing DSET Pulses from Heavy-Ion Broad-Beam Measurements

Abstract

A table-based method for the estimation of heavy-ion-induced Digital Single Event Transient (DSET) voltage pulse-width in a single logic cell has been developed. The estimation method is based on the actual heavy-ion-induced transient current data in a single metal-oxide-semiconductor field effect transistor (MOSFET) used in the logic cell. The DSET pulse waveform in an inverter is obtained from which the pulse-width was estimated to be 420 ps. This DSET pulse-width value (420 ps) falls within the reasonable range of the DSET pulse-width distribution measured by the self-triggering flip-flop latch chain under heavy-ion irradiation test conditions.

1. Introduction

Digital single event transient (DSET) pulses are momentary glitch noises generated at logic gates by incident ions. They can dominate the heavy-ion-induced soft-error response of modern digital logic very-large-scale-integrations (VLSIs) [1]. Since the pulse-width (duration) of DSETs is a key parameter in determining the soft error rates in logic VLSIs, the DSET pulse-widths have been extensively measured by using logic cell chains, and specially built pulse capture circuits such as variable temporal latches, self-triggering latches/flip-flops, and high drive-capability output-buffers [2,3,4,5,6,7,8,9,10]. These circuits allow us to observe the widths of the DSET pulse originating in actual logic cells under ion irradiation test conditions. The heavy-ion-induced DSET pulse-widths and the DSET cross-sections in 0.2 µm fully-depleted silicon-on-insulator (FD-SOI) process inverter cells and NOR cells by using the on-chip self-triggering flip-flop latch chain named Snapshot has also been measured [3,7,9,10].

On the other hand, an analytical estimation method has been proposed to obtain the DSET pulse waveform generated in a logic cell from transient currents in a single MOSFET used in the logic cells [11,12,13]. This method uses radiation-induced transient current waveforms from the off-state single MOSFET under various voltage conditions. The measured and/or simulated transient current waveforms are stored in a look-up table, and the DSET pulses are estimated within a table-based circuit simulation framework. For example, an FD-SOI process inverter cell is composed of an n-type MOSFET (nMOSFET) and a p-type MOSFET (pMOSFET). Thus, we can calculate the time variation of the logic out-put voltage from the time profile of the transient current with various supply bias conditions and static I-V characteristics of the other MOSFET in the inverter cell. By using this table-based estimation method, we can obtain realistic waveforms of the DSET pulses without the need of any external circuit such as variable temporal latches, self-triggering latches/flip-flops, and high drive-capability output-buffers. The estimation method has been verified by a comparison between the DSET pulse waveform estimated by using a simulated transient current waveform and the pulse-laser-induced DSET pulse waveform in an inverter cell measured by monitoring transistors [14]. In other words, we can obtain realistic waveforms of the DSET pulses without the need of any irradiation experiments.

Since heavy-ions create actual initial charge (electron-hole pairs) distributions in MOSFETs, verification of the heavy-ion induced response based DEST pulse waveform estimation method has also been required. However, actual heavy-ion induced transient current waveforms from a single MOSFET have never been applied to the table-based estimation due to the uncertainty associated with the location of an ion strike within the MOSFET in the ion irradiation experiments. In principle, the transient current waveforms used in the table-based estimation method have to originate at the same location in the MOSFET under all voltage conditions.

In this paper, we used the table-based estimation method to estimate the heavy-ion-induced DSET pulse-widths in a single inverter (NOT) cell. We present a methodology for DSET pulse-width estimation from heavy-ion-induced current waveforms with various drain biases in a single n-type MOSFET. Then, we verify the pulse-width and our methodology by comparing our result to the previously measured DSET pulse-widths with a Snapshot circuit (self-triggering flip-flop latch chain).

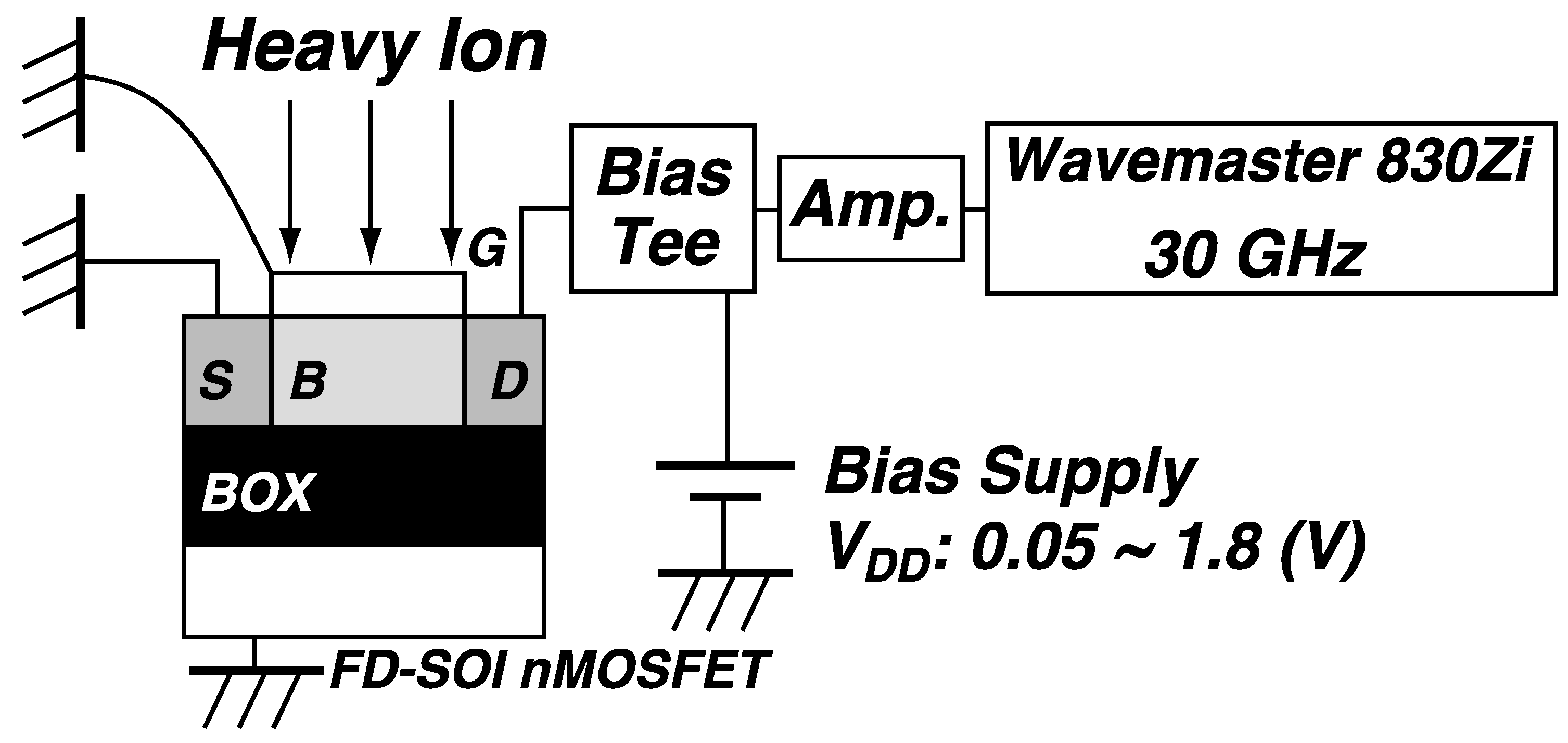
2. Experimental

Figure 1 shows a schematic view of the experimental setup used in this study. The nMOSFET was fabricated by using a 0.2 µm FD-SOI process [15]. The nMOSFET was mounted on a chip carrier with four strip-lines. Each electrode was connected to the corresponding strip-lines with short bonding wires. The gate width of the nMOSFET was 1.56 µm. The nMOSFET was configured as it is in an inverter and was used with self-triggering flip-flop latch chains [3,7,9]. The nominal supply voltage of the process was 1.8 V. The transistor was biased in an off-state where its gate, source, and back-contact were grounded. Transient drain currents induced (I_Dn) by heavy-ion irradiation were measured with various drain biases. The drain’s voltage was varied from 1.8 V to 0.05 V with a power supply. Moreover, the drain bias was swept from 1.8 V to 0.2 V in 0.2 V steps, and from 0.2 V to 0.05 V in 0.05 V steps. Around 150–200 of transient current signals were collected at each drain bias condition. Unlike the tests with laser irradiation where a repetitive signal due to the same irradiation location can be generated and measured by a sampling oscilloscope, a single-shot oscilloscope was required for this study. Thus, we used a 30 GHz high-bandwidth single-shot digital oscilloscope (Model Wavemaster 830Zi) to measure the transient drain currents. It should be noted that the bias-tee, amplifier, and cables limited the bandwidth of the measurement system to 13.5 GHz in this study. The drain transient currents were amplified by a linear pre-amplifier with 21.5 dB before 50-Ω input to the oscilloscope.

The heavy-ion irradiation tests were performed using the azimuthally varying field (AVF) cyclotron at the Takasaki Ion Accelerators for Advanced Radiation Application (TIARA), National Institutes for Quantum and Radiological Science and Technology (QST), Takasaki. The test transistor was irradiated in a vacuum chamber with a broad beam of 322 MeV Krypton (Kr) from a direction perpendicular to the SOI layer to achieve an LET (liner energy transfer) of 40 MeV-cm²/mg at the SOI surface. We assumed that the LET was constant in the SOI layer, since the projected ion range in the Si was 41 µm as evaluated by a SRIM (Stopping and Range of Ion in Matter) calculation [16] and is enough to penetrate the very thin SOI layer without significant energy losses. The beam flux was measured by using a Si solid state detector, and controlled to the same flux as the DSET pulse-widths measurement in [9].

3. Results and Discussion

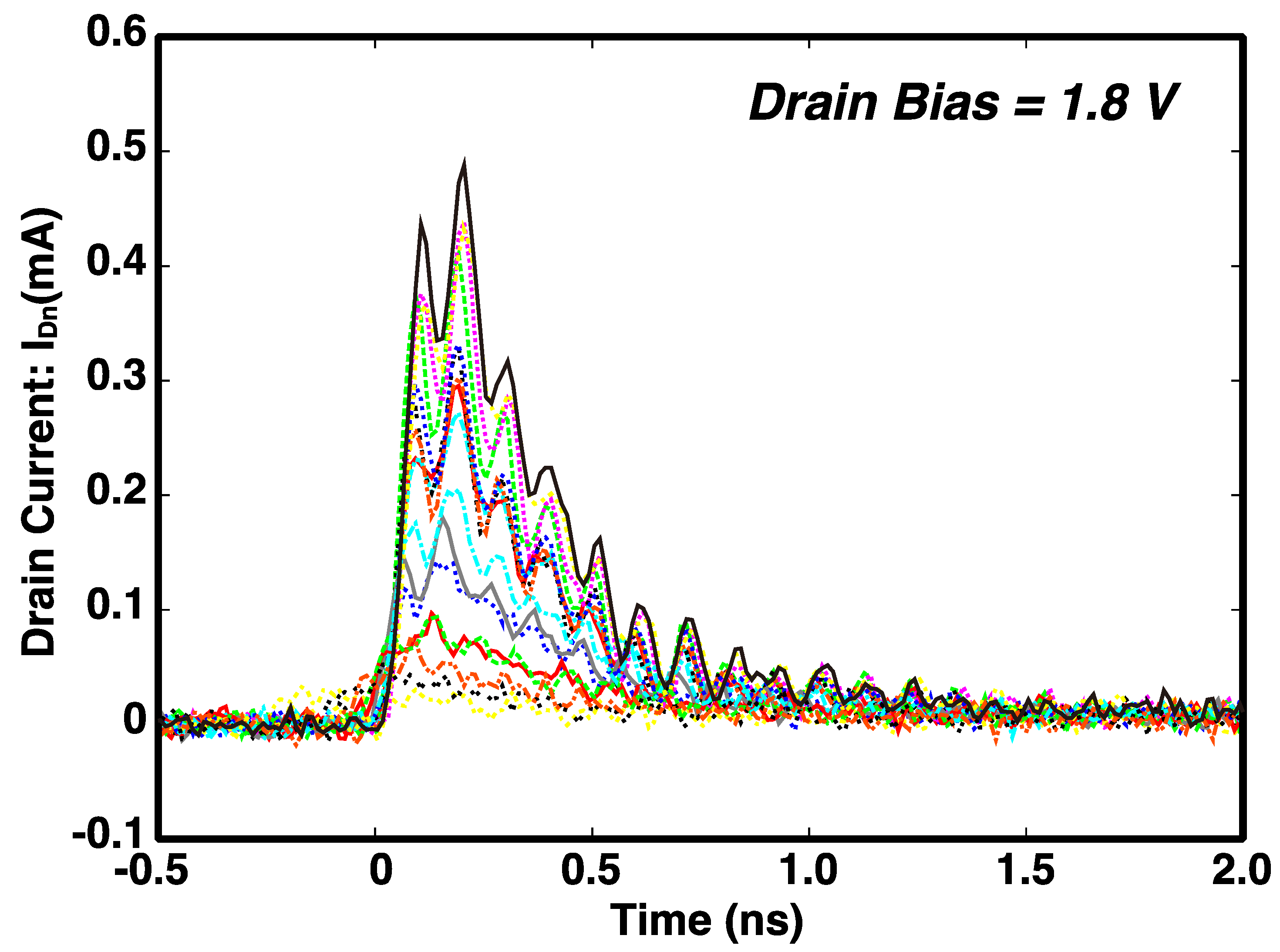
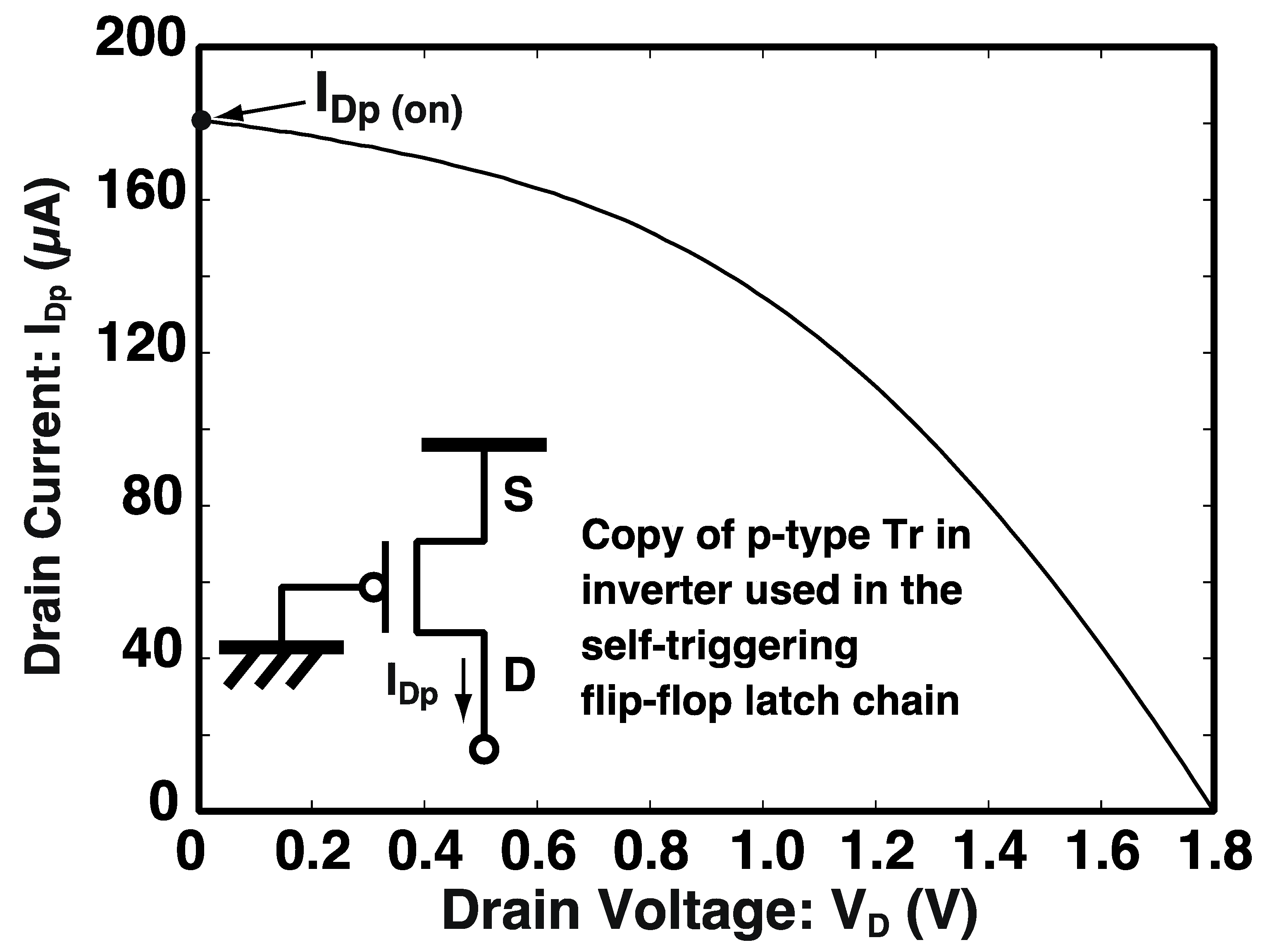
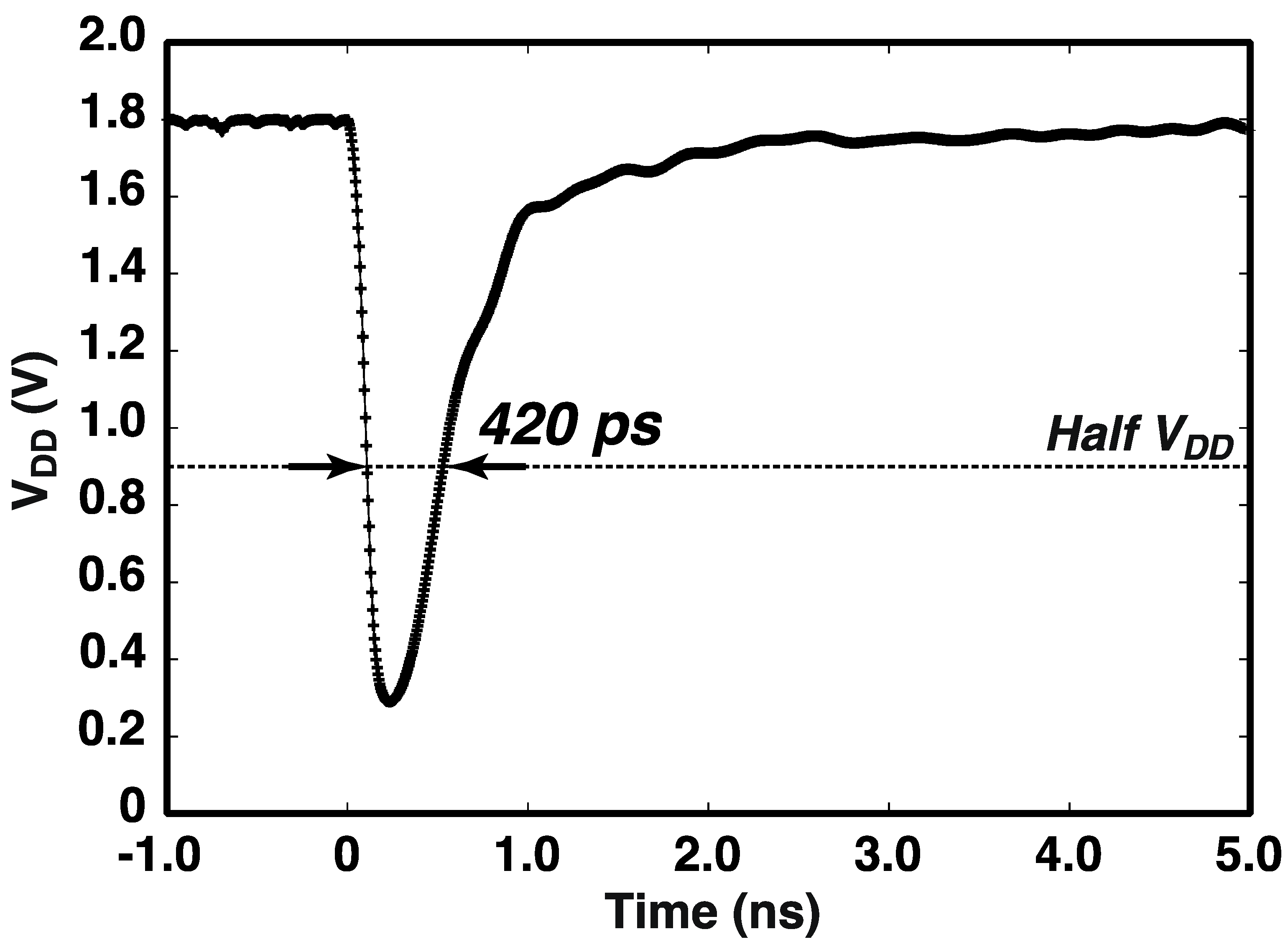
3.1. Experimental Results

Figure 2 shows an example of the measured drain current waveforms at the drain bias of 1.8 V in the experiment. These sampled waveforms from all the measured drain current waveforms were plotted with colors to distinguish each waveform easily. The recorded waveforms exhibited fast rise times and exponentially decaying tails. The measured waveforms showed that the transient current response (pulse-height and decay time) varied even under constant-LET ion irradiation. This result can be explained by the fact that the current waveform varies depending on the location of the ion strikes in the transistor such as a gate hit or a drain hit as is the case for PD-SOI transistors [17,18]. The transient current was not recorded in the case of non-biased nMOSFET by ion incident, since no current pulses were measurable under this condition.

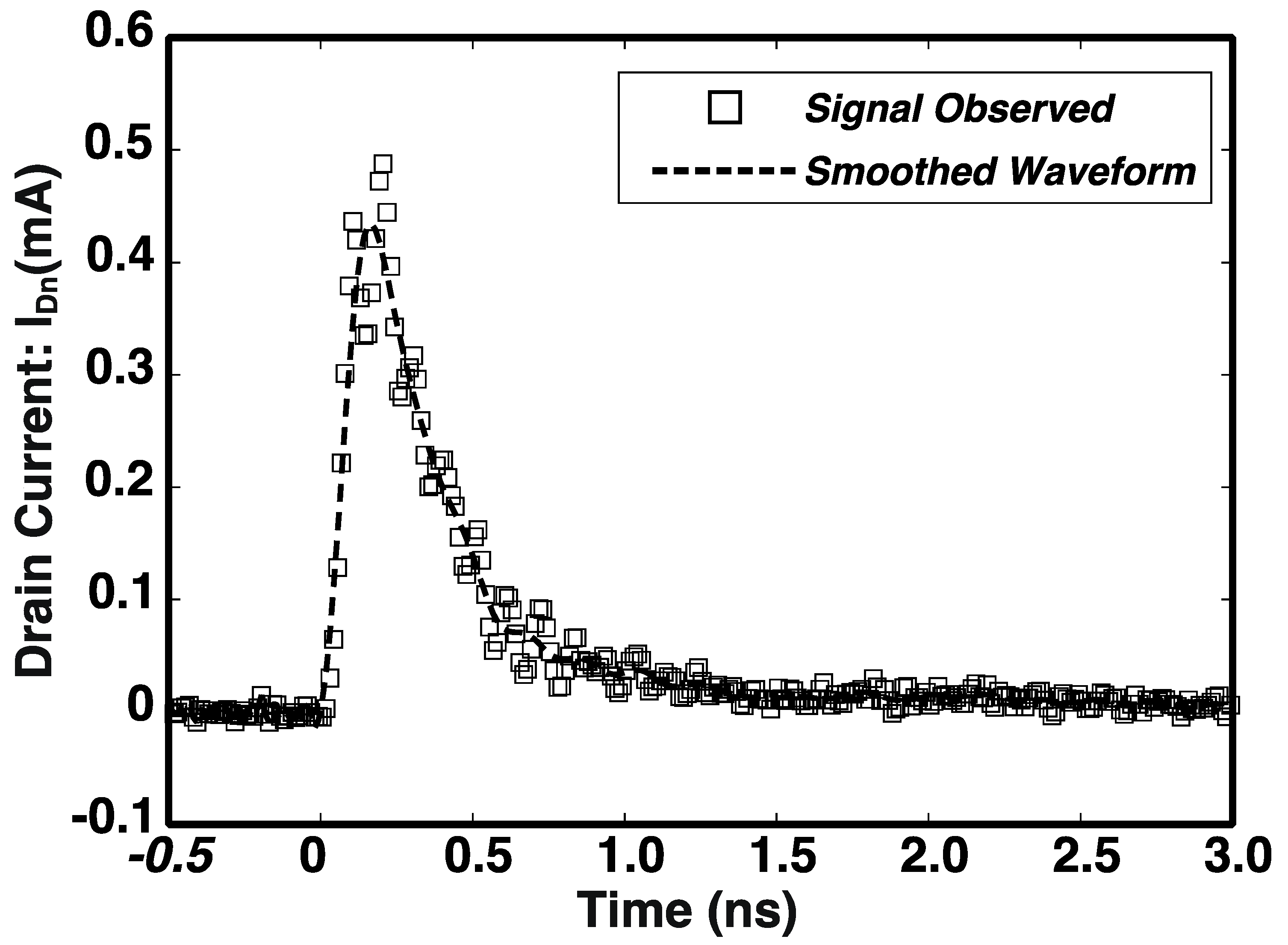
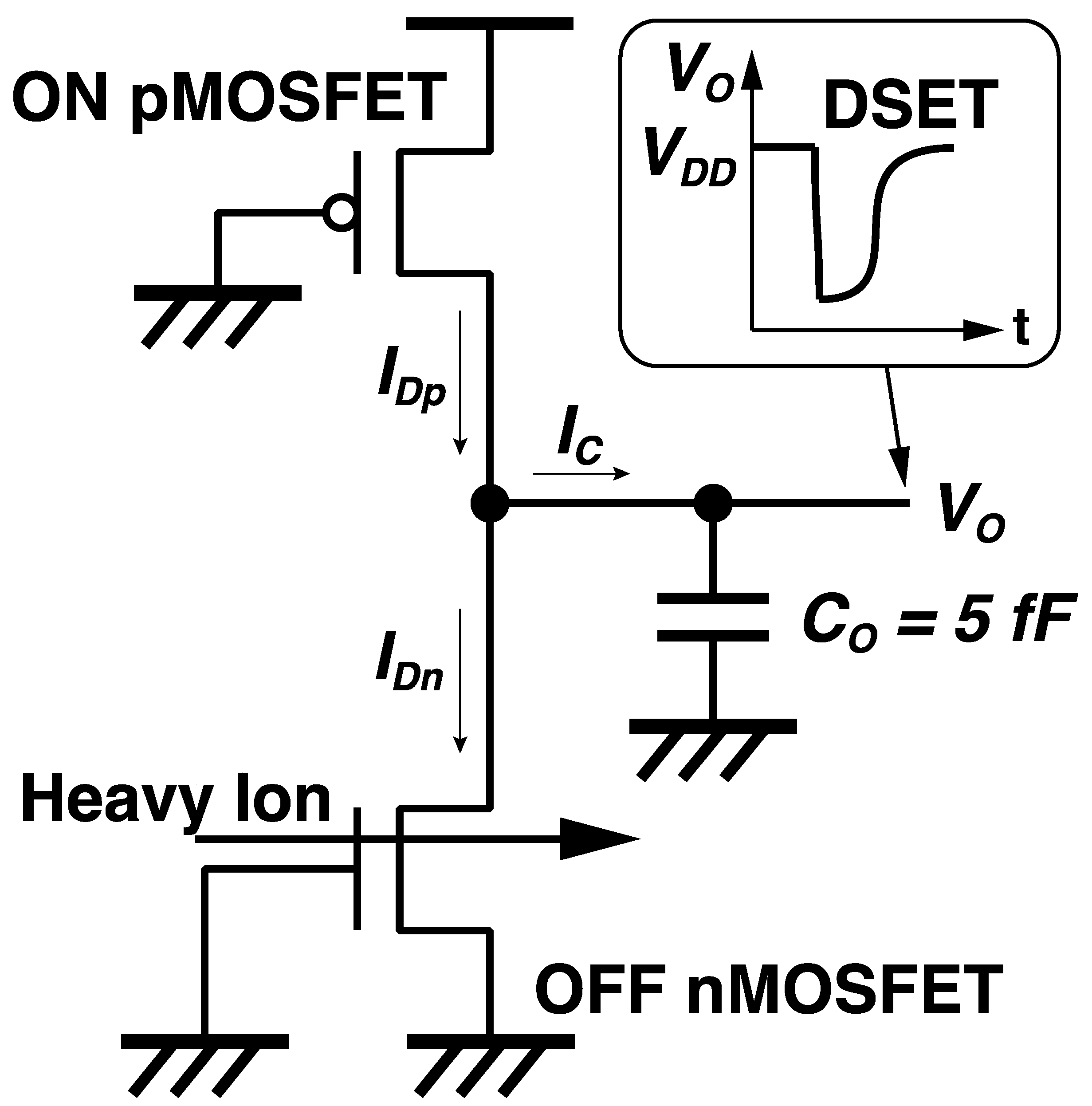
3.2. DSET Pulse-Width Estimation Methodology

As above-mentioned, the DSET pulse-width estimation method ideally requires the transient current waveforms to originate from the same strike location of ions in the MOSFET. However, in this study, it was not possible to obtain information on the relationship between the ion strike locations and the obtained current waveforms due to the use of a broad beam from the accelerator.

A correlation between the collected charge from a nominal biased MOSFET and the DSET pulse-widths in SOI devices was implied in [17]. Thus, we extracted typical waveforms that had the greatest collected charge in each drain bias condition from the random transient current waveforms to estimate a typical DSET pulse waveform. Collected charges were calculated by a numerical integration of the recorded transient current waveforms. Figure 3 shows the extracted waveform from heavy-ion induced drain current waveforms, which had the greatest collected charge at the drain bias of 1.8 V. The waveform corresponds to the waveform plotted as the black solid line in Figure 2. The measured waveform was also smoothed by the fast Fourier transform (FFT) method to minimize the effect of electronic noise. The smoothed waveform is also shown in Figure 3 (broken line). For each drain bias condition, the smoothed transient current was derived in the same way.

Figure 4 shows an equivalent circuit model of an inverter for the table-based estimation method (see [11,12,13,14] for further details of the table-based approach). The nMOSFET and the pMOSFET are modeled in the same configuration as it is used in an inverter connected to a self-triggering flip-flop latch chain to compare the estimated and the measured DSET pulse-widths. Figure 5 shows the measured statistic current-voltage characteristic of the actual pMOSFET. The gate width of the pMOSFET was 2.16 µm. We modeled the output capacitance C_O with a constant capacitance as a next stage inverter model. The value of C_O was theoretically estimated to be 5 fF from the design parameter of the inverter used in the self-triggering flip-flop latch chain. The DSET pulse waveform at V_O was determined from the table-based estimation method by using the measured current waveforms in the nMOSFET and the current-voltage characteristic of the pMOSFET.

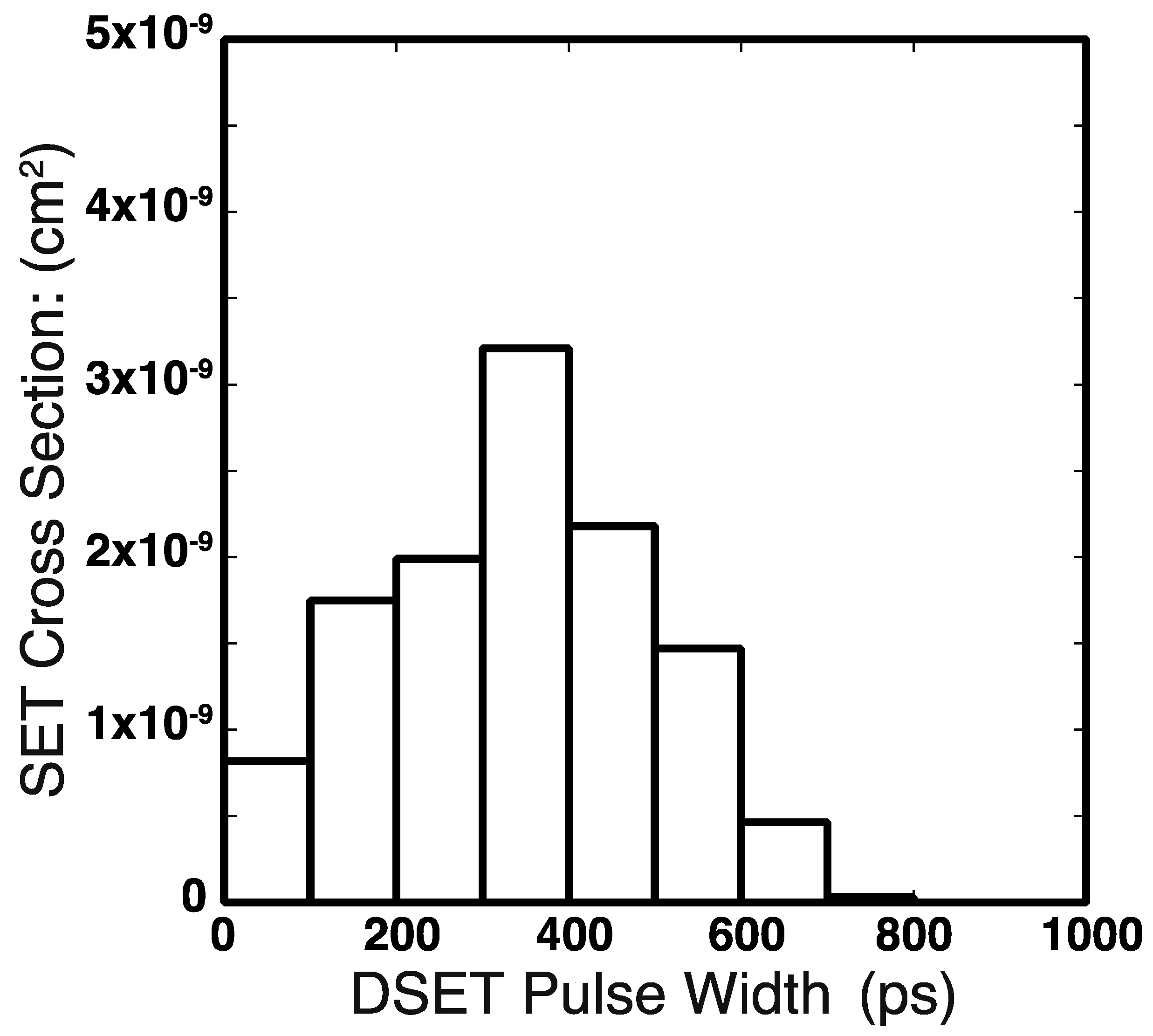
3.3. DSET Pulse-Width Estimation Result

Figure 6 shows an estimated DSET waveform by using the table-based estimation method. This DSET pulse waveform shows the typical characteristics as the estimated waveforms in [14] (e.g., fast falling edge and slow rising tail). This result indicates that our selection method of the current pulses can reconstruct the typical DSET waveforms. The DSET pulse-width is defined at half V_DD (drain voltage) of the transient voltage and was estimated to be 420 ps. The DSET pulse-width determined by the table-based estimation method was compared with the DSET pulse-widths measured by using the self-triggering flip-flop latch chain. Figure 7 shows the DSET cross section (frequency) from a single inverter cell measured by using the self-triggering flip-flop latch chain in [9]. The cross section was plotted as a function of the measured DSET pulse-width in the range up to 750 ps with a peak value at 350 ps. The DSET pulse-width of 420 ps estimated in this study fell within the range from the peak to the worst (longest) pulse-widths in the measured distribution by the self-triggering flip-flop latch chain. We were able to obtain information on the magnitude of the DSET pulse-width in a single inverter cell fabricated by FD-SOI technology by using this estimation method.

The estimated DSET pulse-width by this method was shorter than the measured worst DSET pulse-width (750 ps), though we used waveforms with the greatest collected charge at the drain bias of 1.8 V. In the present method, it is not possible to extract the transient current waveforms set from the MOSFET by having exactly the same position of ion strikes. Therefore, the worst DSET pulse-width must be underestimated. In addition, the bandwidth of the measurement system also contributed to the underestimation of the worst DSET pulse-width. Kobayashi et al. used pulse-laser and a measurement system with a bandwidth of 20 GHz to capture the irradiation response of single transistors, leading to a good agreement between the simulated DSET pulse-waveforms and the table-based estimated DSET pulse-waveform [14]. On the other hand, the bandwidth of our measurement system was 13.5 GHz, and thus, the lower bandwidth of the measurement system resulted in an underestimation in the rise times and the amplitudes of the current pulses induced in the transistor.

4. Conclusions

We estimated the DSET waveform in an inverter from the measured drain currents originating from an nMOSFET fabricated with a 0.2 µm FD-SOI process by using a table-based estimation method. The DSET pulse-width was estimated to be 420 ps at half V_DD. This was consistent with the measured DSET pulse-widths by using the self-triggering flip-flop latch chain, considering the measurement error. Applicability of the table-based DEST pulse waveform estimation method was verified in the case of heavy-ion-induced transient current based DSET pulse-width estimation.