Plasticity Enhancement by Fe-Addition on NiAl Alloy: A Synchrotron X-ray Diffraction Mapping and Molecular Dynamics Simulation Study
Abstract
:1. Introduction
2. Materials and Methods
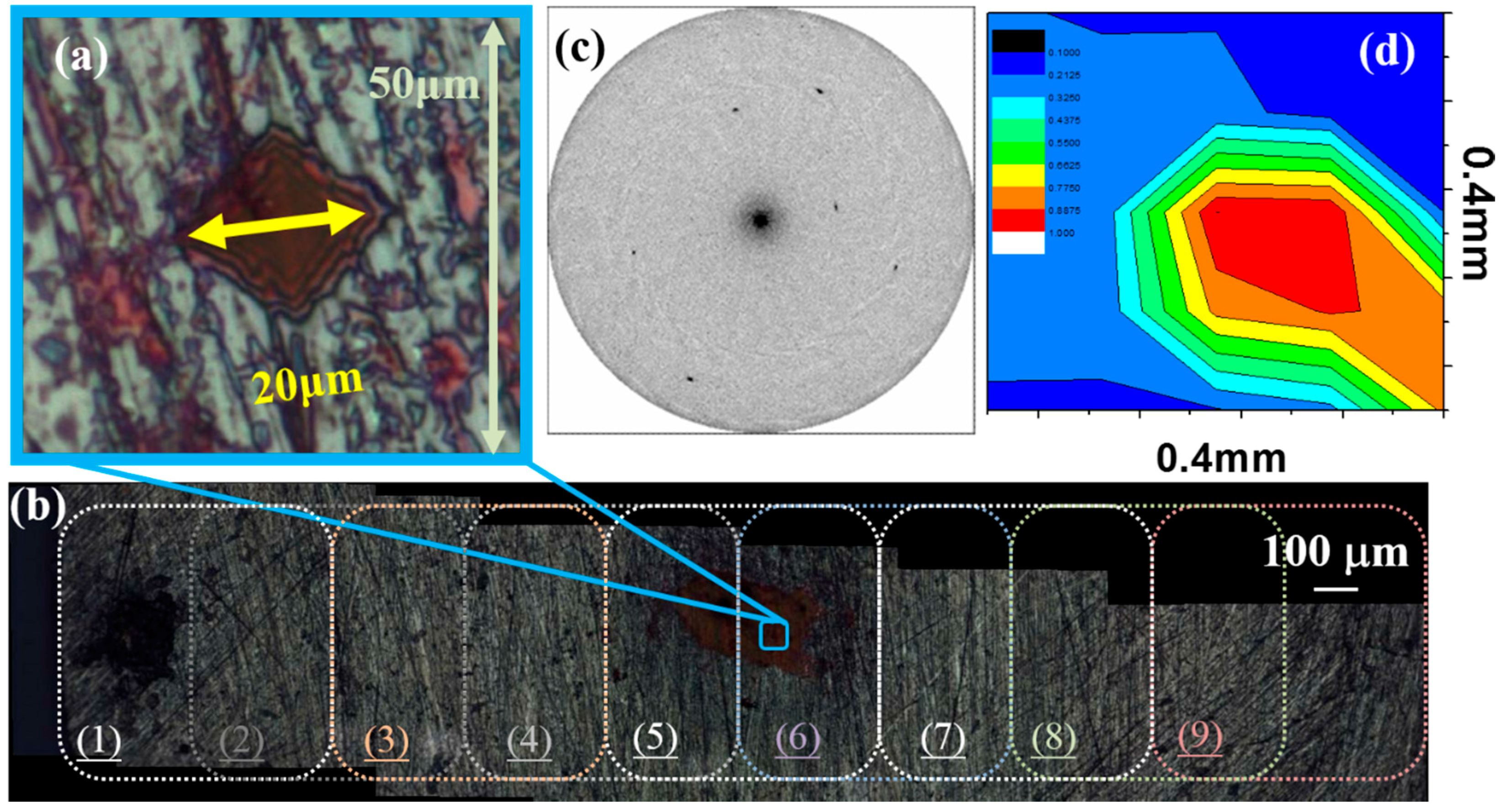
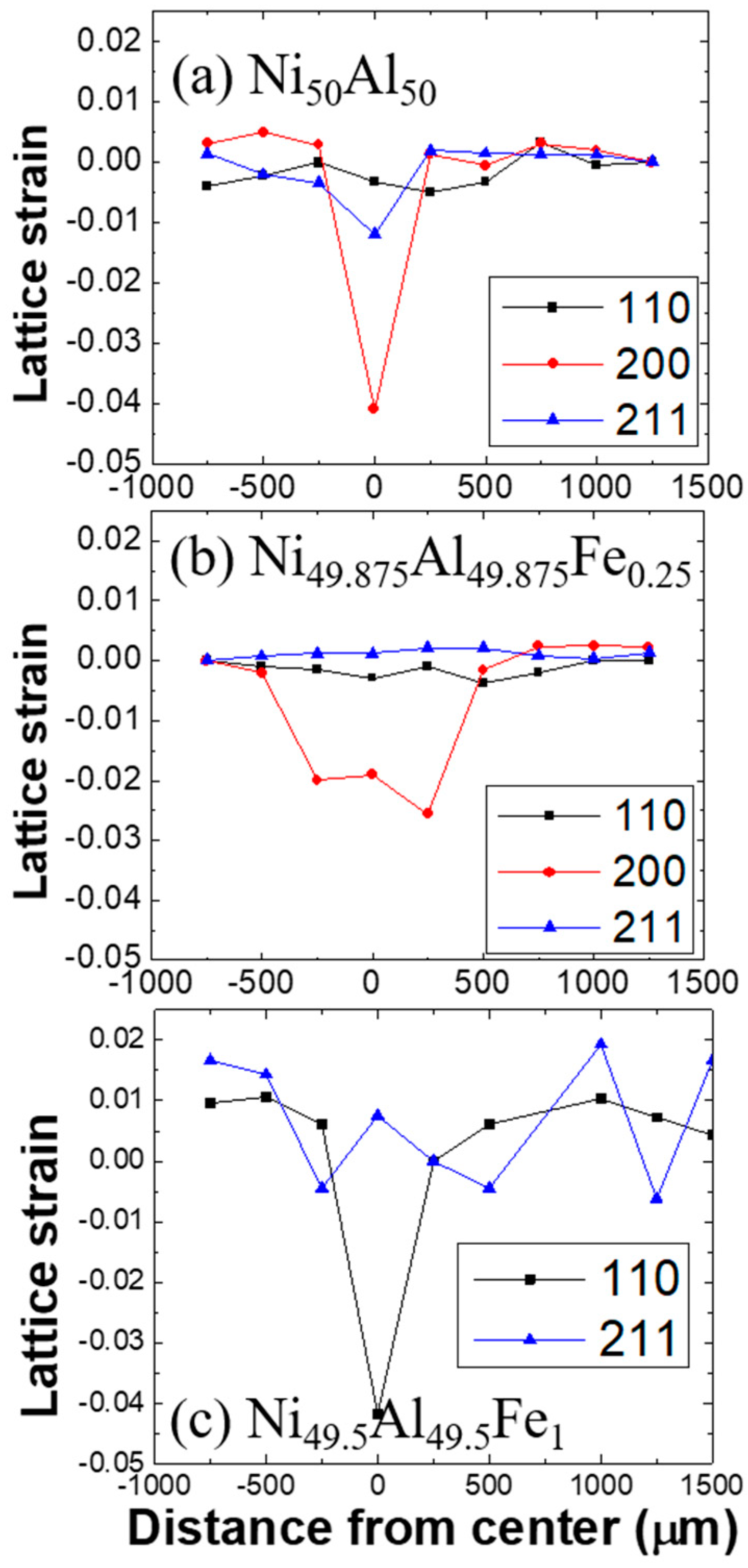
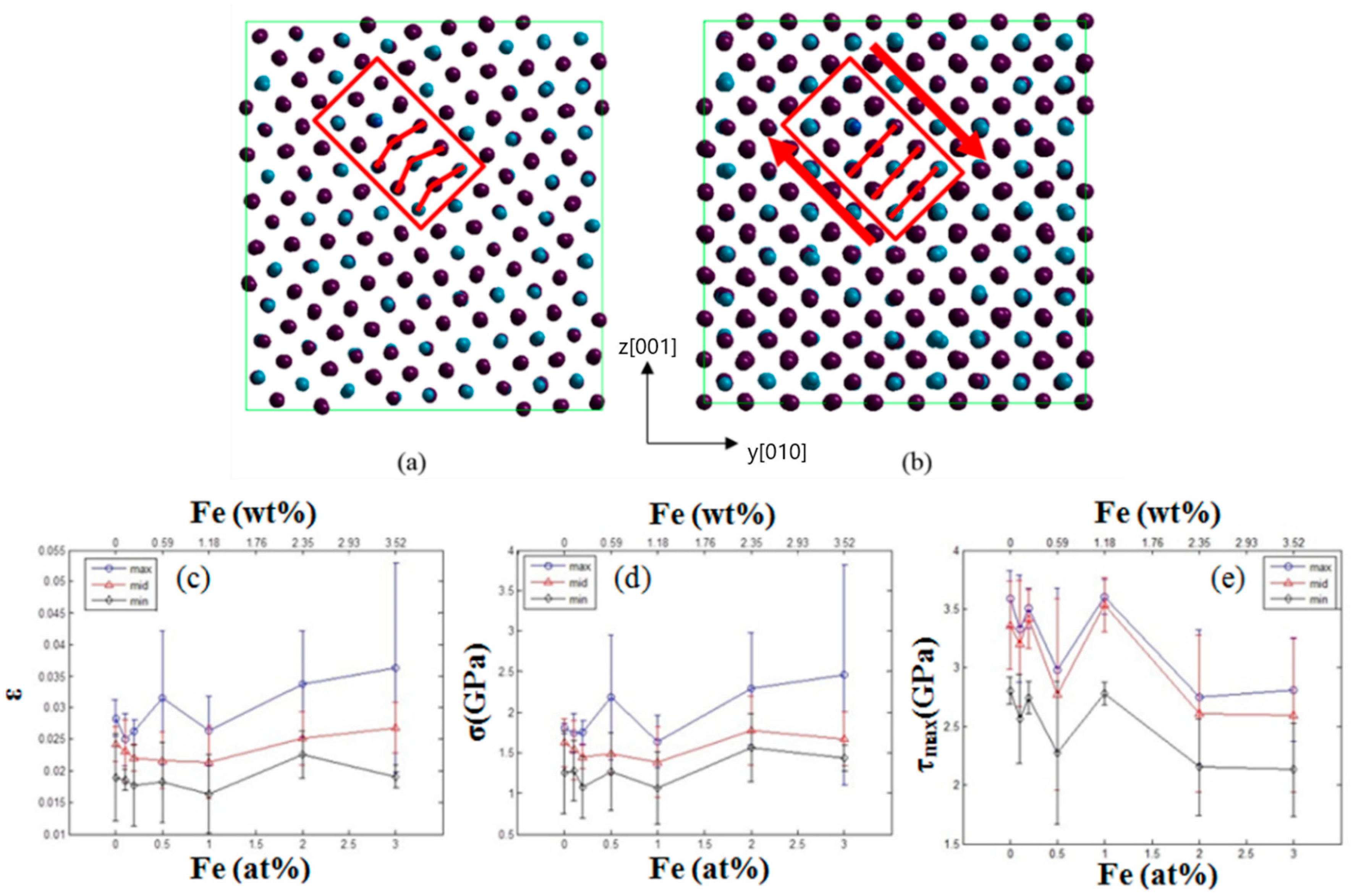
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Han, Y.; Ünal, B.; Evans, J.W. Formation of a novel ordered Ni3Al surface structure by codeposition on NiAl(110). Phys. Rev. Lett. 2012, 108, 216102. [Google Scholar] [CrossRef] [PubMed]
- Ishida, K.; Kainuma, R.; Ueno, N.; Nishizawa, T. Ductility enhancement in NiAl (B2)-base alloys by microstructural control. Metall. Trans. A 1991, 22, 441–446. [Google Scholar] [CrossRef]
- Jiao, Z.; Luan, J.; Zhang, Z.; Miller, M.; Liu, C. High-strength steels hardened mainly by nanoscale NiAl precipitates. Scr. Mater. 2014, 87, 45–48. [Google Scholar] [CrossRef]
- Teng, Z.; Ghosh, G.; Miller, M.K.; Huang, S.; Clausen, B.; Brown, D.; Liaw, P.K. Neutron-diffraction study and modeling of the lattice parameters of a NiAl-precipitate-strengthened Fe-based alloy. Acta Mater. 2012, 60, 5362–5369. [Google Scholar] [CrossRef]
- Fine, M.; Vaynman, S.; Isheim, D.; Chung, Y.-W.; Bhat, S.; Hahin, C. A new paradigm for designing high-fracture-energy steels. Metall. Mater. Trans. A 2010, 41A, 3318–3325. [Google Scholar] [CrossRef]
- Iqbal, F.; Ast, J.; Göken, M.; Durst, K. In situ micro-cantilever tests to study fracture properties of NiAl single crystals. Acta Mater. 2012, 60, 1193–1200. [Google Scholar] [CrossRef]
- Sheng, L.; Zhang, W.; Guo, J.; Yang, F.; Liang, Y.; Ye, H. Effect of Au addition on the microstructure and mechanical properties of NiAl intermetallic compound. Intermetallics 2010, 18, 740–744. [Google Scholar] [CrossRef]
- Darolia, R.; Lahrman, D.; Field, R. The effect of iron, gallium and molybdenum on the room temperature tensile ductility of NiAl. Scr. Metall. Mater. 1992, 26, 1007–1012. [Google Scholar] [CrossRef]
- Hutchings, T.; Withers, P.J.; Holden, T.M.; Lorentzen, T. Introduction to the Characterization of Residual Stress by Neutron Diffraction; Taylor & Francis: London, UK, 2005. [Google Scholar]
- Huang, E.-W.; Lee, S.Y.; Woo, W.; Lee, K.-W. Three-orthogonal-direction stress mapping around a fatigue-crack tip using neutron diffraction. Metall. Mater. Trans. A 2012, 43, 2785–2791. [Google Scholar] [CrossRef]
- Mishin, Y.; Mehl, M.; Papaconstantopoulos, D.; Voter, A.; Kress, J. Structural stability and lattice defects in copper: Ab initio, tight-binding, and embedded-atom calculations. Phys. Rev. B 2001, 63, 224106. [Google Scholar] [CrossRef]
- Rigney, J.D.; Lewandowski, J.J. Fracture toughness of monolithic nickel aluminide intermetallics. Mater. Sci. Eng. 1992, 149, 143–151. [Google Scholar] [CrossRef]
- Giannakopoulos, A.; Larsson, P.-L.; Vestergaard, R. Analysis of Vickers indentation. Int. J. Solids Struct. 1994, 31, 2679–2708. [Google Scholar] [CrossRef]
- Ahn, J.-H.; Kwon, D. Derivation of plastic stress–strain relationship from ball indentations: Examination of strain definition and pileup effect. J. Mater. Res. 2001, 16, 3170–3178. [Google Scholar] [CrossRef]
- Zeng, K.; Chiu, C.H. An analysis of load–penetration curves from instrumented indentation. Acta Mater. 2001, 49, 3539–3551. [Google Scholar] [CrossRef]
- Lin, T.-Y. Using Synchrotron Radiation Mapping to Investigate the Iron-Addition Effects on the Nickel-Aluminide Alloys. Master’s Thesis, National Central University, Taoyuan City, Taiwan, July 2013. [Google Scholar]
- Leonardi, A.; Scardi, P. Dislocation effects on the diffraction line profiles from nanocrystalline domains. Metall. Mater. Trans. A 2015, 47, 5722–5732. [Google Scholar] [CrossRef]
- Zilahi, G.; Ungár, T.; Tichy, G. A common theory of line broadening and rocking curves. J. Appl. Crystallogr. 2015, 48, 418–430. [Google Scholar] [CrossRef]
- Huang, E.W.; Hsu, Y.-H.; Chuang, W.-T.; Ko, W.-C.; Chang, C.-K.; Lee, C.-K.; Chang, W.-C.; Liao, T.-K.; Thong, H.C. Visible-light modulation on lattice dielectric responses of a piezo-phototronic soft material. Adv. Mater. 2015, 27, 7728–7733. [Google Scholar] [CrossRef] [PubMed]
- Huang, E.-W.; Qiao, J.; Winiarski, B.; Lee, W.-J.; Scheel, M.; Chuang, C.-P.; Liaw, P.K.; Lo, Y.-C.; Zhang, Y.; Di Michiel, M. Microyielding of core-shell crystal dendrites in a bulk-metallic-glass matrix composite. Sci. Rep. 2014, 4, 4394. [Google Scholar] [CrossRef] [PubMed]
- Toby, B.H. EXPGUI, a graphical user interface for GSAS. J. Appl. Crystallogr. 2001, 34, 210–213. [Google Scholar] [CrossRef]
- Huang, E.W.; Csiszar, G.; Lo, Y.C.; Huang, Y.L.; Lee, W.J.; Ungar, T.; Liaw, P.K. Plastic deformation of a nano-precipitate strengthened Ni-base alloy investigated by complementary in situ neutron diffraction measurements and molecular-dynamics simulations. Adv. Eng. Mater. 2012, 14, 902–908. [Google Scholar] [CrossRef]
- Li, J. AtomEye: An efficient atomistic configuration viewer. Model. Simul. Mater. Sci. Eng. 2003, 11, 173–177. [Google Scholar] [CrossRef]
- Lathrop, S. Hands-On Paraview Tutorial. Available online: https://hub.vscse.org/resources/89 (accessed on 1 September 2010).
- Liss, K.-D.; Yan, K. Thermo-mechanical processing in a synchrotron beam. Mater. Sci. Eng. A 2010, 528, 11–27. [Google Scholar] [CrossRef]
- Kramer, D.; Huang, H.; Kriese, M.; Robach, J.; Nelson, J.; Wright, A.; Bahr, D.; Gerberich, W. Yield strength predictions from the plastic zone around nanocontacts. Acta Mater. 1998, 47, 333–343. [Google Scholar] [CrossRef]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, E.-W.; Lam, T.-N.; Chang, I.-L.; Hong, W.-J.; Lin, T.-Y.; Su, C.-J.; Liaw, P.K.; Santodonato, L.; Jayant, J.; Fine, M.E. Plasticity Enhancement by Fe-Addition on NiAl Alloy: A Synchrotron X-ray Diffraction Mapping and Molecular Dynamics Simulation Study. Quantum Beam Sci. 2018, 2, 18. https://doi.org/10.3390/qubs2030018
Huang E-W, Lam T-N, Chang I-L, Hong W-J, Lin T-Y, Su C-J, Liaw PK, Santodonato L, Jayant J, Fine ME. Plasticity Enhancement by Fe-Addition on NiAl Alloy: A Synchrotron X-ray Diffraction Mapping and Molecular Dynamics Simulation Study. Quantum Beam Science. 2018; 2(3):18. https://doi.org/10.3390/qubs2030018
Chicago/Turabian StyleHuang, E-Wen, Tu-Ngoc Lam, I-Ling Chang, Wei-Jhih Hong, Tian-Yu Lin, Chun-Jen Su, Peter K. Liaw, Louis Santodonato, Jain Jayant, and Morris E. Fine. 2018. "Plasticity Enhancement by Fe-Addition on NiAl Alloy: A Synchrotron X-ray Diffraction Mapping and Molecular Dynamics Simulation Study" Quantum Beam Science 2, no. 3: 18. https://doi.org/10.3390/qubs2030018
APA StyleHuang, E.-W., Lam, T.-N., Chang, I.-L., Hong, W.-J., Lin, T.-Y., Su, C.-J., Liaw, P. K., Santodonato, L., Jayant, J., & Fine, M. E. (2018). Plasticity Enhancement by Fe-Addition on NiAl Alloy: A Synchrotron X-ray Diffraction Mapping and Molecular Dynamics Simulation Study. Quantum Beam Science, 2(3), 18. https://doi.org/10.3390/qubs2030018






