Shake Table Testing of Voltage and Current Transformers and Numerical Derivation of Corresponding Fragility Curves
Abstract
1. Introduction
2. Shake Table Tests
2.1. Specimens and Instrumentation
2.2. Dynamic Identification of Specimens
2.3. Seismic Simulation Tests
3. FE Numerical Modelling of the Transformers
- Vertical supports of the base plate;
- Metallic base plate;
- Base of the ceramic/porcelain insulator bushing;
- Hollow ceramic/porcelain insulator bushing;
- Metallic hollow top cylinder;
- Secondary terminal box.
- Vertical supports of the base plate;
- Metallic base plate;
- Hollow insulator bushing;
- Metallic head;
- Metallic hollow top cylinder;
- Secondary terminal box.
- For the two VTs: UVC steel model S355J2 + N, with E = 197.41 GPa and yield stress fy = 338.80 MPa;
- For the two CTs: UVC steel model S690QL, with E = 188.63 GPa and yield stress fy = 685.39 MPa.
3.1. Masses, Loads and Damping
3.2. Modelling of the Supporting Column
4. Calibration of the Numerical Models
4.1. Calibration in Terms of Resonant Frequencies
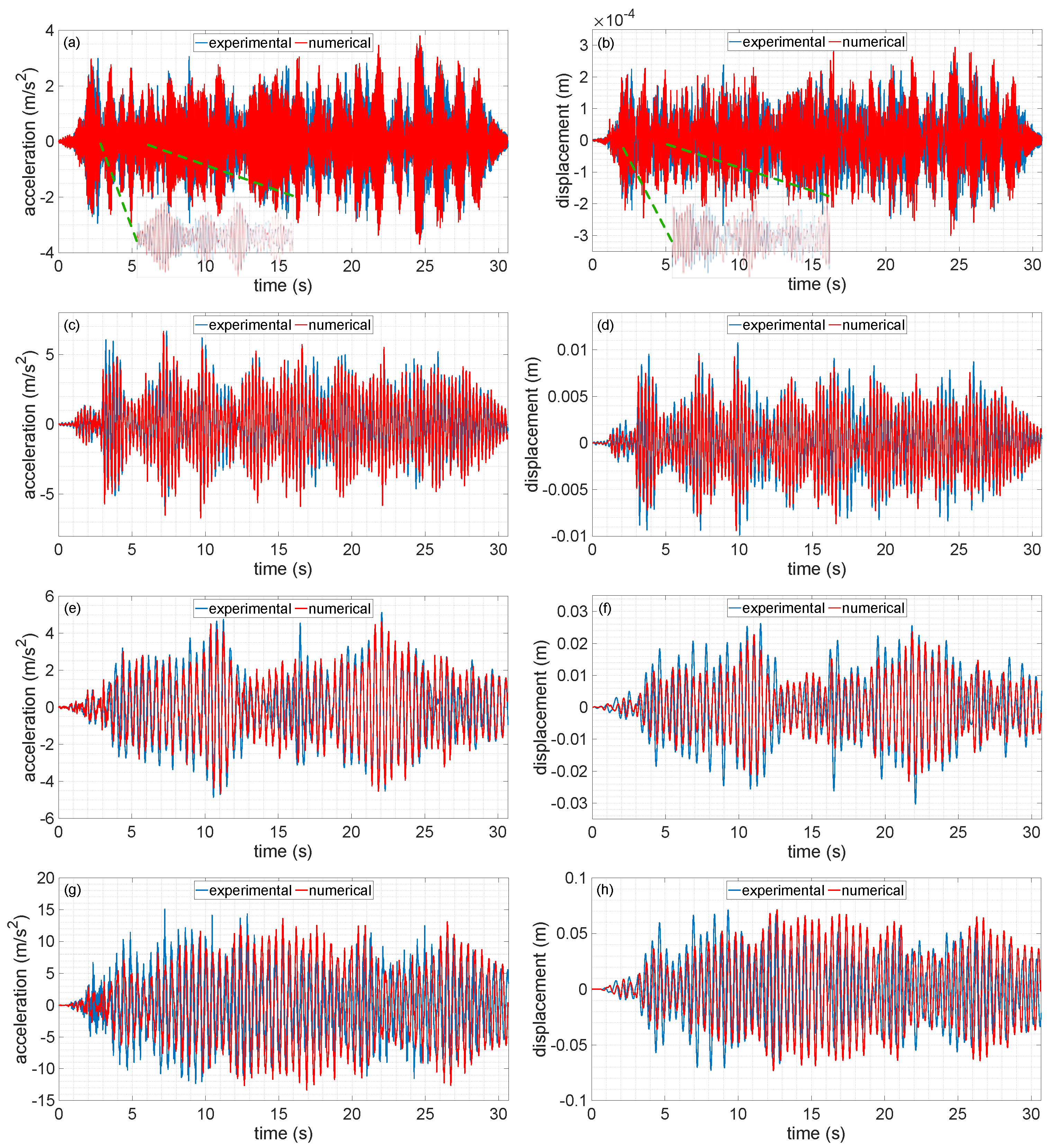
4.2. Calibration in Terms of Dynamic Responses
5. Seismic Fragility Analysis
5.1. Multiple-Stripe Analysis (MSA)
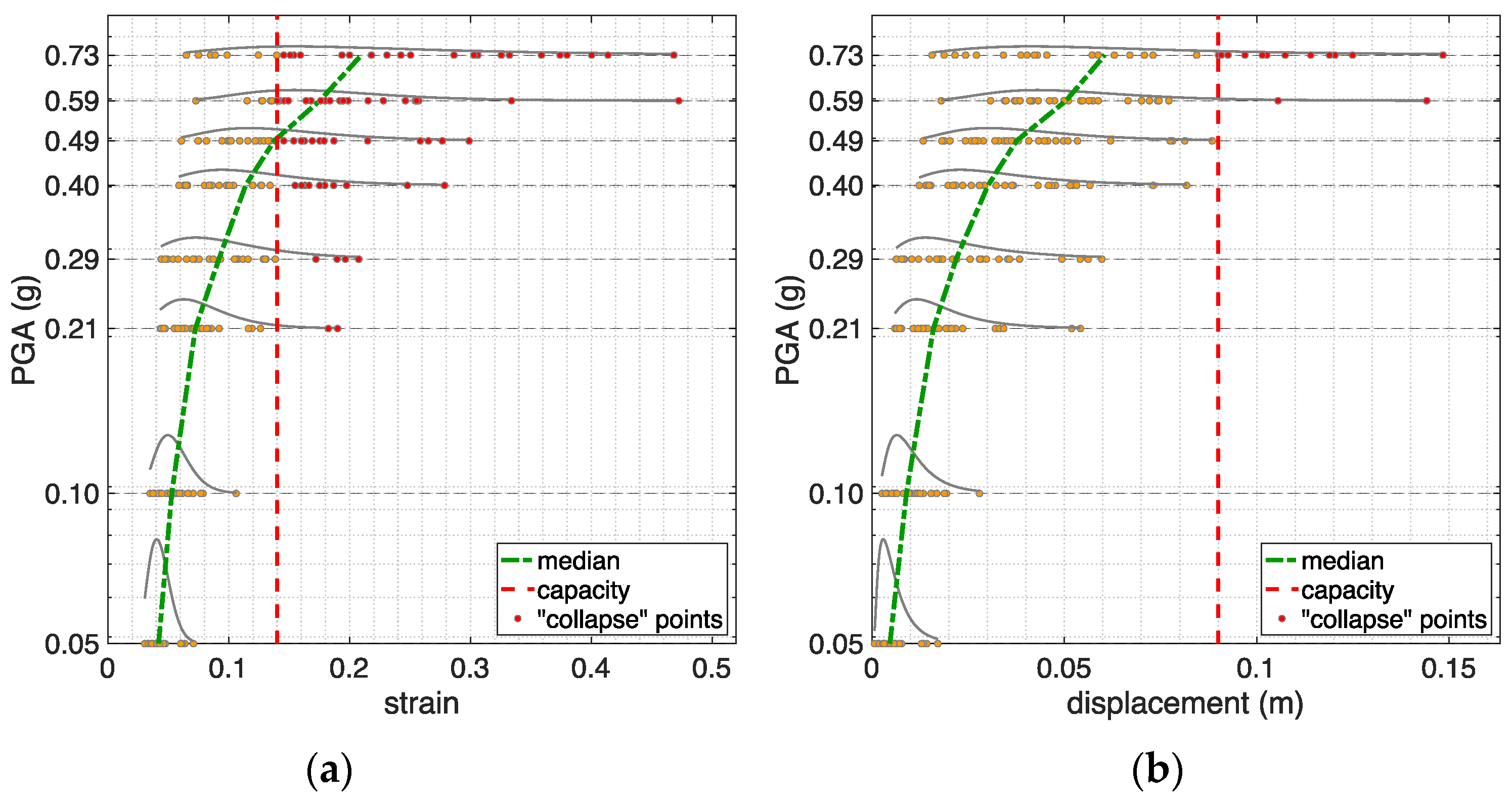
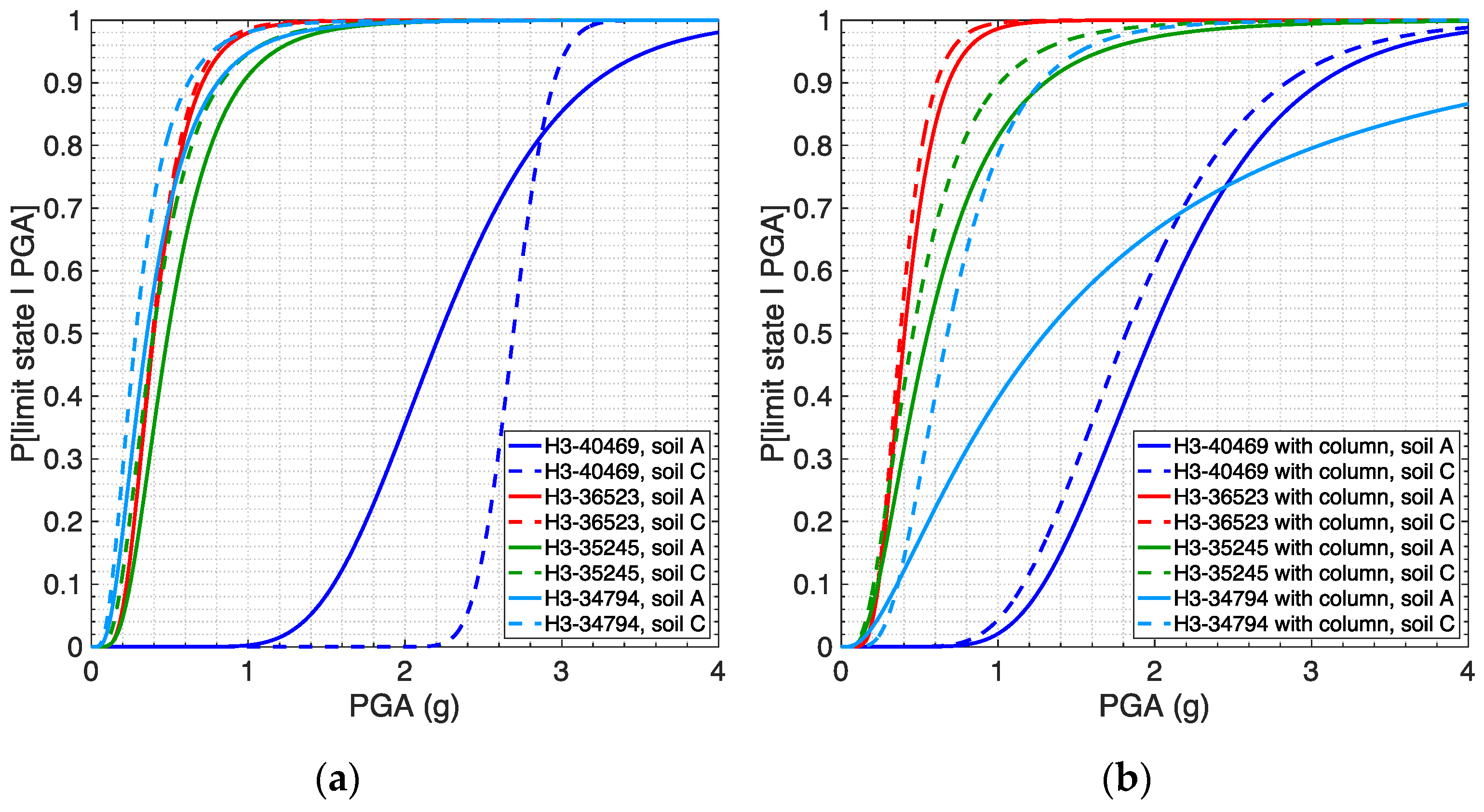
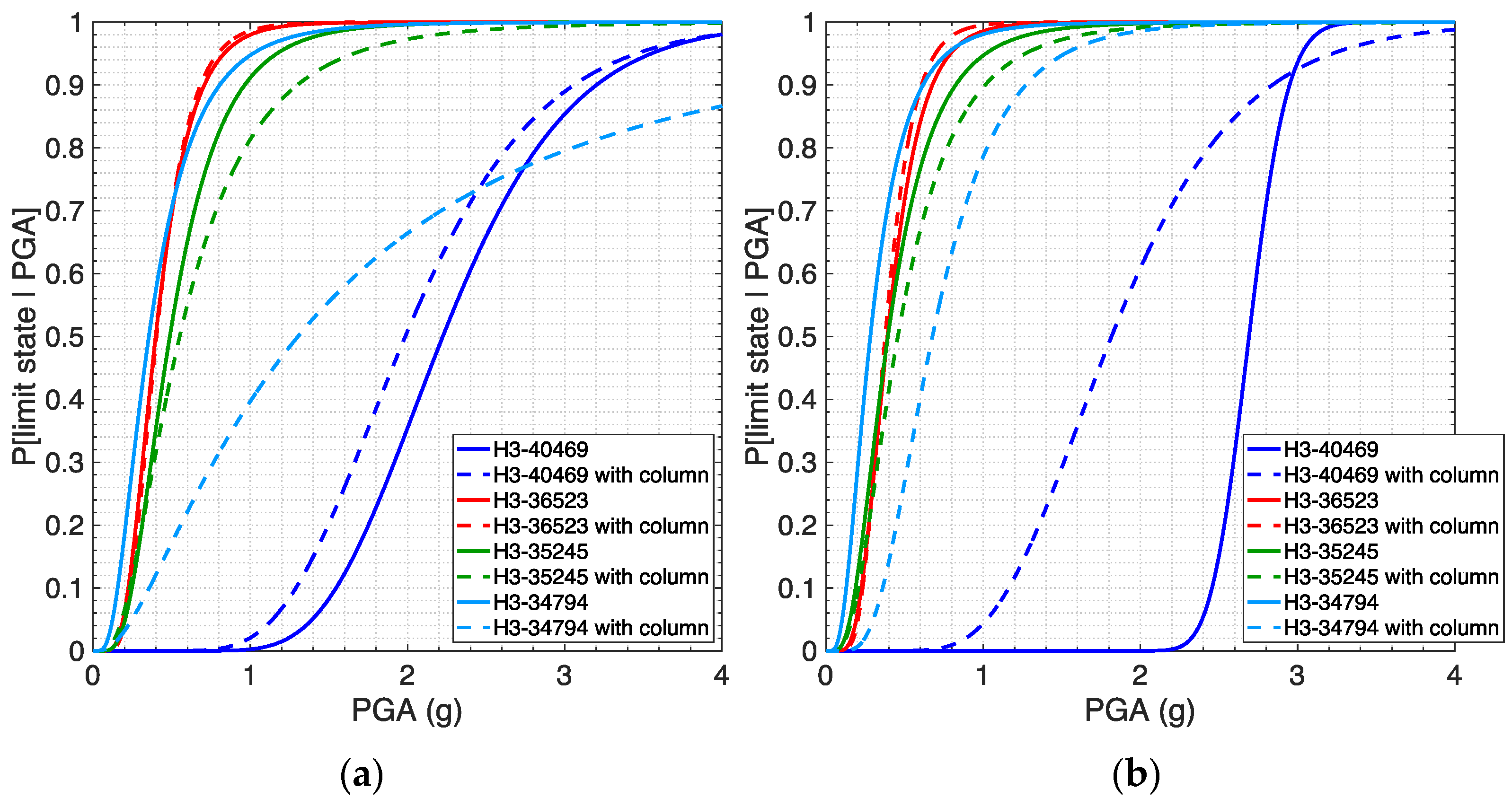
5.2. Fragility Curves
- At each stripe, the number of limit state exceedances was obtained by comparing the demands, in terms of maximum strain in steel (and porcelain, where present) and top displacement, with the relative capacities. Within the counting process, it was decided to take the union (without repetitions) of the exceedances that occurred according to the three failure modes: in this way, for each of the eight models a single fragility curve was derived (actually one per soil type), encompassing all the considered failure modes and limit states. The reason for deriving only one curve per model stands in the fact that, as already pointed out above, utility managers are interested in having substation equipment that is both physically intact and operating normally: in fact, in both cases of mechanical failure and loss of functionality, an electric device is not able to operate anymore and should thus be repaired or replaced.
- The two parameters of the lognormal distribution were retrieved by employing the maximum likelihood (ML) method, developed by Baker (2015) [27], which finds the parameter values such that the resulting distribution has the highest likelihood of having produced the observed “failure” data. The term “failure” here indicates attainment or exceedance of the generic limit state.
- Finally, to obtain an indication of the goodness of fit obtained via ML, the so-called lumped fragility points (Iervolino, 2022) [37] were also computed (one per stripe). They represent empirical percentiles and were retrieved by dividing the number of limit state exceedances by the number of records used (30 per stripe, in this study).
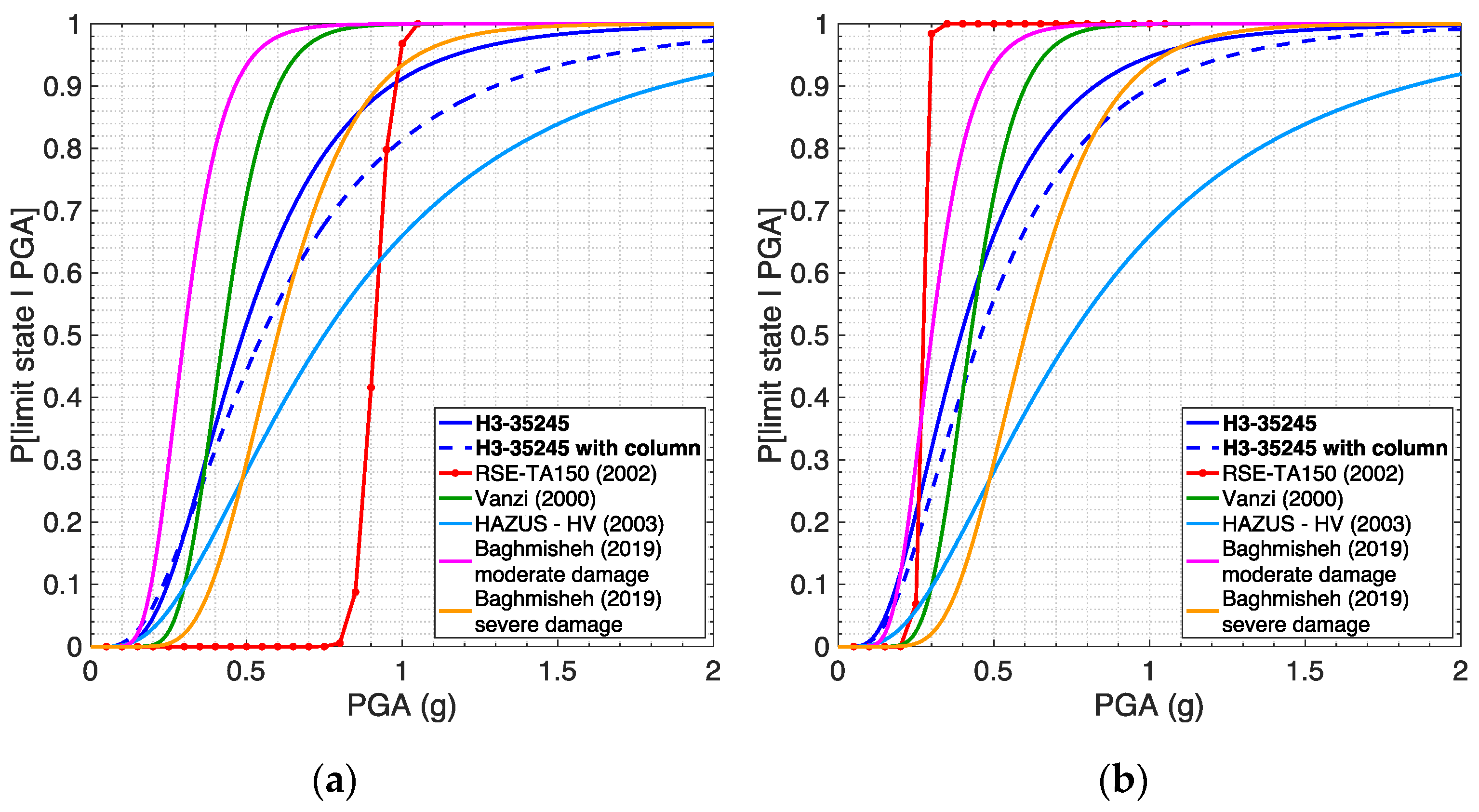
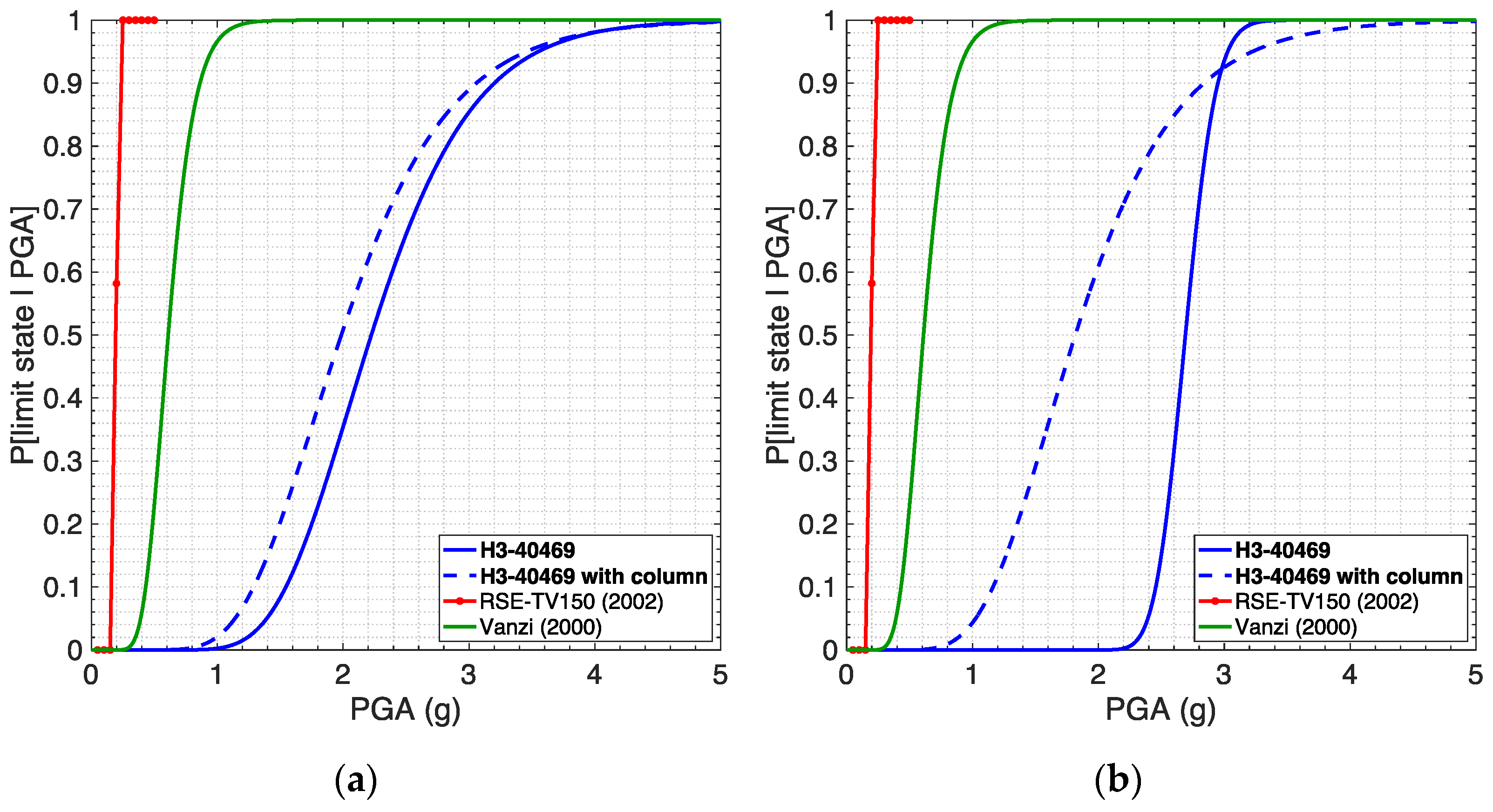
5.3. Comparison with Curves from the Literature
- Database assembled by RSE (Gatti et al., 2002) [10], containing fragility curves for a number of electric devices, all mounted on different types of supporting structure. The curves selected for the comparison are those (i) related to voltage and current transformers operating in substations at 150 kV and 380 kV (voltage values similar to the ones of the devices investigated herein) mounted on a column, (ii) expressed as a function of PGA, and (iii) related to two different site conditions, namely rock and soil (with site amplification), to be compared with the curves obtained herein for soil types A and C, respectively.
- Fragility curves selected and used within the European project SYNER-G (2012) [11], as reported in deliverable 3.3 (Pinto et al., 2010) [38] of SYNER-G and partially in Cavalieri et al. (2014) [39]. In particular, the curves selected for the comparison are those by Vanzi (2000) [12], without voltage distinction, and by HAZUS (FEMA, 2003) [13] for high voltage HV (115 kV) and extra-high voltage EHV (500 kV) for current transformers, whilst for voltage transformers the only available curves are those by Vanzi (2000) [12], also in this case without voltage distinction.
- Fragility curves proposed by Baghmisheh and Estekanchi (2019) [4] for current transformers operating at extra-high voltage EHV (400 kV). The curves selected for the comparison are those related to unconnected devices, consistently with the methodology adopted herein, and two limit states, namely moderate and severe damage. These curves were also included in the plots related to the high voltage device (see Figure 14), for reference.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koliou, M.; Filiatrault, A.; Reinhorn, A.M.; Oliveto, N. Seismic Protection of Electrical Transformer Bushing Systems by Stiffening Techniques; Technical Report MCEER-12-0002; University at Buffalo: Buffalo, NY, USA, 2012. [Google Scholar]
- Zareei, S.A.; Hosseini, M.; Ghafory-Ashtiany, M. Seismic failure probability of a 400 kV power transformer using analytical fragility curves. Eng. Fail. Anal. 2016, 70, 273–289. [Google Scholar] [CrossRef]
- Zareei, S.A.; Hosseini, M.; Ghafory-Ashtiany, M. Evaluation of power substation equipment seismic vulnerability by multivariate fragility analysis: A case study on a 420 kV circuit breaker. Soil Dyn. Earthq. Eng. 2017, 92, 79–94. [Google Scholar] [CrossRef]
- Baghmisheh, A.G.; Estekanchi, H.E. Effects of rigid bus conductors on seismic fragility of electrical substation equipment. Soil Dyn. Earthq. Eng. 2019, 125, 105733. [Google Scholar] [CrossRef]
- Mohammadpour, S.; Hosseini, M. Experimental system identification of a 63 kV substation post insulator and the development of its fragility curves by dynamic finite element analyses. Earthq. Spectra 2017, 33, 1149–1172. [Google Scholar] [CrossRef]
- Gökçe, T.; Orakdöğen, E.; Yüksel, E. Failure mode investigation for High Voltage porcelain insulators. In Proceedings of the 16th European Conference on Earthquake Engineering, Thessaloniki, Greece, 18–21 June 2018. [Google Scholar]
- Gökçe, T.; Yüksel, E.; Orakdöğen, E. Seismic performance enhancement of high-voltage post insulators by a polyurethane spring isolation device. Bull. Earthq. Eng. 2019, 17, 1739–1762. [Google Scholar] [CrossRef]
- Gökçe, T.; Orakdöğen, E.; Yüksel, E. Improvement of the polyurethane spring isolation device for HV post insulators and its evaluation by fragility curves. Earthq. Spectra 2021, 37, 1677–1697. [Google Scholar] [CrossRef]
- Wen, B.; Moustafa, M.A.; Junwu, D. Seismic fragilities of high-voltage substation disconnect switches. Earthq. Spectra 2019, 35, 1559–1582. [Google Scholar] [CrossRef]
- Gatti, F.; Fratelli, M.; Bonacina, G. SISIGEN-SISTEL Impatto di Eventi Eccezionali sui Sistemi di Trasporto e Distribuzione dell’energia Elettrica. Contributo al Rapporto di Sintesi. Milestone 3; Technical Report Doc. RAT-ISMES-2238/2002; CESI S.p.A.: Milan, Italy, 2002. (In Italian) [Google Scholar]
- European Commission. SYNER-G. Collaborative Research Project, Funded by the European Union within Framework Programme 7 (2007–2013) under Grant Agreement No. 244061. 2012. Available online: http://www.vce.at/SYNER-G/files/project/proj-overview.html (accessed on 4 December 2022).
- Vanzi, I. Structural upgrading strategy for electric power networks under seismic action. Earthq. Eng. Struct. Dyn. 2000, 29, 1053–1073. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). HAZUSMH MR4 Multi-Hazard Loss Estimation Methodology—Earthquake Model; Technical Manual; FEMA: Washington, DC, USA, 2003.
- Whittaker, A.S.; Fenves, G.L.; Gilani, A.S. Earthquake performance of porcelain transformer bushings. Earthq. Spectra 2004, 20, 205–223. [Google Scholar] [CrossRef]
- Koliou, M.; Filiatrault, A.; Reinhorn, A.M. Seismic response of high-voltage transformer-bushing systems incorporating flexural stiffeners II: Experimental study. Earthq. Spectra 2013, 29, 1353–1367. [Google Scholar] [CrossRef]
- Moustafa, M.A.; Mosalam, K.M. Substructured dynamic testing of substation disconnect switches. Earthq. Spectra 2016, 32, 567–589. [Google Scholar] [CrossRef]
- Oliveto, N.D.; Reinhorn, A.M. Evaluation of as-installed properties of transformer bushings. Eng. Struct. 2018, 162, 29–36. [Google Scholar] [CrossRef]
- McKenna, F.; Fenves, G.L.; Scott, M.H. OpenSees: Open System for Earthquake Engineering Simulation; Software; University of California: Berkeley, CA, USA, 2000; Available online: http://opensees.berkeley.edu (accessed on 4 December 2022).
- ICC-ES AC156; Seismic Certification by Shake-Table Testing of Nonstructural Components. ICC Evaluation Service: Brea, CA, USA, 2015. Available online: https://icc-es.org/acceptance-criteria/ac156/ (accessed on 4 December 2022).
- Eucentre. Curve di Fragilità di Trasformatori di Tensione e di Corrente; Technical Report RSE177E21_Rec-Report_EUC177-2021E; Eucentre Foundation: Pavia, Italy, 2021. (In Italian) [Google Scholar]
- Cavalieri, F.; Donelli, G.; Pinho, R.; Dacarro, F. Experimental response of voltage and current transformers tested on shake table. In Proceedings of the 17th European Conference on Earthquake Engineering, Bucharest, Romania, 4–9 September 2022. [Google Scholar]
- Yang, T.; Schellenberg, A. OpenSees Navigator; Software; University of California: Berkeley, CA, USA, 2004; Available online: https://openseesnavigator.berkeley.edu/downloads/ (accessed on 4 December 2022).
- MATLAB, Version R2019b. Software. The MathWorks Inc.: Natick, MA, USA, 2019. Available online: https://www.mathworks.com/products/matlab.html (accessed on 4 December 2022).
- OpenSeesWiki Website. Available online: https://opensees.berkeley.edu/wiki/index.php/Main_Page (accessed on 4 December 2022).
- Jalayer, F.; Cornell, C.A. Alternative non-linear demand estimation methods for probability-based seismic assessments. Earthq. Eng. Struct. Dyn. 2009, 38, 951–972. [Google Scholar] [CrossRef]
- Vamvatsikos, D.; Cornell, C.A. Incremental dynamic analysis. Earthq. Eng. Struct. Dyn. 2002, 31, 491–514. [Google Scholar] [CrossRef]
- Baker, J.W. Efficient analytical fragility function fitting using dynamic structural analysis. Earthq. Spectra 2015, 31, 579–599. [Google Scholar] [CrossRef]
- CEN EN 1998-1:2004; Eurocode 8: Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- Bradley, B.A. A generalized conditional intensity measure approach and holistic ground-motion selection. Earthq. Eng. Struct. Dyn. 2010, 39, 1321–1342. [Google Scholar] [CrossRef]
- Bradley, B.A. A ground motion selection algorithm based on the generalized conditional intensity measure approach. Soil Dyn. Earthq. Eng. 2012, 40, 48–61. [Google Scholar] [CrossRef]
- Chiou, B.; Darragh, R.; Gregor, N.; Silva, W. NGA project strong-motion database. Earthq. Spectra 2008, 24, 23–44. [Google Scholar] [CrossRef]
- He, C.; Xie, Q.; Zhou, Y. Influence of flange on seismic performance of 1,100-kV ultra-high voltage transformer bushing. Earthq. Spectra 2019, 35, 447–469. [Google Scholar] [CrossRef]
- Rexton–Steel & Alloys Website. Available online: https://www.rextonsteel.com/s355j2-n-seamless-pipe-supplier-stockist.html#mechanical-properties (accessed on 4 December 2022).
- Henan Gang Iron and Steel Co., Ltd. Website. Available online: https://www.steel-biz.com/s690ql-steel-plates-manufacturer-supplier/#mechanical-properties (accessed on 4 December 2022).
- Gilani, A.S.; Chavez, J.W.; Fenves, G.L.; Whittaker, A.S. Seismic Evaluation of 196 kV Porcelain Transformer Bushings; Technical Report PEER-1998/02; University of California: Berkeley, CA, USA; Pacific Earthquake Engineering Research Center: Richmond, CA, USA, 1998. [Google Scholar]
- Anagnos, T. Development of an Electrical Substation Equipment Performance Database for Evaluation of Equipment Fragilities; Technical Report; Department of Civil and Environmental Engineering, San Jose State University: San Jose, CA, USA, 1999. [Google Scholar]
- Iervolino, I. Estimation uncertainty for some common seismic fragility curve fitting methods. Soil Dyn. Earthq. Eng. 2022, 152, 107068. [Google Scholar] [CrossRef]
- Pinto, P.E.; Cavalieri, F.; Franchin, P.; Vanzi, I. D3.3—Fragility Functions for Electric Power System Elements, Deliverable 3.3 of the SYNER-G Project; Sapienza University of Rome: Rome, Italy, 2010; Available online: http://www.vce.at/SYNER-G/files/dissemination/deliverables.html (accessed on 4 December 2022).
- Cavalieri, F.; Franchin, P.; Pinto, P.E. Fragility Functions of Electric Power Stations. In SYNER-G: Typology Definition and Fragility Functions for Physical Elements at Seismic Risk; Pitilakis, K., Crowley, H., Kaynia, A.M., Eds.; Springer: Dordrecht, The Netherlands; Heidelberg, Germany, 2014; pp. 157–185. ISBN 978-94-007-7872-6. [Google Scholar] [CrossRef]

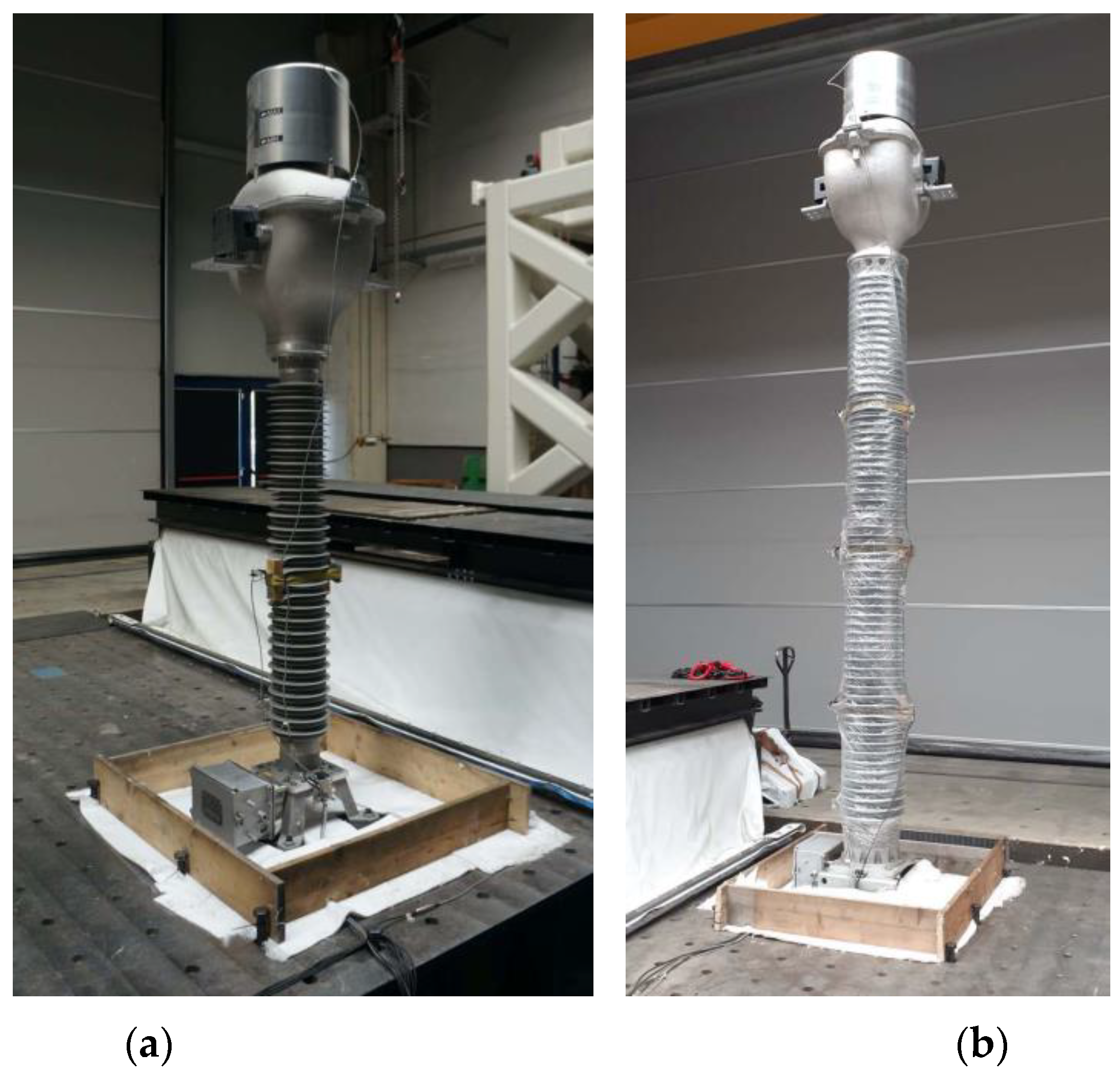





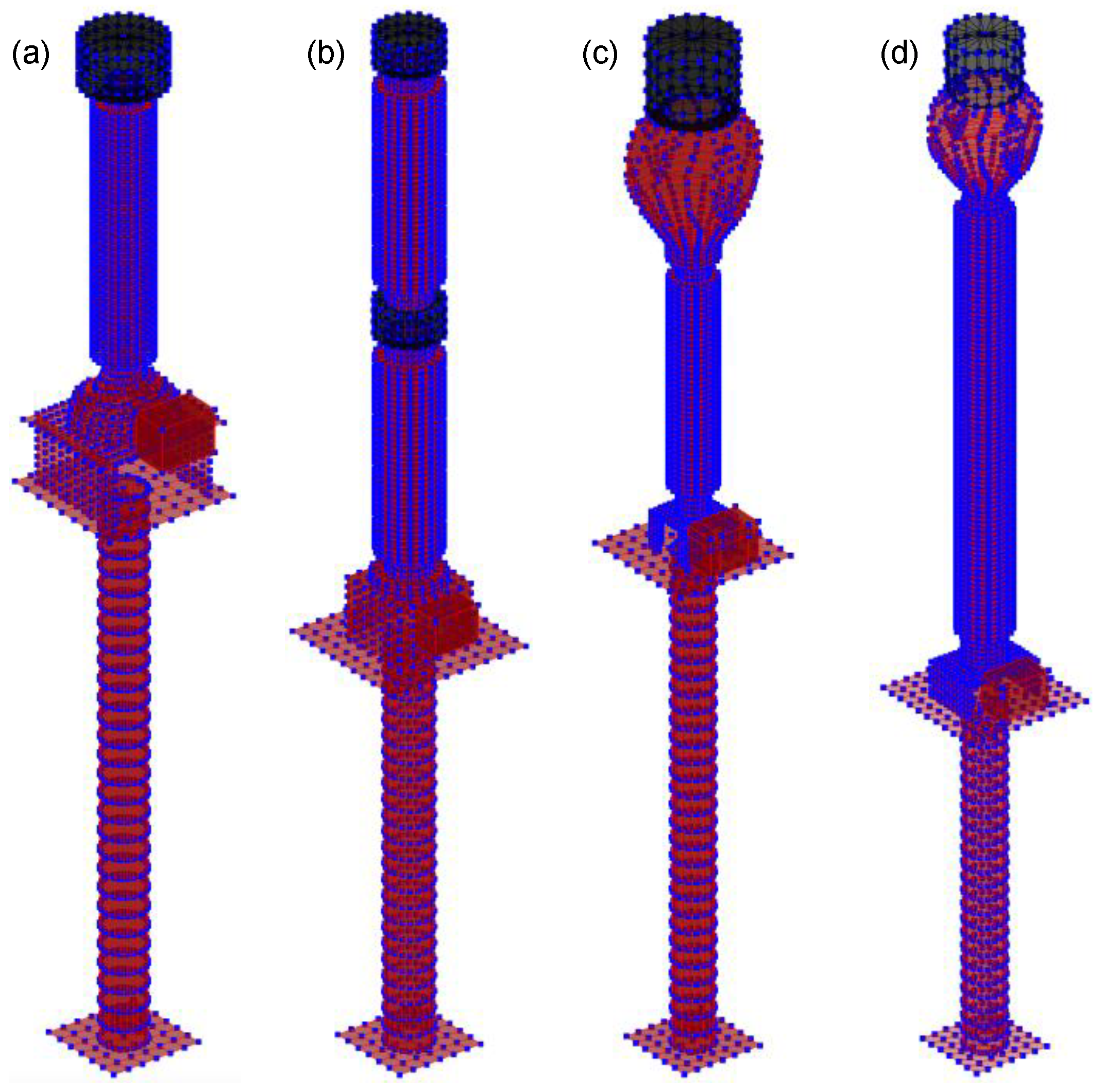



| Specimen | UUT1 | UUT2 | UUT3 | UUT4 |
|---|---|---|---|---|
| Type and voltage (kV) | VT 170 | VT 420 | CT 170 | CT 420 |
| Apparatus | VEOT 170 | TCVT 420 | IOSK 170 | IOSK 420 |
| Production name | H3-40469 | H3-36523 | H3-35245 | H3-34794 |
| Plan dimensions (m) | 0.698 × 0.719 | 0.508 × 0.638 | 0.890 × 0.980 | 0.674 × 0.880 |
| Total height (m) | 2.44 | 3.87 | 2.97 | 4.68 |
| Total mass (kg) | 400 | 533 | 540 | 900 |
| Constitutive materials | Porcelain, steel, aluminium | GFRP 1, silicone rubber HTV 2, steel, aluminium | ||
| Specimen | UUT1 | UUT2 | UUT3 | UUT4 |
|---|---|---|---|---|
| Frequency in X-dir. (Hz) | 19.4 | 4.1 | 2.2 | 2.1 |
| Frequency in Y-dir. (Hz) | 17.5 | 4.1 | 2.2 | 2.1 |
| Specimen | UUT1 | UUT2 | UUT3 | UUT4 |
|---|---|---|---|---|
| Frequency in X-dir. (Hz) | 18.9 | 4.0 | 2.2 | 2.1 |
| Frequency in Y-dir. (Hz) | 17.2 | 4.1 | 2.2 | 2.0 |
| Model | H3-40469 | H3-36523 | H3-35245 | H3-34794 |
|---|---|---|---|---|
| Experimental eigenfrequencies (Hz) | 19.40–17.50 | 4.10–4.10 | 2.20–2.20 | 2.10–2.10 |
| Numerical eigenfrequencies (Hz) | 19.21–17.30 | 4.17–4.18 | 2.22–2.22 | 2.11–2.11 |
| Model | H3-40469 | H3-36523 | H3-35245 | H3-34794 |
|---|---|---|---|---|
| Numerical eigenfrequencies (Hz) | 5.35–4.73 | 3.49–3.72 | 1.88–1.83 | 1.86–1.98 |
| Failure Mode | Limit State | EDP |
|---|---|---|
| Mechanical failure of steel in the base elements | Moderate damage or collapse | Maximum strain in the base steel elements |
| Disconnection of conductors at the top | Loss of functionality | Maximum top displacement |
| Mechanical failure of porcelain in the sheds and base elements | Collapse | Maximum strain in the porcelain sheds and base elements |
| Transformer | Soil Type A | Soil Type C | ||
|---|---|---|---|---|
| Median PGA (g) | Dispersion | Median PGA (g) | Dispersion | |
| H3-40469 | 2.224 | 0.284 | 2.691 | 0.071 |
| H3-36523 | 0.398 | 0.449 | 0.391 | 0.429 |
| H3-35245 | 0.488 | 0.531 | 0.394 | 0.576 |
| H3-34794 | 0.355 | 0.640 | 0.283 | 0.610 |
| H3-40469 with column | 1.986 | 0.337 | 1.817 | 0.348 |
| H3-36523 with column | 0.403 | 0.411 | 0.377 | 0.373 |
| H3-35245 with column | 0.551 | 0.669 | 0.457 | 0.620 |
| H3-34794 with column | 1.303 | 1.010 | 0.678 | 0.491 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cavalieri, F.; Donelli, G.; Pinho, R.; Dacarro, F.; Bernardo, N.; de Nigris, M. Shake Table Testing of Voltage and Current Transformers and Numerical Derivation of Corresponding Fragility Curves. Infrastructures 2022, 7, 171. https://doi.org/10.3390/infrastructures7120171
Cavalieri F, Donelli G, Pinho R, Dacarro F, Bernardo N, de Nigris M. Shake Table Testing of Voltage and Current Transformers and Numerical Derivation of Corresponding Fragility Curves. Infrastructures. 2022; 7(12):171. https://doi.org/10.3390/infrastructures7120171
Chicago/Turabian StyleCavalieri, Francesco, Giuseppe Donelli, Rui Pinho, Filippo Dacarro, Nunzia Bernardo, and Michele de Nigris. 2022. "Shake Table Testing of Voltage and Current Transformers and Numerical Derivation of Corresponding Fragility Curves" Infrastructures 7, no. 12: 171. https://doi.org/10.3390/infrastructures7120171
APA StyleCavalieri, F., Donelli, G., Pinho, R., Dacarro, F., Bernardo, N., & de Nigris, M. (2022). Shake Table Testing of Voltage and Current Transformers and Numerical Derivation of Corresponding Fragility Curves. Infrastructures, 7(12), 171. https://doi.org/10.3390/infrastructures7120171







