Sensitivity of the Flow Number to Mix Factors of Hot-Mix Asphalt
Abstract
1. Introduction
2. Materials
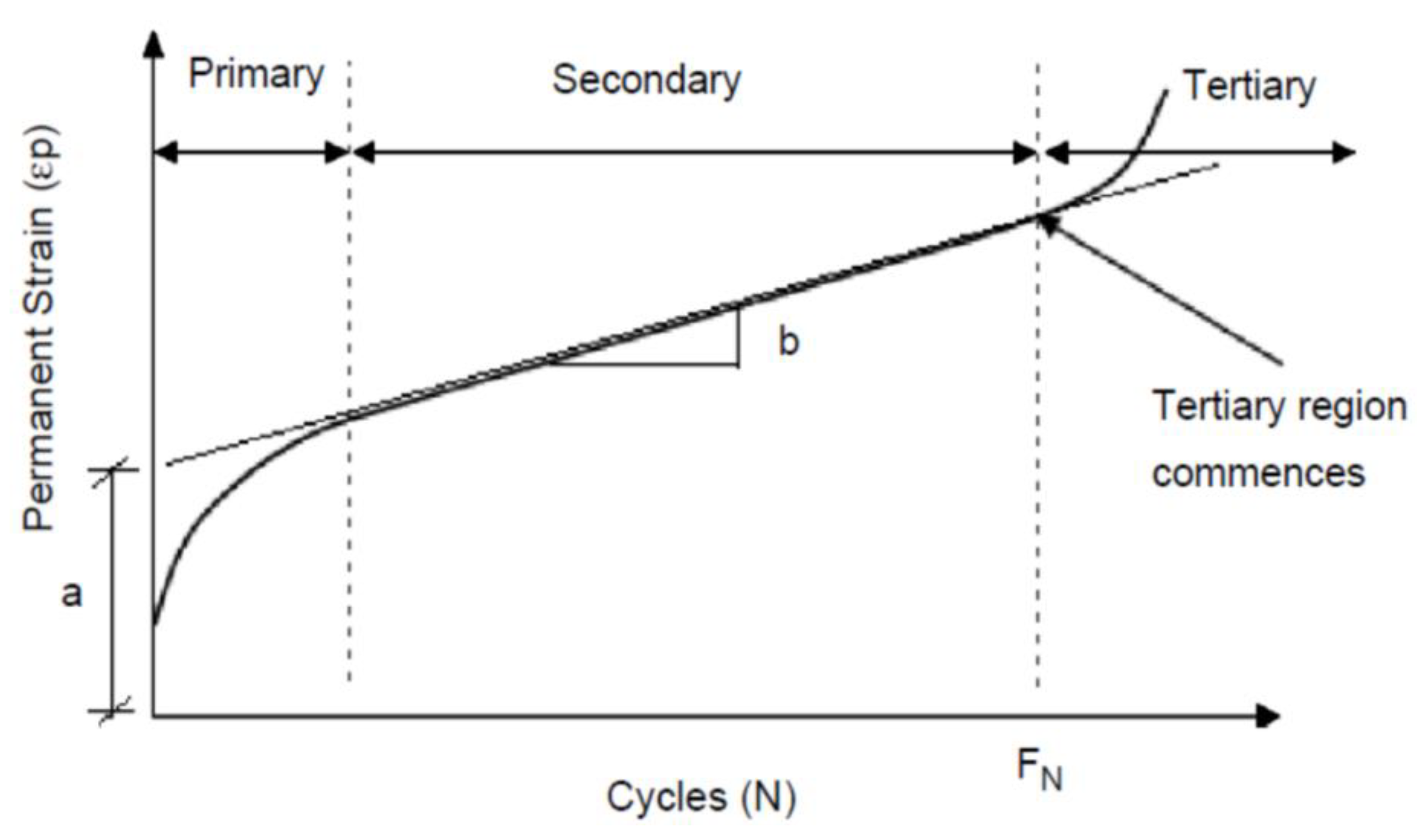
3. Effects on the Flow Number
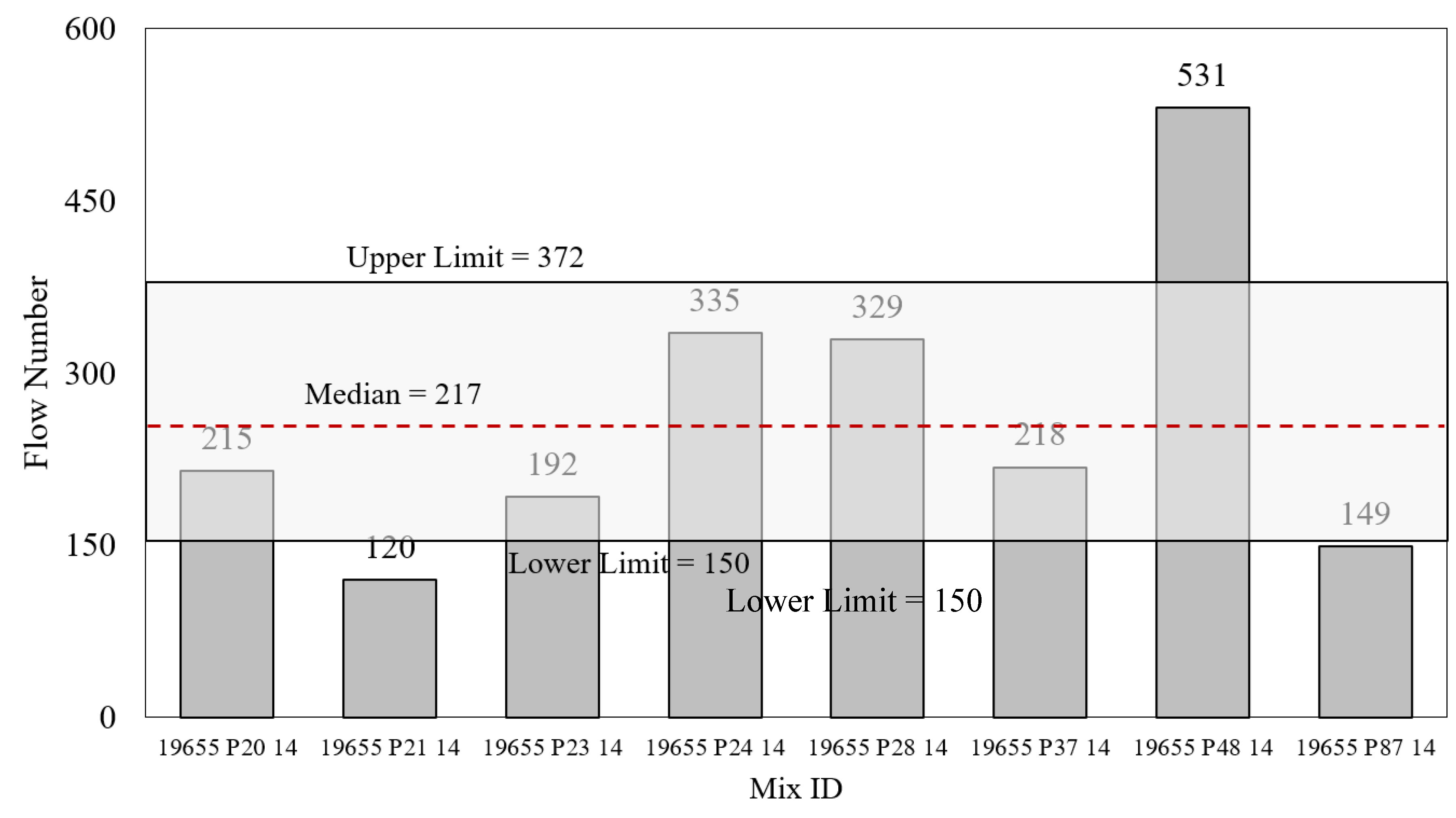
3.1. Same Mix by the Same Contractor
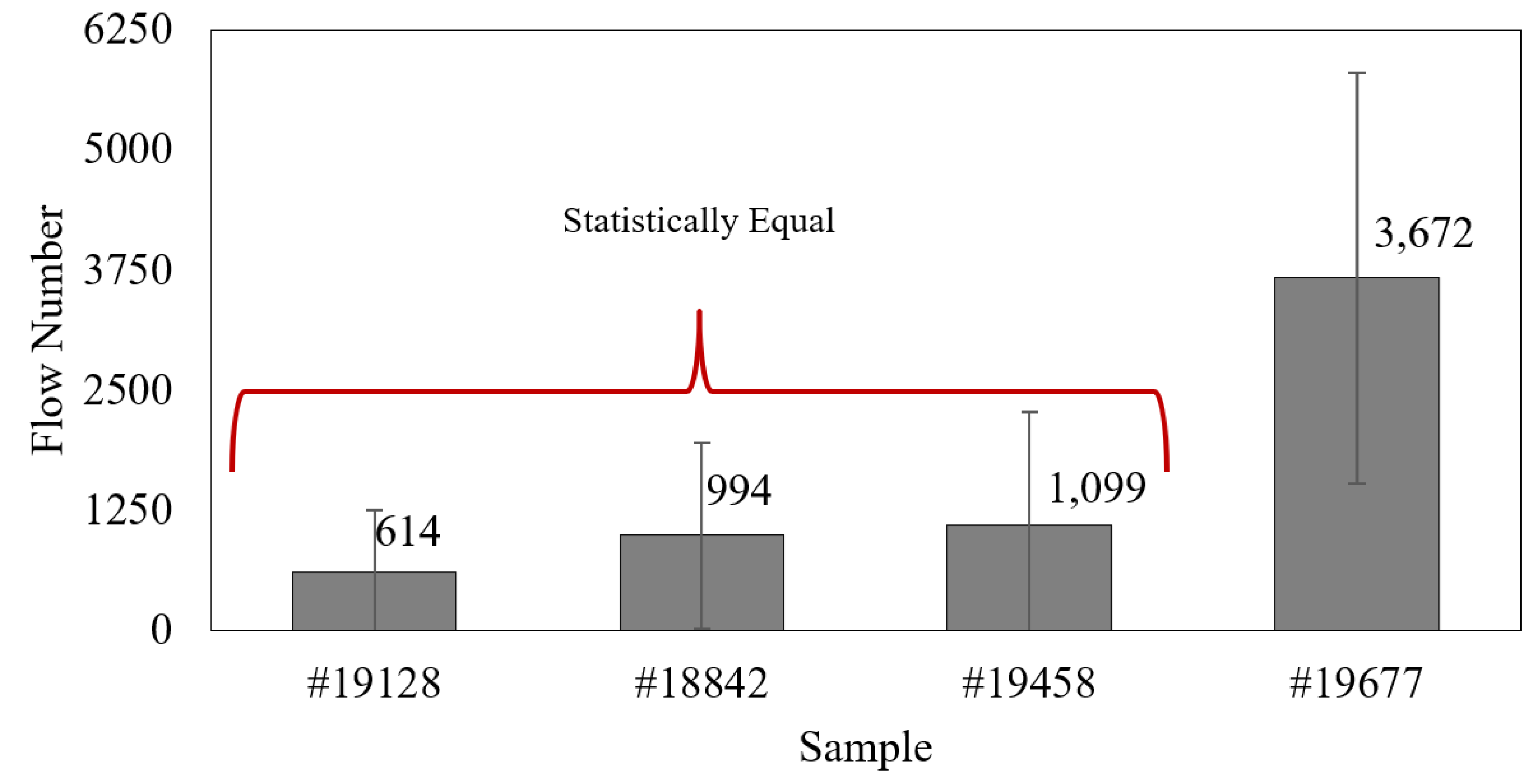
3.2. Same Mix by Different Contractors
3.3. Groupwise Comparison
3.3.1. S(100) PG 64-22
3.3.2. S(100) PG 76-28
3.3.3. SMA PG 76-28
3.3.4. SX(75) PG 58-28
3.3.5. SX(75) PG 58-34
3.3.6. SX(75) PG 64-22
3.3.7. SX(75) PG 64-28
3.3.8. SX(100) PG 58-28
3.3.9. SX(100) PG 64-22
3.3.10. SX(100) PG 64-28
3.3.11. SX(100) PG 76-28
3.4. Analysis Summary
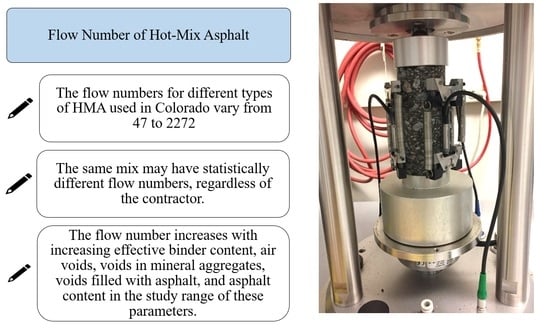
- Only two types of mixtures, SX(100) PG 76-28 and SMA PG 76-28, had flow numbers greater than 740. Thus, only these mixtures are considered good for traffic greater than 30 million ESALs.
- S(100) PG 76-28 had an average flow number of more than 190; thus, it is considered good for traffic between 10 and 30 million ESALs.
- SX(100) PG 64-22, SX(100) PG 64-28, and SX(100) PG 58-28 are considered good for traffic between 3 and 10 million ESALs.
- The other five mixtures—S(100) PG 64-22, SX(75) PG 58-28, SX(75) PG 58-34, SX(75) PG 64-22, and SX(75) PG 64-28—had flow numbers less than 50; thus, they are considered good for traffic of less than 3 million ESALs.
- Comparing SX(100) PG 64-28 and SX(100) PG 76-28, the flow number of HMA increases with an increase in the high-temperature grade of the binder.
- Variable results were observed as to whether the flow number increases or decreases with an increase in the low-temperature grade of the binder. For example, when comparing SX(75) PG 64-22 and SX(75) PG 64-28, the flow number increases with an increase in the low-temperature grade of the binder; however, when comparing SX(75) PG 58-34 and SX(75) PG 58-28, the flow number decreases with an increase in the low-temperature grade of the binder.
- An SX mix has 0.5 in. (12.5 mm) nominal aggregate size, and an S mix has 0.75 in. (19 mm) nominal aggregate size. SX mixes have larger flow numbers, i.e., smaller aggregate size produces a larger flow number, from the comparisons of the flow numbers of SX(100) PG 64-22 with S(100) PG 64-22, and SX(100) PG 76-28 with that of S(100) PG 76-28. However, the differences between these pairs are not statistically significant.
- The (75) and (100) refer to the number of gyrations during design. Greater number of gyrations produce greater flow numbers, as shown from the comparisons of SX(75) PG 58-28 with SX(100) PG 58-28, SX(75) PG 64-22 with SX(100) PG 64-22, and SX(75) PG 64-28 with SX(100) PG 64-28. However, the differences between these pairs were not statistically significant.
4. Conclusions
- The same mix may have statistically different flow numbers, and this is independent of the contractor.
- The flow number increased with increasing Vbe, Va, VMA, VFA, and AC for the range studied in this study.
- Only two types of mixtures, SX(100) PG 76-28 and SMA PG 76-28, had flow numbers greater than 740. Thus, only these mixtures are considered good for traffic greater than 30 million ESALs.
- S(100) PG 76-28 had an average flow number of more than 190; thus, it is considered good for traffic between 10 and 30 million ESALs.
- SX(100) PG 64-22, SX(100) PG 64-28, and SX(100) PG 58-28 are considered good for traffic between 3 and 10 million ESALs.
- The other five mixtures—S(100) PG 64-22, SX(75) PG 58-28, SX(75) PG 58-34, SX(75) PG 64-22, and SX(75) PG 64-28—had flow numbers less than 50; thus, they are considered good for traffic of less than 3 million ESALs.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Biligiri, K.; Way, G. Predicted E* dynamic moduli of the Arizona mixes using asphalt binders placed over a 25-year period. Constr. Build. Mater. 2014, 54, 520–532. [Google Scholar] [CrossRef]
- AASHTO TP 79. Standard Method of Test for Determining the Dynamic Modulus and Flow Number for Asphalt Mixtures Using the Asphalt Mixture Performance Tester (AMPT); American Association of State Highway and Transportation Officials (AASHTO): Washington, DC, USA, 2015; pp. 1–21. [Google Scholar]
- Kaloush, K.E. Simple Performance Test for Permanent Deformation of Asphalt Mixtures. Ph.D. Thesis, Department of Civil and Environmental Engineering, Arizona State University, Tempe, AZ, USA, 2001. [Google Scholar]
- Kvasnak, A.; Robinette, C.J.; Williams, R.C. Statistical development of a flow number predictive equation for the Mechanistic-Empirical Pavement Design Guide. In Proceedings of the TRB Annual Meeting, Washington, DC, USA, 21 January 2007; [CD-ROM]. TRB: Washington, DC, USA, 2007. [Google Scholar]
- Christensen, D.W.; Bonaquist, R.; Jack, D.P. Evaluation of Triaxial Strength as a Simple Test for Asphalt Concrete Rut Resistance; Final Report to the Pennsylvania Department of Transportation, Report No. FHWA-PA-2000-010+97-04 (19); The Pennsylvania Transportation Institute: University Park, PA, USA, August 2000. [Google Scholar]
- Rodezno, M.C.; Kaloush, K.K.; Corrigan, M.R. Development of a Flow Number Predictive Model. In Transportation Research Board [CD-ROM]; TRB: Washington, DC, USA, 2010. [Google Scholar]
- Irfan, M.; Ali, Y.; Iqbal, S.; Ahmed, S.; Hafeez, I. Rutting Evaluation of Asphalt Mixtures Using Static. Dynamic, and Repeated Creep Load Tests. Arab. J. Sci. Eng. 2018, 43, 5143–5155. [Google Scholar] [CrossRef]
- Ogundipe, O.M. Marshall Stability and Flow of Lime-modified Asphalt Concrete. Trans. Res. Proc. 2016, 14, 685–693. [Google Scholar] [CrossRef]
- Irfan, M.; Ali, Y.; Ahmed, S.; Iqbal, S.; Wang, H. Rutting and Fatigue Properties of Cellulose Fiber-Added Stone Mastic Asphalt Concrete Mixtures. Advan. Mat. Sci. Eng. 2019, 2019, 5604197. [Google Scholar] [CrossRef]
- Leiva-Villacorta, F.; Taylor, A.; Willis, R. High-Modulus Asphalt Concrete (HMAC) Uixtures for Use as Base Course; NCAT Report 17-04; 2017; National Center for Asphalt Technology, Auburn University: Auburn, AL, USA, June 2017. [Google Scholar]
- Tripathi, A. Mechanistic analysis and economic benefits of fiber-reinforced asphalt mixtures. Master’s Thesis, Department of Civil Engineering, The University of Texas, Tyler, TX, USA, 2018. [Google Scholar]
- Ziari, H.; Divandari, H. Presenting asphalt mixtures flow number prediction model using gyratory curves. Int. J. Civ. Eng. 2013, 11, 125–133. [Google Scholar]
- Siswanto, H.; Supriyanto, B.; Pranoto, P.R.C.; Hakim, A. Marshall Properties of Asphalt Concrete Using Crumb Rubber Modified of Motorcycle Tire Waste. In Proceedings of the Green Construction and Engineering Education (GCEE) Conference, East Java, Indonesia, 8–9 August 2017. [Google Scholar]


| Traffic Level, Million Equivalent Single Axle Load (ESAL, 80 kN (18 kips)) | Flow Number |
|---|---|
| Less than 3.0 | NA |
| 3.0 to less than 10 | 50 |
| 10 to less than 30 | 190 |
| More than 30 | 740 |
| Mix ID | NMAS, in. (mm) | Binder | Number of Gyrations | Number of Specimens |
|---|---|---|---|---|
| S(100) PG 64-22 | 0.75 (19) | PG 64-22 | 100 | 6 |
| S(100) PG 76-28 | 0.75 (19) | PG 76-28 | 100 | 5 |
| SMA PG 76-28 | 0.50 (12.5) | PG 76-28 | 100 | 12 |
| SX(75) PG 58-28 | 0.50 (12.5) | PG 58-28 | 75 | 8 |
| SX(75) PG 58-34 | 0.50 (12.5) | PG 58-34 | 75 | 4 |
| SX(75) PG 64-22 | 0.50 (12.5) | PG 64-22 | 75 | 8 |
| SX(75) PG 64-28 | 0.50 (12.5) | PG 64-28 | 75 | 4 |
| SX(100) PG 58-28 | 0.50 (12.5) | PG 58-28 | 100 | 2 |
| SX(100) PG 64-22 | 0.50 (12.5) | PG 64-22 | 100 | 15 |
| SX(100) PG 64-28 | 0.50 (12.5) | PG 64-28 | 100 | 10 |
| SX(100) PG 76-28 | 0.50 (12.5) | PG 76-28 | 100 | 31 |
| 19128 | 18842 | 19458 | |
|---|---|---|---|
| 18842 | Equal | - | - |
| 19458 | Equal | Equal | - |
| 19677 | Different | Different | Different |
| Mixes | Lowest Value | Highest Value | 95% CI Lower Limit | 95% CI Upper Limit | Average |
|---|---|---|---|---|---|
| S(100) PG 64-22 | 110 | 252 | 47 | 262 | 155 |
| S(100) PG 76-28 | 626 | 2065 | 253 | 2193 | 1223 |
| SMA PG 76-28 | 426 | 4311 | 1487 | 3057 | 2272 |
| SX(75) PG 58-28 | 29 | 220 | 42 | 140 | 91 |
| SX(75) PG 58-34 | 19 | 75 | 0 | 403 | 47 |
| SX(75) PG 64-22 | 19 | 123 | 28 | 90 | 112 |
| SX(75) PG 64-28 | 32 | 311 | 0 | 323 | 106 |
| SX(100) PG 58-28 | - | - | - | - | 128 |
| SX(100) PG 64-22 | 23 | 388 | 59 | 164 | 112 |
| SX(100) PG 64-28 | 77 | 531 | 134 | 347 | 241 |
| SX(100) PG 76-28 | 82 | 6343 | 893 | 2263 | 1578 |
| Vbe (%) | Va (%) | VMA (%) | VFA (%) | AC (%) | |
|---|---|---|---|---|---|
| S(100) PG 64-22 | Decreases | Increases | Increases | Increases | Decreases |
| S(100) PG 76-28 | - | Decreases | Increases | Decreases | - |
| SMA PG 76-28 | Decreases | Increases | Increases | Increases | Decreases |
| SX(75) PG 58-28 | Increases | Decreases | Decreases | Increases | Increases |
| SX(75) PG 58-34 | NA | NA | NA | NA | NA |
| SX(75) PG 64-22 | Increases | Decreases | Increases | Decreases | Increases |
| SX(75) PG 64-28 | Increases | Decreases | - | Increases | - |
| SX(100) PG 58-28 | NA | NA | NA | NA | NA |
| SX(100) PG 64-22 | Increases | Increases | Decreases | Decreases | Increases |
| SX(100) PG 64-28 | Increases | Increases | Decreases | Decreases | Increases |
| SX(100) PG 76-28 | Increases | Increases | Decreases | Increases | Increases |
| Summary | 6 Increases | 5 Increases | 5 Increases | 5 Increases | 5 Increases |
| 2 Decreases | 4 Decreases | 4 Decreases | 4 Decreases | 2 Decreases | |
| 1 Insensitive | 2 N/A | 1 Insensitive | 2 N/A | 2 Insensitive |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, M.R.; Kalevela, S.A.; Nesselhauf, S.K. Sensitivity of the Flow Number to Mix Factors of Hot-Mix Asphalt. Infrastructures 2019, 4, 34. https://doi.org/10.3390/infrastructures4020034
Islam MR, Kalevela SA, Nesselhauf SK. Sensitivity of the Flow Number to Mix Factors of Hot-Mix Asphalt. Infrastructures. 2019; 4(2):34. https://doi.org/10.3390/infrastructures4020034
Chicago/Turabian StyleIslam, Md Rashadul, Sylvester A. Kalevela, and Shelby K. Nesselhauf. 2019. "Sensitivity of the Flow Number to Mix Factors of Hot-Mix Asphalt" Infrastructures 4, no. 2: 34. https://doi.org/10.3390/infrastructures4020034
APA StyleIslam, M. R., Kalevela, S. A., & Nesselhauf, S. K. (2019). Sensitivity of the Flow Number to Mix Factors of Hot-Mix Asphalt. Infrastructures, 4(2), 34. https://doi.org/10.3390/infrastructures4020034