Simulation of the Effects of Angle of Attack and Projectile Contour in Damage Development in Reinforced Concrete
Abstract
1. Introduction
2. Materials and Methods
3. Results
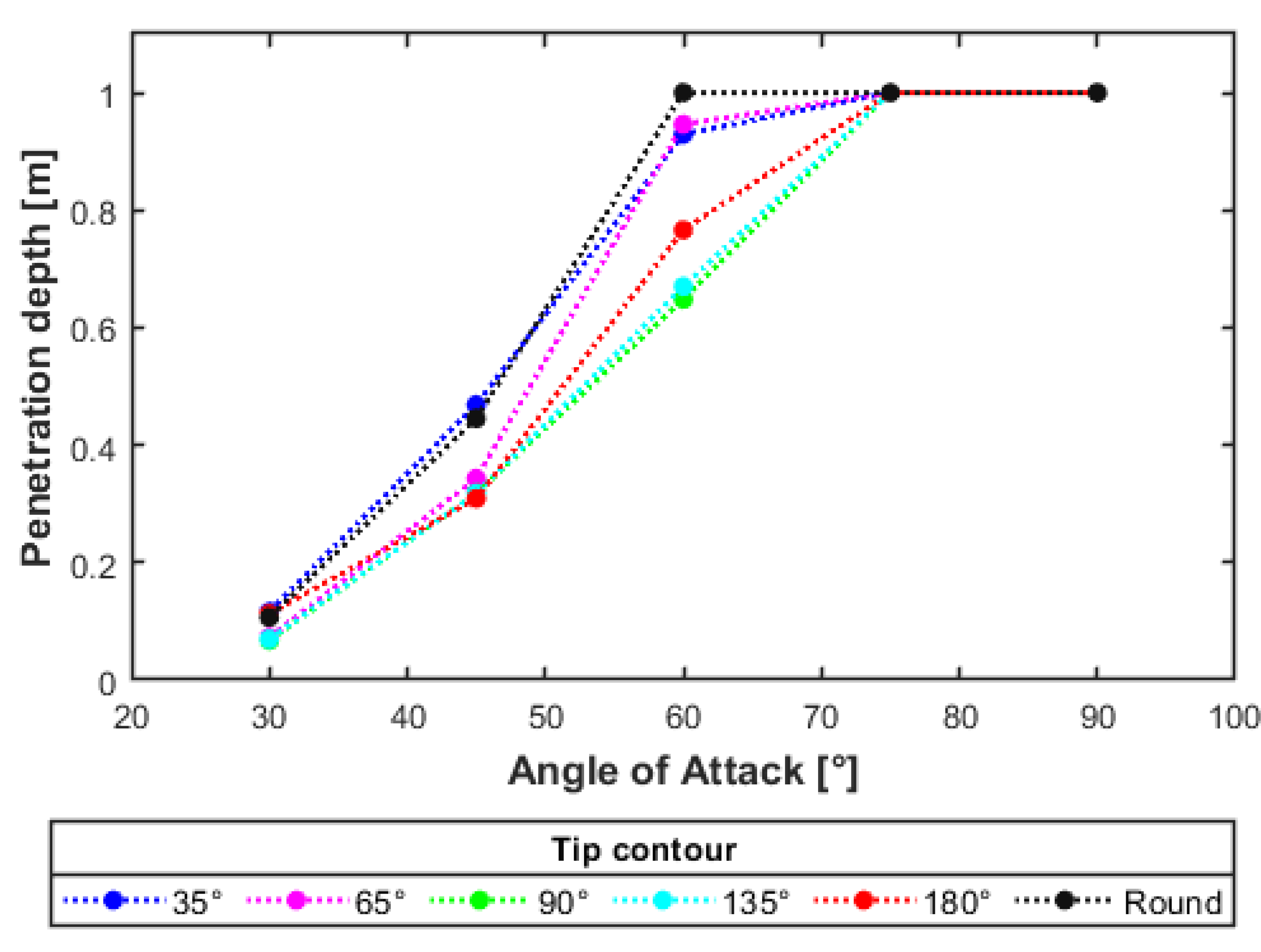
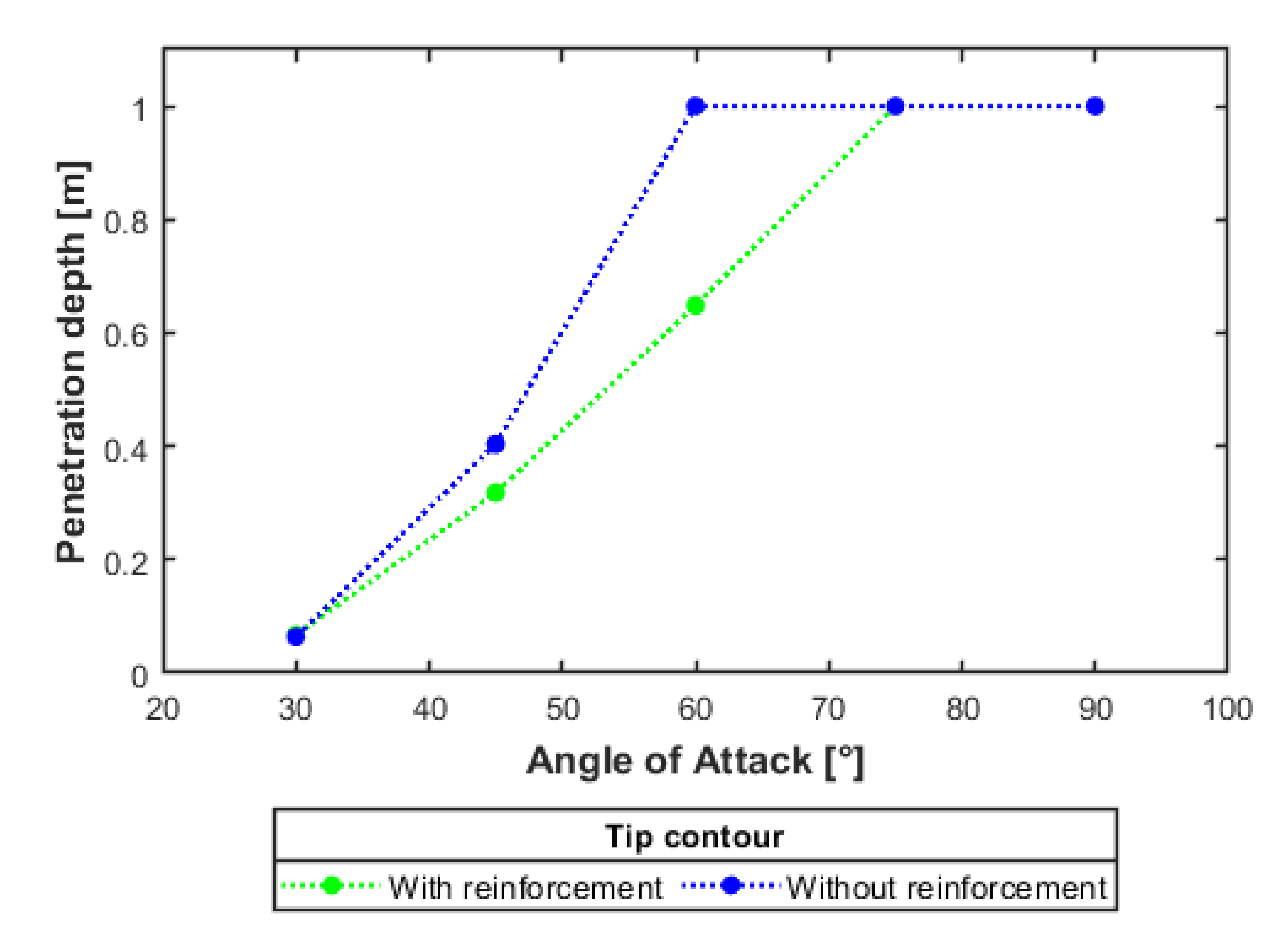
3.1. Penetration
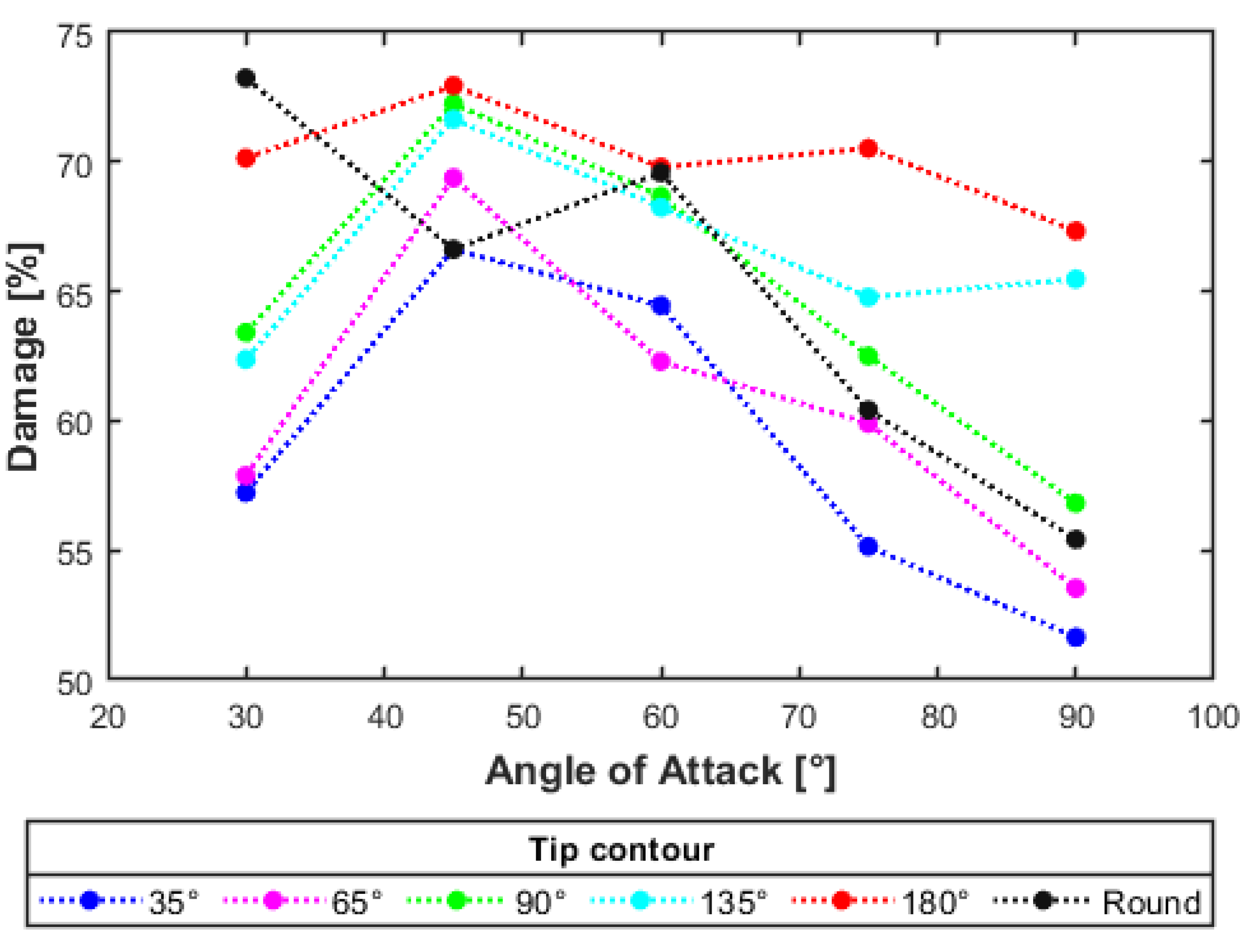
3.2. Damage
4. Conclusions
- The penetration had a similar behavior for all tip contours and mostly depended on the AoA. A flat AoA caused bouncing off of the target and therefore a low penetration.
- A similar tendency for penetration could be also seen in unreinforced concrete. However, the penetration depth for a specific case was significantly reduced with reinforced compared with unreinforced concrete.
- The damage had a similar, but less significant, behavior over all tip contours, while the lowest damage was mostly caused for a flat AoA because of bouncing off of the projectile. A flat tip of the projectile caused the highest damage on the target overall.
- A round tip had a significant difference in damage for an AoA of 30°, which was caused by the projectile digging it into the target right below the surface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, S.; Kong, X.; Fang, Q.; Chen, L.; Wang, Y. Numerical Prediction of Dynamic Failure in Concrete Targets Subjected to Projectile Impact by a Modified Kong-Fang Material Model. Int. J. Impact Eng. 2020, 144, 103633. [Google Scholar] [CrossRef]
- Kong, X.; Fang, Q.; Li, Q.M.; Wu, H.; Crawford, J.E. Modified K&C Model for Cratering and Scabbing of Concrete Slabs under Projectile Impact. Int. J. Impact Eng. 2017, 108, 217–228. [Google Scholar] [CrossRef]
- Rajput, A.; Iqbal, M.A.; Gupta, N.K. Ballistic Performances of Concrete Targets Subjected to Long Projectile Impact. Thin-Walled Struct. 2018, 126, 171–181. [Google Scholar] [CrossRef]
- Xu, X.; Ma, T.; Ning, J. Failure Mechanism of Reinforced Concrete Subjected to Projectile Impact Loading. Eng. Fail. Anal. 2019, 96, 468–483. [Google Scholar] [CrossRef]
- Feng, J.; Gao, X.; Li, J.; Dong, H.; He, Q.; Liang, J.; Sun, W. Penetration Resistance of Hybrid-Fiber-Reinforced High-Strength Concrete under Projectile Multi-Impact. Constr. Build. Mater. 2019, 202, 341–352. [Google Scholar] [CrossRef]
- Tabei, A.; Li, D.S.; Lavender, C.A.; Garmestani, H. Investigation of Precipitate Refinement in Mg Alloys by an Analytical Composite Failure Model. Mech. Mater. 2015, 89, 59–71. [Google Scholar] [CrossRef]
- Tabei, A.; Ahzi, S.; Li, D.S.; Lavender, C.A.; Garmestani, H. Effects of Morphology and Geometry of Inclusions on Two-Point Correlation Statistics in Two Phase Composites. Int. J. Theor. Appl. Multiscale Mech. 2014, 3, 1–17. [Google Scholar] [CrossRef]
- Lyons, C.K.; Tabei, A.; Sobhani, S. Energy Absorbing Cab Guards for Log Trucks. Int. J. For. Eng. 2021, 1–8. [Google Scholar] [CrossRef]
- Wu, C.T.; Wu, Y.; Crawford, J.E.; Magallanes, J.M. Three-Dimensional Concrete Impact and Penetration Simulations Using the Smoothed Particle Galerkin Method. Int. J. Impact Eng. 2017, 106, 1–17. [Google Scholar] [CrossRef]
- Oucif, C.; Kalyana Rama, J.S.; Shankar Ram, K.; Abed, F. Damage Modeling of Ballistic Penetration and Impact Behavior of Concrete Panel under Low and High Velocities. Def. Technol. 2021, 17, 202–211. [Google Scholar] [CrossRef]
- Blasone, M.; Saletti, D.; Baroth, J.; Forquin, P.; Bonnet, E.; Delaplace, A. Ultra-High Performance Fibre-Reinforced Concrete under Impact of an AP Projectile: Parameter Identification and Numerical Modelling Using the DFHcoh-KST Coupled Model. Int. J. Impact Eng. 2021, 152, 103838. [Google Scholar] [CrossRef]
- Ning, J.; Meng, F.; Ma, T.; Xu, X. Failure Analysis of Reinforced Concrete Slab under Impact Loading Using a Novel Numerical Method. Int. J. Impact Eng. 2020, 144, 103647. [Google Scholar] [CrossRef]
- Abdel-Kader, M. Modified Settings of Concrete Parameters in RHT Model for Predicting the Response of Concrete Panels to Impact. Int. J. Impact Eng. 2019, 132, 103312. [Google Scholar] [CrossRef]
- Liu, J.; Wu, C.; Su, Y.; Li, J.; Shao, R.; Chen, G.; Liu, Z. Experimental and Numerical Studies of Ultra-High Performance Concrete Targets against High-Velocity Projectile Impacts. Eng. Struct. 2018, 173, 166–179. [Google Scholar] [CrossRef]
- Hu, F.; Wu, H.; Fang, Q.; Liu, J.C. Impact Resistance of Concrete Targets Pre-Damaged by Explosively Formed Projectile (EFP) against Rigid Projectile. Int. J. Impact Eng. 2018, 122, 251–264. [Google Scholar] [CrossRef]
- Chen, X.; Lu, F.; Zhang, D. Penetration Trajectory of Concrete Targets by Ogived Steel Projectiles–Experiments and Simulations. Int. J. Impact Eng. 2018, 120, 202–213. [Google Scholar] [CrossRef]
- Wu, J.; Ning, J.; Ma, T. The Dynamic Response and Failure Behavior of Concrete Subjected to New Spiral Projectile Impacts. Eng. Fail. Anal. 2017, 79, 547–564. [Google Scholar] [CrossRef]
- Pavlovic, A.; Fragassa, C.; Disic, A. Comparative Numerical and Experimental Study of Projectile Impact on Reinforced Concrete. Compos. Part B Eng. 2017, 108, 122–130. [Google Scholar] [CrossRef]
- Iqbal, M.A.; Rajput, A.; Gupta, N.K. Performance of Prestressed Concrete Targets against Projectile Impact. Int. J. Impact Eng. 2017, 110, 15–25. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, X.; Chen, H.; Wang, J.; Wei, H.; Xiong, W. Experimental and Theoretical Study on Steel Long-Rod Projectile Penetration into Concrete Targets with Elevated Impact Velocities. Int. J. Impact Eng. 2020, 138, 103482. [Google Scholar] [CrossRef]
- Mina, A.L.; Petrou, M.F.; Trezos, K.G. Resistance of an Optimized Ultra-High Performance Fiber Reinforced Concrete to Projectile Impact. Buildings 2021, 11, 63. [Google Scholar] [CrossRef]
- Jamnam, S.; Maho, B.; Techaphatthanakon, A.; Sonoda, Y.; Yoo, D.-Y.; Sukontasukkul, P. Steel Fiber Reinforced Concrete Panels Subjected to Impact Projectiles with Different Caliber Sizes and Muzzle Energies. Case Stud. Constr. Mater. 2020, 13, e00360. [Google Scholar] [CrossRef]
- Elhozayen, A.E.; Lassi, M.Y.; Attia, W.A. Numerical modeling of high-velocity, projectile penetrating concrete blocks reinforced by teflon sheets. WIT Trans. Eng. Sci. 2019, 125, 15–25. [Google Scholar] [CrossRef]
- Leelavanichkul, S.; Brannon, R.M. Survey of Four Damage Models for Concrete; SAND2009-5544, 993922; Sandia National Laboraotories: Albuquerque, NM, USA, 2009. [Google Scholar] [CrossRef][Green Version]
- Tham, C.Y. Numerical and Empirical Approach in Predicting the Penetration of a Concrete Target by an Ogive-Nosed Projectile. Finite Elem. Anal. Des. 2006, 42, 1258–1268. [Google Scholar] [CrossRef]
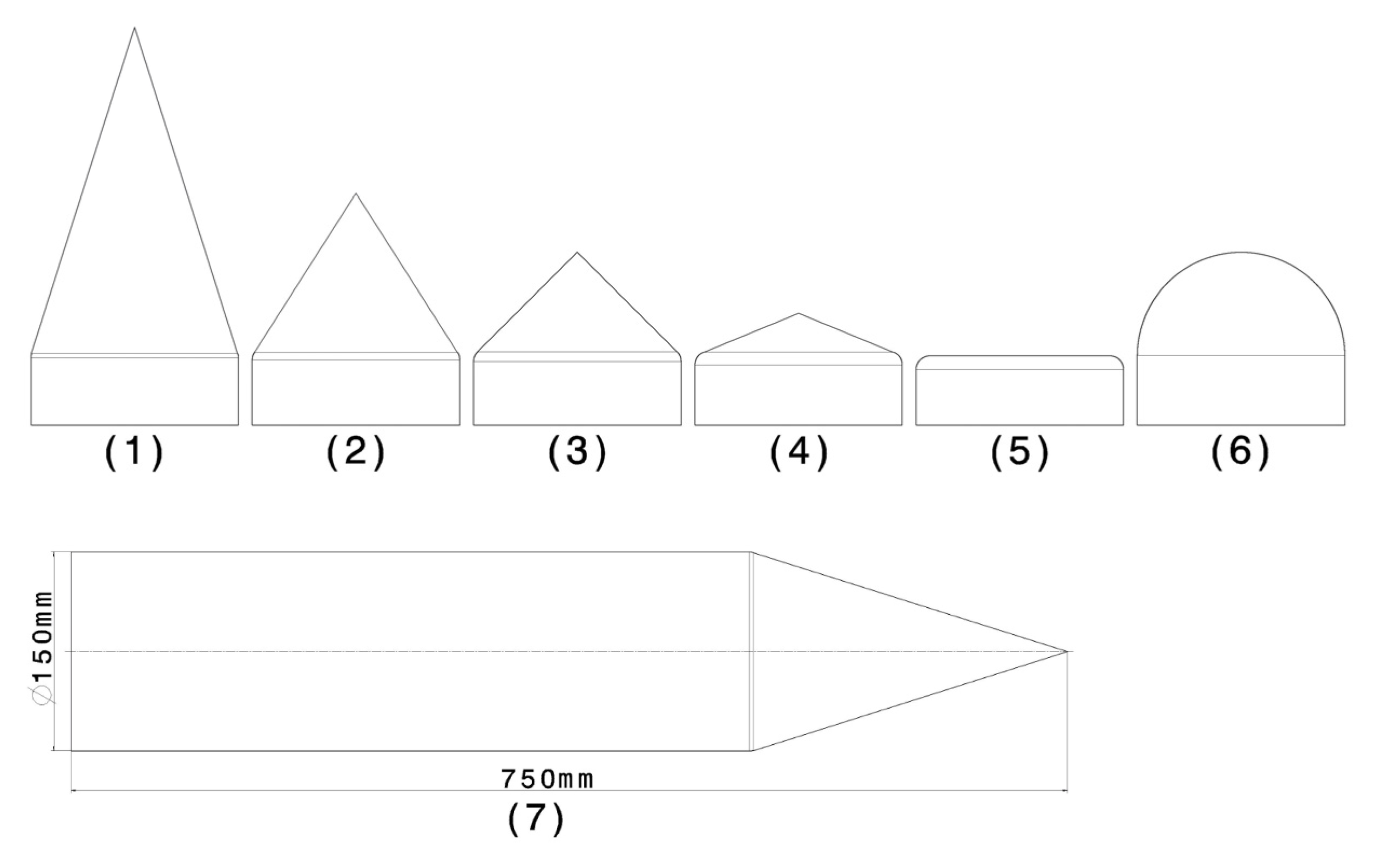
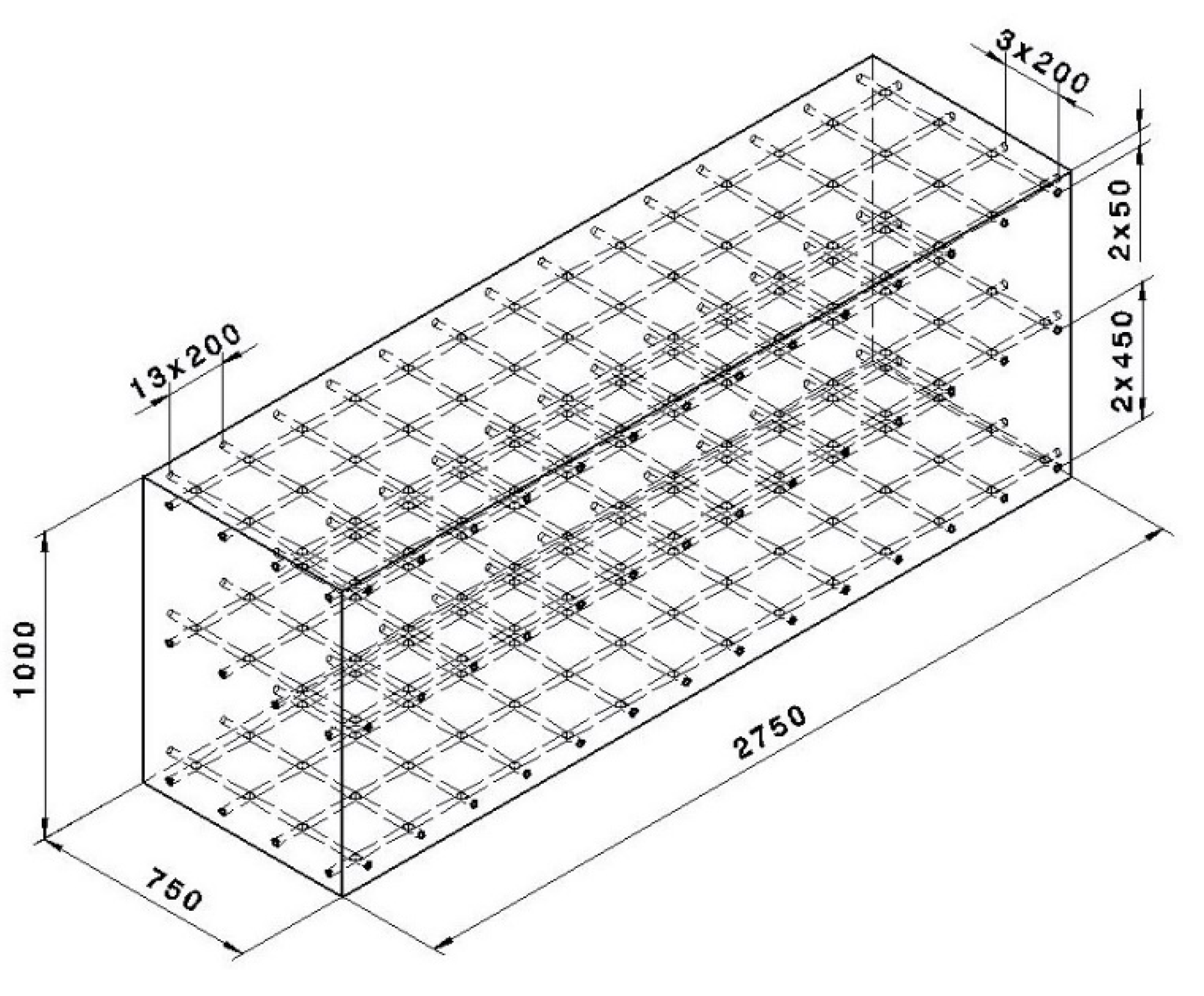



| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Density [kg/m3] | 2314 | Hardening Slope | 2 |
| Specific Heat [J/kgK] | 654 | Elastic Strength/ft | 0.7 |
| Bulk Modulus [MPa] | 35,270 | Elastic Strength/fc | 0.53 |
| Shear Modulus [MPa] | 16,700 | Failure Strength Constant B | 1.6 |
| Strength Model | RHT Concrete | Fracture Strength Exponent m | 0.61 |
| Compressive Strength fc [MPa] | 35 | Compressive Strain Rate Exponent α | 0.032 |
| Tensile Strength ft/fc | 0.1 | Tensile Strain Rate Exponent δ | 0.036 |
| Shear Strength fs/fc | 0.18 | Maximum Fracture Strength Ratio SFMAX | 1 × 1020 |
| Intact Failure Surface Constant A | 1.6 | Damage Constant D1 | 0.04 |
| Intact Failure Surface Exponent n | 0.61 | Damage Constant D2 | 1 |
| Tension/Compression Median Ratio Q2.0 | 0.6805 | Minimum Strain to Failure | 0.01 |
| Brittle to Ductile Transition BQ | 0.0105 | Residual Shear Modulus Fraction | 0.13 |
| Parameter | Value | Parameter | Values |
|---|---|---|---|
| Density [kg/m3] | 7830 | Initial Yield Stress [MPa] | 792 |
| Bulk Modulus [MPa] | 1.59 × 105 | Hardening Constant [MPa] | 510 |
| Shear Modulus [MPa] | 81,800 | Hardening Exponent | 0.26 |
| Strength Model | Johnson-Cook | Strain Rate Exponent | 0.014 |
| Strain Rate Correlation | First-Order | Reference Strain Rate [1/sec] | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jurecs, S.P.; Tabei, A. Simulation of the Effects of Angle of Attack and Projectile Contour in Damage Development in Reinforced Concrete. Designs 2021, 5, 49. https://doi.org/10.3390/designs5030049
Jurecs SP, Tabei A. Simulation of the Effects of Angle of Attack and Projectile Contour in Damage Development in Reinforced Concrete. Designs. 2021; 5(3):49. https://doi.org/10.3390/designs5030049
Chicago/Turabian StyleJurecs, Stefan P., and Ali Tabei. 2021. "Simulation of the Effects of Angle of Attack and Projectile Contour in Damage Development in Reinforced Concrete" Designs 5, no. 3: 49. https://doi.org/10.3390/designs5030049
APA StyleJurecs, S. P., & Tabei, A. (2021). Simulation of the Effects of Angle of Attack and Projectile Contour in Damage Development in Reinforced Concrete. Designs, 5(3), 49. https://doi.org/10.3390/designs5030049





