Operation Safety of a 2-DoF Planar Mechanism for Arm Rehabilitation
Abstract
1. Introduction
2. Materials and Methods
2.1. A Brief Description of Nurse
2.2. Performance Analysis
- Locking the actuators of all the limbs of the parallel mechanism except for the one corresponding to the degree of freedom under analysis (limb i).
- Substituting the non-locked actuator and its limb with the corresponding unit force transmitted to the end-effector.
- Evaluating the instantaneous velocity corresponding to the motion of the end-effector resulting from the unit force applied in the previous point.
2.3. Operation Safety
3. Results and Discussion
3.1. Performance Analysis
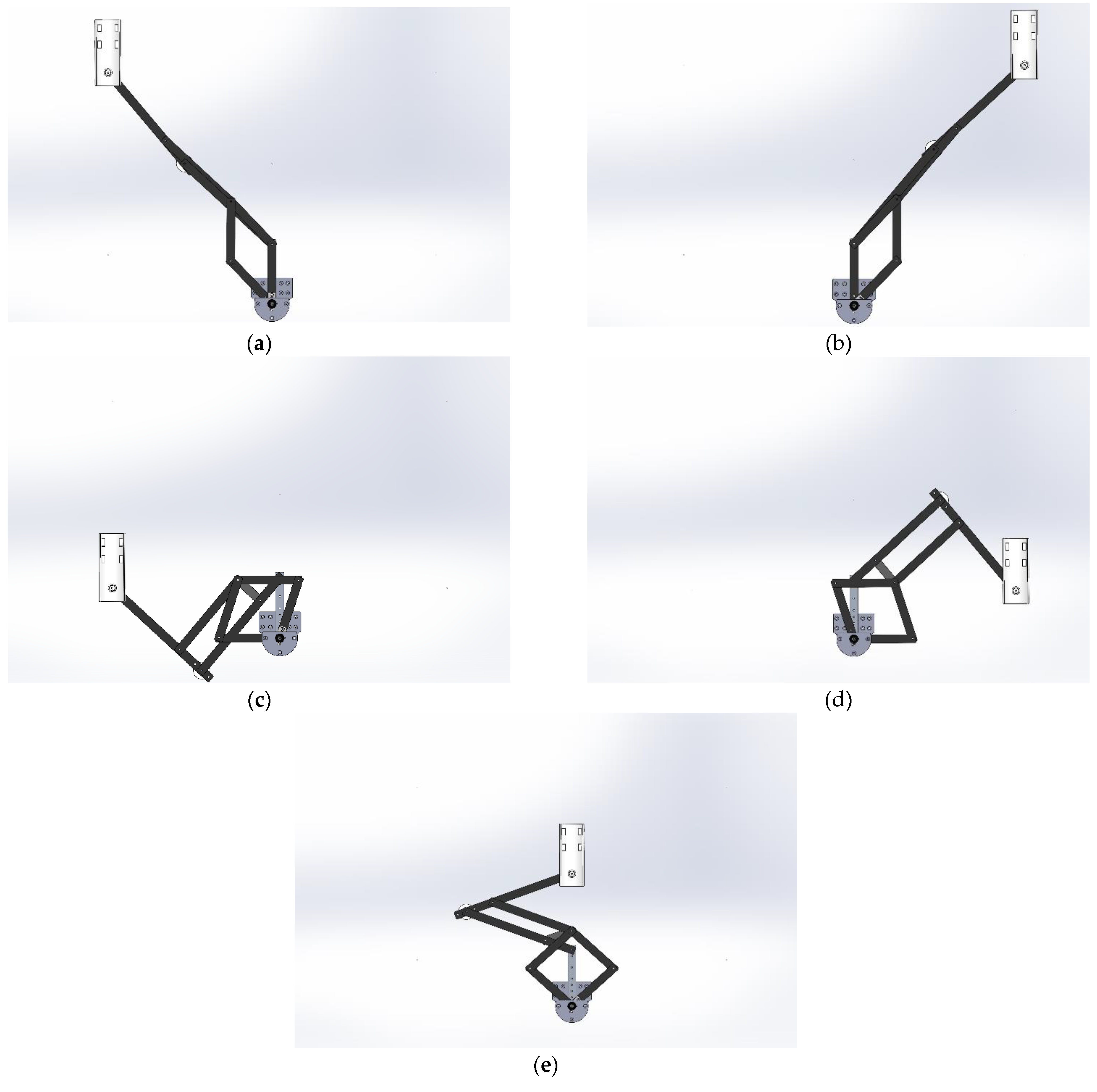
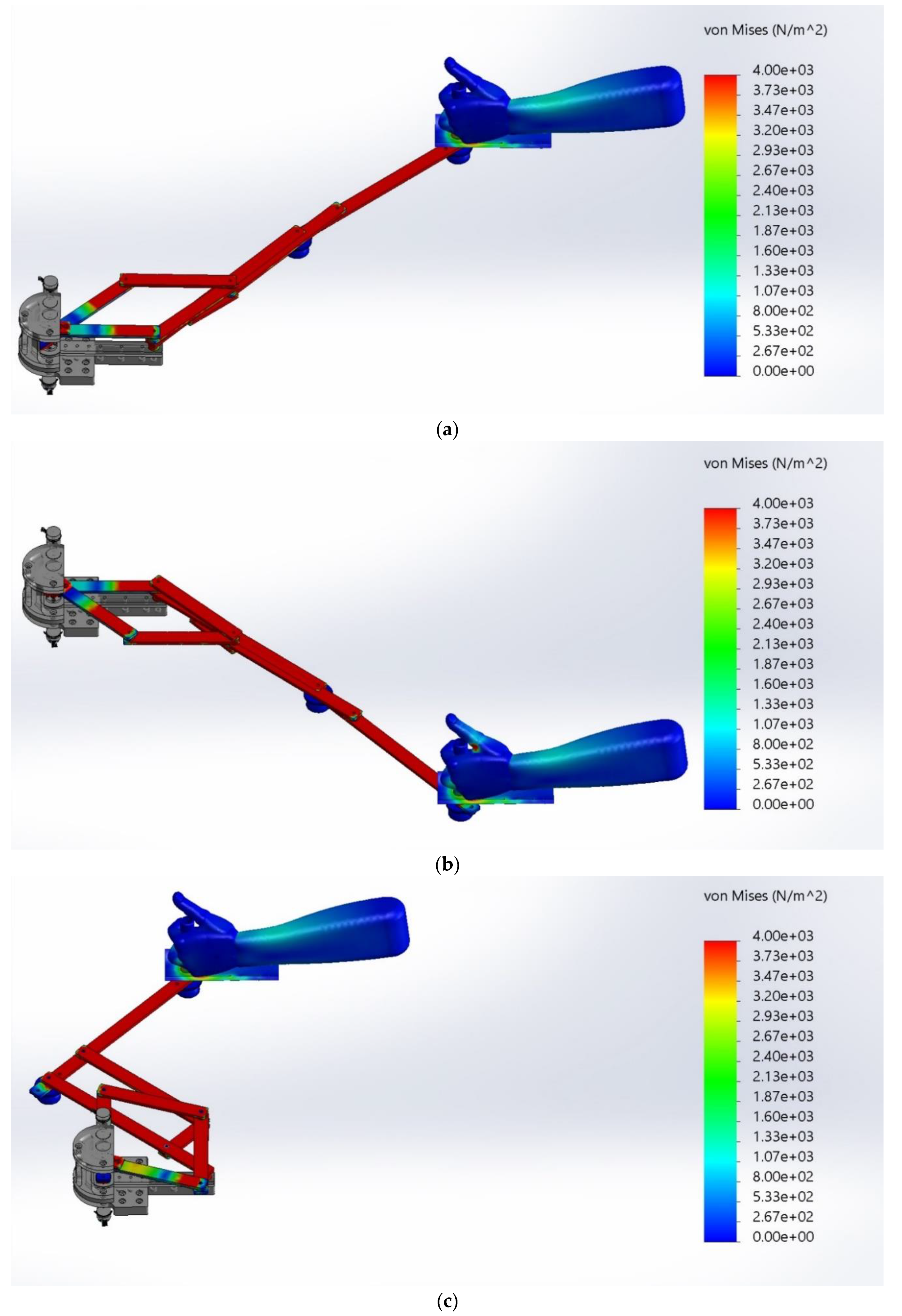
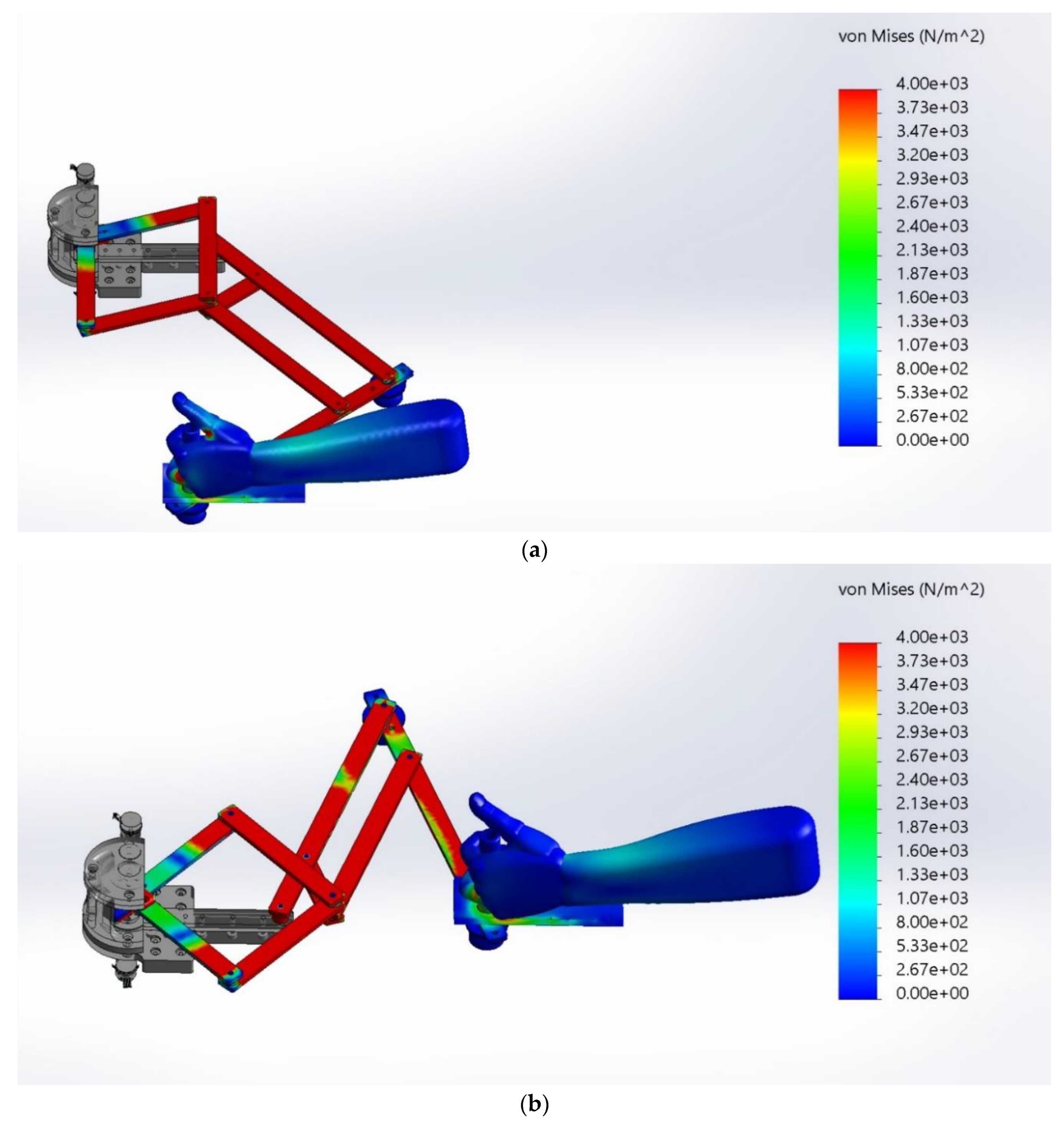
3.2. Operation Safety
4. Conclusions
5. Patents
Author Contributions
Funding
Conflicts of Interest
References
- Burgar, C.G.; Lum, P.S.; Shor, P.C.; Van der Loos, H.M. Development of robots for rehabilitation therapy: The Palo Alto VA/Stanford experience. J. Rehabil. Res. Dev. 2000, 37, 663–673. [Google Scholar]
- Lum, P.S.; Burgar, C.G.; Shor, P.C.; Majmundar, M.; Van der Loos, M. Robot-assisted movement training compared with conventional therapy techniques for the rehabilitation of upper-limb motor function after stroke. Arch. Phys. Med. Rehabil. 2002, 83, 952–959. [Google Scholar] [CrossRef]
- Chu, C.Y.; Patterson, R.M. Soft robotic devices for hand rehabilitation and assistance: A narrative review. J Neuroeng. Rehabil. 2018, 15, 1–14. [Google Scholar] [CrossRef]
- Hesse, S.; Schmidt, H.; Werner, C.; Bardeleben, A. Upper and lower extremity robotic devices for rehabilitation and for studying motor control. Curr. Opin. Neurol. 2003, 16, 705–710. [Google Scholar] [CrossRef]
- Laurentis, K.J.; Won, J.; Alam, M.; Mavroidis, C. Fabrication of Non-Assembly Mechanisms and Robotic Systems Using Rapid Prototyping. ASME. J. Mech. Des. 2001, 123, 516–524. [Google Scholar] [CrossRef]
- Nematollahi, M.; Baghbaderani, K.S.; Amerinatanzi, A.; Zamanian, H.; Elahinia, M. Application of NiTi in Assistive and Rehabilitation Devices: A Review. Bioengineering 2019, 6, 37. [Google Scholar] [CrossRef]
- Riener, R.; Nef, T.; Colombo, G. Robot-aided Neurorehabilitation of the Upper Extremities. Med. Biol. Eng. Comput. 2005, 43, 2–10. [Google Scholar] [CrossRef] [PubMed]
- Gull, M.A.; Bai, S.; Bak, T. A Review on Design of Upper Limb Exoskeletons. Robotics 2020, 9, 16. [Google Scholar] [CrossRef]
- Ball, S.J.; Brown, I.E.; Scott, S.H. A Planar 3dof Robotic Exoskeleton for Rehabilitation and Assessment. In Proceedings of the 29th Annual Conference of the IEEE EMBS Cité Internationale, Lyon, France, 23–26 August 2007; IEEE: Paris, France, 2007; pp. 4024–4027. [Google Scholar] [CrossRef]
- Maciejasz, P.; Eschweiler, J.; Gerlach-Hahn, K.; Jansen-Troy, A.; Leonhardt, S. A survey on robotic devices for upper limb rehabilitation. J. Neuroeng. Rehabil. 2014, 11, 3. [Google Scholar] [CrossRef]
- Nef, T.; Guidali, M.; Riener, R. ARMin III–Arm Therapy Exoskeleton with an Ergonomic Shoulder Actuation. Appl. Bionics Biomech. 2009, 6, 127–142. [Google Scholar] [CrossRef]
- de la Tejera, J.A.; Bustamante-Bello, R.; Ramirez-Mendoza, R.A.; Izquierdo-Reyes, J. Systematic Review of Exoskeletons towards a General Categorization Model Proposal. Appl. Sci. 2021, 11, 76. [Google Scholar] [CrossRef]
- Mao, Y.; Agrawal, S.K. Design of a Cable-Driven Arm Exoskeleton (CAREX) for Neural Rehabilitation. IEEE Trans. Robot. 2012, 28, 922–931. [Google Scholar] [CrossRef]
- Brokaw, E.B.; Murray, T.; Nef, T.; Lum, P.S. Retraining of interjoint arm coordination after stroke using robot-assisted time-independent functional training. J. Rehabil. Res. Dev. 2011, 48, 299–316. [Google Scholar] [CrossRef] [PubMed]
- Binns, M.; Protas, E.D.; Avenarius, S. Shoulder Rehabilitation and Exercise Device. U.S. Patent No. US008251879B2, 28 August 2012. [Google Scholar]
- Campolo, D.; Widjaja, F.; Klein Hubert, J. An Apparatus for Upper Body Movement. U.S. Patent Application 2015/0302777 A1, 22 October 2016. [Google Scholar]
- Annisa, J.; Mohamaddan, S.; Jamaluddin, M.S.; Aliah, A.N.; Omar, A.; Helmy, H.; Norafizah, A. Development of Upper Limb Rehabilitation Robot Prototype for Home Setting. In Proceedings of the 5th Brunei International Conference on Engineering and Technology (BICET 2014), Bandar Seri Begawan, Brunei, 1–3 November 2014; IET: Bandar Seri Begawan, Brunei, 2014; pp. 1–6. [Google Scholar] [CrossRef]
- Krebs, H.I.; Ferraro, M.; Buerger, S.P.; Newbery, M.J.; Makiyama, A.; Sandmann, M.; Lynch, D.; Volpe, B.T.; Hogan, N. Rehabilitation robotics: Pilot trial of a spatial extension for MIT-Manus. J. NeuroEng. Rehabil. 2004, 1, 5. [Google Scholar] [CrossRef] [PubMed]
- Qassim, H.M.; Wan Hasan, W.Z. A Review on Upper Limb Rehabilitation Robots. Appl. Sci. 2020, 10, 6976. [Google Scholar] [CrossRef]
- Rodríguez-León, J.F.; Chaparro-Rico, B.D.M.; Russo, M.; Cafolla, D. An Autotuning Cable-Driven Device for Home Rehabilitation. J. Healthc. Eng. 2021, 2021, 6680762. [Google Scholar] [CrossRef] [PubMed]
- Cafolla, D.; Russo, M.; Carbone, G. Design of CUBE, a Cable-Driven Device for Upper and Lower Limb Exercising. In New Trends in Medical and Service Robotics. Mechanisms and Machine Science; Carbone, G., Ceccarelli, M., Pisla, D., Eds.; Springer: Cham, Switzerland, 2019; Volume 65, pp. 255–263. [Google Scholar] [CrossRef]
- Xiong, H.; Diao, X. A review of cable-driven rehabilitation devices. Disabil. Rehabil. Assist. Technol. 2020, 15, 885–897. [Google Scholar] [CrossRef]
- Chaparro-Rico, B.D.M.; Cafolla, D.; Castillo-Castaneda, E.; Ceccarelli, M. Design of arm exercises for rehabilitation assistance. J. Eng. Res. 2020, 8, 204–218. [Google Scholar] [CrossRef]
- Chaparro-Rico, B.D.M.; Cafolla, D.; Ceccarelli, M.; Castillo-Castaneda, E. Design and Simulation of an Assisting Mechanism for Arm Exercises. In Advances in Italian Mechanism Science. Mechanisms and Machine Science; Boschetti, G., Gasparetto, A., Eds.; Springer: Cham, Switzerland, 2017; Volume 47, pp. 115–123. [Google Scholar] [CrossRef]
- Chaparro-Rico, B.D.M.; Cafolla, D.; Ceccarelli, M.; Castillo-Castaneda, E. NURSE-2 DoF Device for Arm Motion Guidance: Kinematic, Dynamic, and FEM Analysis. Appl. Sci. 2020, 10, 2139. [Google Scholar] [CrossRef]
- Chaparro-Rico, B.D.; Cafolla, D.; Ceccarelli, M.; Castillo-Castaneda, E. Experimental Characterization of NURSE, a Device for Arm Motion Guidance. J. Healthc. Eng. 2018, 2018, 9303282. [Google Scholar] [CrossRef]
- Bessler, J.; Prange-Lasonder, G.B.; Schaake, L.; Saenz, J.F.; Bidard, C.; Fassi, I.; Valori, M.; Lassen, A.B.; Buurke, J.H. Safety Assessment of Rehabilitation Robots: A Review Identifying Safety Skills and Current Knowledge Gaps. Front. Robot. AI 2021, 8, 602878. [Google Scholar] [CrossRef]
- Cafolla, D.; Russo, M.; Carbone, G. Design and validation of an inherently-safe cable-driven assisting device. Int. J. Mech. Control. 2018, 19, 23–32. [Google Scholar]
- Carbone, G.; Gerding, E.C.; Corves, B.; Cafolla, D.; Russo, M.; Ceccarelli, M. Design of a Two-DOFs driving mechanism for a motion-assisted finger exoskeleton. Appl. Sci. 2020, 10, 2619. [Google Scholar] [CrossRef]
- Chen, C.; Angeles, J. Generalized transmission index and transmission quality for spatial linkages. Mech. Mach. Theory 2007, 42, 1225–1237. [Google Scholar] [CrossRef]
- Xie, F.; Liu, X.J.; Wang, J. Performance evaluation of redundant parallel manipulators assimilating motion/force transmissibility. Int. J. Adv. Robot. Syst. 2011, 8, 113–124. [Google Scholar] [CrossRef]
- Wang, J.; Wu, C.; Liu, X.J. Performance evaluation of parallel manipulators: Motion/force transmissibility and its index. Mech. Mach. Theory 2010, 45, 1462–1476. [Google Scholar] [CrossRef]
- Liu, H.; Huang, T.; Kecskeméthy, A.; Chetwynd, D.G. A generalized approach for computing the transmission index of parallel mechanisms. Mech. Mach. Theory 2014, 74, 245–256. [Google Scholar] [CrossRef]
- Liang, X.; Takeda, Y. Transmission index of a class of parallel manipulators with 3-RS (SR) primary structures based on pressure angle and equivalent mechanism with 2-SS chains replacing RS chain. Mech. Mach. Theory 2019, 139, 359–378. [Google Scholar] [CrossRef]
- Russo, M.; Ceccarelli, M.; Takeda, Y. Force transmission and constraint analysis of a 3-SPR parallel manipulator. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 4399–4409. [Google Scholar] [CrossRef]
- ISO International Organization for Standardization. ISO 9283:1998. Available online: https://www.iso.org/standard/22244.html (accessed on 21 October 2021).
- Ansari, M.Z.; Lee, S.K.; Cho, C.D. Hyperelastic Muscle Simulation. KEM 2007, 345–346, 1241–1244. [Google Scholar] [CrossRef]
- Sparks, J.L.; Vavalle, N.A.; Kasting, K.E.; Long, B.; Tanaka, M.L.; Sanger, P.A.; Schnell, K.; Conner-Kerr, T.A. Use of silicone materials to simulate tissue biomechanics as related to deep tissue injury. Adv. Skin Wound Care 2015, 28, 59–68. [Google Scholar] [CrossRef] [PubMed]
- Pineau, J.C.; Delamarche, P.; Bozinovic, S. Average Height of Adolescents in the Dinaric Alps. C. R. Biol. 2005, 328, 841–846. [Google Scholar] [CrossRef]
- Hall, S.J. Basic Biomechanics, 6th ed.; McGraw Hill: New York, NY, USA, 2012; p. 538. [Google Scholar]
- Schmit, J. Human Left Hand. Available online: https://grabcad.com/library/human-left-hand (accessed on 18 October 2021).
- Budynas, R.G.; Nisbett, J.K.; Shigley, J.E. Shigley’s Mechanical Engineering Design, 8th ed.; McGraw-Hill: New York, NY, USA, 2008; p. 216. [Google Scholar]
- Dao, T.T.; Tho, M.-C.H.B. A Systematic Review of Continuum Modeling of Skeletal Muscles: Current Trends, Limitations, and Recommendations. Appl. Bionics. Biomech. 2018, 2018, 7631818. [Google Scholar] [CrossRef] [PubMed]
- Martins, J.A.C.; Pires, E.B.; Salvado, R.; Dinis, P.B. A numerical model of passive and active behavior of skeletal muscles. Comput. Methods Appl. Mech. Eng. 1998, 151, 419–433. [Google Scholar] [CrossRef]
- Toumanidou, T.; Noailly, J. Musculoskeletal modeling of the lumbar spine to explore functional interactions between back muscle loads and intervertebral disk Multiphysics. Front. Bioeng. Biotechnol. 2015, 3, 11. [Google Scholar] [CrossRef][Green Version]
- Fan, A.X.; Dakpé, S.; Dao, T.T.; Pouletaut, P.; Rachik, M.; Ho Ba Tho, M.C. MRI-based finite element modeling of facial mimics: A case study on the paired zygomaticus major muscles. Comput. Methods Biomech. Biomed. Eng. 2017, 20, 919–928. [Google Scholar] [CrossRef]
- Röhrle, O. Simulating the electro-mechanical behavior of skeletal muscles. Comput. Sci. Eng. 2010, 12, 48–58. [Google Scholar] [CrossRef]
- Tang, C.Y.; Tsui, C.P.; Stojanovic, B.; Kojic, M. Finite element modelling of skeletal muscles coupled with fatigue. Int. J. Mech. Sci. 2007, 49, 1179–1191. [Google Scholar] [CrossRef]
- Blemker, S.S.; Pinsky, P.M.; Delp, S.L. A 3D model of muscle reveals the causes of nonuniform strains in the biceps brachii. J. Biomech. 2005, 38, 657–665. [Google Scholar] [CrossRef]
- Singh, D.; Rana, A.; Jhajhria, S.K.; Garg, B.; Pandey, P.M.; Kalyanasundaram, D. Experimental assessment of biomechanical properties in human male elbow bone subjected to bending and compression loads. J. Appl. Biomater. Funct. 2019, 17, 1–13. [Google Scholar] [CrossRef]
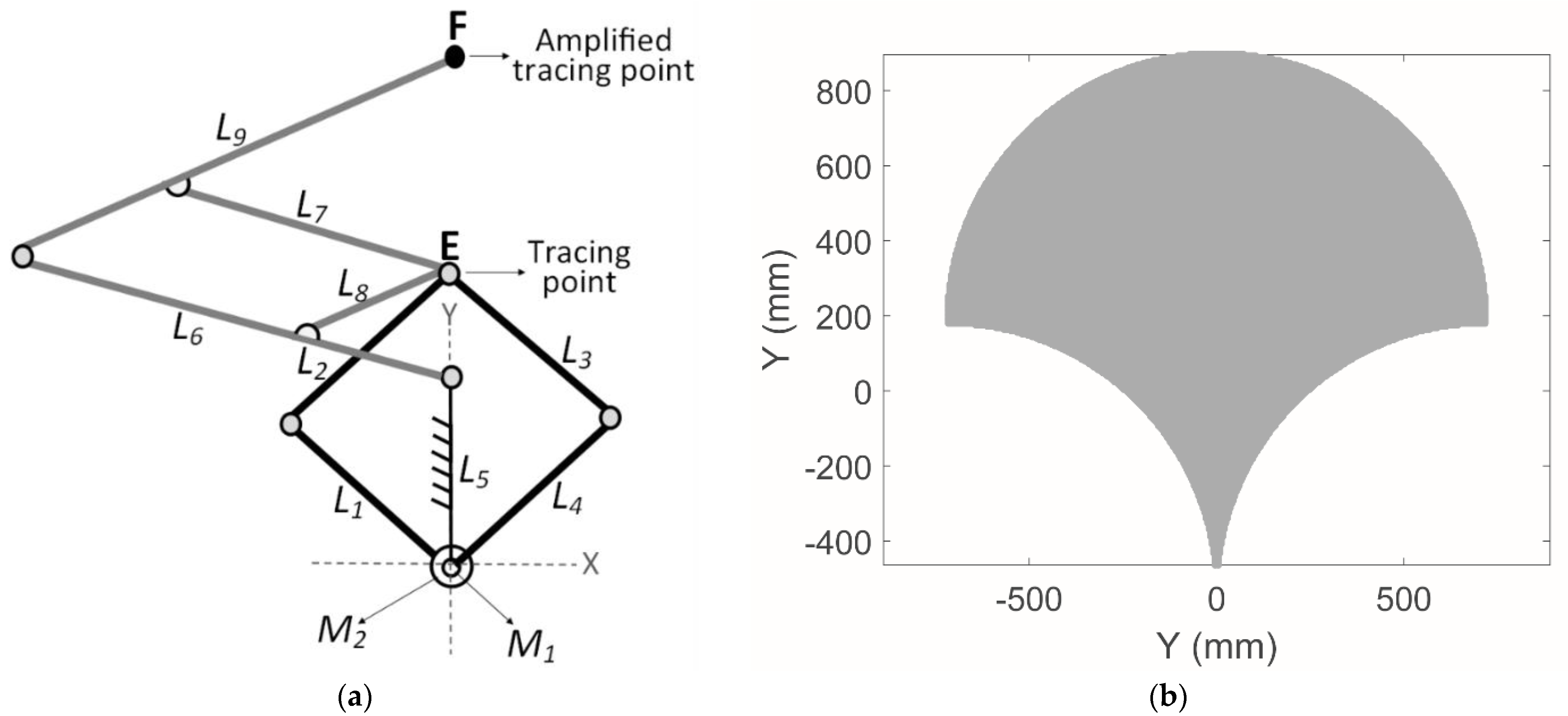


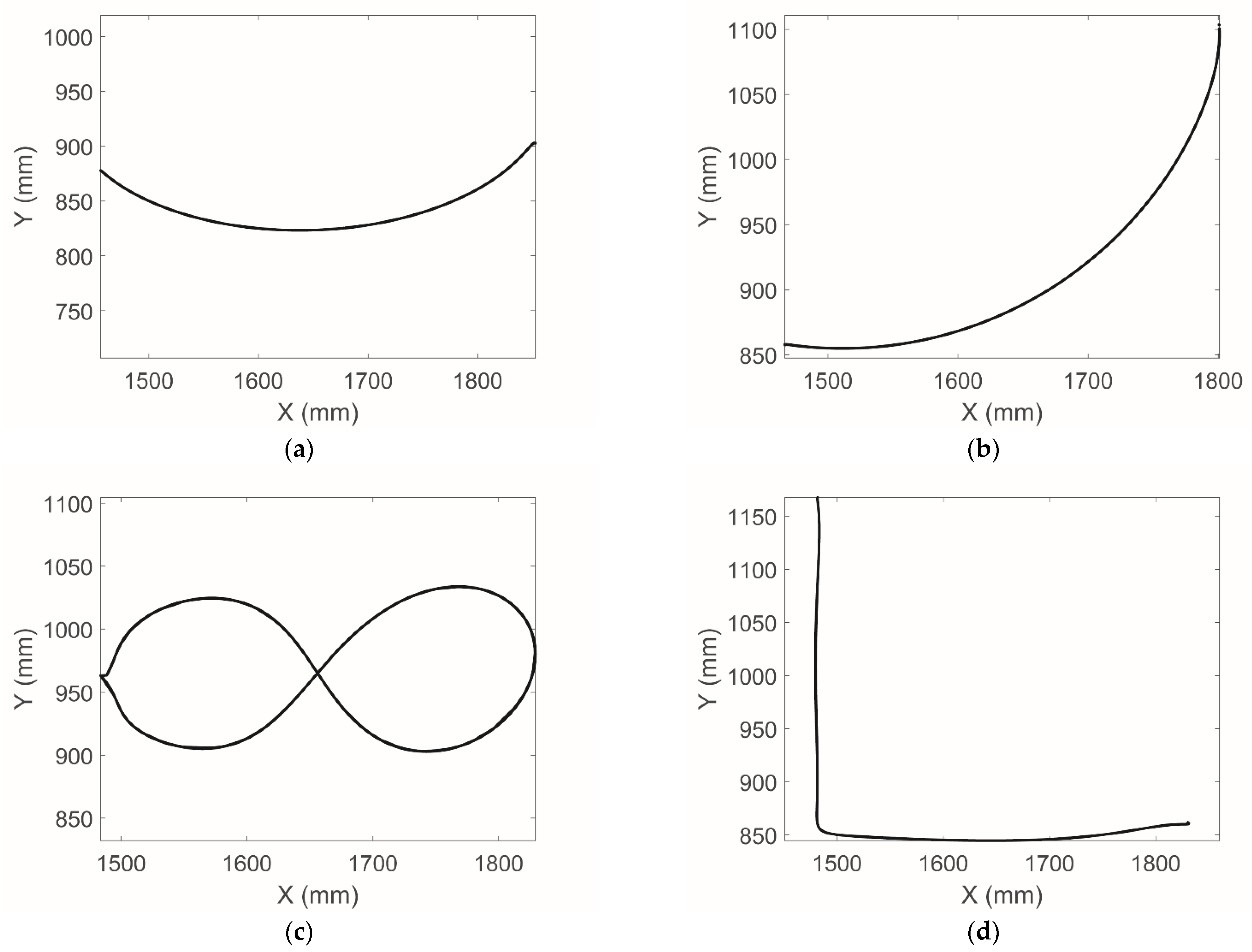
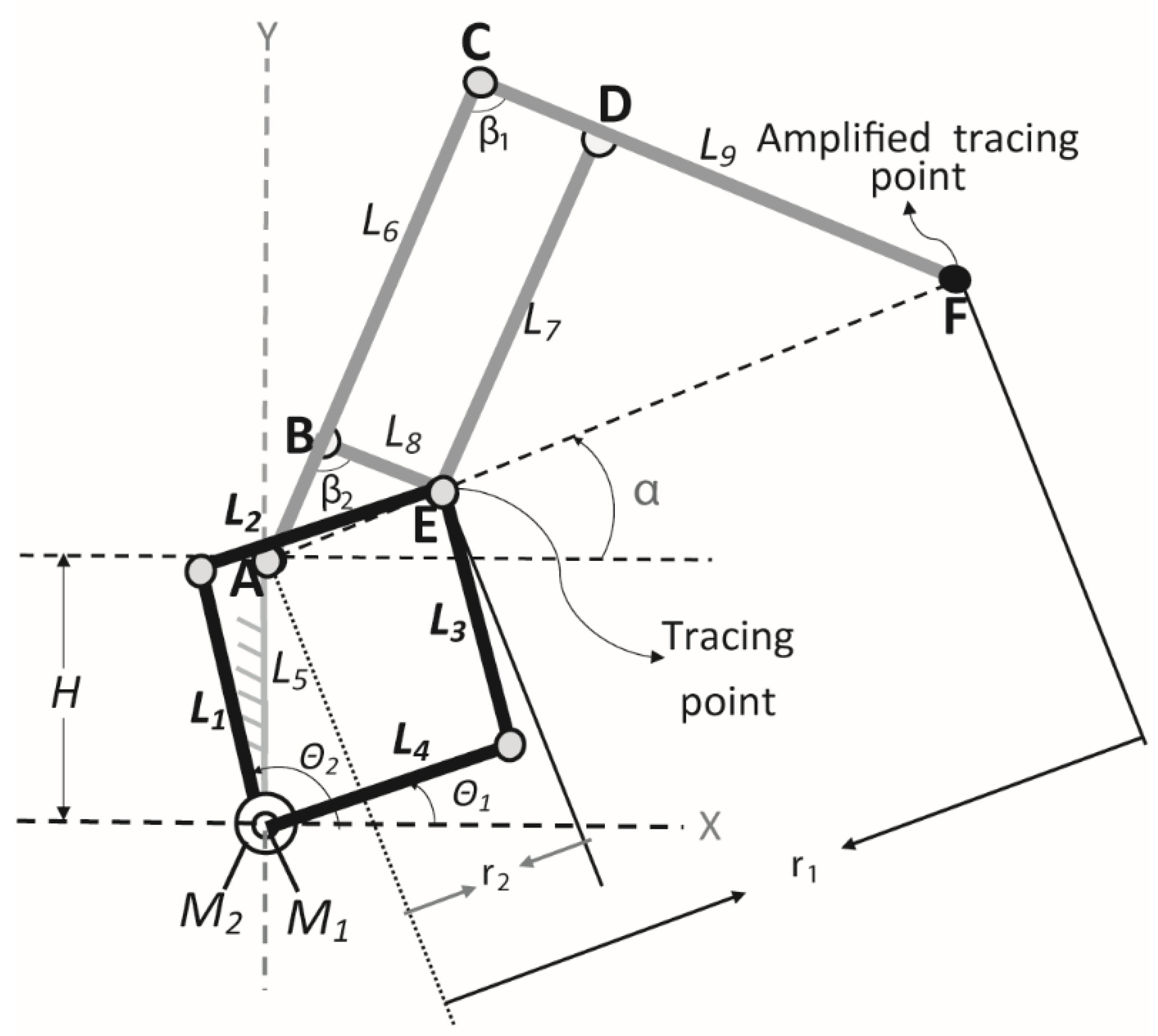
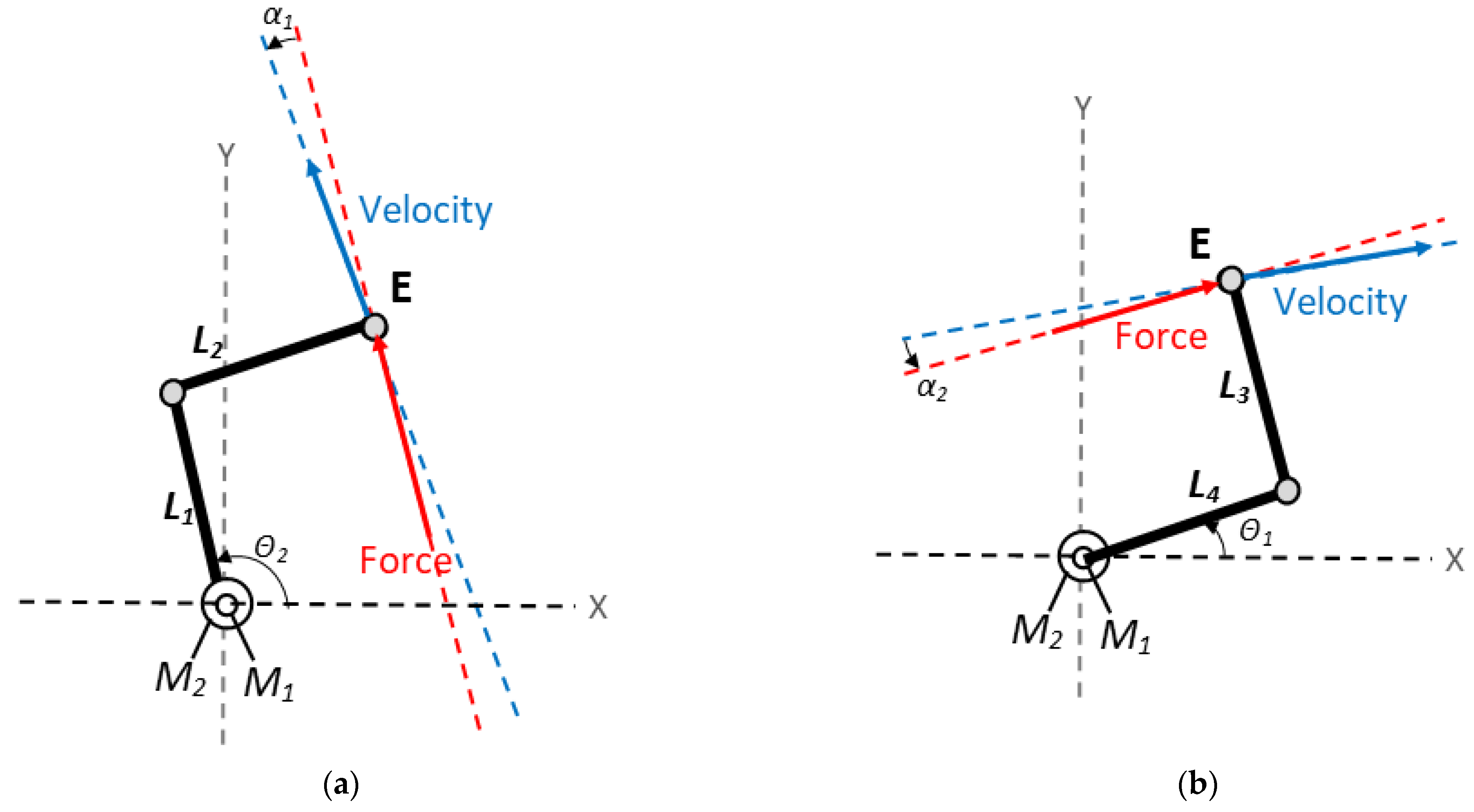


| Properties | Units | 1060 Aluminum |
|---|---|---|
| Elastic Modulus | N/m2 | 6.90 × 1010 |
| Poisson’s Ratio | N/A | 0.33 |
| Shear Modulus | N/m2 | 2.70 × 1010 |
| Mass Density | kg/m3 | 2 700 |
| Tensile Strength | N/m2 | 6.89 × 107 |
| Yield Strength | N/m2 | 2.76 × 107 |
| Properties | Units | ABS 1 |
|---|---|---|
| Elastic Modulus | N/m2 | 0.2 × 1010 |
| Poisson’s Ratio | N/A | 0.39 |
| Shear Modulus | N/m2 | 0.32 × 1010 |
| Mass Density | kg/m3 | 1020 |
| Tensile Strength | N/m2 | 3 × 107 |
| Properties | Units | Silicone |
|---|---|---|
| Elastic Modulus | N/m2 | 11.24 × 1010 |
| Poisson’s Ratio | N/A | 0.28 |
| Shear Modulus | N/m2 | 4.9 × 1010 |
| Mass Density | kg/m3 | 2330 |
| Yield Strength | N/m2 | 12 × 107 |
| Mesh Type | Solid Mesh |
|---|---|
| Mesh type | Mixed Mesh |
| Mesher Used | Curvature-based mesh |
| Jacobian points | 16 Points |
| Maximum element size | 15.40 mm |
| Minimum element size | 3.08 mm |
| Total Nodes | 264,679 |
| Total Elements | 157,419 |
| Variable | Value | Units |
|---|---|---|
| L1 | 180 | mm |
| L2 | 180 | mm |
| L3 | 180 | mm |
| L4 | 180 | mm |
| L5 | 180 | mm |
| L6 | 360 | mm |
| L7 | 270 | mm |
| L8 | 90 | mm |
| L9 | 360 | mm |
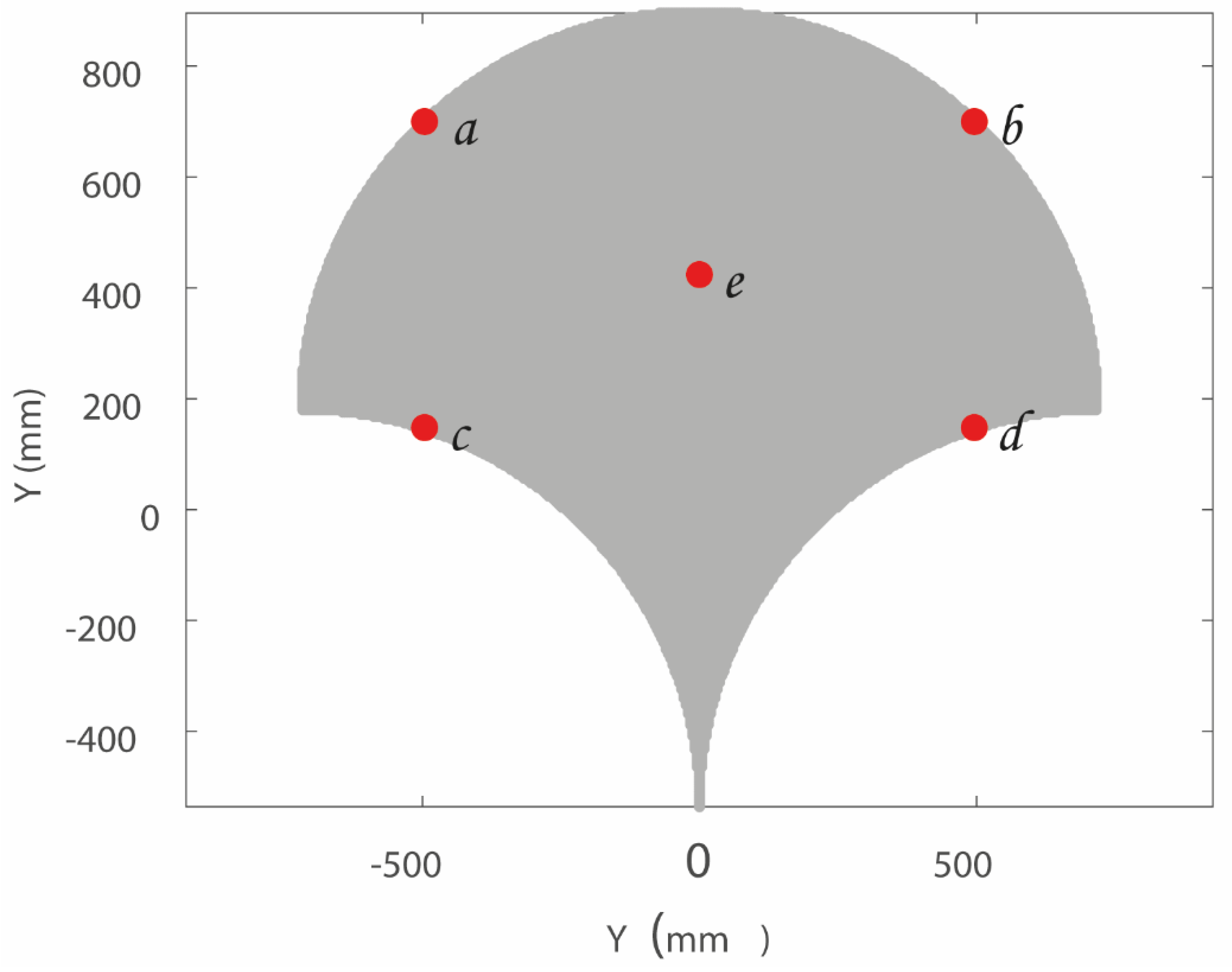
| Critical Positions | Maximum Stress Values |
|---|---|
| a (−496, 700) | 3.99 × 103 N/m2 |
| b (496, 700) | 4.41 × 103 N/m2 |
| c (−496, 148) | 6.53 × 103 N/m2 |
| d (496, 148) | 6.55 × 103 N/m2 |
| e (0, 424) | 4.43 × 103 N/m2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ceccarelli, M.; Russo, M.; Cafolla, D.; Chaparro-Rico, B.D.M. Operation Safety of a 2-DoF Planar Mechanism for Arm Rehabilitation. Inventions 2021, 6, 85. https://doi.org/10.3390/inventions6040085
Ceccarelli M, Russo M, Cafolla D, Chaparro-Rico BDM. Operation Safety of a 2-DoF Planar Mechanism for Arm Rehabilitation. Inventions. 2021; 6(4):85. https://doi.org/10.3390/inventions6040085
Chicago/Turabian StyleCeccarelli, Marco, Matteo Russo, Daniele Cafolla, and Betsy D. M. Chaparro-Rico. 2021. "Operation Safety of a 2-DoF Planar Mechanism for Arm Rehabilitation" Inventions 6, no. 4: 85. https://doi.org/10.3390/inventions6040085
APA StyleCeccarelli, M., Russo, M., Cafolla, D., & Chaparro-Rico, B. D. M. (2021). Operation Safety of a 2-DoF Planar Mechanism for Arm Rehabilitation. Inventions, 6(4), 85. https://doi.org/10.3390/inventions6040085









